Strategic Third-Party Product Entry and Mode Choice under Self-Operating Channels and Marketplace Competition: A Game-Theoretical Analysis
Abstract
1. Introduction
2. Literature Review
2.1. Provider-Side Network Effects in the Context of a Two-Sided Market
2.2. Platform Operations under Different Business Models
2.3. Product Entry in Platform-Based Markets
3. The Model
4. Pricing Analysis
4.1. Pricing Strategies without an Entrant Supplier
4.2. Pricing Strategies with an Entrant Supplier
- (1)
- ,,,, ,;
- (2)
- If si > sj, , ,,, , and ;
- (3)
- If , ; otherwise, ;
- (4)
- ,,,.
- (1)
- ,,;
- (2)
- ,;
- (3)
- ,,.
5. Equilibrium Analysis
5.1. Impact of a Third-Party Supplier’s Entry
- (a)
- Under the reselling self-operating mode, the incumbent supplier charges a higher wholesale price (i.e., ) if v < v1 and gains a higher profit (i.e., ) if v < v3, and the platform charges a higher retail price (i.e., ) if v < v2 and gains a higher profit (i.e., ) if v < v4;
- (b)
- Under the revenue-sharing self-operating mode, the incumbent supplier charges a higher retail price (i.e., ) if v < v5 and gains a higher profit (i.e., ) if v < v6, and the platform gains a higher profit (i.e., ) if v < v7, where vi (i=1,…,7) is defined in Appendix A.
5.2. Equilibrium Partnership
- (a)
- If v < v4, the platform would open its marketplace under both self-operating modes;
- (b)
- If v4 < v < v7, the platform would open its marketplace under the revenue-sharing self-operating mode;
- (c)
- If v > v7, the platform would not open its marketplace, where v4 and v7 are given in Proposition 1.
- (a)
- If ρ < ρ1, the third-party supplier would join the marketplace under both self-operating modes;
- (b)
- If ρ1 < ρ < ρ2, the third-party supplier would join the marketplace under both self-operating modes;
- (c)
- If ρ > ρ2, the third-party supplier would not join the marketplace, where ρ1 and ρ2 are defined in Appendix A.
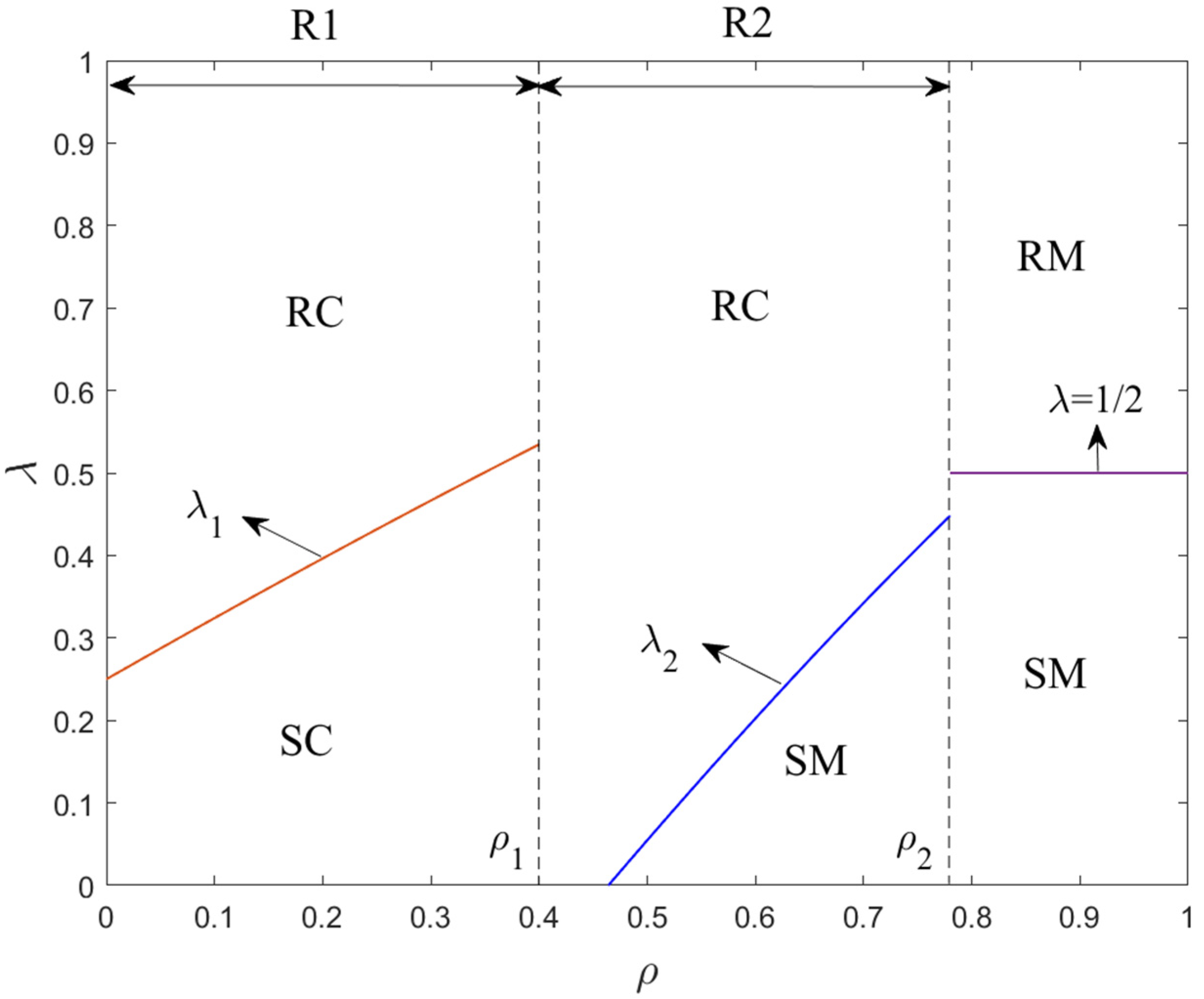
5.3. Self-Operating Mode Choice
- (a)
- When (v, ρ) ∈ R1:(a1) If λ > λ1, the incumbent supplier chooses the reselling mode and scenario RC is under an equilibrium;(a2) If λ < λ1, the incumbent supplier chooses the revenue-sharing mode and scenario SC is under an equilibrium;
- (b)
- When (v, ρ) ∈ R2:(b1) If λ > λ2, the incumbent supplier chooses the reselling mode and scenario RC is under an equilibrium;(b2) If λ < λ2, the incumbent supplier chooses the revenue-sharing mode and scenario SM is under an equilibrium;
- (c)
- When (v, ρ) ∈ R3:(c1) If λ > λ3, the incumbent supplier chooses the reselling mode and scenario RM is under an equilibrium;(c2) If λ < λ3, the incumbent supplier chooses the revenue-sharing mode and scenario SC is under an equilibrium;
- (d)
- Otherwise, the third-party supplier does not appear on the platform’s marketplace and:(d1) If λ > 1/2, the incumbent supplier chooses the reselling mode and scenario RM is under an equilibrium;(d2) If λ < 1/2, the incumbent supplier chooses the reselling mode and scenario SM is under an equilibrium.
6. Conclusions and Discussion
6.1. Theoretical Implications
6.2. Managerial Implications
6.3. Limitations and Future Research
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- (1)
- , ,, , ,, , , , and ;
- (2)
- If s1 > s2 holds, , ,,, , and ; if s1 < s2 holds, the following results can be derived similarly;
- (3)
- > 0 is equivalent to ;
- (4)
- ,,,. □
- (1)
- ,, and ;
- (2)
- , and ;
- (3)
- ;
- (4)
- ,
- (a)
- Denote , , , and . For the wholesale price, we can show that is equivalent to v < v1. For the incumbent supplier’s profit, holds if v < v3 is satisfied. For the retail price, is equivalent to v < v2. For the platform’s profit, holds if v < v4 is satisfied;
- (b)
- Denote , , and . For the retail price, we can show that is equivalent to v < v5. For the incumbent supplier’s profit, holds if v < v6 is satisfied. For the platform’s profit, holds if v < v7 is satisfied. □
References
- Castell, C.; Kiefer, J.; Schubach, S.; Schumann, J.; Graf-Vlachy, L.; Konig, A. Integrating digital platform dynamics into customer orientation research: A systematic review and research agenda. J. Bus. Res. 2023, 163, 113911. [Google Scholar] [CrossRef]
- Song, W.; Chen, J.; Li, W. Spillover Effect of Consumer Awareness on Third Parties’ Selling Strategies and Retailers’ Platform Openness. Inf. Syst. Res. 2021, 32, 172–193. [Google Scholar] [CrossRef]
- Tian, L.; Vakharia, A.J.; Tan, Y.; Xu, Y. Marketplace, reseller, or hybrid: Strategic analysis of an emerging e-commerce model. Prod. Oper. Manag. 2018, 27, 1595–1610. [Google Scholar] [CrossRef]
- Rossmannek, O.; David, N.; Schramm-Klein, H. Suppliers’ loyalty to their sharing platform: The influence of multiple roles. J. Bus. Res. 2022, 143, 272–281. [Google Scholar] [CrossRef]
- Wu, W.; Huang, X.; Wu, C.; Tsai, S. Pricing strategy and performance investment decisions in competitive crowdfunding markets. J. Bus. Res. 2022, 140, 491–497. [Google Scholar] [CrossRef]
- Zhou, G.; Song, P. Third-party apps (TPAs) and software platform performance: The moderating role of competitive entry. Inf. Manag. 2018, 55, 901–911. [Google Scholar] [CrossRef]
- Liu, X.; Zhou, Y.; Shen, Y.; Ge, C.; Jiang, J. Zooming in the impacts of merchants’ participation in transformation from online flash sale to mixed sale e-commerce platform. Inf. Manag. 2021, 58, 103409. [Google Scholar] [CrossRef]
- Ryan, J.K.; Sun, D.; Zhao, X. Competition and coordination in online marketplaces. Prod. Oper. Manag. 2012, 21, 997–1014. [Google Scholar] [CrossRef]
- Chen, J.; Guo, Z. New-Media Advertising and Retail Platform Openness. MIS Q. 2022, 46, 421–556. [Google Scholar] [CrossRef]
- Mantin, B.; Krishnan, H.; Dhar, T. The strategic role of third-party marketplaces in retailing. Prod. Oper. Manag. 2014, 23, 1937–1949. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Q.; Song, P. Third-party sellers’ product entry strategy and its sales impact on a hybrid retail platform. Electron. Commer. Res. Appl. 2012, 47, 101049. [Google Scholar] [CrossRef]
- Chang, S.; Li, A.; Wang, X.; Wang, X. Joint optimization of e-commerce supply chain financing strategy and channel contract. Eur. J. Oper. Res. 2022, 303, 908–927. [Google Scholar] [CrossRef]
- Orji, I.J.; Ojadi, F.; Okwara, U.K. The nexus between e-commerce adoption in a health pandemic and firm performance: The role of pandemic response strategies. J. Bus. Res. 2022, 145, 616–635. [Google Scholar] [CrossRef]
- Zhu, F.; Iansiti, M. Entry into platform-based markets. Strateg. Manag. J. 2012, 33, 88–106. [Google Scholar] [CrossRef]
- Mcintyre, D.P.; Srinivasan, A. Networks, platforms, and strategy: Emerging views and next steps. Strateg. Manag. J. 2017, 38, 141–160. [Google Scholar] [CrossRef]
- Song, P.; Xue, L.; Rai, A.; Zhang, C. The ecosystem of software platform: A study of asymmetric cross-side network effects and platform governance. MIS Q. 2018, 42, 121–142. [Google Scholar] [CrossRef]
- Zhou, X.; Chen, K.; Wen, H.; Lin, J.; Zhang, K.; Tian, X.; Wang, S.; Lev, B. Integration of third-party platforms: Does it really hurt them? Int. J. Prod. Econ. 2021, 234, 108003. [Google Scholar] [CrossRef]
- Anderson, E.G.; Parker, G.G.; Tan, B. Platform Performance Investment in the Presence of Network Externalities. Inf. Syst. Res. 2014, 25, 152–172. [Google Scholar] [CrossRef]
- Chen, J.; Fan, M.; Li, M. Advertising vs. brokerage model for online trading platforms. MIS Q. 2016, 40, 575–596. [Google Scholar] [CrossRef]
- Geng, X.; Tan, Y.; Wei, L. How add-on pricing interacts with distribution contracts. Prod. Oper. Manag. 2018, 27, 605–623. [Google Scholar] [CrossRef]
- Xu, B.; Yao, Z.; Wu, S. Pricing strategies for a bundled channel with services network effects. Int. J. Prod. Res. 2021, 59, 3152–3168. [Google Scholar] [CrossRef]
- Christen, T.; Hess, M.; Grichnik, D.; Wincent, J. Value-based pricing in digital platforms: A machine learning approach to signaling beyond core product attributes in cross-platform settings. J. Bus. Res. 2022, 152, 82–92. [Google Scholar] [CrossRef]
- Kwark, Y.; Chen, J.; Raghunathan, S. Platform or wholesale? A strategic tool for online retailers to benefit from third-party information. MIS Q. 2017, 41, 763–786. [Google Scholar] [CrossRef]
- Hao, L.; Tan, Y. Who wants consumers to be informed? Facilitating information disclosure in a distribution channel. Inf. Syst. Res. 2019, 30, 34–49. [Google Scholar] [CrossRef]
- Belhadj, N.; Laussel, D.; Resende, J. Marketplace or reselling? A signaling model. Inf. Econ. Policy 2020, 50, 100834. [Google Scholar] [CrossRef]
- Wei, J.; Lu, J.; Zhao, J. Interactions of competing manufacturers’ leader–follower relationship and sales format on online platforms. Eur. J. Oper. Res. 2020, 280, 508–522. [Google Scholar] [CrossRef]
- Zennyo, Y. Strategic contracting and hybrid use of agency and wholesale contracts in e-commerce platforms. Eur. J. Oper. Res. 2020, 281, 231–239. [Google Scholar] [CrossRef]
- Vrankić, I.; Herceg, T.; Bach, M. Dynamics and stability of evolutionary optimal strategies in duopoly. Cent. Eur. J. Oper. Res. 2021, 29, 1001–1019. [Google Scholar] [CrossRef]
- Vrankić, I. Conflict and Corporate Social Responsibility in Duopoly. Bus. Syst. Res. Int. J. Soc. Adv. Innov. Res. Econ. 2022, 13, 36–46. [Google Scholar] [CrossRef]
- Georgiou, A. A Case Study of investor R&D Evaluation using Game Theory. Entren. Enterp. Res. Innov. 2022, 8, 91–98. [Google Scholar]
- Qin, X.; Liu, Z.; Tian, L. The optimal combination between selling mode and logistics service strategy in an e-commerce market. Eur. J. Oper. Res. 2021, 289, 639–651. [Google Scholar] [CrossRef]
- Jiang, B.; Jerath, K.; Srinivasan, K. Firm strategies in the “mid tail” of platform-based retailing. Mark. Sci. 2021, 30, 757–775. [Google Scholar] [CrossRef]
- Lu, Q.; Liu, N. Effects of e-commerce channel entry in a two-echelon supply chain: A comparative analysis of single- and dual-channel distribution systems. Int. J. Prod. Econ. 2015, 165, 100–111. [Google Scholar] [CrossRef]
- Li, Z.; Agarwal, A. Platform integration and demand spillovers in complementary markets: Evidence from facebook’s integration of instagram. Manag. Sci. 2017, 63, 3438–3458. [Google Scholar] [CrossRef]
- Forderer, J.; Kude, T.; Mithas, S.; Heinzl, A. Does platform owner’s entry crowd out innovation? Evidence from Google photos. Inf. Syst. Res. 2018, 29, 444–460. [Google Scholar] [CrossRef]
- Zhu, F.; Liu, Q. Competing with complementors: An empirical look at Amazon.com. Strateg. Manag. J. 2018, 39, 2618–2642. [Google Scholar] [CrossRef]
- Wen, W.; Zhu, F. Threat of platform-owner entry and complementor responses: Evidence from the mobile app market. Strateg. Manag. J. 2019, 40, 1336–1367. [Google Scholar] [CrossRef]
- He, S.; Peng, J.; Li, J.; Xu, L. Impact of platform owner’s entry on third-party stores. Inf. Syst. Res. 2020, 31, 1467–1484. [Google Scholar] [CrossRef]
- Zhang, M.; Fu, Y.; Zhao, Z.; Pratap, S.; Huang, Q. Game theoretic analysis of horizontal carrier coordination with revenue sharing in E-commerce logistics. Int. J. Prod. Res. 2019, 57, 1524–1551. [Google Scholar] [CrossRef]
- Cui, R.; Li, M.; Li, Q. Value of High-Quality Logistics: Evidence from a Clash between SF Express and Alibaba. Manag. Sci. 2020, 66, 3879–3902. [Google Scholar] [CrossRef]
- Wang, X.; Xie, J.; Fan, Z. B2C cross-border Ecommerce logistics mode selection considering product returns. Int. J. Prod. Res. 2021, 59, 3841–3860. [Google Scholar] [CrossRef]
- Xu, B.; Yao, Z.; Tang, P. Pricing Strategies for Information Products with Network Effects and Complementary Services in a Duopolistic Market. Int. J. Prod. Res. 2018, 56, 4243–4263. [Google Scholar] [CrossRef]
- Baake, P.; Boom, A. Vertical product differentiation, network externalities, and compatibility decisions. Int. J. Ind. Organ. 2001, 19, 267–284. [Google Scholar] [CrossRef]
- Jing, B. Network externalities and market segmentation in a monopoly. Econ. Lett. 2007, 95, 7–13. [Google Scholar] [CrossRef]
- Zhang, Z.; Nan, G.; Li, M.; Tan, Y. Duopoly Pricing Strategy for Information Products with Premium Service: Free Product or Bundling? J. Manag. Inf. Syst. 2016, 33, 260–295. [Google Scholar] [CrossRef]
- Chen, P.; Zhao, R.; Yan, Y.; Li, X. Promotional pricing and online business model choice in the presence of retail competition. Omega 2020, 94, 102085. [Google Scholar] [CrossRef]

| Notation | Description |
|---|---|
| Decision variables | |
| The retail price set by the platform or the incumbent supplier under scenario i, i ∈{RM, RC, SM, SC} | |
| The retail price set by the entrant supplier under scenario j, j∈{RC, SC} | |
| The wholesale price set by the incumbent supplier under scenario m, m∈{RM, RC} | |
| Other variables | |
| The incumbent supplier’s profit under scenario i | |
| The entrant supplier’s profit under scenario j | |
| The platform’s profit under scenario i The incumbent supplier’s demand under scenario i The entrant supplier’s demand under scenario j | |
| Parameters | |
| s1/s2 | The logistics service level of the platform/entrant supplier |
| v | Product quality of the competitive goods |
| c | The entrant supplier’s opportunity cost incurred from joining the marketplace |
| λ | The revenue-sharing rate under the revenue-sharing self-operating channel mode and λ∈(0, 1) |
| ρ | The commission rate and ρ∈(0, 1) |
| t | The disutility cost per unit distance |
| α | The strength of the indirect network effects |
| N | The supplier number and N∈{1, 2} |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, B.; Huang, J.; Zhang, X.; Alejandro, T.B. Strategic Third-Party Product Entry and Mode Choice under Self-Operating Channels and Marketplace Competition: A Game-Theoretical Analysis. J. Theor. Appl. Electron. Commer. Res. 2024, 19, 73-94. https://doi.org/10.3390/jtaer19010005
Xu B, Huang J, Zhang X, Alejandro TB. Strategic Third-Party Product Entry and Mode Choice under Self-Operating Channels and Marketplace Competition: A Game-Theoretical Analysis. Journal of Theoretical and Applied Electronic Commerce Research. 2024; 19(1):73-94. https://doi.org/10.3390/jtaer19010005
Chicago/Turabian StyleXu, Biao, Jinting Huang, Xiaodan Zhang, and Thomas Brashear Alejandro. 2024. "Strategic Third-Party Product Entry and Mode Choice under Self-Operating Channels and Marketplace Competition: A Game-Theoretical Analysis" Journal of Theoretical and Applied Electronic Commerce Research 19, no. 1: 73-94. https://doi.org/10.3390/jtaer19010005
APA StyleXu, B., Huang, J., Zhang, X., & Alejandro, T. B. (2024). Strategic Third-Party Product Entry and Mode Choice under Self-Operating Channels and Marketplace Competition: A Game-Theoretical Analysis. Journal of Theoretical and Applied Electronic Commerce Research, 19(1), 73-94. https://doi.org/10.3390/jtaer19010005





