1. Introduction
With the rise of the platform economy, e-commerce platform selling has witnessed strong growth in recent decades [
1]. Global e-commerce sales reached
$3.53 trillion in 2019, and electronic retailing revenue is expected to grow to
$6.54 trillion by 2022. Traditionally, the platform has acted as a reseller in which the e-commerce platform owner purchases products from a manufacturer and then sells them to consumers [
2]. However, the rapid growth of online retailing has urged platforms to serve as a marketplace, allowing manufacturers to directly sell products to consumers and charging a platform fee for each sale [
3,
4]. In this paper, the former mode is called the “wholesale selling scheme”, whereas the latter mode is referred to as the “agency selling scheme”. Some giant platforms in the online retail industry, such as Taobao.com in China, Sears in the US, and Flipkart in India, have embraced the agency selling scheme [
5]. The agency selling scheme has also become prevalent in other industries, such as the e-book industry [
6,
7] and the app market [
8]. However, some major e-commerce platforms, e.g., BestBuy.com and Zappos.com, still use the wholesale selling scheme. The wholesale selling scheme continues to prevail as the dominant selling strategy in some industries, such as the music industry and apparel industry [
9]. Some recent literature about the e-commerce platform’s choice of selling scheme includes Wei et al. [
10], Liu and Ke [
11,
12], Chen et al. [
13], and Chen et al. [
14]. The above papers have discussed the impact of different factors, such as product complementarity, promotion, cross-channel spillover, and consumer loyalty, on the e-commerce platform’s selling scheme choice from the pure wholesale selling scheme and pure agency selling scheme. However, the above papers tend to assume that firms or consumers are perfectly rational, but, in fact, they are bounded rational.
As a type of bounded rationality, fairness concern acts as a key role in the decision-making behavior of manufacturers, retailers, and consumers [
15]. In this paper, we specifically focus on discussing the fairness concern from the perspective of the consumer. There are two kinds of consumer fairness concerns in the literature: one is taking the retail prices of peers as a reference point [
16,
17], and the other is treating the profit obtained by a participant of the supply chain as a reference point [
18,
19]. In this paper, we mainly investigate the latter kinds of consumer fairness concerns. We refer the reader to two seminal papers [
15,
20] in behavioral economics that examine the impact of consumer fairness concern on the firm strategy through theoretical models.
With the rapid development of the Internet, consumer fairness concerns toward transactions are growing due to increasing news reports about the trade inequality in different industries. For example, in the gasoline market, more and more news reports cover industry-collusion-increased gasoline prices, and gasoline companies make a large amount of profits [
17]. In the budget hotel industry, consumers who seek fairness in transactions will punish a hotel if the price is high. Under such a market, if consumers feel that the price of a hotel is unfair, they can easily turn to other hotels [
18]. In the catering industry, restaurants cheated the consumers by selling wine at too high prices [
19]. In the pharmaceutical industry, pharmaceutical firms reap huge profits from drugs [
21]. Consumers perceived that the retail prices of drugs are too high and pharmaceutical prices should be regulated. The above industry practices show that fairness-minded consumers are willing to sacrifice their monetary returns and sometimes even abandon deals to punish greedy sellers.
There is also anecdotal evidence that consumers may be concerned about the fairness of transactions. For example, Campbell [
22] found that consumers’ conjecture about corporate profits will affect their perceptions of price fairness. According to a survey of 4058 Americans conducted by BusinessWeek, approximately 73% of consumers deem the trading prices of products of giant American firms to be unfair and unreasonable, and the consumers will choose to give up buying products if they think that the price is unfair [
23]. More recently, Guo and Jiang [
18] conducted a survey of 170 students and showed that over 50% of students will not purchase products, even if the purchasing behavior might bring a return of
$5, when the students realized that the company’s marginal profit was
$18 or more. In the fields of economics and marketing, there is much literature on investigating consumer concerns about fairness, e.g., Guo [
17], Kahneman et al. [
24], and Xia et al. [
25]. Moreover, existing literature has shown that the consumers tend to think that the price of a firm is unfair when the profit of the firm is higher than the surplus of the consumer [
15,
26].
With the development of the Internet and the continuous updating of information technologies (such as blockchain and big data), consumers can better understand the information related to products through various channels. Then, products’ information, such as retail price and production cost, have become more transparent to consumers [
27,
28]. For example, Techinsights, a foreign business data analysis firm, recently disassembled Apple’s iPhone Pro MAX and analyzed its cost structure. The transparency of price and cost information enables fairness-minded consumers to clearly measure the gap between their own consumer surplus and the seller’s profit margin in the process of product purchase. When consumers think that merchants grab too high profits from product sales, consumers will feel that the transaction is unfair, and their willingness to pay will be reduced. Therefore, it is important to pay attention to the impact of consumer fairness concern on the members of the supply chain.
The above industry practices, anecdotal evidence, and existing literature all indicate that, when the profit of a firm is higher than the surplus of consumers, consumers tend to think that the firm’s price is unfair. Therefore, the total utility that consumers obtain from products relies on both the monetary return of the consumer and the profitability of the firm [
18]. Previous literature in behavioral economics has indicated that consumer fairness concerns act as a critical role in the strategies of consumers and firms [
15,
18,
20,
26]. The research focus on the existing literature is how to set a “fair” price for consumers and firms. However, the fairness concerns of consumers hold great consequences. For example, fairness-minded consumers are willing to sacrifice their monetary returns and sometimes even abandon deals to punish greedy sellers. In fact, approximately 85% of people are inclined to punish unfair transactions, even if that behavior is costly and not immediately rewarded [
29]. Therefore, the consumer fairness concern has a huge impact on the operation of firms, which will not only affect the firms’ profitability but also affect the strategic choice of firms, such as the selling scheme choices for e-commerce platforms.
Motivated by the above discussions, this paper is interested in the following research questions:
- (1)
In the presence of consumer fairness concerns, what is the strategic selling scheme for an e-commerce platform?
- (2)
Is the e-commerce platform more inclined to adopt a novel platform pricing scheme rather than a traditional wholesale pricing scheme, and, if so, under what conditions?
- (3)
Which pricing scheme would be preferred by the upstream manufacturer?
- (4)
How does the interaction between fairness concerns and an e-commerce platform’s selling scheme choice affect consumer surplus and social welfare?
To theoretically answer the above research questions, we adopted the rational expectations hypothesis, where economic outcomes are self-fulfilled and in line with people’s expectations [
30,
31,
32,
33]. In our research setting, on the one hand, given the expectation of the wholesale price and retail price, fair-minded consumers make purchase or non-purchase decisions. On the other hand, given the expectation of consumers’ willingness to pay, the manufacturer or platform determines the pricing decision. Furthermore, the expectation of every player in the supply chain system (i.e., manufacturer, platform, and consumers) is in line with actual outcomes. The rational expectations hypothesis is widely used in several domains, such as two-sided market [
34], finance [
35], online platforms [
36], etc.
Based on the rational expectations hypothesis, we examined the desirability of an e-commerce platform’s selling scheme choice in the presence of consumers’ fairness concern behavior. The purpose of this paper is to investigate an e-commerce platform’s selling scheme choice when it adopts a wholesale selling scheme or an agency selling scheme to make a contract with a manufacturer in the presence of consumer fairness concerns. In particular, we established a supply chain game consisting of a single upstream manufacturer and a single downstream platform with a continuum of consumers with fairness concerns. The downstream platform can use the traditional wholesale selling scheme or adopt the novel agency selling scheme. We first obtained equilibrium results under the two selling schemes, and then compared the two schemes from the perspectives of the platform’s profit, the manufacturer’s profit, consumer surplus, and social welfare. For parsimony and analytical tractability, our baseline model characterized consumers as homogenous about fairness concerns. We also extended the baseline model to consider heterogeneous consumers in terms of fairness concerns, proportional platform fee, fairness concerns about the manufacturer’s profit, and endogenous platform fee.
Our research provides several managerial findings. First, the intensity of fairness concern and the platform fee are two key factors affecting the optimal selling scheme choice of the upstream platform. More specifically, our results reveal that, when these two factors are relatively high or low, the traditional wholesale selling scheme outperforms the new agency selling scheme in terms of the e-commerce platform’s profit. Otherwise, the e-commerce platform should adopt the agency selling scheme. Intuitively, when the fairness concern intensity is relatively low, a low platform fee implies that the platform can only retain a small part of the revenue, which is not conducive to the platform’s adoption of the agency selling scheme. However, when the fairness concern intensity is relatively high, as a rational economist, the platform will transfer the control rights for product pricing to the manufacturer to avoid suffering more loss. Consequently, the platform will use the wholesale selling scheme in this case. The above results are consistent with the industry practice wherein both selling schemes coexist. In the luxury industry, many brands, such as Hermes, Armani, and Louis Vuitton, have adopted the agency selling scheme to distribute their products. This is because consumers who buy luxury goods usually care little about fairness: they are fairness-neutral. However, in the budget hotel industry, platforms usually use the wholesale selling scheme. This is because consumers who use budget hotels usually care more about fairness: they are fairness-minded. If consumers feel that the price of a hotel is unfair, they can easily turn to other hotels.
Second, we find that, when the fairness concern intensity is relatively high and the platform fee is relatively low or vice versa, both members of the supply chain system will benefit from the agency selling scheme. The intuition of the win-win result relies on the trade-off between the double marginalization effect and the fairness concern effect. If the fairness concern intensity and the platform fee are either high or low enough, then the fairness concern effect will dominate the double marginalization effect, making both members better off. Otherwise, the double marginalization effect dominates the fairness concern effect, and both members are worse off. Furthermore, we obtain a win-win-win result where the upstream manufacturer, the downstream platform, and end consumers all benefit from the novel agency selling scheme when the platform fee is relatively low.
Third, we find that the e-commerce platform’s profit first increases and then decreases with the platform fee. Moreover, there exists a threshold for the platform fee, below which, adopting the wholesale selling scheme will reduce the market share for the e-commerce platform and, above which, adopting the wholesale selling scheme will increase the platform’s market share. If the platform fee is relatively low, i.e., using the agency selling scheme to sell the product is not expensive for the manufacturer, then the double marginalization effect derived from the decentralized channel structure will dominate the fairness concern effect derived from consumers’ utility inequity. However, if the platform fee is relatively high, i.e., the agency selling scheme is very costly, then the fairness concern effect dominates the double marginalization effect.
To reflect the fact that some consumers are fairness-minded whereas others are fairness-neutral, we extended the core model to capture consumer heterogeneity of the fairness concerns. Our analysis reveals that the main insights derived from the baseline model remain robust. We also obtain several new insights into the extension of considering consumer heterogeneity. Interestingly, the profit of the manufacturer has nothing to do with the fraction of the fairness-minded segment under the wholesale selling scheme, whereas the manufacturer’s profit first increases and then decreases with the fraction of the fairness-minded segment under the agency selling scheme. The intuition behind this insight is as follows. On the one hand, intuitively, a larger fraction of the fairness-minded segment induces the platform to set a lower platform fee, which, in turn, indicates that the transaction is fair, and then more consumers buy the product. The lower platform fee and higher market share give the manufacturer more profits. On the other hand, when the proportion of fairness-minded consumers is small, the platform has no incentive to reduce the platform fee. In this case, consumers feel that the transaction is unfair, and then more transactions are interrupted, which, in turn, hurts the manufacturer. In addition, we also extended our baseline model to investigate the exogenous proportion platform fee, fairness concern about the manufacturer’s profit, and endogenous platform fee. The theoretical analysis reveals that the main conclusions presented in the baseline model remain qualitatively unchanged.
Compared with extant studies, our research contributes to the following two aspects. On the one hand, we contribute to the research stream of fairness concerns in distribution channel management by discussing the impact of the consumer fairness concern on the whole supply chain system and finding that the wholesale selling scheme can derive a win-win outcome for the platform and the manufacturer when the intensity of the fairness concerns and the platform fee is sufficiently large or small. On the other hand, this paper contributes to the literature on selling scheme choice in platform retailing by considering the consumer fairness concern. In the presence of fairness concerns, we illustrate a win-win-win result where the upstream manufacturer, the downstream platform, and end consumers all benefit from the novel agency selling scheme.
The rest of this paper is organized as follows.
Section 2 reviews the related literature. In
Section 3, we set up the main model.
Section 4 presents the equilibrium analysis of the game-theoretical models. In
Section 5, we compare the two models and derive some managerial insights.
Section 6 presents four extensions of the baseline model. In
Section 7, we compare the results obtained with similar previous research and with our paper’s opinion on the differences. We provide several managerial implications and outline future research directions in
Section 8. All proofs of lemmas, corollaries, and propositions are provided in
Appendix A.
5. Comparison
Based on the research results in
Section 4, this section compares the two selling schemes from five perspectives: market share, retail price, profit, consumer surplus, and social welfare. Next, we first discuss the comparison results from the perspective of market share.
5.1. Market Share
Proposition 2. For a lower platform fee (i.e., ), the market share under the agency selling scheme is larger. Otherwise (i.e., , the market share under the wholesale selling scheme is larger.
Proposition 2 illustrates that there exists a threshold for the platform fee, above which, the agency selling scheme will reduce the market share for the e-commerce platform and, below which, it will increase the platform’s market share. Naturally, common wisdom from existing literature suggests that, due to the double-marginalization effect, the market share under the agency selling scheme is larger (see, Hao and Tan [
3] and Geng et al. [
45] for instance). However, in contrast to the results of the conventional literature in the absence of consumers’ fairness concern behavior (e.g., Tan et al. [
6], Hao and Fan [
43], and Tan and Carrillo [
44]), we show that the wholesale selling scheme does not necessarily induce a lower market share compared with the agency selling scheme. The platform fee has two effects on the market share―a direct receding effect and an indirect enhancing effect. First, the direct receding effect leads to a reduction in the market share for the agency selling. Second, the higher the commission fee, the lower the retail price of the product. The lower retail price will increase the market share. We refer to this effect as the indirect enhancing effect. Therefore, for a lower platform fee (i.e.,
), then the indirect enhancing effect dominates the direct receding effect, which, in turn, leads to the platform gaining more market share under the agency selling scheme. However, for a higher platform fee (i.e.,
), the direct receding effect dominates the indirect enhancing effect. Thus, the wholesale selling scheme will create more market share.
5.2. Retail Price
Proposition 3. For a lower platform fee (i.e., ), the retail price in the wholesale selling scheme is larger. Otherwise, (i.e., ), the retail price in the agency selling scheme is larger.
Previous literature in the absence of the fairness concern suggests that wholesale selling will always result in a higher retail price (e.g., [
43,
44]). In contrast to the above common wisdom, our results reveal that which selling scheme results in a high retail price depending on the platform fee. In particular, for a lower platform fee (i.e.,
), the common finding is that the wholesale selling scheme always leads to a higher retail price hold. However, for a higher platform fee (i.e.,
), the reverse result holds. Similar to the explanation of Proposition 2, the above result is caused by two effects—a direct effect and an indirect effect. The indirect effect of the platform fees on the retail price may be positive or negative. Specifically, for a higher platform fee (i.e.,
), the indirect effect of the platform fee on the retail price is positive, and, therefore, the platform will set a higher retail price. For a lower platform fee (i.e.,
), however, the indirect effect of the platform fee on the retail price is negative, and, therefore, the platform will decide on a lower retail price.
5.3. Profit
Based on the above comparison results for market share and retail price, we now provide an equilibrium selling scheme for the platform and the manufacturer and determine the conditions under which the supply chain participants can achieve a win-win situation. For simplicity, we denote two threshold values for the fairness concern intensity: these are and .
Proposition 4. For comparing the platform’s profit under the wholesale selling scheme () and the agency selling scheme (), we have:
- (a)
For a lower platform fee (i.e.,),
- (1)
If the fairness concern intensity is relatively low (i.e., ), then;
- (2)
If the fairness concern intensity is relatively high (i.e.,), then.
- (b)
For a higher platform fee (i.e.,),
- (1)
If the fairness concern intensity is relatively low (i.e.,), then;
- (2)
If the fairness concern intensity is relatively high (i.e.,), then.
Proposition 4 illustrates that the platform’s optimal choice relies on two key factors: the intensity of fairness concern induced by the consumer’s utility inequity and the platform fee charged by the platform for each sale. In particular, Proposition 4(a) illustrates that, when the platform fee and the fairness concern intensity are both relatively low, the platform is inclined to use the wholesale selling scheme. However, for a lower platform fee and a higher fairness concern intensity, the platform will choose the agency selling scheme. The intuition driving this result is as follows. First, for a lower fairness concern intensity, a lower platform fee implies that the platform can only retain a small part of the revenue, which is not conducive to the platform’s adoption of the agency selling scheme. Second, when the fairness concern intensity is relatively high, as a rational economist, the platform will transfer the control rights for product pricing to the manufacturer to avoid suffering more loss. Consequently, the platform will use the wholesale selling scheme in this case.
More interestingly, Proposition 4(b) shows that, when the intensity of fairness concern is high enough (i.e., ), the platforms still tend to adopt the wholesale selling scheme. To pursue more market share, the platform or the manufacturer will aggressively reduce the retail price. This aggressive price reduction dominates the large platform fee in terms of its impacts on the platform’s profit under the agency selling scheme. Under such a situation, consequently, the platform still prefers the wholesale selling scheme. Alternatively, for a higher platform fee and a lower fairness concern intensity, the platform will choose the agency selling scheme.
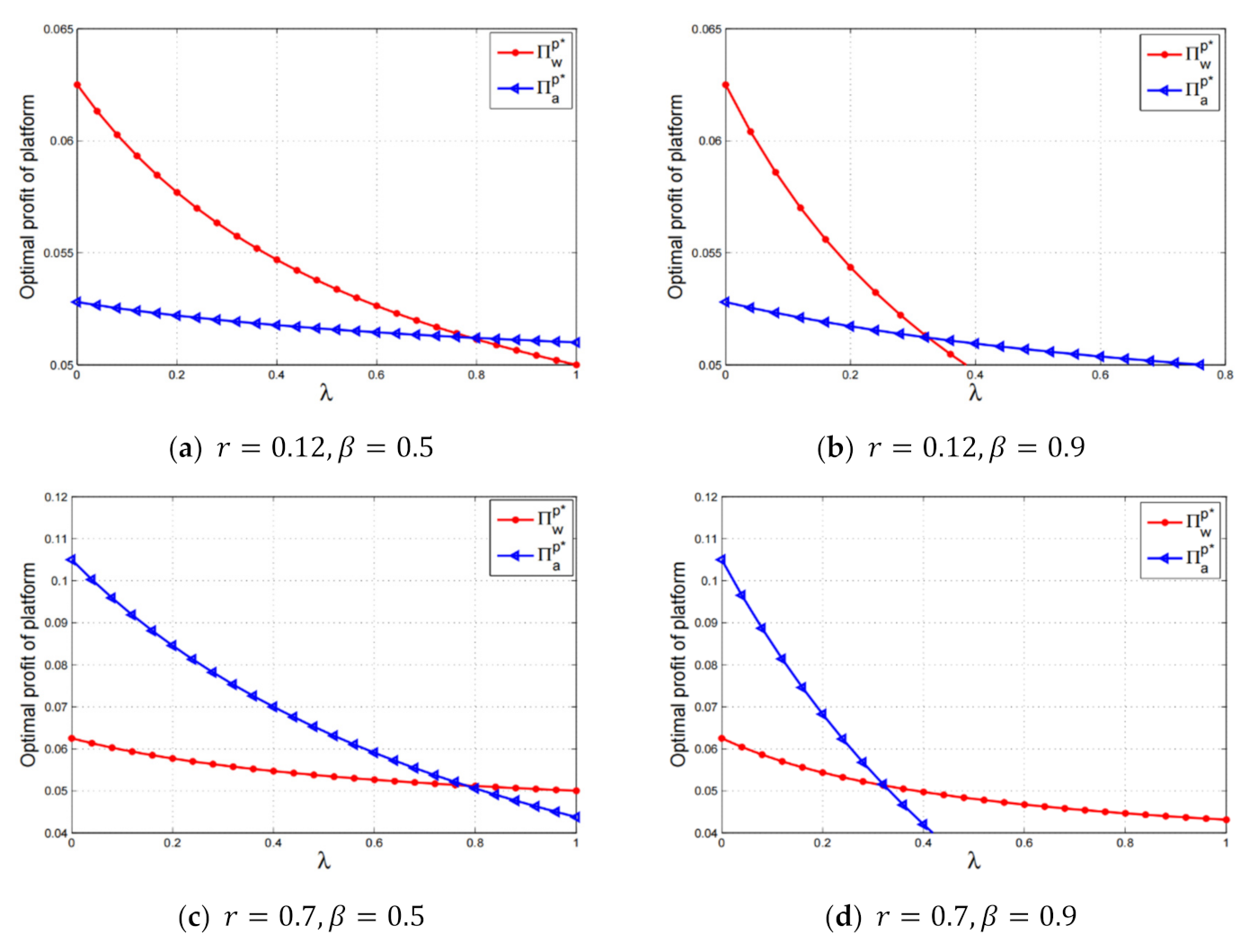
The above results theoretically prove that an increasing number of e-commerce platforms embrace the agency selling scheme. The results shown in Proposition 4 are shown in
Figure 3. The results shown in Lemmas 1 and 2 illustrate that the optimal profits for the platform under the two selling schemes only depend on the fairness concern intensity and the platform fee. Therefore, we set
and
.
Figure 3a,b correspond to the results shown in Proposition 4(a) and Proposition 4(b), respectively.
From the platform’s perspective, Proposition 4 indicates some new managerial insights. Existing literature (e.g., [
43,
44]) investigated the monopoly case and showed that the agency selling scheme always dominates the wholesale selling scheme due to the revenue sharing structure in the agency selling scheme. However, under the same monopoly setting, we show that the agency selling scheme does not necessarily bring more benefits to the platform. Moreover, the agency selling scheme adopted by the platform is consistent with recent business development. Recently, several well-known e-commerce platforms in various industries, such as the travel agency platforms Priceline.com and Expedia.com in the travel industry, the retailing platforms Amazon and JD.com in the online retail industry, and group-buying platforms such as LivingSocial and Groupon in the online sales promotion industry, have begun to adopt the agency selling scheme.
Next, we compare the optimal profit for the upstream manufacturer under the two selling schemes to identify the optimal strategy for the manufacturer. For simplicity, we denote . The following proposition summarizes the comparison result.
Proposition 5. From the perspective of the upstream manufacturer, we have:
- (a)
When the platform fee is relatively low (i.e.,), then.
- (b)
When the platform fee is relatively high (i.e.,1), then.
- (c)
When the platform fee is in the intermediate range (i.e.,),
- (1)
If the fairness concern intensity is sufficiently low (i.e.,), then;
- (2)
If the fairness concern intensity is sufficiently high (i.e.,), then.
Proposition 5 illustrates that the manufacturer’s preference towards the two selling schemes depends on the platform fee and the fairness concern intensity. In particular, Proposition 5(a) indicates that, for a lower platform fee, the manufacturer will choose the agency selling. The manufacturer can gain more profits by using the agency selling scheme for a lower platform fee. Proposition 5(b) illustrates that, for a higher platform fee, the wholesale selling scheme is the dominant strategy for the upstream manufacturer. The driving force of this result is that the manufacturer will suffer more loss by using the agency selling scheme with a higher platform fee. Therefore, the manufacturer prefers to use the wholesale selling scheme in such cases.
However, for the intermediate platform fee, the optimal strategy may be more complex. In such a situation, the manufacturer should consider consumers’ fairness concern behavior when making the selling scheme decision. Proposition 5(c) illustrates that consumers’ desire for fairness in transactions may make the manufacturer deviate from the agency selling scheme to the wholesale selling scheme. When the fairness concern intensity is sufficiently high (i.e., ), then the improvement of the sense of fairness can dominate the market share reduction, and thus make the manufacturer better off. Consequently, the manufacturer may adopt the wholesale selling scheme in such a situation. The above results depend on interactions between the double marginalization effect on the supply side and consumers’ fairness concerns on the demand side.
Based on the results shown in Propositions 4 and 5, we have the following proposition.
Proposition 6. (Win-win result) From the perspective of the whole supply chain system, we have:
- (a)
For a lower platform fee (i.e.,),
- (1)
If the intensity of fairness concern is relatively low (i.e., ), then the wholesale selling scheme is the dominant strategy for the whole supply chain;
- (2)
If the intensity of fairness concern is relatively high (i.e., ), then both the upstream manufacturer and the downstream platform prefer to choose the agency selling scheme.
- (b)
For a higher platform fee (i.e.,),
- (1)
If the intensity of fairness concern is relatively low (i.e., ), then the agency selling scheme is the dominant strategy for the whole supply chain;
- (2)
If the intensity of fairness concern is relatively high (i.e., ), then both the upstream manufacturer and the downstream platform prefer to adopt the wholesale selling scheme.
Proposition 6 shows that, under the low platform fee setting when the fairness concern intensity is relatively low, both the manufacturer and the platform prefer the wholesale selling scheme. Interestingly, when the intensity of fairness concern is relatively high, the agency selling scheme is the dominant strategy for the whole supply chain. Essentially, the double marginalization effect and consumers’ fairness concern effect lead to the above results. Specifically, when consumers are sensitive enough to unfairness in transactions (i.e., ), their fairness concern effect dominates the double marginalization effect. However, when consumers are not sensitive enough to inequity in transactions (i.e., ), then the double marginalization effect dominates the fairness concern effect.
Alternatively, under the high platform fee setting, Proposition 6(b) draws the opposite conclusion compared with Proposition 6(a). First, Proposition 6(b) reveals that, when the fairness concern intensity is low (i.e., ), the manufacturer is inclined to use the agency selling. This is because the manufacturer’s strategy depends on the fairness concern intensity when considering fairness-minded consumers. Second, Proposition 6(b) indicates that, when the fairness concern intensity is relatively high (i.e., ), then the manufacturer’s preference may switch from the agency selling scheme to the wholesale selling scheme. The intuition behind this result is as follows. A higher implies that consumers are sufficiently sensitive to unfairness during the transaction process. As shown in Proposition 3, the retail price in the agency selling scheme is higher when consumers are sufficiently sensitive to unfairness during the transaction process. A higher retail price of the product makes consumers feel that the transaction is more unfair. Note that, under such a situation, the manufacturer should be willing to provide more payoffs to the platform due to the higher platform fee. In order to avoid suffering more losses, the manufacturer should use the wholesale selling scheme. Therefore, when is relatively high (i.e., ), both the upstream manufacturer and the downstream platform prefer the wholesale selling scheme.
5.4. Consumer Surplus and Social Welfare
In this section, we discuss the implications of the platform’s selling scheme decision on consumer surplus and social welfare. Consumer surplus under the wholesale selling scheme is as follows:
whereas, under the agency selling scheme, it can be described as follows:
We compared consumer surpluses under the two selling schemes to determine which scheme is optimal for consumers; that is, whether consumers can gain more utility under the agency or the wholesale selling scheme. The comparison result is summarized in Proposition 7.
Proposition 7. For a lower platform fee (i.e., ), consumers will be better off under the agency selling scheme. Otherwise (i.e., ), consumers will be better off under the wholesale selling scheme.
Proposition 7 shows that the comparison result for the two selling schemes in terms of consumer surplus depends on the platform fee. In general, consumer surplus depends on two factors: retail price and market share. However, based on the results shown in Propositions 2 and 3, consumer surplus in our model depends on three factors: retail price, market share, and platform fee. In particular, a lower platform fee (i.e., ) reduces the retail price and increases the number of consumers who buy the product (i.e., increasing the market share) under the agency selling scheme. Therefore, in such a situation, consumers gain more utility when the platform adopts the agency selling scheme. However, recall that the market share under the agency selling scheme may be smaller for a higher platform fee, which is shown in Proposition 2, and the retail price in the agency selling may be larger for a higher platform fee, which is shown in Proposition 3. The smaller market shares and higher retail prices hurt consumer surplus. Therefore, in such a situation, consumers gain more utility when the platform adopts the wholesale selling scheme. However, for a higher platform fee (i.e., , the reverse conclusion holds.
We then discuss the social welfare under the two pricing schemes. Following Tan and Carrillo [
44] and Kwark et al. [
49], social welfare is the sum of the profits of the manufacturer and e-commerce platform and consumer welfare. Therefore, the social welfare under the wholesale selling is shown as follows:
whereas social welfare under the agency selling is shown as follows:
Due to the complex form of social welfare, analytical comparisons between the agency and wholesale selling schemes are very complicated. We use numerical analysis to gain managerial implications regarding the social welfare comparison.
Figure 4 shows that social welfare under the two selling schemes decreases with the fairness concern intensity. First, for a lower platform fee,
Figure 4a illustrates that the agency selling scheme outperforms the wholesale selling scheme in terms of social welfare. Second, when the platform fee is in the intermediate range,
Figure 4b illustrates that the comparison between social welfare under the two selling schemes changes as
varies. When the platform fee is in the intermediate range, both selling schemes can be used by the manufacturer and the platform. Under the setting of consumers’ fairness concerns, both parties’ choice depends on the fairness concern intensity. In particular, when the fairness concern is small enough, both parties will choose the agency selling scheme, as shown in Proposition 6, and consumers will obtain more utility under the agency selling scheme, as shown in Proposition 7. Therefore, the wholesale selling scheme results in the highest social welfare in this situation. When the fairness concern is large enough, the reverse conclusion holds; that is, the agency selling scheme results in the highest social welfare. Finally, when the platform fee is relatively high,
Figure 4c illustrates that the wholesale selling scheme outperforms the agency selling scheme in terms of social welfare.
6. Extensions
This section investigates two extensions of our baseline model.
Section 6.1 examines how the heterogeneous consumer behavior impacts the platform’s optimal selling scheme selection. In
Section 6.2, we investigate the case in which the platform takes a proportion of the selling price in the agency selling scheme.
Section 6.3 studies the situation where consumers care about fairness between the manufacturer’s profit and their own surplus. In
Section 6.4, we discuss the endogenous commission fee. We find that the main conclusions presented in the baseline model remain qualitatively unchanged in the four extensions.
6.1. Consumer Heterogeneity
In the baseline model, all consumers have the same fairness concern intensity. In reality, however, the consumer market may be heterogeneous; that is, a proportion of consumers are fairness-minded, whereas others are fairness-neutral and focus on their economic surplus [
18]. To describe these kinds of market practices, we next examine how the heterogeneous consumer behavior impacts the platform’s optimal selling scheme selection. Following the model in Guo [
17], the consumer market consists of two segments in this extension. The first segment is fairness-minded consumers, and the fraction of such consumers is
(
1). If the transaction is deemed unfair, even if it results in a higher monetary payoff than not buying, the consumers in this segment may abandon the purchase. The other segment of consumers is fairness-neutral and may not care about whether the transaction is fair or not. The fraction of this segment is
. The first segment is the fairness-minded segment, whereas the other segment is the fairness-neutral segment. The above model setup is consistent with empirical evidence that consumers’ perception of fairness of large US companies is heterogeneous [
17]. Note that, when
, this extension degenerates to the baseline model. Subscripts
and
denote the fairness-minded segment and the fairness-neutral segment, respectively.
For the fairness-minded segment, the utility function of a consumer is the same as that in the baseline model. Under the wholesale selling scheme, the utility function of the consumer is , whereas, under the agency selling scheme, the utility function of the consumer is . Here, the reservation value is uniformly distributed between 0 and 1. For the fairness-neutral segment, consumers may not care about whether the transaction is fair or not. Therefore, under the wholesale selling scheme, the consumer’s utility function is , whereas, under the agency selling scheme, the consumer’s utility function is . Here, the reservation value is uniformly distributed between 0 and 1.
Under the wholesale selling scheme, the fairness-minded consumers will buy one product if
and fairness-neutral consumers will buy one product if
. Note that the reservation values
and
are both uniformly distributed between 0 and 1. According to the theory of rational expectation equilibrium (e.g., [
19,
32,
33]), the market share under the wholesale selling scheme is
Similarly, under the agency selling scheme, the fairness-minded consumers will buy one product if
and fairness-neutral consumers will buy one product if
. Therefore, the market share under the agency selling scheme is
.
6.1.1. Wholesale Selling Scheme
Under the wholesale selling scheme, the game-theoretical models for both parties of the e-commerce supply chain are the same as the baseline model. Based on the first-order condition and backward induction, the equilibrium results under the wholesale selling scheme are summarized in Lemma 3.
Lemma 3. Under the wholesale selling scheme, the manufacturer charges the wholesale price and the platform sets the retail price The market demand is The profits of the manufacturer, the platform, and the supply chain system are , , and , respectively.
It can be verified that the optimal retail price and the platform’s optimal profit are identical to the equilibrium results presented in Lemma 1 by setting . Next, we discuss the effects of the fairness-minded segment fraction on the optimal retail price and the platform’s optimal profit.
Corollary 1. Under and .
Corollary 1 shows that the retail price and the platform’s profit decrease with the fairness-minded segment fraction . This is because, the more the consumers are fairness-minded, the greater the likelihood that a transaction will be interrupted or broken. Therefore, the platform has no choice but to decrease the retail price to avoid losing consumers. The reduction in retail price, in turn, influences the decline in profits.
6.1.2. Agency Selling Scheme
Under the agency selling scheme, the manufacturer maximizes its profit by setting the retail price
given the platform fee
predetermined by the platform owner; that is,
Based on the first-order condition, the equilibrium results under the agency selling scheme are summarized in Lemma 4.
Lemma 4. Under the agency selling scheme, the manufacturer charges the retail price . The market demand is . The profits of the manufacturer, the platform, and the supply chain system are , , and , respectively.
Similar to Lemma 3, Lemma 4 degenerates to the counterpart Lemma 2 shown in the baseline model by setting . Next, we discuss the effects of the fairness-minded segment fraction on the equilibrium results under the agency selling scheme.
Proposition 8. - (a)
,
, and .
- (b)
If the fraction of the fairness-minded consumer is large (i.e., ), then . However, if the fraction of the fairness-minded consumer is small (i.e., ), then .
In line with Corollary 1, Proposition 8(a) shows that the retail price and the platform’s profit decrease with the fairness-minded segment fraction . Proposition 8(a) also illustrates that the market share decreases with . Intuitively, as increases, more transactions will break due to the unfairness in the transaction process. Therefore, the market share will decrease. Proposition 8(b) provides a new insight: when the fraction of the fairness-minded consumers is sufficiently large (i.e., ), the manufacturer’s profit increases with the fraction . Otherwise, the reverse relationship holds. The intuition behind this insight is as follows. On the one hand, intuitively, a larger induces the platform to set a lower platform fee, which, in turn, reveals that the transaction is fair, and then more consumers buy the product. The lower platform fee and the higher market share make the manufacturer gain more profit. Thus, a larger benefits the manufacturer’s profit. On the other hand, when the fraction of the fairness-minded consumers is small (i.e., ), then the platform has no incentive to reduce the platform fee. In such a case, consumers feel that the transaction is very unfair and then the transaction is more likely to break. Therefore, a smaller leads to a lower market share, which, in turn, hurts the manufacturer.
6.1.3. Comparison
The following corollary presents the comparison result about the market share.
Corollary 2. If the platform fee is relatively low (i.e., ), then the market share under the agency selling scheme is larger than that under the wholesale selling scheme. If the platform fee is relatively high (i.e., ), then the market share under the wholesale selling scheme is larger than that under the agency selling scheme.
Corollary 2 illustrates that the comparison result about the market share depends on the platform fee. Particularly, for a lower platform fee, the agency selling scheme leads to more of a market share than the wholesale selling scheme. Otherwise, the reverse relation holds. Corollary 2 confirms that our insight in Proposition 2 under the baseline model remains qualitatively unchanged. We next summarize the comparison result about the retail price under the two selling schemes.
Corollary 3. If the platform fee is relatively low (i.e., ), then the retail price under the wholesale selling scheme is larger than that under the agency selling scheme. If the platform fee is relatively high (i.e., ), then the retail price under the agency selling scheme is larger than that under the wholesale selling scheme.
Corollary 3 illustrates the comparison results in Proposition 3 under the baseline model; that is, a lower platform fee results in a larger retail price under the wholesale selling scheme, which remains qualitatively unchanged.
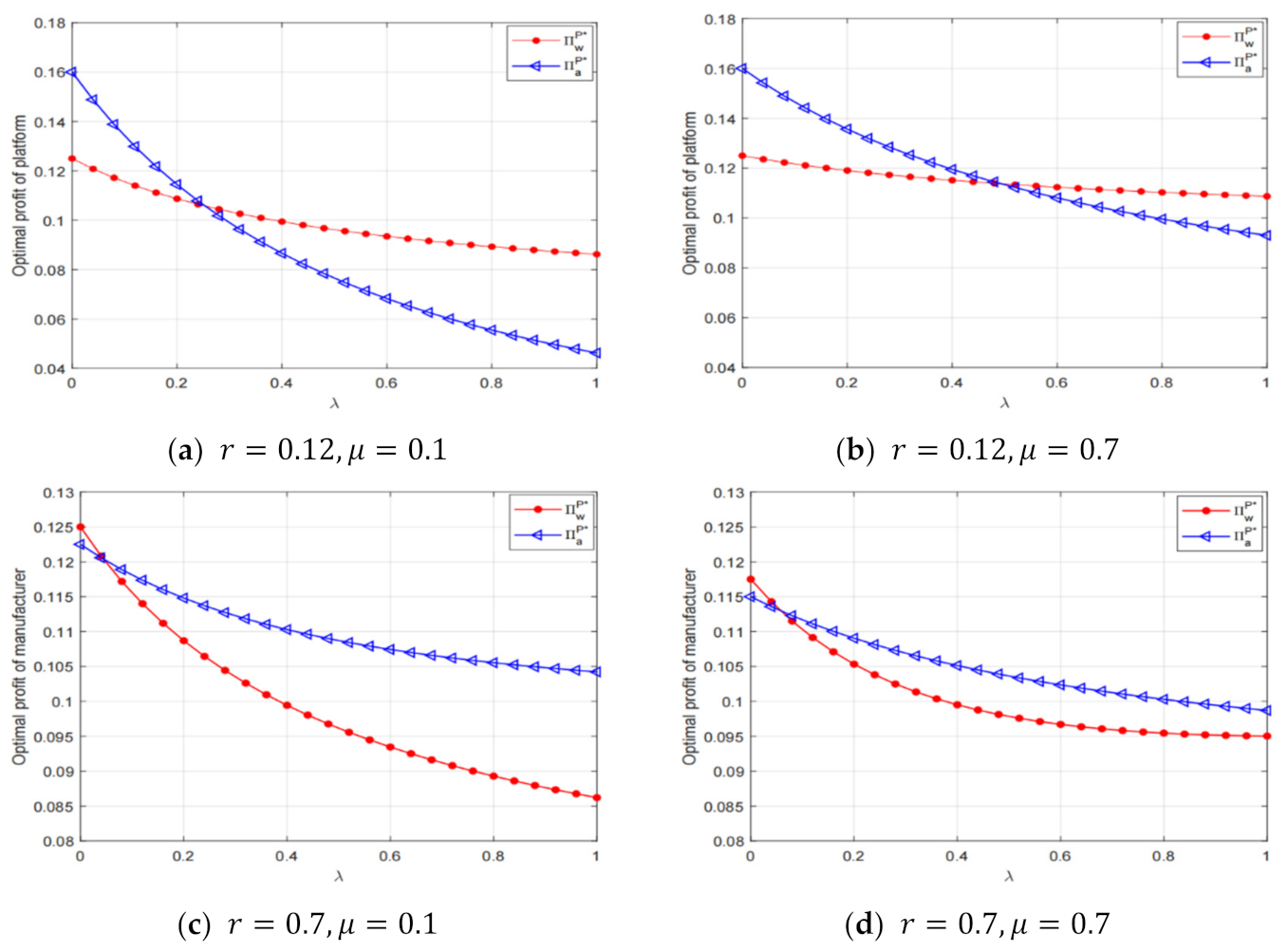
Due to the complexity of the expressions of the equilibrium profit, we present a numerical study. First, from the perspective of the platform, as can be seen from
Figure 5a,b, when the platform fee is low enough, the lower fairness concern intensity induces more profit for the platform under the wholesale selling scheme. However, when the platform fee is low enough, the higher fairness concern intensity generates more profit for the platform under the agency selling scheme.
Figure 5c,d indicate that, for a higher platform fee, the reverse relation of the above results holds. In summary,
Figure 5 illustrates that the comparison of the platform’s profit is independent of the fraction of fairness-minded consumers. Such results illustrate the robustness of Proposition 4 in our baseline model. Second, from the perspective of the manufacturer, we can also verify that the conclusion presented in Proposition 5 remains qualitatively unchanged.
In summary, the main conclusions presented in the baseline model remain qualitatively unchanged. Interestingly, this extension of consumer heterogeneity leads to a new finding: the manufacturer’s profit shows an inverted-U-shape curve relative to the fraction of the fairness-minded segment.
6.2. Proportional Platform Fee
In the baseline model, the platform fee is considered as a fixed fee per one transaction in the agency selling scheme. However, the platform will take a proportion of the selling price in the agency model [
43,
44]. This extension discusses the role of proportional platform fees during the transaction between the manufacturer and the platform. We denote
as the proportional platform fee. The other notations remain the same as the baseline model. Therefore, the purchase utility function under the agency selling scheme is
. Letting
, we have
. Consumers will buy one product if
, i.e.,
. According to the theory of rational expectation equilibrium (e.g., [
19,
32,
33]), under the agency selling scheme, the fairness-minded consumer’s demand function is
. The manufacturer maximizes its profit by setting the retail price
given the proportional platform fee
predetermined by the platform owner; that is,
Based on the first-order condition, the equilibrium results under the agency selling scheme are shown in Lemma 5.
Lemma 5. Under the agency selling scheme, the manufacturer charges the retail price . The market share is . The optimal profits of the manufacturer, the platform, and the supply chain system are , , and , respectively.
Lemma 5 reveals the following findings. As expected, the profit of the manufacturer decreases with the proportional platform fee (i.e., ), whereas the platform’s profit increases with (i.e., ). In other words, the manufacturer will be worse off and the platform will be better off with the higher platform fee. However, the supply chain system’s total profit decreases with the proportional platform fee (i.e., ). Setting a higher commission fee is not a good choice for the whole supply chain system. When the platform obtains more of a profit from the transaction, the fairness consumers will buy fewer products, and may even not buy the product from the platform. Therefore, a moderate proportional commission fee is needed for the sustainable operation of the supply chain system.
For simplicity, we denote two threshold values for the fairness concern intensity; that is, and .
Proposition 9. If and or and , then the agency pricing model benefits the manufacturer and the platform.
Proposition 9 shows that the fairness concern intensity and the commission fee are two key factors affecting the manufacturer’s and platform’s preferences with regard to the selling scheme. In particular, when these two factors are relatively high or low, the wholesale selling scheme outperforms the agency selling scheme in terms of the manufacturer’s and platform’s profits. Otherwise, the agency selling scheme is the dominant strategy for both participants. These results are consistent with Proposition 6 shown in the baseline model. Therefore, our main conclusions remain qualitatively unchanged when the platform fee is a proportion of the selling price in the agency model.
6.3. Fairness Concern about the Manufacturer’s Profit
In the baseline model, we have assumed that the fairness-minded consumers care about the fairness between the platform’s profit and their surplus. In this section, we investigate the situation where consumers care about fairness between the manufacturer’s profit and their surplus. Following Guo and Jiang [
18] and Yi et al. [
19], the purchase utility function of the consumer with fairness concerns under the wholesale selling scheme is
, where
is the monetary payoff derived from a product, and
measures the influence of consumer fairness concern on its utility. Similarly, when the platform adopts the agency selling scheme to sell a product, the purchase utility function of the fairness-minded consumer is
, where the monetary payoff derived from a product is
. The impact of consumer fairness concern on its utility is measured as
. Consumers will only buy a product if its total utility is non-negative; that is,
or
.
Similar to the baseline model, according to the theory of rational expectation equilibrium (e.g., [
19,
32,
33]), the fairness-minded consumer’s demand function under the wholesale selling scheme and the agency selling scheme are
and
, respectively.
When the consumers care about fairness between the manufacturer’s profit and their surplus, the game-theoretical model for both parties of the e-commerce supply chain under the two selling schemes is the same as the baseline model. Based on the backward induction, we can obtain the following equilibrium results.
Lemma 6. - (a)
Under the wholesale selling scheme, the manufacturer charges the wholesale price and the platform determines the retail price . The market share is . The profits of the manufacturer, platform, and supply chain system are , , and , respectively.
- (b)
Under the agency selling scheme, the manufacturer charges the retail price . The market share is . The optimal profits of the manufacturer, the platform, and the supply chain system are , , and , respectively.
Next, we further investigate the situation where the fairness-minded consumers care about the profits of both the manufacturer and the platform. Under such a situation, fairness-minded consumers may weigh on the fairness of trading for both the platform (say,
) and the manufacturer (say,
). Following Guo and Jiang [
18] and Yi et al. [
19], the purchase utility function of the consumer with fairness concerns under the wholesale selling scheme can be expressed as
and the purchase utility function of the consumer with fairness concerns under the agency selling scheme can be expressed as
Consumers will buy a product if and only if the total utility is non-negative; that is, or . Note that, when , this model degenerates to the baseline model, and when , this model degenerates to the model that we have investigated above.
Now, owing to the complexity of the game-theoretical model, we carried out several numerical experiments to address the e-commerce platform’s selling scheme decision. In the numerical experiments, we set the fairness to weigh between the platform and the manufacturer as
and
to drive
Figure 6. The results show that there is no difference in the selling scheme preference for the platform, as reflected in
Figure 3 and
Figure 6. In other words, the main conclusions presented in the baseline model remain qualitatively unchanged.
6.4. Endogenous Platform Fee
In the main model, we assumed that the platform fee charged by the platform is an exogenous constant. A natural extension of this assumption is to investigate what factors may influence the platform fee. In this extension, we discuss the situation where the platform fee is an endogenous variable that is determined through negotiations between the manufacturer and the platform.
To reflect each party’s impact on the platform fee, we employed the standard Nash bargaining solution (Li et al. [
66]), generalized to allow for asymmetric bargaining power. Let
reflect the relative bargaining influence of the platform and
represent the bargaining power of the manufacturer. As a result, the endogenous platform fee,
, is the solution of the following Nash bargaining formulation:
where
and
are the profits of platform and manufacturer under the agency selling scheme, respectively.
The Nash bargaining solution and managerial insights are summarized as follows.
Proposition 10. When the platform fee, , is determined through Nash bargaining:
- (a)
The optimal platform fee is .
- (b)
For , the optimal platform fee increases with the bargaining power of the platform (i.e., ) and decreases with the intensity of consumer fairness concern (i.e., ).
Proposition 10 provides several expected results (which lends confidence to the validity of the model). As expected, the first part of Proposition 10(b) illustrates that the optimal platform fee increases with the bargaining power of the platform. That is, the platform can obtain a higher revenue during the transaction with the manufacturer, as they have more bargaining power. The second part of Proposition 10(b) reveals that the optimal platform fee decreases with the intensity of consumer fairness concerns. The intuition behind this result is as follows. When the intensity of consumer fairness concern becomes large, the consumers are more unwilling to buy products from greedy firms. In order to avoid losing more consumers, the platform will take less from the supply chain system. Therefore, Proposition 10 is consistent with the main insight that a larger platform fee may be harmful to the platform in the presence of fairness-minded consumers.