Numerical Analysis for the Fractional Ambartsumian Equation via the Homotopy Herturbation Method
Abstract
:1. Introduction
2. Basic Concepts
3. Application of the HPM
4. Unified Formula for
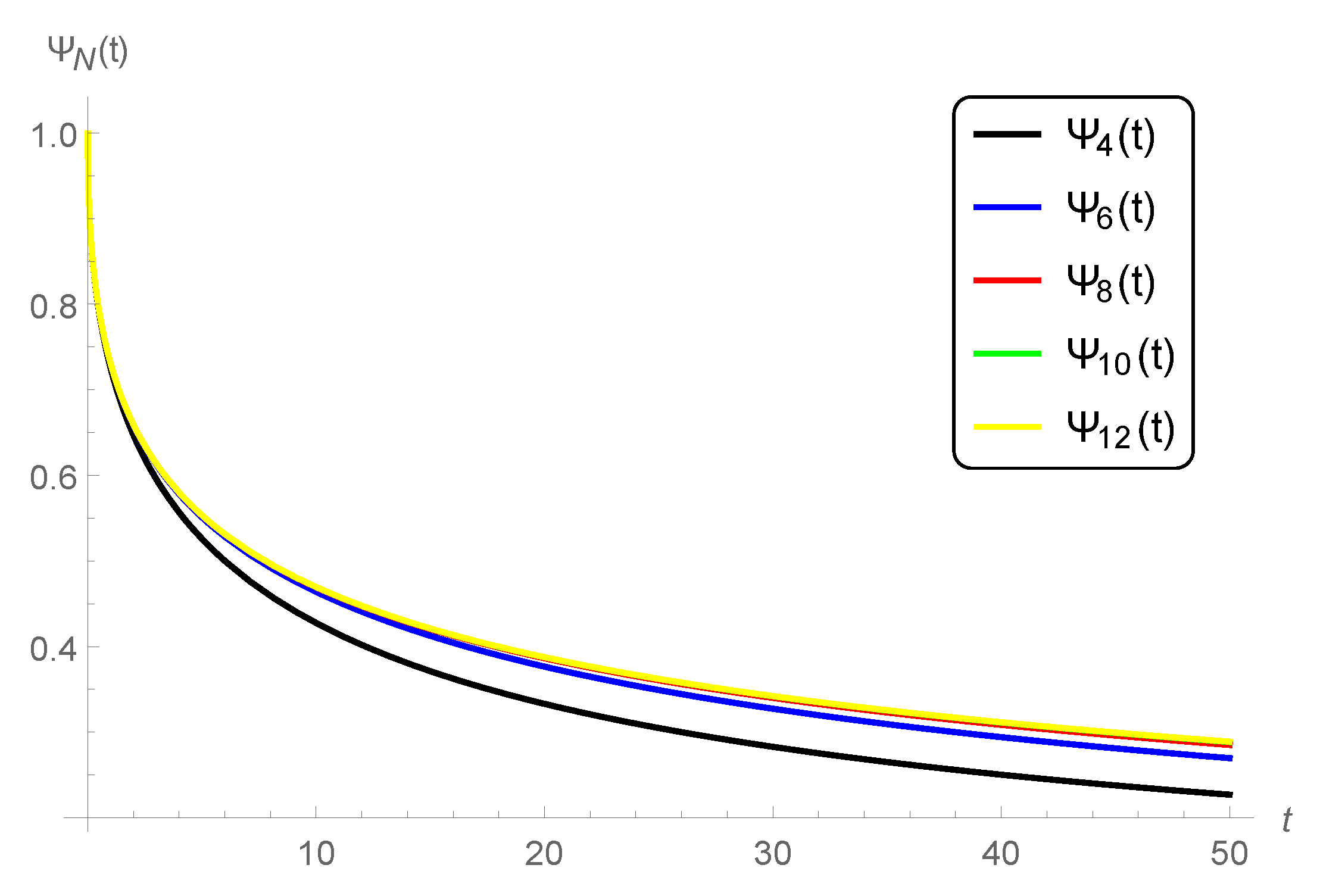
5. Results & Validation
5.1. As
5.2. Convergence
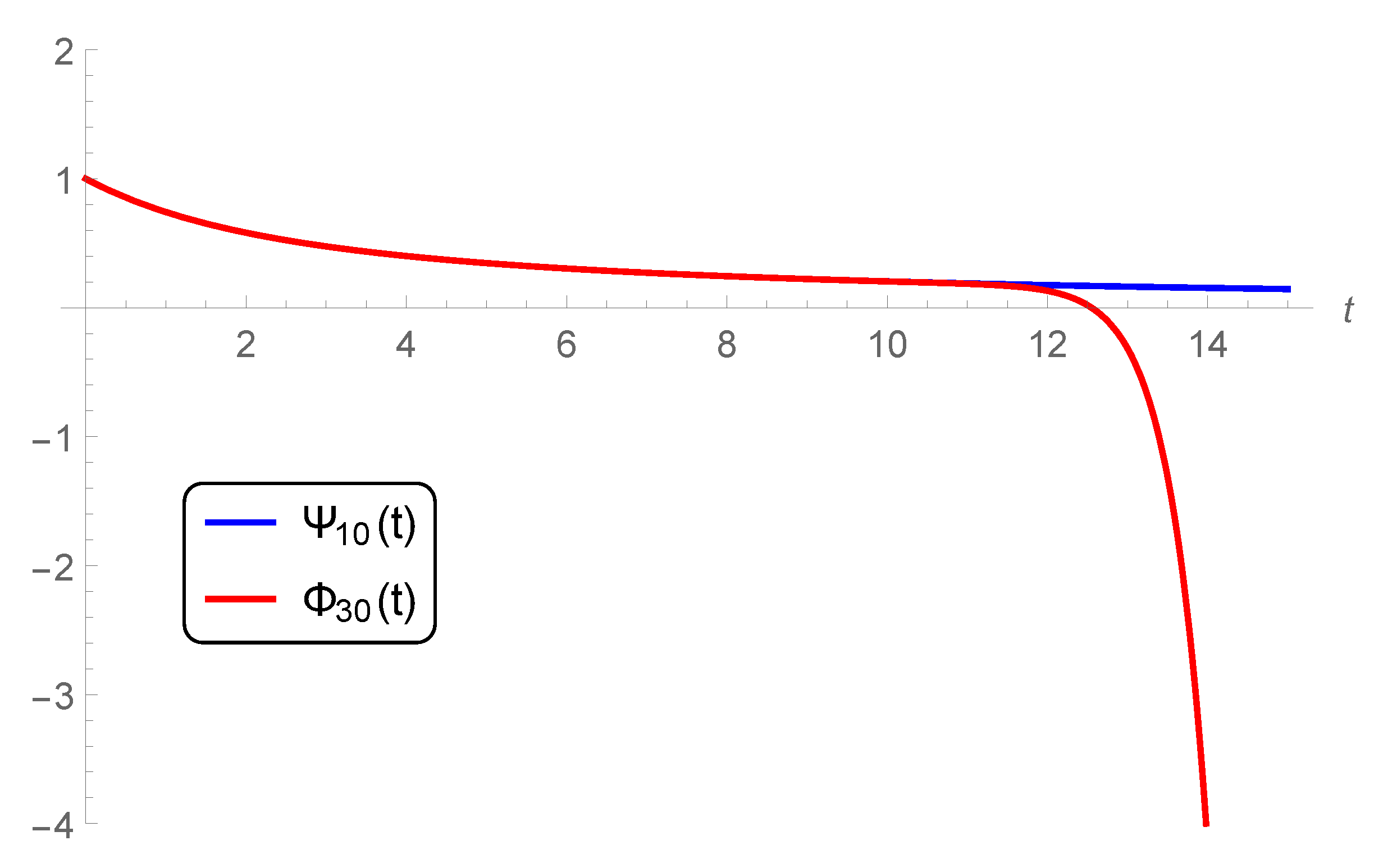
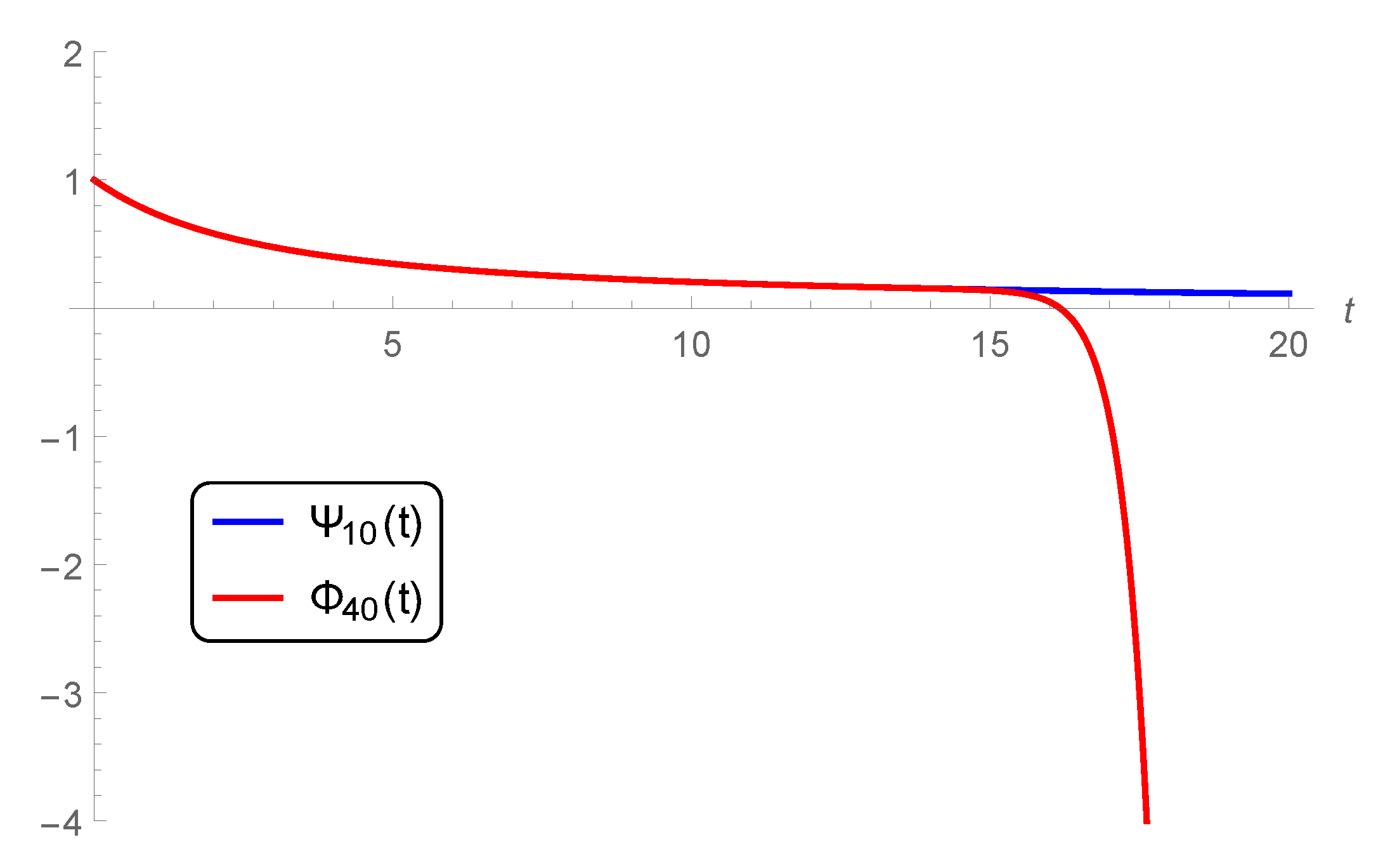
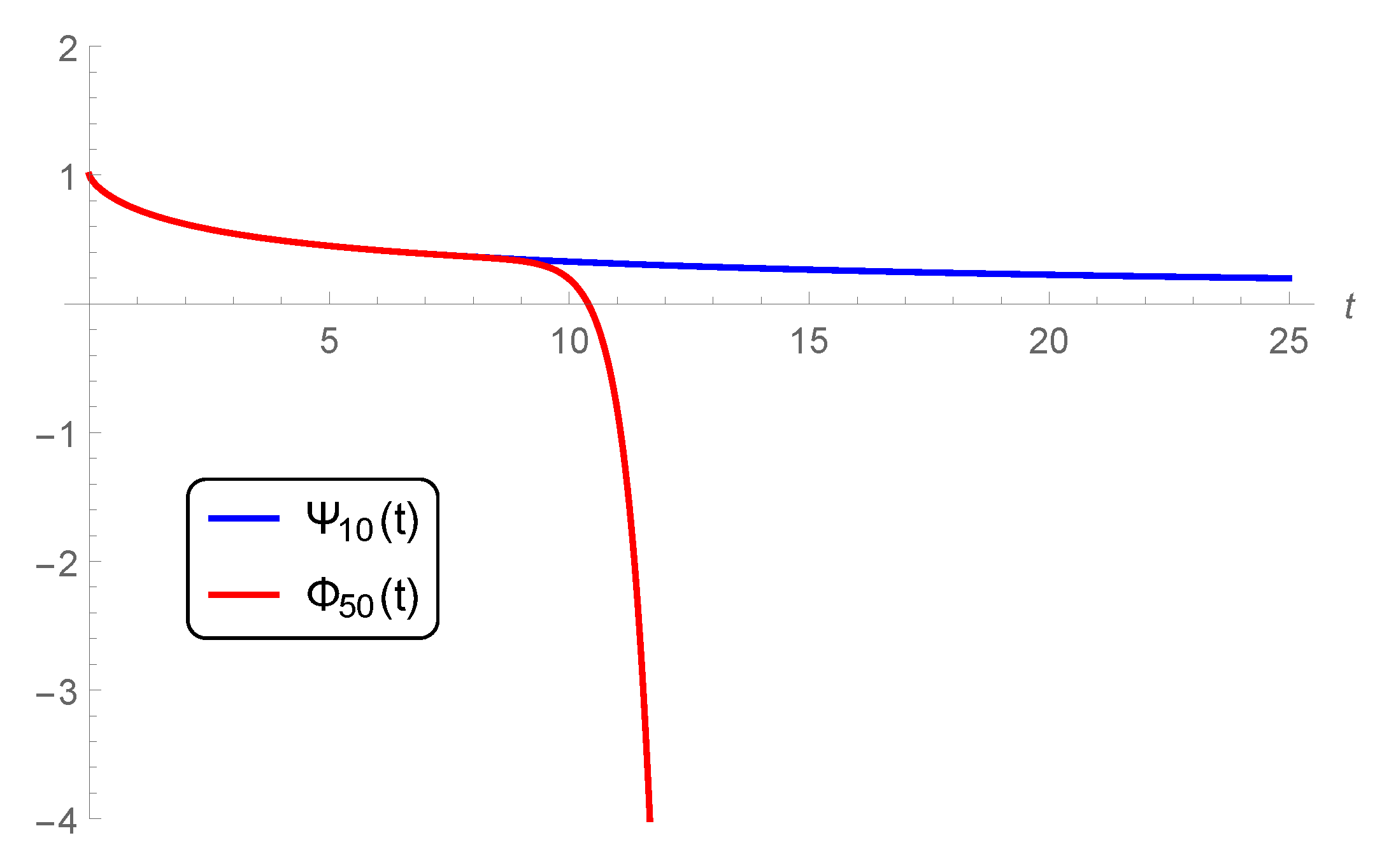
5.3. Comparisons
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ambartsumian, V.A. On the fluctuation of the brightness of the milky way. Dokl. Akad Nauk USSR 1994, 44, 223–226. [Google Scholar]
- Patade, J.; Bhalekar, S. On analytical solution of Ambartsumian equation. Natl. Acad. Sci. Lett. 2017, 40, 291–293. [Google Scholar] [CrossRef]
- Bakodah, H.O.; Ebaid, A. Exact solution of Ambartsumian delay differential equation and comparison with Daftardar-Gejji and Jafari approximate method. Mathematics 2018, 6, 331. [Google Scholar] [CrossRef] [Green Version]
- Ebaid, A.; Al-Enazi, A.; Albalawi, B.Z.; Aljoufi, M.D. Accurate approximate solution of Ambartsumian delay differential equation via decomposition method. Math. Comput. Appl. 2019, 24, 7. [Google Scholar] [CrossRef] [Green Version]
- Algehyne, E.A.; El-Zahar, E.R.; Alharbi, F.M.; Ebaid, A. Development of analytical solution for a generalized Ambartsumian equation. AIMS Math. 2019, 5, 249–258. [Google Scholar] [CrossRef]
- Alatawi, A.A.; Aljoufi, M.; Alharbi, F.M.; Ebaid, A. Investigation of the surface brightness model in the milky way via homotopy perturbation method. J. Appl. Math. Phys. 2020, 8, 434–442. [Google Scholar] [CrossRef] [Green Version]
- Khaled, S.M.; El-Zahar, E.R.; Ebaid, A. Solution of Ambartsumian delay differential equation with conformable derivative. Mathematics 2019, 7, 425. [Google Scholar] [CrossRef] [Green Version]
- Kumar, D.; Singh, J.; Baleanu, D.; Rathore, S. Analysis of a fractional model of the Ambartsumian equation. Eur. Phys. J. Plus 2018, 133, 133–259. [Google Scholar] [CrossRef]
- Patade, J. Series solution of system of fractional order Ambartsumian equations: Application in astronomy. arXiv 2020, arXiv:2008.04904v1. [Google Scholar]
- Patra, A.; Saha Ray, S. Homotopy perturbation Sumudu transform method for solving convective radial fins with temperature-dependent thermal conductivity of fractional order energy balance equation. Int. J. Heat Mass Trans. 2014, 76, 162–170. [Google Scholar] [CrossRef]
- Ayati, Z.; Biazar, J. On the convergence of Homotopy perturbation method. J. Egypt. Math. Soc. 2015, 23, 424–428. [Google Scholar] [CrossRef] [Green Version]
- Golmankhaneh, A.K.; Ali, K.G.; Baleanu, D. Homotopy perturbation method for solving a system of Schrodinger-Korteweg-De Vries equations. Rom. Rep. Phys. 2011, 63, 609–623. [Google Scholar]
- Touchent, K.A.; Hammouch, Z.; Mekkaoui, T.; Belgacem, F.B.M. Implementation and convergence analysis of homotopy perturbation coupled with Smudu transform to construct solutions of local-fractional PDEs. Fractal Fract. 2018, 2, 22. [Google Scholar] [CrossRef] [Green Version]
- Sene, N.; Fall, A.N. Homotopy perturbation ρ-Laplace transform method and its application to the fractional diffusion equation and the fractional diffusion-reaction equation. Fractal Fract. 2019, 3, 14. [Google Scholar] [CrossRef] [Green Version]
- Parvizi, M.; Eslahchi, M.R.; Dehghan, M. Numerical solution of fractional advection-diffusion equation with a nonlinear source term. Numer. Algorithms 2015, 68, 601–629. [Google Scholar] [CrossRef]
- Donatelli, M.; Mazza, M.; Serra-Capizzano, S. Spectral analysis and structure preserving preconditioners for fractional diffusion equation. J. Comput. Phys. 2016, 307, 262–279. [Google Scholar] [CrossRef]
- Lin, X.-L.; Ng, M.K.; Sun, H.-W. A multigrid method for linear systems arising from time-dependent two-dimensional space-fractional diffusion equations. J. Comput. Phys. 2017, 336, 69–86. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific Publishing Company: Singapore, 2000. [Google Scholar]
- Povstenko, Y. Fractional Diffusion-Wave Equation for Scientists and Engineers; Birkhäuser: New York, NY, USA, 2015. [Google Scholar]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions, Related Topics and Applications; Springer: Berlin, Germany, 2014. [Google Scholar]
- Bhalekar, S.; Patade, J. Series solution of the Pantograph equation and its properties. Fractal Fract. 2017, 1, 16. [Google Scholar] [CrossRef] [Green Version]
- Wojciech, S.; Voyiadjis, G.Z. A hyperelastic fractional damage material model with memory. Int. J. Solids Struc. 2017, 124, 151–160. [Google Scholar]

| 0.214582 | 0.252230 | 0.308001 | |
| 0.479368 | 0.530858 | 0.604679 | |
| 0.563455 | 0.607480 | 0.668446 | |
| 0.607715 | 0.644733 | 0.693132 | |
| 0.635007 | 0.666278 | 0.704111 | |
| 0.653379 | 0.679961 | 0.709288 | |
| 0.666460 | 0.689178 | 0.711801 | |
| 0.676140 | 0.695606 | 0.713040 | |
| 0.683504 | 0.700277 | 0.713658 | |
| 0.689225 | 0.703657 | 0.713968 | |
| (Present) | (Ref. [9]) | ||
|---|---|---|---|
| 0 | 1 | 1 | 0 |
| 1 | 0.77178479 | 0.77178478 | 1.0000 × 10−8 |
| 2 | 0.62270840 | 0.62269979 | 8.6100 × 10−6 |
| 3 | 0.51953974 | 0.51907862 | 4.6112 × 10−4 |
| 4 | 0.44464782 | 0.43701082 | 7.6370 × 10−3 |
| 5 | 0.38812397 | 0.32151337 | 6.6612 × 10−2 |
| 6 | 0.34409085 | −0.04360569 | 3.8770 × 10−1 |
| 7 | 0.30888831 | −1.39936299 | 0.1708 × 101 |
| 8 | 0.28013696 | −5.86284892 | 0.6143 × 101 |
| 9 | 0.25623112 | −18.66754017 | 0.1892 × 102 |
| 10 | 0.23605160 | −51.37867472 | 0.5162 × 102 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alharbi, W.; Petrovskii, S. Numerical Analysis for the Fractional Ambartsumian Equation via the Homotopy Herturbation Method. Mathematics 2020, 8, 2247. https://doi.org/10.3390/math8122247
Alharbi W, Petrovskii S. Numerical Analysis for the Fractional Ambartsumian Equation via the Homotopy Herturbation Method. Mathematics. 2020; 8(12):2247. https://doi.org/10.3390/math8122247
Chicago/Turabian StyleAlharbi, Weam, and Sergei Petrovskii. 2020. "Numerical Analysis for the Fractional Ambartsumian Equation via the Homotopy Herturbation Method" Mathematics 8, no. 12: 2247. https://doi.org/10.3390/math8122247
APA StyleAlharbi, W., & Petrovskii, S. (2020). Numerical Analysis for the Fractional Ambartsumian Equation via the Homotopy Herturbation Method. Mathematics, 8(12), 2247. https://doi.org/10.3390/math8122247





