Optimal Conditions for a Multimode Laser Diode with Delayed Optical Feedback in Terahertz Time-Domain Spectroscopy
Abstract
:1. Introduction
2. Methods
2.1. Rate Equations for a Multimode Laser Diode with Delayed Optical Feedback
2.2. Simulation Model for THz Time-Domain Spectroscopy
3. Results and Discussion
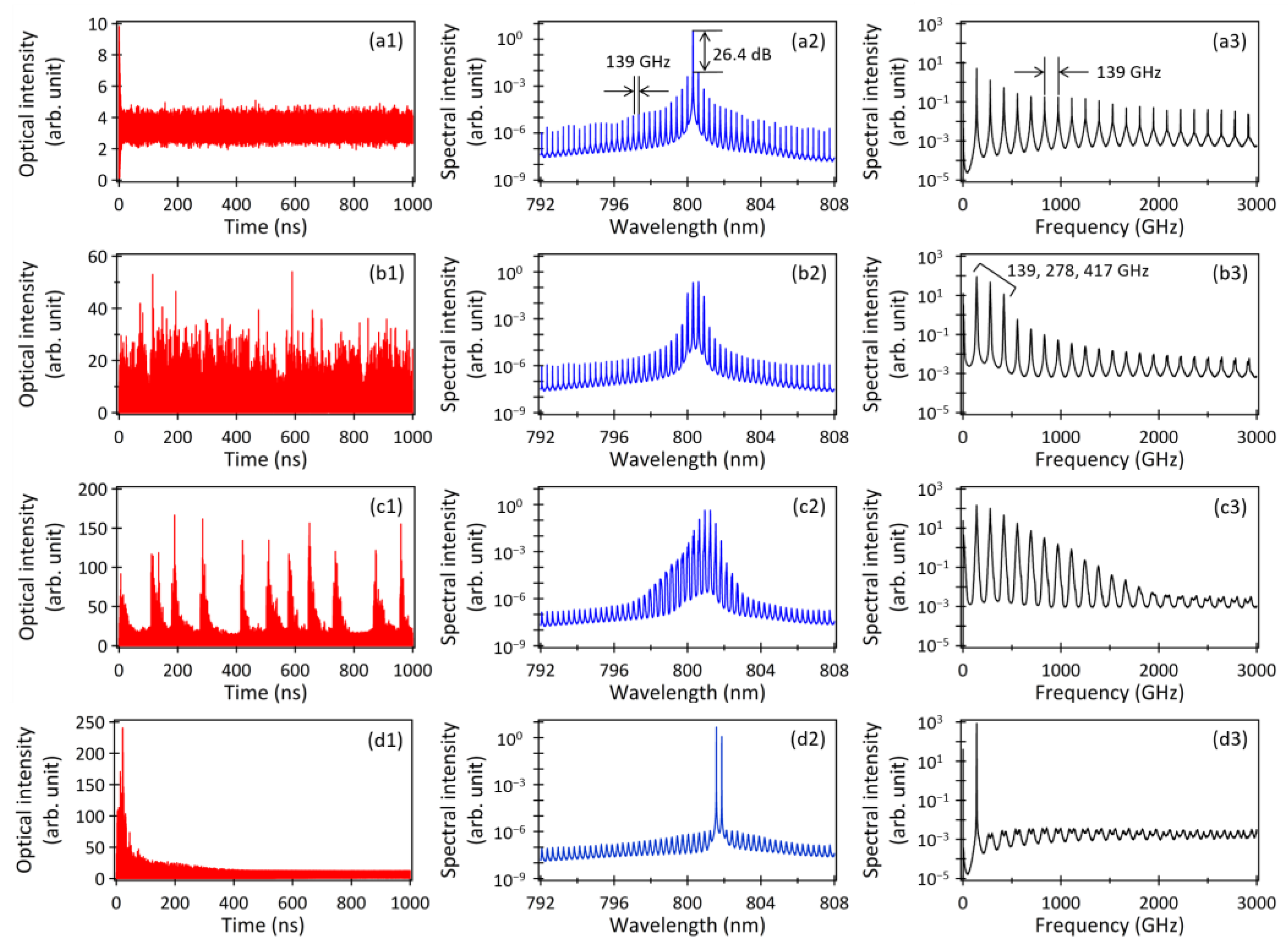
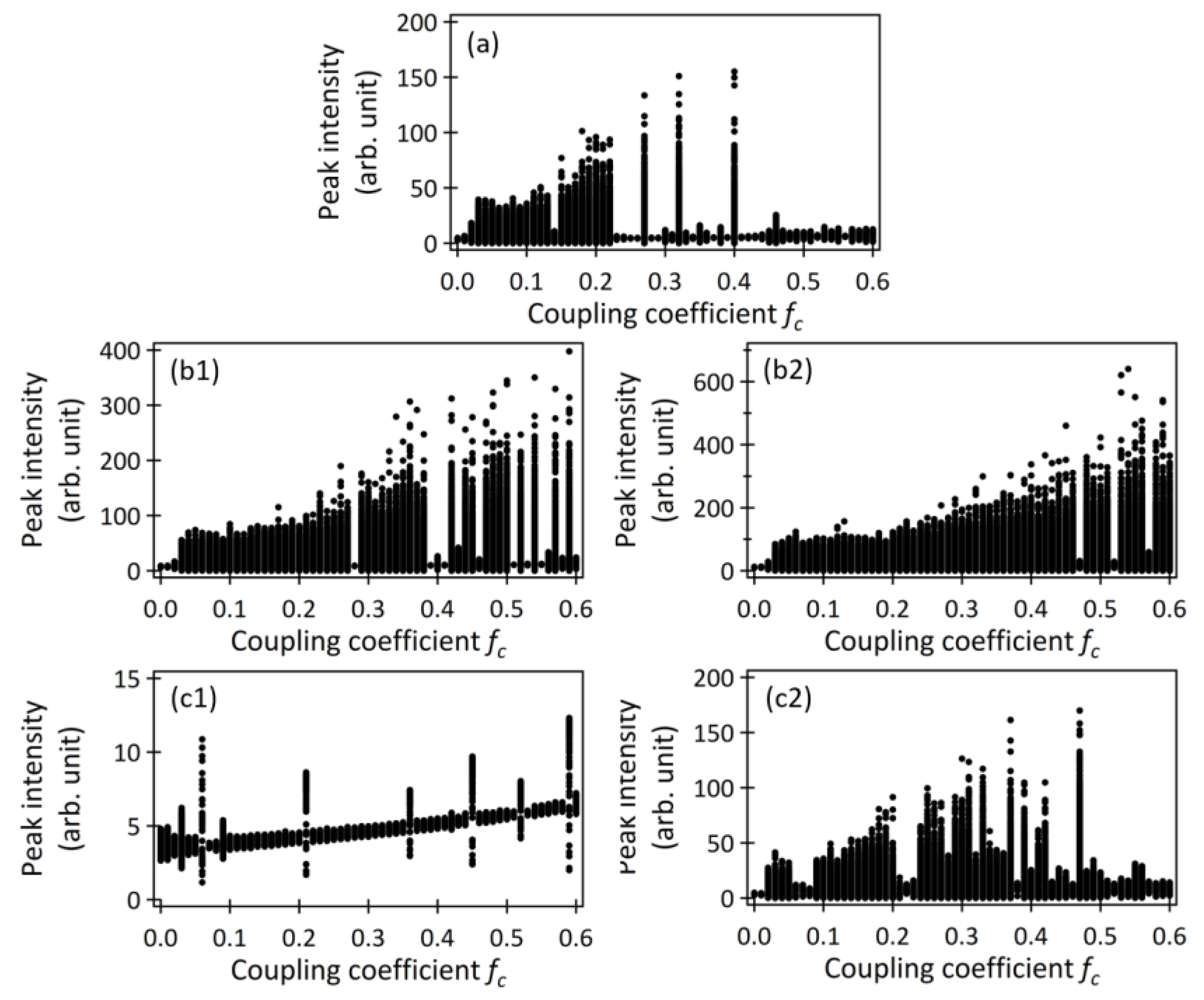
3.1. Classification of the LD Oscillation State by the Coupling Coefficient of the Optical Feedback
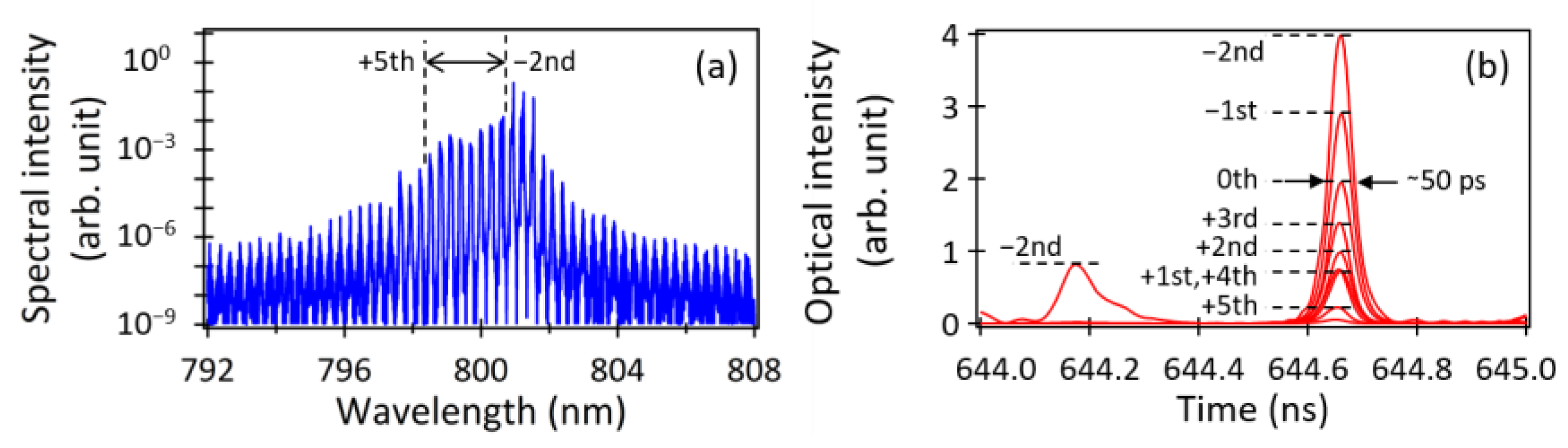
3.2. Characteristics of the Intermittent Chaotic Oscillations
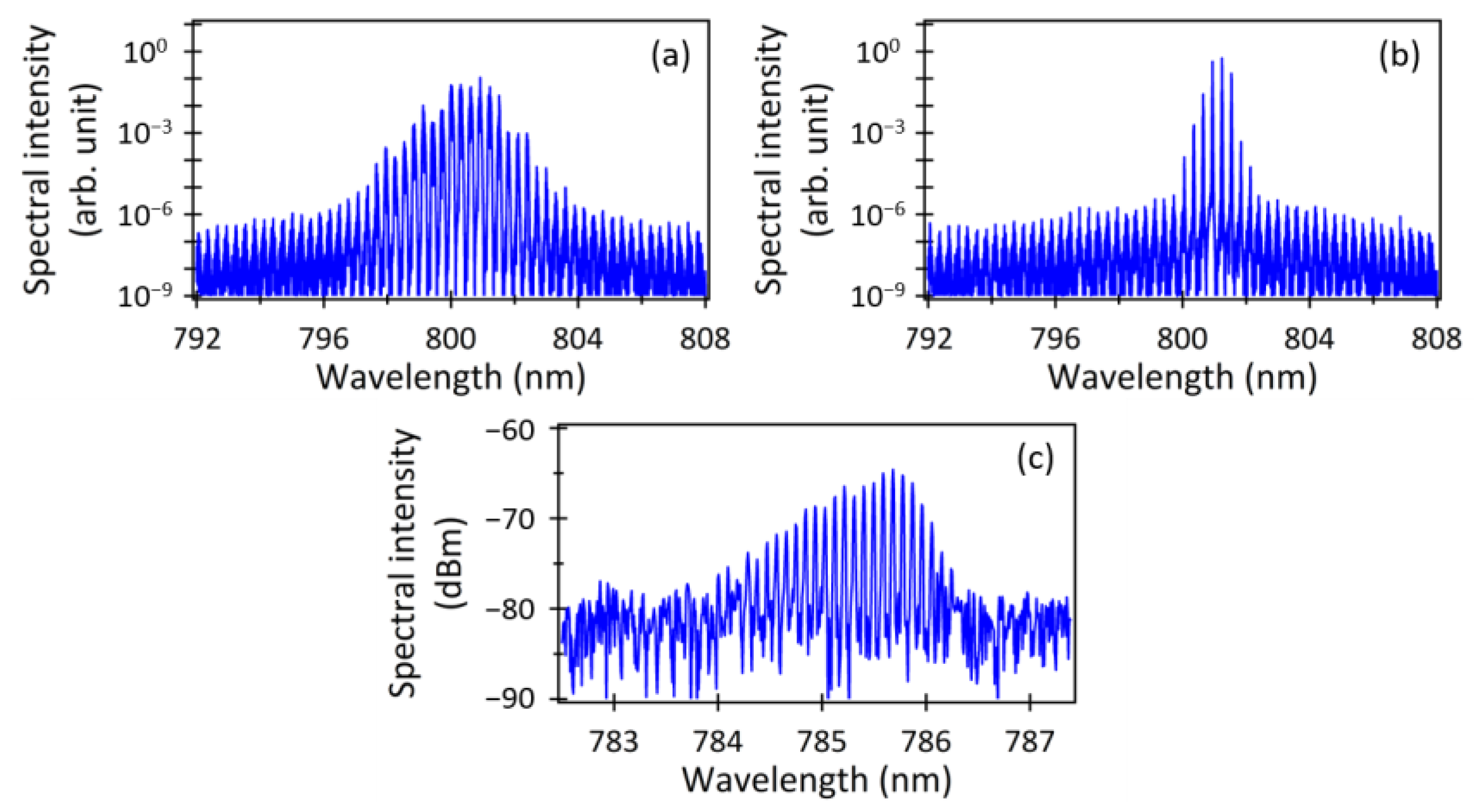
3.3. Time Convergence of the Output Spectral Shapes
3.4. Expansion of the Intermittent Chaotic Oscillation Region
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hangyo, M. Development and future prospects of terahertz technology. Jpn. J. Appl. Phys. 2015, 54, 120101. [Google Scholar] [CrossRef]
- Pavlidis, D. (Ed.) Fundamentals of Terahertz Devices and Applications; John Wiley & Sons Ltd.: Hoboken, NJ, USA, 2021. [Google Scholar]
- Sakai, K. (Ed.) Terahertz Optoelectronics; Springer: Berlin, Germany, 2005. [Google Scholar]
- Zhang, X.-C.; Xu, J. Introduction to THz Wave Photonics; Springer: Berlin, Germany, 2010. [Google Scholar]
- Auston, D.H.; Cheung, K.P.; Smith, P.R. Picosecond photoconducting Hertzian dipoles. Appl. Phys. Lett. 1984, 45, 284–286. [Google Scholar] [CrossRef]
- Pupeza, I.; Wilk, R.; Koch, M. Highly accurate optical material parameter determination with THz time-domain spectroscopy. Opt. Express 2007, 15, 4335–4350. [Google Scholar] [CrossRef]
- Withayachumnankul, W.; Fischer, B.M.; Lin, H.; Abbott, D. Uncertainty in terahertz time-domain spectroscopy measurement. J. Opt. Soc. Am. 2008, 25, 1059–1072. [Google Scholar] [CrossRef]
- Han, N.R.; Chen, Z.C.; Lim, C.S.; Ng, B.; Hong, M.H. Broadband multi-layer terahertz metamaterials fabrication and characterization on flexible substrates. Opt. Express 2011, 19, 6990–6998. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Shutler, A.; Grischkowsky, D. Measurement of the transmission of the atmosphere from 0.2 to 2 THz. Opt. Express 2011, 19, 8830–8838. [Google Scholar] [CrossRef]
- Shrekenhamer, D.; Rout, S.; Strikwerda, A.C.; Bingham, C.; Averitt, R.D.; Sonkusale, S.; Padilla, W.J. High speed terahertz modulation from metamaterials with embedded high electron mobility transistors. Opt. Express 2011, 19, 9968–9975. [Google Scholar] [CrossRef]
- Anthony, J.; Leonhardt, R.; Leon-Saval, S.G.; Argyros, A. THz propagation in kagome hollow-core microstructured fibers. Opt. Express 2011, 19, 18470–18478. [Google Scholar] [CrossRef]
- Niu, T.; Withayachumnankul, W.; Ung, B.S.-Y.; Menekse, H.; Bhaskaran, M.; Sriram, S.; Fumeaux, C. Experimental demonstration of reflectarray antennas at terahertz frequencies. Opt. Express 2013, 21, 2875–2889. [Google Scholar] [CrossRef]
- Islam, M.S.; Cordeiro, C.M.B.; Franco, M.A.R.; Sultana, J.; Cruz, A.L.S.; Abbott, D. Terahertz optical fibers. Opt. Express 2020, 28, 16089–16117. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, G.; Xu, D.; Jiang, B.; Ge, M.; Wu, L.; Yang, C.; Mu, N.; Wang, S.; Chang, C.; et al. Terahertz spectroscopic diagnosis of early blast-induced traumatic brain injury in rats. Biomed. Opt. Express 2020, 11, 4085–4098. [Google Scholar] [CrossRef] [PubMed]
- Tani, M.; Matsuura, S.; Sakai, K.; Hangyo, M. Multiple-frequency generation of sub-terahertz radiation by multimode LD excitation of photoconductive antenna. IEEE Microw. Guid. Wave Lett. 1997, 7, 282–284. [Google Scholar] [CrossRef]
- Morikawa, O.; Tonouchi, M.; Tani, M.; Sakai, K.; Hangyo, M. Sub-THz emission properties of photoconductive antennas excited with multimode laser diode. Jpn. J. Appl. Phy. 1999, 38, 1388–1389. [Google Scholar] [CrossRef]
- Morikawa, O.; Tonouchi, K.; Hangyo, M. Sub-THz spectroscopic system using a multimode laser diode and photoconductive antenna. Appl. Phy. Lett. 1999, 75, 3772–3774. [Google Scholar] [CrossRef]
- Scheller, M.; Koch, M. Terahertz quasi time domain spectroscopy. Opt. Express 2009, 17, 17723–17733. [Google Scholar] [CrossRef]
- Morikawa, O.; Fujita, M.; Takano, K.; Hangyo, M. Sub-terahertz spectroscopic system using a continuous-wave broad-area laser diode and a spatial filter. J. Appl. Phys. 2011, 110, 063107. [Google Scholar] [CrossRef]
- Molter, D.; Kolano, M.; Freymann, G. SLD-driven terahertz cross-correlation spectroscopy. In Proceedings of the 2019 44th Int. Conf. Infrared, Millimeter and Terahertz Waves, Paris, France, 1–6 September 2019; pp. 1–2. [Google Scholar]
- Molter, D.; Klier, J.; Weber., S.; Kolano., M.; Jonuscheit., J.; Freymann, G. Two decades of terahertz cross-correlation spectroscopy. Appl. Phys. Rev. 2021, 8, 021311. Appl. Phys. Rev. 2021, 8, 021311. [Google Scholar] [CrossRef]
- Cherniak, V.; Kubiczek, T.; Kolpatzeck, K.; Balzer, J.C. Laser diode based THz-TDS system with 133 dB peak signal-to-noise ratio at 100 GHz. Sci. Rep. 2023, 13, 13476. [Google Scholar] [CrossRef]
- Kolpatzeck, K.; Liu, X.; Häring, L.; Balzer, J.C.; Czylwik, A. Ultra-High Repetition Rate Terahertz Time-Domain Spectroscopy for Micrometer Layer Thickness Measurement. Sensors 2021, 21, 5389. [Google Scholar] [CrossRef]
- Kuwashima, F.; Taniguchi, S.; Nonaka, K.; Hangyo, M.; Iwasawa, H. Stabilization of THz wave generation by using chaotic oscillation in a laser. In Proceedings of the Int. Conf. Infrared, Millimeter and Terahertz Waves, paper Tu-P.63, Rome, Italy, 5–10 September 2010. [Google Scholar]
- Kuwashima, F.; Shirao, T.; Kishibata, T.; Okuyama, T.; Akamine, Y.; Tani, M.; Kurihara, K.; Yamamoto, K.; Nagashima, T.; Hangyo, M. High efficient THz time domain spectroscopy systems using laser chaos and a metal V grooved waveguide. In Proceedings of the Int. Conf. Infrared, Millimeter and Terahertz Waves, paper W5-P24.2, Tucson, AZ, USA, 14–18 September 2014. [Google Scholar]
- Kuwashima, F.; Jarrahi, M.; Cakmakyapan, S.; Morikawa, O.; Shirao, T.; Iwao, K.; Kurihara, K.; Kitahara, H.; Furuya, T.; Wada, K.; et al. Evaluation of high-stability optical beats in laser chaos by plasmonic photomixing. Opt. Express 2020, 28, 24833–24844. [Google Scholar] [CrossRef]
- Wada, K.; Yoshioka, H.; Jiaxun, Z.; Matsuyama, T.; Horinaka, H. Simple form of multimode laser diode rate equations incorporating the band filling effect. Opt. Express 2011, 19, 3019–3036. [Google Scholar] [CrossRef]
- Wada, K.; Matsuyama, T.; Horinaka, H. Simple gain form of 1.5μm multimode laser diode incorporating band filling and intrinsic gain saturation effects. Jpn. J. Appl. Phy. 2015, 54, 032101. [Google Scholar] [CrossRef]
- Wada, K.; Kitagawa, N.; Matsukura, S.; Matsuyama, T.; Horinaka, H. Timing and amplitude jitter in a gain-switched multimode semiconductor laser. Jpn. J. Appl. Phy. 2016, 55, 042702. [Google Scholar] [CrossRef]
- Wada, K.; Kitagawa, N.; Matsuyama, T. The degree of temporal synchronization of the pulse oscillations from a gain-switched multimode semiconductor laser. Materials 2017, 10, 950. [Google Scholar] [CrossRef] [PubMed]
- Lang, R.; Kobayashi, K. External optical feedback effects on semiconductor injection laser properties. IEEE J. Quantum. Electron. 1980, 16, 347–355. [Google Scholar]
- Siegman, A.E. Lasers. University Science Books: Mill Valley, CA, USA, 1986; pp. 1004–1022. [Google Scholar]
- Ohtsubo, J. Chap. 5, Semiconductor Lasers–Stability, Instability and Chaos, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 133–136. [Google Scholar]
- Cheng, T.; Yang, T.-Y.; Gong, T.; Guo, G.-Q.; Qiu, X.-B.; Li, C.-L.; Zhao, G.; Ma, W.-G. Interference suppression method in optical feedback-cavity enhanced absorption spectroscopy technology. Acta Physica Sinica 2022, 71, 064205. [Google Scholar] [CrossRef]
- Li, P.; Cai, Q.; Zhang, J.; Xu, B.; Liu, Y.; Bogris, A.; Shore, K.A.; Wang, Y. Observation of flat chaos generation using an optical feedback multi-mode laser with a band-pass filter. Opt. Express 2019, 27, 17859–17867. [Google Scholar] [CrossRef]
- Yamada, M. Chap. 7, Theory of Semiconductor Lasers–From Basis of Quantum Electronics to Analyses of the Mode Competition Phenomena and Noise–, Springer Series in Optical Sciences 185; Springer: Tokyo, Japan, 2014; pp. 110–112. [Google Scholar]





| Notation | Parameter | Value | Unit |
|---|---|---|---|
| m | Mode number | −30~+30 | |
| τp | Photon lifetime | 2.0 | ps |
| Trt | Round-trip time of the LD cavity | 7.1 | ps |
| τfb | Delay time of the optical feedback fields | 1 | ns |
| C1 | Nonradiative recombination rate | 2.0 × 108 | s−1 |
| C2 | Radiative recombination coefficient | 2.0 × 10−16 | m3 s−1 |
| C3 | Auger recombination coefficient | 0 | m6 s−1 |
| fc | Coupling coefficient of the optical feedback fields | 0~0.6 | |
| α | Linewidth enhancement factor | 3.0 | |
| β | Spontaneous emission factor | 1.0 × 10−6 | |
| εS | Gain compression factor | 0.05 × 10−23 | m3 |
| δf | Longitudinal mode spacing | 0.139 | THz |
| ∆τc | Coherence time of amplified spontaneous emission | 7.1 | ps |
| e | Elementary electric charge | 1.60 × 10−19 | C |
| L | Laser cavity length | 300 | μm |
| V | Laser cavity volume | 180 | μm3 |
| nr | Refractive index of the active layer | 3.6 | |
| ε0 | Dielectric constant for the vacuum | 8.85 × 10−12 | F/m |
| H | Planck’s constant | 6.63 × 10−34 | Js |
| fm | Central oscillation frequency of the mth mode | 375 + 0.139 m | THz |
| I | Injection current | r × Ith0 | mA |
| r | Pumping rate | 1.5~2.5 | |
| Ith0 | Threshold current for the central mode | 24 | mA |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wada, K.; Kitagawa, T.; Matsuyama, T.; Okamoto, K.; Kuwashima, F. Optimal Conditions for a Multimode Laser Diode with Delayed Optical Feedback in Terahertz Time-Domain Spectroscopy. Spectrosc. J. 2023, 1, 137-151. https://doi.org/10.3390/spectroscj1030012
Wada K, Kitagawa T, Matsuyama T, Okamoto K, Kuwashima F. Optimal Conditions for a Multimode Laser Diode with Delayed Optical Feedback in Terahertz Time-Domain Spectroscopy. Spectroscopy Journal. 2023; 1(3):137-151. https://doi.org/10.3390/spectroscj1030012
Chicago/Turabian StyleWada, Kenji, Tokihiro Kitagawa, Tetsuya Matsuyama, Koichi Okamoto, and Fumiyoshi Kuwashima. 2023. "Optimal Conditions for a Multimode Laser Diode with Delayed Optical Feedback in Terahertz Time-Domain Spectroscopy" Spectroscopy Journal 1, no. 3: 137-151. https://doi.org/10.3390/spectroscj1030012
APA StyleWada, K., Kitagawa, T., Matsuyama, T., Okamoto, K., & Kuwashima, F. (2023). Optimal Conditions for a Multimode Laser Diode with Delayed Optical Feedback in Terahertz Time-Domain Spectroscopy. Spectroscopy Journal, 1(3), 137-151. https://doi.org/10.3390/spectroscj1030012






