Dielectric Stability of Triton X-100-Based Tissue-Mimicking Materials for Microwave Imaging
Abstract
1. Introduction
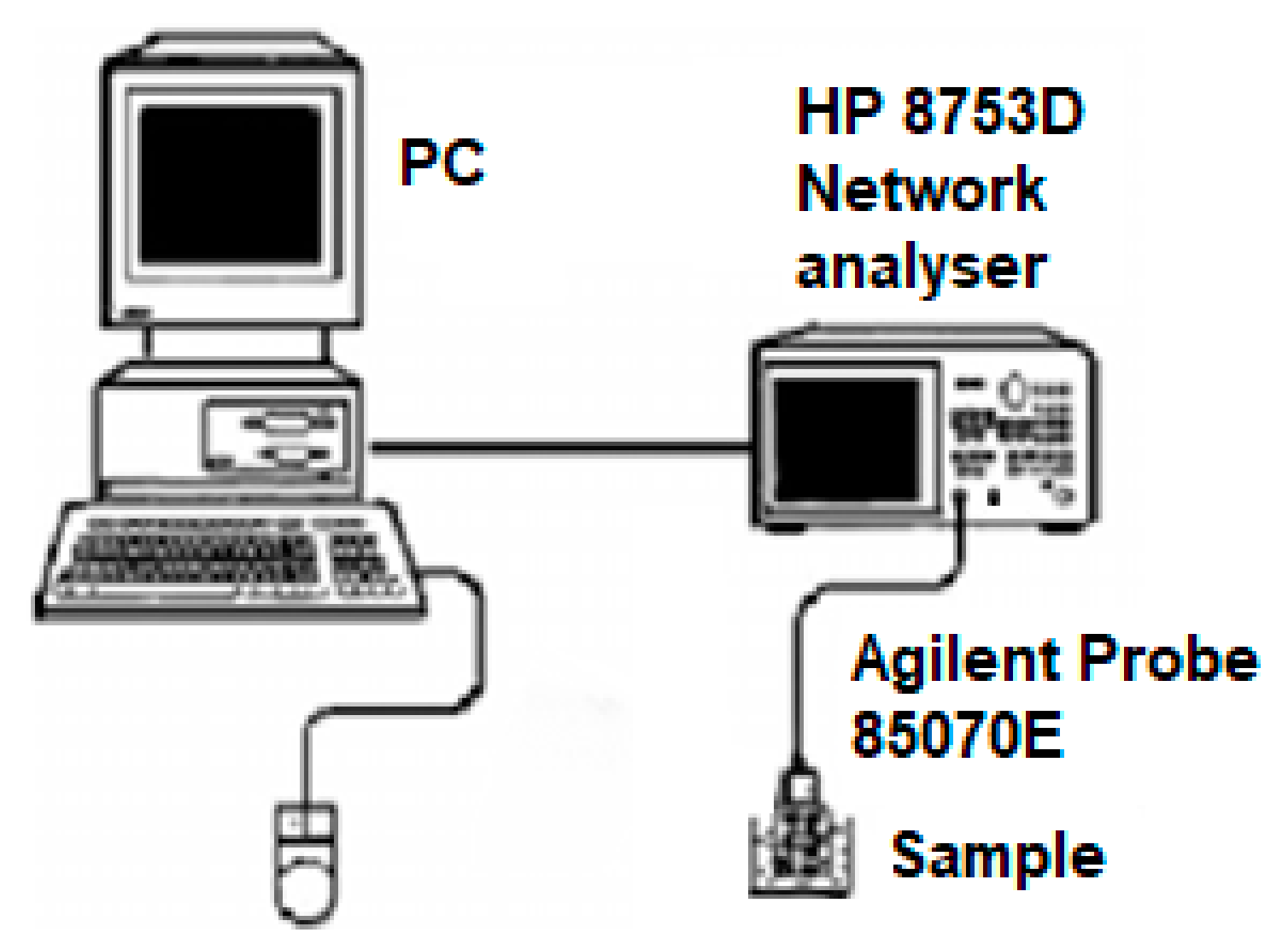
2. Materials and Methods
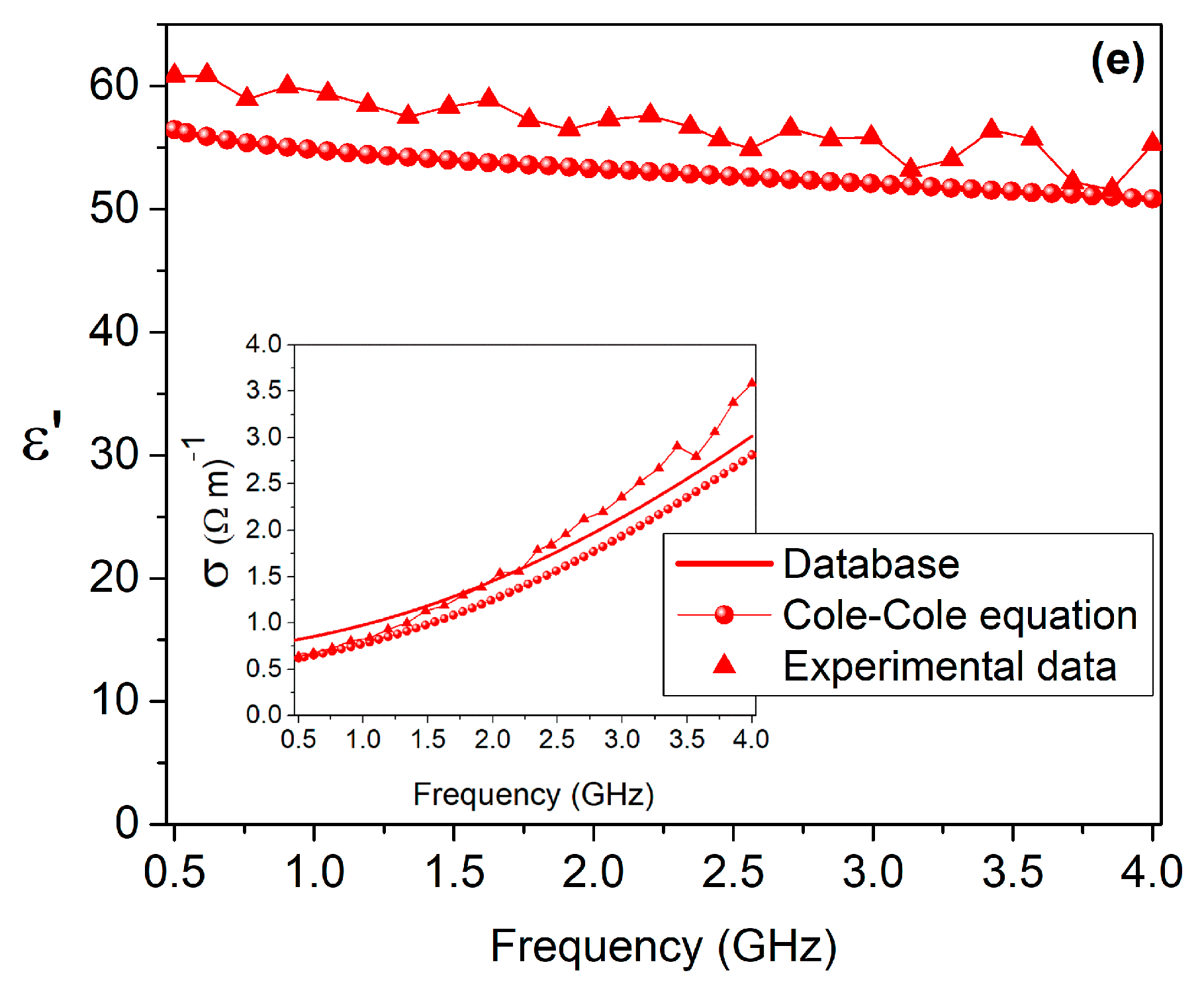
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Amin, B.; Kelly, D.; Shahzad, A.; O’Halloran, M.; Elahi, M.A. Anthropomorphic calcaneus phantom for microwave bone imaging applications. IEEE J. Electromagn. RF Microw. Med. Biol. 2021, 5, 206–213. [Google Scholar] [CrossRef]
- Abedi, S.; Joachimowicz, N.; Meyer, O.; Picard, D.; Roussel, H. Phantoms for a novel generation of medical microwave imaging devices. In Proceedings of the 13th European Conference on Antennas and Propagation, Krakow, Poland, 31 March–5 April 2019; pp. 1–4. [Google Scholar]
- Amin, B.; Elahi, M.A.; Shahzad, A.; Parle, E.; McNamara, L.; O’Halloran, M. An insight into bone dielectric properties variation: A foundation for electromagnetic medical devices. In Proceedings of the 1st World Conference on Biomedical Applications of Electromagnetic Fields, Split, Croatia, 10–13 September 2018; pp. 1–2. [Google Scholar] [CrossRef]
- Joachimowicz, N.; Duchene, B.; Conessa, C.; Meyer, O. Reference phantoms for microwave imaging. In Proceedings of the 11th European Conference on Antennas and Propagation, Paris, France, 19–24 March 2017; pp. 2719–2722. [Google Scholar] [CrossRef]
- Sultan, K.S.; Mohammed, B.; Mills, P.C.; Abbosh, A. Anthropomorphic durable realistic knee phantom for testing electromagnetic imaging systems. IEEE J. Electromagn. RF Microw. Med. Biol. 2020, 5, 132–138. [Google Scholar] [CrossRef]
- Kerketta, S.R.; Ghosh, D. Microwave sensing for human bone health evaluation. AEU-Int. J. Electron. Commun. 2020, 127, 153469. [Google Scholar] [CrossRef]
- Savazzi, M.; Abedi, S.; Ištuk, N.; Joachimowicz, N.; Roussel, H.; Porter, E.; O’Halloran, M.; Costa, J.R.; Fernandes, C.A.; Felício, J.M.; et al. Development of an anthropomorphic phantom of the axillary region for microwave imaging assessment. Sensors 2020, 20, 4968. [Google Scholar] [CrossRef]
- Ramalingam, V.S.; Kanagasabai, M.; Sundarsingh, E.F. A Compact Microwave Device for Fracture Diagnosis of the Human Tibia. IEEE Trans. Compon. Packag. Manuf. Technol. 2019, 9, 661–668. [Google Scholar] [CrossRef]
- Lazebnik, M.; Madsen, E.L.; Frank, G.R.; Hagness, S.C. Tissue-mimicking phantom materials for narrowband and ultrawideband microwave applications. Phys. Med. Biol. 2005, 50, 4245. [Google Scholar] [CrossRef]
- Guy, A.W. Analyses of electromagnetic fields induced in biological tissues by thermographic studies on equivalent phantom models. IEEE Trans. Microw. Theory Tech. 1971, 19, 205–214. [Google Scholar] [CrossRef]
- Cheung, A.Y.; Koopman, D.W. Experimental development of simulated biomaterials for dosimetry studies of hazardous microwave radiation. IEEE Trans. Microw. Theory Tech. 1976, 24, 669–673. [Google Scholar] [CrossRef]
- Chou, C.K.; Chen, G.W.; Guy, A.W.; Luk, K.H. Formulas for preparing phantom muscle tissue at various radiofrequencies. Bioelectromagnetics 1984, 5, 435–441. [Google Scholar] [CrossRef]
- Bini, M.G.; Ignesti, A.; Millanta, L.; Olmi, R.; Rubino, N.; Vanni, R. The polyacrylamide as a phantom material for electromagnetic hyperthermia studies. IEEE Trans. Biomed. Eng. 1984, 3, 317–322. [Google Scholar] [CrossRef]
- Andreuccetti, D.; Bini, M.; Ignesti, A.; Olmi, R.; Rubino, N.; Vanni, R. Use of polyacrylamide as a tissue-equivalent material in the microwave range. IEEE Trans. Biomed. Eng. 1988, 35, 275–277. [Google Scholar] [CrossRef] [PubMed]
- Lagendijk, J.J.W.; Nilsson, P. Hyperthermia dough: A fat and bone equivalent phantom to test microwave/radiofrequency hyperthermia heating systems. Phys. Med. Biol. 1985, 30, 709. [Google Scholar] [CrossRef]
- Marchal, C.; Nadi, M.; Tosser, A.J.; Roussey, C.; Gaulard, M.L. Dielectric properties of gelatine phantoms used for simulations of biological tissues between 10 and 50 MHz. Int. J. Hyperth. 1989, 5, 725–732. [Google Scholar] [CrossRef]
- Robinson, M.P.; Richardson, M.J.; Green, J.L.; Preece, A.W. New materials for dielectric simulation of tissues. Phys. Med. Biol. 1991, 36, 1565. [Google Scholar] [CrossRef]
- Surowiec, A.; Shrivastava, P.N.; Astrahan, M.; Petrovich, Z. Utilization of a multilayer polyacrylamide phantom for evaluation of hyperthermia applicators. Int. J. Hyperth. 1992, 8, 795–807. [Google Scholar] [CrossRef]
- Nikawa, Y.; Chino, M.; Kikuchi, K. Soft and dry phantom modeling material using silicone rubber with carbon fiber. IEEE Trans. Microw. Theory Tech. 1996, 44, 1949–1953. [Google Scholar] [CrossRef]
- Chang, J.T.; Fanning, M.W.; Meaney, P.M.; Paulsen, K.D. A conductive plastic for simulating biological tissue at microwave frequencies. IEEE Trans. Electromagn. Compat. 2000, 42, 76–81. [Google Scholar] [CrossRef]
- Youngs, I.J.; Treen, A.S.; Fixter, G.; Holden, S. Design of solid broadband human tissue simulant materials. IEEE Sci. Meas. Technol. 2002, 149, 323–328. [Google Scholar] [CrossRef]
- Sunaga, T.; Ikehira, H.; Furukawa, S.; Tamura, M.; Yoshitome, E.; Obata, T.; Shinkai, H.; Tanada, S.; Murata, H.; Sasaki, Y. Development of a dielectric equivalent gel for better impedance matching for human skin. Bioelectromagnetics 2003, 24, 214–217. [Google Scholar] [CrossRef]
- Pinto, A.M.; Bertemes-Filho, P.; Paterno, A.S. Caracterização de Gelatina como Fantoma para Medições de Espectroscopia de Impedância elétrica. In Proceedings of the XXIV Congresso Brasileiro de Engenharia Biomédica, Uberlândia, Brazil, 13–17 October 2014; pp. 1317–1320. [Google Scholar] [CrossRef]
- Amin, B.; Kelly, D.; Shahzad, A.; O’Halloran, M.; Elahi, M.A. Microwave calcaneus phantom for bone imaging applications. In Proceedings of the 14th European Conference on Antennas and Propagation (EuCAP), Copenhagen, Denmark, 15–20 March 2020; pp. 1–5. [Google Scholar] [CrossRef]
- Devesa, S.; Graça, M.P.; Henry, F.; Costa, L.C. Microwave dielectric properties of (Bi1−xFex)NbO4 ceramics prepared by the sol-gel method. Ceram. Int. 2015, 41, 8186–8190. [Google Scholar] [CrossRef]
- Fornes-Leal, A.; Cardona, N.; Frasson, M.; Castelló-Palacios, S.; Nevárez, A.; Beltrán, V.P.; Garcia-Pardo, C. Dielectric characterization of in vivo abdominal and thoracic tissues in the 0.5–26.5 GHz frequency band for wireless body area networks. IEEE Access 2019, 7, 31854–31864. [Google Scholar] [CrossRef]
- Sun, J.; Wang, W.; Yue, Q. Review on microwave-matter interaction fundamentals and efficient microwave-associated heating strategies. Materials 2016, 9, 231. [Google Scholar] [CrossRef]
- La Gioia, A.; Porter, E.; Merunka, I.; Shahzad, A.; Salahuddin, S.; Jones, M.; O’Halloran, M. Open-ended coaxial probe technique for dielectric measurement of biological tissues: Challenges and common practices. Diagnostics 2018, 8, 40. [Google Scholar] [CrossRef]
- Gabriel, S.; Lau, R.W.; Gabriel, C. The dielectric properties of biological tissues: II. Measurements in the frequency range 10 Hz to 20 GHz. Phys. Med. Biol. 1996, 41, 2251. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and absorption in dielectrics I. Alternating current characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef]
- Meaney, P.M.; Goodwin, D.; Golnabi, A.H.; Zhou, T.; Pallone, M.; Geimer, S.D.; Burke, G.; Paulsen, K.D. Clinical microwave tomographic imaging of the calcaneus: A first-in-human case study of two subjects. IEEE Trans. Biomed. Eng. 2012, 59, 3304–3313. [Google Scholar] [CrossRef]
- Bjelogrlic, M.; Fuchs, B.; Thiran, J.P.; Mosig, J.R.; Mattes, M. Experimental verification of optimal frequency range for microwave head imaging. In Proceedings of the 19th International Conference on Electromagnetics in Advanced Applications (ICEAA), Verona, Italy, 11–15 September 2017; pp. 1008–1011. [Google Scholar] [CrossRef]
- Keysight Technologies. Keysight 85070E Dielectric Probe Kit 200 MHz to 50 GHz; Keysight Technologies: Santa Rosa, CA, USA, 2017. [Google Scholar]
- Piladaeng, N.; Angkawisittpan, N.; Homwuttiwong, S. Determination of relationship between dielectric properties, compressive strength, and age of concrete with rice husk ash using planar coaxial probe. Meas. Sci. Rev. 2016, 16, 14–20. [Google Scholar] [CrossRef]
- Šarolić, A.; Matković, A. Dielectric permittivity measurement using open-ended coaxial probe—Modeling and simulation based on the simple capacitive-load model. Sensors 2022, 22, 6024. [Google Scholar] [CrossRef]
- Dev, S.R.S.; Raghavan, G.S.V.; Gariepy, Y. Dielectric properties of egg components and microwave heating for in-shell pasteurization of eggs. J. Food Eng. 2008, 86, 207–214. [Google Scholar] [CrossRef]
- Feng, W.; Lin, C.P.; Deschamps, R.J.; Drnevich, V.P. Theoretical model of a multisection time domain reflectometry measurement system. Water Resour. Res. 1999, 35, 2321–2331. [Google Scholar] [CrossRef]
- Hasgall, P.A.; Di Gennaro, F.; Baumgartner, C.; Neufeld, E.; Lloyd, B.; Gosselin, M.C.; Payne, D.; Kuster, N. IT’IS Database for Thermal and Electromagnetic Parameters of Biological Tissues, Version 4.0.; IT’IS Foundation: Zürich, Switzerland, 2018. [Google Scholar]
- Gabriel, C. Compilation of the Dielectric Properties of Body Tissues at RF and Microwave Frequencies; Report N.AL/OE-TR-1996-0037; Occupational and Environmental Health Directorate, Radiofrequency Radiation Division, Brooks Air Force Base: San Antonio, TX, USA, 1996. [Google Scholar]
- Joachimowicz, N.; Conessa, C.; Henriksson, T.; Duchêne, B. Breast phantoms for microwave imaging. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 1333–1336. [Google Scholar] [CrossRef]


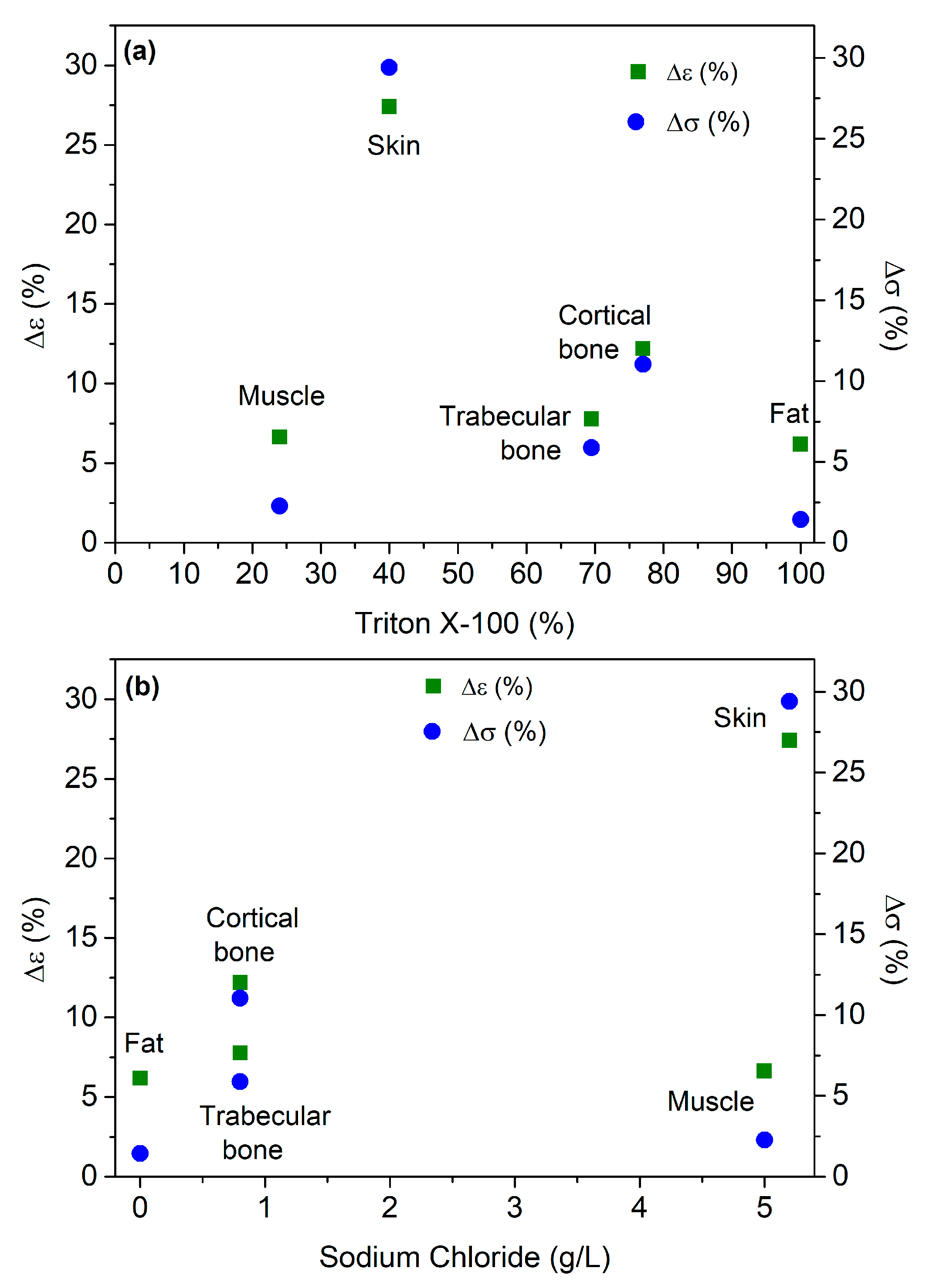
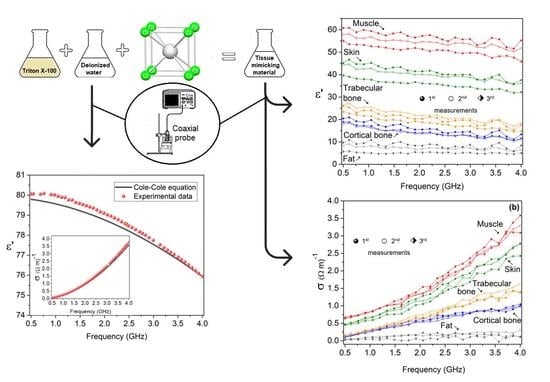
| Target Tissue | Triton X-100 (Volume %) | Deionized Water (Volume %) | NaCl (g/L) |
|---|---|---|---|
| Skin | 40.0 | 60.0 | 5.200 |
| Fat | 100 | 0 | 0 |
| Muscle | 24.0 | 76.0 | 5.000 |
| Cortical bone | 77.0 | 23.0 | 0.800 |
| Trabecular bone | 69.5 | 30.5 | 0.800 |
| Tissue-Mimicking Material | Cole–Cole Model | IT’IS Database | ||
|---|---|---|---|---|
| Δε′ (%) | Δσ (%) | Δε′ (%) | Δσ (%) | |
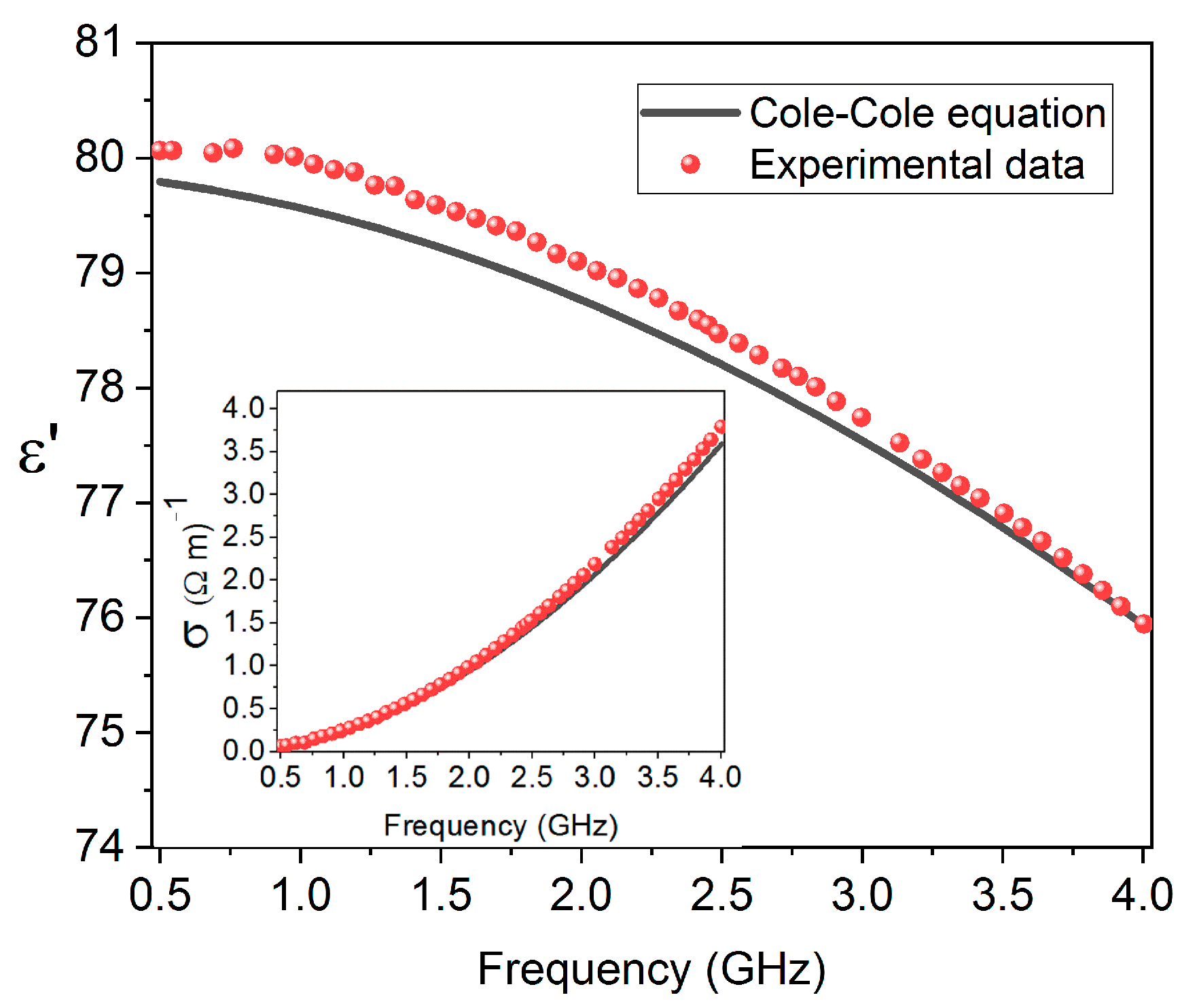
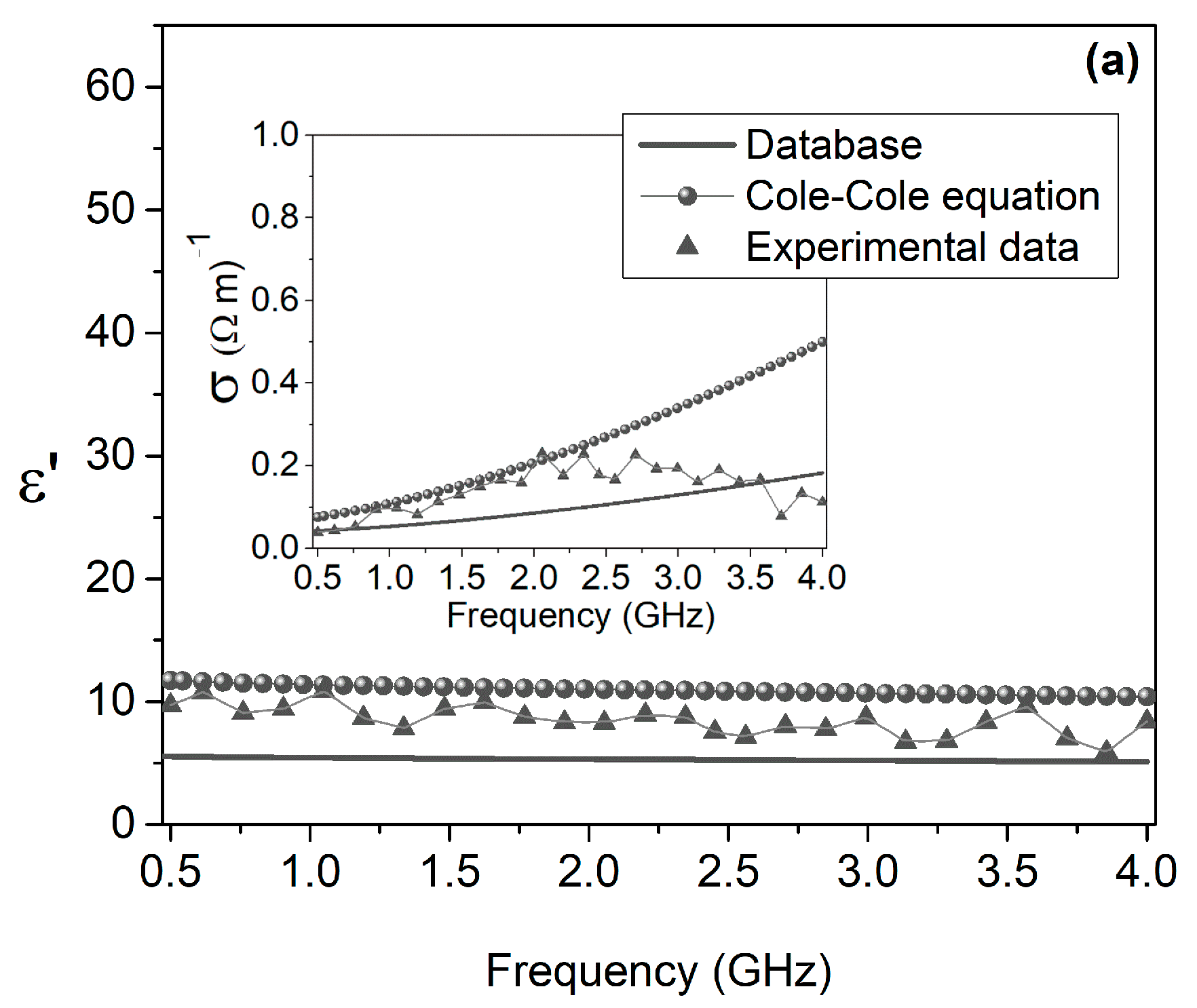
| Skin | 6.09 | 15.17 | 6.20 | 14.77 |
| Fat | 22.83 | 30.43 | 58.14 | 62.65 |
| Muscle | 6.69 | 16.39 | 6.66 | 10.72 |
| Cortical bone | 35.34 | 83.56 | 34.88 | 70.46 |
| Trabecular bone | 8.98 | 11.84 | 9.21 | 8.62 |
| Tissue-Mimicking Material | Dielectric Constant, ε′ | ||||
|---|---|---|---|---|---|
| 1st Measurement | 2nd Measurement | Δε′ (%) | 3rd Measurement | Δε′ (%) | |
| Skin | 7.08 | 6.35 | 10.31 | 5.14 | 27.40 |
| Fat | 13.91 | 12.80 | 8.02 | 13.05 | 6.18 |
| Muscle | 19.01 | 20.21 | 6.29 | 17.75 | 6.63 |
| Cortical bone | 40.32 | 39.25 | 2.67 | 35.41 | 12.18 |
| Trabecular bone | 55.16 | 53.50 | 3.00 | 50.87 | 7.78 |
| Tissue-Mimicking Material | Conductivity, σ | ||||
|---|---|---|---|---|---|
| 1st Measurement | 2nd Measurement | Δσ (%) | 3rd Measurement | Δσ (%) | |
| Skin | 0.17 | 0.13 | 23.99 | 0.12 | 29.41 |
| Fat | 0.70 | 0.66 | 6.88 | 0.69 | 1.43 |
| Muscle | 0.88 | 0.97 | 9.44 | 0.90 | 2.27 |
| Cortical bone | 1.54 | 1.52 | 1.07 | 1.37 | 11.04 |
| Trabecular bone | 1.87 | 1.78 | 4.57 | 1.76 | 5.88 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Relva, M.; Devesa, S. Dielectric Stability of Triton X-100-Based Tissue-Mimicking Materials for Microwave Imaging. Spectrosc. J. 2023, 1, 72-85. https://doi.org/10.3390/spectroscj1020007
Relva M, Devesa S. Dielectric Stability of Triton X-100-Based Tissue-Mimicking Materials for Microwave Imaging. Spectroscopy Journal. 2023; 1(2):72-85. https://doi.org/10.3390/spectroscj1020007
Chicago/Turabian StyleRelva, Mariana, and Susana Devesa. 2023. "Dielectric Stability of Triton X-100-Based Tissue-Mimicking Materials for Microwave Imaging" Spectroscopy Journal 1, no. 2: 72-85. https://doi.org/10.3390/spectroscj1020007
APA StyleRelva, M., & Devesa, S. (2023). Dielectric Stability of Triton X-100-Based Tissue-Mimicking Materials for Microwave Imaging. Spectroscopy Journal, 1(2), 72-85. https://doi.org/10.3390/spectroscj1020007