Electronic Correlations in Ferromagnetic Heusler Alloy ln2MnW: Insights from First-Principles Calculations
Abstract
1. Introduction
2. Computational Method
3. Results and Discussion
3.1. Structural Properties
3.2. Lattice Parameters
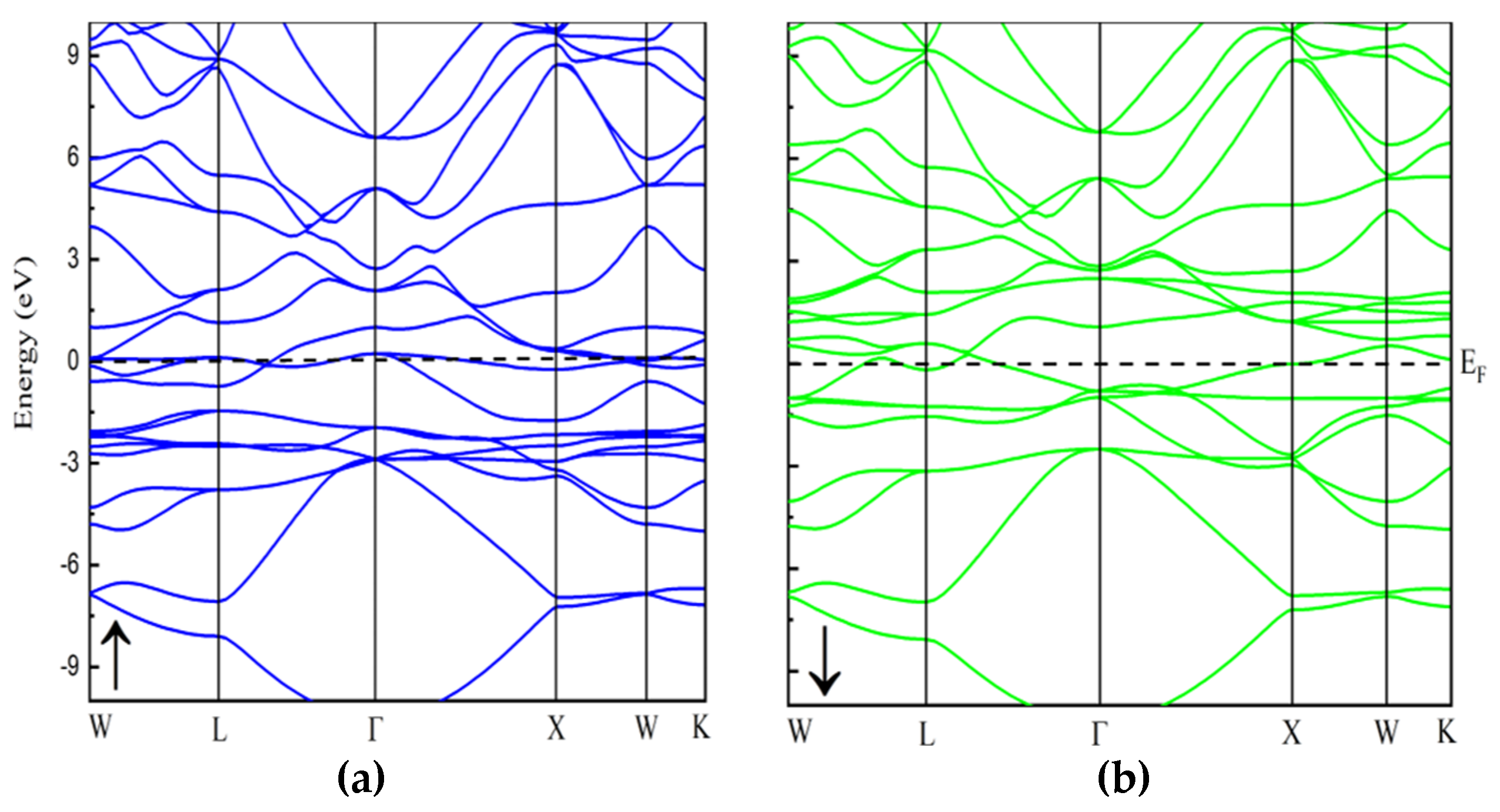
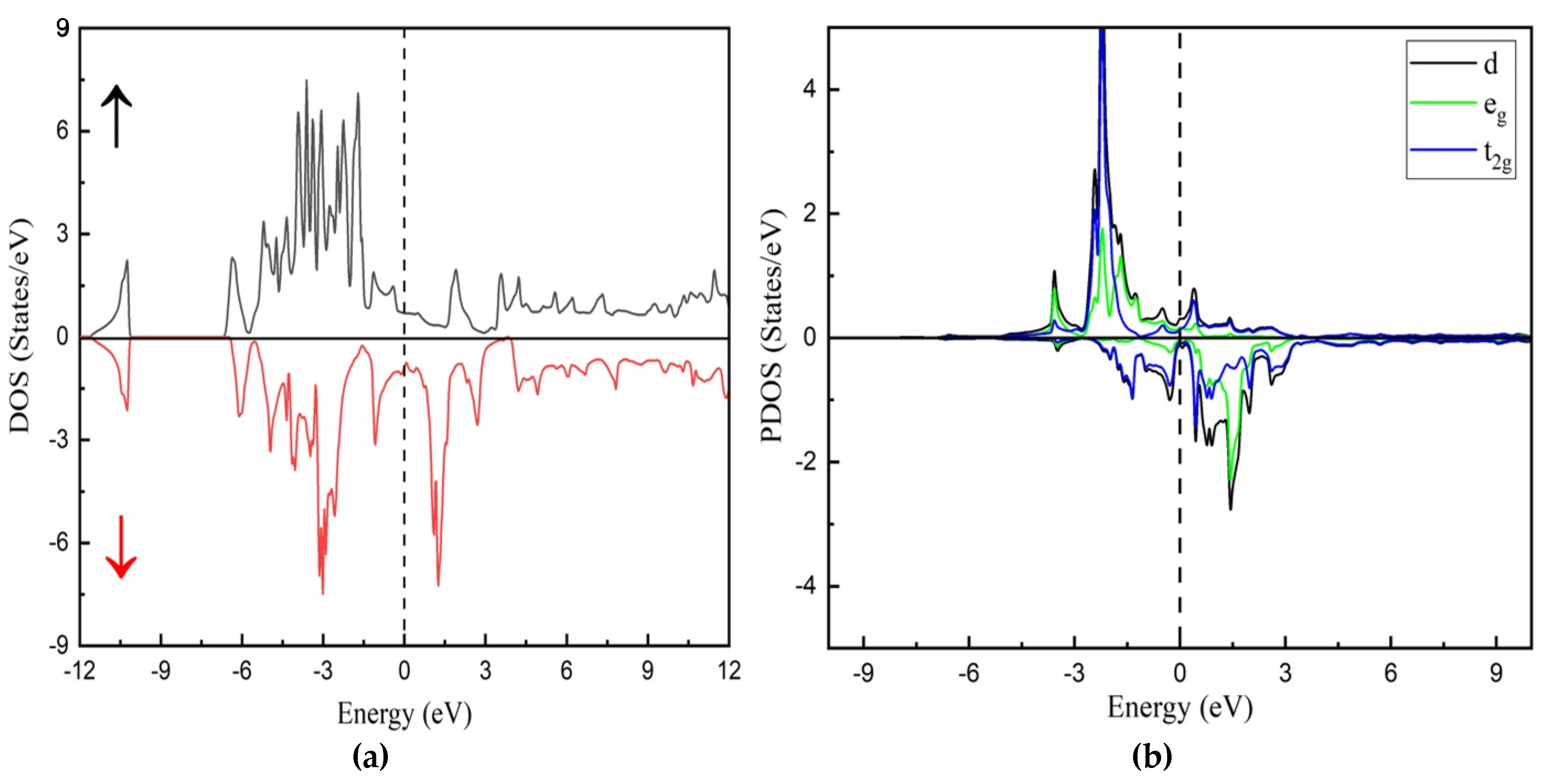
3.3. Electronic Characteristics
3.4. Magnetic Properties
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Graf, T.; Felser, C.; Parkin, S.S.P. Heusler Compounds: Applications in Spintronics. In Handbook of Spintronics; Springer: Dordrecht, The Netherlands, 2016; pp. 335–364. [Google Scholar] [CrossRef]
- Bulman, G.; Cook, B. High-efficiency energy harvesting using TAGS-85/half-Heusler thermoelectric devices. Energy Harvest. Storage: Mater. Devices Appl. V 2014, 9115, 17–24. [Google Scholar] [CrossRef]
- Erkisi, A.; Surucu, G. The investigation of electronic, magnetic, mechanical, and lattice dynamical properties of Pd MX (M = Cr, Fe and X = Si and Ge) ferromagnetic half-Heusler metallics: An ab initio study. Mater. Res. Express. 2017, 4, 066504. [Google Scholar] [CrossRef]
- Wang, C.; Meyer, J.; Teichert, N.; Auge, A.; Rausch, E.; Balke, B.; Hütten, A.; Fecher, G.H.; Felser, C. Heusler nanoparticles for spintronics and ferromagnetic shape memory alloys. J. Vac. Sci. Technol. B 2014, 32, 020802. [Google Scholar] [CrossRef]
- Hirohata, A.; Sukegawa, H.; Yanagihara, H.; Zutic, I.; Seki, T.; Mizukami, S.; Swaminathan, R. Roadmap for Emerging Materials for Spintronic Device Applications. IEEE Trans. Magn. 2015, 51, 1–11. [Google Scholar] [CrossRef]
- Chauhan, N.S.; Miyazaki, Y. Contrasting role of bismuth doping on the thermoelectric performance of VFeSb half-Heusler. J. Alloys Compd. 2022, 908, 164623. [Google Scholar] [CrossRef]
- Agbaoye, R.O.; Adebambo, P.O.; Adetunji, B.I.; Osafile, O.; Adebayo, G.A. Thermoelectric properties, optimal doping levels and high figure of merit in Cobalt-based Half/Full Heusler alloys by First-Principles calculations. Mater. Sci. Eng. B 2019, 248, 114409. [Google Scholar] [CrossRef]
- Adebambo, P.O.; Osafile, O.E.; Laoye, J.A.; Idowu, M.A.; Adebayo, G.A. Electronic fitness function, effective mass and thermoelectric properties of Rh-based (-ScTe; -TiSb; -VSn) alloys for thermoelectric generator applications. Comput. Condens. Matter 2021, 26, e00523. [Google Scholar] [CrossRef]
- Graf, T.; Parkin, S.S.P.; Felser, C. Heusler Compounds—A Material Class With Exceptional Properties. IEEE Trans. Magn. 2011, 47, 367–373. [Google Scholar] [CrossRef]
- Xie, W.; Weidenkaff, A.; Tang, X.; Zhang, Q.; Poon, J.; Tritt, T. Recent Advances in Nanostructured Thermoelectric Half-Heusler Compounds. Nanomaterials 2012, 2, 379–412. [Google Scholar] [CrossRef]
- Osafile, O.E.; Azi, J.O. Structural, electronic, elastic and mechanical properties of novel ZrMnAs half Heusler alloy from first principles. Phys. B Condens. Matter 2019, 571, 41–49. [Google Scholar] [CrossRef]
- Terris, B.D.; Thomson, T. Nanofabricated and self-assembled magnetic structures as data storage media. J. Phys. D Appl. Phys. 2005, 38, R199–R222. [Google Scholar] [CrossRef]
- Kervan, N.; Kervan, S. A first-principle study of half-metallic ferrimagnetism in the Ti2CoGa Heusler compound. J. Magn. Magn. Mater. 2012, 324, 645–648. [Google Scholar] [CrossRef]
- Berri, S.; Maouche, D.; Ibrir, M.; Zerarga, F. A first-principle study of half-metallic ferrimagnetism in the CoFeTiSb quaternary Heusler compound. J. Magn. Magn. Mater. 2014, 354, 65–69. [Google Scholar] [CrossRef]
- Lei, F.; Tang, C.; Wang, S.; He, W. Half-metallic full-Heusler compound Ti2NiAl: A first-principles study. J. Alloys Compd. 2011, 509, 5187–5189. [Google Scholar] [CrossRef]
- Birsan, A. Magnetism in the new full-Heusler compound, Zr2CoAl: A first-principles study. Curr. Appl. Phys. 2014, 14, 1434–1436. [Google Scholar] [CrossRef]
- Zada, Z.; Khan, A.A.; Reshak, A.H.; Khan, A.M.; Shakeel, S.; Ali, D.; Ismail, M.; Ramli, M.M. A first principles study of Palladium-based full Heusler ferromagnetic Pd2MnSb compound. Opt. Quant. Electron. 2024, 56, 248. [Google Scholar] [CrossRef]
- Kulkova, S.E.; Eremeev, S.V.; Kakeshita, T.; Kulkov, S.S.; Rudenski, G.E. The electronic structure and magnetic properties of full-and half-Heusler alloys. Mater. Trans. 2006, 47, 599–606. [Google Scholar] [CrossRef]
- Munir, J.; Jamil, M.; Jbara, A.S.; Fatima, K.; Ain, Q.; Ullah, H.; Yousaf, M. Spin-polarized electromagnetic and optical response of full-Heusler Co2VZ (Z = Al, Be) alloys for spintronic application. Eur. Phys. J. Plus 2021, 136, 1009. [Google Scholar] [CrossRef]
- Dahmane, F.; Mogulkoc, Y.; Doumi, B.; Tadjer, A.; Khenata, R.; Omran, S.B.; Rai, D.P.; Murtaza, G.; Varshney, D. Structural, electronic and magnetic properties of Fe2-based full Heusler alloys: A first principle study. J. Magn. Magn. Mater. 2016, 407, 167–174. [Google Scholar] [CrossRef]
- Page, A.; Uher, C.; Poudeu, P.F.; Van der Ven, A. Phase separation of full-Heusler nanostructures in half-Heusler thermoelectrics and vibrational properties from first-principles calculations. Phys. Rev. B 2015, 92, 174102. [Google Scholar] [CrossRef]
- Rogl, G.; Grytsiv, A.; Gürth, M.; Tavassoli, A.; Ebner, C.; Wünschek, A.; Puchegger, S.; Soprunyuk, V.; Schranz, W.; Bauer, E.; et al. Mechanical properties of half-Heusler alloys. Acta Mater. 2016, 107, 178–195. [Google Scholar] [CrossRef]
- Dahal, R.; Kaphle, G.C. Structural, electronic and magnetic properties of xyz type half-heusler alloys. J. Nepal Phys. Soc. 2019, 5, 97–102. [Google Scholar]
- Fiedler, G.; Kratzer, P. Ternary Semiconductors NiZrSn and CoZrBi with half-Heusler structure: A first-principles study. Phys. Rev. B 2016, 94, 075203. [Google Scholar] [CrossRef]
- Wakeel, M.; Murtaza, G.; Ullah, H.; Khan, S.; Laref, A.; Ameer, Z.; Khan, S.A. Structural, electronic, and magnetic properties of palladium based full Heusler compounds: DFT study. Phys. B Condens. Matter 2021, 608, 412716. [Google Scholar] [CrossRef]
- Schwarz, K.; Blaha, P.; Madsen, G.K.H. Electronic structure calculations of solids using the WIEN2k package for material sciences. Comput. Phys. Commun. 2002, 147, 71–76. [Google Scholar] [CrossRef]
- Blaha, P.; Schwarz, K.; Sorantin, P.; Trickey, S.B. Full-potential, linearized augmented plane wave programs for crystalline systems. Comput. Phys. Commun. 1990, 59, 399–415. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Nasarullah; Yaseen, M.; Aldaghfag, S.A.; Zahid, M.; Misbah. Physical characteristics of X2NaMoBr6 (X = K, Rb): A DFT study. Mater. Sci. Semicond. Process. 2022, 147, 106760. [Google Scholar] [CrossRef]
- Graf, T.; Casper, F.; Winterlik, J.; Balke, B.; Fecher, G.H.; Felser, C. Crystal Structure of New Heusler Compounds. Z. Anorg. Allg. Chem. 2009, 635, 976–981. [Google Scholar] [CrossRef]
- Birch, F. The effect of pressure upon the elastic parameters of isotropic solids, according to Murnaghan’s theory of finite strain. J. Appl. Phys. 1938, 9, 279–288. [Google Scholar] [CrossRef]
- Rauf, S.; Arif, S.; Haneef, M.; Amin, B. The first principle study of magnetic properties of Mn2WSn, Fe2YSn (Y=Ti, V), Co2YSn (Y=Ti, Zr, Hf, V, Mn) and Ni2YSn (Y=Ti, Zr, Hf, V, Mn) heusler alloys. J. Phys. Chem. Solids 2015, 76, 153–169. [Google Scholar] [CrossRef]
- Nazar, M.; Nasarullah; Aldaghfag, S.A.; Yaseen, M.; Waqas, M.; Butt, M.K.; Boukhris, I. Electronic, Structural, Optical, and Magnetic Characteristics A2MgS4 of (A=Dy, Er) Spinel Sulfides: A Density Functional Theory Study. Phys. Status Solidi B Basic Res. 2023, 260, 2300003. [Google Scholar] [CrossRef]
- Nazar, M.; Nasarullah; Aldaghfag, M.I.S.A.; Yaseen, M.; Khera, S.N.R.A.; Abdellattif, M.H. First-principles calculations to investigate structural, magnetic, optical, electronic and thermoelectric properties of X2MgS4(X = Gd, Tm) spinel sulfides. J. Phys. Chem. Solids 2022, 166, 110719. [Google Scholar] [CrossRef]
- Gökoğlu, G. Ab initio electronic structure of NiCoCrGa half-metallic quaternary Heusler compound. Solid State Sci. 2012, 14, 1273–1276. [Google Scholar] [CrossRef]
- Hao, Z.; Liu, R.; Fan, Y.; Wang, L. First-principles calculations of a new half-metallic Heusler alloy FeCrAs. J. Alloys Compd. 2020, 820, 153118. [Google Scholar] [CrossRef]
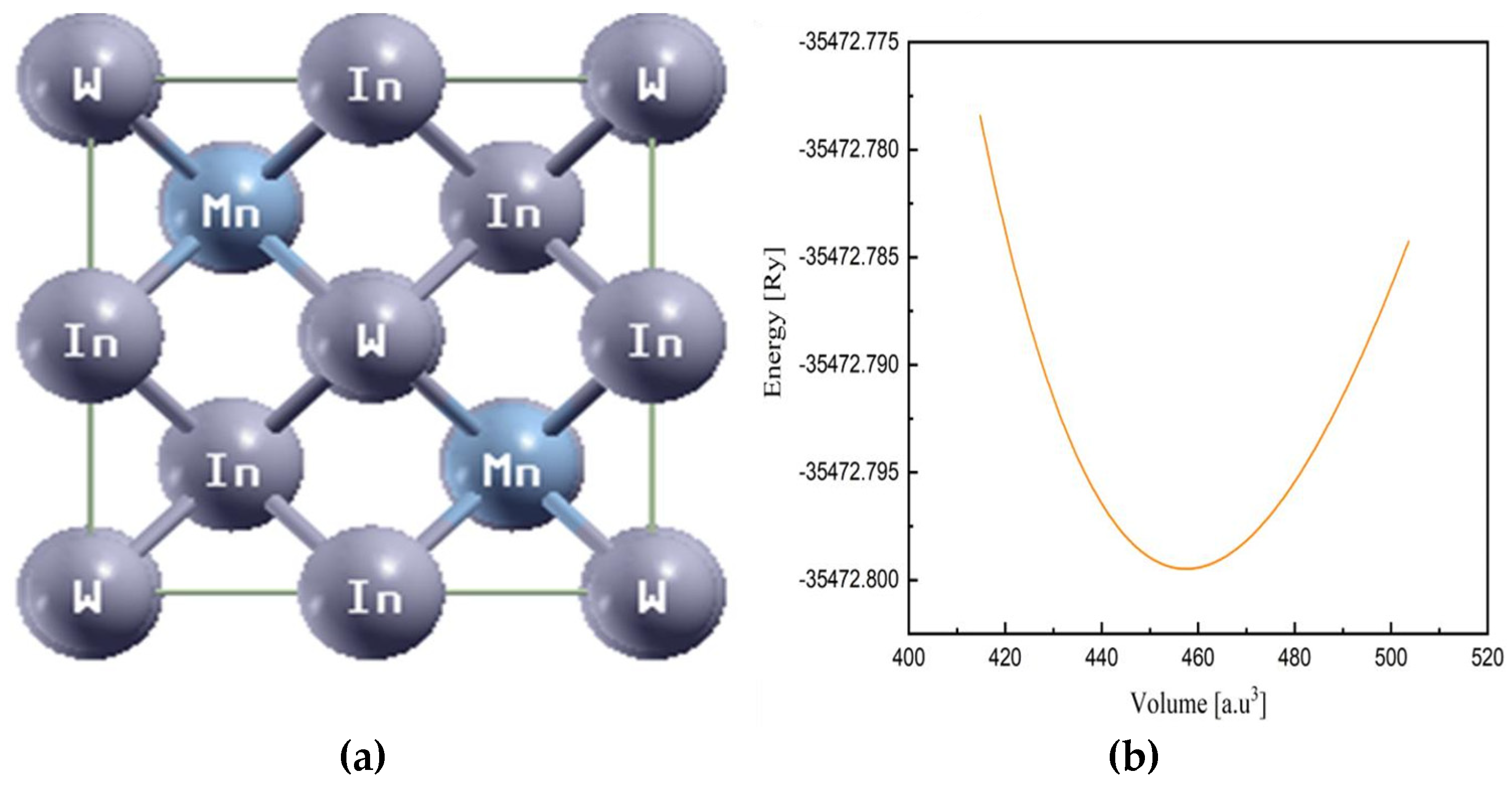



| Lattice Parameters | Lattice Constant | Vo | BP | B (Gpa) | E0 | ||
|---|---|---|---|---|---|---|---|
| PM | FM | AFM | |||||
| ln2MnW Exp. a,c | 6.33 6.413 a 6.420 c | 490.1032 ----------- | 5.00 ----------- | 203.9194 --------- | −58,182.48 ------------- | −58,182.51 ------------- | −581,821.51 -------------- |
| Parameter | GGA | GGA + U |
|---|---|---|
| Total magnetic moment | 4.33 | 4.408 |
| Magnetic moment of Mn | 4.1 | 4.1 |
| Magnetic moment of W | −0.02600 | −0.02500 |
| Magnetic moment of ln | 0.17218 | 0.15 |
| Interstitial | 0.17218 | 0.083 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, A.M.; Zahoor, U. Electronic Correlations in Ferromagnetic Heusler Alloy ln2MnW: Insights from First-Principles Calculations. Alloys 2025, 4, 5. https://doi.org/10.3390/alloys4020005
Khan AM, Zahoor U. Electronic Correlations in Ferromagnetic Heusler Alloy ln2MnW: Insights from First-Principles Calculations. Alloys. 2025; 4(2):5. https://doi.org/10.3390/alloys4020005
Chicago/Turabian StyleKhan, Abdul Munam, and Uzma Zahoor. 2025. "Electronic Correlations in Ferromagnetic Heusler Alloy ln2MnW: Insights from First-Principles Calculations" Alloys 4, no. 2: 5. https://doi.org/10.3390/alloys4020005
APA StyleKhan, A. M., & Zahoor, U. (2025). Electronic Correlations in Ferromagnetic Heusler Alloy ln2MnW: Insights from First-Principles Calculations. Alloys, 4(2), 5. https://doi.org/10.3390/alloys4020005