Theoretical Studies on the Motions of Cloud and Precipitation Particles—A Review
Abstract
1. Introduction
2. General Mathematics Formulation of Hydrometeor Motion in Air
3. Motions of Cloud Drops and Rain Drops
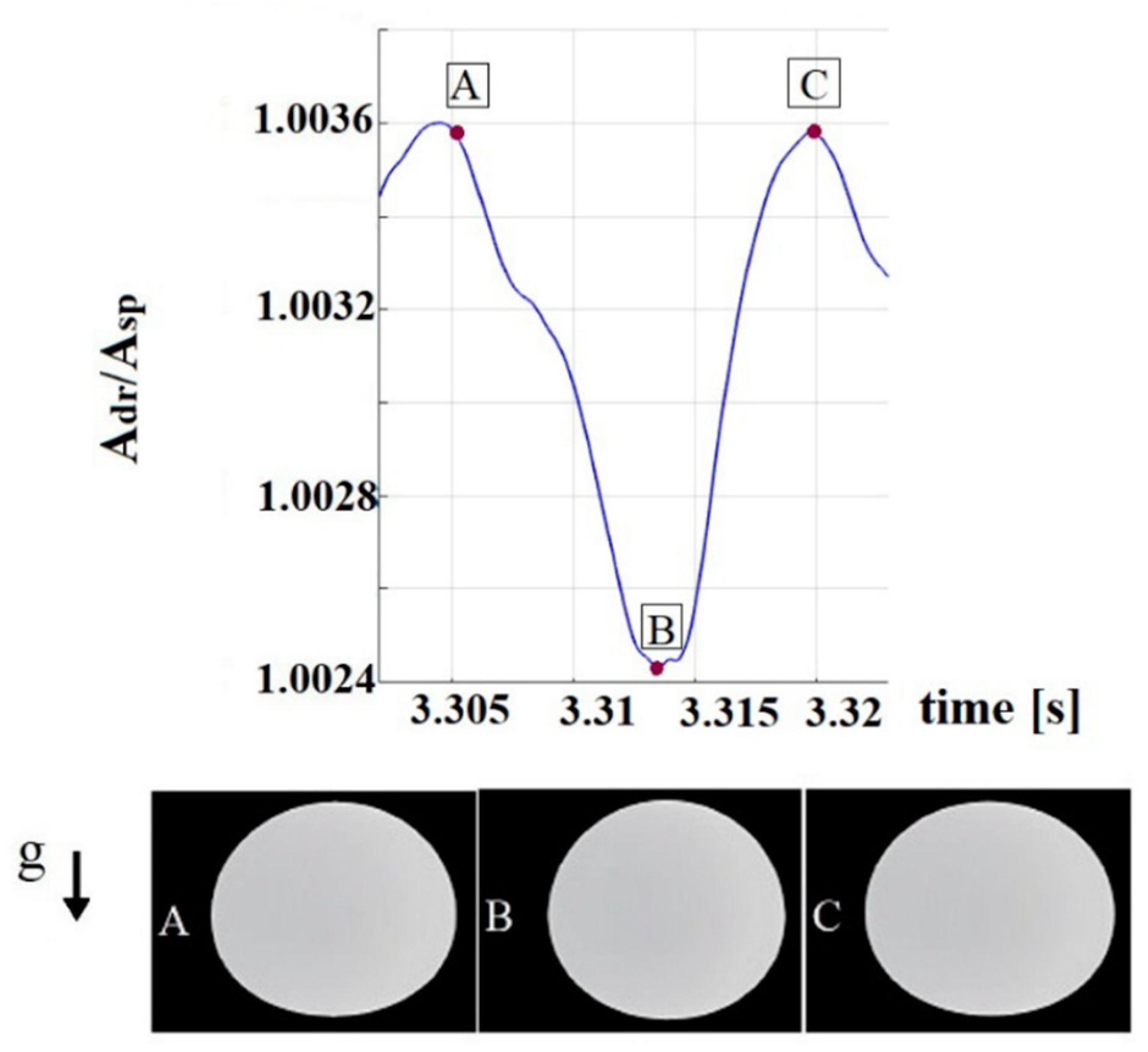
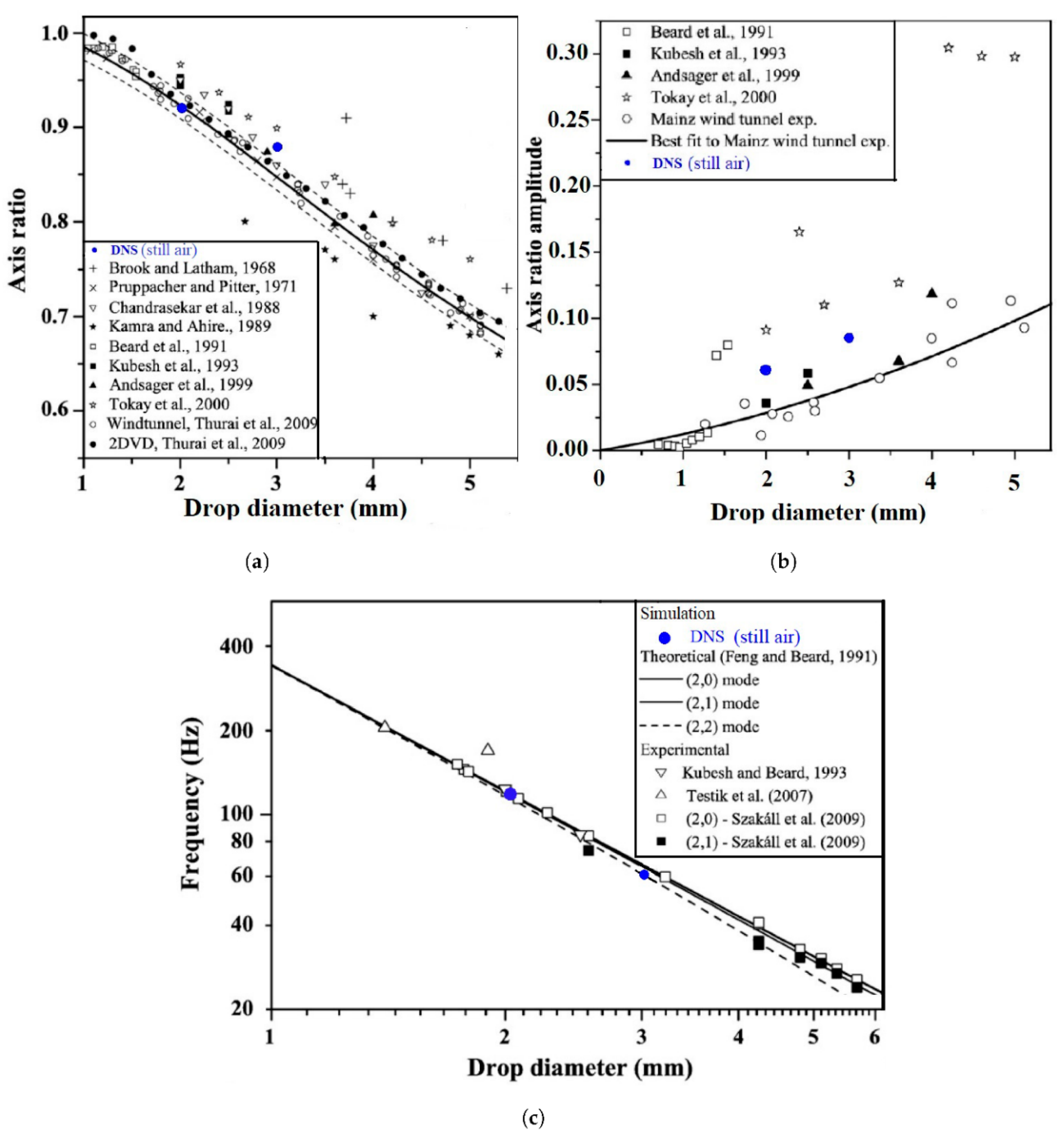
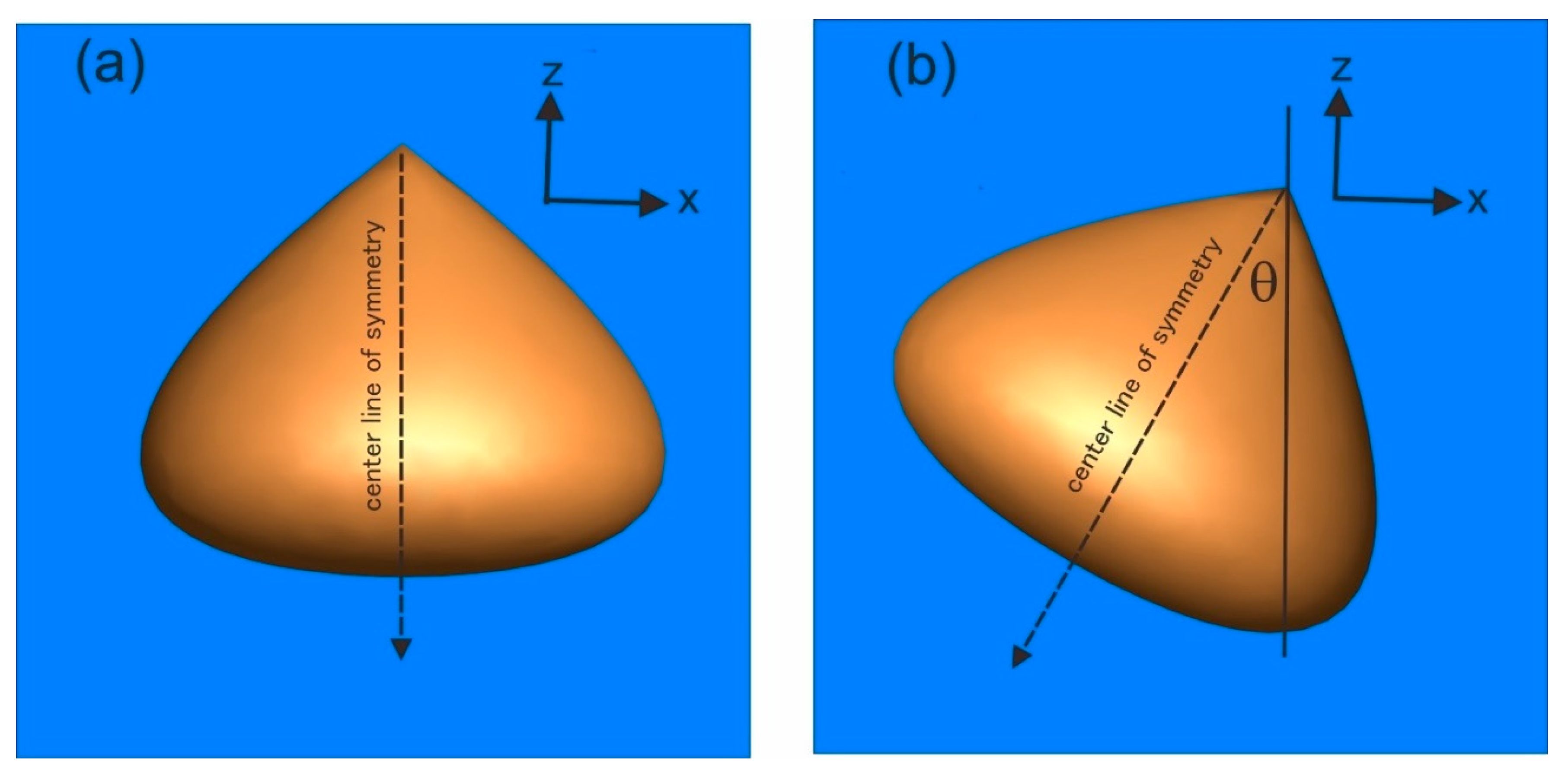
3.1. Observations of the Fall Attitudes of Water Drops
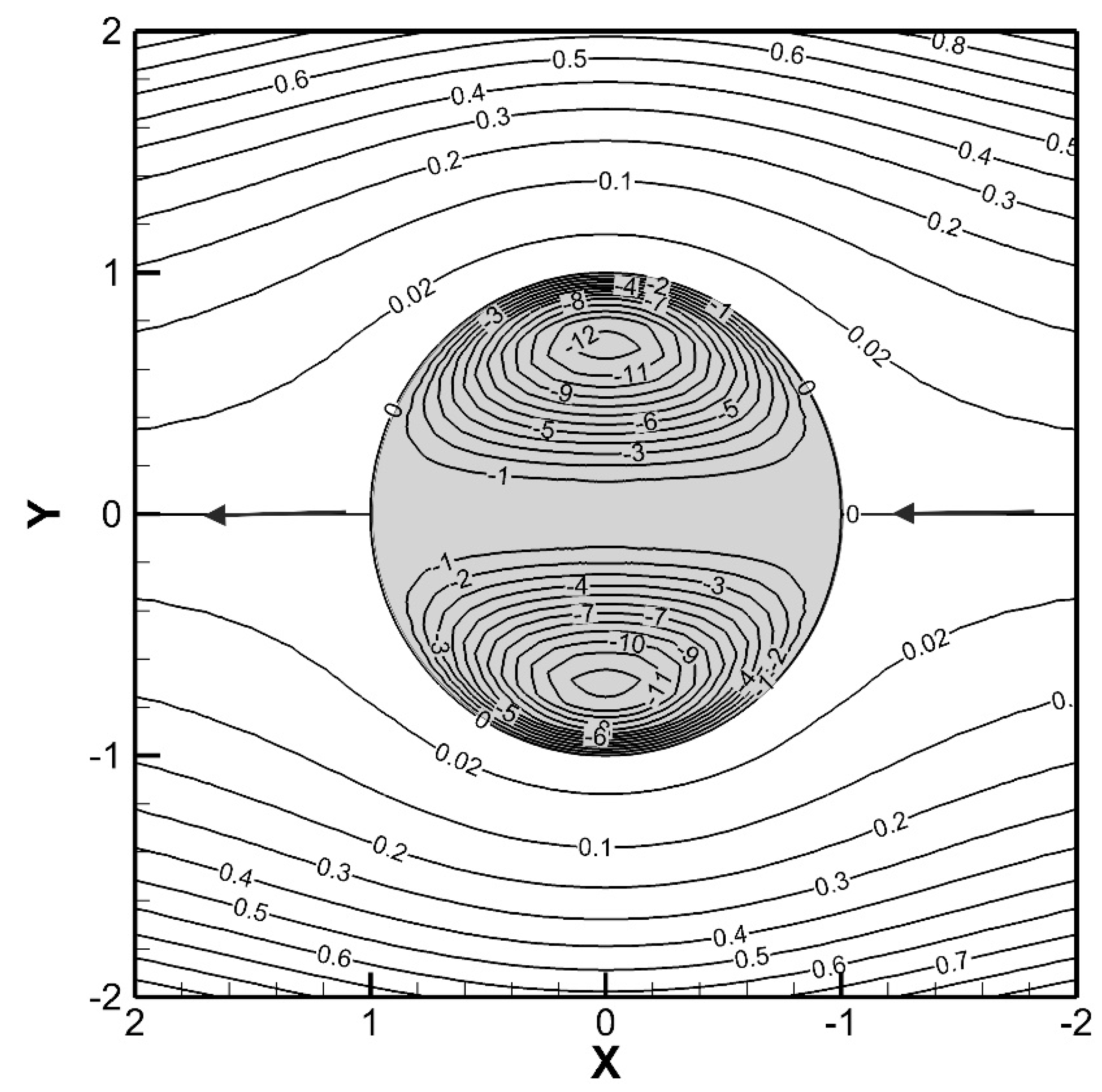
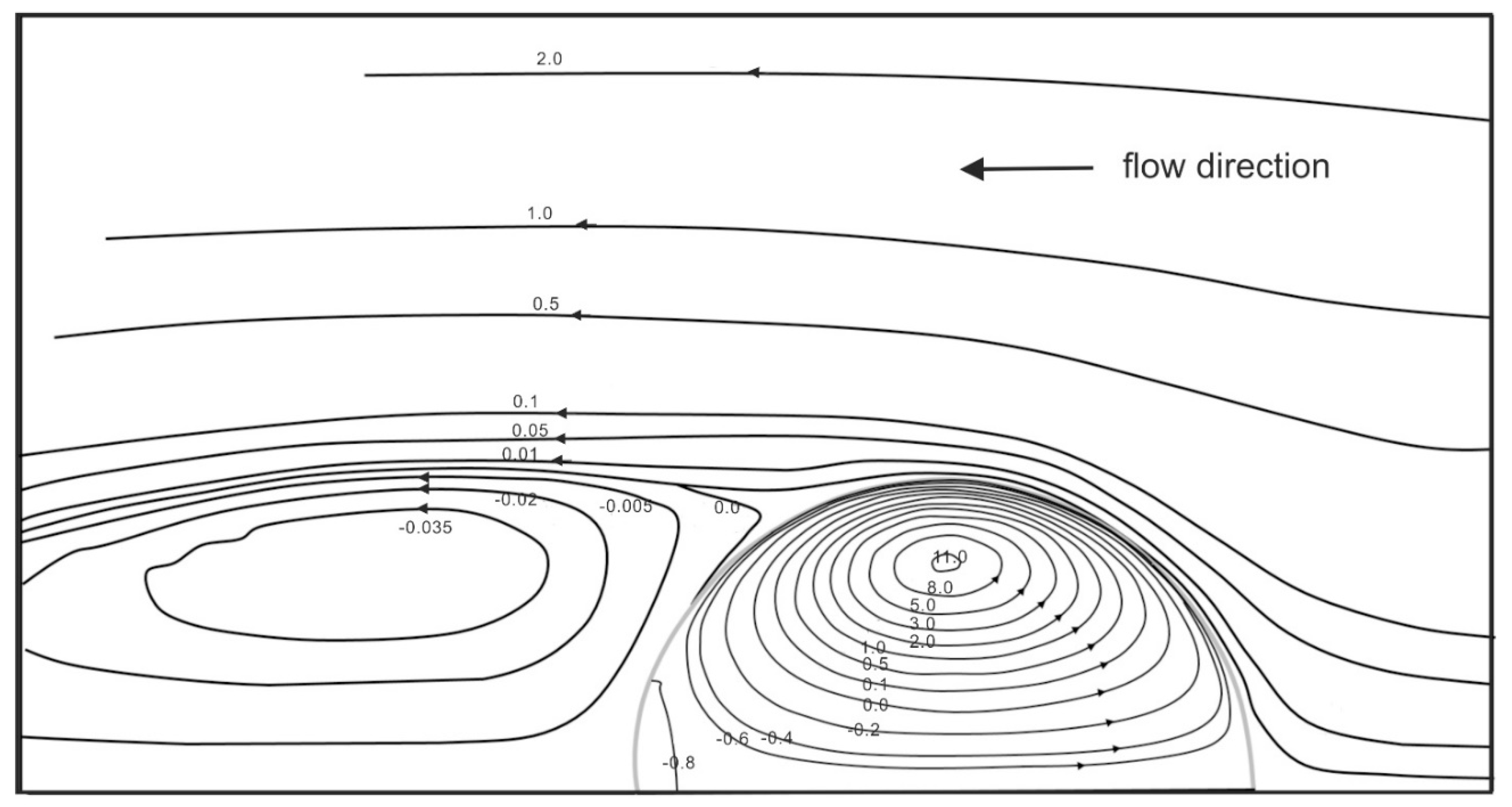
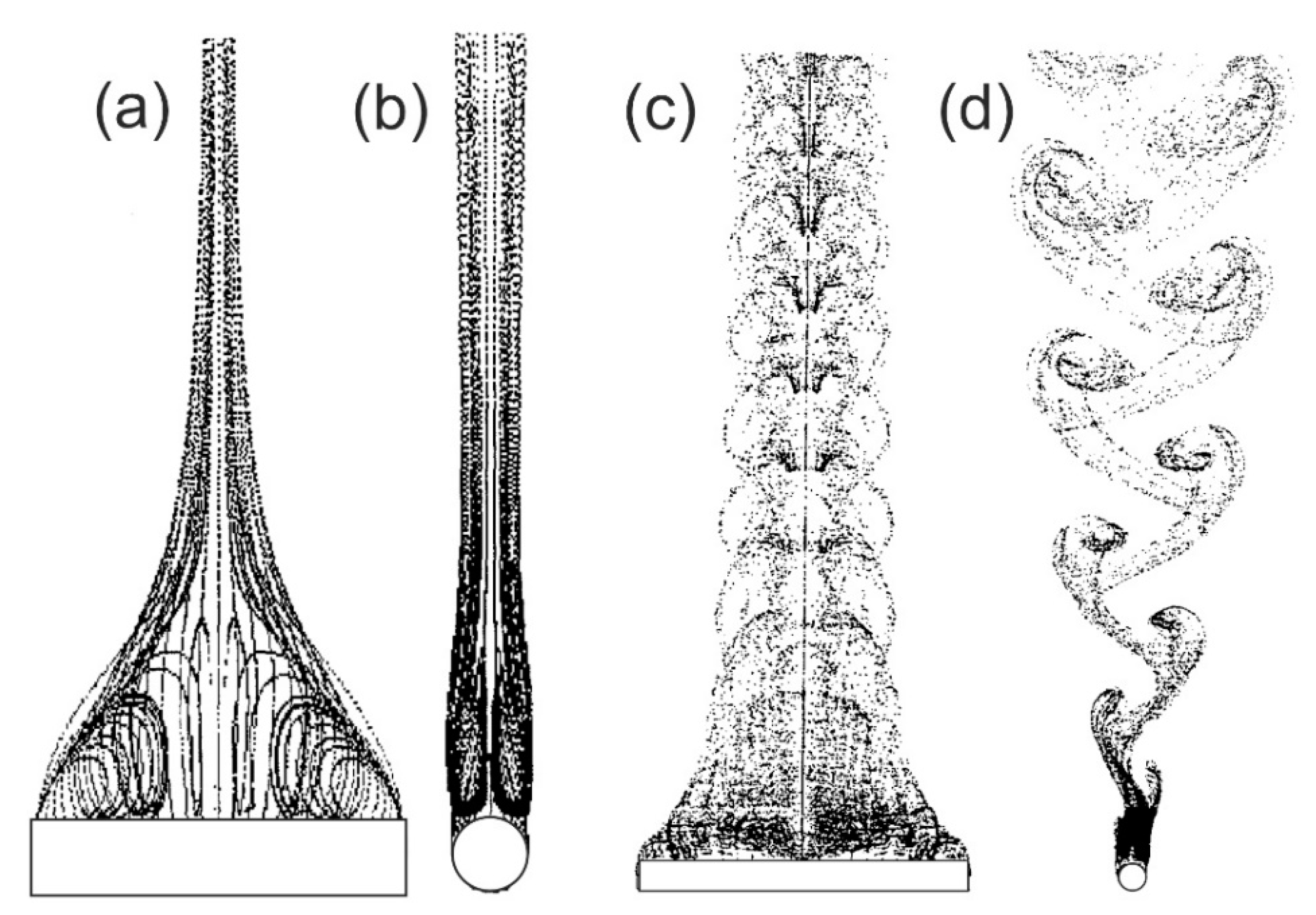
3.2. Flow Fields around Falling Cloud Drops and Raindrops
4. Ice Particles
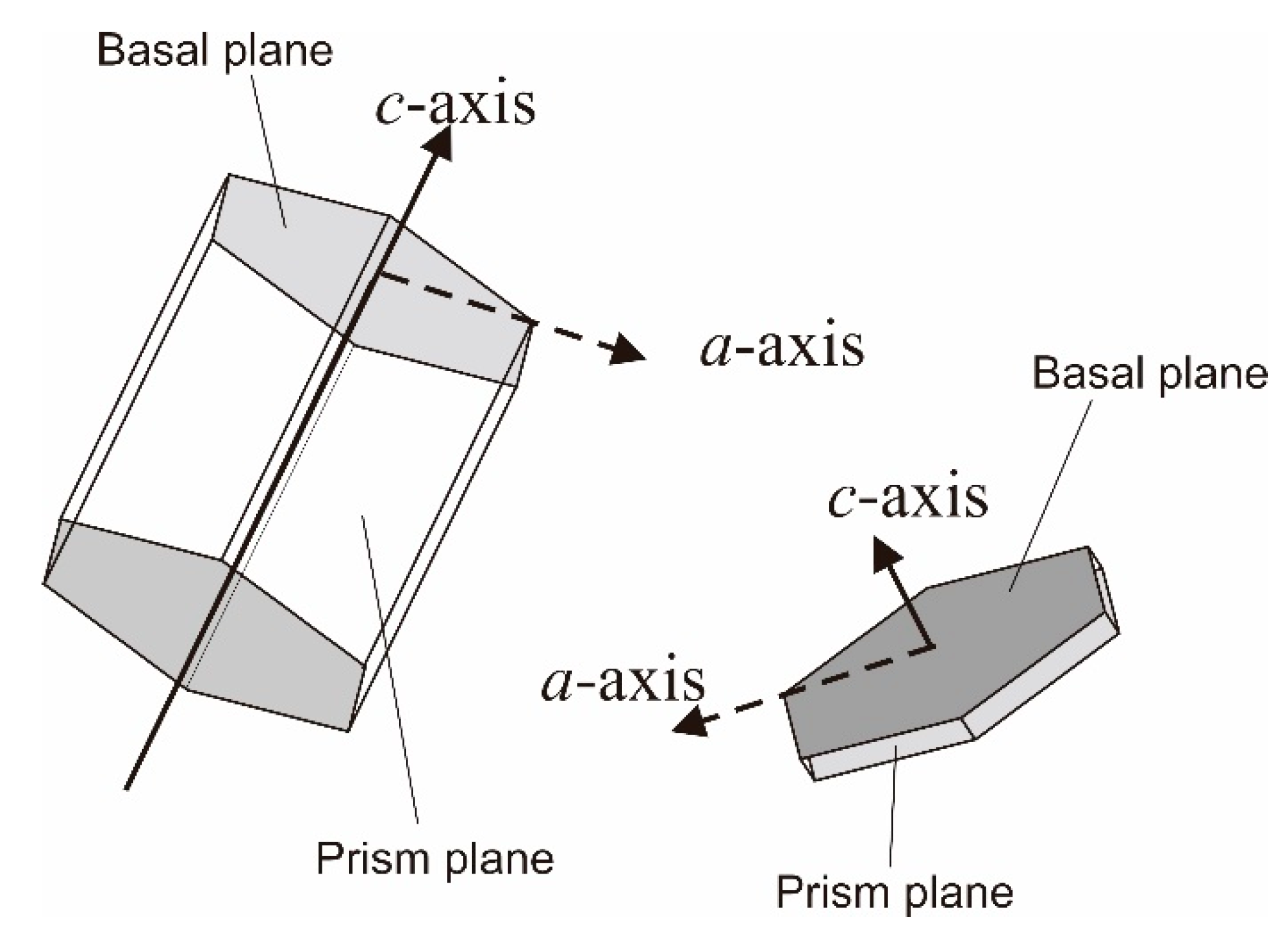
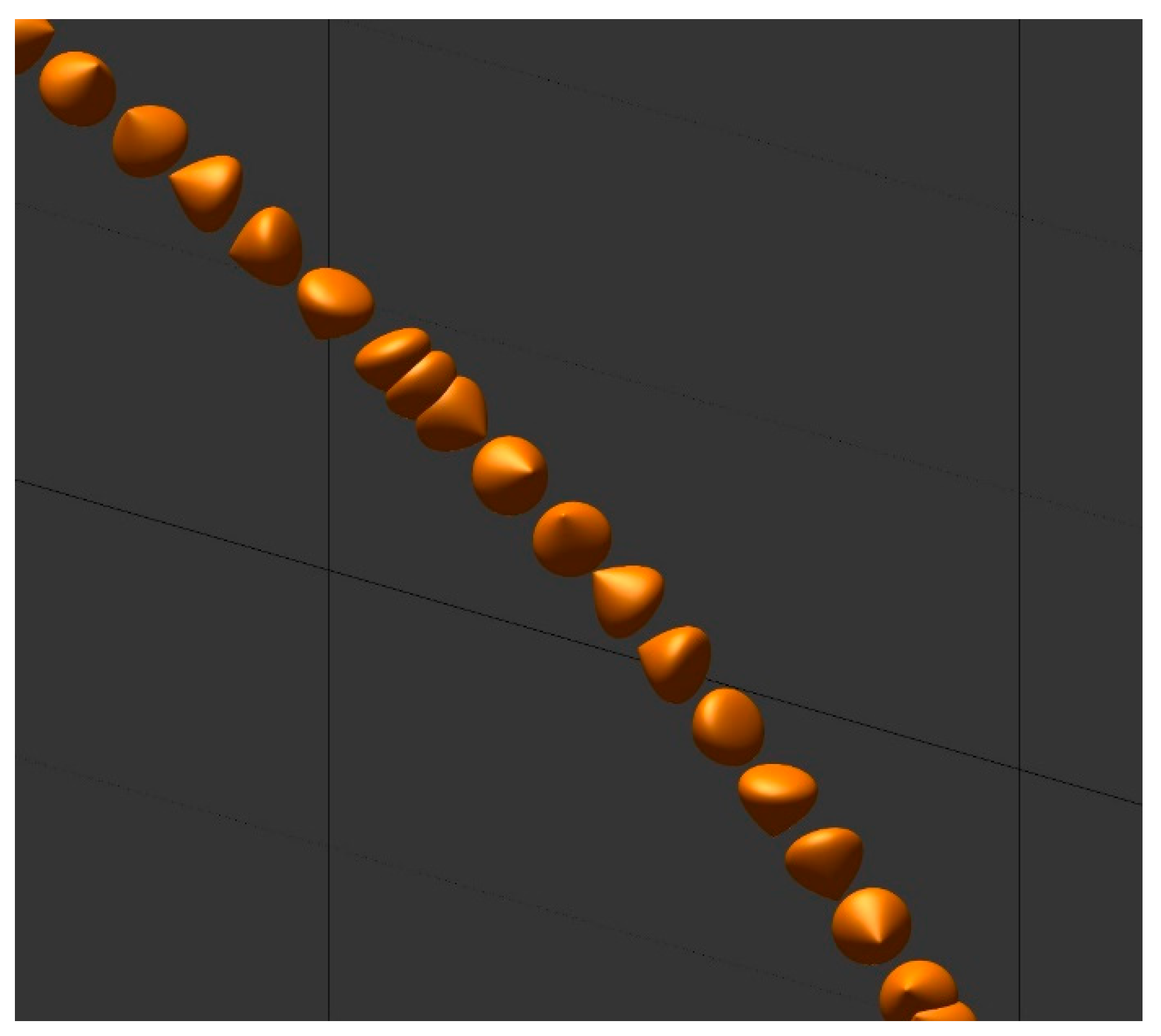
4.1. Observations of Ice Particle Fall Attitudes
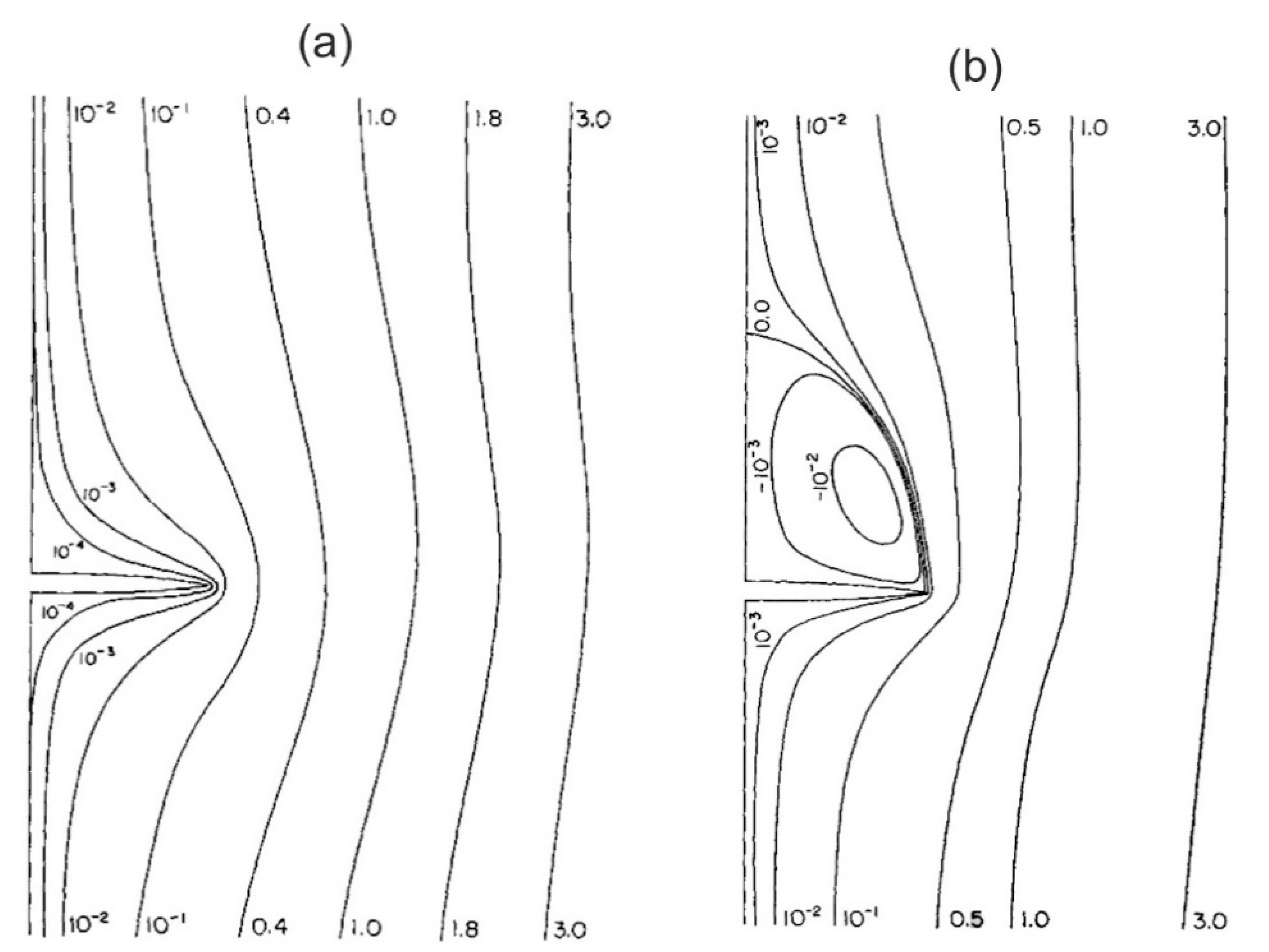
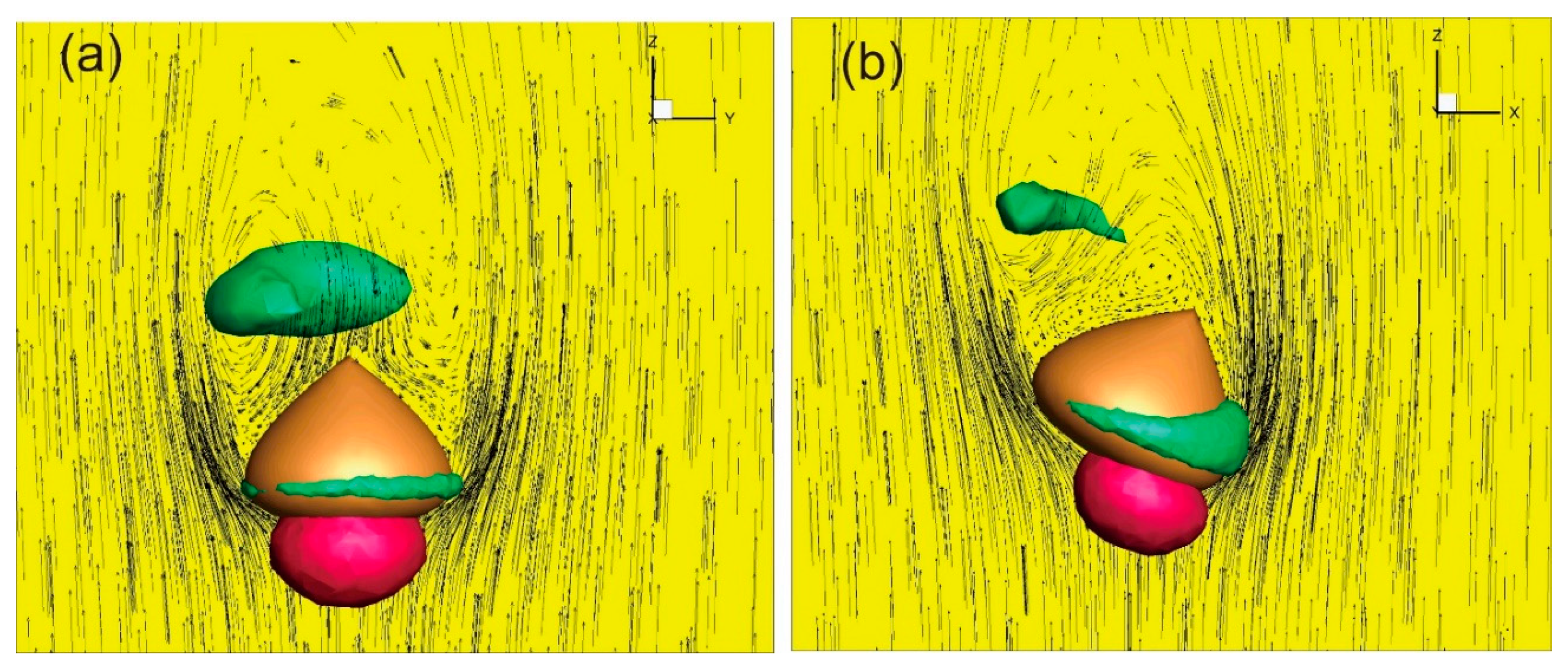
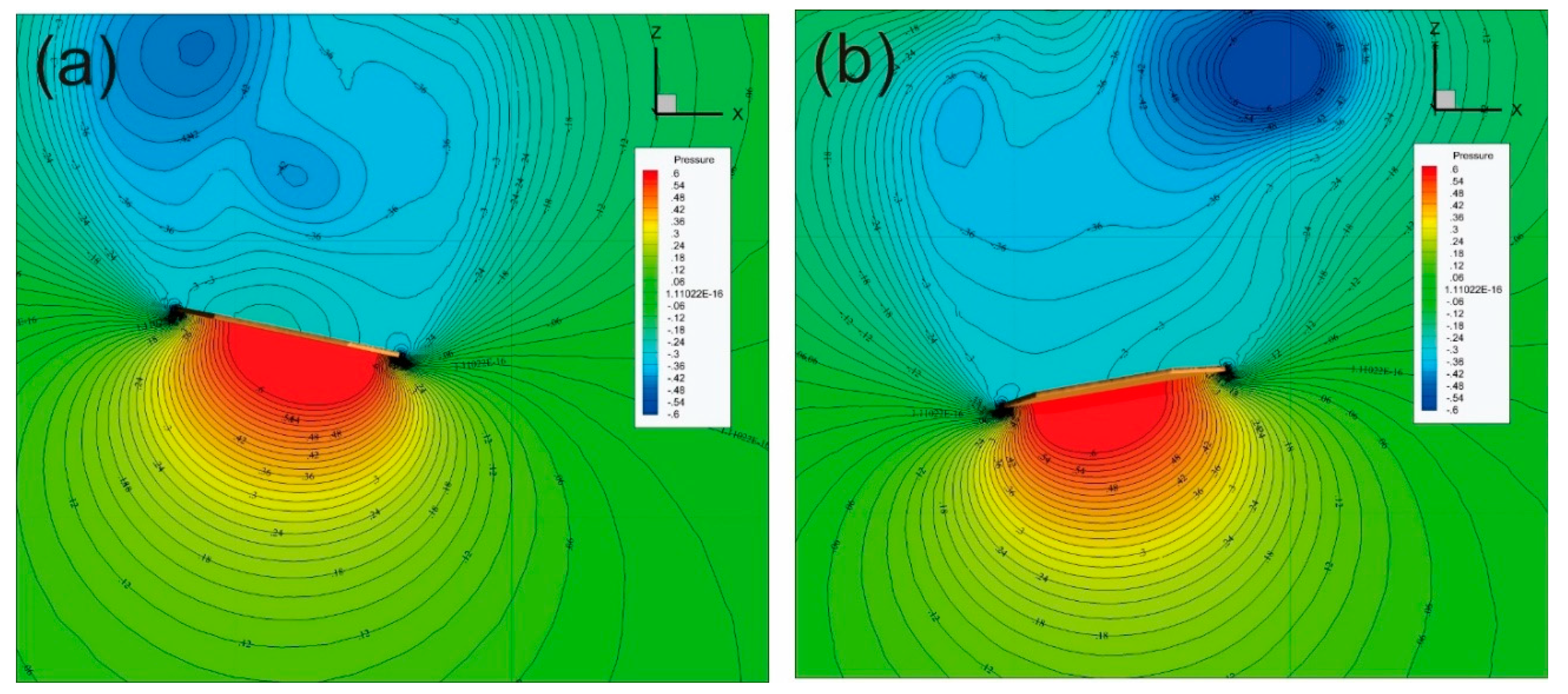
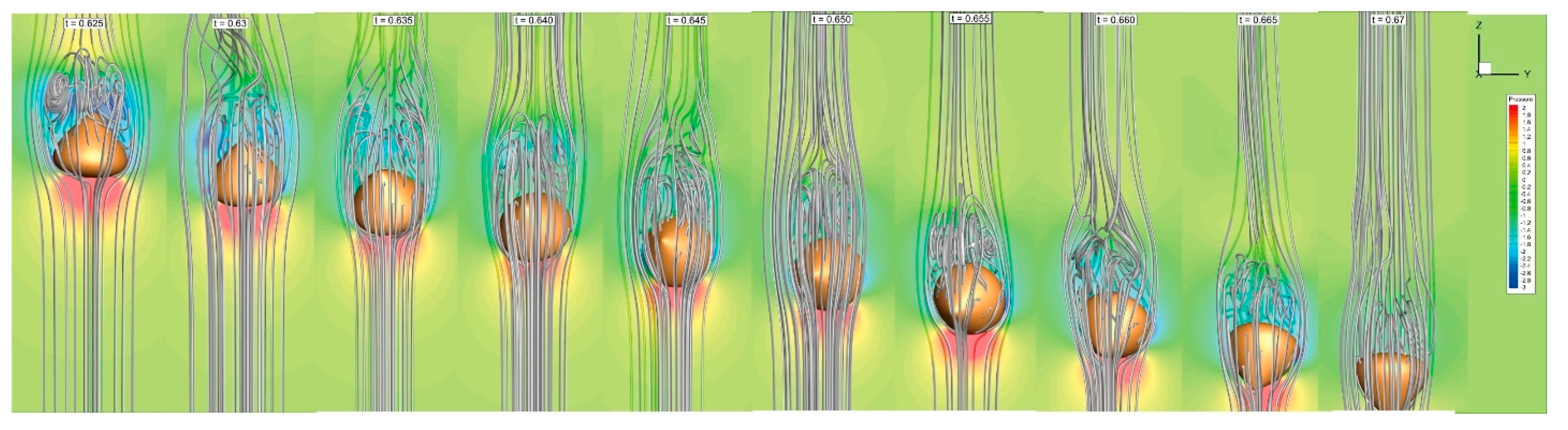
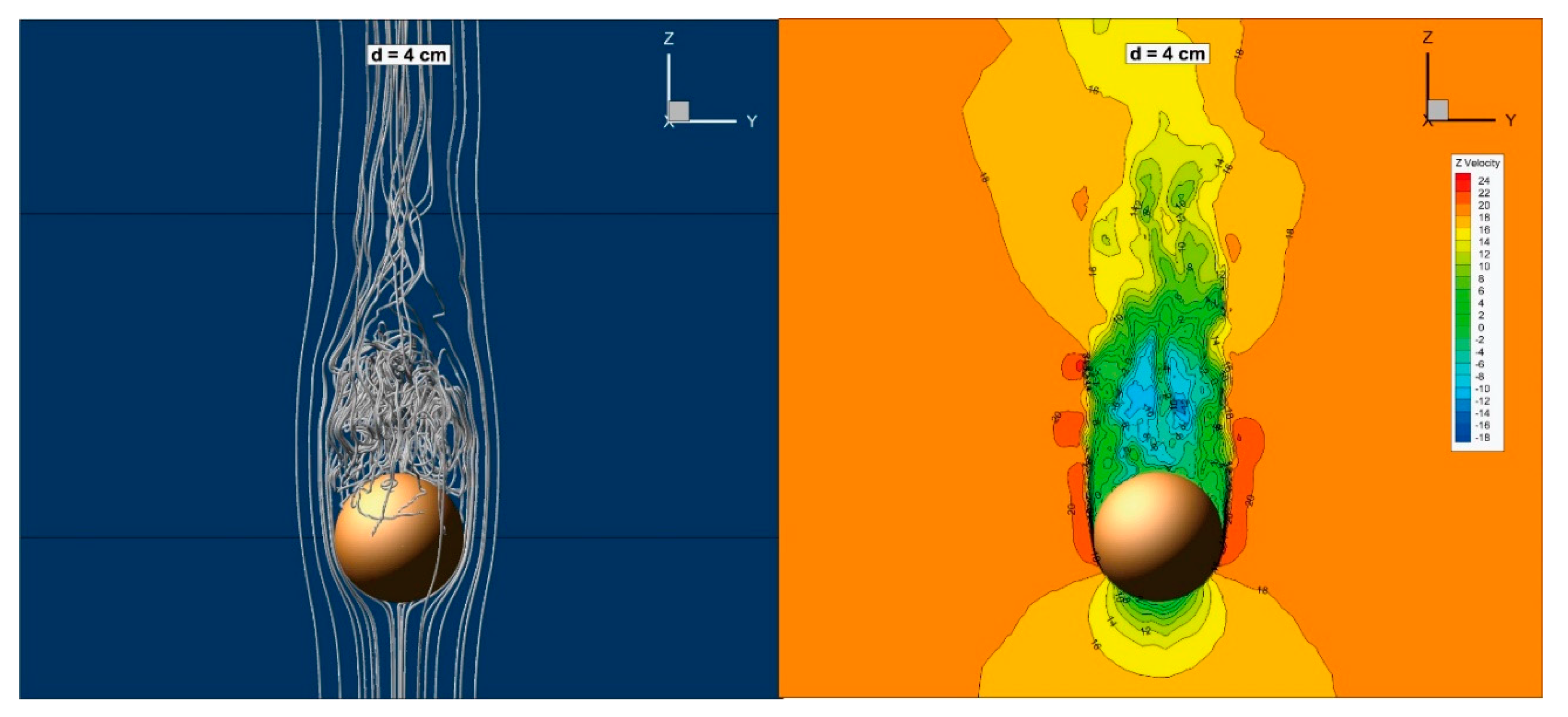
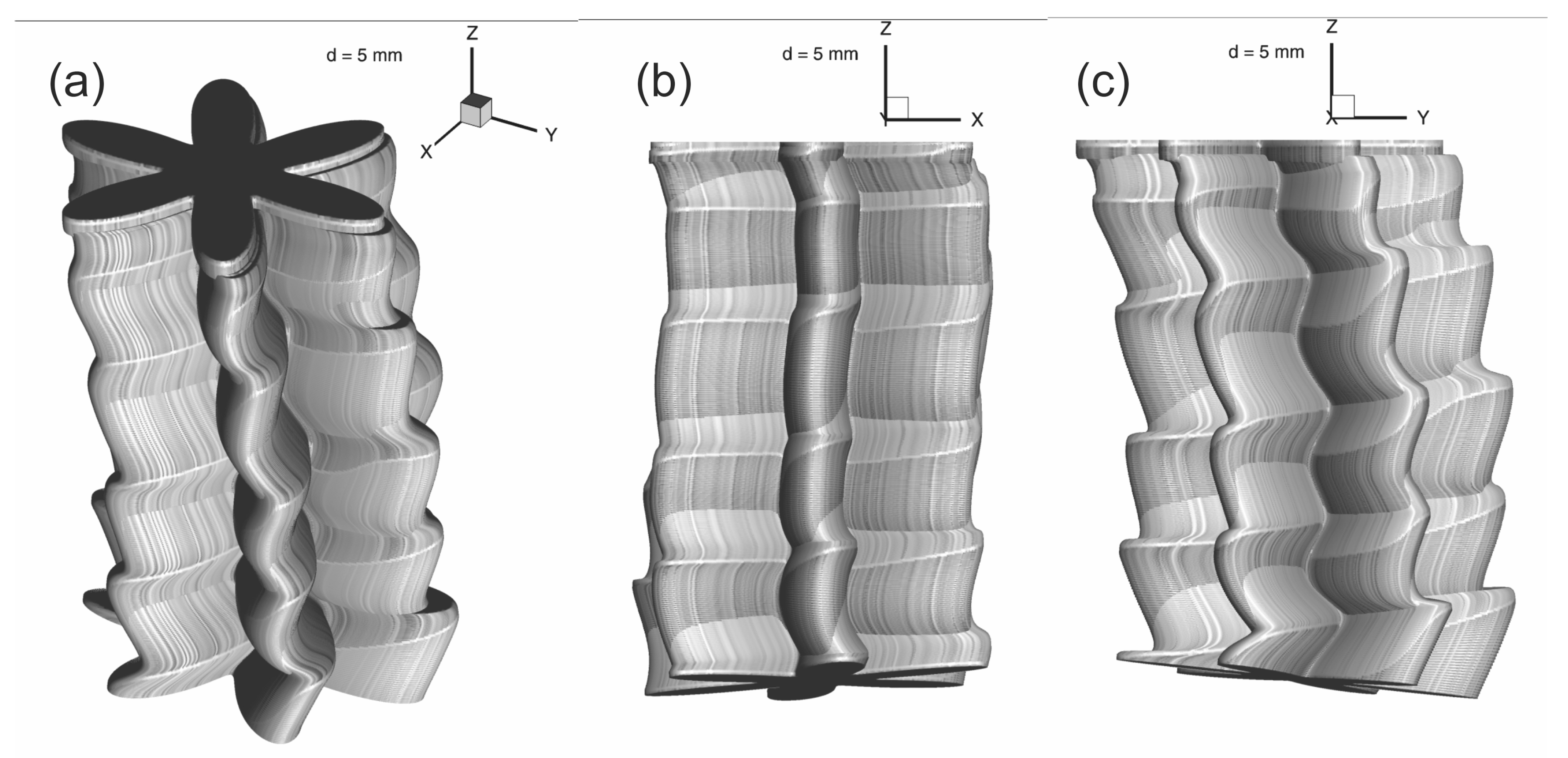
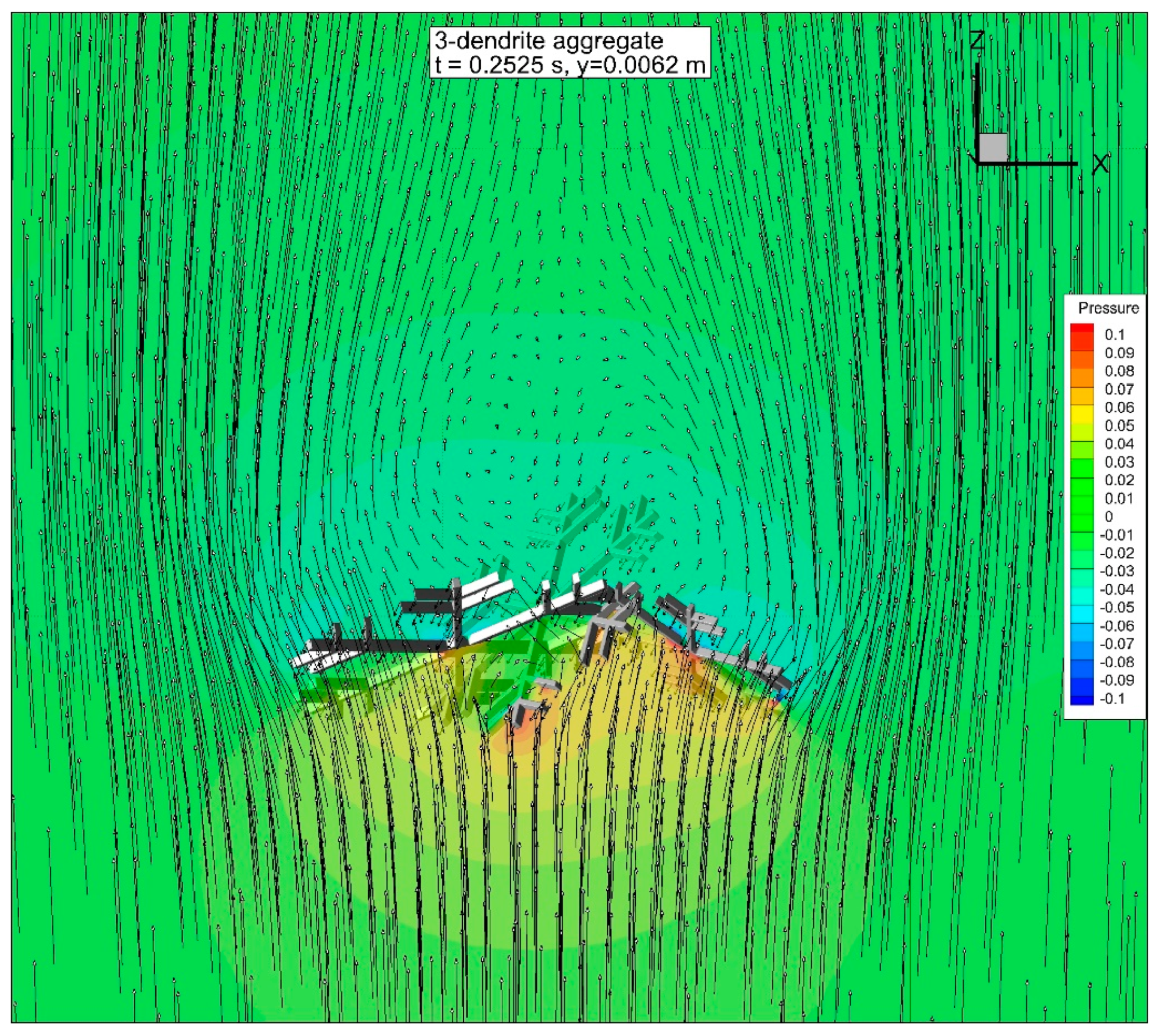
4.2. Flow Fields around Falling Ice Particles
5. Summary and Outlook
Funding
Acknowledgments
Conflicts of Interest
References
- Pruppacher, H.R.; Klett, J.D. Microphysics of Clouds and Precipitation, 2nd ed.; Springer: Dordrecht, The Netherlands, 1997; ISBN 978-0-7923-4211-3. [Google Scholar]
- Wang, P.K. Physics and Dynamics of Clouds and Precipitation; Cambridge University Press: Cambridge, UK, 2013; ISBN 9780511794285. [Google Scholar]
- Beard, K.V.; Pruppacher, H.R. A wind tunnel investigation of collection ker-nels for small water drops in the air. Q. J. R. Meteorol. Soc. 1971, 97, 242–248. [Google Scholar] [CrossRef]
- Beard, K.V.; Pruppacher, H.R. An experimental test of theoret-ically calculated collision efficiencies of cloud drops. J. Geophys. Res. 1968, 73, 6407–6414. [Google Scholar] [CrossRef]
- Hockings, L.M. The collision efficiency of small drops. Q. J. R. Meteorol. Soc. 1959, 85, 44–50. [Google Scholar] [CrossRef]
- Hockings, L.M.; Jonas, P.R. The collision efficiency of small drops. Q. J. R. Meteorol. Soc. 1970, 96, 722–729. [Google Scholar] [CrossRef]
- Jonas, P.R. The collision efficiency of small drops. Q. J. R. Meteorol. Soc. 1972, 98, 681–683. [Google Scholar] [CrossRef]
- Pinsky, M.; Khain, A.; Shapiro, M. Collision Efficiency of Drops in a Wide Range of Reynolds Numbers: Effects of Pressure on Spectrum Evolution. J. Atmos. Sci. 2001, 58, 742–764. [Google Scholar] [CrossRef]
- Cotton, W.R.; Bryan, G.; van den Heever, S.C. (Eds.) Storm and Cloud Dynamics, 2nd ed.; Academic Press: Cambridge, MA, USA, 2010; ISBN 9780120885428. [Google Scholar]
- Blanchard, D.C. The behavior of water drops at terminal velocity in air. Trans. Am. Geophys. Union 1950, 31, 836–842. [Google Scholar] [CrossRef]
- Komabayashi, M.; Gonda, T.; Isono, K. Lifetime of water drops before breaking and size distribution of fragment drops. J. Meteorol. Soc. Jpn. 1964, 42, 330–340. [Google Scholar] [CrossRef]
- Cotton, W.; Gokhale, N.R. Collision, coalescence, and break-up of large water drops in a vertical wind tunnel. J. Geophys. Res. 1967, 72, 4041–4049. [Google Scholar] [CrossRef]
- Spengler, J.D.; Gokhale, N.R. A large, vertical wind tunnel for hydrometeor studies. In Proceedings of the Second National Conference on Weather Modification of the American Meteorological Society, Santa Barbara, CA, USA., 6–9 April 1970; pp. 289–293. [Google Scholar]
- Pruppacher, H.R.; Neiburger, M. The UCLA cloud tunnel. In Proceedings of the International Conference on Cloud Physics, Toronto, ON, Canada, 26–30 August 1968; pp. 389–392. [Google Scholar]
- Beard, K.V.; Pruppacher, H.R. A determination of the terminal velocity and drag of small water drops by means of a wind tunnel. J. Atmos. Sci. 1969, 26, 1066–1072. [Google Scholar] [CrossRef]
- Montgomery, D.N.; Dawson, G.A. Collisional charging of water drops. J. Geophys. Res. 1969, 74, 962–972. [Google Scholar] [CrossRef]
- Gunn, R.; Kinzer, G.D. The Terminal Velocity of Fall for Water Drops in Stagnant Air. J. Meteorol. 1949, 6, 243–248. [Google Scholar] [CrossRef]
- Chowdhury, M.N.; Testik, F.Y.; Hornack, M.C.; Khan, A.A. Free fall of water drops in laboratory rainfall simulations. Atmos. Res. 2016, 168, 158–168. [Google Scholar] [CrossRef]
- Sartor, J.D.; Abbott, C.E. Prediction and measurement of the accelerated motion of water drops in air. J. Appl. Meteorol. 1975, 14, 232–239. [Google Scholar] [CrossRef]
- Diehl, K.; Mitra, S.K.; Szakáll, M.; von Blohn, N.; Borrmann, S.; Pruppacher, H.R. The Mainz vertical wind tunnel facility–A review of 25 years of laboratory experiments on cloud physics and chemistry. In Wind Tunnels: Aerodynamics, Models and Experiments; Pereira, J.D., Ed.; Nova Science Publishers: Hauppauge, NY, USA, 2011; pp. 69–92. [Google Scholar]
- Szakáll, M.; Diehl, K.; Mitra, S.; Borrmann, S.A. Wind tunnel study on the shape, oscillation, and internal circulation of large raindrops with sizes between 2.5 and 7.5 mm. J. Atmos. Sci. 2009, 66, 755–765. [Google Scholar] [CrossRef]
- Szakáll, M.; Mitra, S.; Diehl, K.; Borrmann, S.A. Shapes and oscillations of falling raindrops—A review. Atmos. Res. 2010, 97, 416–425. [Google Scholar] [CrossRef]
- Wang, P.K.; Pruppacher, H.R. Acceleration to Terminal Velocity of Cloud and Rain Drops. J. Appl. Meteorol. 1977, 16, 275–280. [Google Scholar] [CrossRef]
- Abbott, C.E.; Cannon, T.W. A drop generator with electronic control of size, production rate, and charge. Rev. Sci. Instrum. 1972, 43, 1313–1317. [Google Scholar] [CrossRef]
- Hadamard, J.S. Mouvement permanent lent d’une sphere liquide et visqueuse dans un liquide visqueux. CR Hebd. Seances Acad. Sci. 1911, 152, 1735–1738. (In French) [Google Scholar]
- Rybczynsky, W. Über die Fortschreitende Bewegung Einer Flüssigen Kugel in Einem Zähen Medium. Bull. Acad. Sci. Cracovie 1911, 1, 40–46. (In German) [Google Scholar]
- Maxworthy, T. Accurate measurements of sphere drag at low Reynolds numbers. J. Fluid Mech. 1965, 23, 369–372. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Steinberger, E.H. An experimental determination of the drag on a sphere at low Reynolds numbers. J. Appl. Phys. 1968, 39, 4129–4132. [Google Scholar] [CrossRef]
- LeClair, B.P.; Hamielec, A.E.; Pruppacher, H.R.; Hall, W.D. A theoretical and experimental study of the internal circulation in water drops falling at terminal velocity in air. J. Atmos. Sci. 1972, 29, 728–740. [Google Scholar] [CrossRef]
- Hamielec, A.E.; Hoffman, T.W.; Ross, L.L. Numerical solution to the Navier-Stokes equation of motion for flow past spheres. AIChE J. 1967, 13, 213–219. [Google Scholar] [CrossRef]
- Taneda, S. Experimental investigation of the wake behind a sphere at low Reynolds numbers. J. Phys. Soc. Jpn. 1956, 11, 1101–1108. [Google Scholar] [CrossRef]
- Diehl, K. Eine Theoretische und Experimentelle Untersuchung Uber Die Zirkulation in Wolkentropfen und Regentropfen. Diploma Thesis, Institute of Atmospheric Physics, University of Mainz, Mainz, Germany, 1989. [Google Scholar]
- Magarvey, R.H.; Bishop, R.L. Wakes in liquid-liquid systems. Phys. Fluids 1961, 4, 800. [Google Scholar] [CrossRef]
- Ren, W.; Reutzsch, J.; Weigand, B. Direct numerical simulation of water drops in turbulent flow. Fluids 2020, 5, 158. [Google Scholar] [CrossRef]
- Eisenschmidt, K.; Ertl, M.; Gomaa, H.; Kieffer-Roth, C.; Meister, C.; Rauschenberger, P.; Reitzle, M.; Schlottke, K.; Weigand, B. Direct numerical simulations for multiphase flows: An overview of the multiphase code FS3D. Appl. Math. Comput. 2016, 272, 508–517. [Google Scholar] [CrossRef]
- Hirt, C.; Nichols, B. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Rider, W.J.; Kothe, D.B. Reconstructing volume tracking. J. Comput. Phys. 1998, 141, 112–152. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Beard, K. A wind tunnel investigation of the internal circulation and shape of water drops falling at terminal velocity in air. Q. J. R. Meteorol. Soc. 1970, 96, 247–256. [Google Scholar] [CrossRef]
- Beard, K.V. Terminal velocity and shape of cloud and precipitation drops aloft. J. Atmos. Sci. 1976, 33, 851–864. [Google Scholar] [CrossRef]
- Tokay, A.; Beard, K.V. A Field Study of Raindrop Oscillations. Part I: Observation of Size Spectra and Evaluation of Oscillation Causes. J. Appl. Meteorol. 1996, 35, 1671–1687. [Google Scholar] [CrossRef]
- Nakaya, U.; Terada, T. Simultaneous Observations of the Mass, Falling Velocity and Form of Individual Snow Crystals. J. Fac. Sci. Hokkaido Univ. 1935, 1, 191–200. [Google Scholar]
- Magono, C. On the fall velocity of snowflakes. J. Meteorol. 1951, 8, 199–200. [Google Scholar] [CrossRef]
- Magono, C. On the growth of snowflakes and graupel. Sci. Rep. Yokohama Nat. Univ. Sect. I 1953, 2, 18–40. [Google Scholar]
- Magono, C. On the falling velocity of solid precipitation elements. Sci. Rep. Yokohama Nat. Univ. Sect. I 1954, 3, 33–40. [Google Scholar]
- Litvinov, I.V. Determination of falling velocity of snow particles. Izv. Akad. Nauk SSR Ser. Geofiz. 1956, 7, 853–856. [Google Scholar]
- Bashkirova, T.A.; Pershina, T.A. On the mass of snow crystals and their fall velocity. Tr. GI. Geofiz. Observ. 1964, 165, 83–100. [Google Scholar]
- Jayaweera, K.O.L.F.; Mason, B.J. The behavior of freely falling cylinders and cones in a viscous fluid. J. Fluid Mech. 1965, 22, 709–720. [Google Scholar] [CrossRef]
- Jayaweera, K.O.L.F.; Cottis, R.E. Fall velocities of plate-like and columnar ice crystals. Q. J. R. Meteorol. Soc. 1969, 95, 203–209. [Google Scholar] [CrossRef]
- Magono, C.; Nakamura, T. Aerodynamic studies of falling snowflakes. J. Meteorol. Soc. Jpn. 1965, 43, 139–147. [Google Scholar] [CrossRef]
- Podzimek, J. Movement of ice particles in the atmosphere. In Proceedings of the International Conference on Cloud Physics, Tokyo and Sapporo, Japan, 24 May–1 June 1965; pp. 224–230. [Google Scholar]
- Podzimek, J. Aerodynamic conditions of ice crystal aggregation. In Proceedings of the International Conference on Cloud Physics, Toronto, ON, Canada, 26–30 August 1968; pp. 295–299. [Google Scholar]
- Fukuta, N. Experimental studies on the growth of ice crystals. J. Atmos. Sci. 1969, 26, 522–531. [Google Scholar] [CrossRef]
- Brown, S.R. Terminal Velocities of Ice Crystals. Master’s Thesis, Dept. of Atmospheric Science, Colorado State University, Fort Collins, CO, USA, 1970. [Google Scholar]
- Jiusto, J.E.; Bosworth, G.E. Fall velocity of snowflakes. J. Appl. Meteorol. 1971, 10, 1352–1354. [Google Scholar] [CrossRef]
- Heymsfield, A. Ice crystal terminal velocities. J. Atmos. Sci. 1972, 29, 1348–1357. [Google Scholar] [CrossRef]
- Jayaweera, K.O.L.F.; Ryan, B.F. Terminal velocities of ice crystals. Q. J. R. Meteorol. Soc. 1972, 98, 193–197. [Google Scholar] [CrossRef]
- Zikmunda, J.; Vali, G. Fall patterns and fall velocities of rimed ice crystals. J. Atmos. Sci. 1972, 29, 1334–1347. [Google Scholar] [CrossRef][Green Version]
- Wang, P.K. Motions of Ice Hydrometeors in the Atmosphere-Numerical Studies and Implications; Springer-Nature: Singapore, 2021; ISBN 978-981-33-4430-3. [Google Scholar]
- Kajikawa, M. Measurement of falling velocity of individual snow crystals. J. Meteorol. Soc. Jpn. 1972, 50, 577–584. [Google Scholar] [CrossRef][Green Version]
- Kajikawa, M. Laboratory measurement of falling velocity of individual ice crystals. J. Meteorol. Soc. Jpn. 1973, 51, 263–272. [Google Scholar] [CrossRef]
- Kajikawa, M. Experimental formula of falling velocity of snow crystals. J. Meteorol. Soc. Jpn. 1975, 53, 267–275. [Google Scholar] [CrossRef][Green Version]
- Kajikawa, M. Measurement of falling velocity of individual graupel particles. J. Meteorol. Soc. Jpn. 1975, 53, 476–481. [Google Scholar] [CrossRef][Green Version]
- Kajikawa, M. Observation of the falling motion of early snowflakes Part I. Relationship between the free-fall pattern and the number and shape of component snow crystals. J. Meteorol. Soc. Jpn. 1982, 60, 797–803. [Google Scholar] [CrossRef]
- Kajikawa, M. Observation of the falling motion of early snowflakes Part II. On the variation of falling velocity. J. Meteorol. Soc. Jpn. 1989, 67, 731–737. [Google Scholar] [CrossRef]
- Kajikawa, M. Observation of the falling motion of plate-like snow crystals Part I: The free-fall patterns and velocity variations of unrimed crystals. J. Meteorol. Soc. Jpn. 1992, 70, 1–9. [Google Scholar] [CrossRef]
- Westbrook, C.D.; Sephton, E.K. Using 3-D-printed analogues to investigate the fall speeds and orientations of complex ice particles. Geophys. Res. Lett. 2017, 44, 7994–8001. [Google Scholar] [CrossRef]
- List, R.; Schemenauer, R. Free-fall behaviour of planar snow crystals, conical graupel and small hail. J. Atmos. Sci. 1971, 28, 110–115. [Google Scholar] [CrossRef]
- List, R. Kennzeichen atmosphärischer Eispartikeln. I. Teil. Graupeln als Wachstumszentren von Hagelkörner. Z. Angew. Math. Phys. 1958, 9A, 180–192. [Google Scholar] [CrossRef]
- Pflaum, J.C.; Martin, J.J.; Pruppacher, H.R. A wind tunnel investigation of the hydrodynamic behaviour of growing, freely falling graupel. Q. J. R. Meteorol. Soc. 1978, 104, 179–187. [Google Scholar] [CrossRef]
- List, R. Der Hagelversuchskanal. Z. Angew. Math. Phys. 1959, 10, 381–415. [Google Scholar] [CrossRef]
- List, R.; Rentsch, U.W.; Byram, A.C.; Lozowski, E.P. On the aerodynamics of spheroidal hailstone models. J. Atmos. Sci. 1973, 30, 653–661. [Google Scholar] [CrossRef]
- Lesins, G.B.; List, R. Sponginess and drop shedding of gyrating hailstones in a pressure-controlled wind tunnel. J. Atmos. Sci. 1986, 43, 2813–2825. [Google Scholar] [CrossRef][Green Version]
- Knight, C.A.; Knight, N.C. The falling behavior of hailstones. J. Atmos. Sci. 1970, 27, 672–681. [Google Scholar] [CrossRef][Green Version]
- Heymsfield, A.J.; Westbrook, C.D. Advances in the estimation of ice particle fall speeds using laboratory and field measurements. J. Atmos. Sci. 2010, 67, 2469–2482. [Google Scholar] [CrossRef]
- Bürgesser, R.E.; Ávila, E.E.; Castellano, N.E. Laboratory measurements of sedimentation velocity of columnar ice crystals. Q. J. R. Meteorol. Soc. 2016, 142, 1713–1720. [Google Scholar] [CrossRef]
- Garrett, T.J.; Fallgater, C.; Shkurko, K.; Howlett, D. Fall speed measurement and high-resolution multi-angle photography of hydrometeors in free fall. Atmos. Meas. Tech. 2012, 5, 2625–2633. [Google Scholar] [CrossRef]
- Thorn, A. The flow past circular cylinders at low speeds. Proc. Roy. Soc. 1933, A141, 651–669. [Google Scholar]
- Dennis, S.C.R.; Chang, G.Z. Numerical integration of the Navier–Stokes equations for two-dimensional flow. Phys. Fluid 1969, 12, II-88–II-93. [Google Scholar] [CrossRef]
- Hamielec, A.C.; Raal, J.D. Numerical studies of viscous flow around a circular cylinder. Phys. Fluids 1969, 12, 11–22. [Google Scholar] [CrossRef]
- Takami, H.; Keller, H.B. Steady two-dimensional viscous flow of an incompressible fluid past a circular cylinder. Phys. Fluids 1969, 12, 51–56. [Google Scholar] [CrossRef]
- Schlamp, R.J.; Pruppacher, H.R.; Hamielec, A.E. A Numerical Investigation of the Efficiency with which Simple Columnar Ice Crystals Collide with Supercooled Water Drops. J. Atmos. Sci. 1975, 32, 2330–2337. [Google Scholar] [CrossRef]
- Rimon, Y.; Lugt, H.J. Laminar flow past oblate spheroids of various thicknesses. Phys. Fluids 1969, 12, 2465–2472. [Google Scholar] [CrossRef]
- Masliyah, J.H.; Epstein, N. Numerical study of steady flow past spheroids. J. Fluid Mech. 1970, 44, 493–512. [Google Scholar] [CrossRef]
- Pitter, R.L.; Pruppacher, H.R.; Hamielec, A.E. A Numerical Study of Viscous Flow Past a Thin Oblate Spheroid at Low and Intermediate Reynolds Numbers. J. Atmos. Sci. 1973, 30, 125–134. [Google Scholar] [CrossRef]
- Pitter, R.L.; Pruppacher, H.R. A numerical investigation of collision efficiencies of simple ice plates colliding with supercooled drops. J. Atmos. Sci. 1974, 31, 551–559. [Google Scholar] [CrossRef][Green Version]
- Ji, W.; Wang, P.K. Numerical simulation of three-dimensional unsteady viscous flow past fixed hexagonal ice crystals in the air—Preliminary results. Atmos. Res. 1990, 25, 539–557. [Google Scholar] [CrossRef]
- Ji, W.; Wang, P.K. Numerical simulation of three-dimensional unsteady viscous flow past finite cylinders in an unbounded fluid at low intermediate Reynolds numbers. Theor. Comput. Fluid Dyn. 1991, 3, 43–59. [Google Scholar]
- Wang, P.K.; Ji, W. Numerical Simulation of Three-Dimensional Unsteady Flow past Ice Crystals. J. Atmos. Sci. 1997, 54, 2261–2274. [Google Scholar] [CrossRef]
- Kubicek, A.; Wang, P.K. A numerical study of the flow fields around a typical conical graupel falling at various inclination angles. Atmos. Res. 2012, 118, 15–26. [Google Scholar] [CrossRef]
- Wang, P.K.; Kubicek, A. Flow fields of graupel falling in air. Atmos. Res. 2013, 124, 158–169. [Google Scholar] [CrossRef]
- Wang, P.K. Mathematical Description of the Shape of Conical Hydrometeors. J. Atmos. Sci. 1982, 39, 2615–2622. [Google Scholar] [CrossRef]
- Hashino, T.; Chiruta, M.; Polzin, D.; Kubicek, A.; Wang, P.K. Numerical simulation of the flow fields around falling ice crystals with inclined orientation and the hydrodynamic torque. Atmos. Res. 2014, 150, 79–96. [Google Scholar] [CrossRef]
- Cheng, K.Y.; Wang, P.K.; Hashino, T. A numerical study on the attitudes and aerodynamics of freely falling hexagonal ice plates. J. Atmos. Sci. 2015, 72, 3685–3698. [Google Scholar] [CrossRef]
- Hashino, T.; Cheng, K.Y.; Chueh, C.C.; Wang, P.K. Numerical study of motion and stability of falling columnar crystals. J. Atmos. Sci. 2016, 73, 1923–1942. [Google Scholar] [CrossRef]
- Chueh, C.C.; Wang, P.K.; Hashino, T. A preliminary numerical study on the time-varying fall attitudes and aerodynamics of freely falling conical graupel particles. Atmos. Res. 2017, 183, 58–72. [Google Scholar] [CrossRef]
- Chueh, C.C.; Wang, P.K.; Hashino, T. Numerical Study of Motion of Falling Conical Graupel. Atmos. Res. 2018, 199, 82–92. [Google Scholar] [CrossRef]
- Takahashi, T. Riming Electrification as a Charge Generation Mechanism in Thunderstorms. J. Atmos. Sci. 1978, 35, 1536–1548. [Google Scholar] [CrossRef]
- Cui, Z.; Davies, S.; Carslaw, K.S.; Blyth, A.M. The response of precipitation to aerosol through riming and melting in deep convective clouds. Atmos. Chem. Phys. 2011, 11, 3495–3510. [Google Scholar] [CrossRef]
- Johnson, J.S.; Cui, Z.; Lee, L.A.; Gosling, J.P.; Blyth, A.M.; Carslaw, K.S. Evaluating uncertainty in convective cloud microphysics using statistical emulation. J. Adv. Model. Earth Syst. 2015, 7, 162–187. [Google Scholar] [CrossRef]
- Cheng, K.Y.; Wang, P.K. A numerical study of the flow fields around falling hails. Atmos. Res. 2013, 132–133, 253–263. [Google Scholar] [CrossRef]
- Grover, S.N.; Pruppacher, H.R.; Hamielec, A.E. A Numerical Determination of the Efficiency with Which Spherical Aerosol Particles Collide with Spherical Water Drops Due to Inertial Impaction and Phoretic and Electrical Forces. J. Atmos. Sci. 1977, 34, 1655–1663. [Google Scholar] [CrossRef]
- Wang, P.K.; Chueh, C.C.; Wang, C.K. A Numerical Study of Flow Fields of Lobed Hailstones Falling in Air. Atmos. Res. 2015, 160, 1–14. [Google Scholar] [CrossRef]
- Nettesheim, J.; Wang, P.K. A Numerical Study on the Aerodynamics of Freely Falling Planar Ice Crystals. J. Atmos. Sci. 2018, 75, 2849–2865. [Google Scholar] [CrossRef]
- Rasmussen, R.M.; Levizzani, V.; Pruppacher, H.R. A wind tunnel and theoretical study of the melting behavior of atmospheric ice particles. III. Experiment and theory for spherical ice particles of radius > 500 μm. J. Atmos. Sci. 1984, 41, 381–388. [Google Scholar]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, P.K. Theoretical Studies on the Motions of Cloud and Precipitation Particles—A Review. Meteorology 2022, 1, 288-310. https://doi.org/10.3390/meteorology1030019
Wang PK. Theoretical Studies on the Motions of Cloud and Precipitation Particles—A Review. Meteorology. 2022; 1(3):288-310. https://doi.org/10.3390/meteorology1030019
Chicago/Turabian StyleWang, Pao K. 2022. "Theoretical Studies on the Motions of Cloud and Precipitation Particles—A Review" Meteorology 1, no. 3: 288-310. https://doi.org/10.3390/meteorology1030019
APA StyleWang, P. K. (2022). Theoretical Studies on the Motions of Cloud and Precipitation Particles—A Review. Meteorology, 1(3), 288-310. https://doi.org/10.3390/meteorology1030019





