Testing Cosmic Acceleration from the Late-Time Universe
Abstract
1. Introduction
2. Theoretical Background
2.1. Standard Cosmological Model
2.2. Flat Constant wCDM Model
2.3. CPL Parametrization
3. Data and Methodology
| Observable | Measurement | Error | Year | Dataset Survey | Reference | |
|---|---|---|---|---|---|---|
| 0.106 | 0.336 | 0.015 | 2011 | 6dFGS BAO | [50] | |
| 0.15 | 4.47 | 0.17 | 2021 | SDSS Main Galaxy Sample | [53] | |
| 0.31 | 6.29 | 0.14 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.36 | 7.09 | 0.16 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.38 | 25.00 | 0.76 | 2021 | SDSS BOSS Galaxy Sample | [54] | |
| 0.40 | 7.70 | 0.16 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.44 | 8.20 | 0.13 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.48 | 8.64 | 0.11 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.51 | 13.36 | 0.21 | 2021 | SDSS BOSS Galaxy Sample | [53] | |
| 0.52 | 8.90 | 0.12 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.56 | 9.16 | 0.14 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.59 | 9.45 | 0.17 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.64 | 9.62 | 0.22 | 2017 | SDSS-III BOSS-DR12 | [54] | |
| 0.697 | 1529 | 73 | 2020 | DECaLS DR8 Footprint LRG | [49] | |
| 0.698 | 19.77 | 0.47 | 2020 | eBOSS DR16 LRG Sample | [44] | |
| 0.698 | 17.65 | 0.30 | 2020 | eBOSS DR16 LRG Sample | [44] | |
| 0.70 | 17.96 | 0.51 | 2021 | eBOSS DR16 ELG Sample | [55] | |
| 0.835 | 18.92 | 0.51 | 2022 | Dark Energy Survey Year 3 | [48] | |
| 0.845 | 20.91 | 2.86 | 2021 | eBOSS DR16 ELG Sample | [55] | |
| 0.874 | 1680 | 109 | 2020 | DECaLS DR8 Footprint LRG | [49] | |
| 1.48 | 13.23 | 0.47 | 2021 | eBOSS DR16 Quasar Sample | [46] | |
| 1.48 | 30.21 | 0.79 | 2021 | eBOSS DR16 Quasar Sample | [46] | |
| 2.33 | 8.99 | 0.19 | 2020 | eBOSS DR16 Ly-Quasar | [47] | |
| 2.33 | 37.5 | 1.1 | 2020 | eBOSS DR16 Ly-Quasar | [47] |
4. Analysis and Results
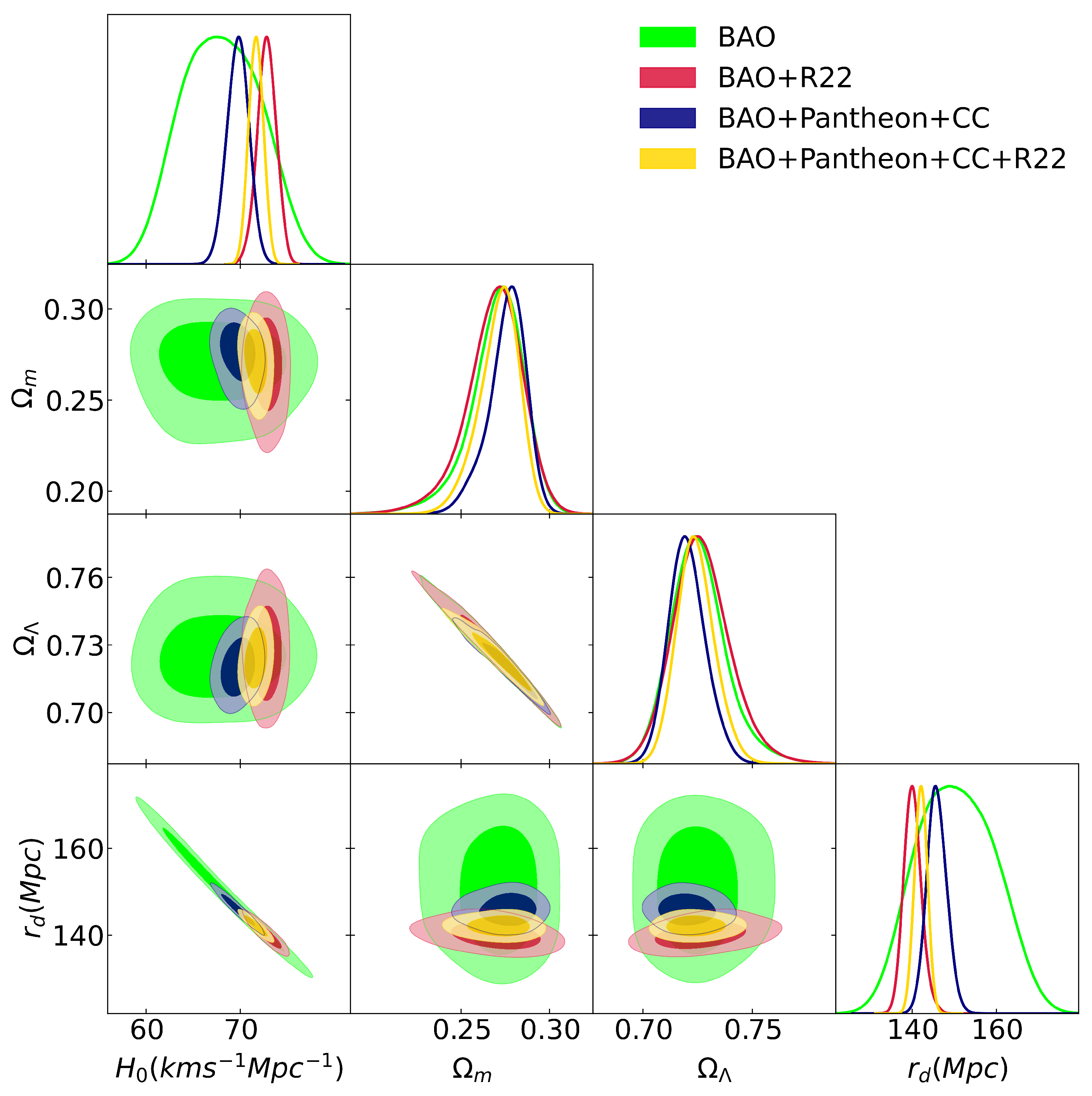
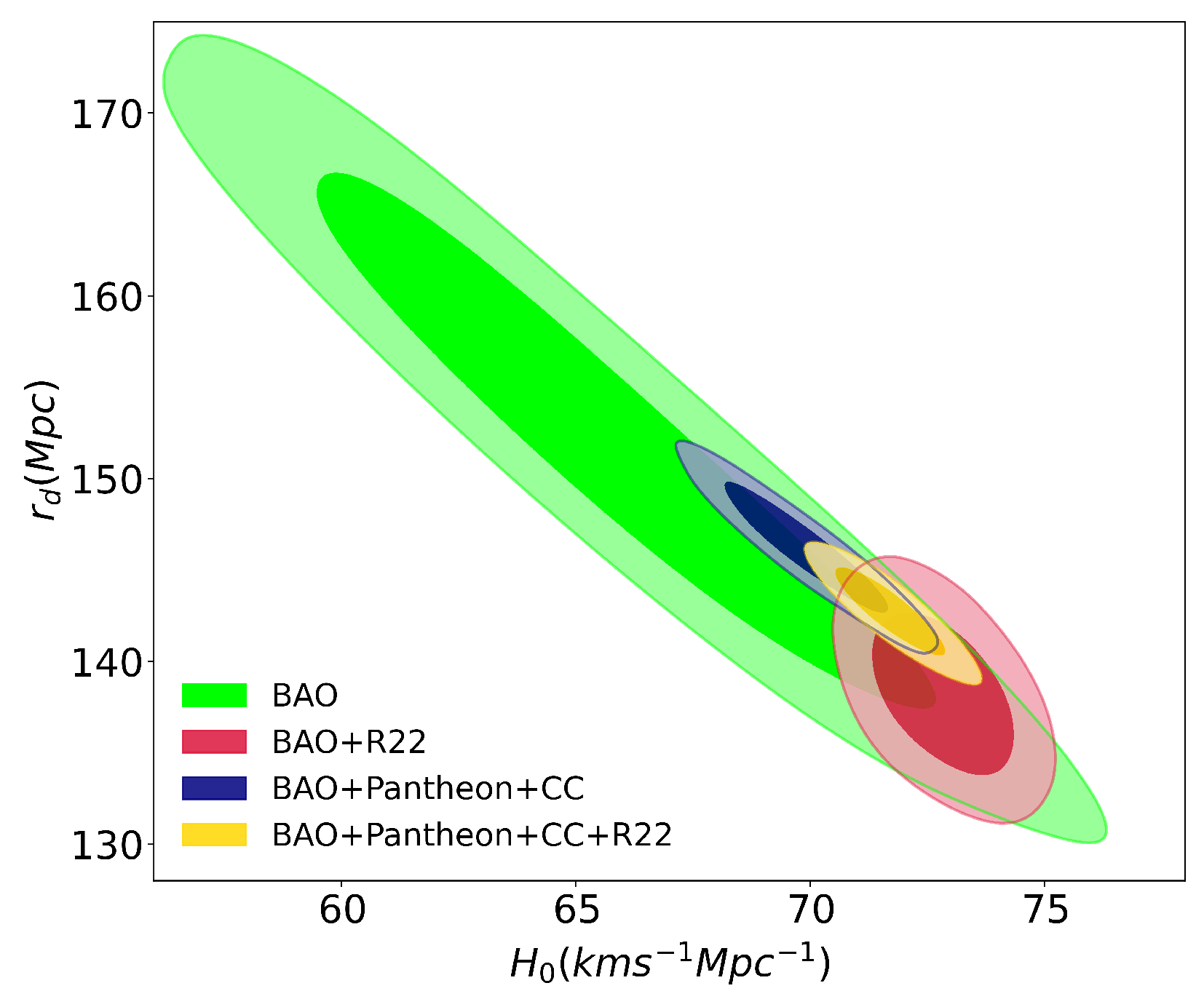
4.1. Standard Cosmological Model
4.2. Models beyond Standard Model
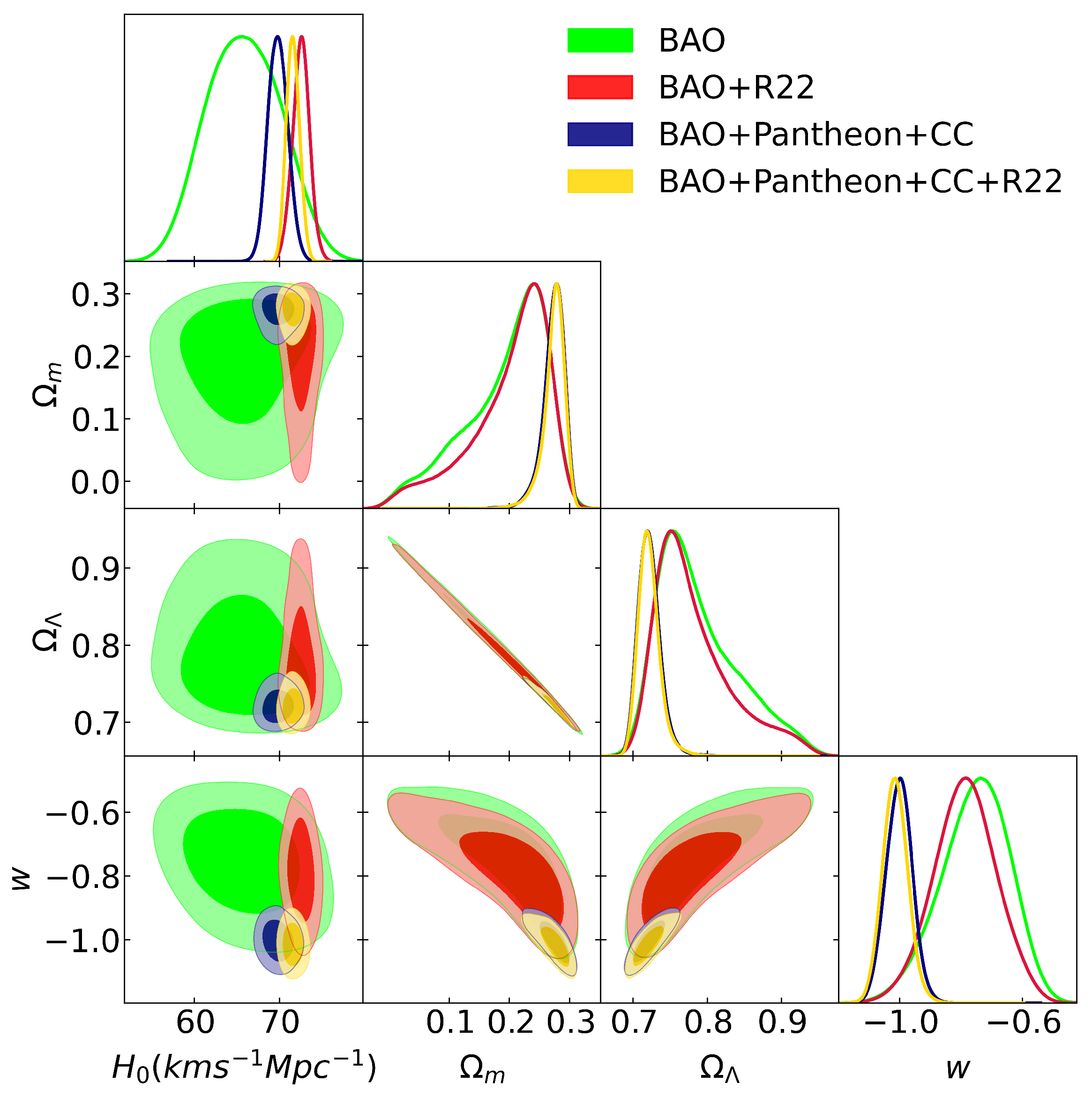
4.2.1. wCDM Model
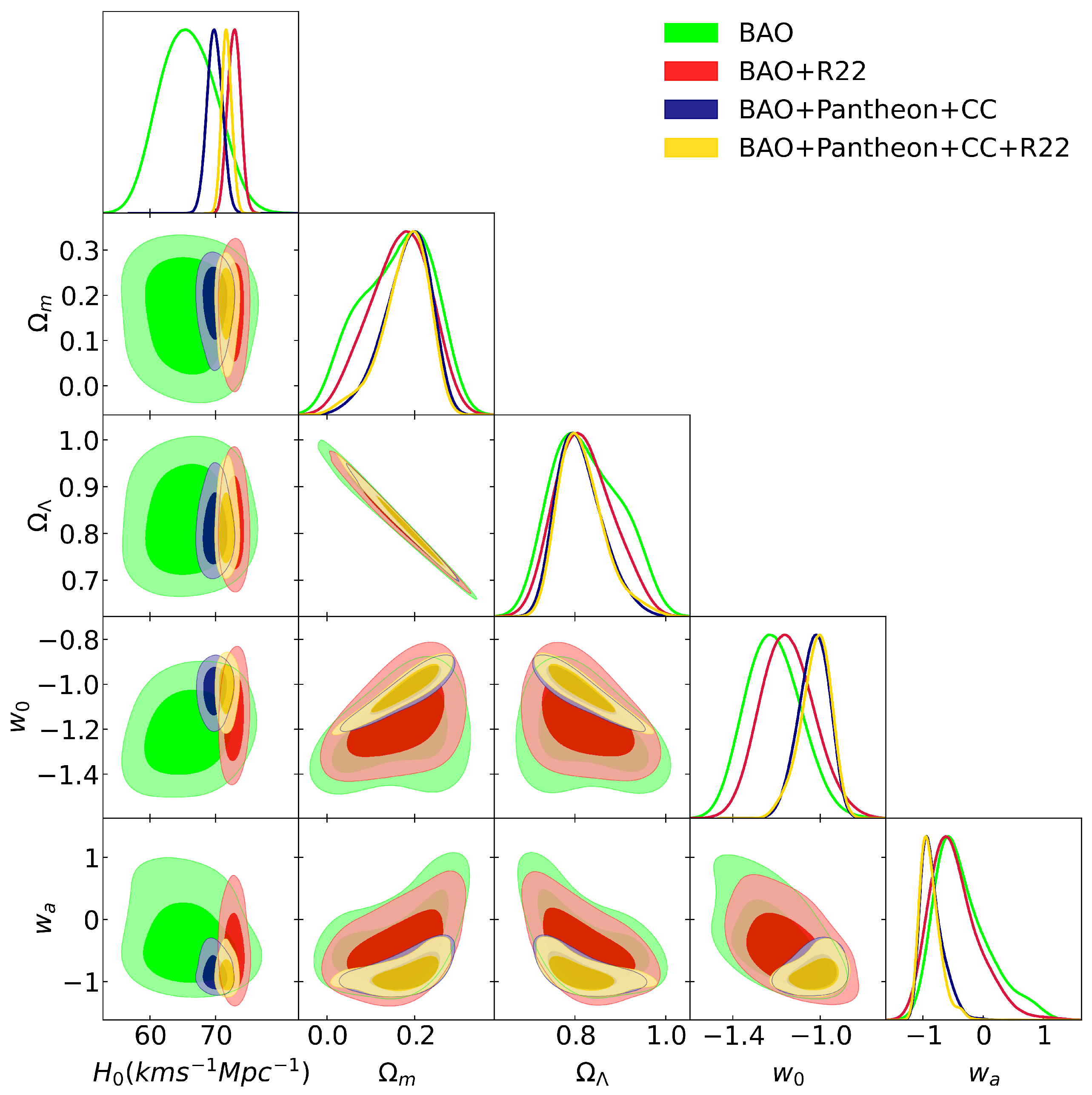
4.2.2. CDM Model
5. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Planck Collaboration. Planck 2018 Results—VI. Cosmological Parameters. Astron. Astrophys. 2020, A6, 641. [Google Scholar]
- Bennett, C.L.; Larson, D.; Weil, J.L.; Jarosik, N.; Hinshaw, G.; Odegard, N.; Smith, K.M.; Hill, R.S.; Gold, B.; Halpern, M.; et al. Nine-year Wilkinson microwave anisotropy probe (WMAP) observations: Final maps and results. Astrophys. J. Suppl. Ser. 2013, 208, 20. [Google Scholar] [CrossRef]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gillil, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational Evidence from Supernovae for an Accelerating Universe and a Cosmological Constant. Astron. J. 1998, 116, 1009. [Google Scholar] [CrossRef]
- Riess, A.G.; Macri, L.; Casertano, S.; Lampeitl, H.; Ferguson, H.C.; Filippenko, A.V.; Jha, S.W.; Li, W.; Chornock, R. A 3% solution: Determination of the Hubble constant with the Hubble space telescope and wide field camera 3. Astron. J. 2011, 730, 119. [Google Scholar] [CrossRef]
- Riess, A.G.; Macri, L.M.; Hoffmann, S.L.; Scolnic, D.; Casertano, S.; Filippenko, A.V.; Tucker, B.E.; Reid, M.J.; Jones, D.O.; Silverman, J.M.; et al. A 2.4% determination of the local value of the Hubble constant. Astron. J. 2016, 826, 56. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.M.; Scolnic, D. Large Magellanic Cloud Cepheid Standards Provide a 1% Foundation for the Determination of the Hubble Constant and Stronger Evidence for Physics beyond ΛCDM. Astron. J. 2019, 876, 55. [Google Scholar] [CrossRef]
- Riess, A.G.; Yuan, W.; Macri, L.M.; Scolnic, D.; Brout, D.; Casertano, S.; Jones, D.O.; Murakami, Y.; Anand, G.S.; Breuval, L.; et al. A Comprehensive Measurement of the Local Value of the Hubble Constant with 1 km/s/Mpc Uncertainty from the Hubble Space Telescope and the SH0ES Team. Astrophys. J. Lett. 2022, 934, L7. [Google Scholar] [CrossRef]
- Huang, Q.G.; Wang, K. How the dark energy can reconcile Planck with local determination of the Hubble constant. Eur. Phys. J. 2016, 76, 506. [Google Scholar] [CrossRef]
- Di Valentino, E.; Melchiorri, A.; Silk, J. Reconciling Planck with the local value of H0 in extended parameter space. Phys. Lett. B 2016, 761, 242–246. [Google Scholar] [CrossRef]
- Xu, L.; Huang, Q.G. Detecting the neutrinos mass hierarchy from cosmological data. Sci. China Phys. Mech. Astron. 2018, 61, 039521. [Google Scholar] [CrossRef]
- Yang, W.; Pan, S.; Di Valentino, E.; Saridakis, E.N.; Chakraborty, S. Observational constraints on one-parameter dynamical dark-energy parametrizations and the H0 tension. Phys. Rev. D 2019, 99, 043543. [Google Scholar] [CrossRef]
- Poulin, V.; Smith, T.L.; Karwal, T.; Kamionkowsk, M. Early Dark Energy can Resolve the Hubble Tension. Phys. Rev. Lett. 2019, 122, 221301. [Google Scholar] [CrossRef] [PubMed]
- Vagnozzi, S. New physics in light of the H0 tension: An alternative view. Phys. Rev. D 2020, 102, 023518. [Google Scholar] [CrossRef]
- Liu, M.; Huang, Z.; Luo, X.; Miao, H.; Singh, N.K.; Huang, L. Can non-standard recombination resolve the Hubble tension? Sci. China Phys. Mech. Astron. 2020, 63, 290405. [Google Scholar] [CrossRef]
- Ding, Q.; Nakama, T.; Wang, Y. A gigaparsec-scale local void and the Hubble tension. Sci. China Phys. Mech. Astron. 2020, 63, 290403. [Google Scholar] [CrossRef]
- Ryan, J.; Chen, Y.; Ratra, B. Baryon acoustic oscillation, Hubble parameter, and angular size measurement constraints on the Hubble constant, dark energy dynamics, and spatial curvature. Mon. Not. R. Astron. Soc. 2019, 488, 3844–3856. [Google Scholar] [CrossRef]
- Zhao, G.B.; Raveri, M.; Pogosian, L.; Wang, Y.; Crittenden, R.G.; Handley, W.J.; Percival, W.J.; Beutler, F.; Brinkmann, J.; Chuang, C.; et al. Dynamical dark energy in light of the latest observations. Nat. Astron. 2017, 1, 627–632. [Google Scholar] [CrossRef]
- Li, X.; Shafieloo, A. A Simple Phenomenological Emergent Dark Energy Model can Resolve the Hubble Tension. Astrophys. J. Lett. 2019, 883, L3. [Google Scholar] [CrossRef]
- Di Valentino, E. Investigating Cosmic Discordance. Astrophys. J. Lett. 2021, 908, L9. [Google Scholar] [CrossRef]
- Haitao, M.; Zhiqi, H. The H0 Tension in Non-flat QCDM Cosmology. Astron. J. 2018, 868, 20. [Google Scholar]
- Millon, M.; Galan, A.; Courbin, F.; Treu, T.; Suyu, S.H.; Ding, X.; Birrer, S.; Chen, G.C.-F.; Shajib, A.J.; Sluse, D.; et al. An exploration of systematic uncertainties in the inference of H0 from time-delay cosmography. Astron. Astrophys. 2020, 639, A101. [Google Scholar] [CrossRef]
- Wong, K.C.; Suyu, S.H.; Chen, G.C.-F.; Rusu, C.E.; Millon, M.; Sluse, D.; Bonvin, V.; Fassnacht, C.D.; Taubenberger, S.; Auger, M.W.; et al. H0LiCOW—XIII. A 2.4 percent measurement of H0 from lensed quasars: 5.3σ tension between early- and late-Universe probes. Mon. Not. R. Astron. Soc. 2020, 498, 1420–1439. [Google Scholar] [CrossRef]
- Mooley, K.P.; Deller, A.T.; Gottlieb, O.; Nakar, E.; Hallinan, G.; Bourke, S.; Frail, D.A.; Horesh, A.; Corsi, A.; Hotokezaka, K. Superluminal motion of a relativistic jet in the neutron-star merger GW170817. Nature 2018, 561, 355–359. [Google Scholar] [CrossRef]
- The LIGO Scientific Collaboration and The Virgo Collaboration; The 1M2H Collaboration; The Dark Energy Camera GW-EM Collaboration and the DES Collaboration; The DLT40 Collaboration; The Las Cumbres Observatory Collaboration; The VINROUGE Collaboration; The MASTER Collaboration. A gravitational-wave standard siren measurement of the Hubble constant. Nature 2017, 551, 85–88. [Google Scholar] [CrossRef] [PubMed]
- Hotokezaka, K.; Nakar, E.; Gottlieb, O.; Nissanke, S.; Masuda, K.; Hallinan, G.; Mooley, K.P.; Deller, A.T. A Hubble constant measurement from the superluminal motion of the jet in GW170817. Nat. Astron. 2019, 3, 940–944. [Google Scholar] [CrossRef]
- Wu, Q.; Zhang, G.-Q.; Wang, F.-Y. An 8 percent determination of the Hubble constant from localized fast radio bursts. Mon. Not. R. Astron. Soc. Lett. 2022, 515, L1–L5. [Google Scholar] [CrossRef]
- James, C.W.; Ghosh, E.M.; Prochaska, J.X.; Bannister, K.W.; Bhandari, S.; Day, C.K.; Deller, A.T.; Glowacki, M.; Gordon, A.C.; Heintz, K.E.; et al. A measurement of Hubble’s Constant using Fast Radio Bursts. Mon. Not. R. Astron. Soc. 2022, 516, 4862–4881. [Google Scholar] [CrossRef]
- Pesce, D.W.; Braatz, J.A.; Reid, M.J.; Riess, A.G.; Scolnic, D.; Condon, J.J.; Gao, F.; Henkel, C.; Impellizzeri, C.M.V.; Kuo, C.Y.; et al. The Megamaser Cosmology Project. XIII. Combined Hubble Constant Constraints. Astrophys. J. Lett. 2020, 891, L1. [Google Scholar] [CrossRef]
- Reid, J.; Pesce, D.W.; Riess, A.G. An Improved Distance to NGC 4258 and Its Implications for the Hubble Constant. Astrophys. J. Lett. 2019, 886, L27. [Google Scholar] [CrossRef]
- Kuo, C.Y.; Braatz, J.A.; Lo, K.Y.; Reid, M.J.; Suyu, S.H.; Pesce, D.W.; Condon, J.J.; Henkel, C.; Impellizzeri, C.M.V. The Megamaser Cosmology Project. VI. Observations of NGC 6323. Astron. J. 2015, 800, 26. [Google Scholar] [CrossRef]
- Freedman, W.L.; Madore, B.F.; Hatt, D.; Hoyt, T.J.; Jang, I.S.; Beaton, R.L.; Burns, C.R.; Lee, M.G.; Monson, A.J.; Neeley, J.R.; et al. The Carnegie-Chicago Hubble Program. VIII. An Independent Determination of the Hubble Constant Based on the Tip of the Red Giant Branch. Astron. J. 2019, 882, 34. [Google Scholar] [CrossRef]
- Freedman, W.L.; Madore, B.F.; Hoyt, T.; Jang, I.S.; Beaton, R.; Lee, M.G.; Monson, A.; Neeley, J.; Jeffrey, R. Calibration of the Tip of the Red Giant Branch. Astron. J. 2020, 891, 57. [Google Scholar] [CrossRef]
- Freedman, W.L. Measurements of the Hubble Constant: Tensions in Perspective. Astron. J. 2021, 919, 16. [Google Scholar] [CrossRef]
- Addison, G.E.; Watts, D.J.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Weil, J.L. Elucidating ΛCDM: Impact of Baryon Acoustic Oscillation Measurements on the Hubble Constant Discrepancy. Astron. J. 2021, 853, 119. [Google Scholar] [CrossRef]
- Moresco, M.; Amati, L.; Amendola, L.; Birrer, S.; Blakeslee, J.P.; Cantiello, M.; Cimatti, A.; Darling, J.; Valle, M.D.; Fishbach, M.; et al. Unveiling the Universe with emerging cosmological probes. Living Rev. Relativ. 2022, 25, 6. [Google Scholar] [CrossRef]
- Suyu, S.H.; Bonvin, V.; Courbin, F.; Fassnacht, C.D.; Rusu, C.E.; Sluse, D.; Treu, T.; Wong, K.C.; Auger, M.W.; Ding, X.; et al. H0LiCOW—I. H0 Lenses in COSMOGRAIL’s Wellspring: Program overview. Mon. Not. R. Astron. Soc. 2017, 468, 2590–2604. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, Q.G. Measuring H0 from low-z datasets. Sci. China Phys. Mech. Astron. 2020, 63, 290402. [Google Scholar] [CrossRef]
- Kazantzidis, L.; Perivolaropoulos, L. Evolution of the fσ8 tension with the Planck15/ΛCDM determination and implications for modified gravity theories. Phys. Rev. D 2018, 97, 103503. [Google Scholar] [CrossRef]
- Linder, E.V. Probing gravitation, dark energy, and acceleration. Phys. Rev. D 2004, 70, 023511. [Google Scholar] [CrossRef]
- Chevallier, M.; Polarski, D. Accelerating universes with scaling dark matter. Int. J. Mod. Phys. D 2001, 10, 213–223. [Google Scholar] [CrossRef]
- Linder, E.V. Exploring the Expansion History of the Universe. Phys. Rev. Lett. 2003, 90, 091301. [Google Scholar] [CrossRef] [PubMed]
- Ross, A.J.; Samushia, L.; Howlett, C.; Percival, W.J.; Burden, A.; Manera, M. The clustering of the SDSS DR7 main Galaxy sample—I. A 4 per cent distance measure at z = 0.15. Mon. Not. R. Astron. Soc. 2015, 449, 835–847. [Google Scholar] [CrossRef]
- Alam, S.; Ata, M.; Bailey, S.; Beutler, F.; Bizyaev, D.; Blazek, J.A.; Bolton, A.S.; Brownstein, J.R.; Burden, A.; Chuang, C.-H.; et al. The clustering of galaxies in the completed SDSS-III Baryon Oscillation Spectroscopic Survey: Cosmological analysis of the DR12 galaxy sample. Mon. Not. R. Astron. Soc. 2017, 470, 2617–2652. [Google Scholar] [CrossRef]
- Gil-Marin, H.; Bautista, J.E.; Paviot, R.; Vargas, M.; De la Torre, S.; Fromenteau, S.; Alam, S.; Avila, S.; Burtin, E.; Chuang, C.-H.; et al. The Completed SDSS-IV extended Baryon Oscillation Spectroscopic Survey: Measurement of the BAO and growth rate of structure of the luminous red galaxy sample from the anisotropic power spectrum between redshifts 0.6 and 1.0. Mon. Not. R. Astron. Soc. 2020, 498, 2492–2531. [Google Scholar] [CrossRef]
- Raichoor, A.; De Mattia, A.; Ross, A.J.; Zhao, C.; Alam, S.; Avila, S.; Bautista, J.; Brinkmann, J.; Brownstein, J.R.; Burtin, E.; et al. The completed SDSS-IV extended Baryon Oscillation Spectroscopic Survey: Large-scale structure catalogues and measurement of the isotropic BAO between redshift 0.6 and 1.1 for the Emission Line Galaxy Sample. Mon. Not. R. Astron. Soc. 2021, 500, 3254–3274. [Google Scholar] [CrossRef]
- Hou, J.; Sanchez, A.G.; Ross, A.J.; Smith, A.; Neveux, R.; Bautista, J.; Burtin, E.; Zhao, C.; Scoccimarro, R.; Dawson, K.S.; et al. The completed SDSS-IV extended Baryon Oscillation Spectroscopic Survey: BAO and RSD measurements from anisotropic clustering analysis of the quasar sample in configuration space between redshift 0.8 and 2.2. Mon. Not. R. Astron. Soc. 2021, 500, 1201–1221. [Google Scholar] [CrossRef]
- du Mas des Bourboux, H.; Rich, J.; Font-Ribera, A.; de Sainte Agathe, V.; Farr, J.; Etourneau, T.; Le Goff, J.-M.; Cuceu, A.; Balland, C.; Bautista, J.E.; et al. The Completed SDSS-IV Extended Baryon Oscillation Spectroscopic Survey: Baryon Acoustic Oscillations with Lyα Forests. Astron. J. 2020, 901, 153. [Google Scholar] [CrossRef]
- DES Collaboration. Dark Energy Survey Year 3 results: A 2.7 percent measurement of baryon acoustic oscillation distance scale at redshift 0.835. Phys. Rev. D 2022, 105, 043512. [Google Scholar] [CrossRef]
- Sridhar, S.; Song, Y.-S.; Ross, A.J.; Zhou, R.; Newman, J.A.; Chuang, C.-H.; Blum, R.; Gaztanaga, E.; Landriau, M.; Prada, F. Clustering of LRGs in the DECaLS DR8 Footprint: Distance Constraints from Baryon Acoustic Oscillations Using Photometric Redshifts. Astron. J. 2020, 904, 69. [Google Scholar] [CrossRef]
- Beutler, F.; Blake, C.; Colless, M.; Jones, D.H.; Staveley-Smith, L.; Campbell, L.; Parker, Q.; Saunders, W.; Watson, F. The 6dF Galaxy Survey: Baryon acoustic oscillations and the local Hubble constant. Mon. Not. R. Astron. Soc. 2011, 416, 3017–3032. [Google Scholar] [CrossRef]
- Handley, W.J.; Hobson, M.P.; Lasenby, A.N. POLYCHORD: Nested sampling for cosmology. Mon. Not. R. Astron. Soc. Lett. 2015, 450, L61–L65. [Google Scholar] [CrossRef]
- Riess, A.G.; Anand, G.S.; Yuan, W.; Casertano, S.; Dolphin, A.; Macri, L.M.; Breuval, L.; Scolnic, D.; Perrin, M.; Anderson, R.I. Crowded No More: The Accuracy of the Hubble Constant Tested with High-resolution Observations of Cepheids by JWST. Astrophys. J. Lett. 2023, 956, L18. [Google Scholar] [CrossRef]
- Alam, S.; Aubert, M.; Avila, S.; Ball, C.; Bautista, J.E.; Bershady, M.A.; Bizyaev, D.; Blanton, M.R.; Bolton, A.S.; Bovy, J.; et al. Completed SDSS-IV extended Baryon Oscillation Spectroscopic Survey: Cosmological implications from two decades of spectroscopic surveys at the Apache Point Observatory. Phys. Rev. D 2021, 103, 083533. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, G.; Chuang, C.; Ross, A.J.; Percival, W.J.; Gil-Marín, H.; Cuesta, A.J. The clustering of galaxies in the completed SDSS-III Baryon Oscillation Spectroscopic Survey: Tomographic BAO analysis of DR12 combined sample in configuration space. Mon. Not. R. Astron. Soc. 2017, 469, 3762–3774. [Google Scholar] [CrossRef]
- Zhao, G.-B.; Wang, Y.; Taruya, A.; Zhang, W.; Gil-Marin, H.; de Mattia, A.; Ross, A.J.; Raichoor, A.; Zhao, C.; Percival, W.J.; et al. The completed SDSS-IV extended Baryon Oscillation Spectroscopic Survey: A multitracer analysis in Fourier space for measuring the cosmic structure growth and expansion rate. Mon. Not. R. Astron. Soc. 2021, 504, 33–52. [Google Scholar] [CrossRef]
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Huber, M.E.; Kessler, R.; Narayan, G.; Riess, A.G.; et al. The Complete Light-curve Sample of Spectroscopically Confirmed SNe Ia from Pan-STARRS1 and Cosmological Constraints from the Combined Pantheon Sample. Astron. J. 2018, 859, 101. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, H.; Yuan, S.; Liu, S.; Zhang, T.-J.; Sun, Y.-C. Four new observational H(z) data from luminous red galaxies in the Sloan Digital Sky Survey data release seven. Res. Astron. Astrophys. 2014, 14, 1221. [Google Scholar] [CrossRef]
- Simon, J.; Verde, L.; Jimenez, R. Constraints on the redshift dependence of the dark energy potential. Phys. Rev. D 2005, 71, 123001. [Google Scholar] [CrossRef]
- Moresco, M.; Cimatti, A.; Jimenez, R.; Pozzetti, L.; Zamorani, G.; Bolzonella, M.; Dunlop, J.; Lamareille, F.; Mignoli, M.; Pearce, H.; et al. Improved constraints on the expansion rate of the Universe up to z ≈ 1.1 from the spectroscopic evolution of cosmic chronometers. J. Cosmol. Astropart. Phys. 2012, 8, 6. [Google Scholar] [CrossRef]
- Moresco, M.; Pozzetti, L.; Cimatti, A.; Jimenez, R.; Maraston, C.; Verde, L.; Thomas, D.; Citro, A.; Tojeiro, R.; Wilkinson, D. A 6 percent measurement of the Hubble parameter at z ≈ 0.45: Direct evidence of the epoch of cosmic re-acceleration. J. Cosmol. Astropart. Phys. 2016, 5, 14. [Google Scholar] [CrossRef]
- Ratsimbazafy, A.L.; Loubser, S.I.; Crawford, S.M.; Cress, C.M.; Bassett, B.A.; Nichol, R.C.; Väisänen, P. Age-dating luminous red galaxies observed with the Southern African Large Telescope. Mon. Not. R. Astron. Soc. 2017, 467, 3239–3254. [Google Scholar] [CrossRef]
- Stern, D.; Jimenez, R.; Verde, L.; Kamionkowski, M.; Stanford, S.A. Cosmic chronometers: Constraining the equation of state of dark energy. I: H(z) measurements. J. Cosmol. Astropart. Phys. 2010, 2, 8. [Google Scholar] [CrossRef]
- Borghi, N.; Moresco, M.; Cimatti, A. Toward a Better Understanding of Cosmic Chronometers: A New Measurement of H(z) at z ≈ 0.7. Astrophys. J. Lett. 2022, 928, L4. [Google Scholar] [CrossRef]
- Jiao, K.; Borghi, N.; Moresco, M.; Zhang, T.-J. New Observational H(z) Data from Full-spectrum Fitting of Cosmic Chronometers in the LEGA-C Survey. Astrophys. J. Suppl. Ser. 2023, 265, 48. [Google Scholar] [CrossRef]
- Moresco, M. Raising the bar: New constraints on the Hubble parameter with cosmic chronometers at z ≈ 2. Mon. Not. R. Astron. Soc. Lett. 2015, 450, L16–L20. [Google Scholar] [CrossRef]
- Nunes, R.C.; Yadav, S.K.; Jesus, J.F.; Bernui, A. Cosmological parameter analyses using transversal BAO data. Mon. Not. R. Astron. Soc. 2020, 497, 2133–2141. [Google Scholar] [CrossRef]
- Nunes, R.C.; Bernui, A. BAO signatures in the 2-point angular correlations and the Hubble tension. Eur. Phys. J. C 2020, 80, 1025. [Google Scholar] [CrossRef]
- Verde, L.; Bernal, J.L.; Heavens, A.F.; Jimenez, R. The length of the low-redshift standard ruler. Mon. Not. R. Astron. Soc. 2017, 467, 731–736. [Google Scholar] [CrossRef][Green Version]
- Lemos, T.; Ruchika; Carvalho, J.C.; Alcaniz, J. Low-redshift estimates of the absolute scale of baryon acoustic oscillations. Eur. Phys. J. C 2023, 83, 495. [Google Scholar] [CrossRef]
- Pogosian, L.; Zhao, G.-B.; Jedamzik, K. Recombination-independent Determination of the Sound Horizon and the Hubble Constant from BAO. Astrophys. J. Lett. 2020, 904, L7. [Google Scholar] [CrossRef]
- Akaike, H. A new look at the statistical model identification. IEEE Trans. Autom. Control. 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Schwarz, G. Estimating the Dimension of a Model. Ann. Statist. 1978, 6, 461–464. [Google Scholar] [CrossRef]


| z | Method | Reference | ||
|---|---|---|---|---|
| 0.07 | 69 | 19.6 | Full-spectrum fitting | [57] |
| 0.09 | 69 | 12 | Full-spectrum fitting | [58] |
| 0.12 | 68.6 | 26.2 | Full-spectrum fitting | [57] |
| 0.17 | 83 | 8 | Full-spectrum fitting | [58] |
| 0.179 | 75 | 4 | Calibrated D4000 | [59] |
| 0.199 | 75 | 5 | Calibrated D4000 | [59] |
| 0.20 | 72.9 | 29.6 | Full-spectrum fitting | [57] |
| 0.27 | 77 | 14 | Full-spectrum fitting | [58] |
| 0.28 | 88.8 | 36.6 | Full-spectrum fitting | [57] |
| 0.352 | 83 | 14 | Calibrated D4000 | [59] |
| 0.38 | 83 | 13.5 | Calibrated D4000 | [60] |
| 0.4 | 95 | 17 | Full-spectrum fitting | [58] |
| 0.4004 | 77 | 10.2 | Calibrated D4000 | [60] |
| 0.425 | 87.1 | 11.2 | Calibrated D4000 | [60] |
| 0.445 | 92.8 | 12.9 | Calibrated D4000 | [60] |
| 0.47 | 89.0 | 49.6 | Full-spectrum fitting | [61] |
| 0.4783 | 80.9 | 9 | Calibrated D4000 | [60] |
| 0.48 | 97 | 62 | Full-spectrum fitting | [62] |
| 0.593 | 104 | 13 | Calibrated D4000 | [59] |
| 0.68 | 92 | 8 | Calibrated D4000 | [59] |
| 0.75 | 98.8 | 33.6 | Lick indices | [63] |
| 0.781 | 105 | 12 | Calibrated D4000 | [59] |
| 0.80 | 113.1 | 28.5 | Full-spectrum fitting | [64] |
| 0.875 | 125 | 17 | Calibrated D4000 | [59] |
| 0.88 | 90 | 40 | Full-spectrum fitting | [62] |
| 0.9 | 117 | 23 | Full-spectrum fitting | [58] |
| 1.037 | 154 | 20 | Calibrated D4000 | [59] |
| 1.3 | 168 | 17 | Full-spectrum fitting | [58] |
| 1.363 | 160 | 33.6 | Calibrated D4000 | [65] |
| 1.43 | 177 | 18 | Full-spectrum fitting | [58] |
| 1.53 | 140 | 14 | Full-spectrum fitting | [58] |
| 1.75 | 202 | 40 | Full-spectrum fitting | [58] |
| 1.965 | 186.5 | 50.4 | Calibrated D4000 | [65] |
| n Correlated Pairs | BAO | BAO + R22 |
|---|---|---|
| n = 0 | = 0.269 ± 0.015 | = 0.269 ± 0.017 |
| = 0.725 ± 0.011 | = 0.725 ± 0.013 | |
| n = 6 | = 0.263 ± 0.015 | = 0.264 ± 0.015 |
| = 0.731 ± 0.015 | = 0.730 ± 0.014 | |
| n = 12 | = 0.262 ± 0.017 | = 0.263 ± 0.015 |
| = 0.732 ± 0.012 | = 0.732 ± 0.011 |
| Parameter | BAO | BAO + R22 | BAO + Pantheon + CC | BAO + Pantheon + CC + R22 |
|---|---|---|---|---|
| (km s Mpc) | 68.01 ± 4.53 | 72.82 ± 1.01 | 69.76 ± 1.71 | 71.68 ± 1.65 |
| 0.270 ± 0.039 | 0.268 ± 0.037 | 0.275 ± 0.025 | 0.271 ± 0.026 | |
| 0.725 ± 0.022 | 0.726 ± 0.023 | 0.720 ± 0.014 | 0.724 ± 0.015 | |
| (Mpc) | 150.45 ± 9.89 | 140.14 ± 3.16 | 145.88 ± 3.32 | 142.10 ± 2.49 |
| 0.999 ± 0.074 | 0.938 ± 0.023 | 0.971 ± 0.028 | 0.949 ± 0.024 |
| Parameter | BAO | BAO + R22 | BAO + Pantheon + CC | BAO + Pantheon + CC + R22 |
|---|---|---|---|---|
| (km s Mpc) | 65.83 ± 4.73 | 72.56 ± 2.10 | 69.83 ± 1.06 | 71.60 ± 1.02 |
| 0.193 ± 0.077 | 0.201 ± 0.068 | 0.273 ± 0.015 | 0.273 ± 0.016 | |
| 0.786 ± 0.050 | 0.780 ± 0.046 | 0.721 ± 0.013 | 0.721 ± 0.013 | |
| w | −0.753 ± 0.168 | −0.786 ± 0.210 | −1.001 ± 0.040 | −1.014 ± 0.053 |
| (Mpc) | 150.67 ± 10.32 | 136.90 ± 2.48 | 145.73 ± 3.45 | 142.44 ± 2.24 |
| 0.996 ± 0.069 | 0.924 ± 0.018 | 0.970 ± 0.029 | 0.949 ± 0.025 |
| Parameters | BAO | BAO+R22 | BAO+Pantheon+CC | BAO + Pantheon + CC + R22 |
|---|---|---|---|---|
| (km s Mpc) | 65.82 ± 4.43 | 72.83 ± 1.41 | 69.90 ± 1.06 | 71.71 ± 1.06 |
| 0.159 ± 0.098 | 0.165 ± 0.073 | 0.183 ± 0.056 | 0.178 ± 0.050 | |
| 0.826 ± 0.080 | 0.820 ± 0.065 | 0.810 ± 0.050 | 0.814 ± 0.050 | |
| −1.214 ± 0.130 | −1.149 ± 0.121 | −1.027 ± 0.069 | −1.020 ± 0.072 | |
| −0.344 ± 0.432 | −0.478 ± 0.390 | −0.848 ± 0.180 | −0.878 ± 0.161 | |
| (Mpc) | 152.01 ± 10.18 | 138.26 ± 2.82 | 146.18 ± 2.35 | 142.73 ± 2.36 |
| 1.002 ± 0.066 | 0.930 ± 0.022 | 0.974 ± 0.033 | 0.950 ± 0.035 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lozano Torres, J.A. Testing Cosmic Acceleration from the Late-Time Universe. Astronomy 2023, 2, 300-314. https://doi.org/10.3390/astronomy2040020
Lozano Torres JA. Testing Cosmic Acceleration from the Late-Time Universe. Astronomy. 2023; 2(4):300-314. https://doi.org/10.3390/astronomy2040020
Chicago/Turabian StyleLozano Torres, Jose Agustin. 2023. "Testing Cosmic Acceleration from the Late-Time Universe" Astronomy 2, no. 4: 300-314. https://doi.org/10.3390/astronomy2040020
APA StyleLozano Torres, J. A. (2023). Testing Cosmic Acceleration from the Late-Time Universe. Astronomy, 2(4), 300-314. https://doi.org/10.3390/astronomy2040020






