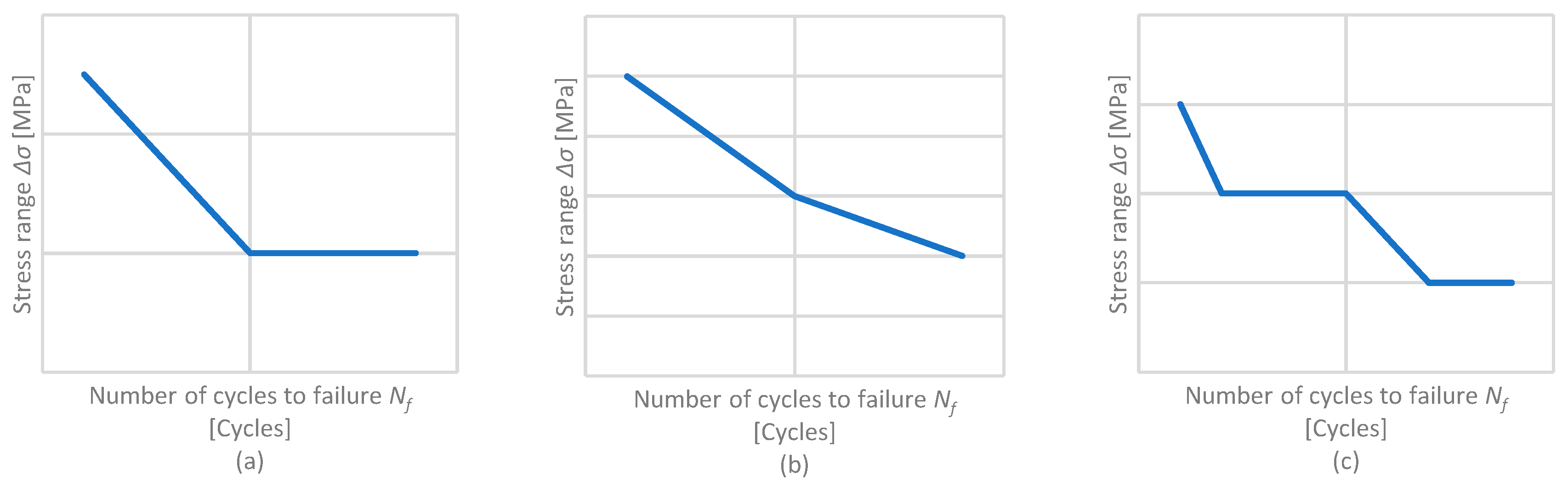
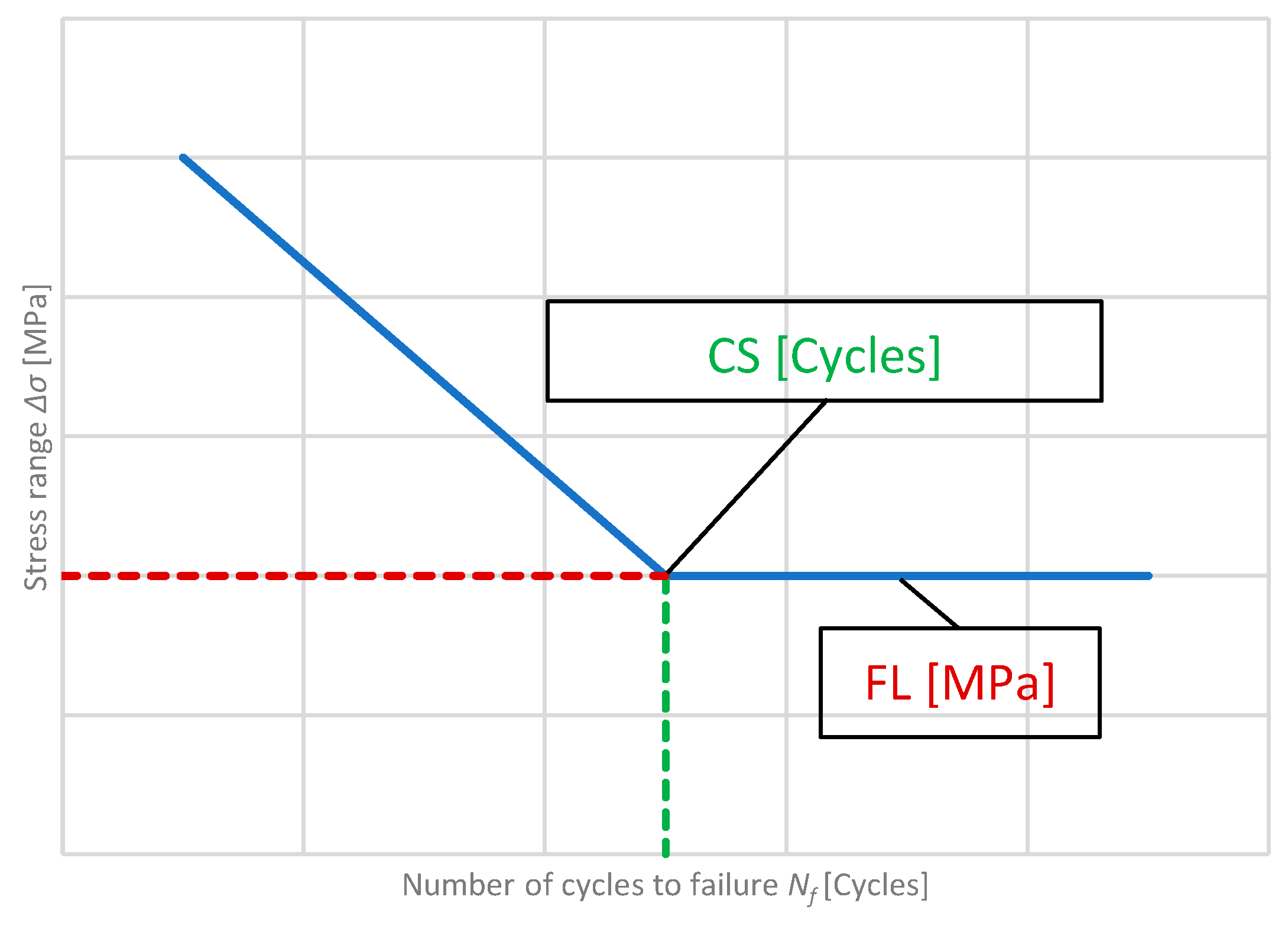
Figure 2.
Schematic illustration of S-N curve showing the positions of the CS and FL.
Figure 2.
Schematic illustration of S-N curve showing the positions of the CS and FL.
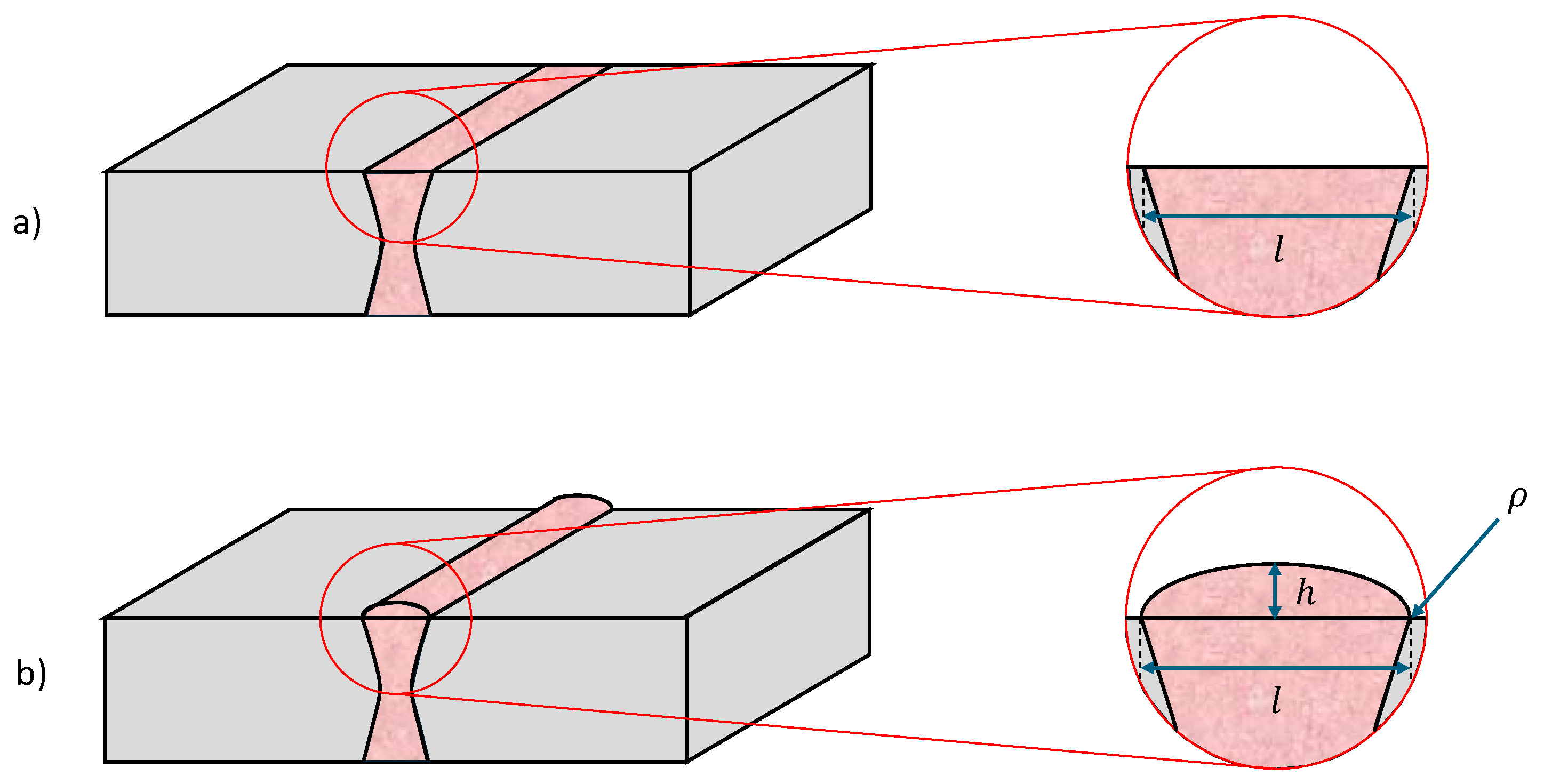
Figure 3.
Difference between (a) ground flush and (b) as-welded conditions. The parameters h (height of the weld convexity), l (length of the weld seam), and ρ (weld toe radius) are reported.
Figure 3.
Difference between (a) ground flush and (b) as-welded conditions. The parameters h (height of the weld convexity), l (length of the weld seam), and ρ (weld toe radius) are reported.
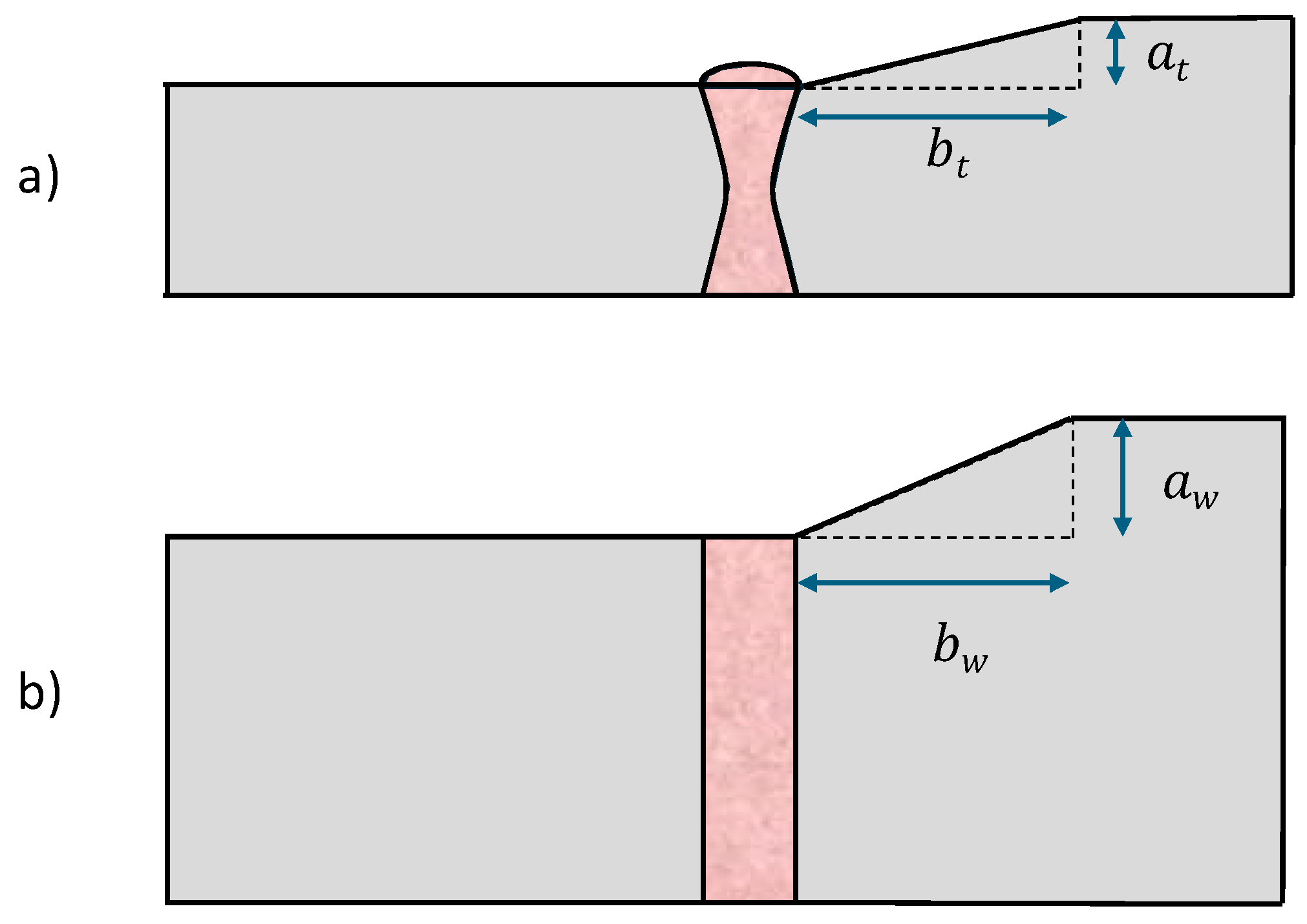
Figure 4.
Explanation of the elevation by means of an as-welded joint seen from both a (a) side and (b) top view.
Figure 4.
Explanation of the elevation by means of an as-welded joint seen from both a (a) side and (b) top view.
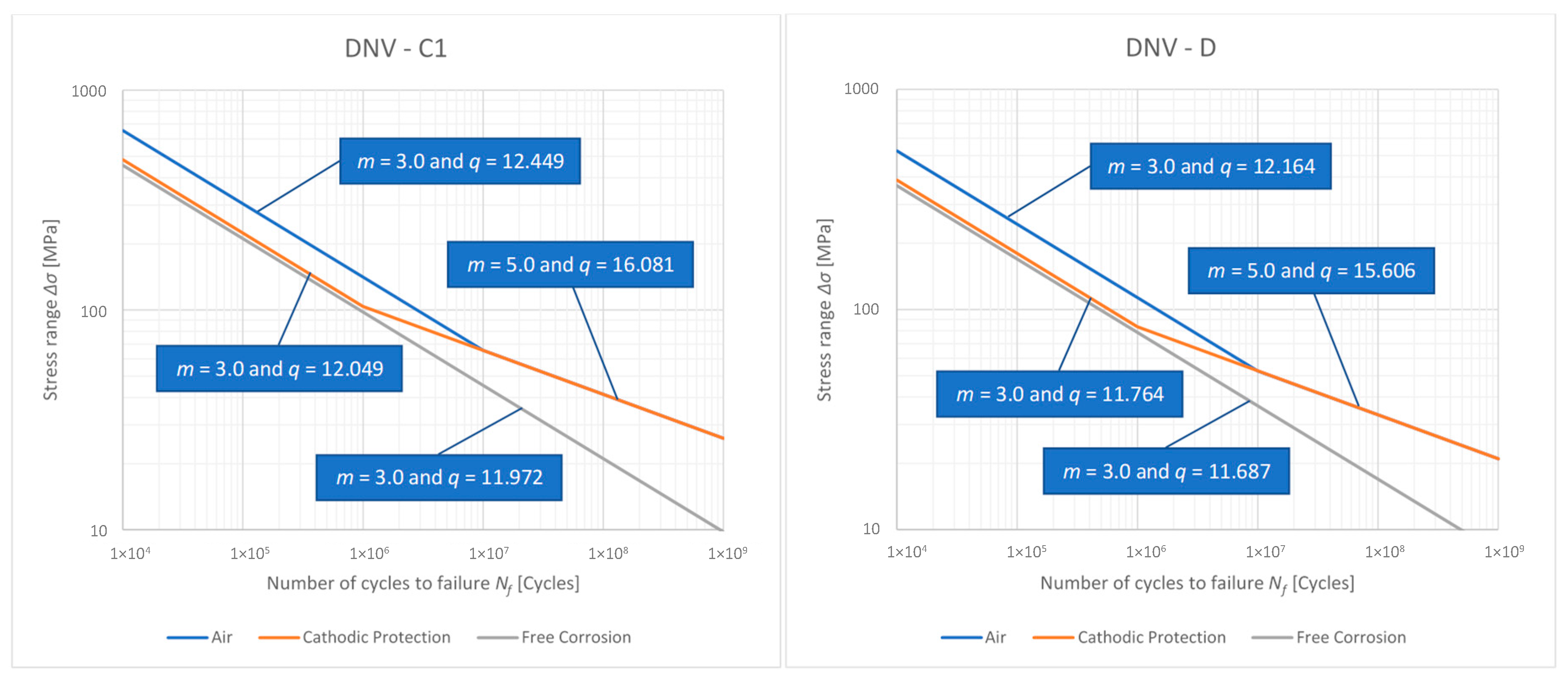
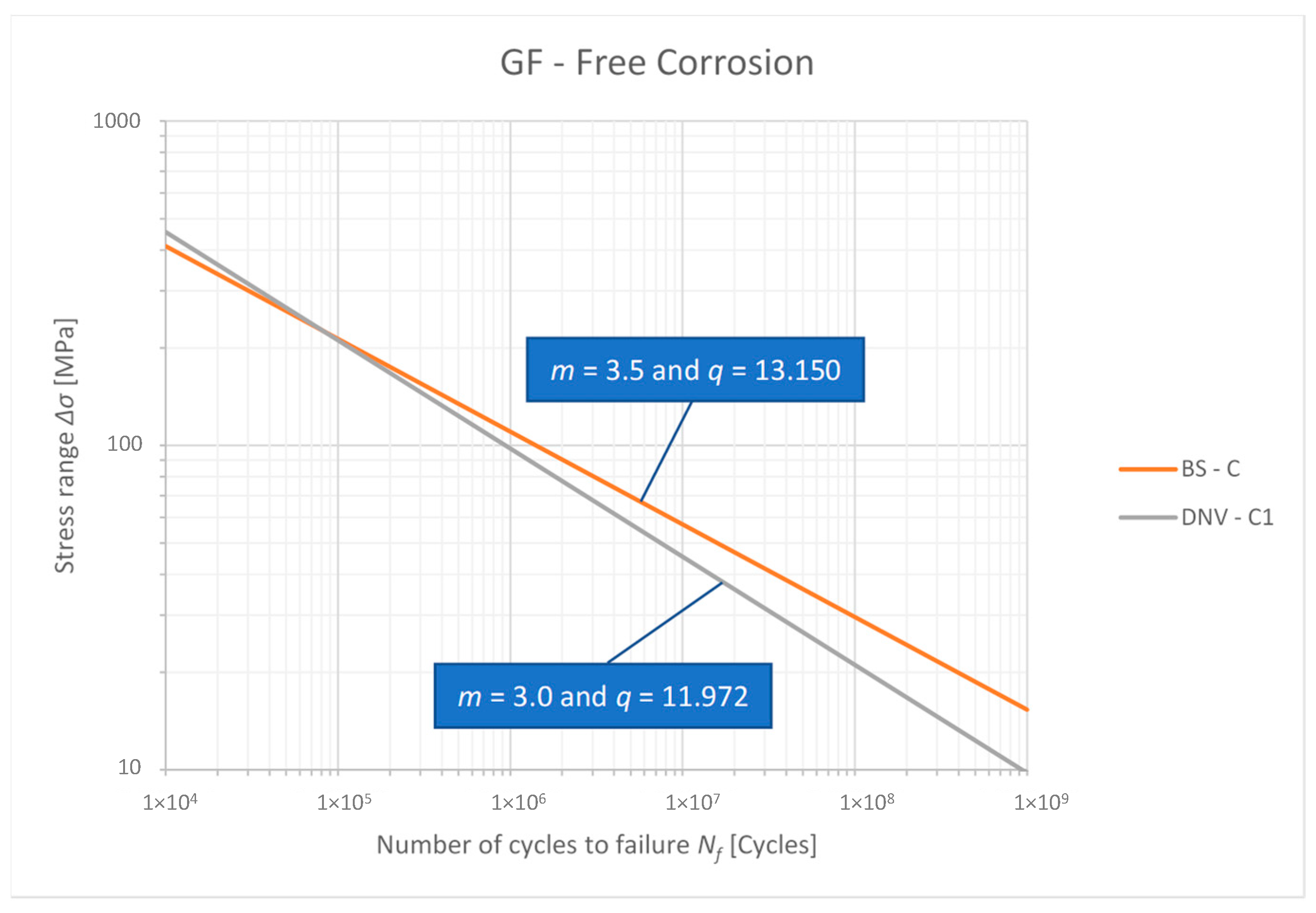
Figure 5.
S-N curves recommended in the DNV standard for classes C1 (left) and D (right) in various environments.
Figure 5.
S-N curves recommended in the DNV standard for classes C1 (left) and D (right) in various environments.
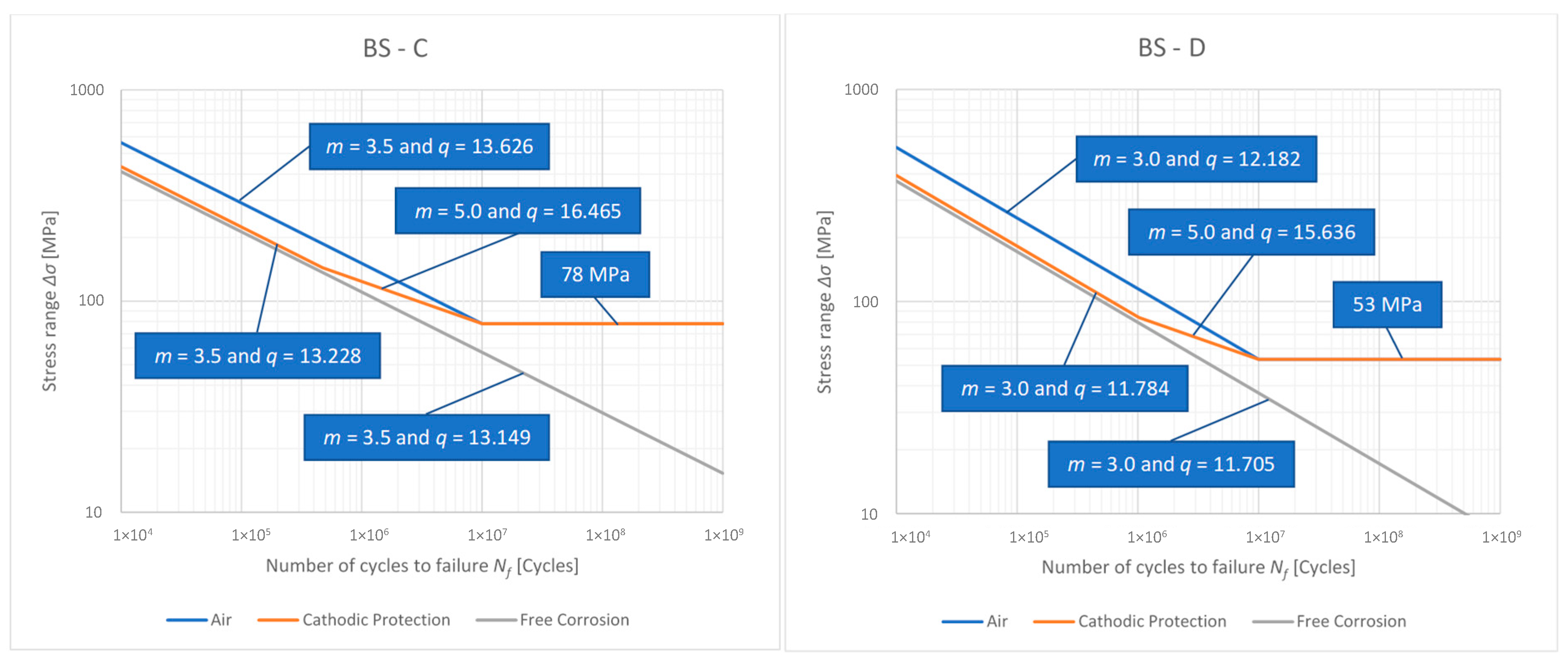
Figure 6.
S-N curves from BS for classes C (left) and D (right) in various environments.
Figure 6.
S-N curves from BS for classes C (left) and D (right) in various environments.
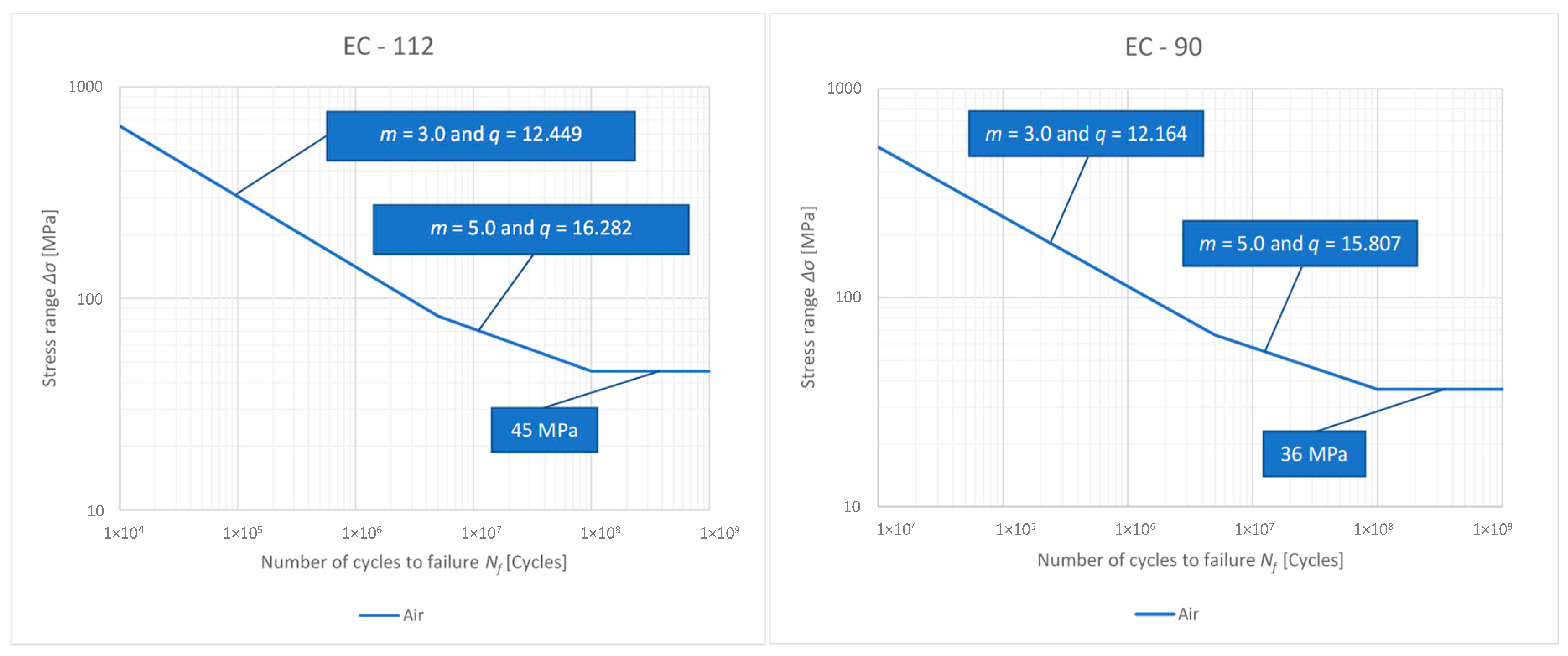
Figure 7.
S-N curves from EC for classes 112 (left) and 90 (right) in air.
Figure 7.
S-N curves from EC for classes 112 (left) and 90 (right) in air.
Figure 8.
S-N curves comparison for classes C1, C, and 112 from DNV, BS, and EC, respectively, in air.
Figure 8.
S-N curves comparison for classes C1, C, and 112 from DNV, BS, and EC, respectively, in air.
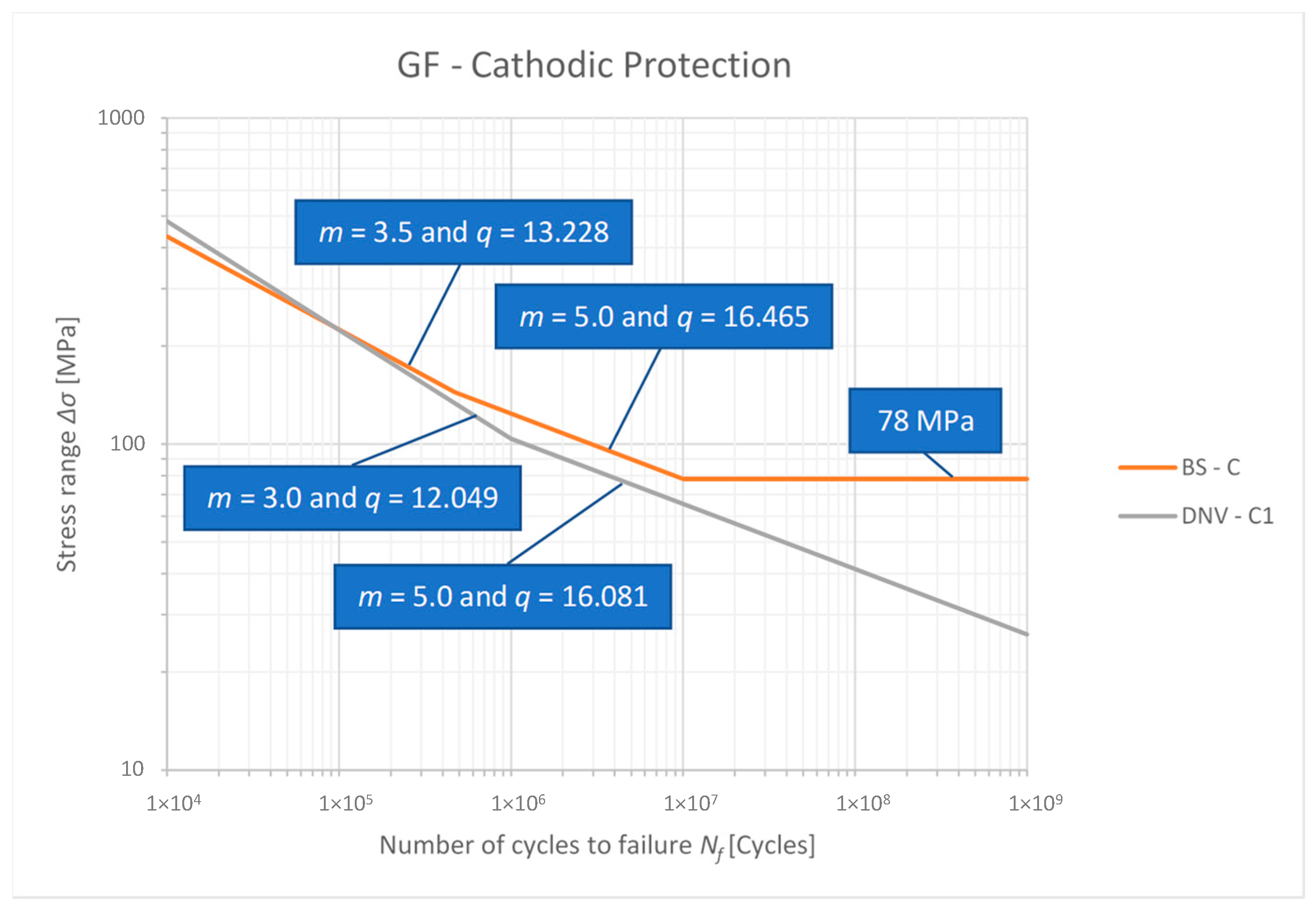
Figure 9.
S-N curves comparison from DNV and BS for classes C1 and C, where the environment is seawater with cathodic protection.
Figure 9.
S-N curves comparison from DNV and BS for classes C1 and C, where the environment is seawater with cathodic protection.
Figure 10.
S-N curves comparison from DNV and BS for classes C1 and C, where the environment is seawater without cathodic protection.
Figure 10.
S-N curves comparison from DNV and BS for classes C1 and C, where the environment is seawater without cathodic protection.
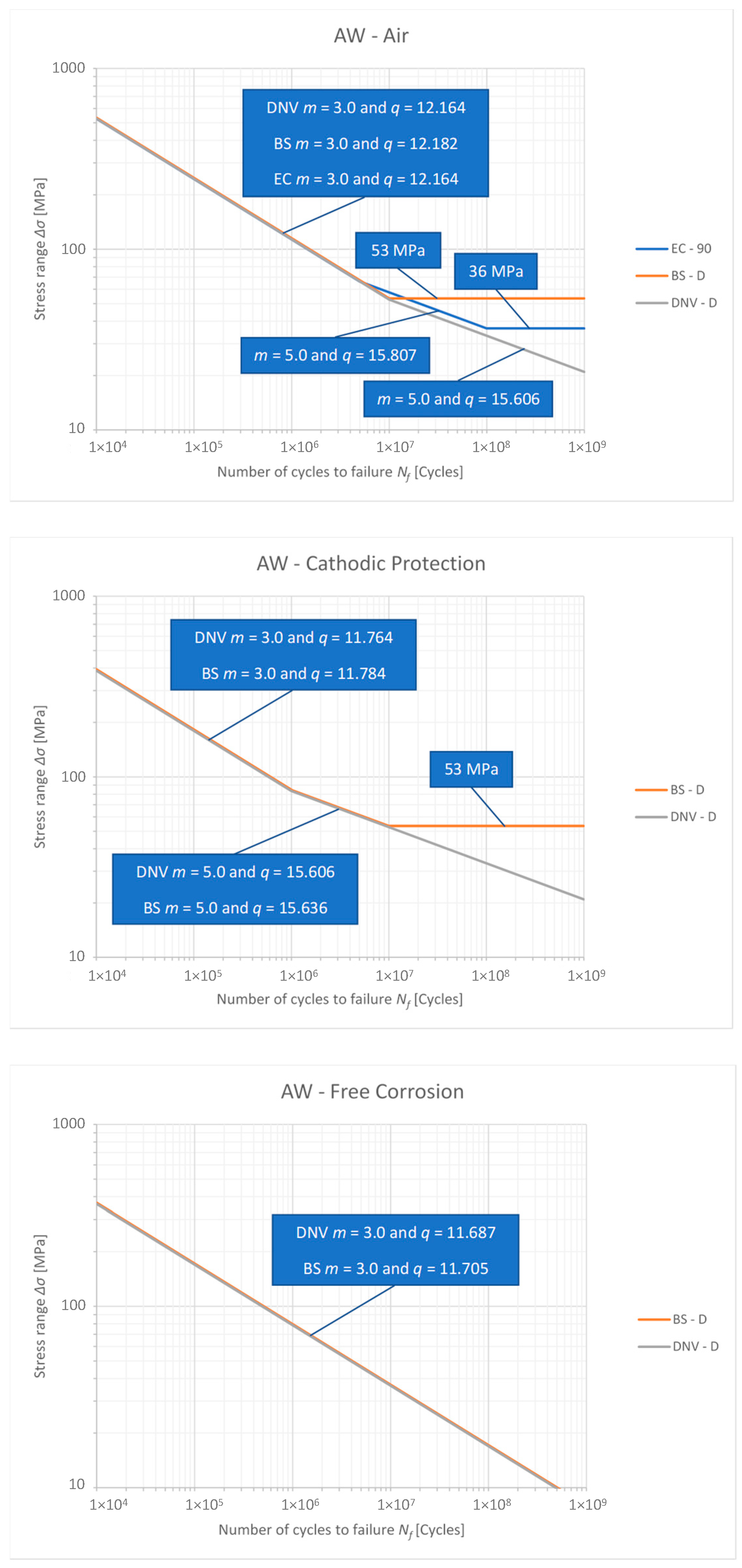
Figure 11.
S-N curves comparison from DNV, BS, and EC for AW classes in air, seawater with cathodic protection, and seawater with free-corrosion conditions (from top to bottom, respectively).
Figure 11.
S-N curves comparison from DNV, BS, and EC for AW classes in air, seawater with cathodic protection, and seawater with free-corrosion conditions (from top to bottom, respectively).
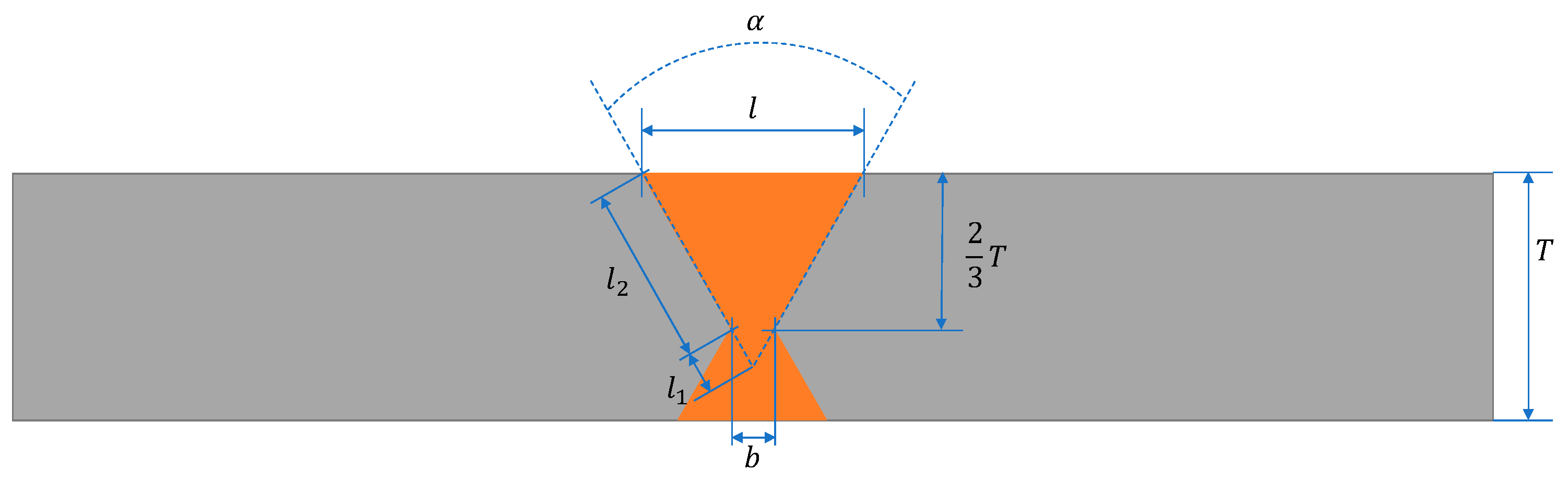
Figure 12.
Welded joint showing geometrical parameters b (weld gap), l (weld width), α (groove angle), T (thickness of the plates), and other parameters useful to correlate the weld width with the thickness of the plates.
Figure 12.
Welded joint showing geometrical parameters b (weld gap), l (weld width), α (groove angle), T (thickness of the plates), and other parameters useful to correlate the weld width with the thickness of the plates.
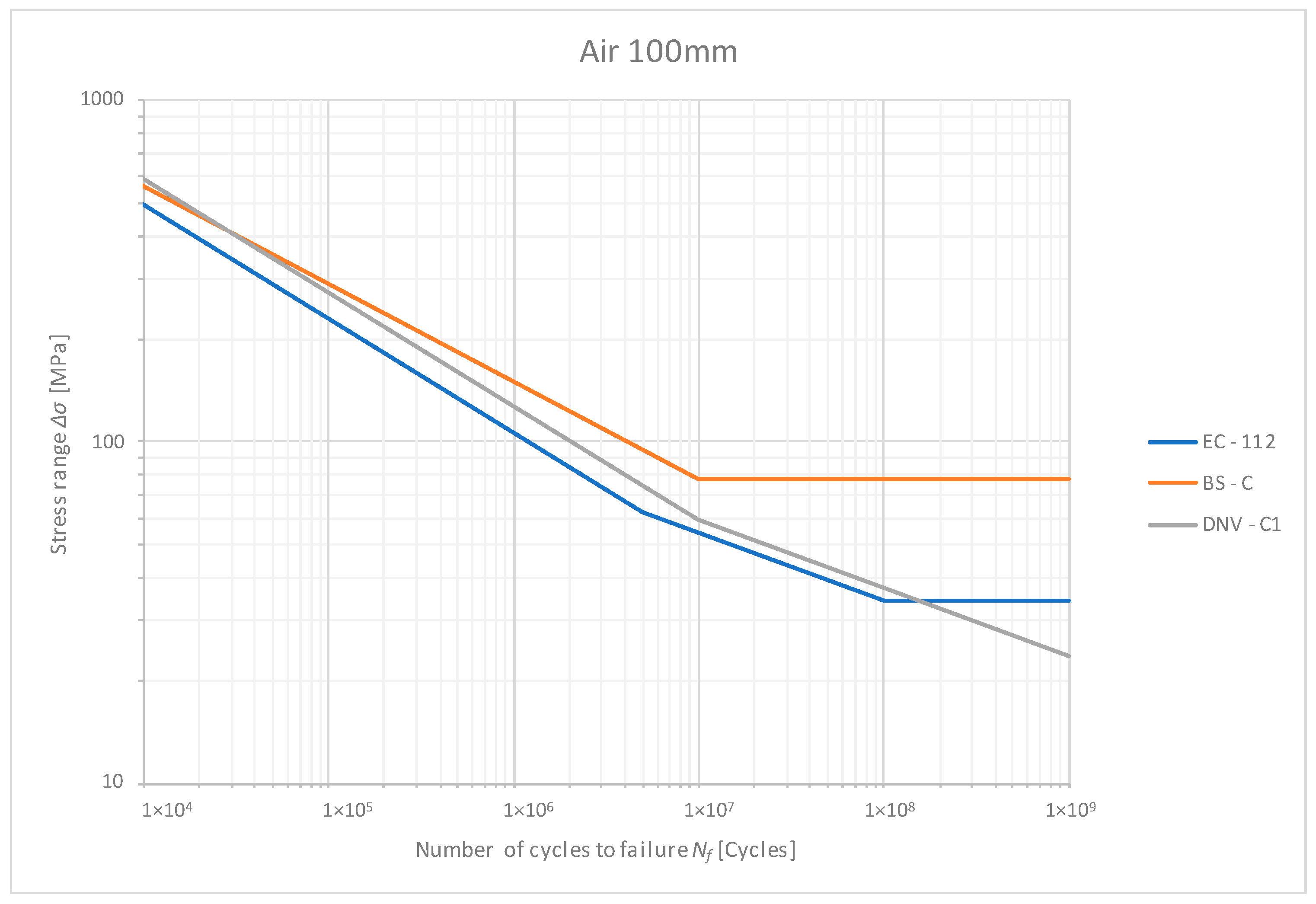
Figure 13.
S-N curves comparison for classes C1, C, and 112 from DNV, BS, and EC, respectively, where the environment is air and the thickness is 100 mm.
Figure 13.
S-N curves comparison for classes C1, C, and 112 from DNV, BS, and EC, respectively, where the environment is air and the thickness is 100 mm.
Figure 14.
The percentage variation of number of cycles to failure between DNV and linear unfixed in air for class D.
Figure 14.
The percentage variation of number of cycles to failure between DNV and linear unfixed in air for class D.
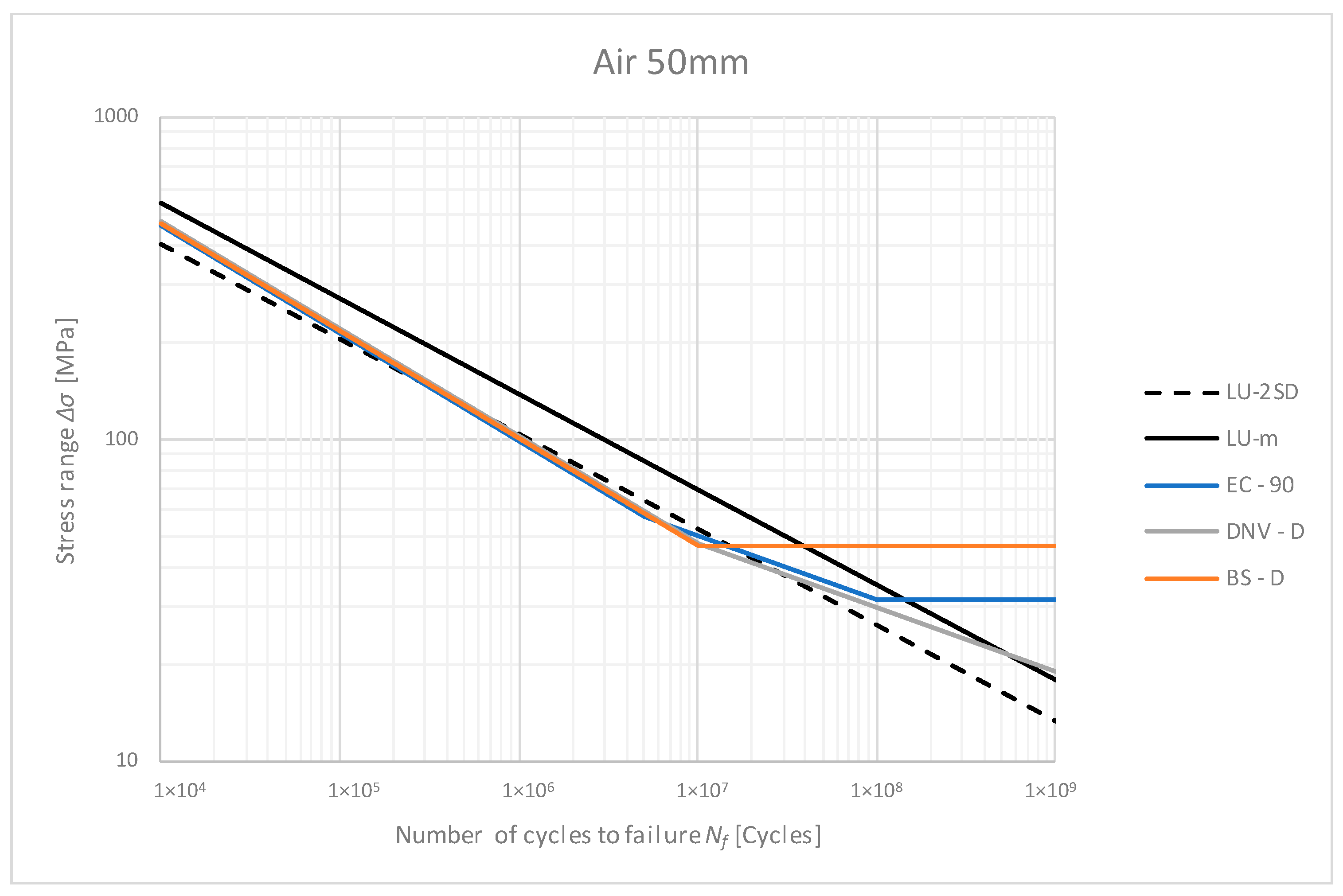
Figure 15.
Comparison between the S-N curves coming from the standards and the linear unfixed model by Mehmanparast et al. in air with a thickness of 50 mm for AW classes.
Figure 15.
Comparison between the S-N curves coming from the standards and the linear unfixed model by Mehmanparast et al. in air with a thickness of 50 mm for AW classes.
Table 1.
Weld classifications for double-V transverse butt welds taken from DNV, BS, and EC.
Table 1.
Weld classifications for double-V transverse butt welds taken from DNV, BS, and EC.
| Standard | Class Name | Type of Weld | Allowed Height of Convexity | Allowed Elevation | Notes | Ref. |
|---|
| DNV | C1 | GF | 0 | 0.25 | Specific acceptance criteria and NDT are required for a weld to belong to this class. | Table A-5 in DNV [10] |
| D | AW | | 0.25 | |
| BS | C | GF | 0 | Not allowed | It is reported that this class should not be employed for structural purposes. | Table 5 in BS [11] |
| D | AW | Not reported | 0.25 | |
| EC | 112 | GF | 0 | 0.25 | NDT are required, but it is not specified which ones. No S-N curves in seawater. | Table 8.3 in EC [12] |
| 90 | AW | | 0.25 | NDT are required, but it is not specified which ones. No S-N curves in seawater. |
Table 2.
HCF data for ground flush welds (classes C1, C, and 112 according to DNV, BS, and EC, respectively). The calculated values have been marked with a star sign (*).
Table 2.
HCF data for ground flush welds (classes C1, C, and 112 according to DNV, BS, and EC, respectively). The calculated values have been marked with a star sign (*).
| GF–HCF | Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
|---|
| 3.0 | 3.5 | 3.0 | 3.0 | 3.5 | - | 3.0 | 3.5 | - |
| 12.449 | 13.626 * | 12.449 * | 12.049 | 13.228 * | - | 11.972 | 13.149 * | - |
| 1st CS [Cycles] | | | | | * | - | - | - | - |
Table 3.
UHCF data for ground flush welds (classes C1, C, and 112 according to DNV, BS, and EC, respectively). The calculated values have been marked with a star sign (*).
Table 3.
UHCF data for ground flush welds (classes C1, C, and 112 according to DNV, BS, and EC, respectively). The calculated values have been marked with a star sign (*).
| GF–UHCF | Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
|---|
| 5.0 | - | 5.0 | 5.0 | 5.0 | - | 3.0 | 3.5 | - |
| 16.081 | - | 16.282 * | 16.081 | 16.465 * | - | 11.972 | 13.149 * | - |
| 2nd CS [Cycles] | - | - | | - | | - | - | - | - |
| FL [MPa] | - | 78 | 45* | - | 78 | - | - | - | - |
Table 4.
HCF data for as-welded conditions (class D according to DNV and BS, and class 90 according to EC). The calculated values have been marked with a star sign (*).
Table 4.
HCF data for as-welded conditions (class D according to DNV and BS, and class 90 according to EC). The calculated values have been marked with a star sign (*).
| AW–HCF | Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
|---|
| 3.0 | 3.0 | 3.0 | 3.0 | 3.0 | - | 3.0 | 3.0 | - |
| 12.164 | 12.182 * | 12.164 * | 11.764 | 11.784 * | - | 11.687 | 11.705 * | - |
| 1st CS [Cycles] | | | | | * | - | - | - | - |
Table 5.
UHCF data for as-welded conditions (class D according to DNV and BS, and class 90 according to EC). The calculated values have been marked with a star sign (*).
Table 5.
UHCF data for as-welded conditions (class D according to DNV and BS, and class 90 according to EC). The calculated values have been marked with a star sign (*).
| AW–UHCF | Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
|---|
| 5.0 | - | 5.0 | 5.0 | 5.0 | - | 3.0 | 3.0 | - |
| 15.606 | - | 15.807 * | 15.606 | 15.636 * | - | 11.687 | 11.705 * | - |
| 2nd CS [Cycles] | - | - | | - | | - | - | - | - |
| FL [MPa] | - | 53 | 36 * | - | 53 | - | - | - | - |
Table 6.
The percentage increase in Nf when switching from AW curves to GF curves for all of the analyzed standards and environments.
Table 6.
The percentage increase in Nf when switching from AW curves to GF curves for all of the analyzed standards and environments.
| AW to GF | Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
|---|
| 200 MPa | 93% | 97% | 93% | 93% | 97% | - | 93% | 97% | - |
| 50 MPa | 199% | 0% | 199% | 199% | 0% | - | 93% | 294% | - |
| 10 MPa | 199% | 0% | 0% | 199% | 0% | - | 93% | 780% | - |
Table 7.
Thickness correction exponents from DNV, BS, and EC for both GF and AW classes, depending on the environment.
Table 7.
Thickness correction exponents from DNV, BS, and EC for both GF and AW classes, depending on the environment.
| Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
| GF | 0.10 | NA | 0.20 | 0.10 | NA | - | 0.15 | NA | - |
| AW | 0.20 | 0.20 | 0.20 | 0.20 | 0.20 | - | 0.20 | 0.20 | - |
Table 8.
The percentage decrease in Nf when thickness increases from 25 mm to 100 mm for all of the analyzed standards and environments.
Table 8.
The percentage decrease in Nf when thickness increases from 25 mm to 100 mm for all of the analyzed standards and environments.
| GF | Air | Cathodic Protection | Free Corrosion |
|---|
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
|---|
| 200 MPa | −25% | 0% | −56% | −25% | 0% | - | −36% | 0% | - |
| 50 MPa | −39% | 0% | −75% | −39% | 0% | - | −36% | 0% | - |
| 10 MPa | −39% | 0% | 0% | −39% | 0% | - | −36% | 0% | - |
| AW | Air | Cathodic Protection | Free Corrosion |
| Standard | DNV | BS | EC | DNV | BS | EC | DNV | BS | EC |
| 200 MPa | −45% | −56% | −56% | −45% | −56% | - | −45% | −56% | - |
| 50 MPa | −50% | −inf | −75% | −63% | −inf | - | −45% | −56% | - |
| 10 MPa | −63% | 0% | 0% | −63% | 0% | - | −45% | −56% | - |
Table 9.
Slopes, intercepts, standard deviations, and number of cycles to failure at 200 and 80 MPa for the four models developed by Mehmanparast et al. and the three standards under investigation.
Table 9.
Slopes, intercepts, standard deviations, and number of cycles to failure at 200 and 80 MPa for the four models developed by Mehmanparast et al. and the three standards under investigation.
| | Linear Fixed | Linear Unfixed | Bayesian Fixed | Bayesian Unfixed | DNV | BS | EC |
|---|
| 3.00 | 3.37 | 3.00 | 3.36 | 3.0 | 3.0 | 3.0 |
| 11.953 | 12.786 | 11.940 | 12.765 | 12.033 | 12.001 | 11.983 |
| SD | 0.21 | 0.21 | 0.22 | 0.22 | 0.200 | 0.2095 | NP |
| at 200 MPa | 1.12 × 105 | 1.08 × 105 | 1.09 × 105 | 1.08 × 105 | 1.35 × 105 | 1.25 × 105 | 1.20 × 105 |
| at 80 MPa | 1.75 × 106 | 2.36 × 106 | 1.70 × 106 | 2.35 × 106 | 2.11 × 106 | 1.96 × 106 | 1.88 × 106 |
Table 10.
The percentage increase or decrease in the number of cycles to failure at 200 and 80 MPa calculated by comparing the results obtained from the four models developed by Mehmanparast et al. with those from DNV.
Table 10.
The percentage increase or decrease in the number of cycles to failure at 200 and 80 MPa calculated by comparing the results obtained from the four models developed by Mehmanparast et al. with those from DNV.
| | Linear Fixed | Linear Unfixed | Bayesian Fixed | Bayesian Unfixed |
|---|
| Change in at 200 MPa | −17% | −20% | −19% | −20% |
| Change in at 80 MPa | −17% | 12% | −19% | 11% |