Power Curtailment Analysis of DC Series–Parallel Offshore Wind Farms
Abstract
:1. Introduction
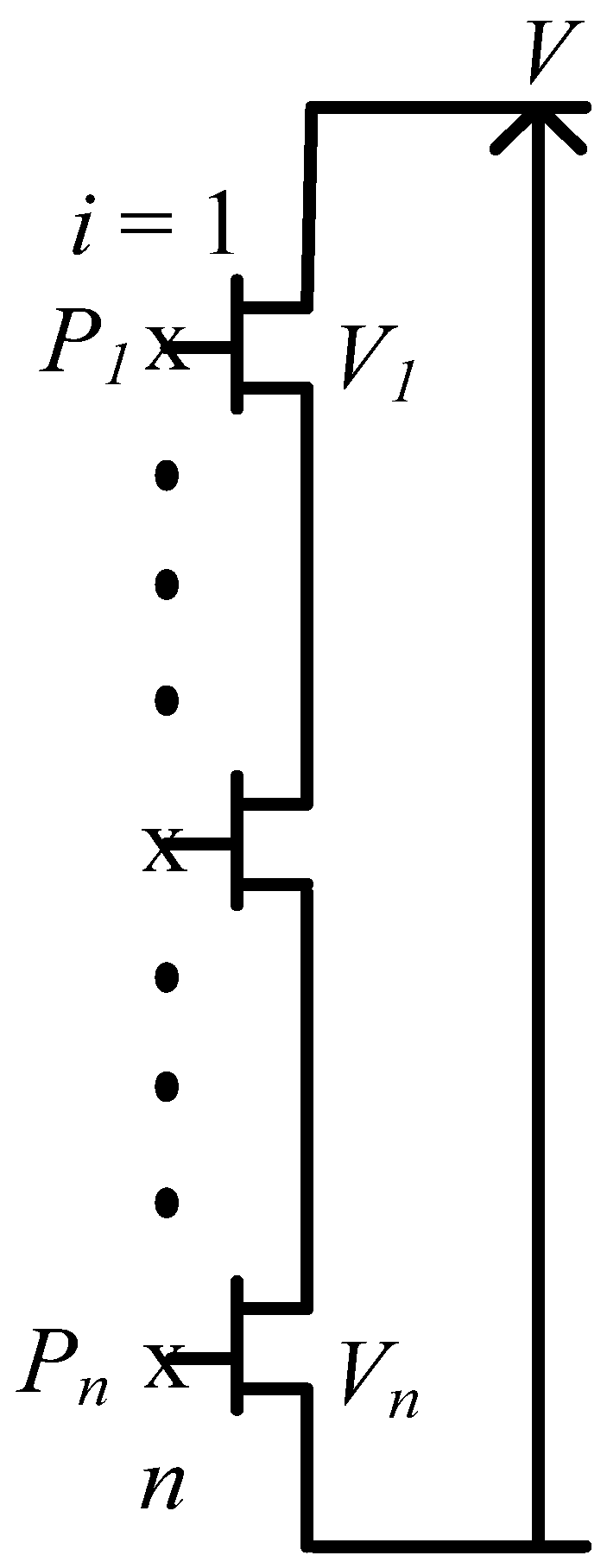
2. DC Series–Parallel Collection System Configuration
2.1. Effect of Voltage Tolerance Levels of MVDC Converters on Energy Curtailment
2.2. Defining Upper-Voltage Tolerance Levels by Redundancy Requirements
2.3. String Voltage Limits
3. Calculation of Power Curtailment Losses
- The windfarm layout for the DC series–parallel collection system is defined with respect to the number of wind turbines connected in the series, the number of strings connected in parallel, and the horizontal and vertical distances between the wind turbines.
- By using the well-known wake model developed by Jensen [31], the wind speed differences among the series-connected turbines are calculated considering both single and multiple wake effects. This has been carried out for the wind speeds ranging from the cut-in wind speed to the cut-out wind speed, taking into account the different wind direction sectors.
- The wind speed of each wind turbine in the string is calculated using step 2, and the output power of each wind turbine is calculated. The power outputs are assumed to be at the rated values beyond the rated speeds.
- The output power differences of the series-connected wind turbines decides the MVDC output voltage of the series-connected wind turbines in each case. Now, considering the ‘n − 1’ redundancy requirement in Equation (4), the upper-voltage tolerance levels for the wind turbine MVDC converter are identified. This factor varies concerning the configuration of the considered wind farms, depending on the number of wind turbines connected in the series.
- The quantity of the required power curtailment in order to maintain the identified upper-voltage tolerance levels of the wind turbine MVDC converter is calculated. This has been explained in [26] with respect to the different power curtailment modes. This has been carried out for all the strings in the wind farm for each combination of the wind speed and wind direction sectors. The total power curtailment losses of the wind farm are calculated as
- 6.
- The energy curtailment losses can be estimated using the total power curtailment losses of the wind farms calculated in the previous step.
4. Case Study for Different Configurations of DC Series–Parallel Wind Farms
4.1. Upper-Voltage Tolerance Levels for Wind Turbine MVDC Converters
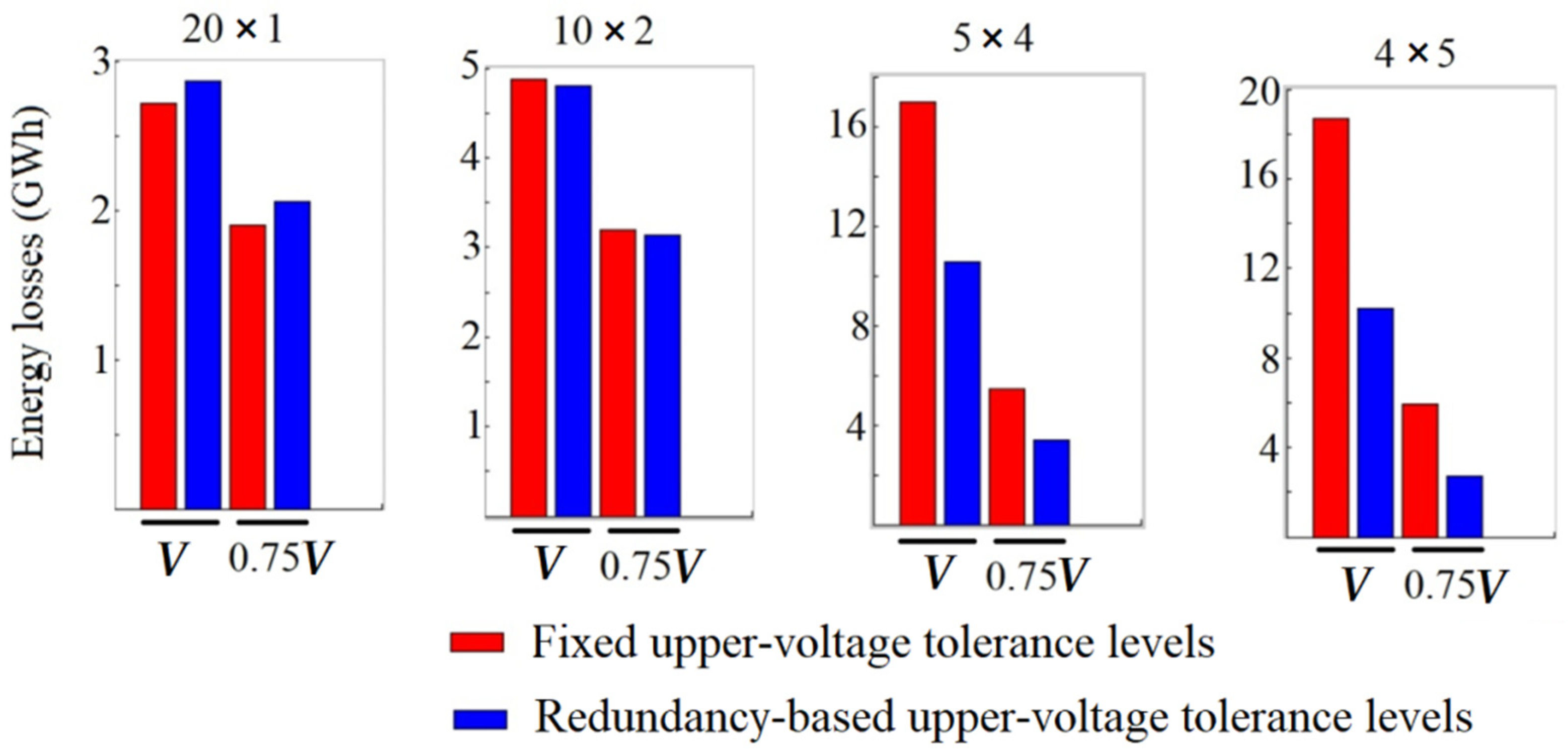
4.2. Comparision of Energy Curtailment Losses with Fixed and Redundancy-Based Upper-Voltage Tolerance Levels
- DC-based wind farms with a greater number of series-connected turbines and a smaller number of parallel-connected strings (cases 1 and 2) can be designed with fixed upper-voltage tolerance levels. The curtailment losses were evaluated to be similar in the comparative study by considering fixed upper-voltage tolerance levels and redundancy-based upper-voltage tolerance levels for the MVDC converters of a DC series–parallel offshore wind farm. Hence, these configurations can be designed with fixed nominal upper-voltage tolerance levels, without oversizing the MVDC converters of the DC series–parallel wind farms.
- DC-based wind farm configurations with a greater number of parallel-connected strings and a smaller number of series-connected turbines (cases 3 and 4) can be designed with redundancy-based upper-voltage tolerance levels. The curtailment losses were evaluated to be lower with redundancy-based upper-voltage tolerance levels in the comparative study between fixed upper-voltage tolerance levels and redundancy-based upper-voltage tolerance levels for the MVDC converters of a DC series–parallel offshore wind farm. Hence, these configurations need to be designed with a redundancy-based upper-voltage tolerance level, which makes it essential to oversize the MVDC converters of the DC series–parallel wind farms.
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Bahirat, H.J.; Mork, B.A.; Hoidalen, H.K. Comparison of wind farm topologies for offshore applications. In Proceedings of the 2012 IEEE Power and Energy Society General Meeting, San Diego, CA, USA, 22–26 July 2012; pp. 1–8. [Google Scholar] [CrossRef]
- Bresesti, P.; Kling, W.; Hendriks, R.; Vailati, R. HVDC connection of offshore wind farms to the transmission system. IEEE Trans. Energy Convers. 2007, 22, 37–43. [Google Scholar] [CrossRef]
- Rahman, S.; Khan, I.; Alkhammash, H.; Nadeem, M. A Comparison Review on Transmission Mode for Onshore Integration of Offshore Wind Farms: HVDC or HVAC. Electronics 2021, 10, 1489. [Google Scholar] [CrossRef]
- Jiang, R.; Wang, J.; Guan, Y. Overview of Multi-MW Wind Turbines and Wind Parks. IEEE Trans. Ind. Electron. 2011, 58, 1081–1095. [Google Scholar] [CrossRef]
- Chen, Z.; Guerrero, J.M.; Blaabjerg, F. A review of the state of the art of power electronics for wind turbines. IEEE Trans. Power Electron. 2009, 24, 1859–1875. [Google Scholar] [CrossRef]
- Gomis-Bellmunt, O.; Junyent-Ferré, A.; Sumper, A.; Bergas-Jané, J. Control of a wind farm based on synchronous generators with a central HVDC-VSC converter. IEEE Trans. Power Syst. 2011, 26, 1632–1640. [Google Scholar] [CrossRef] [Green Version]
- Jovcic, D. Offshore wind farm with a series multiterminal CSI HVDC. Electr. Power Syst. Res. 2008, 78, 747–755. [Google Scholar] [CrossRef]
- Robinson, J.; Jovcic, D.; Joos, G. Analysis and design of an offshore wind farm using a MV DC Grid. IEEE Trans. Power Deliv. 2010, 25, 2164–2173. [Google Scholar] [CrossRef]
- Meyer, C. Key Components for Future Offshore Dc Grids. Ph.D. Thesis, Rheinisch-Westfallischen Technischen Hochschule Aachen, Aachen, Germany, 2007. [Google Scholar]
- Jovcic, D.; Strachan, N. Offshore wind farm with centralized power conversion and DC interconnection. IET Gener. Transm. Distrib. 2009, 3, 586–595. [Google Scholar] [CrossRef]
- Meyer, C.; Hoing, M.; Peterson, A.; De Doncker, R.W. Control and design of DC grids for offshore wind farms. IEEE Trans. Ind. Appl. 2007, 43, 1475–1482. [Google Scholar] [CrossRef]
- Lakshmanan, P.; Liang, J.; Jenkins, N. Assessment of collection systems for HVDC connected offshore wind farms. Electr. Power Syst. Res. 2015, 129, 75–82. [Google Scholar] [CrossRef] [Green Version]
- Holtsmark, N.; Bahirat, H.J.; Molinas, M.; Mork, B.A.; Hoidalen, H.K. An All-DC offshore wind farm with series-connected turbines: An alternative to the classical parallel AC model? IEEE Trans. Ind. Electron. 2013, 60, 2420–2428. [Google Scholar] [CrossRef]
- Veilleux, E.; Lehn, P.W. Interconnection of Direct-Drive Wind Turbines Using a Series-Connected DC Grid. IEEE Trans. Sustain. Energy 2013, 5, 139–147. [Google Scholar] [CrossRef] [Green Version]
- Bahirat, H.J.; Kjolle, G.H.; Mork, B.A.; Hoidalen, H.K. Reliability assessment of DC wind farms. In Proceedings of the IEEE Power and Energy Society General Meeting, San Diego, CA, USA, 22–26 July 2012; pp. 1–7. [Google Scholar]
- Tatsuta, F.; Nishikata, S. Dynamic performance analysis of a wind turbine generating system with series connected wind generators and bypass diodes using a current source thyristor inverter. In Proceedings of the 2010 International Power Electronics Conference (TPEC2010), Sapporo, Japan, 21–24 June 2010; pp. 1830–1836. [Google Scholar] [CrossRef]
- Nishikata, S.; Tatsuta, F. A New Interconnecting Method for Wind Turbine/Generators in a Wind Farm. IEEE Trans. Ind. Electron. 2010, 57, 468–475. [Google Scholar] [CrossRef]
- Popat, M.; Wu, B.; Liu, F.; Zargari, N. Coordinated Control of Cascaded Current-Source Converter Based Offshore Wind Farm. IEEE Trans. Sustain. Energy 2012, 3, 557–565. [Google Scholar] [CrossRef]
- Garcés, A.; Molinas, M. Coordinated control of series-connected offshore wind park based on matrix converters. Wind Energy 2012, 15, 827–845. [Google Scholar] [CrossRef]
- Rong, F.; Wu, G.; Li, X.; Huang, S.; Zhou, B. ALL-DC Offshore Wind Farm With Series-Connected Wind Turbines to Over-come Unequal Wind Speeds. IEEE Trans. Power Electron. 2019, 34, 1370–1381. [Google Scholar] [CrossRef]
- Bahirat, H.J.; Mork, B.A. Operation of DC Series–Parallel Connected Offshore Wind Farm. IEEE Trans. Sustain. Energy 2018, 10, 596–603. [Google Scholar] [CrossRef]
- Zhang, H.; Gruson, F.; Rodriguez, D.M.F.; Saudemont, C. Overvoltage Limitation Method of an Offshore Wind Farm with DC Series-Parallel Collection Grid. IEEE Trans. Sustain. Energy 2018, 10, 204–213. [Google Scholar] [CrossRef] [Green Version]
- Lundberg, S. Wind Farm Configuration and Energy Efficiency Studies—Series DC versus AC Layouts. Ph.D. Thesis, Chalmers University of Technology, Goteborg, Sweden, 2006. [Google Scholar]
- Chuangpishit, S.; Tabesh, A.; Moradi-Sharbabk, Z.; Saeedifard, M. Topology Design for Collector Systems of Offshore Wind Farms with Pure DC Power Systems. IEEE Trans. Ind. Electron. 2013, 61, 320–328. [Google Scholar] [CrossRef]
- Pape, M.; Kazerani, M. A Generic Power Converter Sizing Framework for Series-Connected DC Offshore Wind Farms. IEEE Trans. Power Electron. 2021, 37, 2307–2320. [Google Scholar] [CrossRef]
- Lakshmanan, P.; Guo, J.; Liang, J. Energy curtailment of DC series–parallel connected offshore wind farms. IET Renew. Power Gener. 2018, 12, 576–584. [Google Scholar] [CrossRef]
- Frandsen, S.; Barthelmie, R.; Pryor, S.; Rathmann, O.; Larsen, S.; Højstrup, J.; Thøgersen, M. Analytical modelling of wind speed deficit in large offshore wind farms. Wind Energy 2006, 9, 39–53. [Google Scholar] [CrossRef]
- Barthelmie, R.J.; Larsen, G.C.; Frandsen, S.T.; Folkerts, L.; Rados, K.; Pryor, S.C.; Lange, B.; Schepers, G. Comparison of Wake Model Simulations with Offshore Wind Turbine Wake Profiles Measured by Sodar. J. Atmos. Ocean. Technol. 2006, 23, 888–901. [Google Scholar] [CrossRef]
- Barthelmie, R.; Frandsen, S.T.; Rathmann, O.; Hansen, K.; Politis, E.S.; Prospathopoulos, J.; Schepers, J.G.; Rados, K.; Cabezón, D.; Schlez, W.; et al. Flow and Wakes in Large Wind Farms: Final Report for Upwind WP8, February 2011. Available online: https://www.osti.gov/etdeweb/servlets/purl/1016226 (accessed on 10 May 2022).
- Guo, J.; Wang, X.; Zhang, Z.; Li, H.; Lakshmanan, P.; Liang, J. Energy curtailment analysis of offshore wind farms with DC series-parallel collection systems. In Proceedings of the DRPT-2015, Changsha, China, 26–29 November 2015. [Google Scholar] [CrossRef]
- Jensen, N.O. A Note on Wind Generator Interaction; Technical Report; Risø National Laboratory: Roskilde, Denmark; p. RISØ-M-24111983.
- Hou, P.; Hu, W.; Soltani, M.; Chen, Z. Optimized Placement of Wind Turbines in Large-Scale Offshore Wind Farm Using Particle Swarm Optimization Algorithm. IEEE Trans. Sustain. Energy 2015, 6, 1272–1282. [Google Scholar] [CrossRef] [Green Version]
- Bak, C.; Zahle, F.; Bitsche, R.; Kim, T.; Yde, A.; Henriksen, L.C.; Hansen, M.H.; Blasques, J.P.A.A.; Gaunaa, M.; Natarajan, A.; et al. Description of the DTU 10 MW Reference Wind Turbine; DTU Wind Energy: Fredericia, Denmark, 2013. [Google Scholar]
- R&D centre Kiel University of Applied Sciences GmbH, FINO3—Research Platform in the North Sea and the Baltic No. 3 [Online]. Available online: http://www.fino3.de/en/ (accessed on 10 May 2022).


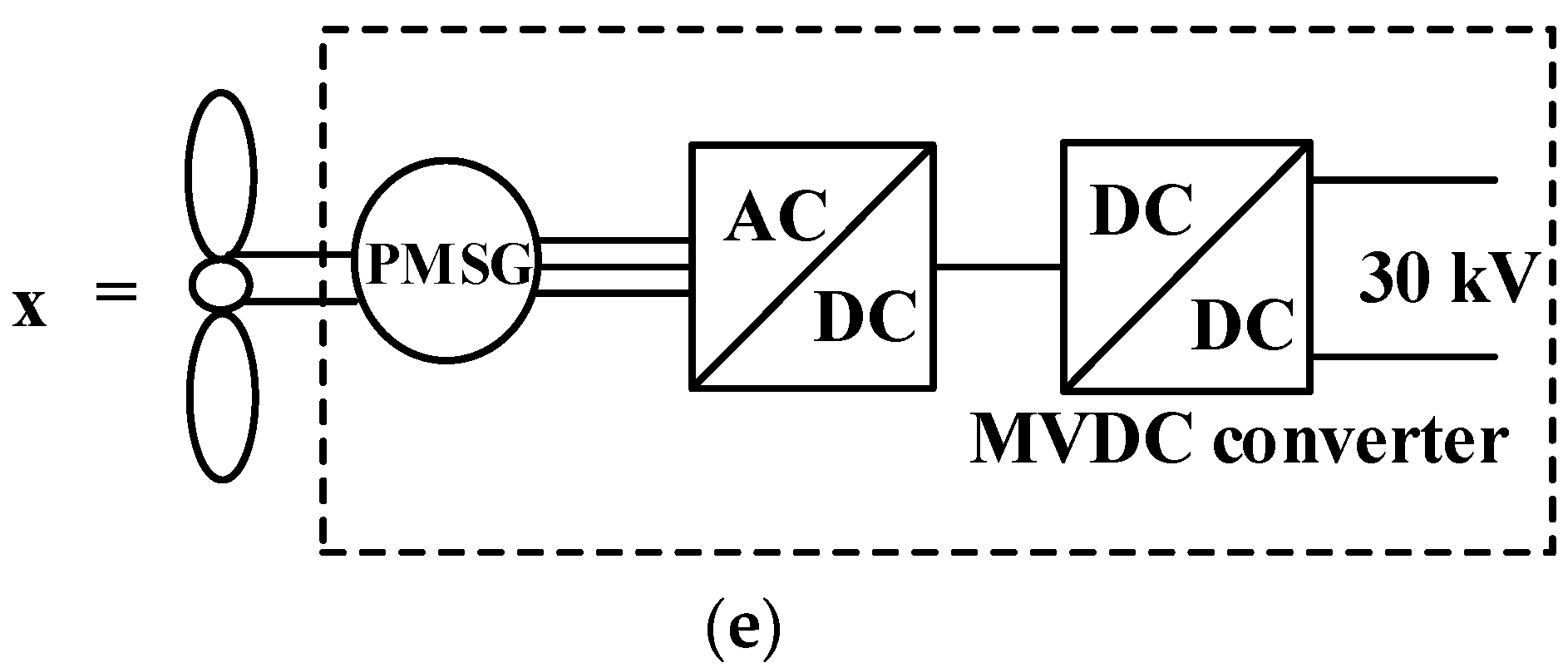
| Parameter | 10 MW DTU Wind Turbine [32,33,34] |
|---|---|
| Cut-in wind speed | 4 m/s |
| Rated wind speed | 11.4 m/s |
| Cut-out wind speed | 25 m/s |
| Rotor diameter | 178.3 m |
| Rated power | 10 MW |
| Cases | 20 × 1 V = 600 kV | 10 × 2 V = 300 kV | 5 × 4 V = 150 kV | 4 × 5 V = 120 kV |
|---|---|---|---|---|
| Upper-voltage tolerance levels of wind turbine MVDC converters Vn (1 + α) | 31.6 kV | 33.3 kV | 37.5 kV | 40 kV |
| α | 5.3% | 11% | 25% | 33.3% |
| Annual Energy Production for a 200 MW DC Wind Farm: 960.02 GWh. | ||||
|---|---|---|---|---|
| Losses in GWh (%) | ||||
| 20 × 1 V = 600 kV | 10 × 2 V = 300 kV | 5 × 4 V = 150 kV | 4 × 5 V = 120 kV | |
| AEC losses with a fixed upper-voltage tolerance level (10%) for wind turbine MVDC converters | 2.7154 (0.28%) | 4.8695 (0.51%) | 16.9492 (1.77%) | 18.7045 (1.95%) |
| AEC losses with redundancy-based upper-voltage tolerance levels for wind turbine MVDC converters | 2.8662 (0.30%) | 4.8089 (0.50%) | 10.5349 (1.10%) | 10.1851 (1.07%) |
| Annual Energy Production for a 200 MW DC Wind Farm: 960.02 GWh. | ||||
|---|---|---|---|---|
| Losses in GWh (%) | ||||
| 20 × 1 V = 600 kV | 10 × 2 V = 300 kV | 5 × 4 V = 150 kV | 4 × 5 V = 120 kV | |
| AEC losses with a fixed upper-voltage tolerance level (10%) for wind turbine MVDC converters | 1.8977 (0.20%) | 3.1880 (0.33%) | 5.4676 (0.57%) | 5.9247 (0.62%) |
| AEC losses with redundancy-based upper-voltage tolerance levels for wind turbine MVDC converters | 2.0557 (0.21%) | 3.1307 (0.33%) | 3.3706 (0.35%) | 2.6947 (0.28%) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lakshmanan, P. Power Curtailment Analysis of DC Series–Parallel Offshore Wind Farms. Wind 2022, 2, 466-478. https://doi.org/10.3390/wind2030025
Lakshmanan P. Power Curtailment Analysis of DC Series–Parallel Offshore Wind Farms. Wind. 2022; 2(3):466-478. https://doi.org/10.3390/wind2030025
Chicago/Turabian StyleLakshmanan, Padmavathi. 2022. "Power Curtailment Analysis of DC Series–Parallel Offshore Wind Farms" Wind 2, no. 3: 466-478. https://doi.org/10.3390/wind2030025
APA StyleLakshmanan, P. (2022). Power Curtailment Analysis of DC Series–Parallel Offshore Wind Farms. Wind, 2(3), 466-478. https://doi.org/10.3390/wind2030025





