Can Deviation from Maximal θ₂₃ Be Resolved in DUNE? ^†

Abstract

Current global analyses of 3$\nu$ oscillation data point towards non-maximal ${\theta }_{23}$, but the maximal value of ${\theta }_{23}={45}^{\circ }$ is still allowed at 3$\sigma$ confidence level. It is expected that DUNE will establish the non-maximal value of ${\theta }_{23}$ at high confidence level if ${\theta }_{23}\ne {45}^{\circ }$ in nature. In this work, we present, in detail, the sensitivity of DUNE to establish the deviation from maximal ${\theta }_{23}$. We find that a 3$\sigma$ (5$\sigma$) determination of non-maximal ${\theta }_{23}$ is possible in DUNE with an exposure of 336 kt·MW·years, if the true value of ${sin}^2{\theta }_{23}\le 0.465\left(0.450\right)$ or ${sin}^2{\theta }_{23}\ge 0.554\left(0.572\right)$ for any value of true ${\delta }_{\mathrm{CP}}$ and true choice of normal mass ordering. We also discuss the extent of individual contributions from appearance and disappearance channels and the impacts of systematic uncertainties and total exposure, while addressing the discovery of non-maximal of ${\theta }_{23}$ in DUNE.

1. Introduction

Present 3$\nu$ global oscillation data favor normal mass ordering (NMO) at 2.5$\sigma$ and provide hints for lower octant of atmospheric mixing angle (${\theta }_{23}$) and leptonic CP violation at around 90% confidence level [1]. In this work, we explore the capabilities of the upcoming high-precision long-baseline experiment DUNE [2] to establish the non-maximal ${\theta }_{23}$ using an on-axis, wide-band neutrino beam with most of its flux peaking around 2.5 GeV. DUNE is enriched with substantial Earth’s matter effect, having a baseline of 1285 km with a line-averaged constant Earth matter density (${\rho }_{\mathrm{avg}}$) of 2.848 g/cm${}^3$. To simulate our results, we make use of the publicly available GLoBES software [3]. For generating our prospective data, we use the following benchmark values of oscillation parameters from Ref. [1]: ${sin}^2{\theta }_{12}=0.303,{sin}^2{\theta }_{13}=0.0223,{sin}^2{\theta }_{23}=0.455,\Delta m_{31}^2=2.522\times {10}^{-3}{\mathrm{eV}}^2,\Delta m_{21}^2=7.36\times {10}^{-5}{\mathrm{eV}}^2,\mathrm{and}{\delta }_{\mathrm{CP}}={223}^{\circ }$, assuming NMO.

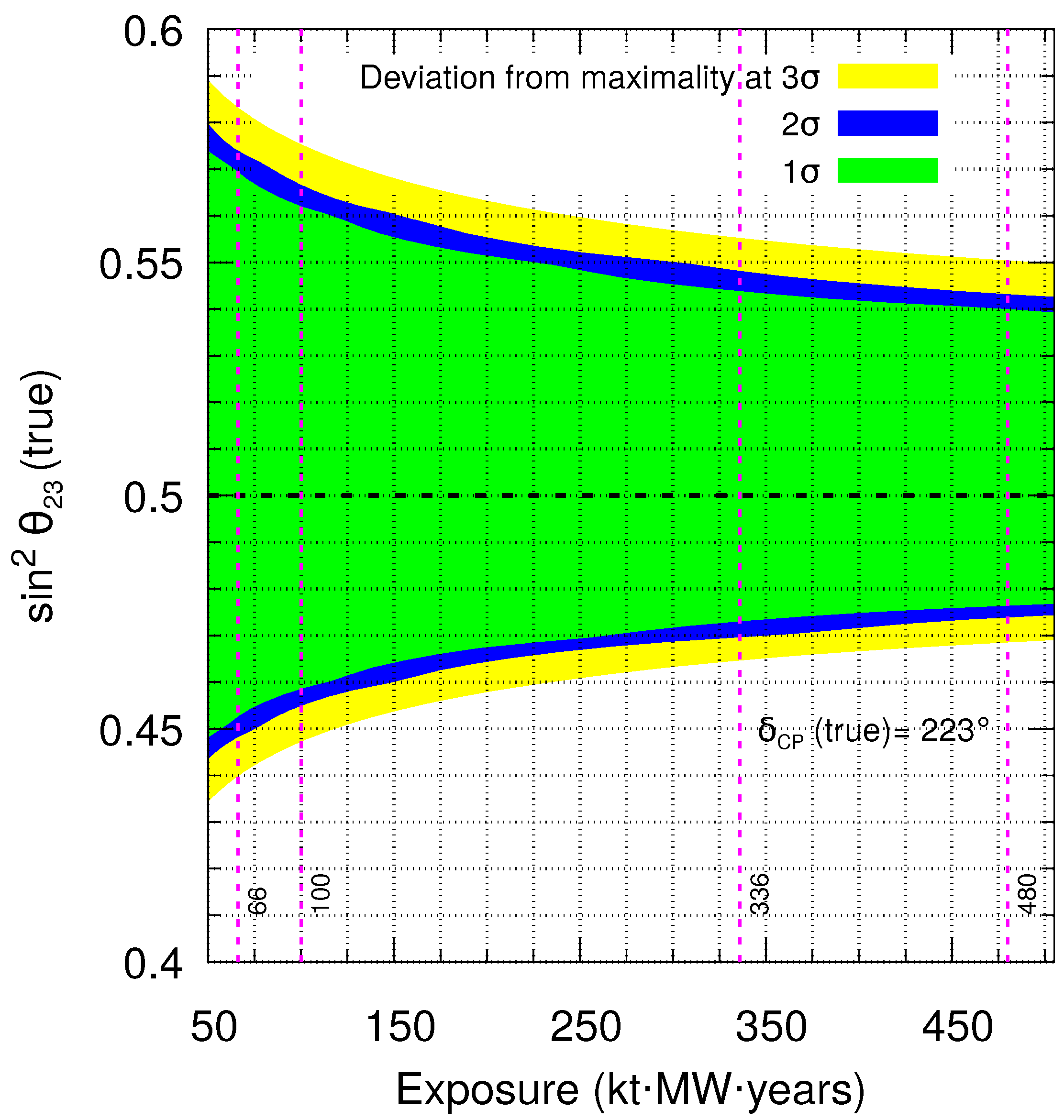
2. Results and Discussions

To estimate the median sensitivities in the frequentist approach, we use the Poissonian ${\chi }^2$ [4]. For estimating the sensitivity towards establishing deviation from non-maximal ${\theta }_{23}$ in DUNE, we define the following:

$$\Delta {\chi }_{\mathrm{DM}}^2=\underset{(\overrightarrow{\lambda },{\kappa }_s,{\kappa }_b)}{\min }\left\{{\chi }^2\left({sin}^2{\theta }_{23}^{\mathrm{true}}\in [0.4,0.6]\right)-{\chi }^2\left({sin}^2{\theta }_{23}^{\mathrm{test}}=0.5\right)\right\},$$

(1)

where $\overrightarrow{\lambda }=\{{\delta }_{\mathrm{CP}},\Delta m_{31}^2\}$ is the set of oscillation parameters over which we marginalize within their current 3$\sigma$ allowed ranges (as mentioned in Ref. [1]). Here, ${\kappa }_s\mathrm{and}{\kappa }_b$ are the respective systematic pulls on signal and background. In Figure 1, we show the sensitivity of DUNE in establishing deviation from non-maximal ${\theta }_{23}$ using an exposure of 336 kt·MW·years. Figure 1 depicts that, for the present best-fit values of oscillation parameters (assuming NMO), DUNE can establish deviation from non-maximal ${\theta }_{23}$ at 4.2$\sigma$ C.L., assuming a total run-time of 7 years, equally divided in both neutrino and antineutrino modes. Furthermore, we observe that a 3$\sigma$ (5$\sigma$) determination of non-maximal ${\theta }_{23}$ is possible in DUNE if the true value of ${sin}^2{\theta }_{23}\le 0.465\left(0.450\right)$ or ${sin}^2{\theta }_{23}\ge 0.554\left(0.572\right)$, irrespective of any value of true ${\delta }_{\mathrm{CP}}$. The narrow width of the red-colored band in Figure 1 shows that this sensitivity hardly depends on the choice of true ${\delta }_{\mathrm{CP}}$. This is because the contribution from the disappearance channel dominates while establishing deviation from maximal ${\theta }_{23}$ (see Figure 2), and this channel is almost independent of ${\delta }_{\mathrm{CP}}$ [5].

Figure 2 portrays the individual contributions from appearance (blue curves), disappearance (red curves), and their combination (black curve) as a function of true ${sin}^2{\theta }_{23}$ in establishing non-maximal ${\theta }_{23}$. Note that, when ${sin}^2{\theta }_{23}$ lies in higher octant but remains close to maximal mixing, the appearance channel provides better sensitivity than the disappearance channel in excluding maximal mixing solution. However, for ${sin}^2{\theta }_{23}\ge 0.56$, the disappearance channel dominates, causing a sharp rise in total $\Delta {\chi }_{\mathrm{DM}}^2$. Throughout the lower octant, the disappearance channel contributes the most towards the sensitivity. The solid and dashed curves depict the sensitivities with and without systematic uncertainties, suggesting that both the channels are affected due to the systematic errors in DUNE. In

Table 1. $\Delta {\chi }_{\mathrm{DM}}^2$ for various choices of normalization uncertainties on appearance and disappearance events: [(2%, 5%), (0%, 0%), (5%, 5%), (5%, 10%), and (10%, 10%)], where (2%, 5%) is the nominal choice [2]. Results are shown for ${sin}^2{\theta }_{23}$ (true) = 0.455 (current best-fit) and ${sin}^2{\theta }_{23}$ (true) = 0.473 (current $1\sigma$ upper bound), assuming true NMO and ${\delta }_{\mathrm{CP}}$ (true) = 223${}^{\circ }$ with 336 kt·MW·years of exposure. This table is from Ref. [6].

True ${sin}^2{\mathit{\theta }}_{23}$	Channels	2, 5%	0, 0%	5, 5%	5, 10%	10, 10%
	App. + Disapp.	$\mathbf{17.64}$	$\mathbf{24.13}$	$\mathbf{16.88}$	$\mathbf{16.74}$	$\mathbf{15.42}$
$\mathbf{0.455}$	App.	$\mathbf{3.52}$	$\mathbf{4.05}$	$\mathbf{2.33}$	$\mathbf{2.33}$	$\mathbf{1.05}$
(Best-fit)	Disapp.	$\mathbf{14.31}$	$\mathbf{18.79}$	$\mathbf{14.31}$	$\mathbf{14.16}$	$\mathbf{14.16}$
	App. + Disapp.	$\mathbf{4.28}$	$\mathbf{5.72}$	$\mathbf{3.88}$	$\mathbf{3.84}$	$\mathbf{3.42}$
$\mathbf{0.473}$	App.	$\mathbf{1.27}$	$\mathbf{1.47}$	$\mathbf{0.84}$	$\mathbf{0.84}$	$\mathbf{0.38}$
($1\sigma$ upper bound)	Disapp.	$\mathbf{2.99}$	$\mathbf{3.88}$	$\mathbf{2.99}$	$\mathbf{2.97}$	$\mathbf{2.97}$

, we quantify the sensitivity towards non-maximal ${\theta }_{23}$ considering five different sets of normalization uncertainties on appearance and disappearance events, keeping the systematic errors in various backgrounds fixed at their nominal values. We observe that the sensitivity from the disappearance channel is deteriorated more than the appearance channel when we transition from a scenario with no systematic uncertainties $(0\%,0\%)$ to their nominal values $(2\%,5\%)$. Nevertheless, if we increase the uncertainties further, then the deterioration in the sensitivity is quite substantial in the appearance channel, while the disappearance channel is hardly affected. Since, in this study, disappearance is the primary contributing channel, we expect, therefore, that the performance of DUNE in establishing non-maximal ${\theta }_{23}$ will remain mostly unaffected if the systematic uncertainties are enhanced in DUNE.

In Figure 3, we depict the true values of ${sin}^2{\theta }_{23}$ that DUNE can distinguish from ${sin}^2{\theta }_{23}^{\mathrm{test}}=0.5$ as a function of total exposure. With the proposed setup, we expect a significant improvement in the discovery of a non-maximal ${\theta }_{23}$ when exposure increases from 50 kt·MW·years to 100 kt·MW·years. Further, increasing the total exposure to its benchmark value of 336 kt·MW·years shows only marginal improvement. Any further increase in the statistics, does not result in much improvement in the sensitivity.

3. Conclusions

We explore, in detail, the performance of DUNE in establishing deviation from non-maximal ${\theta }_{23}$, which plays a crucial role in constructing neutrino mass models, addressing the issue of leptonic CP violation, and performing precision measurement of other oscillation parameters. In light of the global fit of the current oscillation data assuming NMO, we find that DUNE can exclude ${sin}^2{\theta }_{23}=0.5$ at 4.2$\sigma$ with 336 kt·MW·years of exposure. This sensitivity can further deteriorate to 2$\sigma$ or enhance to 6.5$\sigma$, depending upon the true value of ${sin}^2{\theta }_{23}$ in its current 1$\sigma$ upper (0.47) and lower (0.44) bounds, respectively. We further observe that the disappearance channel plays a significant role and the impact of systematic uncertainties is minimal in this study.