Neutrino Mass Ordering with IceCube DeepCore ^†

Abstract

The neutrino mass ordering (NMO) is one of the last undetermined properties in the three-neutrino paradigm. NMO studies aim to answer the question of whether the neutrino mass ordering is normal ($m_3>m_2>m_1$) or inverted ($m_2>m_1>m_3$). We conduct a study of the NMO sensitivity with atmospheric neutrinos using 9.3 years of IceCube DeepCore data, where a new event selection, reconstruction method, particle identification, and systematic uncertainty modeling are used. The goals of this analysis consist of: (1) probing the NMO at neutrino baselines that are not accessible to long-baseline accelerator experiments, (2) contributing to NMO global fit studies in an important and unique way, (3) serving as a detailed study on the NMO in preparation for the upcoming IceCube Upgrade, which should significantly improve the DeepCore NMO sensitivity.

1. Introduction

The neutrino mass ordering (NMO) is one of the last open questions that are left in the three-neutrino paradigm. NMO analyses look for the true ordering of the three neutrino mass states: ${\nu }_1$, ${\nu }_2$, and ${\nu }_3$. Specifically, these analyses look to answer whether the mass ordering is normal ($m_3>m_2>m_1$) or inverted ($m_2>m_1>m_3$), and as such, determine the sign of $\Delta m_{31}^2=m_3^2-m_1^2$, commonly referred to as the mass splitting, as shown in Figure 1. Neutrino mass states are related to neutrino flavor states through the Pontecorvo–Maki–Nakagawa–Sakata (PMNS) matrix, so that

$$|{\nu }_{\alpha }\rangle =\sum _i^{}{U}_{\alpha i}|{\nu }_i\rangle$$

(1)

where $\alpha$ ∈ $\left\{e,\mu ,\tau \right\}$ and i ∈ $\left\{1,2,3\right\}$. The PMNS matrix, U, is parameterized by three mixing angles, ${\theta }_{12},{\theta }_{13},$ and ${\theta }_{23}$, and one CP-violating phase, ${\delta }_{\mathrm{CP}}$.

As neutrinos propagate, mass states with differing masses will propagate with different velocities, causing interference among the flavor states. This allows the detection of a different flavor state than the one originally produced. This phenomenon is called neutrino oscillations. The oscillation probability is

$$\begin{array}{cc}\hfill P_{\alpha \to \beta }={\delta }_{\alpha \beta }& - 4\sum _{i>j}Re\left[U_{\alpha i}^{*}{U}_{\beta i}{U}_{\alpha j}{U}_{\beta j}^{*}\right]{sin}^2\left(\frac{\Delta m_{ij}^{2}L}{4E_{\nu }}\right)\hfill \\ & + 2\sum _{i>j}Im\left[U_{\alpha i}^{*}{U}_{\beta i}{U}_{\alpha j}{U}_{\beta j}^{*}\right]sin\left(\frac{\Delta m_{ij}^{2}L}{2E_{\nu }}\right)\hfill \end{array}$$

(2)

where L and $E_{\nu }$ are the propagation length and energy of the neutrino, respectively, $\Delta m_{ij}^2$ is the mass splitting for mass states i and j, and $\alpha$ and $\beta$ are different neutrino flavors.

Neutrino oscillations were discovered in 1998 by Super-Kamiokande [2] and later confirmed in 2001 by the Sudbury Neutrino Observatory (SNO) [3], showing evidence for physics beyond the standard model. With the discovery of neutrino oscillations, the ordering of the neutrino masses became a crucial piece of the neutrino puzzle as most of the currently open questions regarding neutrinos depend greatly on whether the mass ordering is normal or inverted. Presently, the combined global fit for the NMO, obtained by combining neutrino oscillation data from various experiments, prefers the normal ordering (NO) over the inverted ordering (IO) by 1.6–2.6$\sigma$ [1].

The IceCube Neutrino Observatory is an ice-Cherenkov neutrino detector located at the South Pole. It consists of a lattice of 5160 digital optical module photosensors (DOMs) embedded in Antarctic ice greater than 1.5 km below the surface [4]. Neutrinos interact with the ice via weak charged-current and neutral-current interactions and produce charged particles that emit detectable Cherenkov radiation. The DeepCore subarray is the more densely instrumented region of IceCube [5]. It was built for the detection of neutrino events with energies ranging from about 5 GeV to 300 GeV.

At energies below about 15 GeV, atmospheric neutrinos, muon-flavored and electron-flavored neutrinos from cosmic ray interactions, exhibit distortions in their oscillation probabilities when they traverse the core of the Earth. This is due to coherent forward scattering of neutrinos with electrons in matter, a process called the Mikheyev–Smirnov–Wolfenstein (MSW) effect. As can be seen in Figure 2, Earth matter effects introduce differences in the oscillation probabilities such that, for a true NO, matter effects manifest for neutrinos, while for a true IO, they manifest for anti-neutrinos. Because DeepCore cannot distinguish between neutrinos and anti-neutrinos, the signal cannot be found simply by looking at whether these differences are coming from either the neutrino or anti-neutrino channel. Therefore, we rely on both the atmospheric neutrino flux and the neutrino-nucleon cross-section, which are greater for neutrinos than anti-neutrinos, to determine the NMO by observing more pronounced Earth matter effects for the NO than for the IO.

2. Materials and Methods

For this study, we use a Monte-Carlo-simulated event sample for 9.3 years of IceCube DeepCore data (about 145,000 more events as compared to previous DeepCore studies [8]) with an improved signal to background ratio, where the background in DeepCore consists mainly of atmospheric muons and random noise. The sample starts off with about three orders of magnitude more background than neutrino signal. Boosted decision tree (BDT) classifiers are used for both muon and noise background rejection, followed by more cuts on muon events that pass the veto region undetected and fiducial volume containment cuts. The final level event rates of the sample can be seen in

Table 1. Final level rates of each event type in the DeepCore sample.

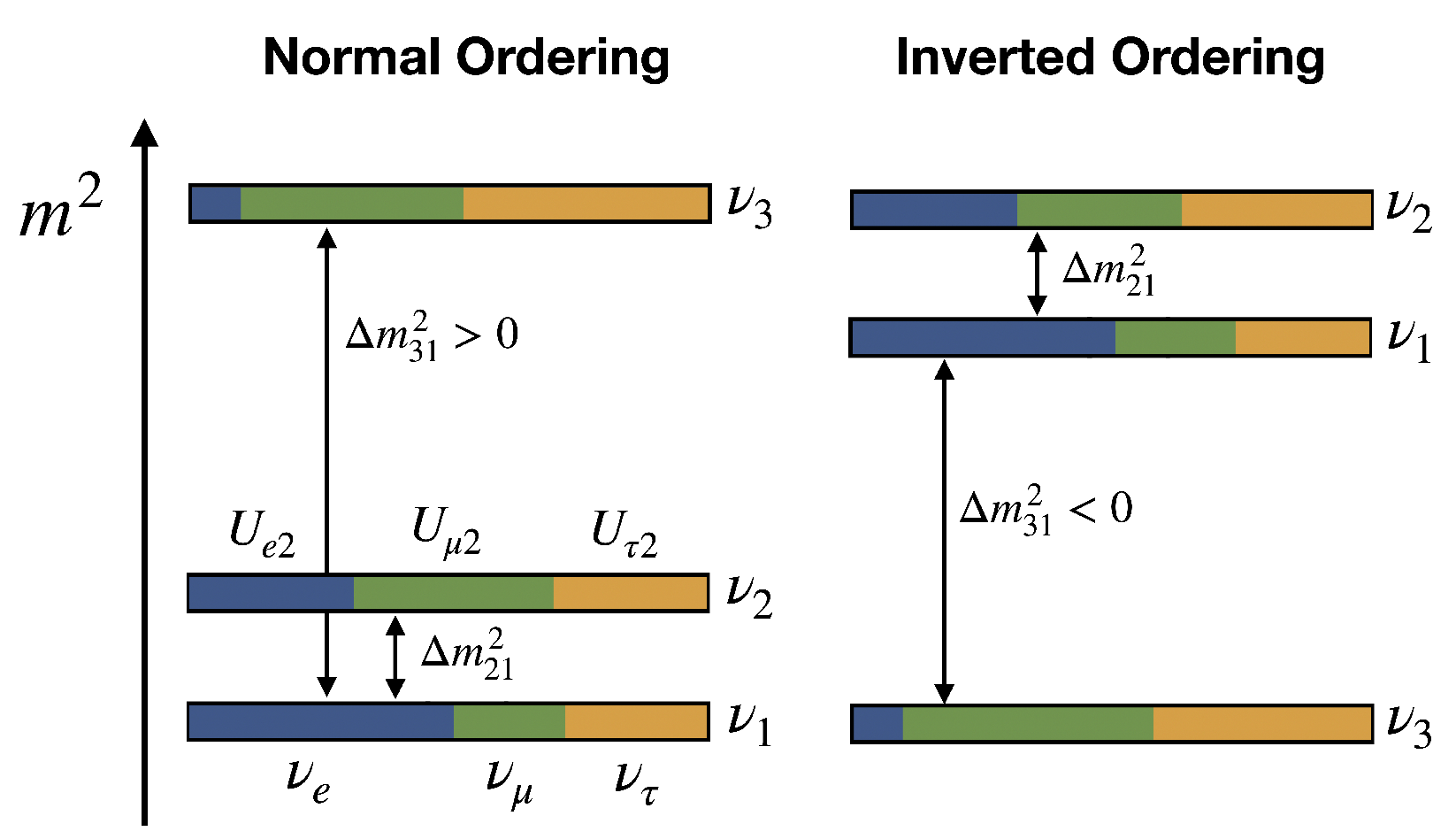
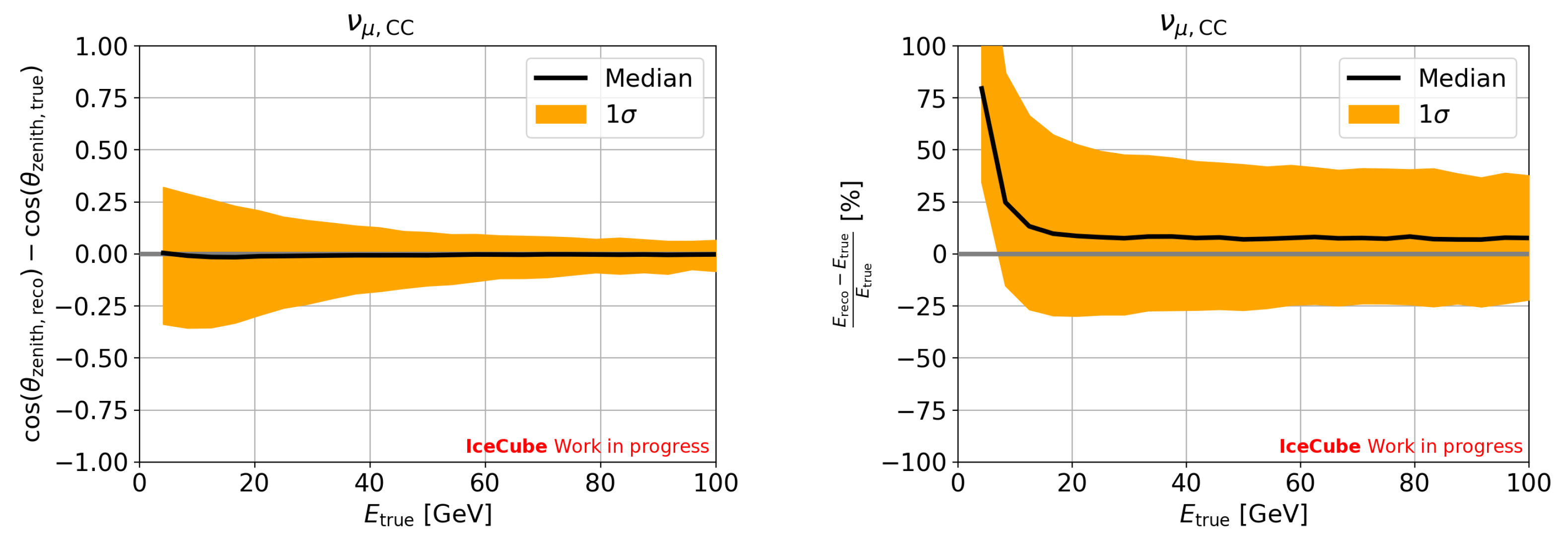
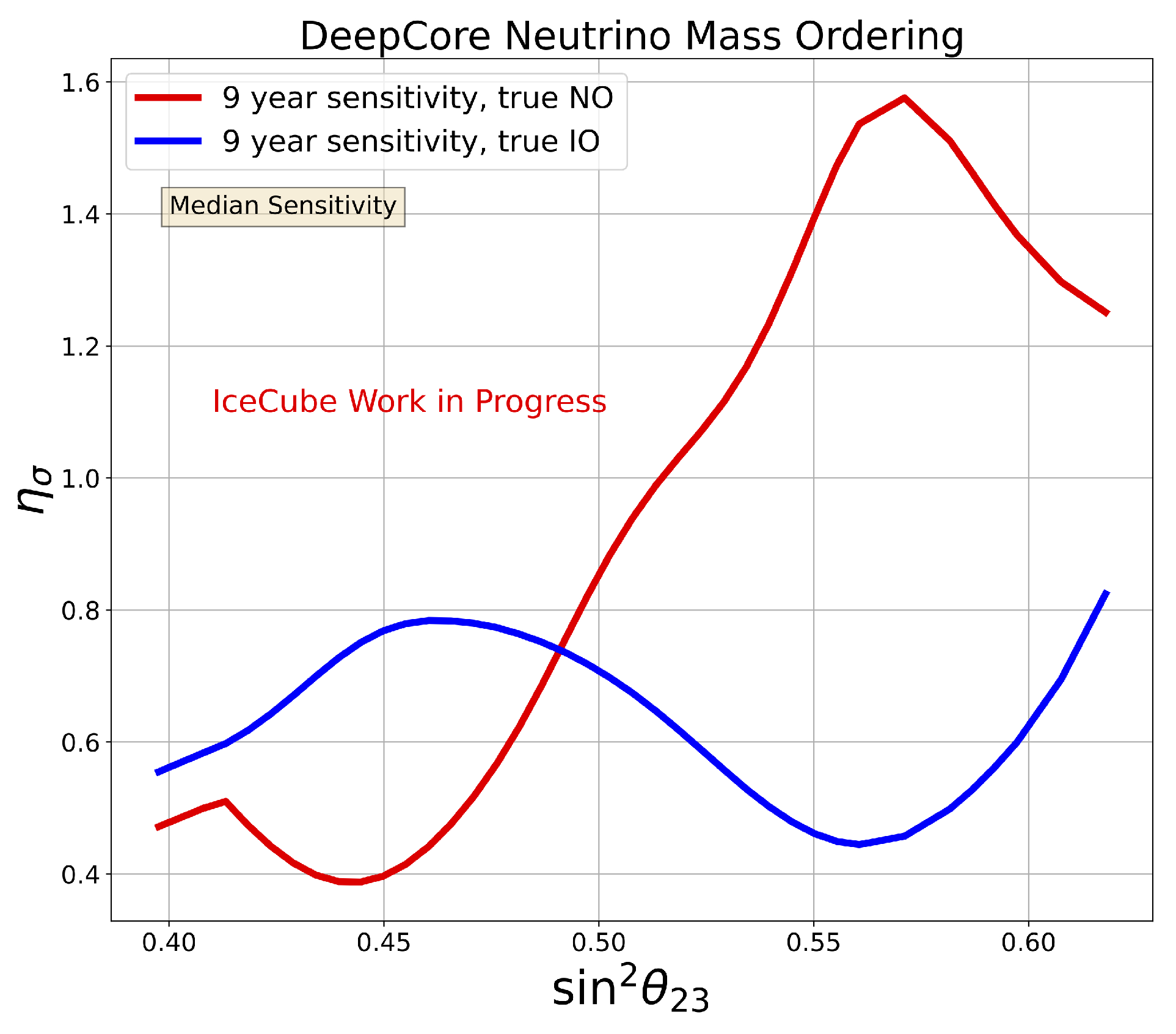
Type	Rate [mHz]	Num Events [9.3 yr]	% of Sample
${\nu }_e\mathrm{CC}$	0.162	47,541 ± 73	23.0
${\nu }_{\mu }\mathrm{CC}$	0.432	126,411 ± 126	61.1
${\nu }_{\tau }\mathrm{CC}$	0.032	9510 ± 21	4.6
$\nu \mathrm{NC}$	0.075	21,966 ± 50	10.6
${\mu }_{\mathrm{atm}}$	0.005	1463 ± 87	0.7
Total	0.707	206,894 ± 179	-

.

Figure 3 shows the reconstruction resolution for ${\nu }_{\mu }CC$ events, which dominate the DeepCore signal as can be seen in Figure 1, as a function of the true neutrino energy [9]. Since the NMO sensitivity for DeepCore lies at energies below 15 GeV, reconstruction is the main challenge in this analysis as it proves difficult to precisely reconstruct the neutrino energy in this region. Finally, a BDT classifier is applied to distinguish ${\nu }_{\mu }CC$ events, which produce a different event topology from all other events. We perform this classification to prevent further dilution of the signal between the different neutrino flavors.

The statistical uncertainties from limited Monte Carlo production are found to have a negligible impact. The systematic uncertainties include neutrino flux, cross-section, and detection-related uncertainties. For the cosmic ray neutrino flux uncertainties, we use the Honda flux model [10] as our baseline flux model with the MCEq scheme for greater model flexibility [11]. We use the Barr parametrization [12] for our systematic parameters in the analysis to account for pion and kaon production uncertainties. For neutrino cross-section uncertainties, we assign one systematic parameter for deep inelastic scattering to account for the disagreement between CSMS [13] and GENIE [14]. We also include systematic parameters for the axial mass of resonant and quasi-elastic charged-current interactions. For detector-related systematics, we use parameters for the photon ice scattering and absorption as well as for the optical efficiency of the photomultiplier tubes (PMTs) located in the DOMs. The parametrization of detector systematics is performed separately for every bin in the event sample distribution. By comparing corresponding bin counts for multiple simulation sets with differing detector systematic properties, we are able to perform a linear fit per bin to obtain a re-weighting factor for any given detector systematic parameter value.

3. Results

Figure 4 shows the projected DeepCore median NMO sensitivity [15] using 9.3 years of data as a function of the ${\theta }_{23}$ mixing angle. A few key observations that can be made from this plot are that the upper ${\theta }_{23}$ octant is most favorable for resolving the ordering provided that the NO is true. Furthermore, the lower ${\theta }_{23}$ octant is most favorable for resolving the ordering provided the IO is true. The characteristic sensitivity shape that is seen can be attributed to the ${\theta }_{23}$ octant dependence in the full three-flavor oscillation probability in the presence of matter effects with a combined neutrino–antineutrino weighted average. Specifically, the kink that is created near ${sin}^2\left({\theta }_{23}\right)=0.42$ for a true NO appears due to the well-known ${\theta }_{23}$-octant mass-ordering degeneracy in neutrino oscillation probabilities [16]. The disappearance channel alone cannot break this degeneracy because the leading term in the muon neutrino disappearance channel is proportional to ${sin}^2\left(2{\theta }_{23}\right)$. However, this degeneracy is partially broken when you take the appearance channel into account. Therefore, the farther away from maximal, the easier it becomes to break this degeneracy.

4. Discussion

DeepCore has opened up a new window in neutrino oscillations with its ability to look at neutrinos traversing the core of the Earth with energies ranging from a few GeV to hundreds of GeV. Furthermore, we are now at the stage of analyzing 9.3 years of detector data, which makes for robust analyses due to the increase in DeepCore’s statistical power with over 200,000 atmospheric neutrino events. Therefore, the current DeepCore NMO analysis can play an important role in the NMO global fit studies.

Looking ahead, it should also be noted that synergistic effects between DeepCore and the Jiangmen Underground Neutrino Observatory (JUNO) experiment [17] can provide an enhancement in the NMO signal. JUNO will be a multi-purpose neutrino observatory in southeast China. It will rely on the precise determination of sub-leading terms in the oscillation probability of reactor anti-neutrinos to determine the NMO. By performing a joint fit, synergistic effects are observed for the combined sensitivity when performing a fit of the NO to a true IO or of the IO to a true NO. These synergistic effects have been previously studied specifically in the context of a combined fit with the IceCube Upgrade and JUNO [18,19,20].

The IceCube Upgrade is the upcoming fully funded extension of DeepCore, adding seven more strings with tighter module spacing [21]. The upgrade is expected to detect neutrinos down to an energy of about 1 GeV as well as significantly improve the resolution of events at the higher energies. This will especially improve the NMO analysis where the signal region spans between 2.5 GeV and 15 GeV.