Differentially Rotating Relativistic Stars beyond the J-Constant Law †
Abstract
:1. Introduction
2. Methods
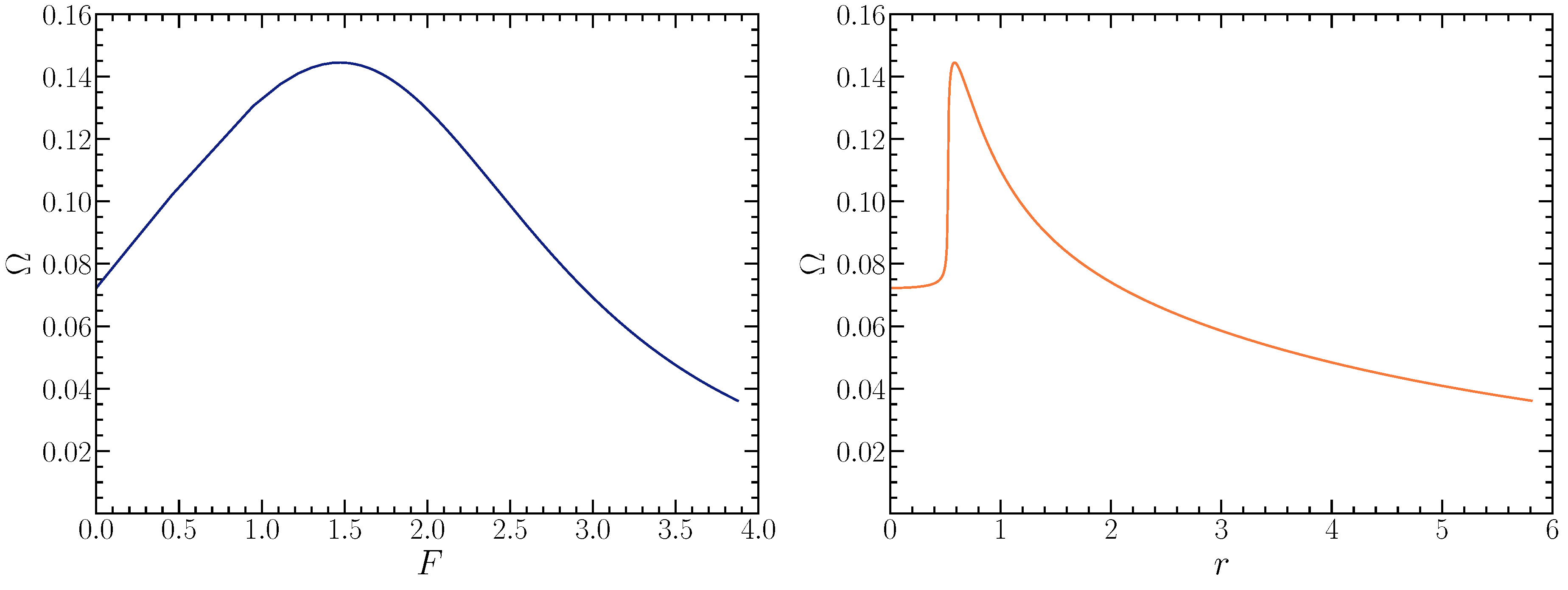
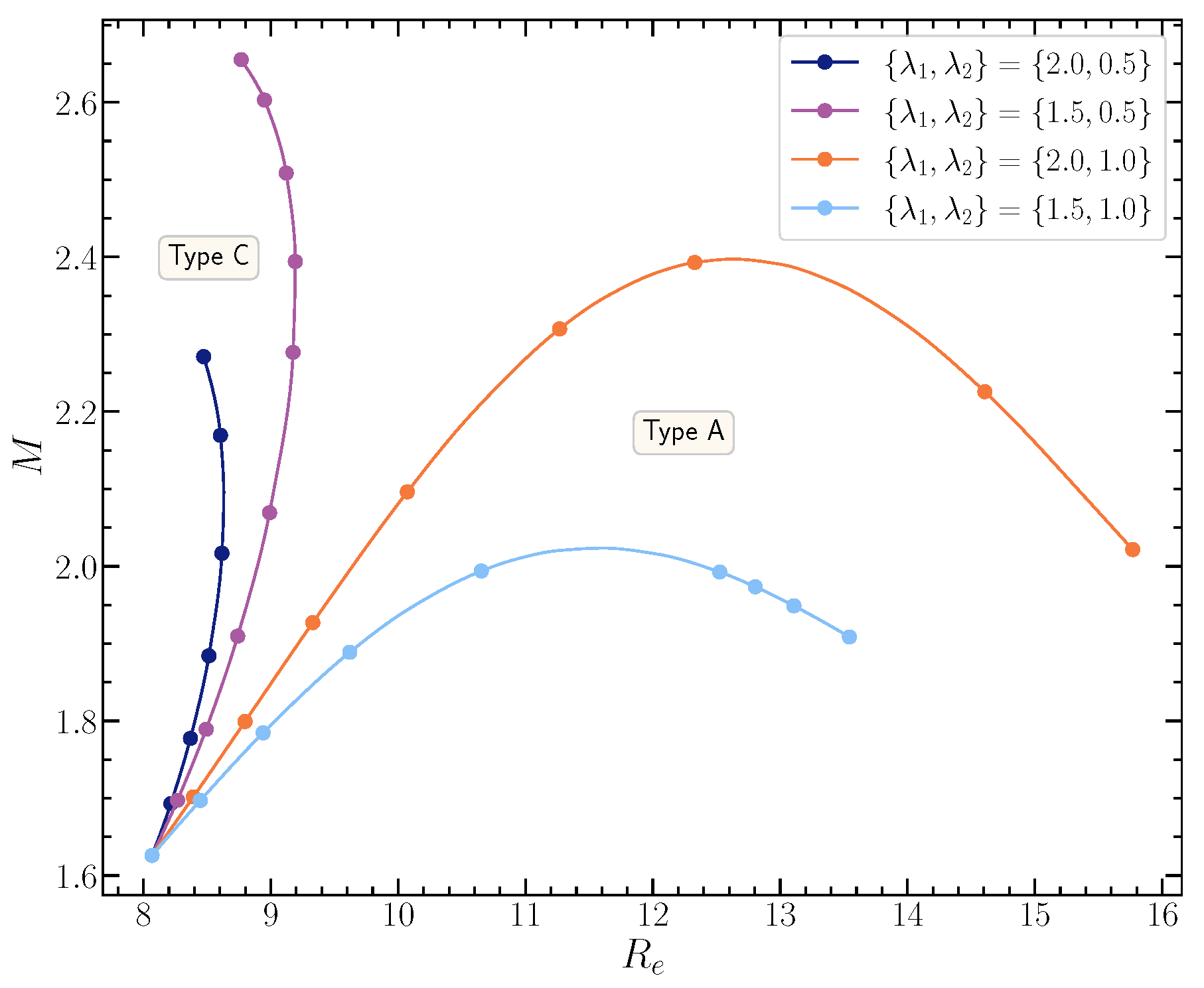
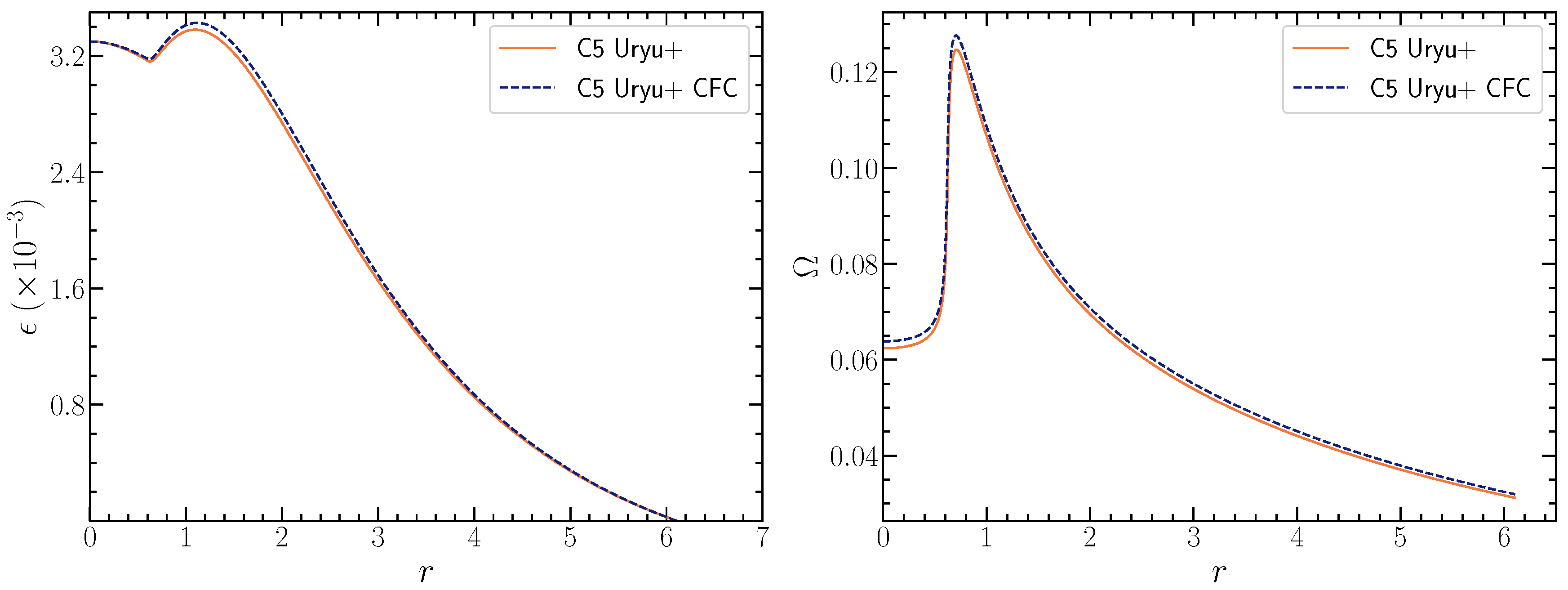
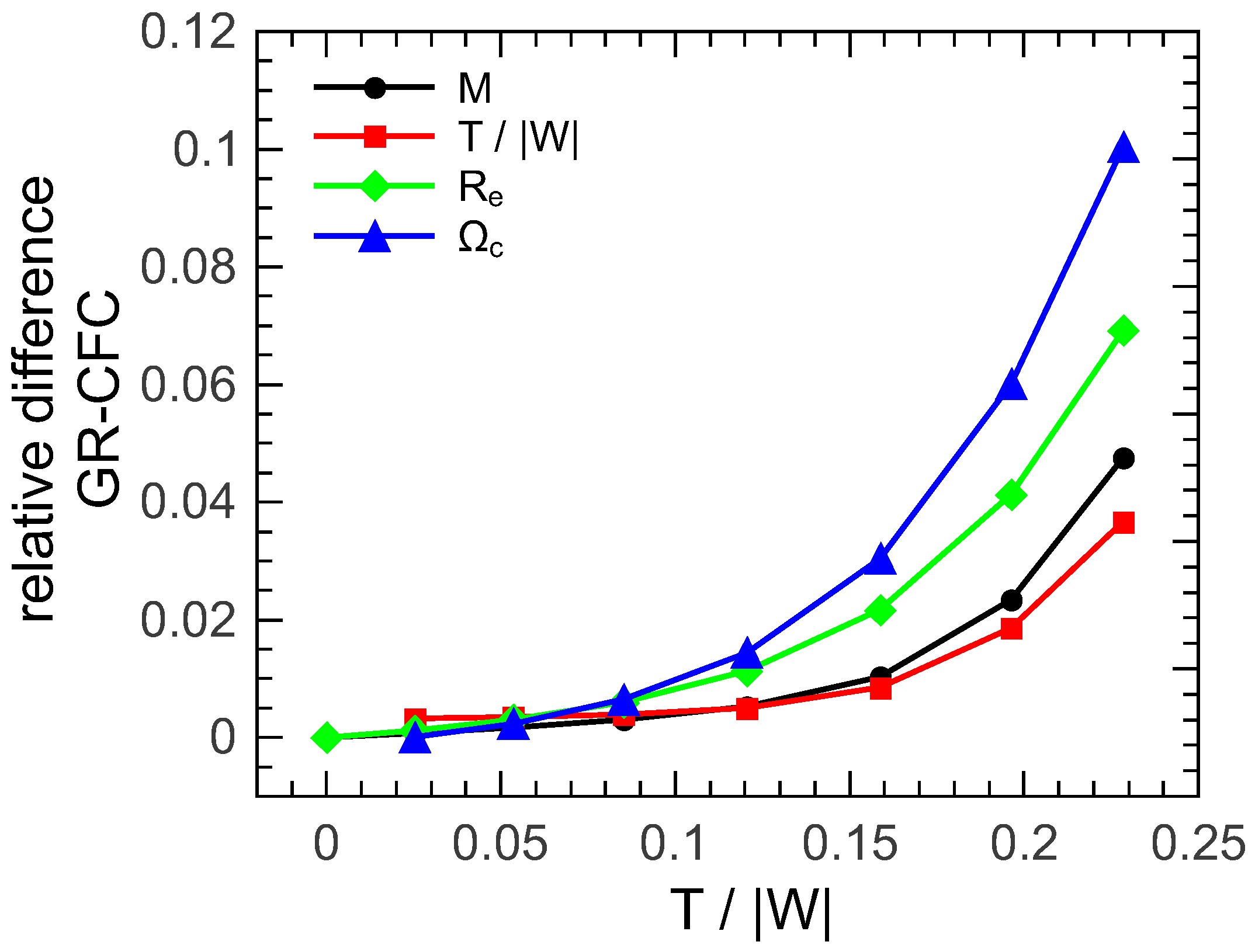
3. Results
- Sequence A is a constant rest mass sequence with .
- Sequence B is a constant central energy density sequence with .
- Sequence C is a constant central energy density sequence with .
4. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| KEH | Komatsu, Eriguchi and Hachisu |
| EOS | equation of state |
| BNS | binary neutron star |
| IWM | Isenberg, Wilson, Mathews |
| CFC | conformal flatness condition |
| GR | general relativity |
References
- Stergioulas, N.; Bauswein, A.; Zagkouris, K.; Janka, H.T. Gravitational waves and non-axisymmetric oscillation modes in mergers of compact object binaries. Mon. Not. R. Astron. Soc. 2011, 418, 427–436. [Google Scholar] [CrossRef] [Green Version]
- Bauswein, A.; Stergioulas, N. Unified picture of the post-merger dynamics and gravitational wave emission in neutron star mergers. Phys. Rev. D 2015, 91, 124056. [Google Scholar] [CrossRef] [Green Version]
- Bauswein, A.; Stergioulas, N.; Janka, H.T. Exploring properties of high-density matter through remnants of neutron-star mergers. Eur. Phys. J. A 2016, 52, 56. [Google Scholar] [CrossRef]
- Bauswein, A.; Stergioulas, N. Spectral classification of gravitational-wave emission and equation of state constraints in binary neutron star mergers. J. Phys. G Nucl. Part. Phys. 2019, 46, 113002. [Google Scholar] [CrossRef] [Green Version]
- Komatsu, H.; Eriguchi, Y.; Hachisu, I. Rapidly rotating general relativistic stars. I - Numerical method and its application to uniformly rotating polytropes. Mon. Not. R. Astron. Soc. 1989, 237, 355–379. [Google Scholar] [CrossRef]
- Uryū, K.; Tsokaros, A.; Baiotti, L.; Galeazzi, F.; Taniguchi, K.; Yoshida, S. Modeling differential rotations of compact stars in equilibriums. Phys. Rev. D 2017, 96, 103011. [Google Scholar] [CrossRef] [Green Version]
- De Pietri, R.; Feo, A.; Font, J.A.; Löffler, F.; Pasquali, M.; Stergioulas, N. Numerical-relativity simulations of long-lived remnants of binary neutron star mergers. Phys. Rev. D 2020, 101, 064052. [Google Scholar] [CrossRef] [Green Version]
- Iosif, P.; Stergioulas, N. Equilibrium sequences of differentially rotating stars with post-merger-like rotational profiles. arXiv 2020, arXiv:gr-qc/2011.10612. [Google Scholar]
- Friedman, J.L.; Stergioulas, N. Rotating Relativistic Stars; Cambridge Monographs on Mathematical Physics, Cambridge University Press: Cambridge, UK, 2013. [Google Scholar] [CrossRef] [Green Version]
- Cook, G.B.; Shapiro, S.L.; Teukolsky, S.A. Spin-up of a Rapidly Rotating Star by Angular Momentum Loss: Effects of General Relativity. Astrophys. J. 1992, 398, 203. [Google Scholar] [CrossRef]
- Stergioulas, N.; Apostolatos, T.A.; Font, J.A. Non-linear pulsations in differentially rotating neutron stars: Mass-shedding-induced damping and splitting of the fundamental mode. Mon. Not. R. Astron. Soc. 2004, 352, 1089–1101. [Google Scholar] [CrossRef] [Green Version]
- Bauswein, A.; Stergioulas, N. Semi-analytic derivation of the threshold mass for prompt collapse in binary neutron-star mergers. Mon. Not. R. Astron. Soc. 2017, 471, 4956–4965. [Google Scholar] [CrossRef] [Green Version]
- Stergioulas, N.; Friedman, J.L. Comparing Models of Rapidly Rotating Relativistic Stars Constructed by Two Numerical Methods. Astrophys. J. 1995, 444, 306. [Google Scholar] [CrossRef]
- Stergioulas, N. RNS Public Domain Code. Available online: http://www.gravity.phys.uwm.edu/rns (accessed on 1st April 2020).
- Zhou, E.; Tsokaros, A.; Uryū, K.; Xu, R.; Shibata, M. Differentially rotating strange star in general relativity. Phys. Rev. D 2019, 100, 043015. [Google Scholar] [CrossRef] [Green Version]
- Hanauske, M.; Takami, K.; Bovard, L.; Rezzolla, L.; Font, J.A.; Galeazzi, F.; Stöcker, H. Rotational properties of hypermassive neutron stars from binary mergers. Phys. Rev. D 2017, 96, 043004. [Google Scholar] [CrossRef] [Green Version]
- Iosif, P.; Stergioulas, N. On the accuracy of the IWM–CFC approximation in differentially rotating relativistic stars. Gen. Relativ. Gravit. 2014, 46, 1800. [Google Scholar] [CrossRef] [Green Version]
- Ansorg, M.; Gondek-Rosińska, D.; Villain, L. On the solution space of differentially rotating neutron stars in general relativity. Mon. Not. R. Astron. Soc. 2009, 396, 2359–2366. [Google Scholar] [CrossRef] [Green Version]
- Isenberg, J.A. Waveless Approximation Theories of Gravity. Int. J. Mod. Phys. D 2008, 17, 265–273. [Google Scholar] [CrossRef] [Green Version]
- Wilson, J.R.; Mathews, G.J.; Marronetti, P. Relativistic numerical model for close neutron-star binaries. Phys. Rev. D 1996, 54, 1317–1331. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vretinaris, S.; Stergioulas, N.; Bauswein, A. Empirical relations for gravitational-wave asteroseismology of binary neutron star mergers. Phys. Rev. D 2020, 101, 084039. [Google Scholar] [CrossRef] [Green Version]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iosif, P.; Stergioulas, N. Differentially Rotating Relativistic Stars beyond the J-Constant Law. Phys. Sci. Forum 2021, 2, 62. https://doi.org/10.3390/ECU2021-09312
Iosif P, Stergioulas N. Differentially Rotating Relativistic Stars beyond the J-Constant Law. Physical Sciences Forum. 2021; 2(1):62. https://doi.org/10.3390/ECU2021-09312
Chicago/Turabian StyleIosif, Panagiotis, and Nikolaos Stergioulas. 2021. "Differentially Rotating Relativistic Stars beyond the J-Constant Law" Physical Sciences Forum 2, no. 1: 62. https://doi.org/10.3390/ECU2021-09312
APA StyleIosif, P., & Stergioulas, N. (2021). Differentially Rotating Relativistic Stars beyond the J-Constant Law. Physical Sciences Forum, 2(1), 62. https://doi.org/10.3390/ECU2021-09312





