1. Introduction
Observations carried out indicate that visible matter and detected radiation make only a small contribution to the total mass of the Universe. Evidence for the existence of dark matter comes for example from studies of gravitational lensing, cosmic microwave background radiation (CMB) or rotation curves of spiral galaxies [
1,
2,
3,
4,
5,
6,
7]. According to the results of observations, dark matter plays a dominant role in the evolution and acceleration of the Universe. In this connection, the urgent task is to search for physical systems (models) for which the presence of dark matter is an intrinsic property.
One of such physical systems can be a “null string” gas, i.e., a multi-string system that structurally consists of gravitationally-interacting closed thin tubes of a massless scalar field in the form of a circle. In works [
8,
9], a study of the influence of the shape of a closed null string on its gravitational properties was started. This study showed that closed null strings in the shape of a circle are stable when moving in an external gravitational field. That is, the shape of a closed null string in the form of a circle should be preserved when moving in an external gravitational field. It was noted that the observed manifestation of the action of an external gravitational field on such a null string can only be a change in its size (radius) or a change in the direction of its motion.
The possibility of taking into account the gravitational interaction in a gas consisting of closed null strings in the form of a circle was investigated in the works [
10,
11]. The result of these works was the ability to qualitatively take into account the mutual change in the trajectories of motion for two interacting null strings. The algorithm proposed to take into account the mutual influence is based on the study of the trajectories of the probe null strings in the gravitational field of the null string of the source. It should be noted that an interesting feature of the gravitational interaction in a gas of null strings is the possibility of the formation of coordinated motions of closed null strings, in which they perform oscillatory motions within a region limited in space. In this case, we can say that two gravitationally-interacting null strings that oscillate inside a space-limited region form a primary particle with an effective nonzero rest mass.
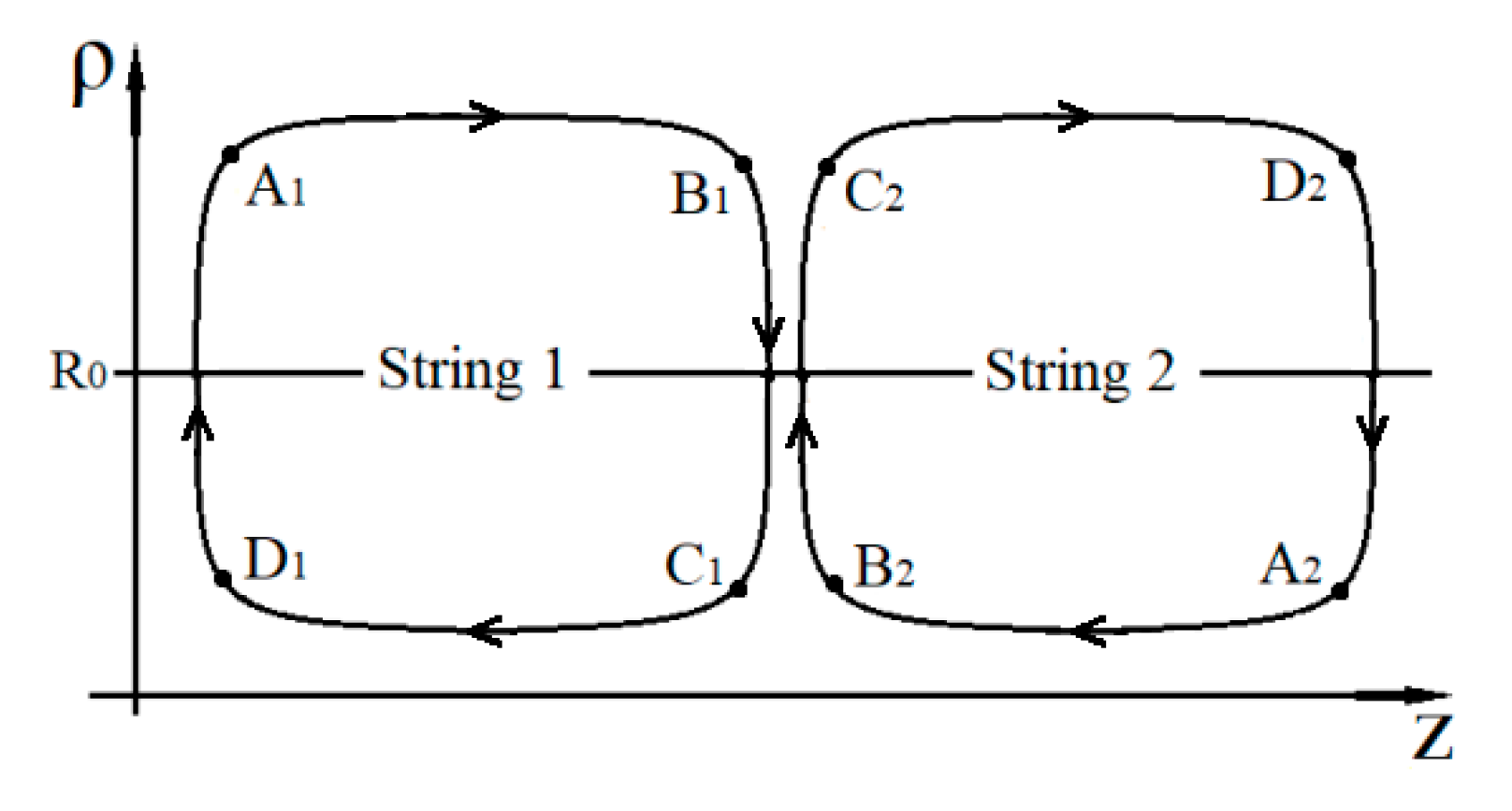
Figure 1 and
Figure 2 in a cylindrical coordinate system, qualitatively, show examples of trajectories of motion of two null strings, the gravitational interaction between which leads to oscillations of each null string inside a region limited in space (the repeating trajectories of motion of null strings forming the corresponding primary particles are bounded both in the variable z and in the variable
) [
10,
11]. The examples given are distinguished by the location in space of the regions within which the oscillations of the null strings that form the primary particles occur.
For the cases shown in
Figure 1 and
Figure 2, two gravitationally-interacting closed null strings have the shape of a circle, they are located in parallel planes and move “towards” each other. For the situation shown in
Figure 1, the meeting surface (i.e., the surface on which two null strings “meet” when moving towards them) is
. For the situation shown in
Figure 2, the meeting surface is the surface
. In
Figure 1 and
Figure 2, the direction of motion for string 1 defines a sequence of points: A
1, B
1, C
1, D
1, …, and for string 2, a sequence of points A
2, B
2, C
2, D
2, and so on. In these figures, the points on the trajectories of motion, which are designated by the same letter (but with different indices), correspond to the positions of null strings forming the primary particle for the same time value
.
If each primary particle shown in
Figure 1 and
Figure 2 is considered separately (i.e., outside the gas null strings), then the “lifetime” of such a particle will be unlimited. However, the “lifetime” of the primary particle located inside the gas of null strings, due to the gravitational interaction with external null strings, can be both long and very short. Long-term existence (“lifetime”) of primary particles is possible if they are combined into many-particle spatial structures.
Among such structures, the most interesting are axially symmetric (“one-dimensional”) objects—“threads”, and spherically symmetric domains—“macro” objects.
It should be noted that the possibility of combining primary particles (pairs of gravitationally-interacting null strings) into complex many-particle structures depends on the direction of motion of the null strings involved in the formation of the primary particle [
10,
11]. To characterize the direction of motion of the null strings that form the primary particle, a vector orthogonal to the plane of the location of the null strings that form the primary particle can be constructed. To unite interacting null strings into complex multiparticle formations, for example, into a “one-dimensional” object (“thread”), the directions of the vectors that characterize the direction of movement of individual null strings (locally) must coincide.
For the case of combining primary particles into a spherically symmetric domain, the forming “threads” are located at the radii of such a domain, and null strings belonging to different “threads” form spherical surfaces (form layers of the domain).
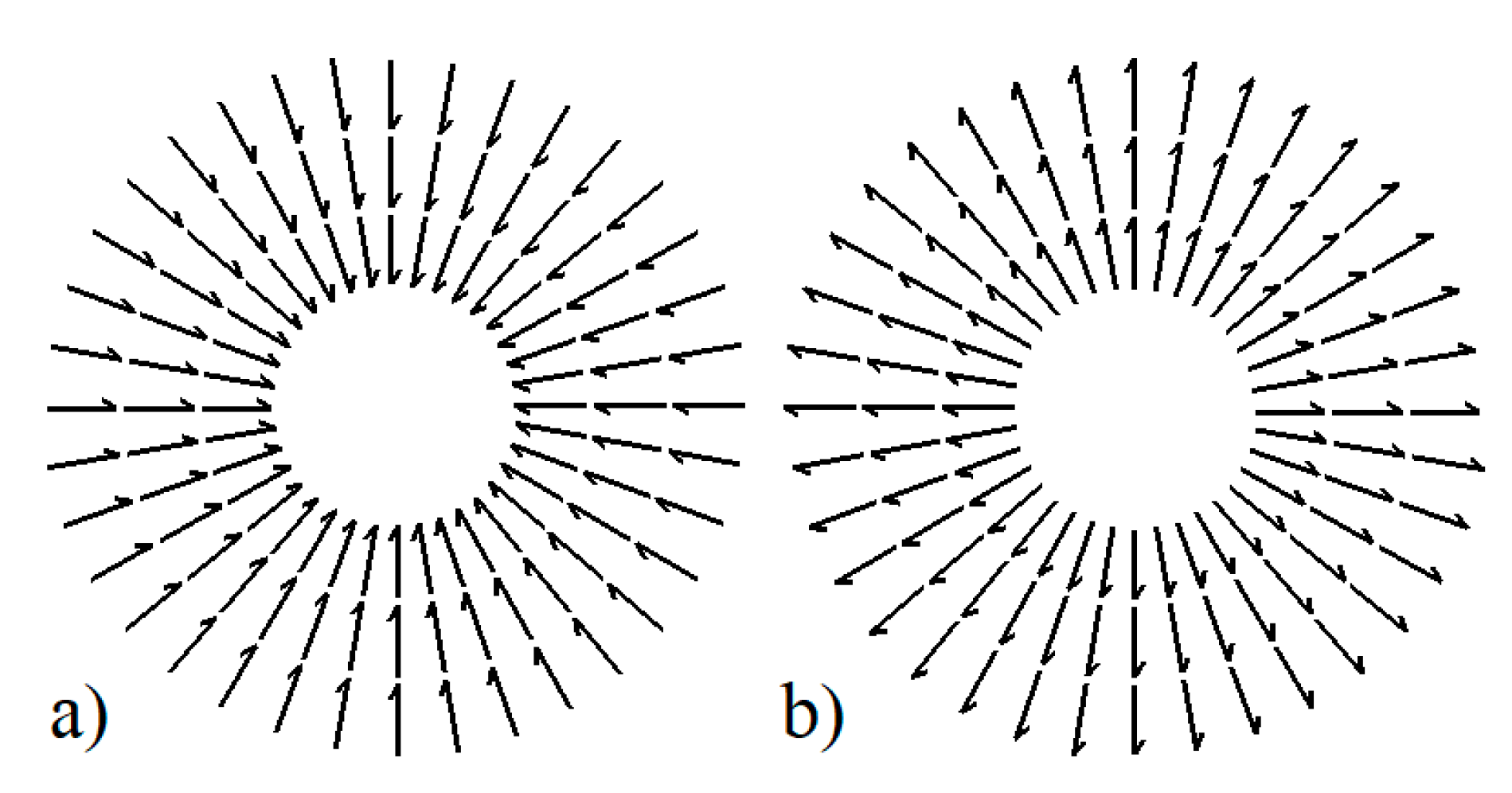
Figure 3 shows two possibilities of such a combination (schematically). In
Figure 3, each vector corresponds to a null string (the vector determines the direction of motion of the null string, which is involved in the formation of the “thread”). Null strings that participate in the formation of a spherically symmetric domain are always located orthogonal to the direction of the corresponding vector [
10,
11].
It can be noted that an interesting feature of such “macro” structures in a null string gas is the fundamental impossibility of having a fully formed (finished) structure. The following reasons can be named that lead to the impossibility of having a fully formed structure for “macro” objects (“macro” structures) in a gas of null strings:
The listed reasons should lead to a random (dynamic) change in the number of null strings that gravitationally “belong” to a specific (considered) “macro” object (specific “macro” objects). It is clear that due to the random change in the number of null strings that gravitationally “belong” to the considered “macro” formation, the spatial form of the “macro” object (domain) will also randomly (dynamically) change.
Considering the spatial distributions of “macro” formations in a gas of null strings on different time scales, one can introduce the concepts of “substance” and “field”. Namely, the “macro” formations averaged over a long period of time form the “substance”, and the structures that are associated with the “macro” objects but whose “lifetime” is less will form the “field”. Obviously, with a decrease in the observation time, various ordered structures (“particles”) will be observed in the “field”, which can be interpreted as interaction “particles”. Moreover, with decreasing observation time, the number of “particles” forming the “field” should increase, and the structure of the “particles” forming the “field” will become more diverse.
In this model, dark matter can be formed by extremely numerous and spatially diverse structures with nonzero rest mass, which are formed in a null string gas (gas of thin closed tubes of a massless scalar field) as a result of gravitational interaction. It is important to note that the “lifetime” of such structures in the gas can be extremely short, but their number and permanent formation of new ones can make a considerable contribution to the overall mass.
An interesting problem is the study of the gravitational field of such structures, in particular, the study of the gravitational field of two gravitationally-interacting null strings in the form of a circle (primary particles with nonzero rest mass), the trajectories of which are shown in
Figure 1 and
Figure 2.
In the articles [
17,
18,
19,
20,
21], the gravitational field of solitary closed null strings was investigated, the trajectories of which did not change over time. Namely, in the articles [
20,
21], a solution for the Einstein equations was found for a closed null string of constant radius, which moves along the
axis and at each moment of time
lies entirely in the plane orthogonal to this axis. In the articles [
17,
18,
19], solutions are given that describe the gravitational field of a solitary closed null string, for the cases in which the null string radially increases or radially decreases its size (radius). One of the results of these papers is the statement that the flat Minkowski space cannot be considered as asymptotic for such gravitational fields. On the other hand, in accordance with Petrov’s classification [
22], the gravitational field of particles with nonzero rest mass should be of the type
, and respectively, the asymptotics of the gravitational field of particles with nonzero rest mass should be flat Minkowski space.
This article is devoted to the study of the asymptotics of the gravitational field of primary particles with nonzero rest mass. These particles are structurally composed of two closed null strings in the shape of a circle and they are formed in a gas of null strings as a result of gravitational interaction. In this work, a unit system is chosen in which the speed of light c = 1.
2. Construction of the Quadratic Form
We will assume that the source of the gravitational field is two gravitationally-interacting null strings in the form of a circle (primary particles), the trajectories of which are shown in
Figure 1 and
Figure 2.
When constructing a quadratic form describing the field of two gravitationally-interacting null strings, we can assume that the trajectory of motion of each of the interacting null strings consists of four segments (see
Figure 1 and
Figure 2). Moreover, on the segments
and
, the null string moves with a constant radius. On the segments
and
, the null string radially decreases or increases its size (radius).
It is important to note that on each of the given segments, the null strings that form the particle move in opposite directions (“towards” each other). So, for example, for the case shown in
Figure 1, on the segment
, string 1 moves in the positive direction of the
axis, and string 2, at the same time, on the segment
moves in the negative direction of the
axis. On the segment
, string 1 radially decreases its size (radius), and string 2, at the same time, on the segment
, radially increases its size (radius).
If a closed null string, on a certain segment, moves along the
axis without changing the size (radius), then its “trajectory” of motion (world surface) is determined by the equalities
where
and
are parameters on the world surface of the null string;
and
constants that determine the value of the
parameter on the boundaries of the segment;
null string radius; and the constants
and
, respectively, determine the initial time value and the initial position of the null string on the
axis. The motion of a closed null string in the negative direction of the z axis corresponds to the case
. The motion of a closed null string in the positive direction of the z axis describes the case
.
If, on some segment, a closed null string radially increases or decreases its size (radius) while being on the surface
, then the “trajectory” of motion is determined by the equalities
where the constants
and
, respectively, determine the initial time value and the initial radius of the null string. The case
describes radial compression, and the case
describes the radial expansion of a closed null string. It is assumed that
In Equalities (3), the constant is the radius of the null string at the initial moment of time () for the case of radial expansion, and is the radius of the null string at the final moment of time () for the case of radial compression.
Since for trajectories of a null string (1) and (2), the shape of a null string is preserved during motion (remains a circle), the metric functions as . Then, using the invariance of the quadratic form with respect to the inversion of on , we obtain .
It can also be noted that for the cases shown in
Figure 1 and
Figure 2, on time scales much larger than the time of one full cycle of oscillation of the null string (or at distances for which the geometric dimensions of the particles shown in
Figure 1 and
Figure 2 can be neglected), the functions of the required quadratic form must be invariant under the simultaneous inversion
,
, i.e.,
The consequence of (4) is
. Also, using the freedom to choose coordinate systems in GR, we partially fix it by the requirement
. Thus, the quadratic form for the problem being solved can be represented as
where
are functions of the variables
.
3. Null String Motion
The motion of a null string in a pseudo-Riemannian space-time is determined by the system of equations [
23]:
where
is the metric tensor, and
are Christoffel symbols for the external space-time,
,
; the indices
take values 0, 1, 2, 3; and the functions
determine the motion trajectory (the world surface) of the null string.
Since the Trajectories (1) and (2) simulate the motion of gravitationally-interacting null strings, on the corresponding segments of the trajectories shown in
Figure 1 and
Figure 2, then the functions
,
, must be particular solutions of the equations of motion of a null string. In this case, the analysis of the equations of motion can give additional restrictions on the functions of the quadratic form (5).
For Trajectory (1), Equation (7) leads to the equality
and for Trajectory (2), to the equality
For (8) and (9), the quadratic form (5) takes the form
where
are functions of the variables
.
Note that on each of the segments:
,
,
,
,
(see
Figure 1 and
Figure 2), the null strings forming the primary particle move towards each other (in opposite directions). Then, the functions defining the trajectories of the interacting null strings, for each of the segments, must simultaneously satisfy the equations of motion (6).
For the case of motion of a closed null string of constant radius in the negative direction of the
axis, equations of motion (6), for (1) and (10), lead to the only equation
For the case of motion of a closed null string of constant radius in the positive direction of the
axis, equations of motion (6), for (1) and (10), lead to the equation
The joint solution of equations (11) and (12) is
Equations of motion (6), taking into account (2), for the case of radial compression and radial expansion of a closed null string located in the plane, lead to the equations
The joint solution of Equations (14) and (15) is
The consequence of the equalities (13) and (16) is
. Without loss of generality, we can fix the value of the constant
, then we finally get
4. Einstein’s Equations Solution
If functions
define the trajectory of motion (world surface) of a null string, which moves in a pseudo-Riemannian space-time with the metric tensor
, then the components of the energy-momentum tensor of such a null string are determined by the equalities [
23]
where indexes
take values 0, 1, 2, 3,
,
According to (18) outside the null string, i.e., in the region where , , all components of the null string energy-momentum tensor are identically zero. In this article, we investigate the asymptotics of the gravitational field of primary particles (i.e., we are looking for a solution in empty space surrounding the primary particle). Then, without loss of generality, we can assume that all components of the energy-momentum tensor of gravitationally-interacting null strings in the investigated region are equal to zero.
In the investigated region of space-time, the Einstein system of equations, for the quadratic form (10) and (17), can be represented as
The solution to Equations (19)–(24) can be represented as
where
,
, and
are constants.
Since the function
must be invariant under the simultaneous inversion of
,
, the consequence of (4) is
Also, without loss of generality, you can fix the value of the constant
For constant values (26) and (27), we find
Applying (17) and (28) for (10), we obtain the expression for the required quadratic form
6. Conclusions
It is interesting that since by the term “null string’” we mean a thin tube of a massless scalar field, then, in fact, physical processes in a gas of null strings are processes in a structured massless scalar field. The gravitational (curvature) interaction between the structural elements of this field (thin tubes) leads to the formation of primary particles that have a nonzero rest mass. By interacting, such particles can combine into various multiparticle structures (“macro” objects). The “lifetime” of both primary particles and “macro” objects formed in a structured scalar field can be different, but the result of their existence is the appearance of mass in the scalar field. This occurs because the interaction between primary particles depends on the “direction” of motion of the null strings forming the particle (it depends on the direction of the vector that characterizes the “direction” of motion of the null strings forming the primary particle). The consequence of gravitational (curvature) interaction in a structured scalar field is the appearance of a vector field, the formation of which is associated with the peculiarity of interaction between various global structures of the scalar field. In this case, time-stable “macro” objects are sources of structures that form a “field” and also are sources of an external vector field.
It seems interesting to consider a structured massless scalar field (a gas of thin closed tubes) as the initial matter of the Universe. With such a choice, it may become possible to reduce all known types of interaction to various realizations of curvature (gravitational) interaction between elements (formed structures) of such a gas. In fact, it becomes possible to combine the concepts of “substance” and “field”. The advantages of such a model include its four-dimensionality, as well as the obvious quantum properties of “macro” structures and “macro” processes that can be observed in such a gas. In essence, a future theory describing processes in a gas of thin tubes of a massless scalar field can be considered as a possible example of a hybrid theory. This is the case, since this theory should be partly quantum (for “macro” structures and “macro” processes) and partly classical (motion of the “null strings” forming a gas).
To my friend and colleague Marina Glumova. She fought bravely, but lost her personal battle with COVID-19.