Chaotic Behavior of the Zakharov-Kuznetsov Equation with Dual-Power Law and Triple-Power Law Nonlinearity
Abstract
:1. Introduction
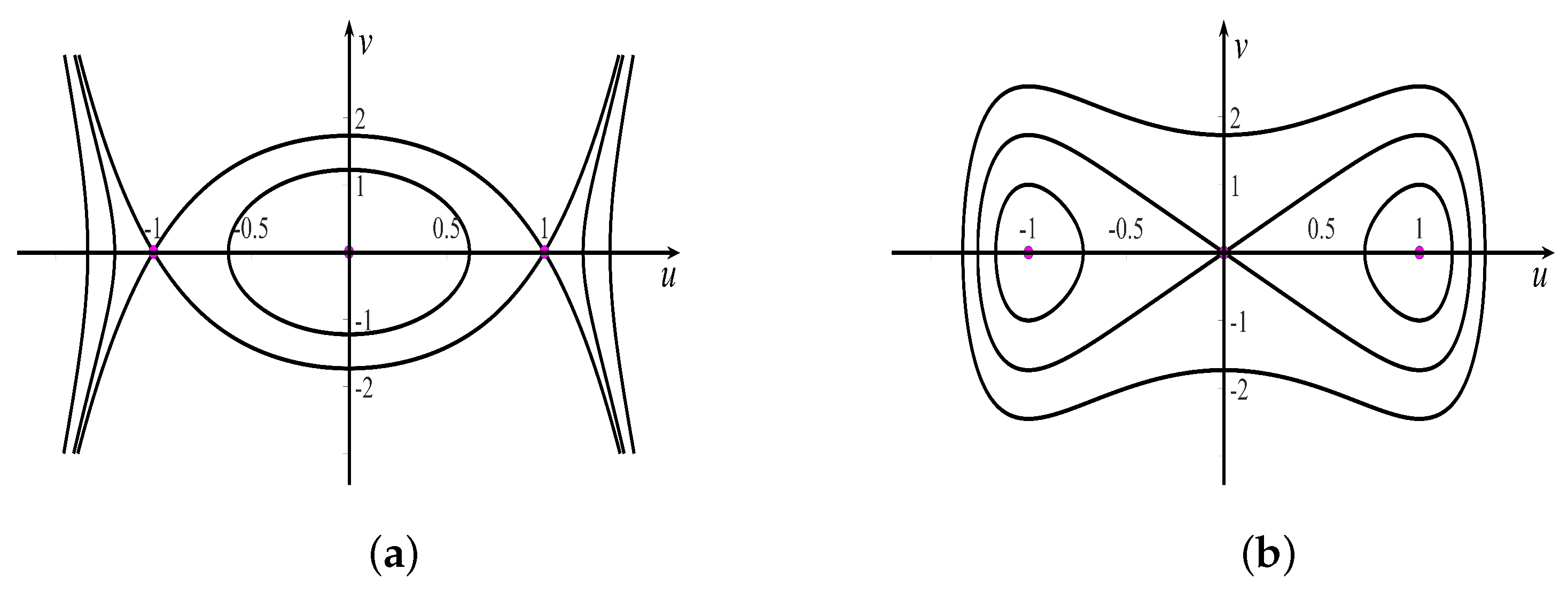
2. Qualitative Analysis for ZK Equation
2.1. ZK Equation with Dual-Power Law Nonlinearity
2.2. ZK Equation with Triple-Power Law Nonlinearity
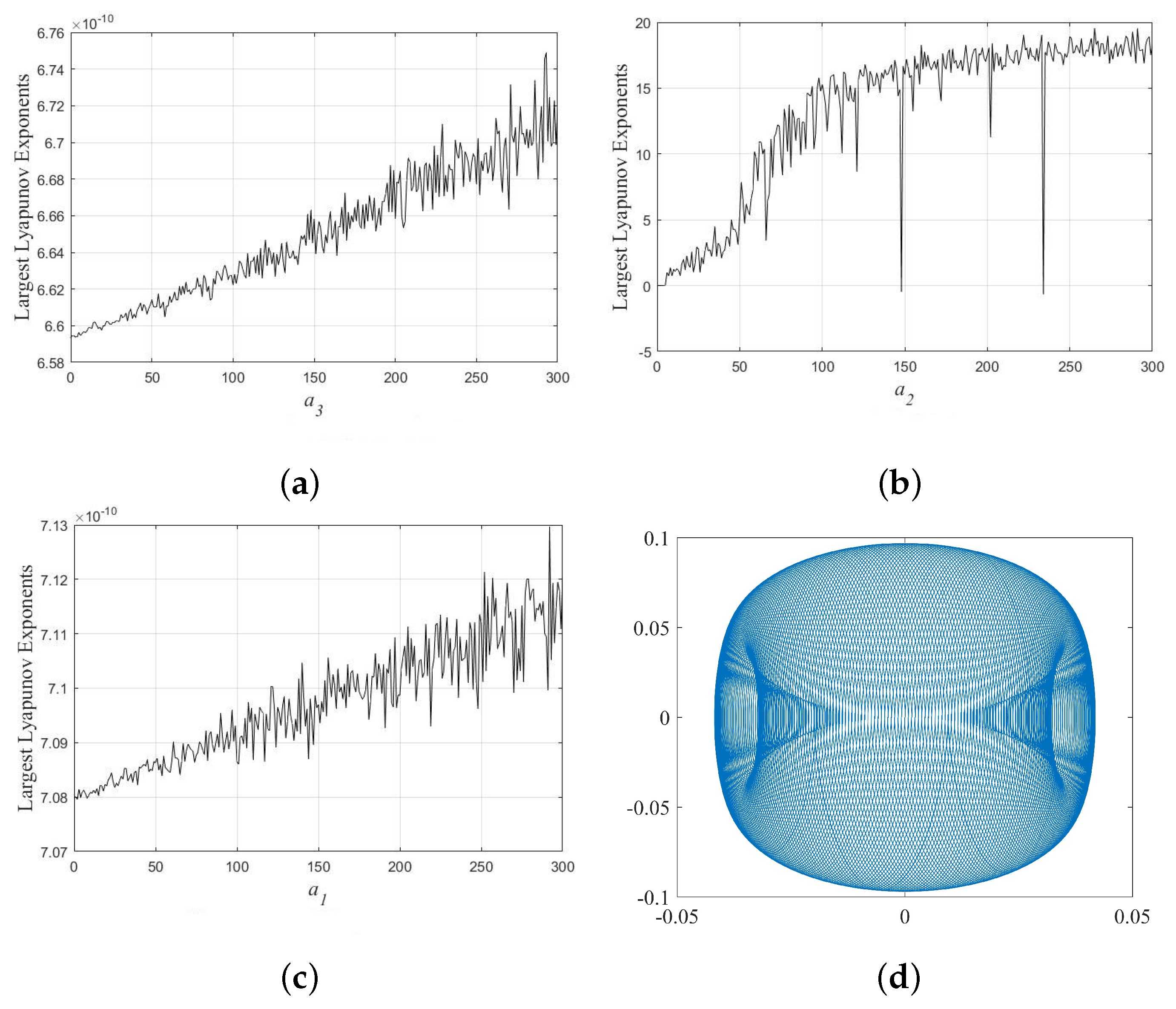
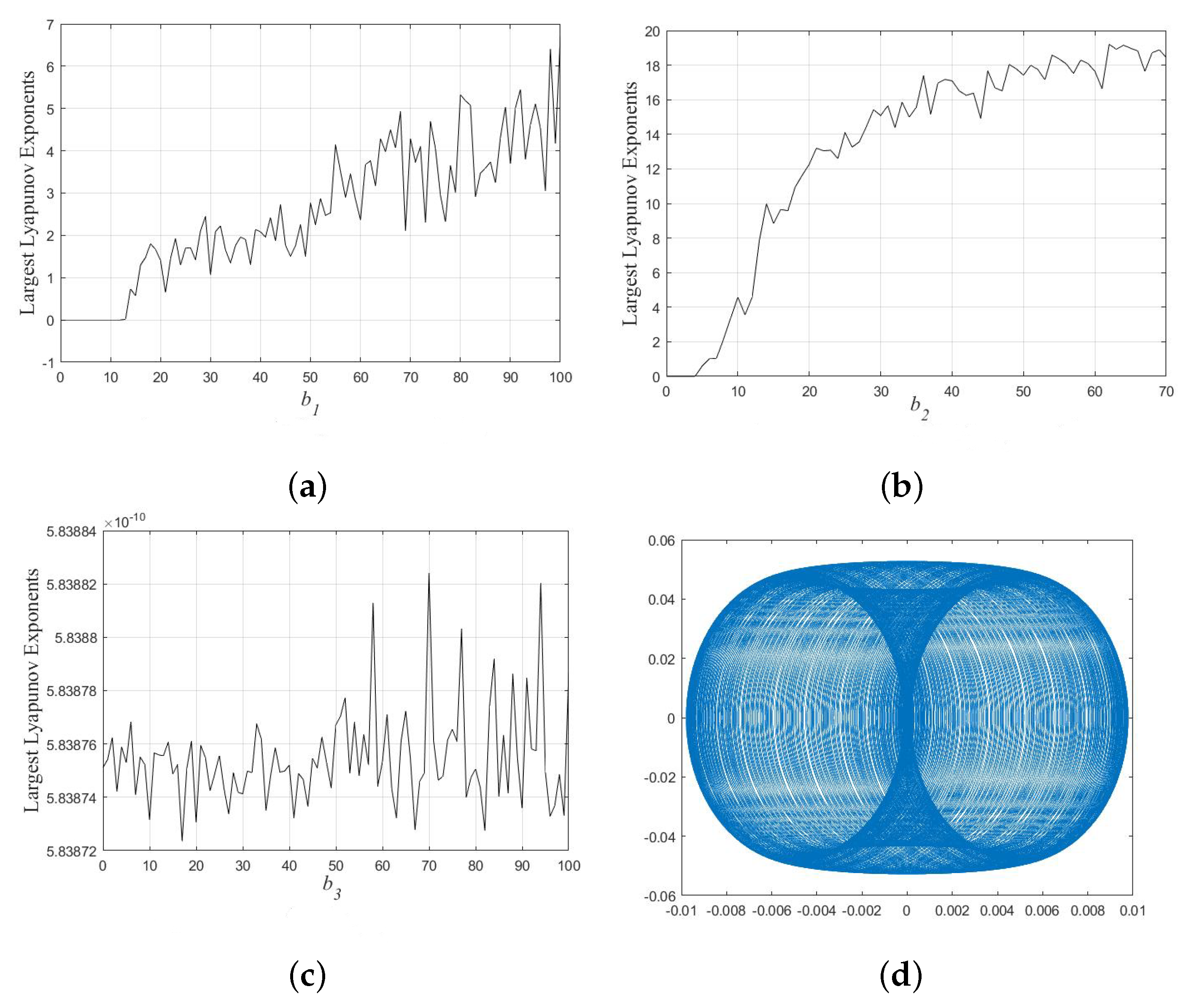
3. The Chaotic Motions
3.1. The Chaotic Motions of System (7)
3.2. The Chaotic Motions of System (18)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zakharov, V.E.; Kuznetsov, E.A. Three-dimensional solitons. Zhurnal Eksperimentalnoi Teroreticheskoi Fiz. 1974, 29, 594–597. [Google Scholar]
- Munro, S.; Parkes, E.J. The derivation of a modified Zakharov-Kuznetsov equation and the stability of its solutions. J. Plasma Phys. 2000, 62, 305–317. [Google Scholar] [CrossRef]
- Munro, S.; Parkes, E.J. Stability of solitary-wave solutions to a modified Zakharov-Kuznetsov equation. J. Plasma Phys. 2000, 64, 411–426. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Exact solutions with solitons and periodic structures for the Zakharov-Kuznetsov (ZK) equation and its modified form. Commun. Nonlinear Sci. Numer. Simul. 2005, 10, 597–606. [Google Scholar] [CrossRef]
- Li, B.; Yong, C.; Zhang, H. Exact travelling wave solutions for a generalized Zakharov-Kuznetsov equation. Appl. Math. Comput. 2003, 146, 653–666. [Google Scholar] [CrossRef]
- Shivamoggi, B.K. The Painlev analysis of the Zakharov-Kuznetsov equation. Phys. Scr. 1990, 42, 641. [Google Scholar] [CrossRef]
- Schamel, H. A modified Korteweg-de Vries equation for ion acoustic waves due to resonant electrons. J. Plasma Phys. 1973, 9, 377–387. [Google Scholar] [CrossRef]
- Zhao, X.; Zhou, H.; Tang, Y.; Jia, H. Travelling wave solutions for modified Zakharov-Kuznetsov equation. Appl. Math. Comput. 2006, 181, 634–648. [Google Scholar] [CrossRef]
- Biswas, A.; Zerrad, E. 1-soliton solution of the Zakharov-Kuznetsov equation with dual-power law nonlinearity. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 3574–3577. [Google Scholar] [CrossRef]
- Du, X.X.; Tian, B.; Qu, Q.X.; Yuan, Y.Q.; Zhao, X.H. Lie group analysis, solitons, self-adjointness and conservation laws of the modified Zakharov-Kuznetsov equation in an electron-positron-ion magnetoplasma. Chaos Solitons Fractals 2020, 134, 109709. [Google Scholar] [CrossRef]
- Jhangeer, A.; Hussain, A.; Tahir, S.; Sharif, S. Solitonic, super nonlinear, periodic, quasiperiodic, chaotic waves and conservation laws of modified Zakharov-Kuznetsov equation in transmission line. Commun. Nonlinear Sci. Numer. Simul. 2020, 86, 105254. [Google Scholar] [CrossRef]
- Yin, H.M.; Tian, B.; Hu, C.C.; Zhao, X.C. Chaotic motions for a perturbed nonlinear Schröinger equation with the power-law nonlinearity in a nano optical fiber. Appl. Math. Lett. 2019, 93, 139–146. [Google Scholar] [CrossRef]
- Kai, Y.; Li, Y.; Huang, L. Topological properties and wave structures of Gilson-Pickering equation. Chaos Solitons Fractals 2022, 157, 111899. [Google Scholar] [CrossRef]
- Kai, Y.; Chen, S.; Zhang, K.; Yin, Z. Exact solutions and dynamic properties of a nonlinear fourth-order time-fractional partial differential equation. Waves Random Complex Media 2022, 1–12. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Kai, Y. Chaotic Behavior of the Zakharov-Kuznetsov Equation with Dual-Power Law and Triple-Power Law Nonlinearity. AppliedMath 2023, 3, 1-9. https://doi.org/10.3390/appliedmath3010001
Li Y, Kai Y. Chaotic Behavior of the Zakharov-Kuznetsov Equation with Dual-Power Law and Triple-Power Law Nonlinearity. AppliedMath. 2023; 3(1):1-9. https://doi.org/10.3390/appliedmath3010001
Chicago/Turabian StyleLi, Yaxi, and Yue Kai. 2023. "Chaotic Behavior of the Zakharov-Kuznetsov Equation with Dual-Power Law and Triple-Power Law Nonlinearity" AppliedMath 3, no. 1: 1-9. https://doi.org/10.3390/appliedmath3010001
APA StyleLi, Y., & Kai, Y. (2023). Chaotic Behavior of the Zakharov-Kuznetsov Equation with Dual-Power Law and Triple-Power Law Nonlinearity. AppliedMath, 3(1), 1-9. https://doi.org/10.3390/appliedmath3010001




