Investigation of Possible Effects of Wearing Exoskeletons during Welding on Heart Rate

Abstract

This study aims to investigate the possible effects of wearing exoskeletons during welding on heart rate. Additionally, the validity of a measuring instrument for determining acute heart rate is to be assessed. N = 15 young healthy subjects with welding experience took part in the study. The study design defines a one-hour workflow that abstracts welding and grinding tasks. The sequence is based on the internationally recognized standard DIN EN ISO 9606-1 and reproduces authentic work sequences in constrained body positions. Each subject completed the workflow once with and once without an exoskeleton. Recorded measures were the heart rates measured by a wrist-worn smartwatch and by Impedance Cardiography (ICG). The average heart rate shows no statistically significant differences in the measurement series with and without exoskeletons. The temporal variation of the heart rate shows a statistically significant influence of wearing exoskeletons and provides a moderate to strong effect, corresponding to a Cohens d of d = 0.78. Only 28.57% of all data series obtained with a smartwatch were equivalent to ICG-data after analysis with t-test, Pearson’s correlation, and orthogonal regression. Using averaged heart rates to assess exoskeleton effects is not a suitable measure. A trend analysis using linear regression shows moderate to strong statistically significant effects in the time course of heart rates and provides an approach to evaluate exoskeleton-induced effects.

1. Introduction

Musculoskeletal diseases (MSD) such as back pain or shoulder issues resulting from heavy physical work are the main reasons for absence from the workplace [1]. This can lead to long-term negative outcomes for the worker, reducing quality of life and increasing sick leave days, and also result in negative outcomes for the employer due to absence of skilled workers [2]. Several ergonomic measures or automation can modify workplaces in order to avoid physically over-demanding work tasks. However, this is not feasible for many workplaces [3]. One option to prevent work-related MSD is exoskeletons, which can reduce the loading on certain joints by supporting lifting and carrying tasks or overhead work [4]. The use of industrial exoskeletons for various types of manual tasks such as manual handling, overhead work, or welding has drastically increased in recent years [5].

Exoskeletons can be grouped according to the supported body region—the most common and most studied being back-supporting exoskeletons and shoulder-supporting exoskeletons [4,6,7]. Back-supporting exoskeletons, which aim to support the user during lifting and lowering tasks as well as forward bending work, have been shown to significantly reduce the muscle activity of the back [8,9,10,11], the perceived strain of the back [10,12], as well as the perceived general strain of the body [13,14]. An influence of back-support exoskeletons on heart rate and metabolic cost could not be shown [15,16,17]. Shoulder support exoskeletons are aimed at supporting the arms during overhead work. It has previously been shown that this type of exoskeleton significantly reduces the activity of the muscles responsible for shoulder elevation and shoulder rotation [18,19,20,21,22,23] and significantly reduces the perceived strain of the shoulders [24,25,26], as well as the whole body. While no effect on heart rate could be shown, the metabolic cost could be significantly reduced [27,28,29]. To conclude, most findings on the effectiveness of exoskeletons are limited to the area of the body, which the exoskeleton aimed to support. Findings on the reduction in whole-body loading are mostly limited to subjective parameters, while objective measures such as heart rate and metabolic cost show ambiguous results. Subjective findings suggest reduced whole-body loading when using exoskeletons; therefore, more research has to be conducted to confirm this in the form of objective measures.

One industrial application of upper body exoskeletons, aimed at supporting the arms during overhead or in front of the body work, is manual welding. The occupation of welding is highly strainful, which is related to a high number of shoulder diseases, which can lead to incapacity to work or even disability [30]. Therefore, this industry could enormously benefit from exoskeletons that unload the upper extremities and the whole body. This paper is focused on assessing the effects of exoskeletons on cardiovascular load by means of the heart rate.

The aims of this paper are:

To assess the effect of different exoskeletons on the heart rate during a simulated manual welding task.

To assess the validity of a wrist-worn smartwatch-based measurement of heart rate compared with heart rate measurement by ICG, an approved medical device.

For the assessment of manual welding, two different types of physiological measurements were included—the measurement of the heart rate with a smartwatch and the measurement of Impedance Cardiography (ICG), a complex measurement of hemodynamics and electrical conduction of the heart, resulting in several parameters, including heart rate. The heart rate data from the smartwatch are based on an optoelectric measurement of the pulse wave, while the measurement of heart rate by ICG is based on electrodes attached to the thorax and neck. It has previously been shown that the measurement of heart rate by wrist-worn trackers is inferior to heart rate data measured by a chest strap [31].

2. Materials and Methods

2.1. Participants and Ethical Approval

A total of n = 15 subjects participated in the study, all subjects were young professional trainees with welding experience from AUDI AG in Neckarsulm, Ford-Werke GmbH in Cologne and Wilhelm-Maybach-Schule in Stuttgart. All subjects were healthy, had no contraindicating musculoskeletal or cardiological diseases and gave written informed consent to participate in the study. The average age was 23 years. A total of 86.6% of the participants were male and 13.4% female. Body mass index (BMI) averaged 27.7. The experiment received prior approval by the ethical committee of the University of Stuttgart.

2.2. Experimental Design

In order to ensure a safe experimental procedure, welding simulators “Soldamatic” from the company Seabery (Seabery Soluciones, Huelva, Spain), as well as grinding simulators designed by the Institute of Industrial Engineering and Management at the University of Stuttgart and the Fraunhofer IPA, were used. These simulators accurately mimic the task of welding a seam and reworking the piece with an angle grinder under laboratory conditions. Standard DIN EN ISO 9606-1 for welding education served as a basis for the simulated workplaces, allowing to define real processes under authentic framework conditions. DIN EN ISO 9606-1 describes and defines welding in constrained positions. Since it is the welding process with the highest industrial impact, the metal active gas (MAG) welding process was chosen for this study. The following welding positions for this experiment were defined in cooperation with SLV Nord, Hamburg:

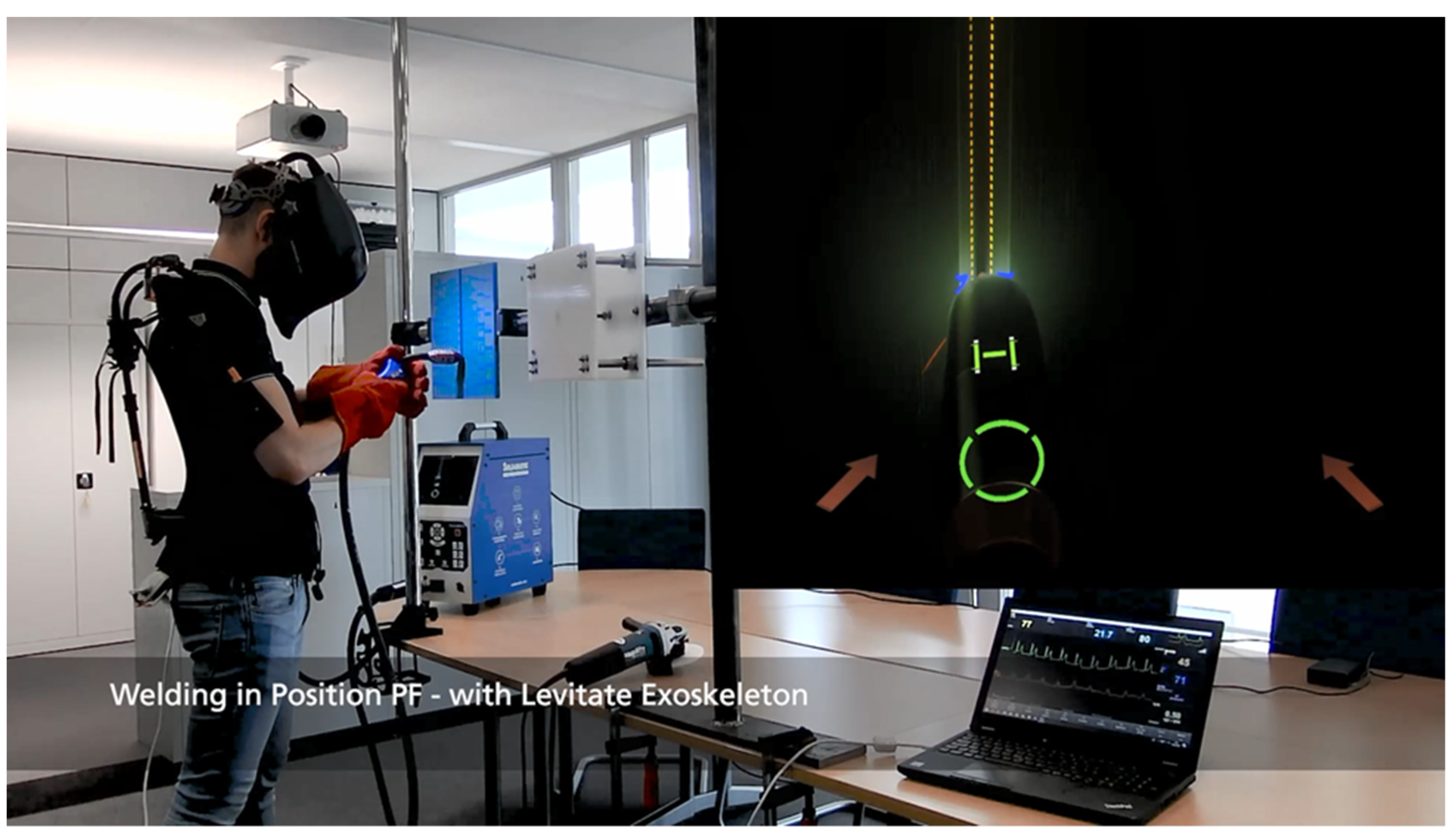
• PF Position—vertical uphill (workpiece located in front of the body, end position slightly below eye level) (see Figure 1).
• PE Position—overhead (workpiece positioned above head, approximately 300 mm in front of the eyes) (see Figure 2).

Each subject welded a 250 mm seam in PF Position followed by simulated grinding in this position. This procedure was repeated 10 times.

Directly following, each subject completed the same process in the PE Position (10 times welding and grinding of the seam).

The total time for the workflow was approx. one hour.

Each study participant completed the defined workflow twice: once with an exoskeleton and once without an exoskeleton. The order of the two runs was randomized. In total, 53.33% of the participants started without exoskeletons, and the other 46.66% with exoskeletons. The subjects were given at least one hour to rest between the runs.

2.3. Equipment

2.3.1. Exoskeletons

Different passive industrial exoskeletons were used in this study. Since the design of the workflow strains the arms and shoulder area, all exoskeletons aimed at supporting work in front of the upper body and overhead by supporting the upper arm. The ones used within this study were:

• Airframe ^®—Levitate Technologies, Inc., 9540 Waples St., Suite F, San Diego, CA 92121, USA.
  • Supporting force: 1.7 to 4.7 kg per arm.
• Paexo Shoulder—Ottobock SE & Co. KGaA, Max-Näder-Straße 15, D-37115 Duderstadt, Germany.
  • Supporting force: 1 to 4.5 kg per arm.
• 360 XFR—Skel-Ex B.V., Scheepsbouwweg 8, G4, 3089 JW Rotterdam, Netherlands.
  • Supporting force: 1 to 4.9 kg per arm.

The selection of exoskeletons was randomly determined. All systems were used in equal proportions in the study. In order to be market-neutral and not to create a competitive advantage, no manufacturer-selective evaluation was performed. This is possible as the build, the point of force application at the upper arm and the supporting force of all three exoskeletons, is similar.

2.3.2. Impedance Cardiography (ICG)

Impedance cardiography was recorded with the Medis Cardioscreen 1000 (medis Medizinische Messtechnik GmbH, Gewerbegebiet Am Ehrenberg, Werner-von-Siemens-Straße 8, 98693 Ilmenau, Germany; REF CG1000).

Two pairs of electrodes were placed at the neck and the thorax. An additional ACM sensor was placed at the earlobe. The cardiac parameters were recorded over the complete duration of the trial. The system works with a sampling rate of 200 Hz.

2.3.3. Smartwatch

Heart rate data was also simultaneously recorded by means of wrist-worn smartwatches. This technology is based on optoelectronic measurement of the pulse wave at the wrist. The watch used here was a Samsung Galaxy Watch 3 (Samsung Electronics GmbH, Am Kronberger Hang 6, 65,824 Schwalbach am Taunus, Germany).

• Model Number: SM-R840
• One UI Watch Version: 2.0
• Tizen Version: 5.5.0.2
• Software Version: R840XXU1BUE3
• Knox Version: 2.5.0

Smartwatch data were recorded over the complete duration of the trial, with a rate of two measurements per minute. The entire measurement series were stored in the smartwatch’s internal storage. After finishing the measurement, the data were transmitted via wireless network. The raw data were then saved locally from the network.

2.3.4. Grinding Simulator

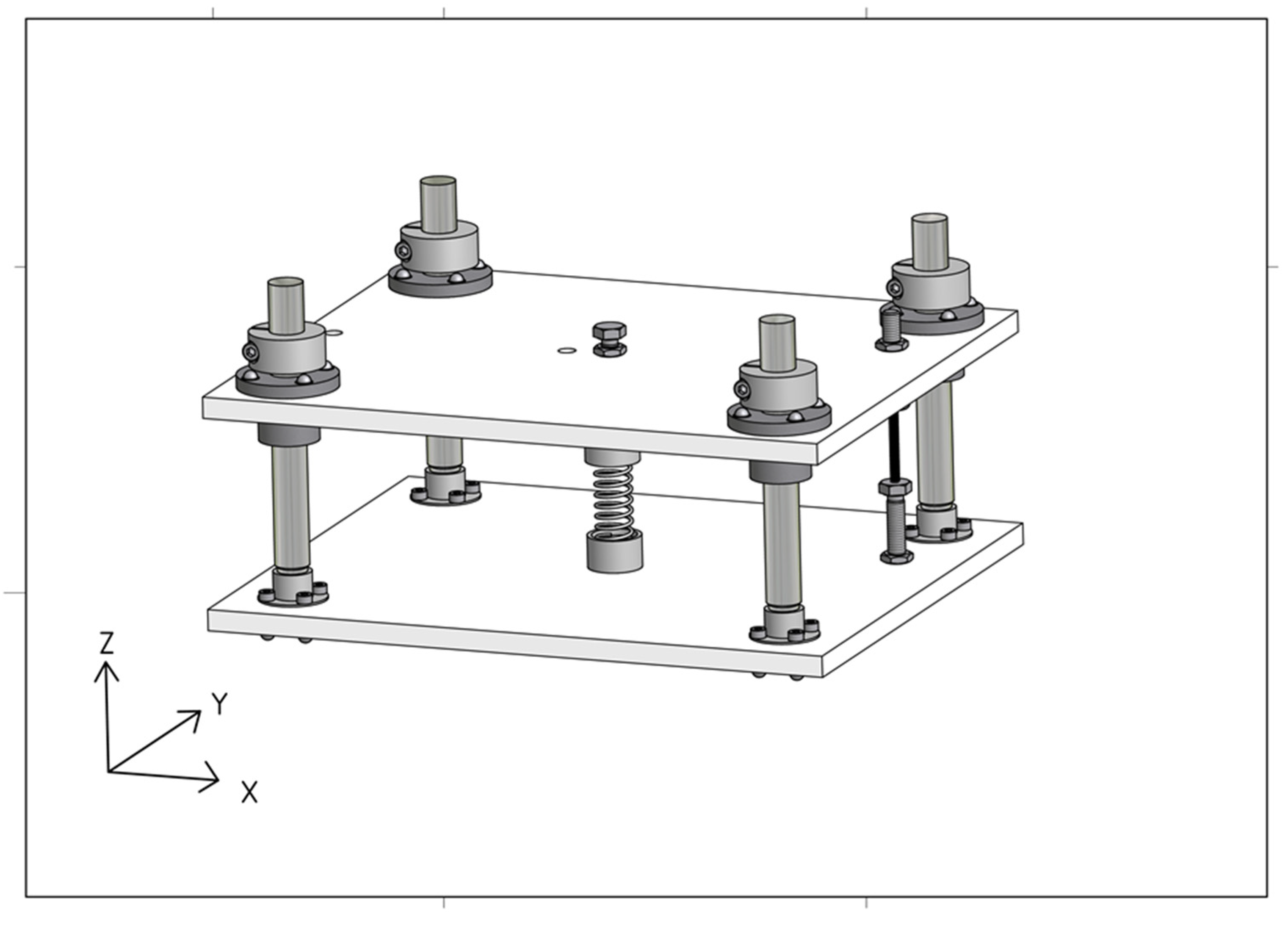
A grinding simulator was developed to ensure appropriate force on the work piece of 35 N in z direction (see Figure 3). This working point is based on a welding experiment that was conducted and analyzed in preparation of this study. The welding parameters in accordance with DIN EN ISO 9606-1 were physically welded in an internal test workshop and processed using an angle grinder. The forces occurring during grinding were determined and simulated using force transducers. The constructed test stand consists of a force-absorbing linearly mounted plate that provides visual feedback when a force of 35 N is reached in the z direction.

The operating point is not rigid and leaves around 5 mm space for movement as in real grinding. The counterpart is a commercially available angle grinder. Its functionality is deactivated by isolating the power connector. The grinder is combined with a dummy cutting disc manufactured of polyoxymethylene with the identical dimensions of a 125 mm cutting disc.

2.4. Data Analysis

All data were analyzed using Minitab statistics software, version 20.1.2 (64 bit). The analysis was categorized into two parts: the effect of exoskeletons on the heart rate (based on ICG data) and the validity of the smartwatch data compared with ICG data. The effect of exoskeletons was examined by comparing the average heart rates over the last 10 min of each trial.

In a second step, the time series of the last 30 min of each trial were examined to assess changes in heart rate over time.

To assess the validity of the heart rate data collected using the smartwatches, the last 10 min of each trial were used. In this part of the study, the heart rate data sets collected with ICG were synchronized with the heart rate data sets collected with smartwatches and examined regarding their deviation. For this purpose, both systems were gauged using the absolute time stamp. Afterwards, each value measured by the smartwatch was assigned to the simultaneously measured heart rate by ICG, resulting in two pairs of values so that both samples were of equal size.

• Effects of exoskeletons on heart rate:

To evaluate the averaged effects of exoskeletons on heart rate, an Anderson–Darling test was performed to assess normality. Afterwards, based on a test for equal variances, a paired two-sample t-test was executed. To visualize the averaged effects of exoskeletons on the heart rate, confidence intervals as well as error bar charts were used.

Effects over time were examined by additionally using trend analysis based on linear regression.

• Validity of the smartwatch heart rate data:

The Anderson–Darling test was used to assess normality. A paired two-sample t-test was used to evaluate the deviation in heart rate, considering the respective measurement method.

The correlation of heart rate data measured with smartwatch and heart rate data measured with ICG was analyzed with Pearson’s correlation coefficient. Based on a two-variance test, an orthogonal regression analysis was performed to determine the linear relationships of the two measurement methods.

3. Results

3.1. Effects of Exoskeletons on Heart Rate

All subjects completed the study, and no data were lost. This results in n = 15 pairs of measurement series (corresponding to valid data from 15 subjects), each consisting of n = 20 measurement points (corresponding to two measurements per minute).

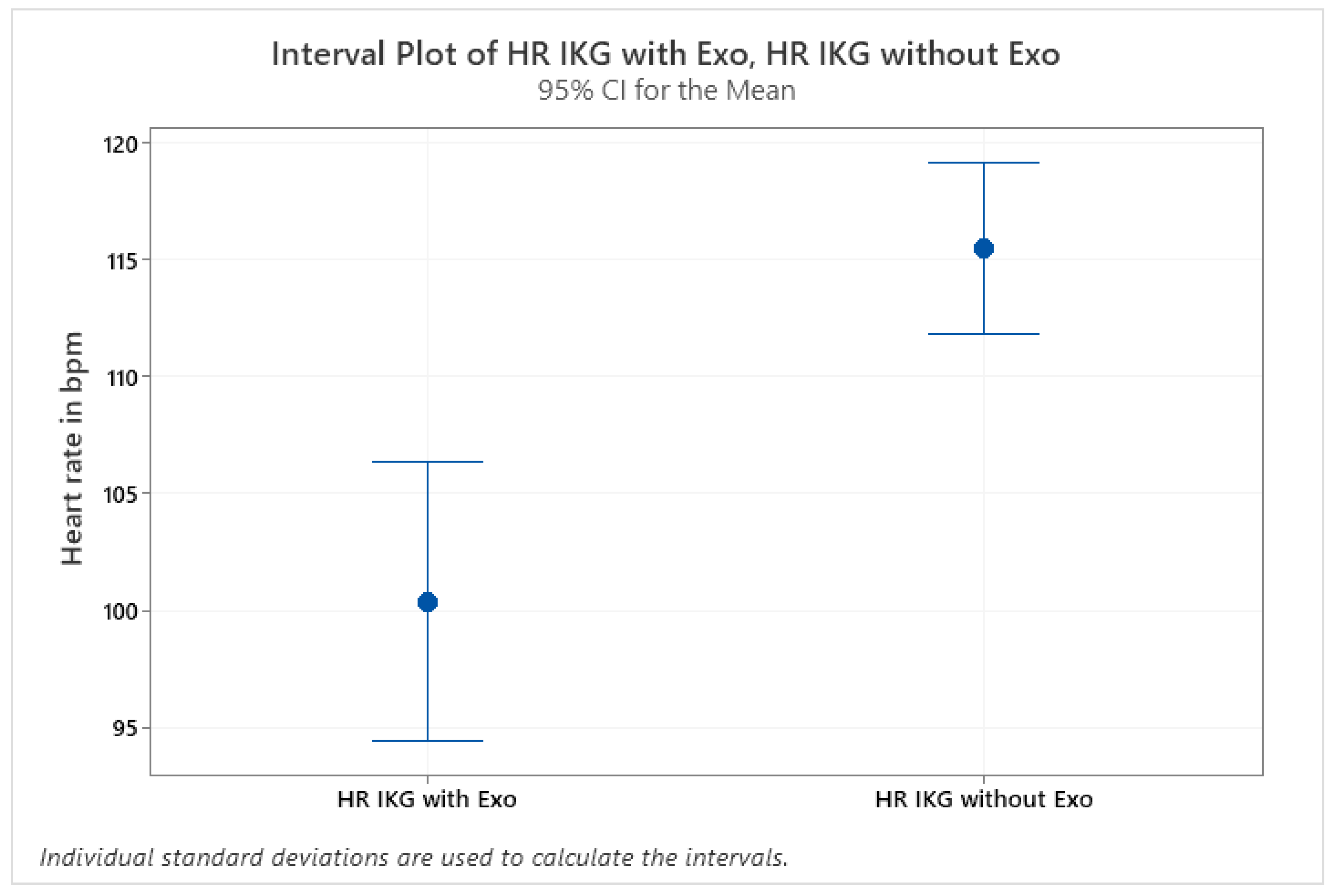
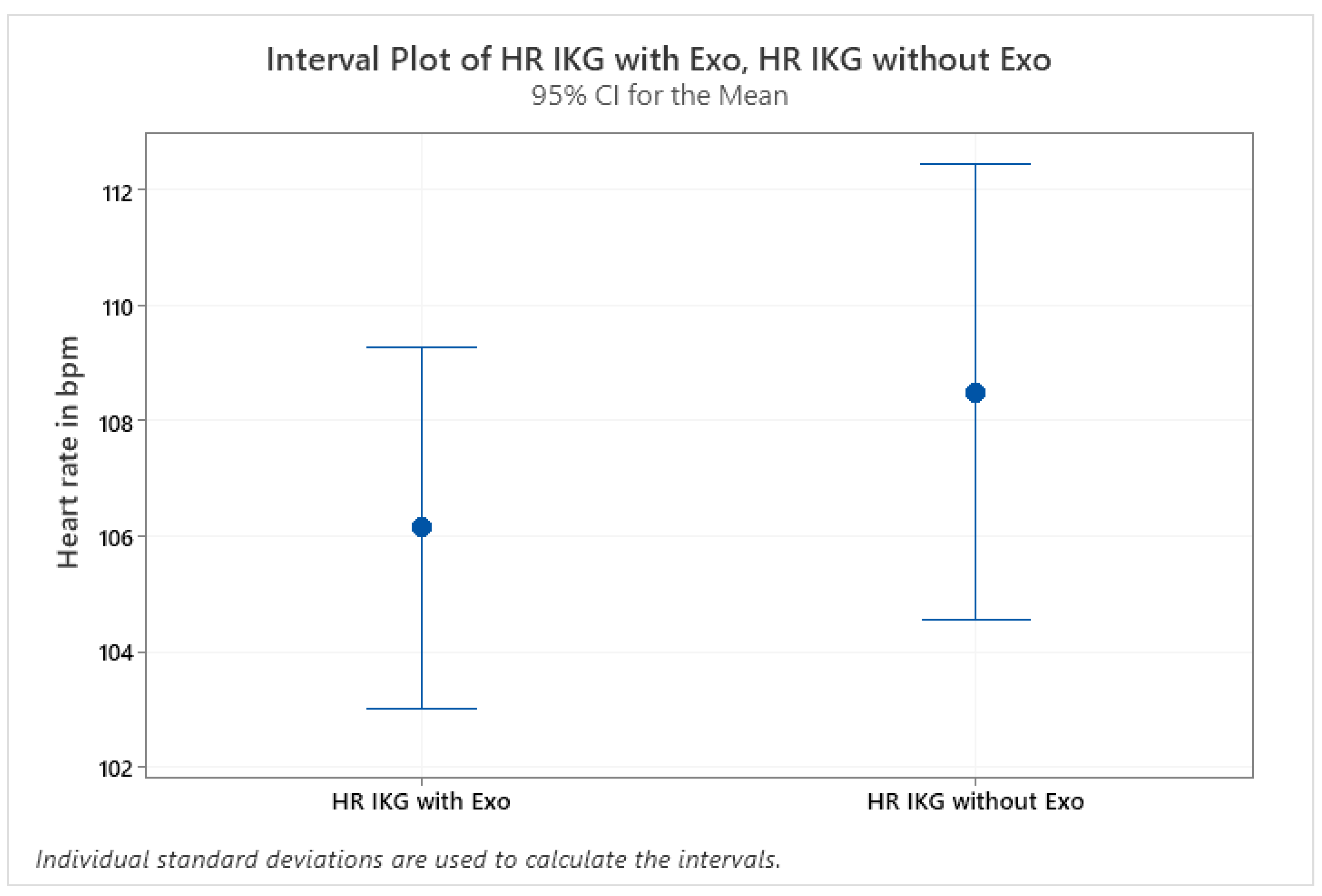
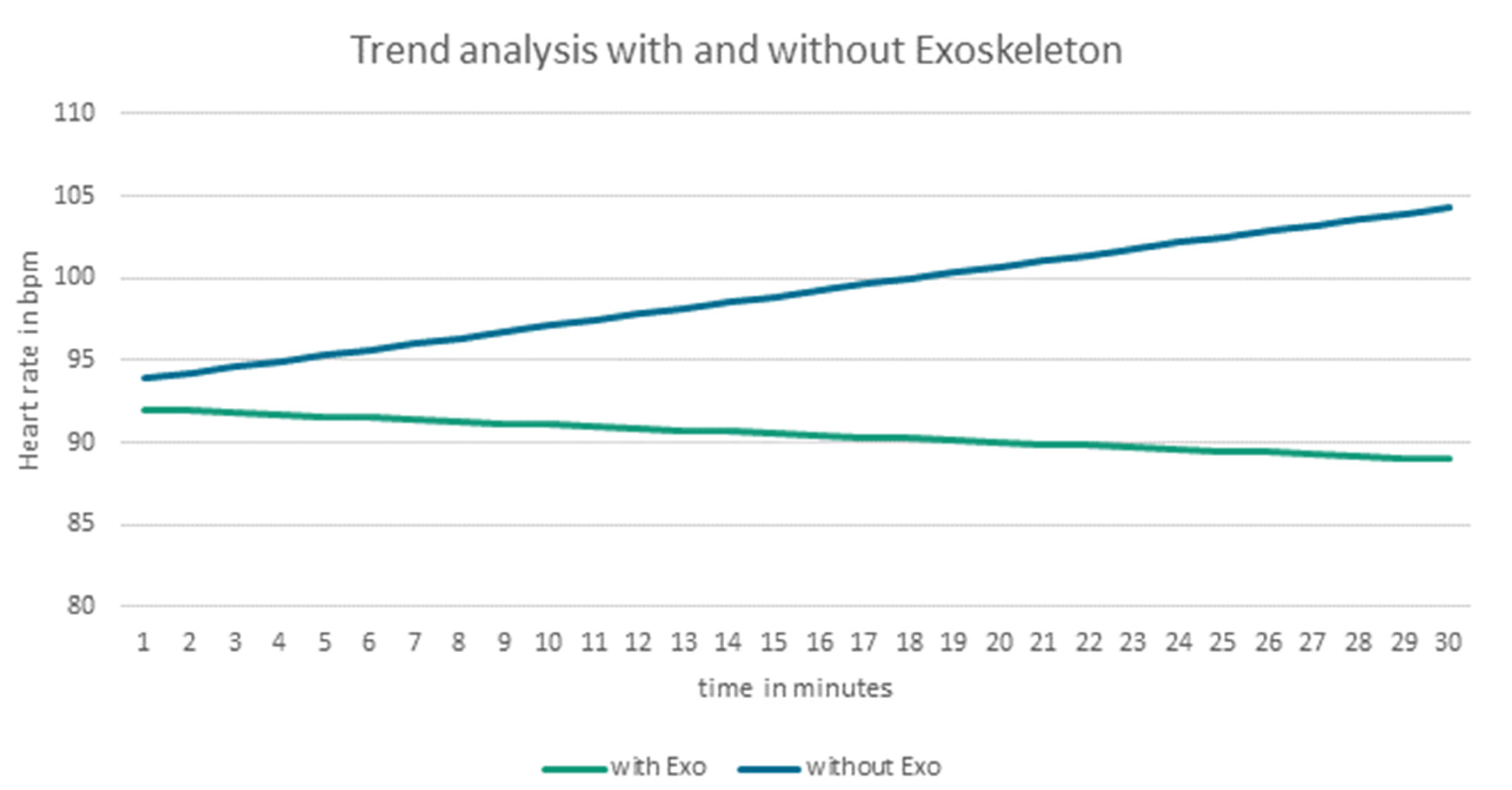
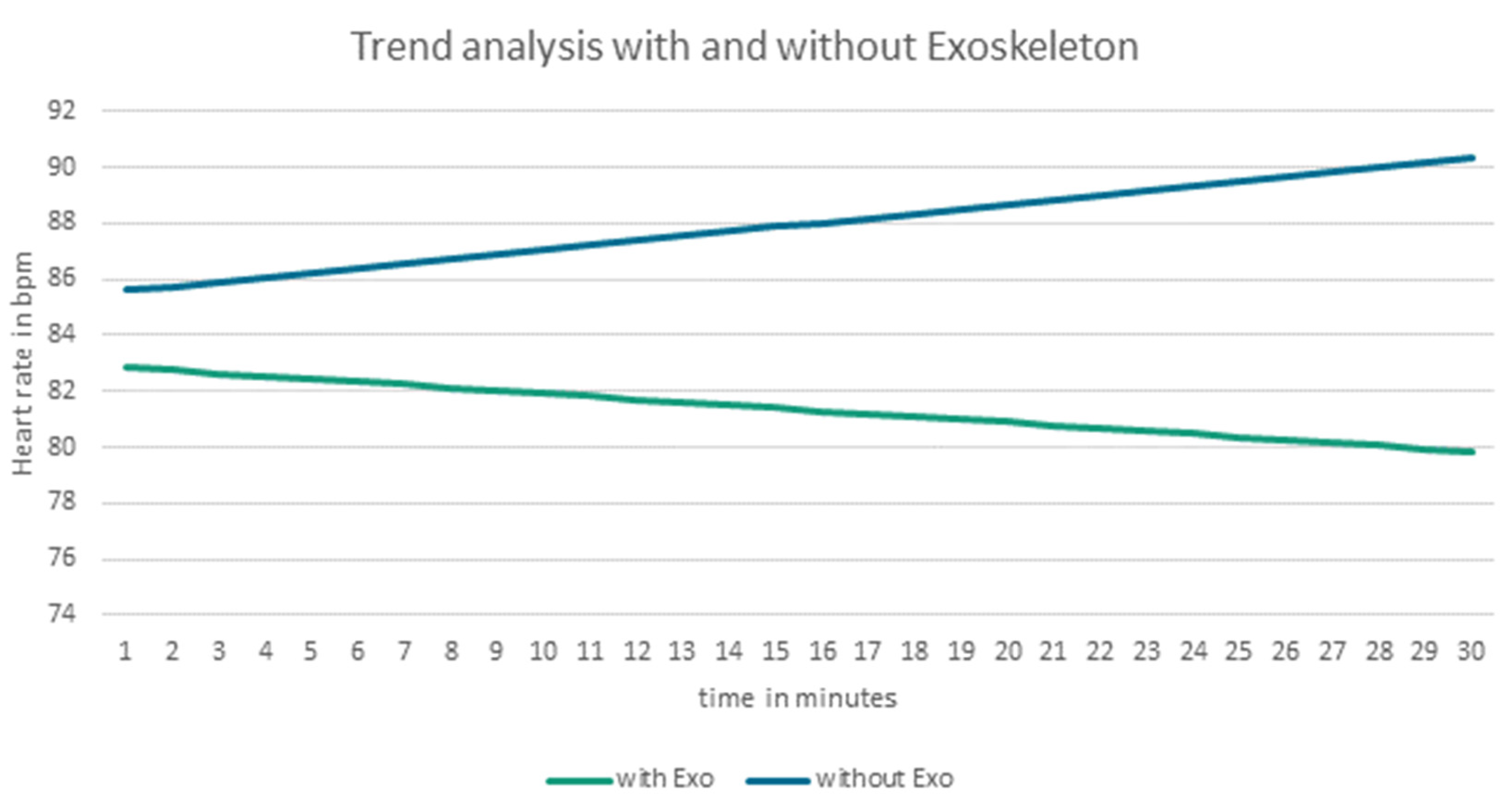
The effects of the exoskeletons regarding the mean heart rate show strong individual differences. Considering individual participants separately, effects range from a significant reduction in mean heart rate to a significant increase in mean heart rate when wearing an exoskeleton. Two different effects can be seen in Figure 4 and Figure 5. For one subject, no effect of the exoskeleton on mean heart rate could be found.

Table 1. Mean values of the measurement series with and without exoskeletons and results of the mean value analysis; p ≤ 0.05 was considered statistically significant.

Subject	Setup	Mean (95% CI) bpm	t-Test (Paired) p
0301	With Exo	89.4	0.000
	Without Exo	101.25
0302	With Exo	106.05	0.000
	Without Exo	118.80
0303	With Exo	100.40	0.001
	Without Exo	115.50
0304	With Exo	97.45	0.007
	Without Exo	102.65
0305	With Exo	106.15	0.382
	Without Exo	108.50
0306	With Exo	105.55	0.000
	Without Exo	111.20
0307	With Exo	114.55	0.001
	Without Exo	108.44
0308	With Exo	128.30	0.016
	Without Exo	132.10
0309	With Exo	81.30	0.000
	Without Exo	89.75
0312	With Exo	94.75	0.000
	Without Exo	78.40
0313	With Exo	90.10	0.039
	Without Exo	95.35
0314	With Exo	132.15	0.000
	Without Exo	109.20
0315	With Exo	114.75	0.000
	Without Exo	101.65
0316	With Exo	91.55	0.000
	Without Exo	84.30
Averaged	With Exo	103.75	0.704
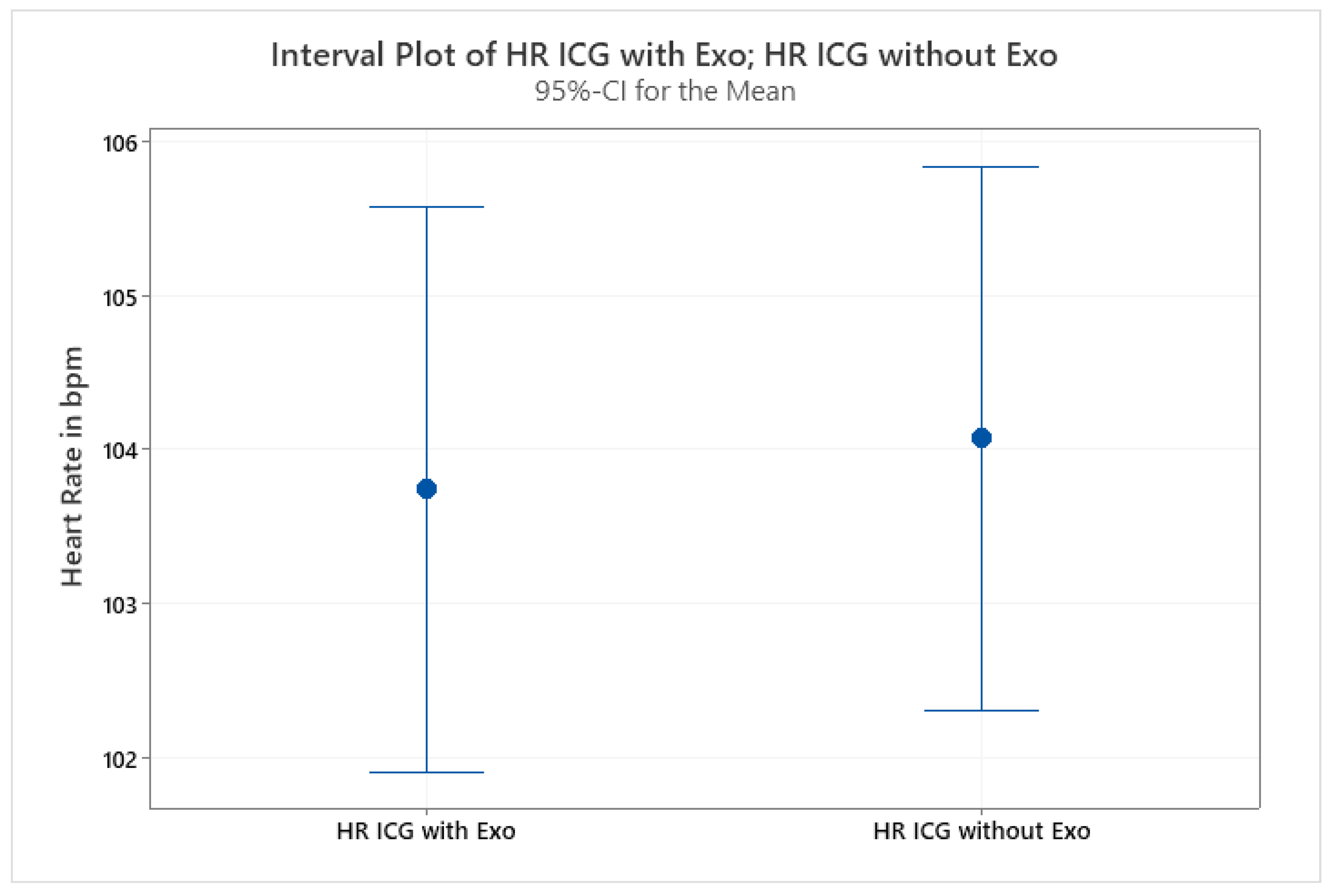
	Without Exo	104.08

shows the means for each subject with and without exoskeleton as well as the means for all subjects as well as the corresponding statistical analysis. As can be seen in the table, for a total of eight subjects, mean heart rate could be significantly reduced when using an exoskeleton, while it was significantly increased for a total of five subjects. A comparison of means of all subjects did not show a significant effect of exoskeleton use on heart rate during a simulated overhead welding task.

No effect of exoskeletons on the mean heart rate of the last 10 min of the task could be found (see Figure 6). This finding does not correspond to subjective ratings, which show a significantly decreased rating of perceived exertion when wearing an exoskeleton. This led us to further analyze heart rate over time in the form of the slope of the last 30 min of the activity.

Considering the slope of the linear regression of each trial divided into the categories “with exoskeleton” and “without exoskeleton”, a reduction in the incline could be found in 80% of the subjects.

As shown in

Table 2. Slope of the regression lines of all measurement series and result of the mean value analysis over all subjects.

Subject	With Exo [Slope]	Without Exo [Slope]	t-Test (Paired) p
0301	−0.001048	0.003600	-
0302	0.001880	0.001923	-
0303	0.002755	0.004938	-
0304	0.003023	0.006046	-
0305	0.002664	0.004007	-
0306	0.001609	0.005852	-
0307	0.002980	0.002658	-
0308	0.006790	0.003020	-
0309	−0.001035	0.001626	-
0311	0.001141	0.001913	-
0312	0.000282	0.002703	-
0313	0.001385	0.001975	-
0314	0.006248	0.006480	-
0315	0.003684	0.004067	-
0316	0.004356	0.003558	-
Averaged	0.002448	0.003624	0.051

, a decrease in heart rate over time can be observed for two subjects (subjects 0301 and 0309) when using an exoskeleton (see Figure 7 and Figure 8).

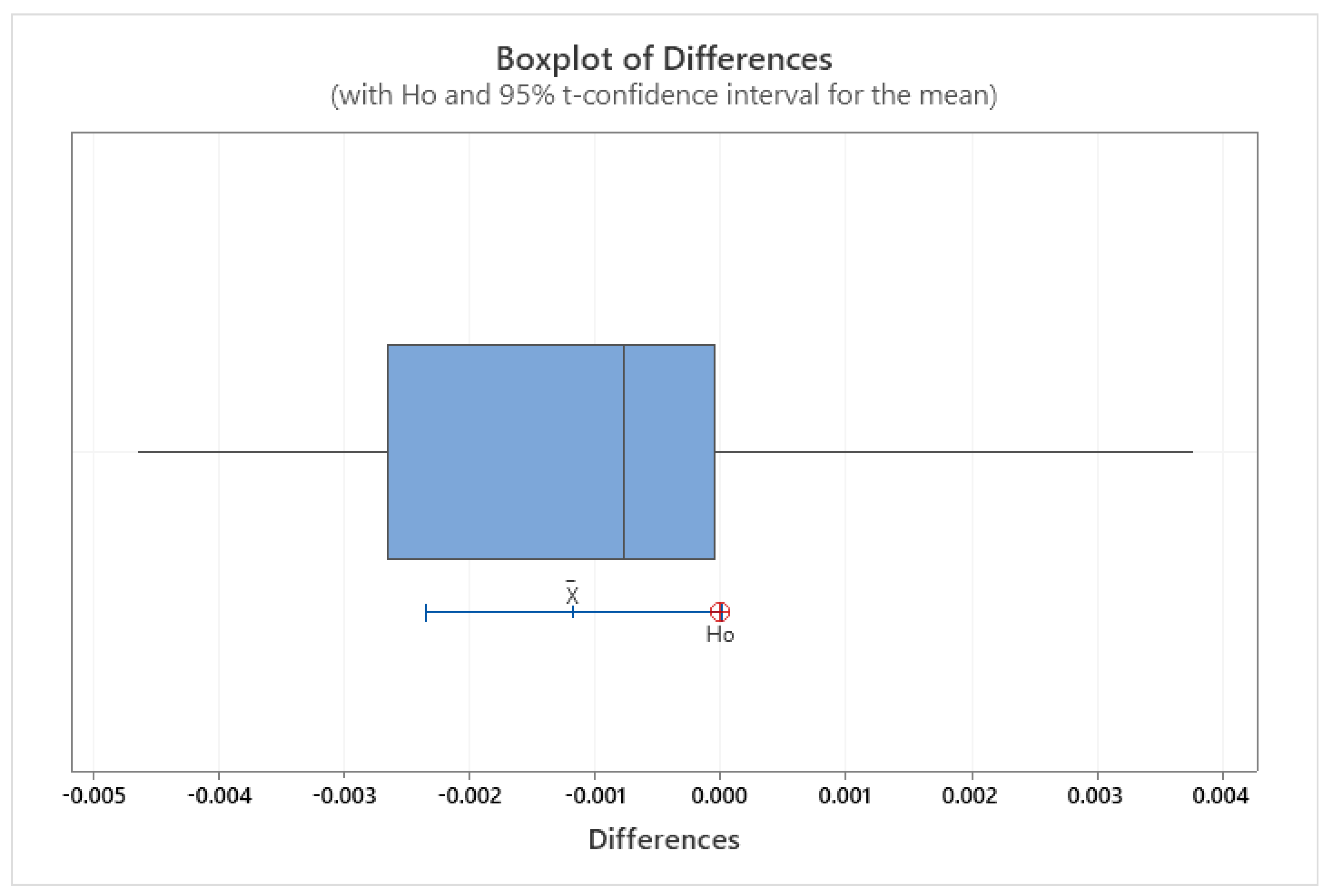
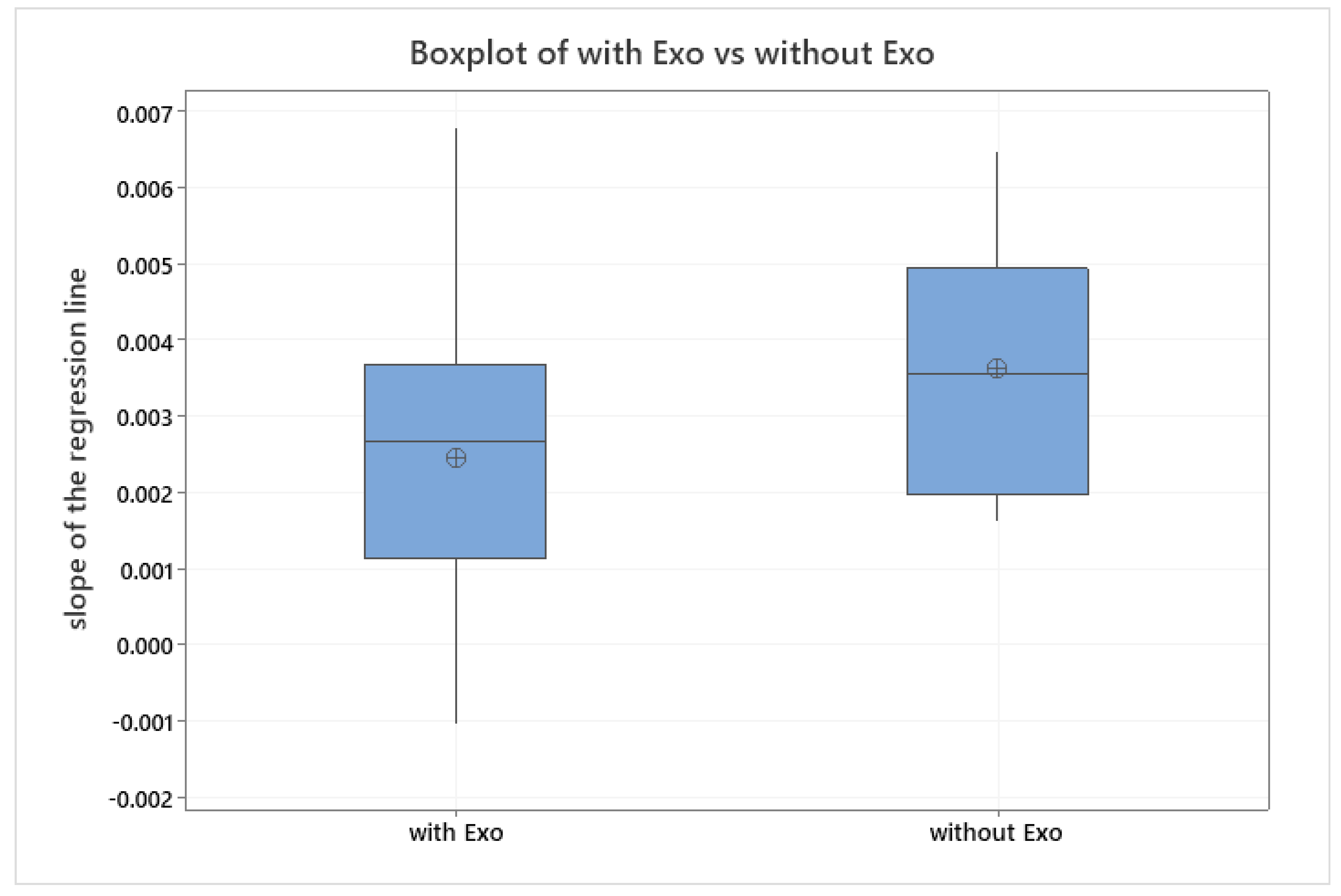
The comparison of means of the slope of heart rate with and without exoskeleton provides a borderline case (see Figure 9) with a p-value of 0.051 resulting from the paired sample t-test.

The visualized 95% confidence interval as boxplot (see Figure 10) and the preceding single value consideration lead to the conclusion that the null hypothesis has to be rejected. This results in a significant difference in the increase in heart rate over time using an exoskeleton. This results in a medium-to-strong effect size d according to a Cohen of 0.78.

3.2. Validity of the Heart Rate Data Measured with a Smartwatch by ECG Recorded with ICG

N = 30 measurement series are available, each containing 20 measurement points. Not all measurement series could be evaluated for the following reasons:

• 0308 without exoskeleton: The smartwatch delivered the same value 20 times for this subject. Thus, a normal distribution is not given. Therefore, the application of different statistical methods is not possible, and the data was considered invalid.
• 0311 with exoskeleton: The smartwatch did not record any values in this measurement.

The comparison of means, using a t-test, revealed that 72.4% of the total n = 28 valid series of measurements showed no significant difference between heart rates recorded by smartwatch and those recorded by impedance cardiography (see

Table 3. List of results of all statistical evaluation methods (subject identifier 0310 was not assigned).

Subject	Setup	t-Test (Paired) [p]	Pearson Correlation [r/p]	Orth. Regression Analysis
0301	With Exo	0.389	0.773/0.000	Equivalent
	Without Exo	0.876	0.602/0.005	Equivalent
0302	With Exo	0.177	0.575/0.008	Equivalent
	Without Exo	0.953	0.044/0.855	Not equivalent
0303	With Exo	0.547	0.205/0.385	Not equivalent
	Without Exo	0.469	0.007/0.759	Not equivalent
0304	With Exo	0.664	0.699/0.001	Equivalent
	Without Exo	0.629	0.600/0.005	Not equivalent
0305	With Exo	0.420	0.347/0.133	Equivalent
	Without Exo	0.901	0.540/0.014	Not equivalent
0306	With Exo	0.000	0.008/0.728	Not equivalent
	Without Exo	0.000	0.248/0.292	Not equivalent
0307	With Exo	0.002	0.403/0.078	Not equivalent
	Without Exo	0.045	0.013/0.958	Not equivalent
0308	With Exo	0.046	0.273/0.244	Not equivalent
	Without Exo	-	-	-
0309	With Exo	0.635	0.250/0.287	Not equivalent
	Without Exo	0.385	0.678/0.001	Not equivalent
0311	With Exo	-	-	-
	Without Exo	0.882	0.134/0.573	Not equivalent
0312	With Exo	0.592	0.641/0.002	Equivalent
	Without Exo	0.531	0.637/0.002	Equivalent
0313	With Exo	0.852	0.149/0.531	Not equivalent
	Without Exo	0.141	0.428/0.059	Not equivalent
0314	With Exo	0.001	0.430/0.059	Not equivalent
	Without Exo	0.393	0.538/0.014	Not equivalent
0315	With Exo	0.042	0.494/0.027	Not equivalent
	Without Exo	0.418	0.471/0.036	Equivalent
0316	With Exo	0.627	0.516/0.020	Equivalent
	Without Exo	0.359	0.334/0.151	Not equivalent

).

N = 13 of the n = 28 valid measurement series show a statistically significant correlation according to Pearson (see Table 3); this corresponds to 46.4% of the population. Of these, n = 8 correlate moderately to strongly and n = 5 correlate moderately.

For n = 9 measurement series, orthogonal regression analysis provides no evidence that the measurement instruments are different, which represents 32.14% of the population (see Table 3).

The number of measurement series that do not show a significant difference in the comparison of means, show a moderate-to-strong correlation according to Pearson, and do not provide any indication of different measurement values of the instruments based on the orthogonal regression amounts to n = 8. With a total population of n = 28, this results in 28.57%.

4. Discussion

In the first step of the analysis, we analyzed the effect of exoskeleton use on the mean of the heart rate of the last ten minutes of a standardized one-hour activity. The last ten minutes of the activity were chosen, as this demonstrates the highest level of strain and fatigue. As several studies have shown an effect of exoskeletons on perceived exertion and muscle activity [4], we hypothesized a significant reduction in mean heart rate when wearing an exoskeleton. This hypothesis had to be rejected according to the non-significant result of the statistical analysis. On further investigation, the effect of the exoskeleton was highly individual, with eight subjects experiencing a reduced heart rate, five subjects experiencing an increased heart rate, and two subjects experiencing no change in heart rate when wearing an exoskeleton. One explanation for the highly individual effects of the exoskeleton could be the body composition of the subjects. Of the seven subjects that experienced either no effect of the exoskeleton or an increase in heart rate, six had a BMI of over 25, which corresponds to overweight subjects. The one subject that experienced an increased heart rate when wearing an exoskeleton and was not overweight had a BMI of 19, which is close to underweight (BMI < 18.5). Of the eight subjects that experienced a positive effect of exoskeleton use on the heart rate, only three had a BMI of over 25. This could be explained by a fixed maximal support force of the exoskeleton that might not be able to compensate for the weight of heavier arms. The heavier a subject’s arms are, the lower the percentage of support the exoskeleton can offer. On the other side, when considering a very light subject, the weight of the exoskeleton contributes a greater added weight in relation to the body weight. This could lead to higher energy expenditure due to the exoskeletons weight. Another possible explanation could be that the fit of the exoskeletons is not optimal for either heavier subjects or very slim subjects, as the adjustability of all exoskeletons has limitations. This finding has to be confirmed in further studies with a wide range of subject sizes and weights that allows for an analysis of the correlation between weight and exoskeleton effectiveness.

In total, the mean heart rate of the last ten minutes does not seem to be an optimal parameter to assess the effect of exoskeletons. Therefore, we hypothesized that there is an effect of exoskeleton on the temporal variation of heart rate, measured in the slope of heart rate over the last 30 min of the activity.

Despite the borderline case regarding the comparison of means of the regression line slope over time, a significant difference was observed when using an exoskeleton. In two individual cases, a steady decrease in heart rate was observed with the help of an exoskeleton. A Cohen’s d of d = 0.78 can be calculated, corresponding to a medium to strong effect. This clearly shows that the slope of heart rate over time is a more suitable measure for the evaluation of the effect of exoskeletons. If we assume a high significance of the method and, according to Cohen, a medium-to-strong effect of the exoskeleton on the temporal increase in heart rate, the statement would be that 80% of all subjects experience less of an increasing heart rate over time when wearing an exoskeleton. Since an increase in heart rate while the workload stays constant is an indicator of fatigue [32], it can be concluded that an exoskeleton could delay or positively influence fatigue. In this analysis, 12 out of 15 subjects experienced a decreased slope of heart rate when wearing an exoskeleton. The three subjects that did not experience this effect all had a BMI over 30. This confirms the finding that the effect of the exoskeleton is reduced in overweight subjects either due to lack of support from the exoskeleton or due to improper fit of the exoskeleton.

To conclude from both analyses, the effect of exoskeletons on heart rate seems to be highly individual and might be dependent on the weight of the subject. The slope of heart rate over a longer period of time (here 30 min) seems to be more adequate to assess the effect of exoskeletons than the mean of heart rate at the end of a task (here 10 min).

For the second section of this paper, we examined the validity of the heart rates measured by smartwatch compared with the heart rates measured by ICG. As the ICG is a licensed medical product, it can be assumed that it provides valid heart rate measurements. The statistical analysis concluded that only n = 8 measurement series of the smartwatch heart rate data, which corresponds to 28.57% of the total population, provide valid measurement data. The reasons for this deviation cannot be fully determined at this point, but a possible reason could be the measurement of heart rate by the smartwatch at the wrist, which has previously been shown to be inferior to other heart rate measurements (chest strap and ECG) [31]. It is also possible that the type of movement in this work task, which consists of prolonged static positions with raised arms, contributes to the inferior measurement of heart rate by the smartwatch. The optoelectric measurement of heart rate at the wrist is based on the photoplethysmographic measurement of the pulse wave. During the activity tested here, the arms are held above the height of the heart for most of the time. Raising the arms above heart height leads to a decrease in blood pressure at the wrist, which could lead to an increase in measurement errors by the smartwatch. More research has to be conducted to assess the suitability of smartwatch-based heart rate measurements for varying work tasks with and without overhead work.

All in all, the measurement of heart rate with a wrist-worn smartwatch does not seem adequate for the task analyzed here.

5. Conclusions

It seems that using mean heart rate to determine effects of exoskeletons is not an appropriate measure. However, when analyzing the variation of heart rate over a longer period of time, a positive effect of an exoskeleton can be found. This suggests that exoskeletons for the upper extremities can reduce the loading of the body and delay the onset of fatigue. As this study only analyzed a one-hour period, further studies have to investigate whether this effect also occurs in significantly shorter or longer working periods.

Furthermore, these results show that while the mean heart rate may not be an appropriate measure for unloading of the body using exoskeletons, the time trend of the heart rate is a more promising measure. Additional research should be conducted to confirm these findings.

Moreover, the results indicate that there might be a correlation between body size and the effectiveness of exoskeletons, with underweight and overweight subjects experiencing less benefit from exoskeleton use.

The second focus of this study was to determine whether a wrist-worn smartwatch could measure the heart rate as accurately as impedance cardiography, a licensed medical product. The measurement of heart rate by means of a wrist-worn smartwatch is exposed to high individual fluctuations. In conclusion, wrist-worn smartwatches do not seem to measure heart rate accurately. Therefore, further research is necessary to find the origin of these substantial variations.