SPH Simulation of Molten Metal Flow Modeling Lava Flow Phenomena with Solidification
Abstract
1. Introduction
2. Computational Method
2.1. Governing Equations
2.2. Discretization of Governing Equations in SPH Method
2.2.1. Discretization of Navier–Stokes Equation
2.2.2. Algorithm of Incompressibility
2.2.3. Discretization of Energy Transport Equation
2.3. Computational Conditions
3. Results and Discussion
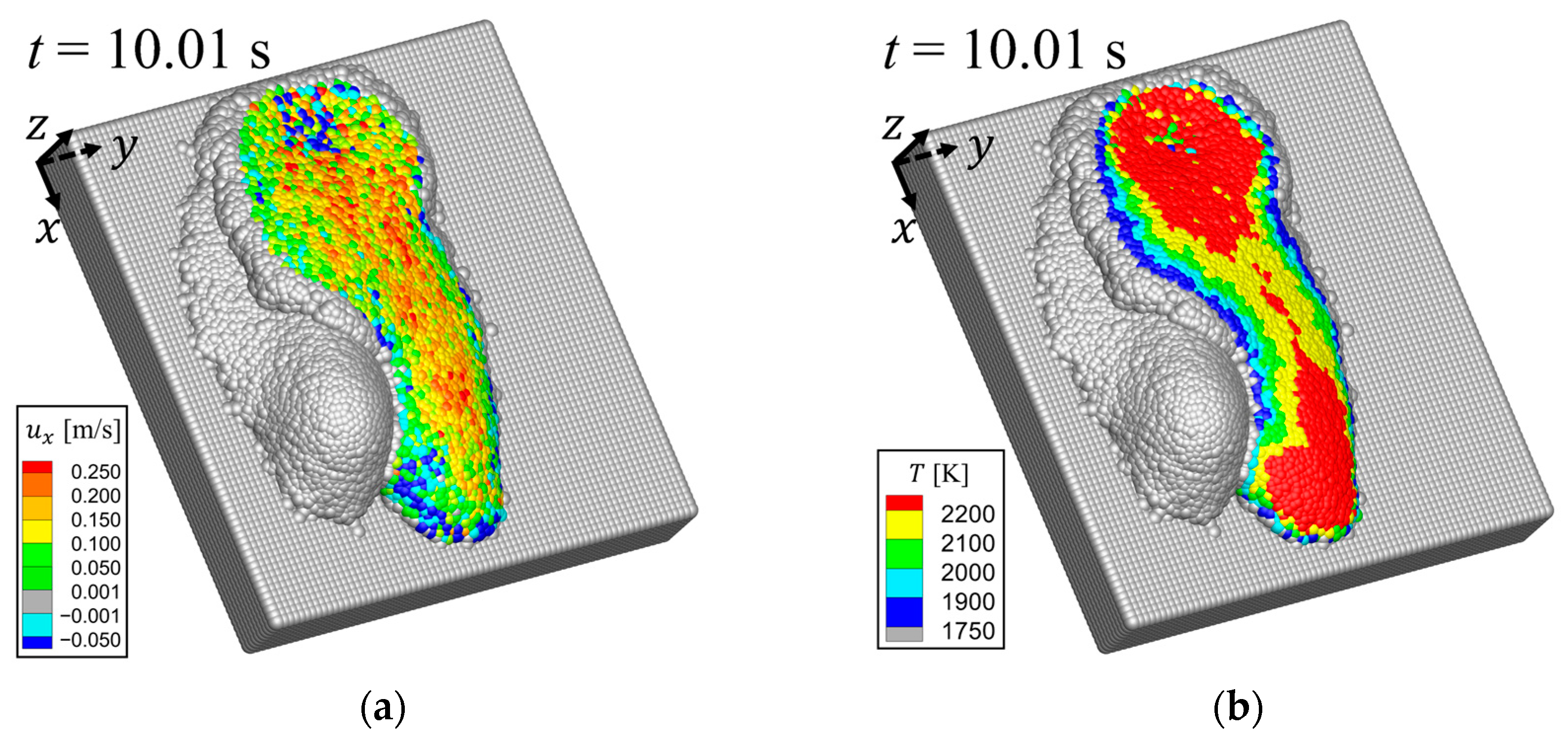
3.1. Molten Metal Behavior on Tilted Surface
3.2. Discussion of the Formation Process of Structures Unique to Lava Flow
3.2.1. Levee-like Structure
3.2.2. Shape of Tip
4. Conclusions
- The levee-like shape was formed during the molten metal flowing down in this simulation. The outer edge of the flow was solidified and stationary due to its high cooling rate. On the other hand, the inside flowed as a liquid phase due to its low cooling rate. These flows resulted in formations that were qualitatively similar to the formations of lava levees in actual lava;
- The tip of the flow became rounded in this simulation. It was similar to the toe-like shape at the tip of lava. The tip became rounded and raised up after solidification. This was similar to the solidified tip of lava. It was also successfully simulated that molten metal flows on a surface that changes topographically due to the solidification of the molten metal.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gregg, T.K.P.; Fink, J.H. A laboratory investigation into the effects of slope on lava flow morphology. J. Volcanol. Geotherm. Res. 2000, 96, 145–159. [Google Scholar] [CrossRef]
- Miyamoto, H.; Crown, D.A. A simplified two-component model for the lateral growth of pahoehoe lobes. J. Volcanol. Geotherm. Res. 2006, 157, 331–342. [Google Scholar] [CrossRef]
- Bokharaeian, M.; Novák, L.; Csámer, Á. Rheological study of lava flow analog mixtures. Acta Geodyn. Geomater. 2023, 20, 11–18. [Google Scholar] [CrossRef]
- Hon, K.; Kauahikaua, J.; Denlinger, R.; Mackay, K. Emplacement and inflation of pahoehoe sheet flows: Observations and measurements of active lava flows on Kilauea volcano, Hawaii. Geol. Soc. Am. Bull. 1994, 106, 351–370. [Google Scholar] [CrossRef]
- Quareni, F.; Tallarico, A.; Dragoni, M. Modeling of the steady-state temperature field in lava flow levées. J. Volcanol. Geotherm. Res. 2004, 132, 241–251. [Google Scholar] [CrossRef]
- Tsepelev, I.; Ismail-Zadeh, A.; Melnik, O.; Korotkii, A. Numerical modeling of fluid flow with rafts: An application to lava flows. J. Geodyn. 2016, 97, 31–41. [Google Scholar] [CrossRef]
- Starodubtsev, I.; Vasev, P.; Starodubtseva, Y.; Tsepelev, I. Numerical simulation and visualization of lava flows. Sci. Vis. 2022, 14, 66–76. [Google Scholar] [CrossRef]
- Tsepelev, I.; Ismail-Zadeh, A.; Starodubtseva, Y.; Korotkii, A.; Melnik, O. Crust development inferred from numerical models of lava flow and its surface thermal measurements. Ann. Geophys. 2019, 62, VO226. [Google Scholar]
- Starodubtsev, I.S.; Starodubtseva, Y.V.; Tsepelev, I.A.; Ismail-Zadeh, A.T. Three-dimensional numerical modeling of lava dynamics using the smoothed particle hydrodynamics method. J. Volcanol. Seismol. 2023, 17, 175–186. [Google Scholar] [CrossRef]
- Zago, V.; Bilotta, G.; Cappello, A.; Dalrymple, R.A.; Fortuna, L.; Ganci, G.; Hérault, A.; Del Negro, C. Simulating complex fluids with smoothed particle hydrodynamics. Ann. Geophys. 2017, 60, 669–679. [Google Scholar] [CrossRef]
- Zago, V.; Bilotta, G.; Cappello, A.; Dalrymple, R.A.; Fortuna, L.; Ganci, G.; Hérault, A.; Del Negro, C. Preliminary validation of lava benchmark tests on the GPUSPH particle engine. Ann. Geophys. 2018, 62, 224–236. [Google Scholar] [CrossRef]
- Zago, V.; Bilotta, G.; Hérault, A.; Dalrymple, R.A.; Fortuna, L.; Cappello, A.; Ganci, G.; Del Negro, C. Semi-implicit 3D SPH on GPU for lava flows. J. Comput. Phys. 2018, 375, 854–870. [Google Scholar] [CrossRef]
- Hérault, A.; Bilotta, G.; Vicari, A.; Rustico, E.; Del Negro, C. Numerical simulation of lava flow using a GPU SPH model. Ann. Geophys. 2011, 54, 600–620. [Google Scholar]
- Bašić, M.; Blagojević, B.; Peng, C.; Bašić, J. Lagrangian differencing dynamics for time-independent non-Newtonian materials. Materials 2021, 14, 6210. [Google Scholar] [CrossRef]
- Peshkov, I.; Dumbser, M.; Boscheri, W.; Romenski, E.; Chiocchetti, S.; Ioriatti, M. Simulation of non-Newtonian viscoplastic flows with a unified first order hyperbolic model and a structure-preserving semi-implicit scheme. Comput. Fluids 2021, 224, 104963. [Google Scholar] [CrossRef]
- Peng, C.; Bašić, M.; Blagojević, B.; Bašić, J.; Wu, W. A Lagrangian differencing dynamics method for granular flow modeling. Comput. Geotech. 2021, 137, 104297. [Google Scholar] [CrossRef]
- Castillo-Sánchez, H.A.; de Souza, L.F.; Castelo, A. Numerical simulation of rheological models for complex fluids using hierarchical grids. Polymers 2022, 14, 4958. [Google Scholar] [CrossRef] [PubMed]
- Shao, S.; Lo, E.Y.M. Incompressible SPH method for simulating Newtonian and non-Newtonian flows with a free surface. Adv. Water Resour. 2003, 26, 787–800. [Google Scholar] [CrossRef]
- Laigle, D.; Lachamp, P.; Naaim, M. SPH-based numerical investigation of mudflow and other complex fluid flow interactions with structures. Comput. Geosci. 2007, 11, 297–306. [Google Scholar] [CrossRef]
- Pan, W.; Tartakovsky, A.M.; Monaghan, J.J. Smoothed particle hydrodynamics non-Newtonian model for ice-sheet and ice-shelf dynamics. J. Comput. Phys. 2013, 242, 828–842. [Google Scholar] [CrossRef]
- Bilotta, G.; Zago, V.; Centorrino, V.; Dalrymple, R.A.; Hérault, A.; Del Negro, C.; Saikali, E. A numerically robust, parallel-friendly variant of BiCGSTAB for the semi-implicit integration of the viscous term in smoothed particle hydrodynamics. J. Comput. Phys. 2022, 466, 111413. [Google Scholar] [CrossRef]
- Shi, H.; Huang, Y. A GPU-based δ-plus-SPH model for non-Newtonian multiphase flows. Water 2022, 14, 1734. [Google Scholar] [CrossRef]
- Lan, Y.; Zhang, Y.; Tian, W.; Su, G.H.; Qiu, S. An enhanced implicit viscosity ISPH method for simulating free-surface flow coupled with solid-liquid phase change. J. Comput. Phys. 2023, 474, 111809. [Google Scholar] [CrossRef]
- Dingwell, D.B.; Webb, S.L. Structural relaxation in silicate melts and non-Newtonian melt rheology in geologic processes. Phys. Chem. Miner. 1989, 16, 508–516. [Google Scholar] [CrossRef]
- Lejeune, A.; Richet, P. Rheology of crystal-bearing silicate melts: An experimental study at high viscosities. J. Geophys. Res. 1995, 100, 4215–4229. [Google Scholar] [CrossRef]
- Monaghan, J.J. Smoothed particle hydrodynamics. Annu. Rev. Astron. Astrophys. 1992, 30, 543–574. [Google Scholar] [CrossRef]
- Koshizuka, S.; Oka, Y. Moving-particle semi-implicit method for fragmentation of incompressible fluid. Nucl. Sci. Eng. 1996, 123, 421–434. [Google Scholar] [CrossRef]
- Ito, M.; Izawa, S.; Fukunishi, Y.; Shigeta, M. Numerical simulation of a weld pool formation in a TIG welding using an incompressible SPH method. Q. J. Jpn. Weld. Soc. 2014, 32, 213–222. (In Japanese) [Google Scholar] [CrossRef][Green Version]
- Shigeta, M.; Watanabe, T.; Izawa, S.; Fukunishi, Y. Incompressible SPH simulation of double-diffusive convection phenomena. Int. J. Emerg. Multidiscip. Fluid Sci. 2009, 1, 1–18. [Google Scholar] [CrossRef]
- Ito, M.; Nishio, Y.; Izawa, S.; Fukunishi, Y.; Shigeta, M. Numerical simulation of joining process in a TIG welding system using incompressible SPH method. Q. J. Jpn. Weld. Soc. 2015, 33, 34s–38s. [Google Scholar] [CrossRef]
- Komen, H.; Shigeta, M.; Tanaka, M. Numerical simulation of molten metal droplet transfer and weld pool convection during gas metal arc welding using incompressible smoothed particle hydrodynamics method. Int. J. Heat Mass Transf. 2018, 121, 978–985. [Google Scholar] [CrossRef]
- Tsujimura, Y. Gas Metal arc Yousetsu ni okeru Kinzokujouki wo Tomonau arc Genshou to sono Netsugentokusei ni Kansuru Kenkyu. Ph.D. Thesis, Osaka University, Osaka, Japan, 2012. (In Japanese). [Google Scholar]
- Komen, H.; Matsui, S.; Konishi, K.; Shigeta, M.; Tanaka, M.; Kamo, T. Modeling of submerged arc welding phenomena and experimental study of the heat source characteristics. Q. J. Jpn. Weld. Soc. 2017, 35, 93–101. (In Japanese) [Google Scholar] [CrossRef][Green Version]
- Sahoo, P.; Debroy, T.; McNallan, M.J. Surface tension of binary metal—Surface active solute systems under conditions relevant to welding metallurgy. Metall. Trans. B 1988, 19, 483–491. [Google Scholar] [CrossRef]
- Komen, H.; Shigeta, M.; Tanaka, M.; Nakatani, M.; Abe, Y. Numerical simulation of slag forming process during submerged arc welding using DEM-ISPH hybrid method. Weld. World 2018, 62, 1323–1330. [Google Scholar] [CrossRef]
- Fukazawa, T.; Tanaka, K.; Komen, H.; Shigeta, M.; Tanaka, M.; Murphy, A.B. Numerical investigation for dominant factors in slag transfer and deposition process during metal active gas welding using incompressible smoothed particle hydrodynamics method. Q. J. Jpn. Weld. Soc. 2021, 39, 277–290. [Google Scholar] [CrossRef]
- Hawaiian Regional Geology. Available online: https://www.flickr.com/photos/rschott/310299013/in/photostream/ (accessed on 15 April 2024).



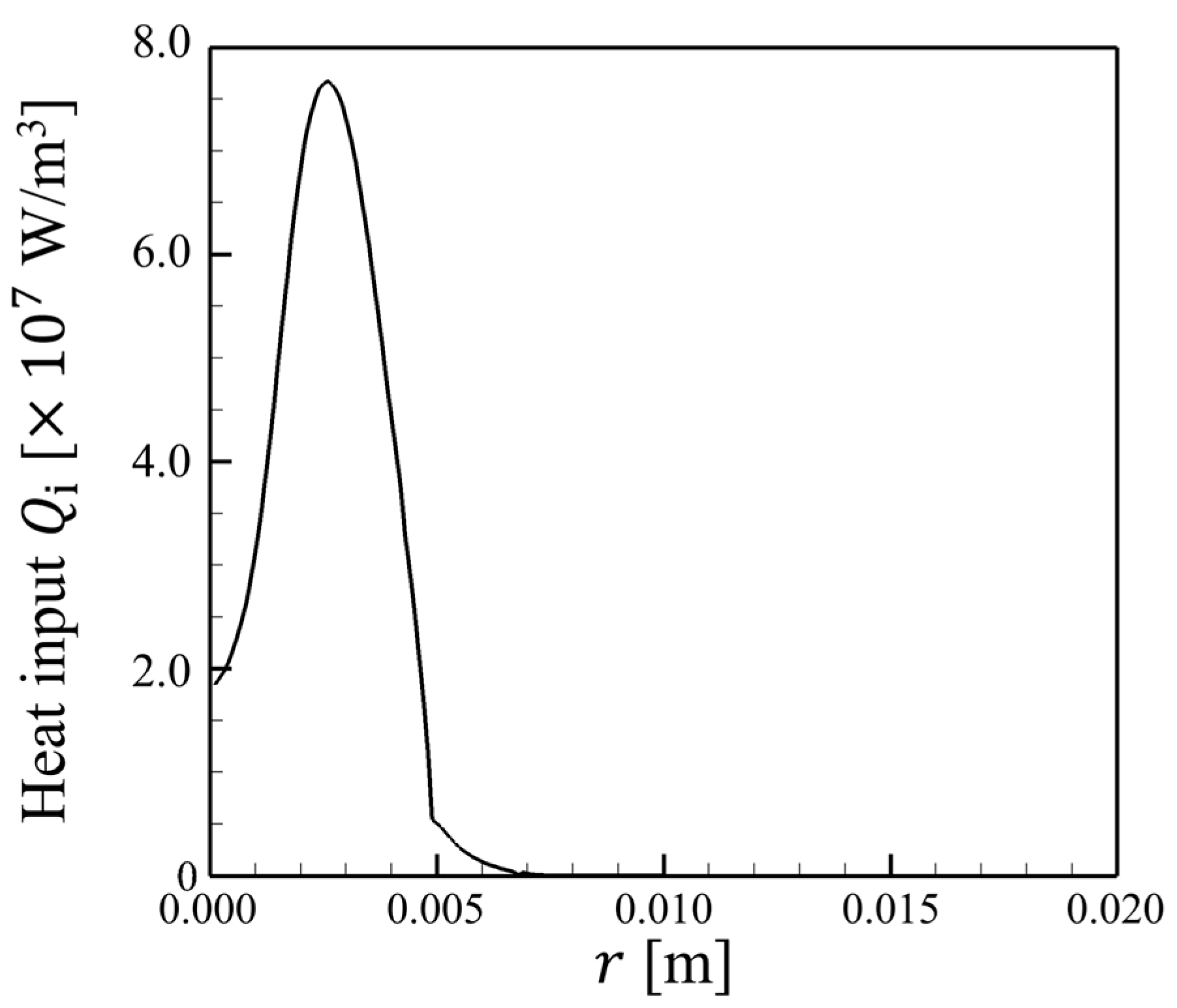






| Property | Parameter |
|---|---|
| Density | 7850 kg·m−3 |
| Melting point | 1750 K |
| Viscosity | 4.0 × 10−3 Pa·s |
| Thermal conductivity | 30.0∼73.0 W·m−1·K−1 |
| Specific heat | 0.44∼1.04 kJ·kg−1·K−1 |
| Latent heat | 270 kJ·kg−1 |
| Emissivity | 0.1 |
| Thermal expansion | 5.54 × 10−5 K−1 |
| Surface tension | 1.0 N·m−1 |
| Temperature gradient in surface tension | −1.0 × 10−4 N·m−1·K−1 |
| Property | Parameter |
|---|---|
| Diameter of computational particle | 5.0 × 10−4 m |
| Time step width | 1.0 × 10−4 s |
| Gravitational acceleration | 9.8 m·s−2 |
| Thermal conductivity of the gas | 30.0∼89.5 W·m−1·K−1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tomita, S.; Yoshikawa, J.; Sugimoto, M.; Komen, H.; Shigeta, M. SPH Simulation of Molten Metal Flow Modeling Lava Flow Phenomena with Solidification. Dynamics 2024, 4, 287-302. https://doi.org/10.3390/dynamics4020017
Tomita S, Yoshikawa J, Sugimoto M, Komen H, Shigeta M. SPH Simulation of Molten Metal Flow Modeling Lava Flow Phenomena with Solidification. Dynamics. 2024; 4(2):287-302. https://doi.org/10.3390/dynamics4020017
Chicago/Turabian StyleTomita, Shingo, Joe Yoshikawa, Makoto Sugimoto, Hisaya Komen, and Masaya Shigeta. 2024. "SPH Simulation of Molten Metal Flow Modeling Lava Flow Phenomena with Solidification" Dynamics 4, no. 2: 287-302. https://doi.org/10.3390/dynamics4020017
APA StyleTomita, S., Yoshikawa, J., Sugimoto, M., Komen, H., & Shigeta, M. (2024). SPH Simulation of Molten Metal Flow Modeling Lava Flow Phenomena with Solidification. Dynamics, 4(2), 287-302. https://doi.org/10.3390/dynamics4020017







