Entropy
Definition
1. Introduction
2. Basics
2.1. Definitions and Properties of Entropy
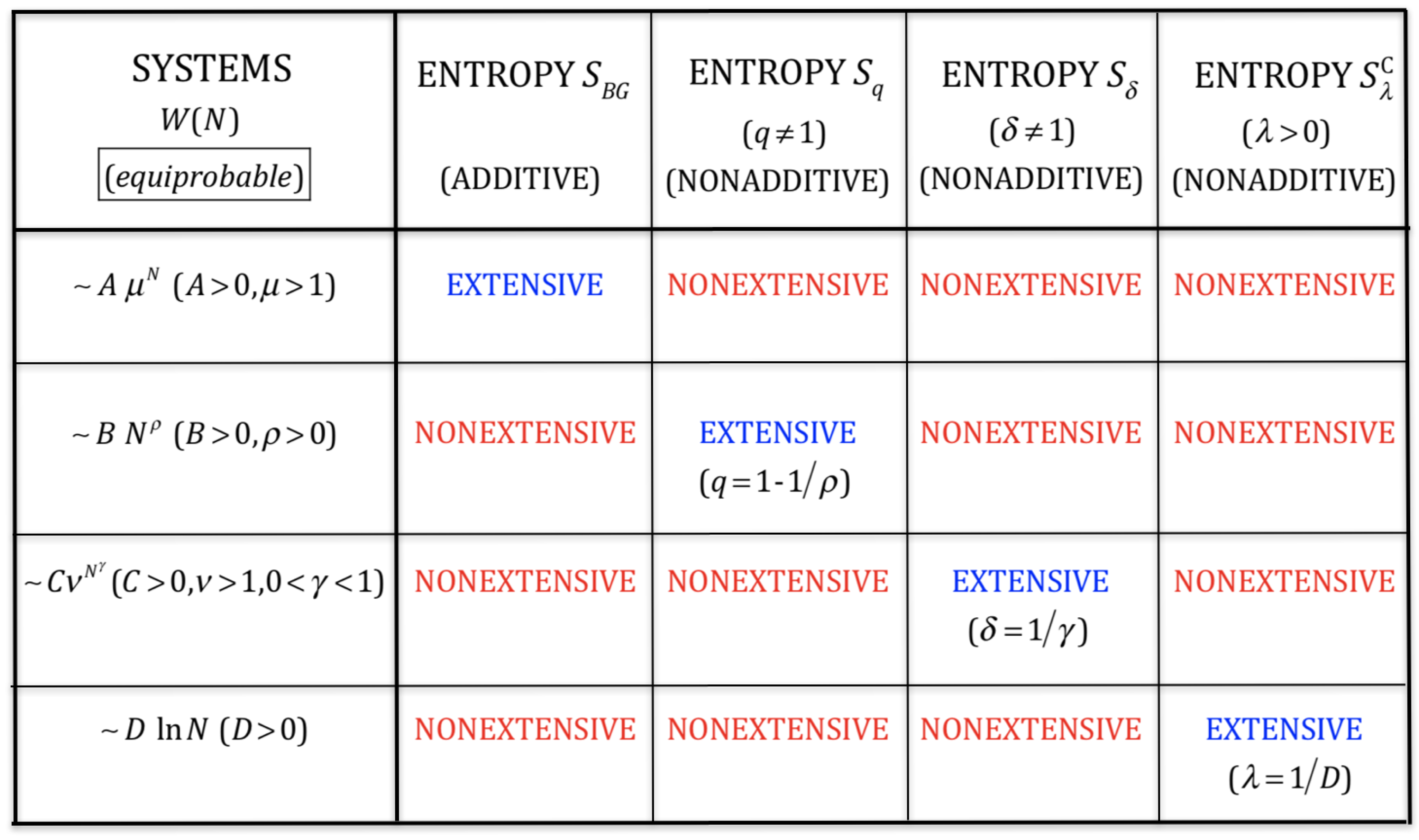
2.2. Additivity versus Extensivity
- Exponential class :This is the typical case within the BG theory. We have , therefore is extensive, as thermodynamically required.
- Power-law class :We should not use since it implies , thus violating thermodynamics. We verify instead that , as thermodynamically required.
- Stretched exponential class :In this instance, no value of q exists which would imply an extensive entropy . We can instead used with . Indeed, , as thermodynamically required.
- Logarithmic class :In this case, no values of exist which imply an extensive entropy . Instead, we can use the Curado entropy [33] with . Indeed, we can verify that , as thermodynamically required.
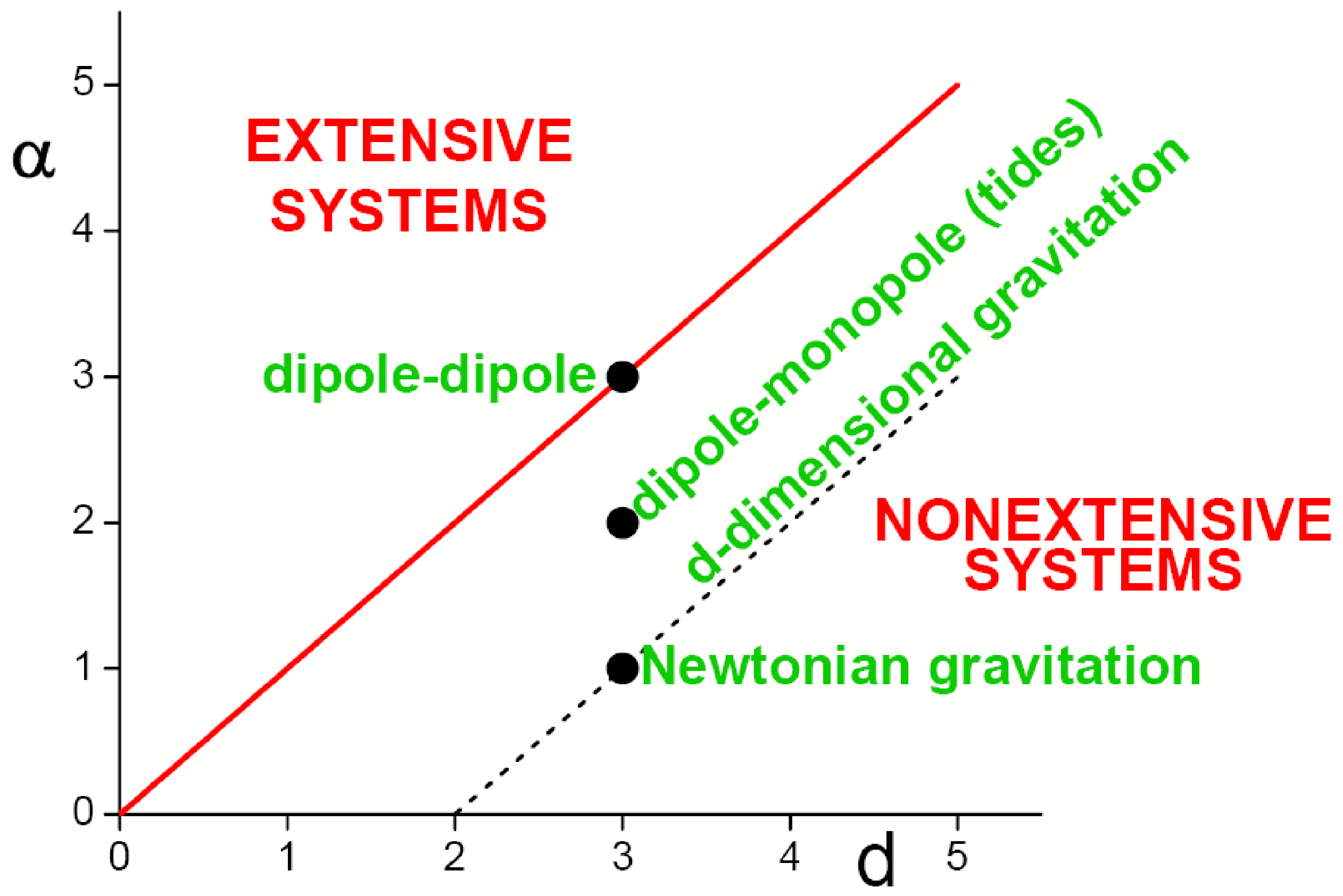
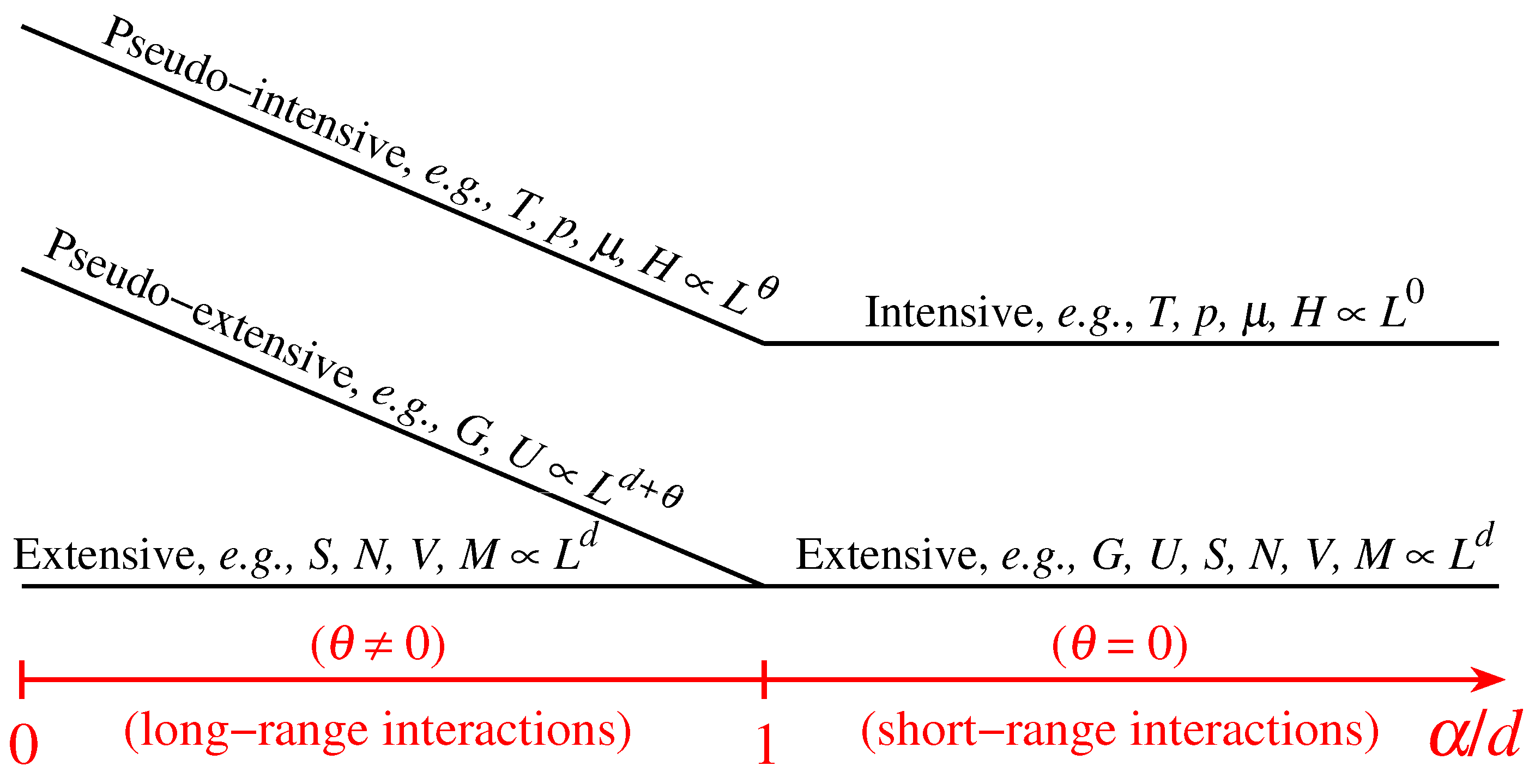
2.3. Range of Interactions
2.4. Thermodynamics and Legendre Transformations
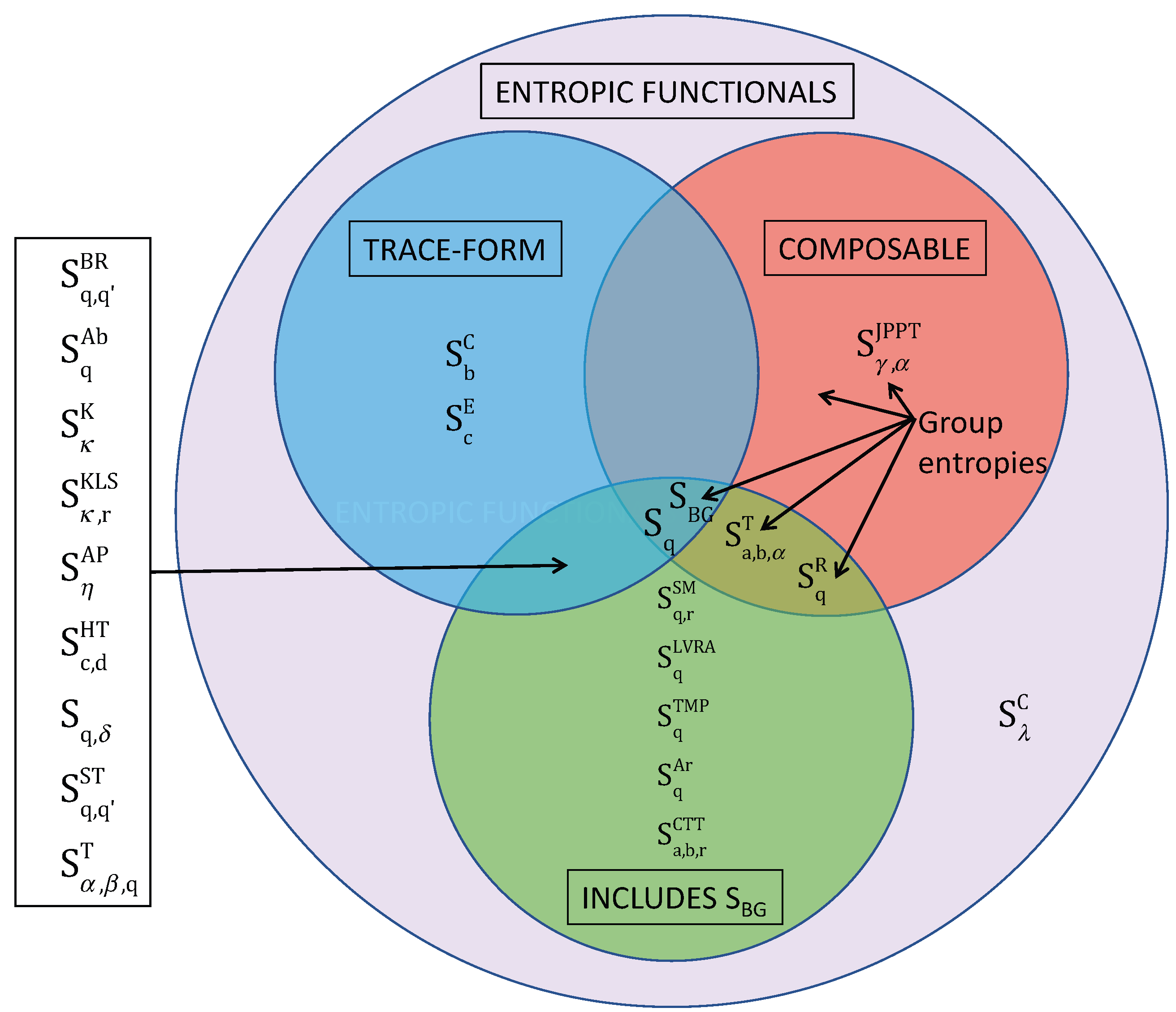
2.5. Classification of Entropic Functionals
2.6. Boltzmann–Gibbs and Nonextensive Statistical Mechanics
2.6.1. Boltzmann–Gibbs Statistical Mechanics
2.6.2. q-Generalization of the Boltzmann–Gibbs Theory
3. Results and Applications
3.1. In Physics
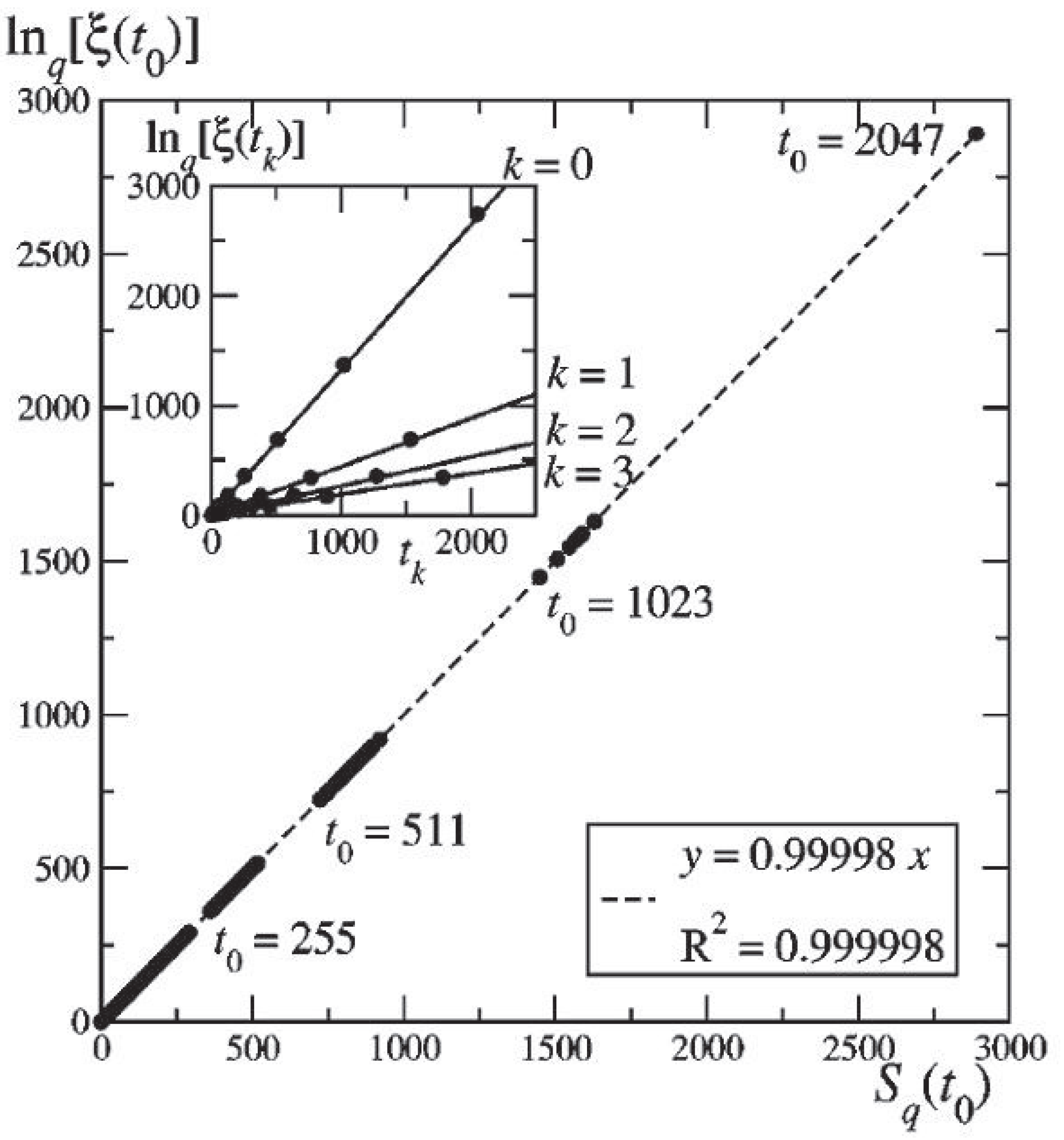
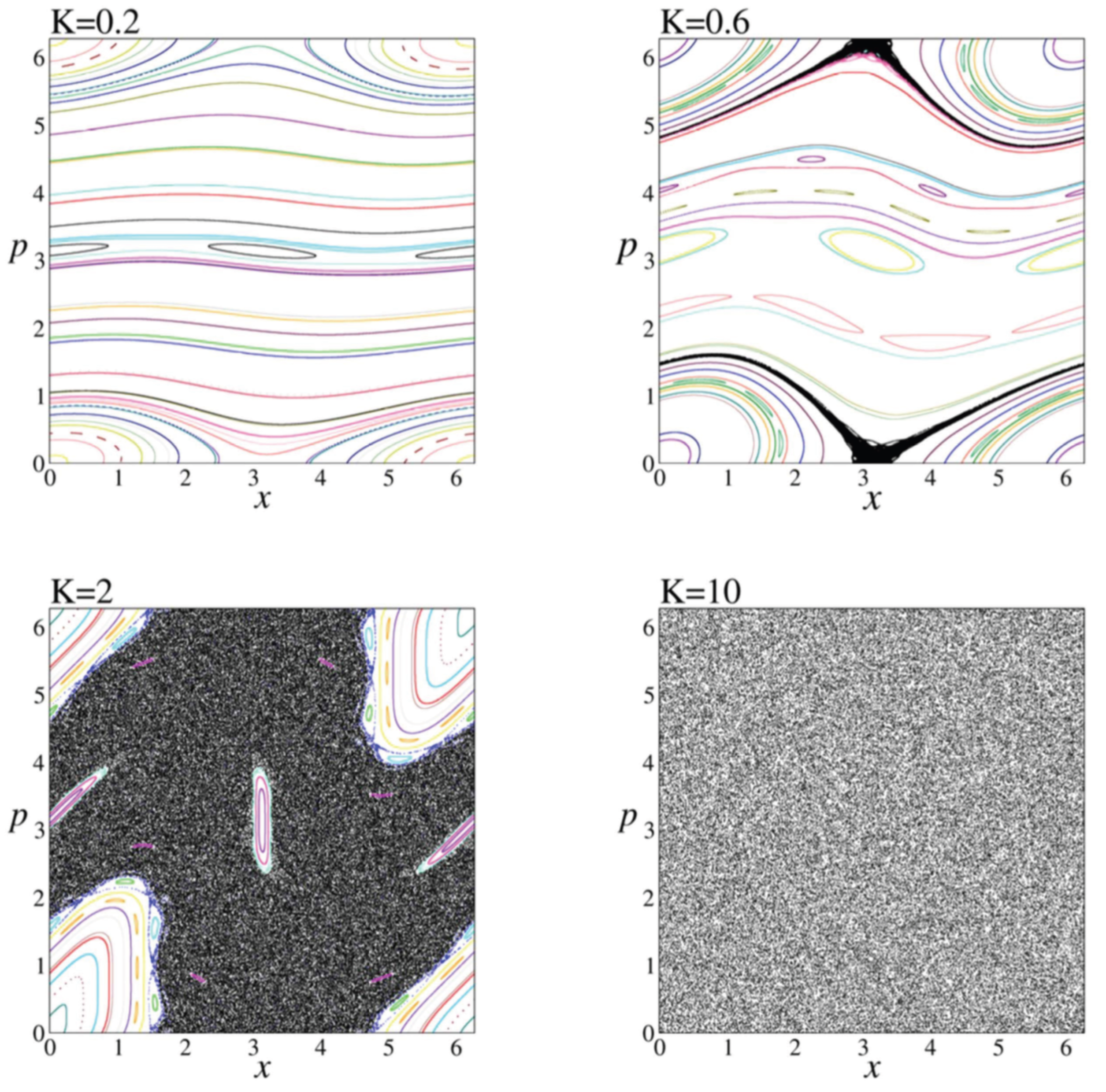
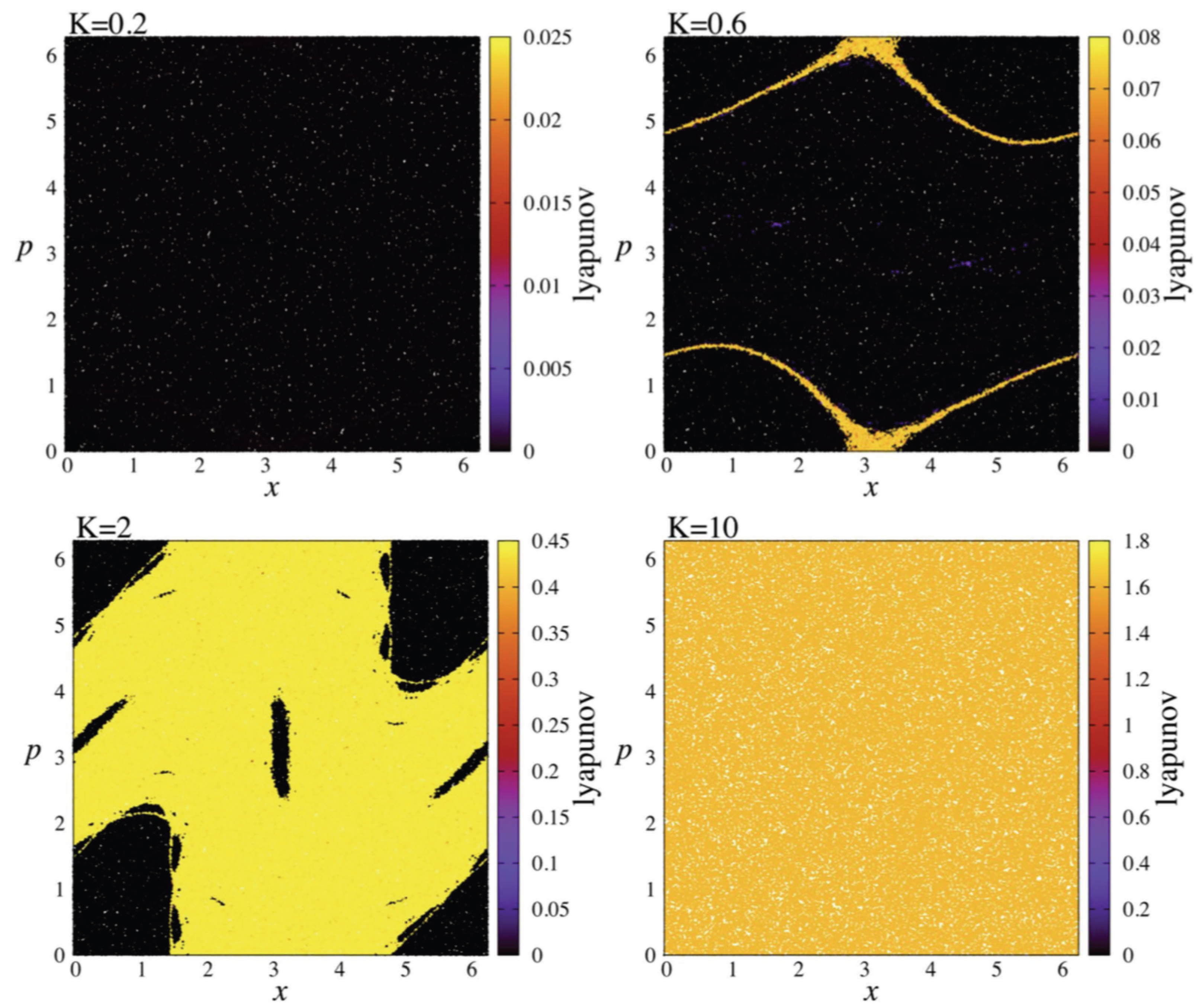
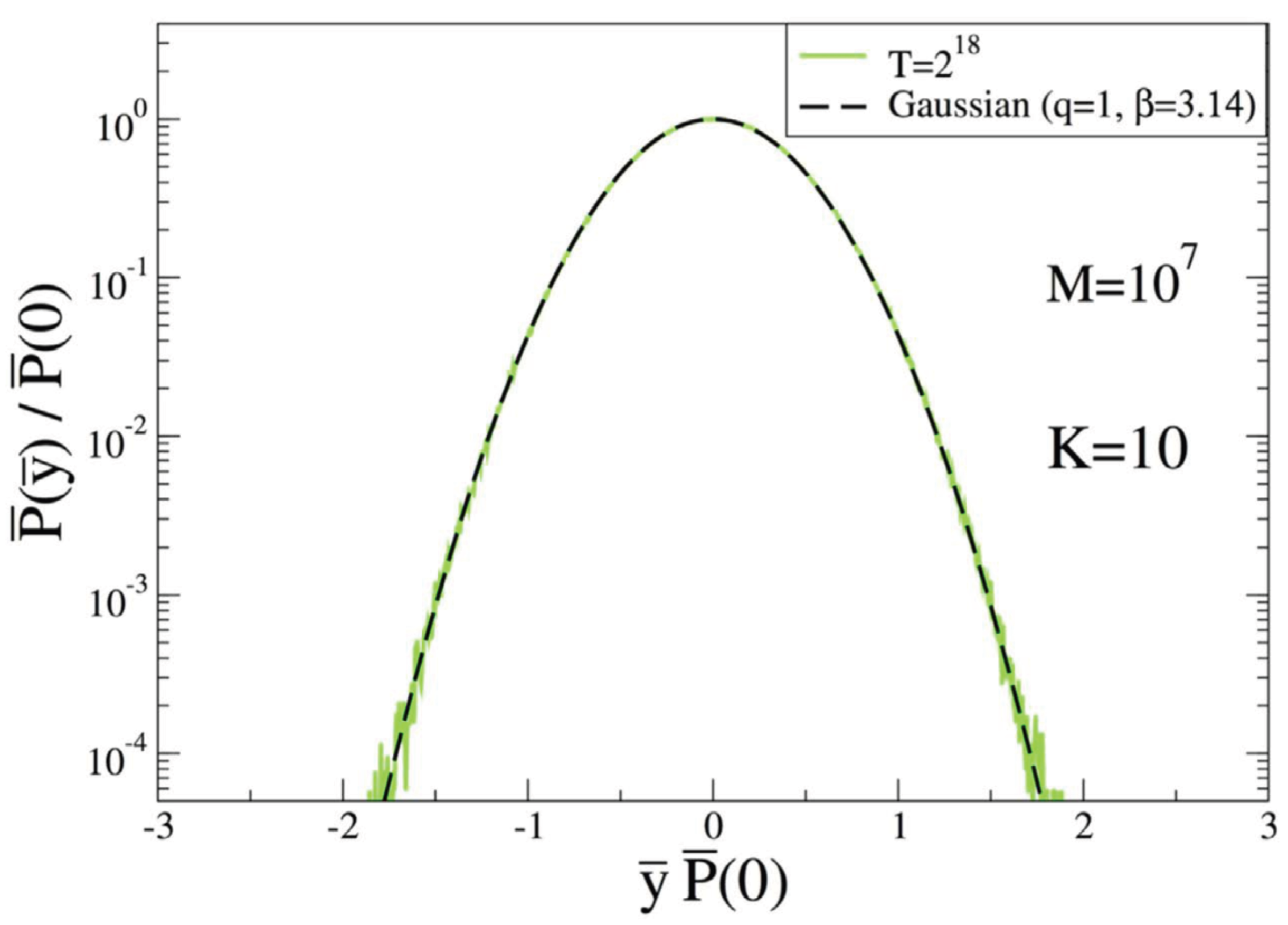
3.1.1. Nonlinear Dynamical Systems
3.1.2. First-Principle Calculation of q for a Quantum Hamiltonian System
3.1.3. Long-Range Interactions
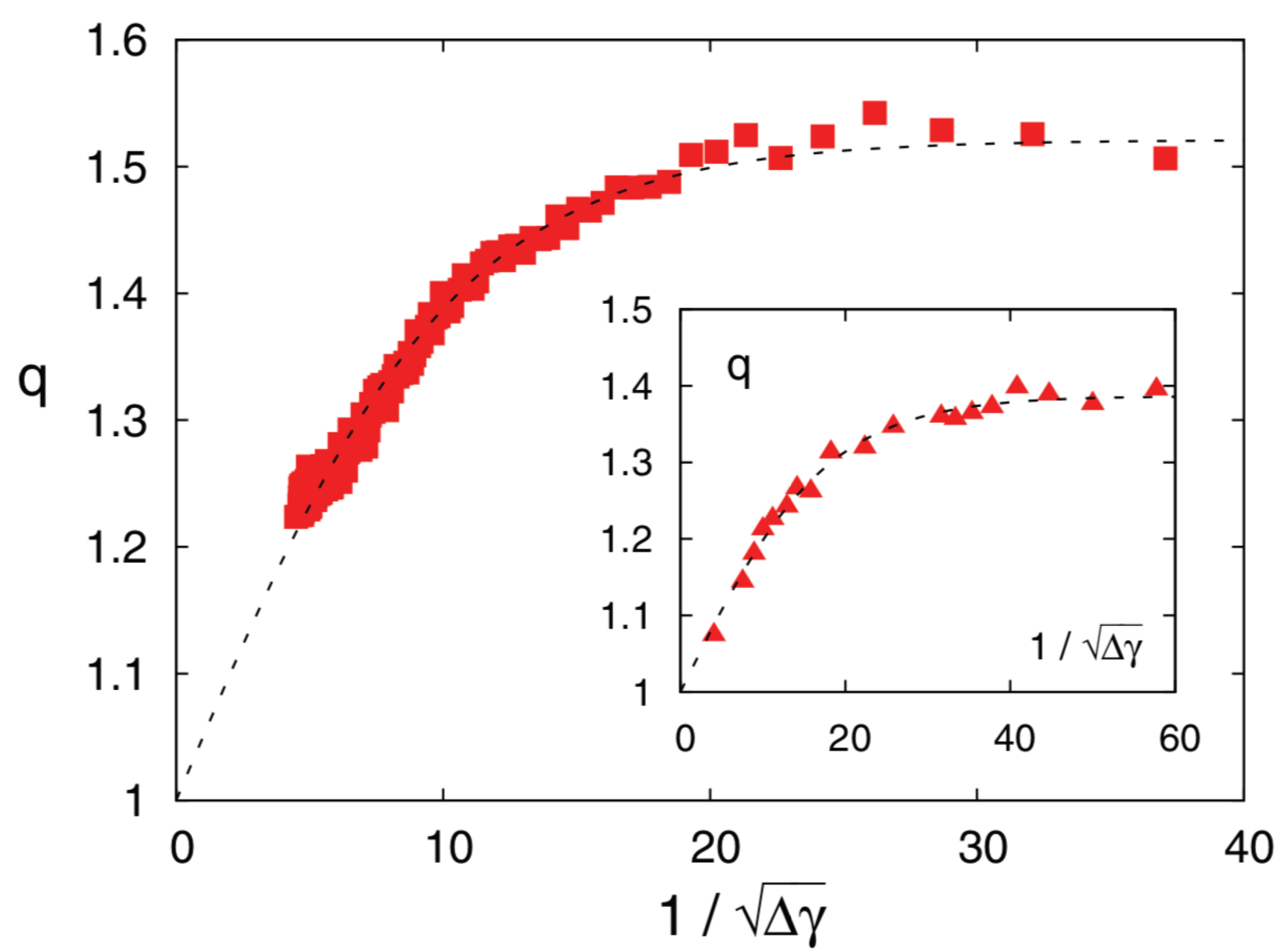
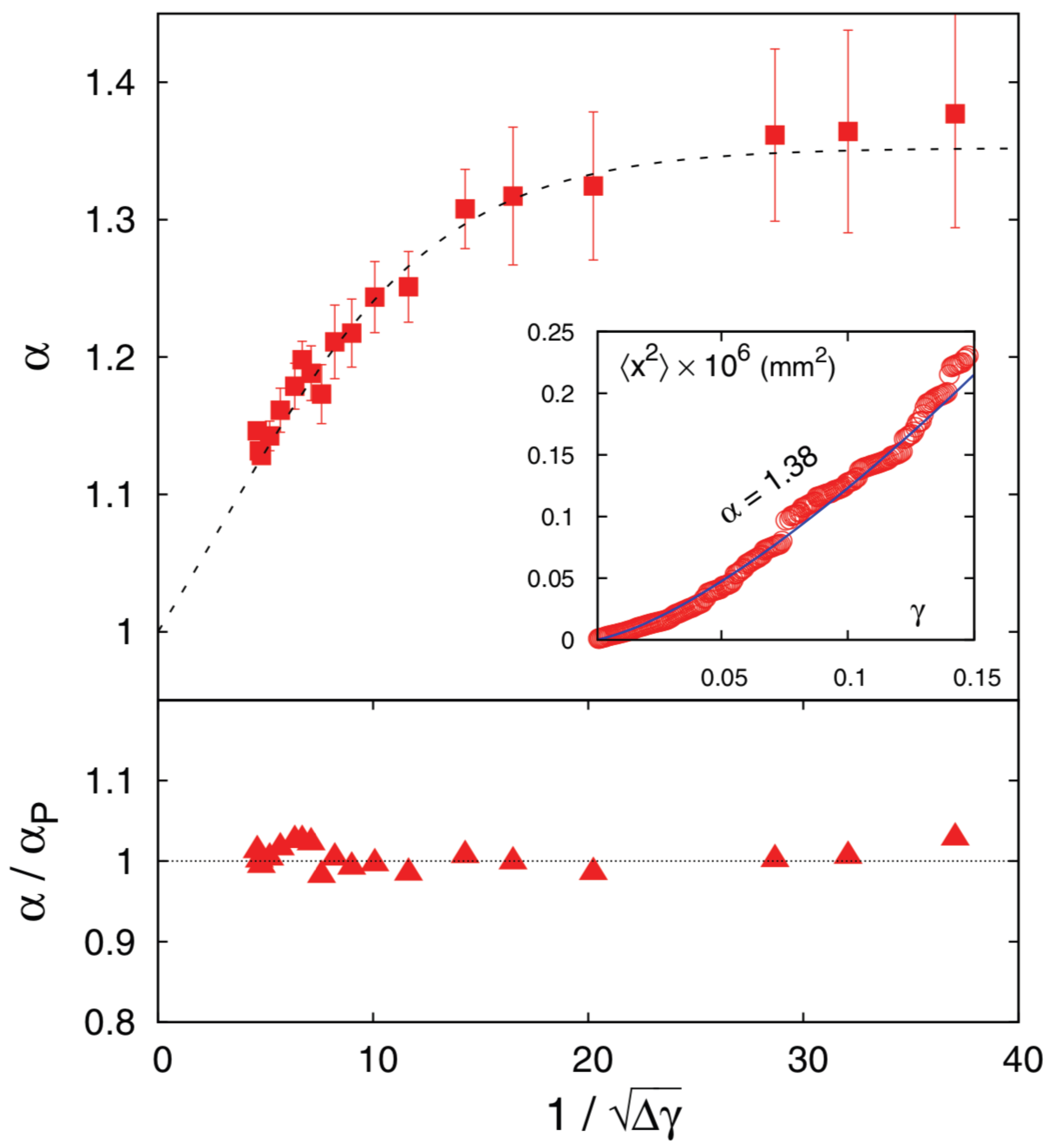
3.1.4. Overdamped Many-Body Systems
3.1.5. Low Energy Physics
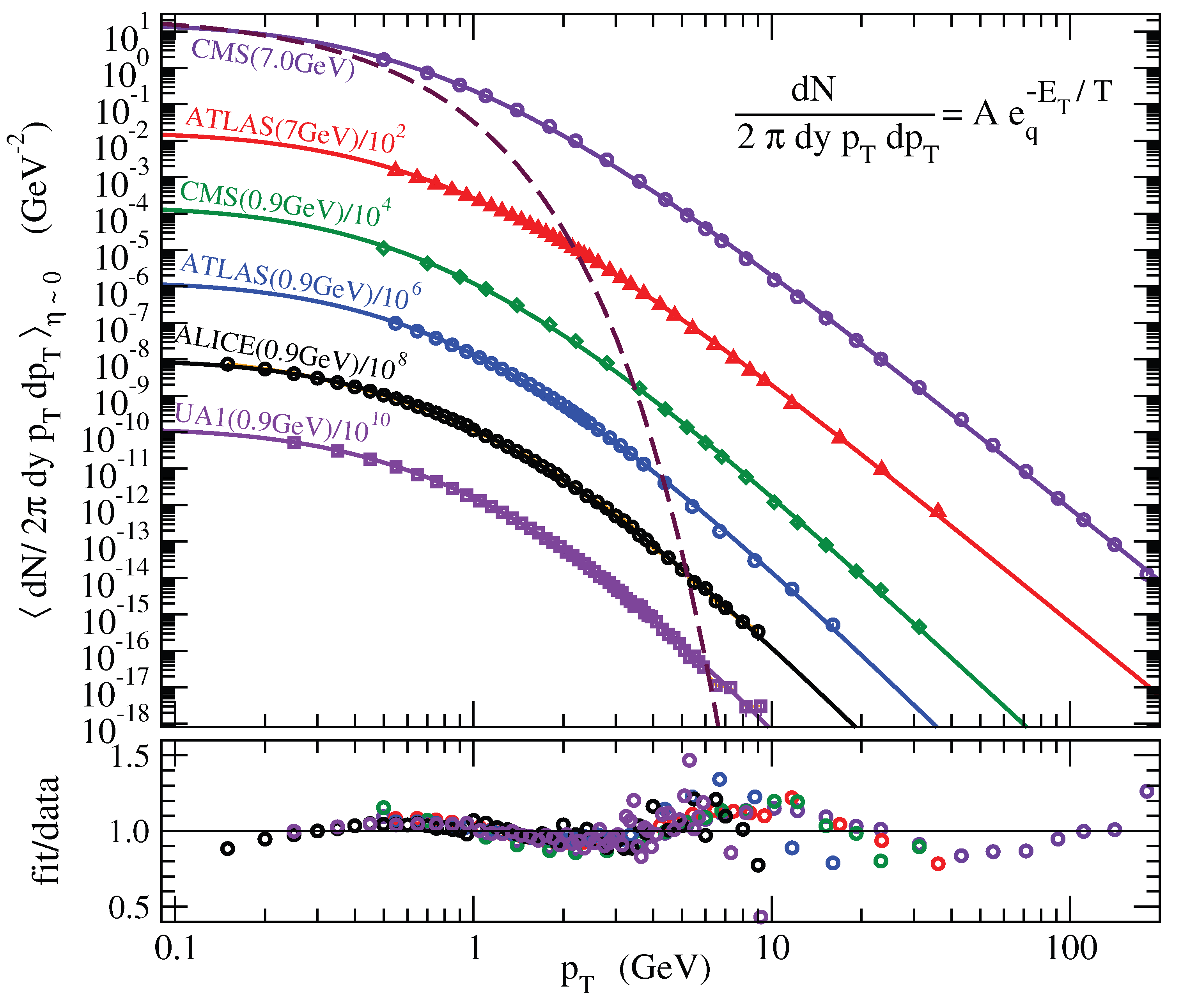
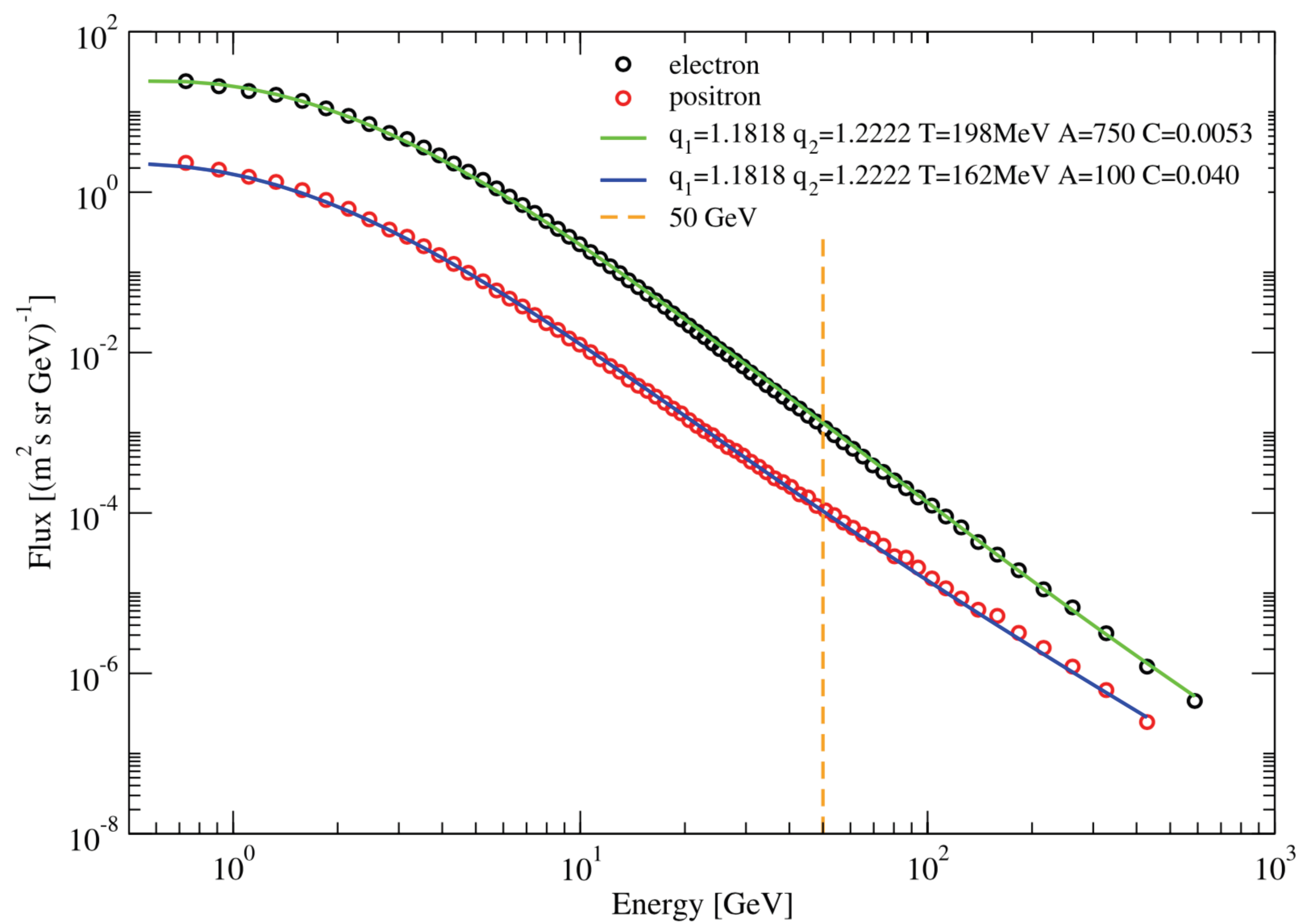
3.1.6. High Energy Physics
3.2. Beyond Physics
3.2.1. Mathematics
3.2.2. Chemistry
3.2.3. Economics
3.2.4. Biology and Life Sciences
3.2.5. Computer Sciences
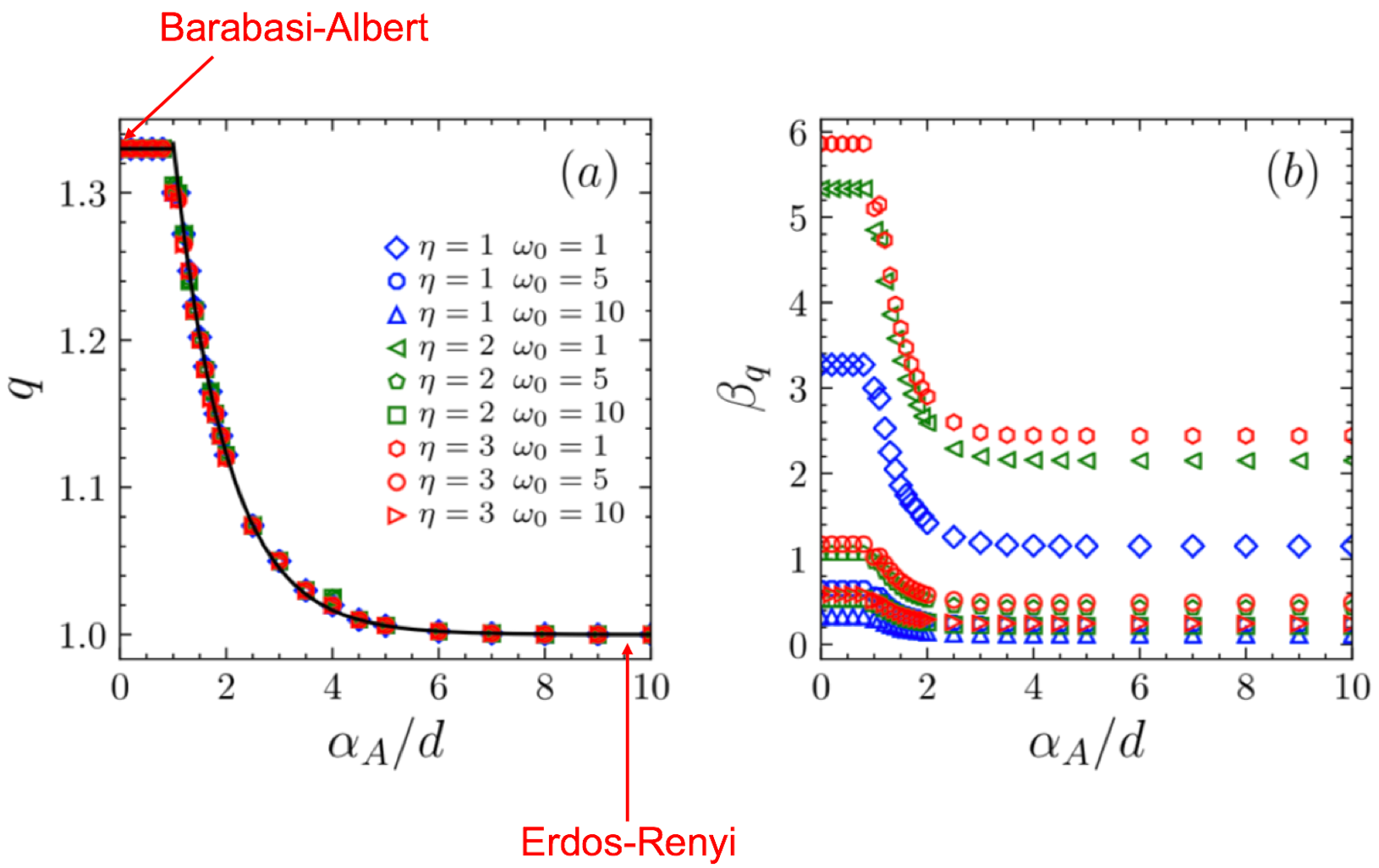
3.2.6. Random Networks
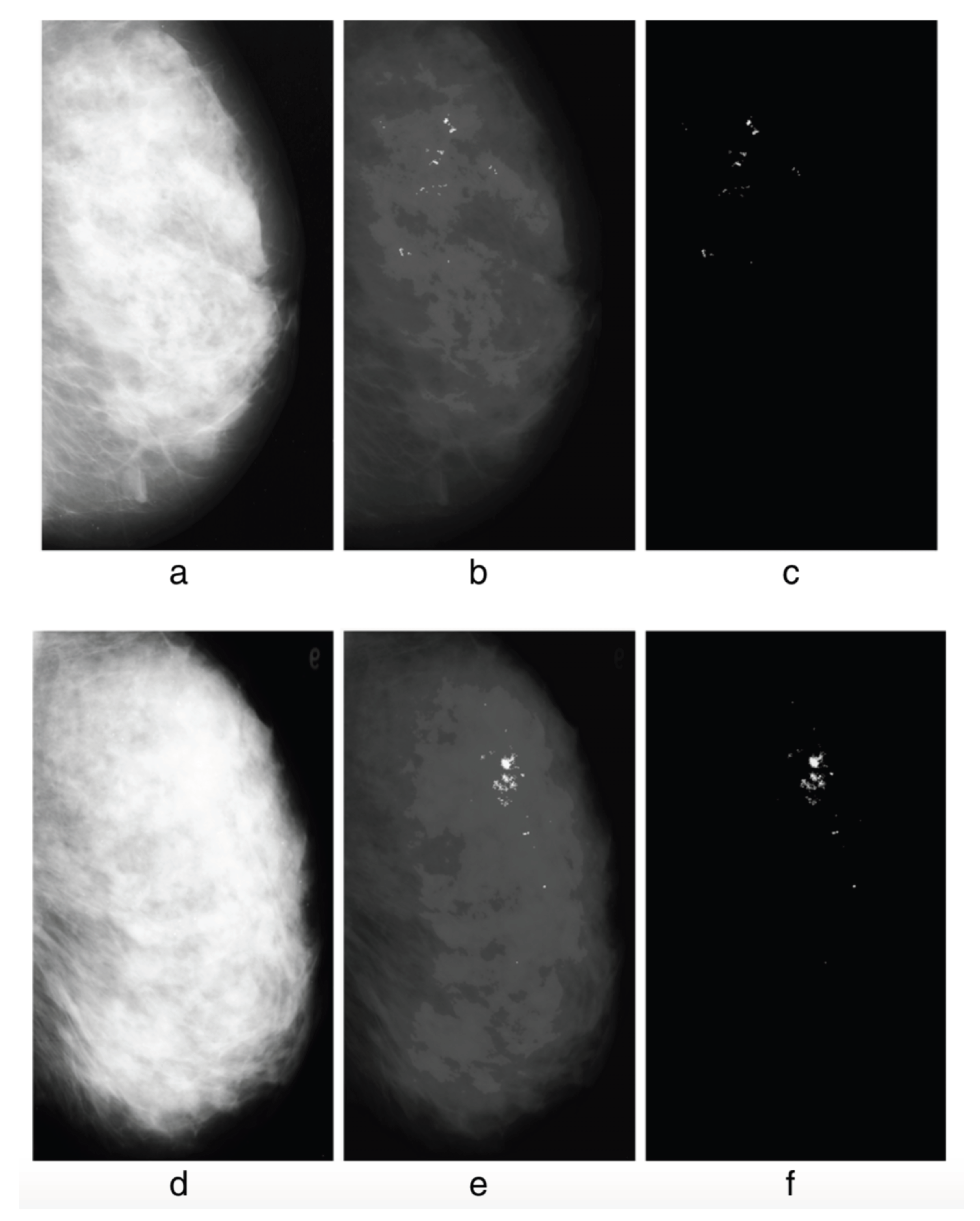
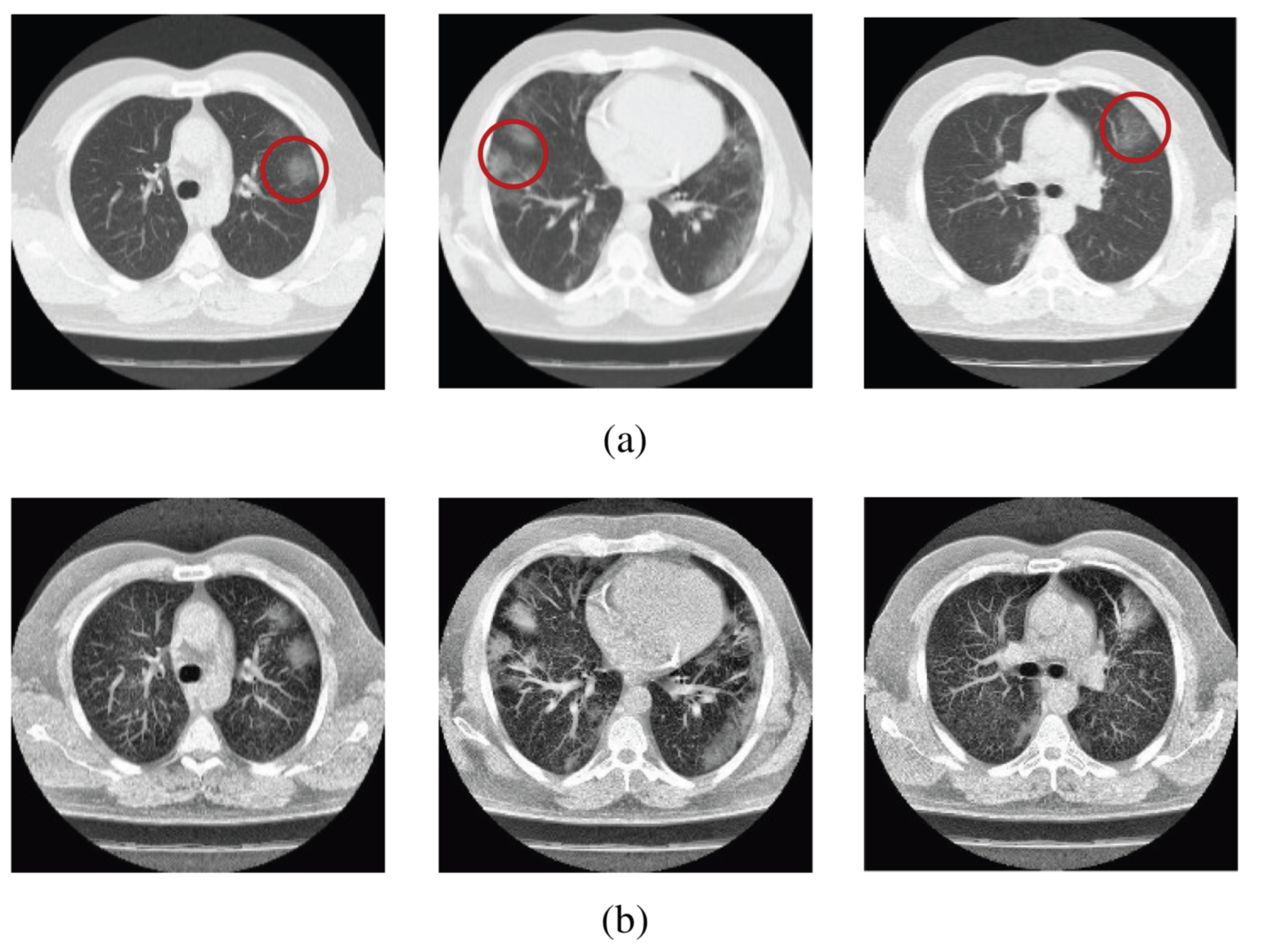
3.2.7. Image and Signal Processing
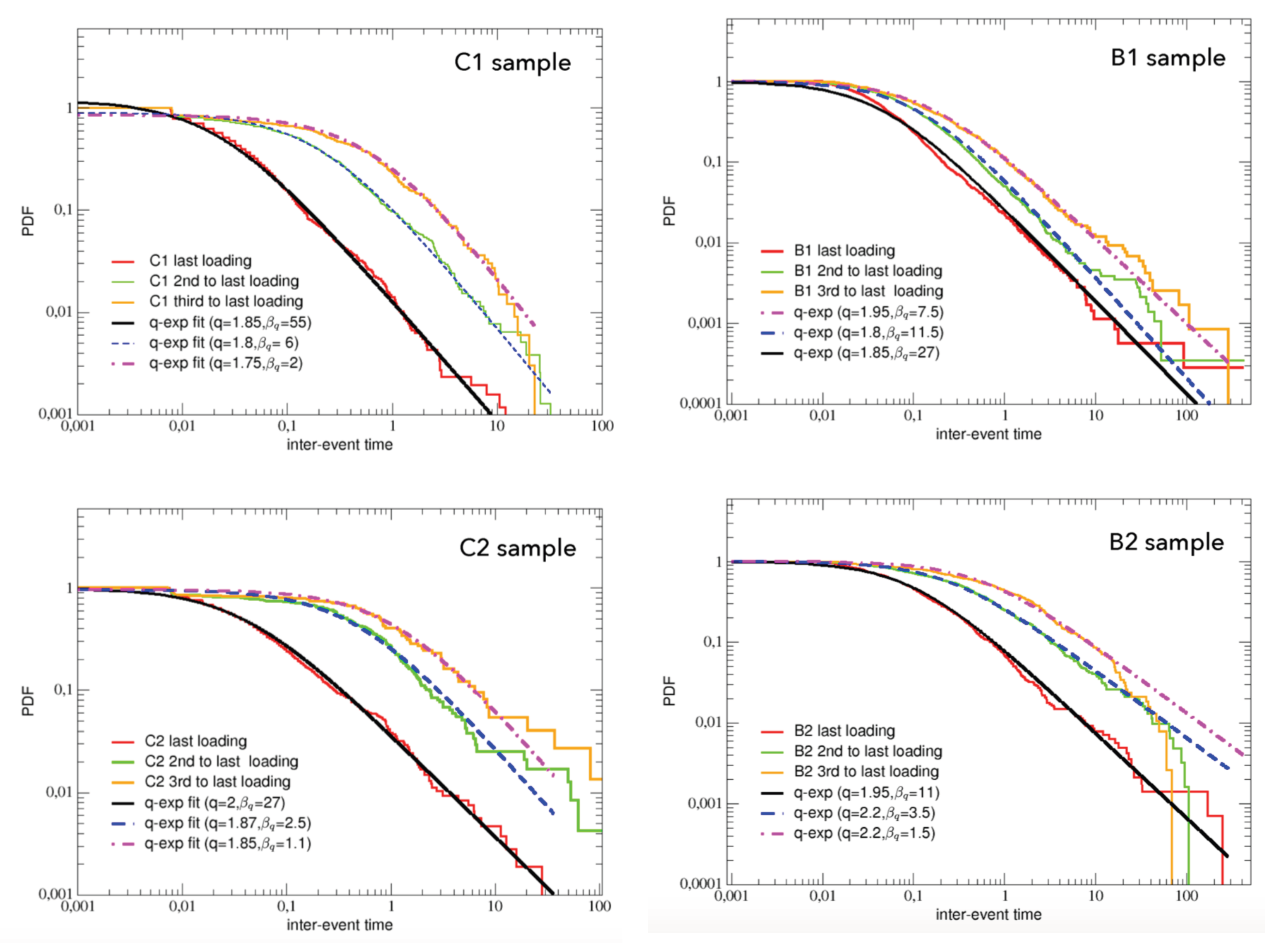
3.2.8. Engineering
4. Final Remarks
Funding
Acknowledgments
Conflicts of Interest
Entry Link on the Encyclopedia Platform
References
- Clausius, R. Uber die Wärmeleitung gasförmiger Körper. Ann. Phys. 1865, 125, 353–400. [Google Scholar] [CrossRef]
- Clausius, R. The Mechanical Theory of Heat with Its Applications to the Steam Engine and to Physical Properties of Bodies; John van Voorst, 1 Paternoster Row. MDCCCLXVII: London, UK, 1865. [Google Scholar]
- Boltzmann, L. Weitere Studien u̇ber das Wȧrmegleichgewicht unter Gas moleku̇len [Further Studies on Thermal Equilibrium Between Gas Molecules]. Wien. Ber. 1872, 66, 275. [Google Scholar]
- Boltzmann, L. Uber die Beziehung eines allgemeine mechanischen Satzes zum zweiten Haupsatze der Warmetheorie Sitzungsberichte, K. Akademie der Wissenschaften in Wien, Math. Naturwissenschaften 1877, 75, 67–73. [Google Scholar]
- Gibbs, J.W. Elementary Principles in Statistical Mechanics—Developed with Especial Reference to the Rational Foundation of Thermodynamics; C. Scribner’s Sons: New York, NY, USA, 1902. [Google Scholar]
- Gibbs, J.W. The collected works. In Thermodynamics; Yale University Press: New Haven, CT, USA, 1948; Volume 1. [Google Scholar]
- Gibbs, J.W. Elementary Principles in Statistical Mechanics; OX Bow Press: Woodbridge, CT, USA, 1981. [Google Scholar]
- von Neumann, J. Thermodynamik quantenmechanischer Gesamtheiten. Nachrichten Ges. Wiss. Gott. 1927, 1927, 273–291. [Google Scholar]
- Shannon, C.E. A mathematical theory of communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Shannon, C.E. A mathematical theory of communication. Bell Syst. Tech. J. 1948, 27, 623–656. [Google Scholar] [CrossRef]
- Shannon, C.E. The Mathematical Theory of Communication; University of Illinois Press: Urbana, IL, USA, 1949. [Google Scholar]
- Jaynes, E.T. Information theory and statistical mechanics. Phys. Rev. 1957, 106, 620–630. [Google Scholar] [CrossRef]
- Jaynes, E.T. Information theory and statistical mechanics. II. Phys. Rev. 1957, 108, 171–190. [Google Scholar] [CrossRef]
- Renyi, A. On measures of information and entropy. In Proceedings of the Fourth Berkeley Symposium; University of California Press: Los Angeles, CA, USA, 1961; Volume 1, pp. 547–561. [Google Scholar]
- Renyi, A. Probability Theory; Dover Publications Inc.: New York, NY, USA, 1970. [Google Scholar]
- Balatoni, J.; Renyi, A. Remarks on entropy. Publ. Math. Inst. Hung. Acad. Sci. 1956, 1, 9–40. [Google Scholar]
- Renyi, A. On the dimension and entropy of probability distributions. Acta Math. Acad. Sci. Hung. 1959, 10, 193–215. [Google Scholar] [CrossRef]
- Havrda, J.; Charvat, F. Quantification method of classification processes - Concept of structural α-entropy. Kybernetika 1967, 3, 30–35. [Google Scholar]
- Lindhard, J.; Nielsen, V. Det Kongelige Danske Videnskabernes Selskab Matematisk-fysiske Meddelelser (Denmark). Stud. Stat. Mech. 1971, 38, 1–42. [Google Scholar]
- Sharma, B.D.; Mittal, D.P. New non-additive measures of entropy for discrete probability distributions. J. Math. Sci. 1975, 10, 28. [Google Scholar]
- Sharma, B.D.; Taneja, I.J. Entropy of type (α,β) and other generalized measures in information theory. Metrika 1975, 22, 205. [Google Scholar] [CrossRef]
- Mittal, D.P. On some functional equations concerning entropy, directed divergence and inaccuracy. Metrika 1975, 22, 35. [Google Scholar] [CrossRef]
- Jaynes, E.T. Gibbs vs. Boltzmann entropies. Am. J. Phys. 1965, 33, 391–398. [Google Scholar] [CrossRef]
- Landauer, R. Information is physical. Phys. Today 1991, 44, 23. [Google Scholar] [CrossRef]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487, [First appeared as preprint in 1987: CBPF-NF-062/87, ISSN 0029–3865, Centro Brasileiro de Pesquisas Fisicas, Rio de Janeiro]. [Google Scholar] [CrossRef]
- Tsallis, C. Nonextensive Statistical Mechanics—Approaching a Complex World, 1st ed.; Springer: New York, NY, USA, 2009. [Google Scholar]
- Watanabe, S. Knowing and Guessing; Wiley: New York, NY, USA, 1969. [Google Scholar]
- Barlow, H. Conditions for versatile learning, Helmholtz’s unconscious inference, and the task of perception. Vis. Res. 1990, 30, 1561. [Google Scholar] [CrossRef]
- Penrose, O. Foundations of Statistical Mechanics: A Deductive Treatment; Pergamon: Oxford, UK, 1970; p. 167. [Google Scholar]
- Tsallis, C.; Cirto, L.J.L. Black hole thermodynamical entropy. Eur. Phys. J. C 2013, 73, 2487. [Google Scholar] [CrossRef]
- Ubriaco, M.R. Entropies based on fractional calculus. Phys. Lett. A 2009, 373, 2516. [Google Scholar] [CrossRef]
- Lima, H.S.; Tsallis, C. Exploring the neighborhood of q-exponentials. Entropy 2020, 22, 1402. [Google Scholar] [CrossRef]
- Tsallis, C. Nonextensive Statistical Mechanics—Approaching a Complex World, 2nd ed.; Springer: New York, NY, USA, 2022; in press. [Google Scholar]
- Holton, G.; Elkana, Y. (Eds.) Albert Einstein: Historical and Cultural Perspectives; Dover Publications: Mineola, NY, USA, 1997; p. 227. [Google Scholar]
- Antoni, M.; Ruffo, S. Clustering and relaxation in Hamiltonian long-range dynamics. Phys. Rev. E 1995, 52, 2361–2374. [Google Scholar] [CrossRef]
- Anteneodo, C.; Tsallis, C. Breakdown of the exponential sensitivity to the initial conditions: Role of the range of the interaction. Phys. Rev. Lett. 1998, 80, 5313. [Google Scholar] [CrossRef]
- Nobre, F.D.; Tsallis, C. Classical infinite-range-interaction Heisenberg ferromagnetic model: Metastability and sensitivity to initial conditions. Phys. Rev. E 2003, 68, 036115. [Google Scholar] [CrossRef]
- Rodriguez, A.; Nobre, F.D.; Tsallis, C. d-Dimensional classical Heisenberg model with arbitrarily-ranged interactions: Lyapunov exponents and distributions of momenta and energies. Entropy 2019, 21, 31. [Google Scholar] [CrossRef]
- Rodriguez, A.; Nobre, F.D.; Tsallis, C. Quasi-stationary-state duration in d-dimensional long-range model. Phys. Rev. Res. 2020, 2, 023153. [Google Scholar] [CrossRef]
- Dauxois, T. Fermi, Pasta, Ulam, and a mysterious lady. Phys. Today 2008, 6, 55–57. [Google Scholar] [CrossRef]
- Bagchi, D.; Tsallis, C. Fermi-Pasta-Ulam-Tsingou problems: Passage from Boltzmann to q-statistics. Phys. A Stat. Mech. Appl. 2018, 491, 869–873. [Google Scholar] [CrossRef]
- Christodoulidi, H.; Tsallis, C.; Bountis, T. Fermi-Pasta-Ulam model with long-range interactions: Dynamics and thermostatistics. EPL 2014, 108, 40006. [Google Scholar] [CrossRef]
- Christodoulidi, H.; Bountis, T.; Tsallis, C.; Drossos, L. Dynamics and Statistics of the Fermi–Pasta–Ulam β–model with different ranges of particle interactions. J. Stat. Mech. Theory Exp. 2016, 2016, 123206. [Google Scholar] [CrossRef]
- Bagchi, D.; Tsallis, C. Sensitivity to initial conditions of d-dimensional long-range-interacting quartic Fermi-Pasta-Ulam model: Universal scaling. Phys. Rev. E 2016, 93, 062213. [Google Scholar] [CrossRef]
- Landsberg, P.T. Thermodynamics and Statistical Mechanics; Oxford University Press: New York, NY, USA, 1978. [Google Scholar]
- Landsberg, P.T. Thermodynamics and Statistical Mechanics; Dover: New York, NY, USA, 1990. [Google Scholar]
- Tsallis, C.; Cirto, L.J.L. Thermodynamics is more powerful than the role to it reserved by Boltzmann-Gibbs statistical mechanics. Eur. Phys. J. Spec. Top. 2014, 223, 2161. [Google Scholar] [CrossRef][Green Version]
- Tsallis, C. Approach of complexity in nature: Entropic nonuniqueness. Axioms 2016, 5, 20. [Google Scholar] [CrossRef]
- Tsallis, C. Beyond Boltzmann-Gibbs-Shannon in physics and elsewhere. Entropy 2019, 21, 696. [Google Scholar] [CrossRef]
- Tsallis, C. Nonextensive statistics: Theoretical, experimental and computational evidences and connections. Braz. J. Phys. 1999, 29, 1–35. [Google Scholar] [CrossRef]
- Tsallis, C. Talk at the IMS Winter School on Nonextensive Generalization of Boltzmann-Gibbs Statistical Mechanics and Its Applications; Institute for Molecular Science: Okazaki, Japan, 1999. [Google Scholar]
- Tsallis, C. Nonextensive Statistical Mechanics and Thermodynamics: Historical Background and Present Status. In Nonextensive Statistical Mechanics and Its Applications; Abe, S., Okamoto, Y., Eds.; Series Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Hotta, M.; Joichi, I. Composability and generalized entropy. Phys. Lett. A 1999, 262, 302. [Google Scholar] [CrossRef]
- Enciso, A.; Tempesta, P. Uniqueness and characterization theorems for generalized entropies. J. Stat. Mech. 2017, 2017, 123101. [Google Scholar] [CrossRef]
- Tsallis, C. Entropy. In Springer Encyclopedia of Complexity and Systems Science; Springer: Berlin/Heidelberg, Germany, 2008; pp. 2859–2883. [Google Scholar]
- Chafai, D. Entropies, convexity, and functional inequalities–On Φ-entropies and Φ-Sobolev inequalities. J. Math. Kyoto Univ. 2004, 44, 325–363. [Google Scholar] [CrossRef]
- Tempesta, P. Formal groups and Z-entropies. Proc. R. Soc. A 2016, 472, 20160143. [Google Scholar] [CrossRef]
- Jensen, H.J.; Pazuki, R.H.; Pruessner, G.; Tempesta, P. Statistical mechanics of exploding phase spaces: Ontic open systems. J. Phys. A Math. Theor. 2018, 51, 375002. [Google Scholar] [CrossRef]
- Landsberg, P.T.; Vedral, V. Distributions and channel capacities in generalized statistical mechanics. Phys. Lett. A 1998, 247, 211. [Google Scholar] [CrossRef]
- Landsberg, P.T. Entropies galore! In Nonextensive Statistical Mechanics and Thermodynamics. Braz. J. Phys. 1999, 29, 46. [Google Scholar] [CrossRef]
- Rajagopal, A.K.; Abe, S. Implications of form invariance to the structure of nonextensive entropies. Phys. Rev. Lett. 1999, 83, 1711. [Google Scholar] [CrossRef]
- Arimoto, S. Information-theoretical considerations on estimation problems. Inf. Control. 1971, 19, 181–194. [Google Scholar] [CrossRef]
- Curado, E.M.F.; Tempesta, P.; Tsallis, C. A new entropy based on a group-theoretical structure. Ann. Phys. 2016, 366, 22–31. [Google Scholar] [CrossRef]
- Borges, E.P.; Roditi, I. A family of non-extensive entropies. Phys. Lett. A 1998, 246, 399–402. [Google Scholar] [CrossRef]
- Abe, S. A note on the q-deformation theoretic aspect of the generalized entropies in nonextensive physics. Phys. Lett. A 1997, 224, 326–330. [Google Scholar] [CrossRef]
- Kaniadakis, G. Non linear kinetics underlying generalized statistics. Phys. A Stat. Mech. Appl. 2001, 296, 405. [Google Scholar] [CrossRef]
- Kaniadakis, G. Statistical mechanics in the context of special relativity. Phys. Rev. E 2002, 66, 056125. [Google Scholar] [CrossRef] [PubMed]
- Kaniadakis, G. Statistical mechanics in the context of special relativity. II. Phys. Rev. E 2005, 72, 036108. [Google Scholar] [CrossRef]
- Kaniadakis, G.; Lissia, M.; Scarfone, A.M. Deformed logarithms and entropies. Phys. A Stat. Mech. Appl. 2004, 340, 41. [Google Scholar] [CrossRef]
- Anteneodo, C.; Plastino, A.R. Maximum entropy approach to stretched exponential probability distributions. J. Phys. A 1999, 32, 1089. [Google Scholar] [CrossRef]
- Hanel, R.; Thurner, S. A comprehensive classification of complex statistical systems and an axiomatic derivation of their entropy and distribution functions. Europhys. Lett. 2011, 93, 20006. [Google Scholar] [CrossRef]
- Hanel, R.; Thurner, S. When do generalised entropies apply? How phase space volume determines entropy. Europhys. Lett. 2011, 96, 50003. [Google Scholar] [CrossRef]
- Schwammle, V.; Tsallis, C. Two-parameter generalization of the logarithm and exponential functions and Boltzmann-Gibbs-Shannon entropy. J. Math. Phys. 2007, 48, 113301. [Google Scholar] [CrossRef]
- Tempesta, P. Beyond the Shannon-Khinchin formulation: The composability axiom and the universal-group entropy. Ann. Phys. 2016, 365, 180. [Google Scholar] [CrossRef]
- Curado, E.M.F. General aspects of the thermodynamical formalism. Braz. J. Phys. 1999, 29, 36. [Google Scholar] [CrossRef]
- Curado, E.M.F.; Nobre, F.D. On the stability of analytic entropic forms. Phys. A Stat. Mech. Appl. 2004, 335, 94. [Google Scholar] [CrossRef]
- Tsekouras, G.A.; Tsallis, C. Generalized entropy arising from a distribution of q-indices. Phys. Rev. E 2005, 71, 046144. [Google Scholar] [CrossRef]
- Pal, N.R.; Pal, S.K. Object-background segmentation using new definitions of entropy. IEE Proc. E Comput. Digit. Tech. 1989, 136, 284–295. [Google Scholar] [CrossRef]
- Callen, H.B. Thermodynamics and an Introduction to Thermostatistics, 2nd ed.; Wiley: Hoboken, NJ, USA, 1985. [Google Scholar]
- Balian, R. From Microphysics to Macrophysics; Springer: Berlin, Germany, 1991. [Google Scholar]
- Ferri, G.L.; Martinez, S.; Plastino, A. Equivalence of the four versions of Tsallis’ statistics. J. Stat. Mech. Theory Exp. 2005, 2005, P04009. [Google Scholar] [CrossRef]
- Ferri, G.L.; Martinez, S.; Plastino, A. The role of constraints in Tsallis’ nonextensive treatment revisited. Phys. A Stat. Mech. Appl. 2005, 345, 493. [Google Scholar] [CrossRef]
- Tsallis, C.; Mendes, R.S.; Plastino, A.R. The role of constraints within generalized nonextensive statistics. Phys. A Stat. Mech. Appl. 1998, 261, 534. [Google Scholar] [CrossRef]
- Tsallis, C.; Plastino, A.R.; Alvarez-Estrada, R.F. Escort mean values and the characterization of power-law-decaying probability densities. J. Math. Phys. 2009, 50, 043303. [Google Scholar] [CrossRef]
- Andrade, J.S., Jr.; da Silva, G.F.T.; Moreira, A.A.; Nobre, F.D.; Curado, E.M.F. Thermostatistics of overdamped motion of interacting particles. Phys. Rev. Lett. 2010, 105, 260601. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro, M.S.; Nobre, F.D.; Curado, E.M.F. Classes of N-Dimensional nonlinear Fokker-Planck equations associated to Tsallis entropy. Entropy 2011, 13, 1928–1944. [Google Scholar] [CrossRef]
- Ribeiro, M.S.; Nobre, F.D.; Curado, E.M.F. Time evolution of interacting vortices under overdamped motion. Phys. Rev. E 2012, 85, 021146. [Google Scholar] [CrossRef]
- Curado, E.M.F.; Souza, A.M.C.; Nobre, F.D.; Andrade, R.F.S. Carnot cycle for interacting particles in the absence of thermal noise. Phys. Rev. E 2014, 89, 022117. [Google Scholar] [CrossRef]
- Andrade, R.F.S.; Souza, A.M.C.; Curado, E.M.F.; Nobre, F.D. A thermodynamical formalism describing mechanical interactions. EPL 2014, 108, 20001. [Google Scholar] [CrossRef]
- Nobre, F.D.; Curado, E.M.F.; Souza, A.M.C.; Andrade, R.F.S. Consistent thermodynamic framework for interacting particles by neglecting thermal noise. Phys. Rev. E 2015, 91, 022135. [Google Scholar] [CrossRef]
- Ribeiro, M.S.; Casas, G.A.; Nobre, F.D. Second law and entropy production in a nonextensive system. Phys. Rev. E 2015, 91, 012140. [Google Scholar] [CrossRef]
- Vieira, C.M.; Carmona, H.A.; Andrade, J.S., Jr.; Moreira, A.A. General continuum approach for dissipative systems of repulsive particles. Phys. Rev. E 2016, 93, 060103(R). [Google Scholar] [CrossRef]
- Ribeiro, M.S.; Nobre, F.D. Repulsive particles under a general external potential: Thermodynamics by neglecting thermal noise. Phys. Rev. E 2016, 94, 022120. [Google Scholar] [CrossRef]
- Tsallis, C.; Haubold, H.J. Boltzmann-Gibbs entropy is sufficient but not necessary for the likelihood factorization required by Einstein. EPL 2015, 110, 30005. [Google Scholar] [CrossRef]
- Latora, V.; Baranger, M.; Rapisarda, A.; Tsallis, C. The rate of entropy increase at the edge of chaos. Phys. Lett. A 2000, 273, 97. [Google Scholar] [CrossRef]
- Baldovin, F.; Robledo, A. Nonextensive Pesin identity - Exact renormalization group analytical results for the dynamics at the edge of chaos of the logistic map. Phys. Rev. E 2004, 69, 045202(R). [Google Scholar] [CrossRef]
- Tirnakli, U.; Tsallis, C.; Beck, C. A closer look at time averages of the logistic map at the edge of chaos. Phys. Rev. E 2009, 79, 056209. [Google Scholar] [CrossRef]
- Tirnakli, U.; Tsallis, C. Extensive numerical results for integrable case of standard map. Nonlinear Phenom. Complex Syst. 2020, 23, 149–152. [Google Scholar] [CrossRef]
- Bountis, A.; Veerman, J.J.P.; Vivaldi, F. Cauchy distributions for the integrable standard map. Phys. Lett. A 2020, 384, 126659. [Google Scholar] [CrossRef]
- Tirnakli, U.; Borges, E.P. The standard map: From Boltzmann-Gibbs statistics to Tsallis statistics. Nat. Sci. Rep. 2016, 6, 23644. [Google Scholar] [CrossRef] [PubMed]
- Caruso, F.; Tsallis, C. Nonadditive entropy reconciles the area law in quantum systems with classical thermodynamics. Phys. Rev. E 2008, 78, 021102. [Google Scholar] [CrossRef] [PubMed]
- Cirto, L.J.L.; Assis, V.R.V.; Tsallis, C. Influence of the interaction range on the thermostatistics of a classical many-body system. Physica A 2014, 393, 286–296. [Google Scholar] [CrossRef]
- Cirto, L.J.L.; Rodriguez, A.; Nobre, F.D.; Tsallis, C. Validity and failure of the Boltzmann weight. EPL 2018, 123, 30003. [Google Scholar] [CrossRef]
- Moreira, A.A.; Vieira, C.M.; Carmona, H.A.; Andrade, J.S., Jr.; Tsallis, C. Overdamped dynamics of particles with repulsive power-law interactions. Phys. Rev. E 2018, 98, 032138. [Google Scholar] [CrossRef]
- Lutz, E. Anomalous diffusion and Tsallis statistics in an optical lattice. Phys. Rev. A 2003, 67, 051402(R). [Google Scholar] [CrossRef]
- Douglas, P.; Bergamini, S.; Renzoni, F. Tunable Tsallis distributions in dissipative optical lattices. Phys. Rev. Lett. 2006, 96, 110601. [Google Scholar] [CrossRef]
- Lutz, E.; Renzoni, F. Beyond Boltzmann-Gibbs statistical mechanics in optical lattices. Nat. Phys. 2013, 9, 615–619. [Google Scholar] [CrossRef]
- Tsallis, C.; Bukman, D.J. Anomalous diffusion in the presence of external forces: Exact time-dependent solutions and their thermostatistical basis. Phys. Rev. E 1996, 54, R2197. [Google Scholar] [CrossRef]
- Plastino, A.R.; Plastino, A. Non-extensive statistical mechanics and generalized Fokker-Planck equation. Physica A 1995, 222, 347. [Google Scholar] [CrossRef]
- Combe, G.; Richefeu, V.; Stasiak, M.; Atman, A.P.F. Experimental validation of nonextensive scaling law in confined granular media. Phys. Rev. Lett. 2015, 115, 238301. [Google Scholar] [CrossRef]
- Wong, C.Y.; Wilk, G.; Cirto, L.J.L.; Tsallis, C. From QCD-based hard-scattering to nonextensive statistical mechanical descriptions of transverse momentum spectra in high-energy pp and pp collisions. Phys. Rev. D 2015, 91, 114027. [Google Scholar] [CrossRef]
- Yalcin, G.C.; Beck, C. Generalized statistical mechanics of cosmic rays: Application to positron-electron spectral indices. Sci. Rep. 2018, 8, 1764. [Google Scholar] [CrossRef]
- Nivanen, L.; Mehaute, A.L.; Wang, Q.A. Generalized algebra within a nonextensive statistics. Rep. Math. Phys. 2003, 52, 437. [Google Scholar] [CrossRef]
- Borges, E.P. A possible deformed algebra and calculus inspired in nonextensive thermostatistics. Phys. A Stat. Mech. Appl. 2004, 340, 95–101, Corrigenda in Phys. A Stat. Mech. Appl. 2021, 581, 126206. [Google Scholar] [CrossRef]
- Umarov, S.; Tsallis, C.; Steinberg, S. On a q-central limit theorem consistent with nonextensive statistical mechanics. Milan J. Math. 2008, 76, 307–328. [Google Scholar] [CrossRef]
- Umarov, S.; Tsallis, C.; Gell-Mann, M.; Steinberg, S. Generalization of symmetric α-stable Lévy distributions for q > 1. J. Math. Phys. 2010, 51, 033502. [Google Scholar] [CrossRef]
- Umarov, S.; Tsallis, C. Mathematical Foundations of Nonextensive Statistical Mechanics; World Scientific: Singapore, 2022; in press. [Google Scholar]
- Tsallis, C.; Gell-Mann, M.; Sato, Y. Asymptotically scale-invariant occupancy of phase space makes the entropy Sq extensive. Proc. Natl. Acad. Sci. USA 2005, 102, 15377. [Google Scholar] [CrossRef]
- Gazeau, J.-P.; Tsallis, C. Moebius transforms, cycles and q-triplets in statistical mechanics. Entropy 2019, 21, 1155. [Google Scholar] [CrossRef]
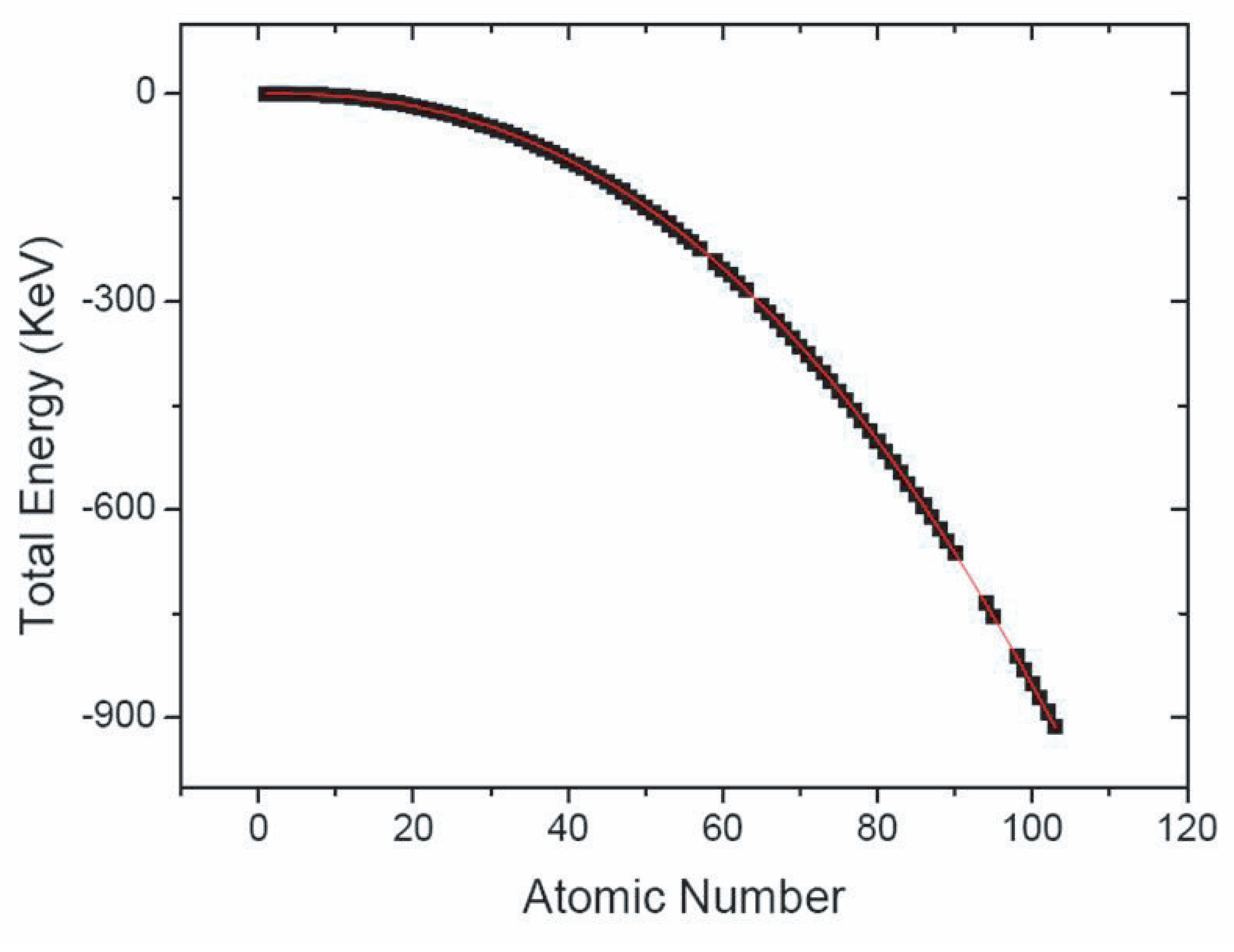
- Amador, C.H.S.; Zambrano, L.S. Evidence for energy regularity in the Mendeleev periodic table. Phys. A Stat. Mech. Appl. 2010, 389, 3866–3869. [Google Scholar] [CrossRef]
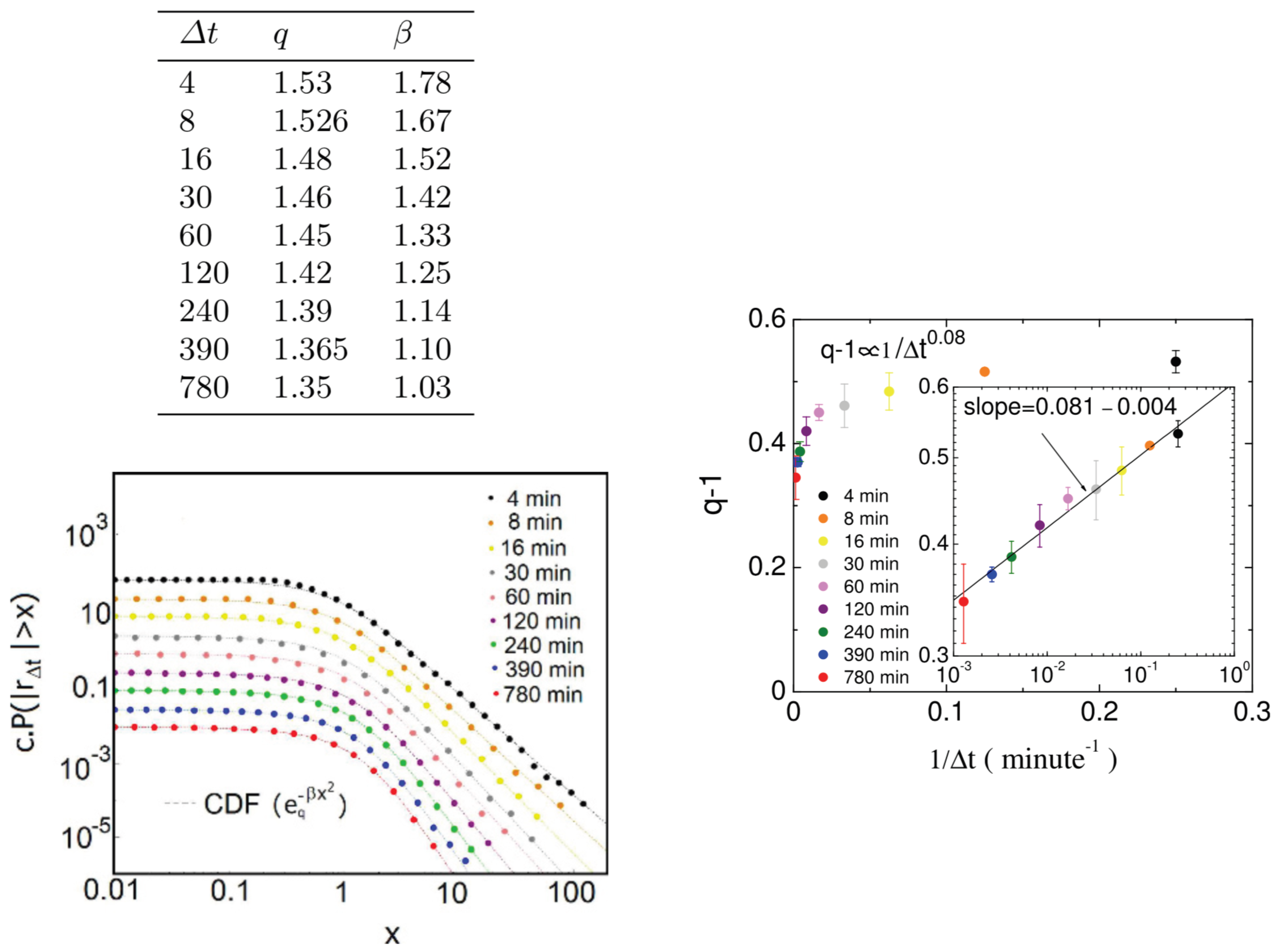
- Ludescher, J.; Tsallis, C.; Bunde, A. Universal behaviour of interoccurrence times between losses in financial markets: An analytical description. Europhys. Lett. 2011, 95, 68002. [Google Scholar] [CrossRef]
- Ludescher, J.; Bunde, A. Universal behavior of the interoccurrence times between losses in financial markets: Independence of the time resolution. Phys. Rev. 2014, 90, 062809. [Google Scholar] [CrossRef]
- Tsallis, C. Economics and finance: q-statistical features galore. Entropy 2017, 19, 457. [Google Scholar] [CrossRef]
- Ruiz, G.; de Marcos, A.F. Evidence for criticality in financial data. Eur. Phys. J. B 2018, 91, 1. [Google Scholar] [CrossRef]
- Bogachev, M.I.; Kayumov, A.R.; Bunde, A. Universal internucleotide statistics in full genomes: A footprint of the DNA structure and packaging? PLoS ONE 2014, 9, e112534. [Google Scholar] [CrossRef]
- Tsallis, C.; Stariolo, D.A. Generalized simulated annealing. Phys. A Stat. Mech. Appl. 1996, 233, 395, First appeared in C. Tsallis and D.A. Stariolo, Generalized simulated annealing. Notas de Fisica/CBPF 026 (June 1994). [Google Scholar] [CrossRef]
- Price, D.J.D.S. Networks of scientific papers. Science 1965, 149, 510–515. [Google Scholar] [CrossRef]
- Watts, D.J.; Strogatz, S.H. Collective dynamics of “small-world” networks. Nature 1998, 393, 440–442. [Google Scholar] [CrossRef] [PubMed]
- Barabasi, A.L.; Albert, R. Emergence of scaling in random networks. Science 1999, 286, 509–512. [Google Scholar] [CrossRef] [PubMed]
- Newman, M.E.J. The structure of scientific collaboration networks. Proc. Natl. Acad. Sci. USA 2001, 98, 404–409. [Google Scholar] [CrossRef] [PubMed]
- Soares, D.J.; Tsallis, C.; Mariz, A.M.; da Silva, L.R. Preferential attachment growth model and nonextensive statistical mechanics. EPL 2005, 70, 70. [Google Scholar] [CrossRef]
- Thurner, S.; Tsallis, C. Nonextensive aspects of self-organized scale-free gas-like networks. EPL 2005, 72, 197. [Google Scholar] [CrossRef]
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
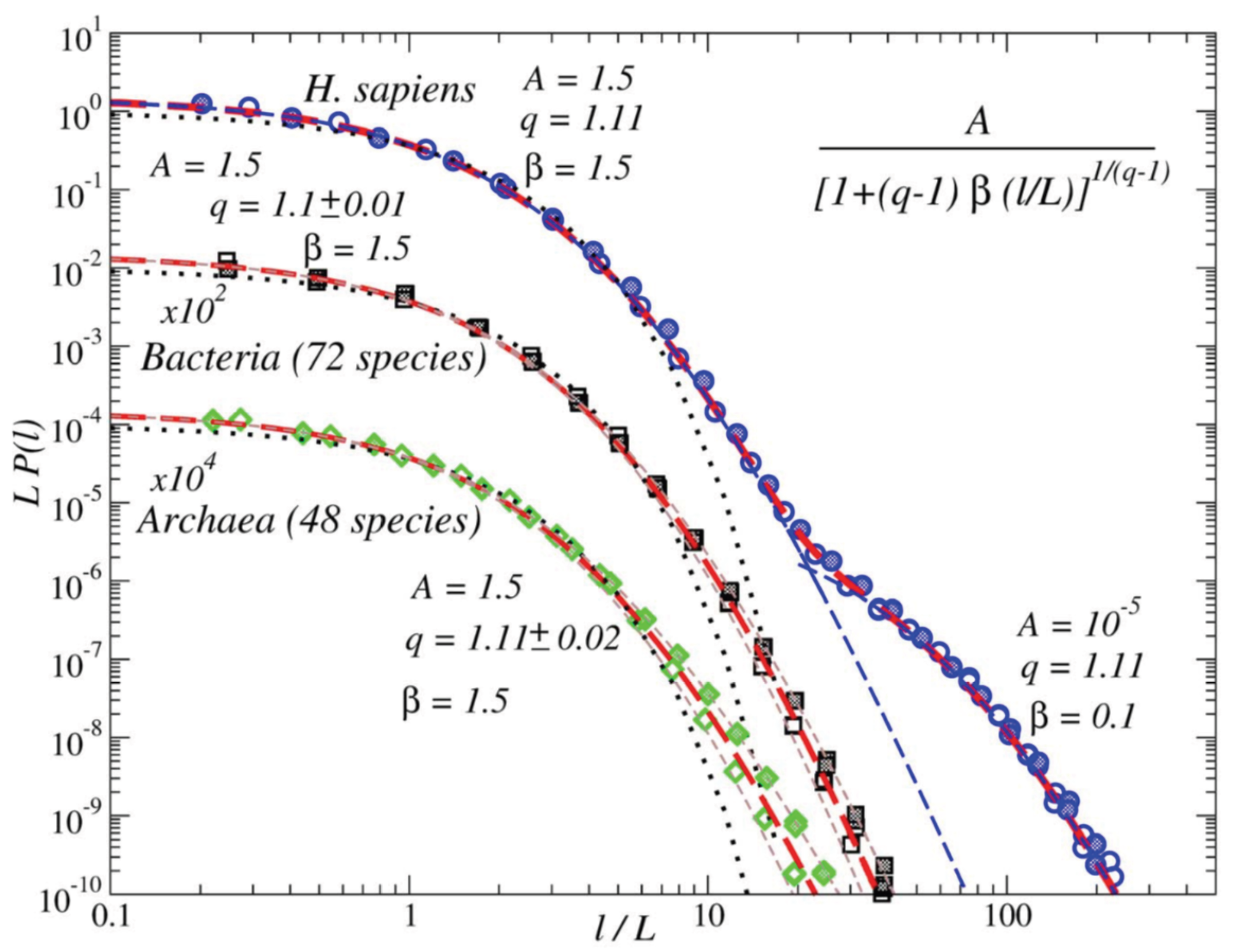
- Brito, S.; da Silva, L.R.; Tsallis, C. Role of dimensionality in complex networks. Sci. Rep. 2016, 6, 27992. [Google Scholar] [CrossRef]
- Cinardi, N.; Rapisarda, A.; Tsallis, C. A generalised model for asymptotically-scale-free geographical networks. J. Stat. Mech. Theory Exp. 2020, 2020, 043404. [Google Scholar] [CrossRef]
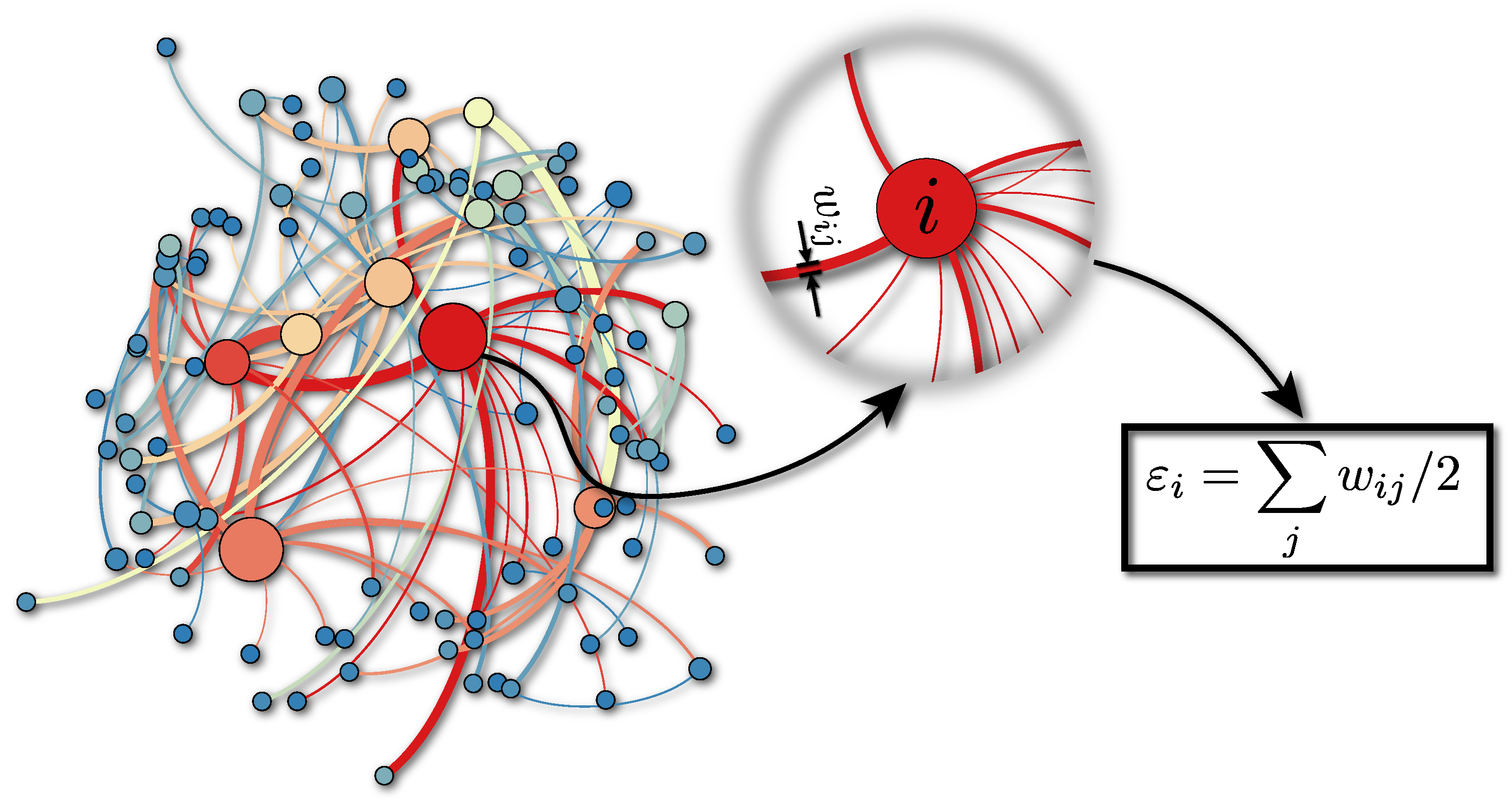
- de Oliveira, R.M.; Brito, S.; da Silva, L.R.; Tsallis, C. Connecting complex networks to nonadditive entropies. Sci. Rep. 2021, 11, 1130. [Google Scholar] [CrossRef]
- Mohanalin, J.; Beenamol, B.; Kalra, P.K.; Kumar, N. A novel automatic microcalcification detection technique using Tsallis entropy and a type II fuzzy index. Comput. Math. Appl. 2010, 60, 2426–2432. [Google Scholar] [CrossRef]
- Al-Azawi, R.J.; Al-Saidi, N.M.G.; Jalab, H.A.; Kahtan, H.; Ibrahim, R.W. Efficient classification of COVID-19 CT scans by using q-transform model for feature extraction. PeerJ. Comput. Sci. 2021, 7, e553. [Google Scholar] [CrossRef]
- Greco, A.; Tsallis, C.; Rapisarda, A.; Pluchino, A.; Fichera, G.; Contrafatto, L. Acoustic emissions in compression of building materials: Q-statistics enables the anticipation of the breakdown point. Eur. Phys. J. Spec. Top. 2020, 229, 841–849. [Google Scholar] [CrossRef]
- Stavrakas, I.; Triantis, D.; Kourkoulis, S.K.; Pasiou, E.D.; Dakanali, I. Acoustic emission analysis of cement mortar specimens during three point bending tests. Lat. Am. J. Solids Struct. 2016, 13, 2283–2297. [Google Scholar] [CrossRef]


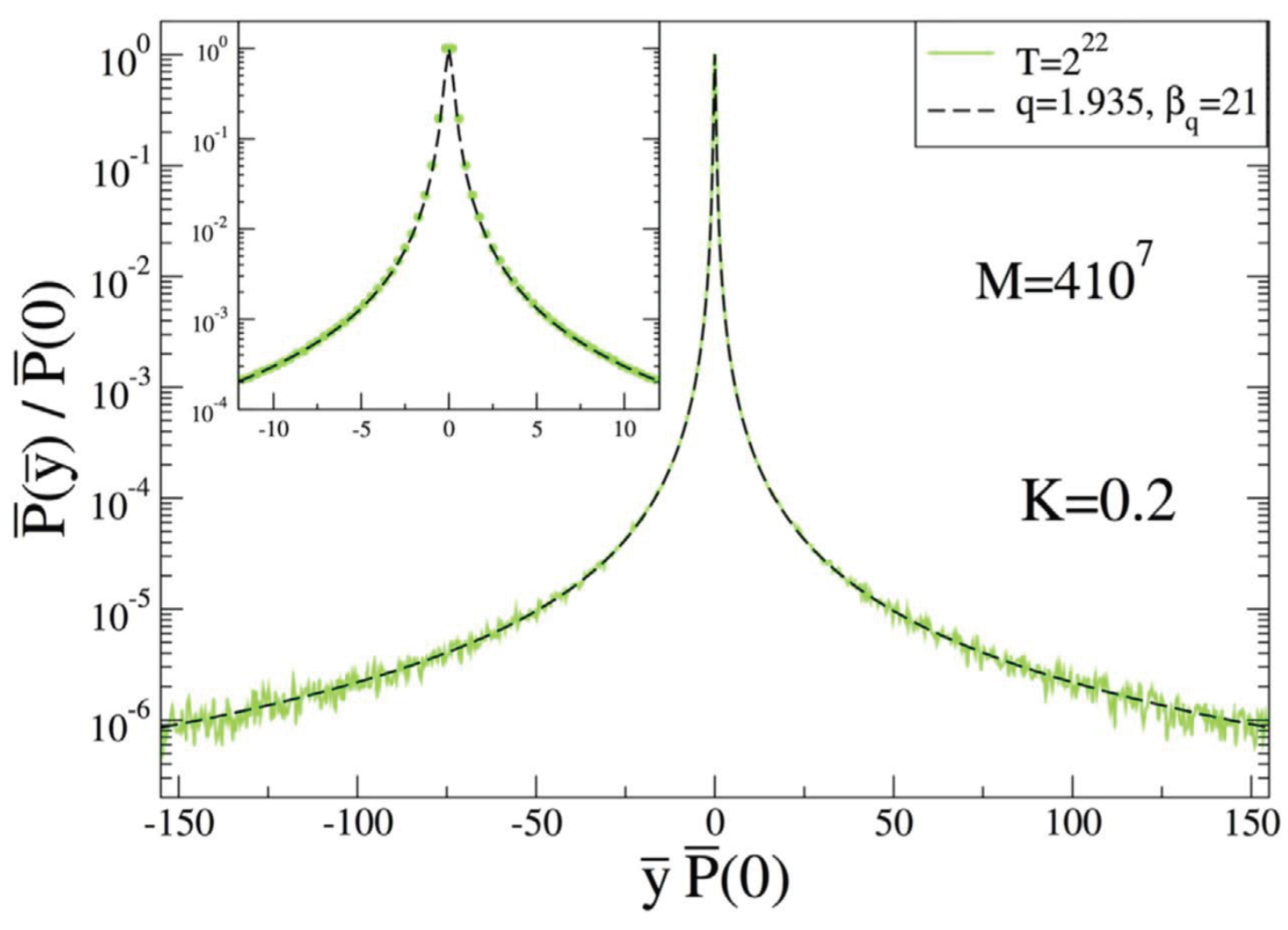
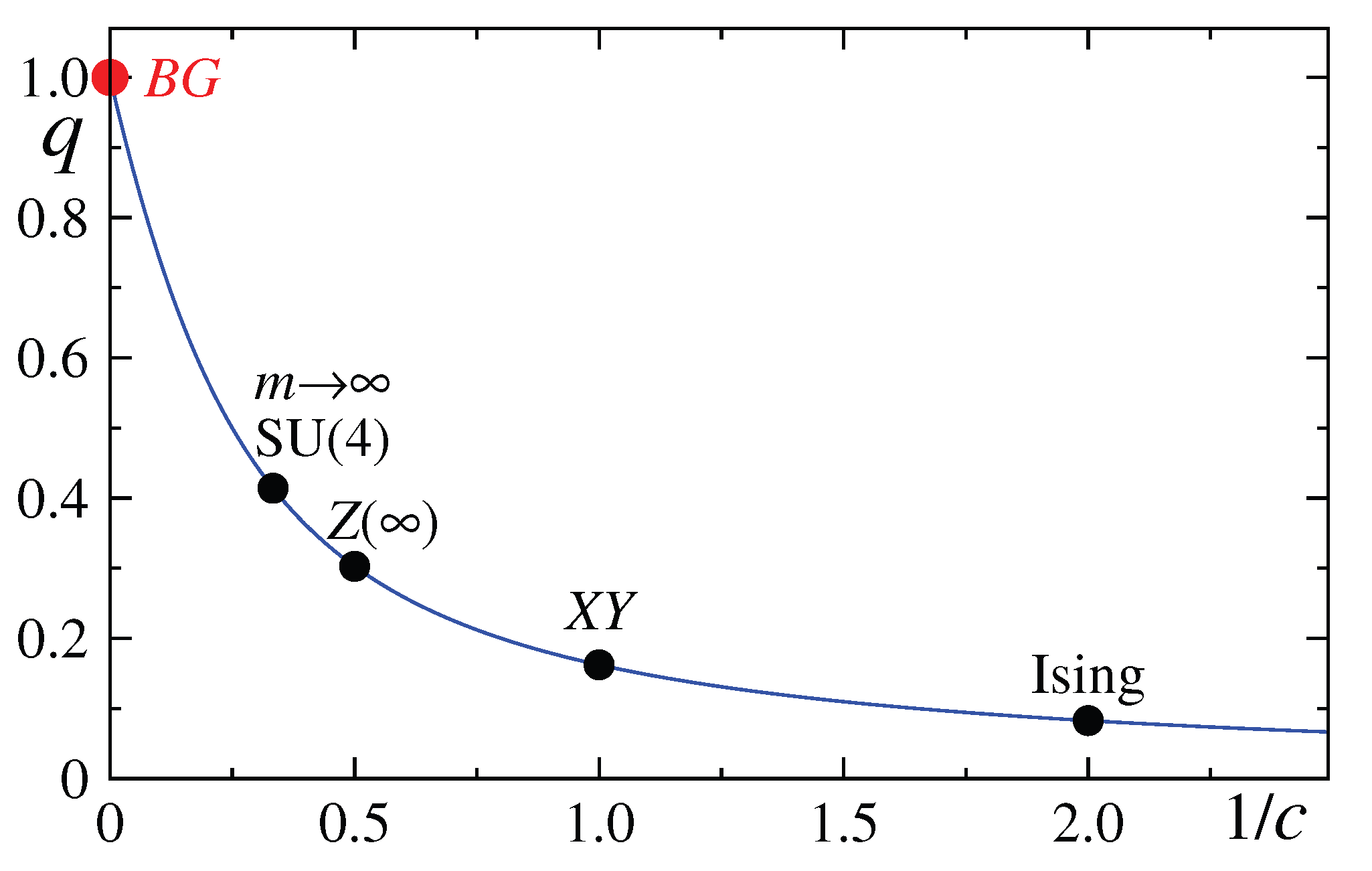

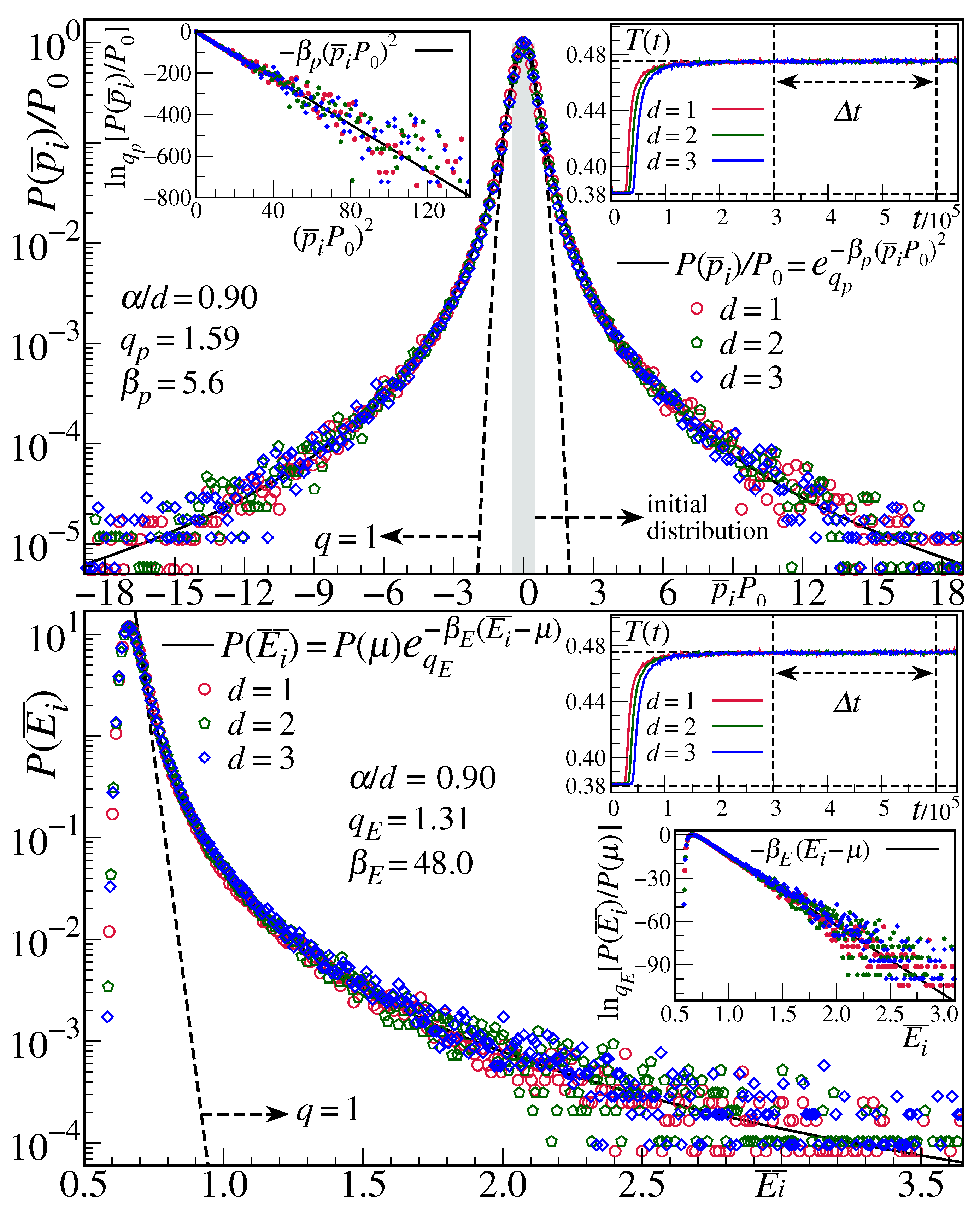
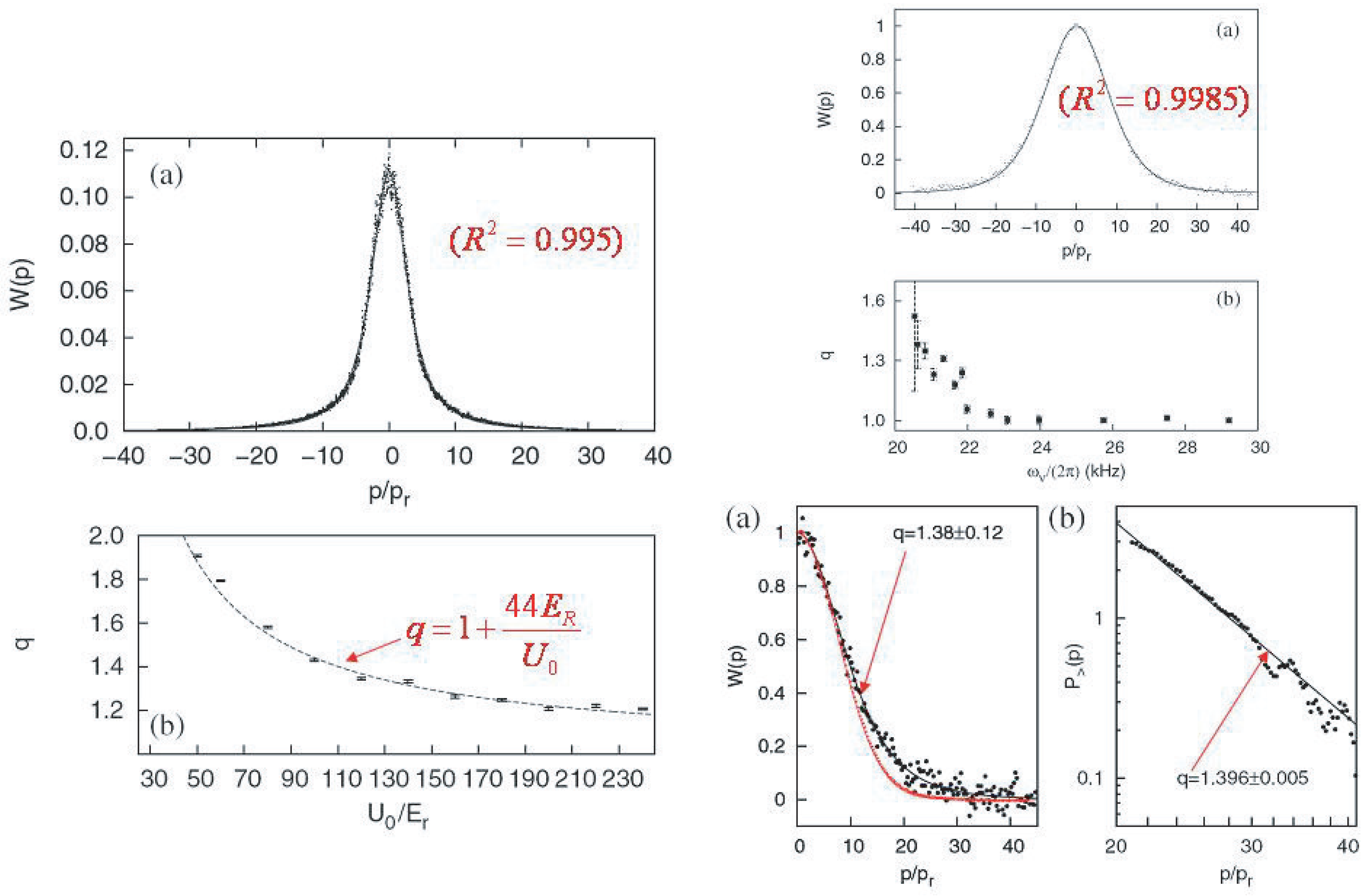

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tsallis, C. Entropy. Encyclopedia 2022, 2, 264-300. https://doi.org/10.3390/encyclopedia2010018
Tsallis C. Entropy. Encyclopedia. 2022; 2(1):264-300. https://doi.org/10.3390/encyclopedia2010018
Chicago/Turabian StyleTsallis, Constantino. 2022. "Entropy" Encyclopedia 2, no. 1: 264-300. https://doi.org/10.3390/encyclopedia2010018
APA StyleTsallis, C. (2022). Entropy. Encyclopedia, 2(1), 264-300. https://doi.org/10.3390/encyclopedia2010018





