Analyzing Power Law Extensions of Newtonian Gravity Using Differential Force Measurements

Abstract

The Standard Model is not a complete description of reality; it omits the existence of dark matter, dark energy, and an explanation as to why no CP violation has been observed. However, some of these phenomena could be explained through a new force mediated by a new boson. If such a boson were massless it would result in a power law potential and if massive the interaction would be Yukawa-like. A previous experiment employing the interactions of a micromechanical oscillator attached to spherical test mass was successful in placing the best limits on a mass–mass Yukawa-like interaction, but the data were never analyzed in the context of a power law. Here, those data are analyzed considering a power law for powers n = 1–5 where n is the number of boson exchanges. The results show that the limits obtained through power law analysis of these data are not better than the currently accepted limits. A discussion of an experiment design capable of producing better limits on power law extensions to the Standard Model is presented, and suggests that a micromechanical-oscillator-based experiment remains capable of improving the limits by at least one order of magnitude.

1. Introduction

The success of the Standard Model (SM) in describing matter and interactions cannot be overstated, but it is not a complete description: it does not explain dark matter and dark energy [1], it predicts charge-parity (CP) violations in the strong force, which have not yet been observed [1,2,3], and there is no quantized description of gravity [1]. This incompleteness has lead to many theories to fill the gaps in the SM.

One such approach is hypothesizing an interaction mediated by an as-yet undiscovered boson [4,5]. If the hypothetical boson is massive it leads to Yukawa-like interactions [6], but if it is massless, the interaction will be parameterized with a power law [7]. This power law is typically written as a correction to Newtonian gravity and for two point masses is expressed as

$$U=\frac{-G{m}_1{m}_2}{r}\left(1+{\Lambda }_n{\left(\frac{r_0}{r}\right)}^{n-1}\right)$$

(1)

where G is Newton’s gravitational constant, $m_1$ and $m_2$ are point masses separated by a distance r, ${\Lambda }_n$ is the strength of the correction relative to gravity for a particular power of n, and $r_0$ is a constant used to preserve the dimension of the interaction; in this work $r_0=1\times {10}^{-15}$ m. The value of $r_0$ was chosen to be the same as in Ref. [8] so that the ${\Lambda }_n$ reported in this work can be directly compared to the ${\Lambda }_n$ found in Ref. [8].

Previous experiments to probe both power law and Yukawa-like deviations in Newtonian gravity were reported in Refs. [8,9,10]. One experiment to probe hypothetical Yukawa-like interactions was carried out in 2016 [11]. The Yukawa-like interaction of the form

$$U=\frac{-G{m}_1{m}_2}{r}\left(1+\alpha e^{r/\lambda }\right)$$

(2)

was probed and the experiment placed the best limits on $\alpha$ for a range of $\lambda \in (30,8000)$ nm, where $\alpha$ is the strength of the correction and $\lambda$ is the Compton wavelength of a massive hypothetical boson [11]. The experiment consisted of a spherical test mass attached to a micromechanical oscillator that was brought within 200 nm of a source mass made of alternating Au and Si sections, and the force was measured between the two masses. The set-up was not designed to probe power law extensions of the SM and was expected to not be sensitive enough to improve those limits. However, a reanalysis of the force measurements from [11] and a full analysis with the data was never carried out in the context of a power law.

This work carries out a full analysis considering power law extensions of the SM on the force measurements in [11] as well as on new data. The limits obtained are not an improvement over the current best limits [8,10], so we discuss what would need to be done for the approach in [11] to improve limits on power law extensions to the SM.

2. Materials and Methods

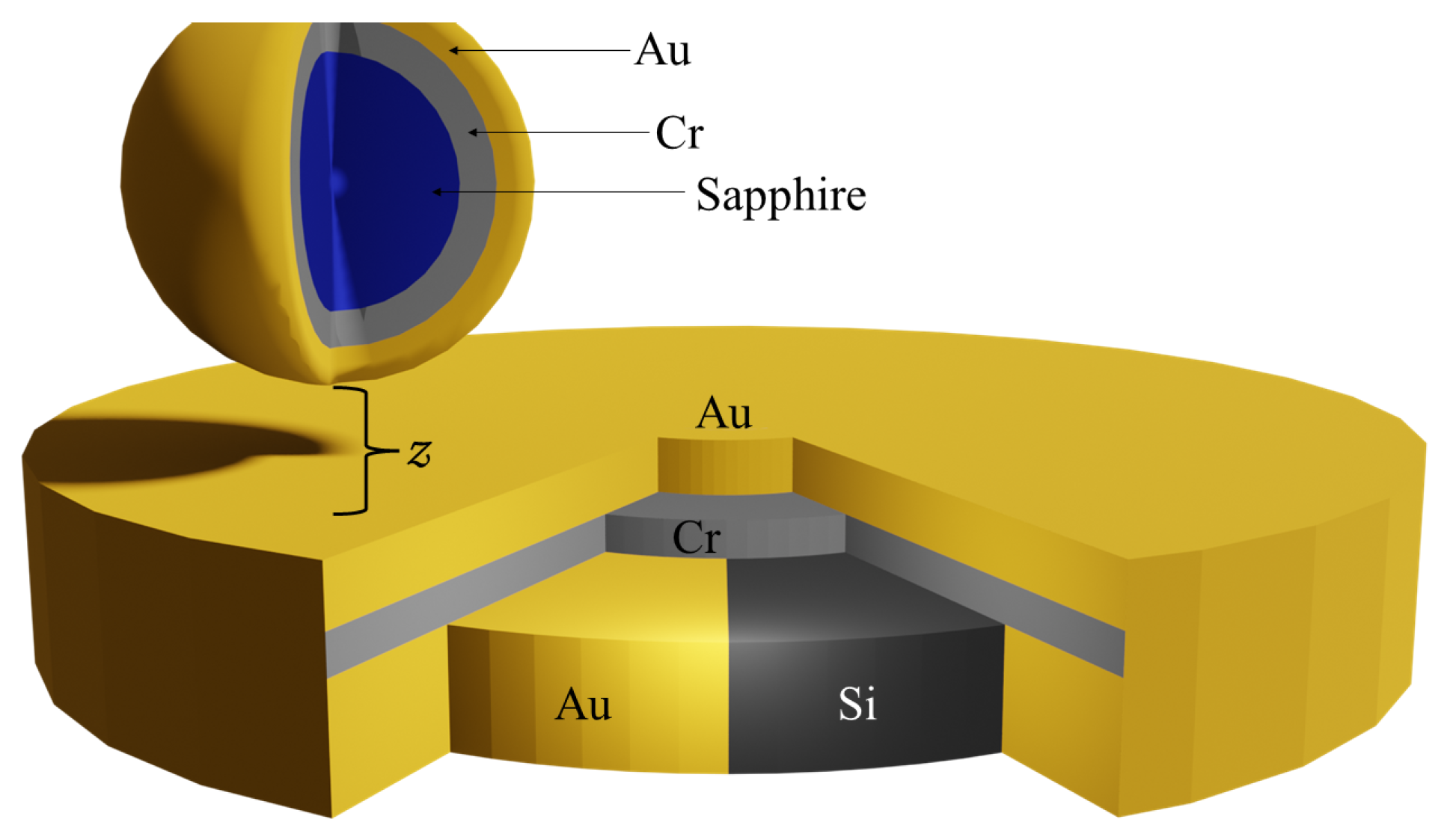
Ref. [11] used a differential force measurement technique between a spherical test mass attached to a micromechanical oscillator and a source mass. The test mass was a sphere composed of 3 layers; a central sapphire core with a 149.3 ± 0.2 $\mathsf{\mu }$m radius covered by 10 nm of Cr followed by 250 nm of Au (see Figure 1 for a cross-sectional diagram of the test and source mass). The sphere was glued to a 500 × 500 $\mathsf{\mu }$m micromechanical oscillator and the system had a quality factor Q ∼ 7200. The deflection of the test mass was measured through a change in capacitance between the oscillator’s plate and electrodes located below the plate.

The source mass was a layered structure consisting of BK7 Schott glass followed by a 2.10 ± 0.02 $\mathsf{\mu }$m layer of alternating sections of Au and Si. The Au and Si sectors shared a common layer of a 10 ± 1 nm Cr wetting layer, on top of which was a 150 nm Au layer covering the sample. The shared top Au layer thickness was chosen to be larger than the effective penetration depth of the Casimir force. In this way, the contribution due to the Casimir force is the same whether the test mass is located over a Si sector or an Au sector and leads to a Casimir-less measuring technique as described in [12].

The test mass was brought to within 200 nm of the source mass’s surface, and at this separation the minimum detectable force is 12 fN/$\sqrt{\mathrm{Hz}}$. The closest the masses could be brought is ∼200 nm without the Casimir force making the sphere jump into contact and ruining the whole experiment. The sensitivity also decreases as the separation increases.

The source mass was rotated so that the sections alternated under the test mass at the oscillator’s resonant frequency. Doing so makes the experiment select the first harmonic of the force commensurate with the period of the samples.

While the source masses used to set limits on the Yukawa-like interaction [11] had upwards of 300 Au and Si sections, there were source masses that had only two, 1 mm wide, sections of alternating gold and silicon, as depicted in Figure 2. One sample had an inner radius of 2.5 mm and the other had an inner radius of 5 mm. The layered structure is the same as the 300 section samples, except the wetting layer is Ti. The data taken with these larger source masses were not previously analyzed.

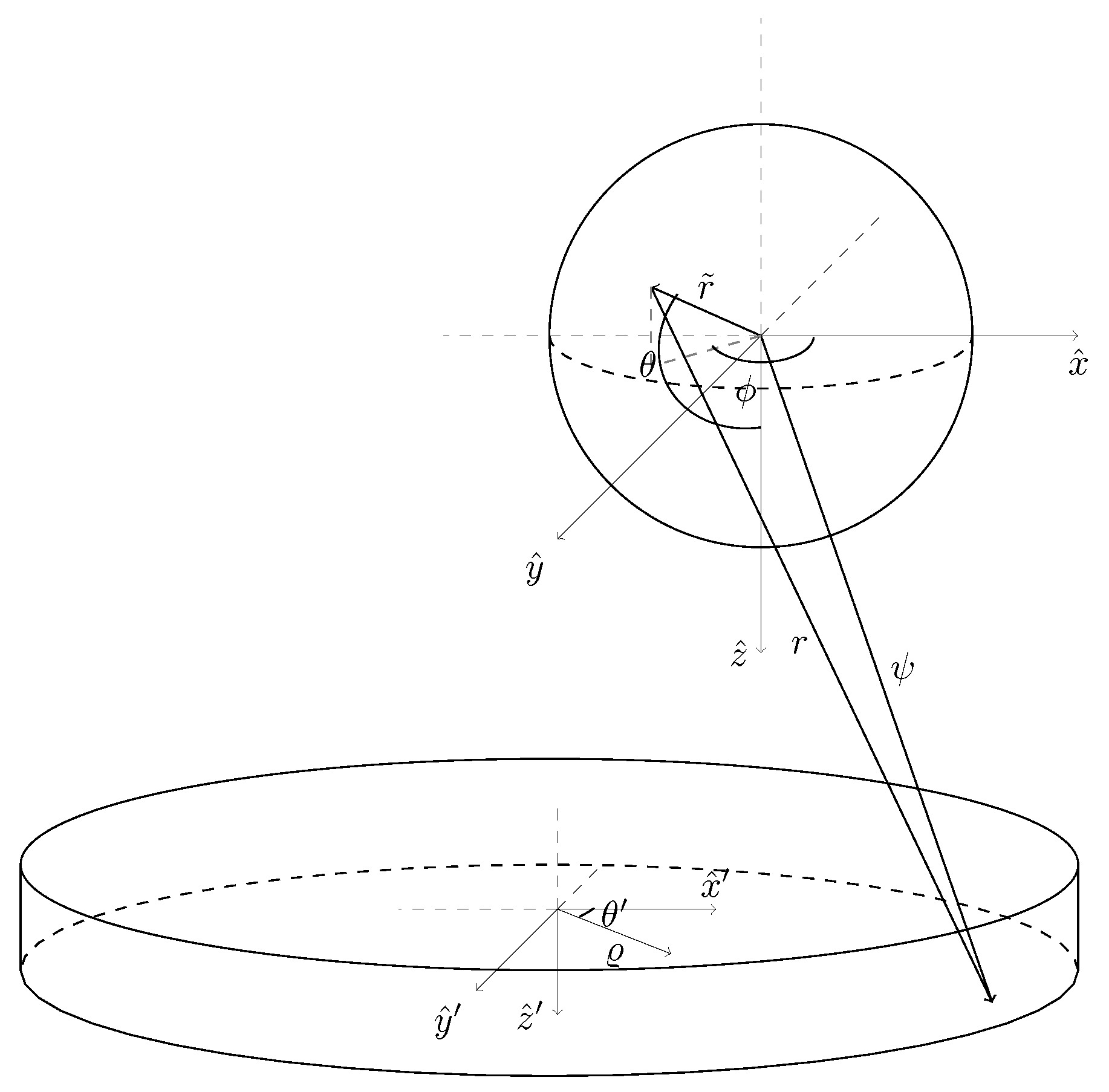
To extract limits on ${\Lambda }_n$, the force due to the potential expressed in Equation (1) was calculated by integrating over the experimental geometry. First, the interaction between a spherical test mass and an arbitrary point in the source mass was calculated analytically with a coordinate system centered at the sphere, shown as the unprimed coordinate system in Figure 3, using spherical coordinates ($\tilde{r},\theta ,\varphi$), where $\theta$ and $\varphi$ are the polar and azimuthal angle, respectively. The potential energy between the sphere and the source mass is

$$\begin{array}{cc}\hfill U& =-2\pi G{\rho }_1{\rho }_2{\Lambda }_n{r}_0^{n-1}\int \int \int \left(\frac{1}{\psi (2-n)}\left[\frac{R{(\psi -R)}^{3-n}}{3-n}\right.\right.\hfill \\ & +\left.\left.\frac{{(\psi -R)}^{4-n}}{(4-n)(3-n)}+\frac{R{(\psi +R)}^{3-n}}{3-n}-\frac{{(\psi +R)}^{4-n}}{(4-n)(3-n)}\right]\right)d{V}_{sm}\hfill \end{array}$$

(3)

where R is the radius of the sphere, ${\rho }_1$ and ${\rho }_2$ are the densities of the sphere and the point, respectively, $\psi$ is the distance from the center of the sphere to the arbitrary point in the source mass, and $d{V}_{sm}$ is the differential volume of the source mass; see Appendix A for details. It was verified that Equation (3) does not diverge for $n=$ 2, 3, and 4 by taking the limit of Equation (3) as $n\to$ 2, 3, and 4, respectively. These limits can be seen in Appendix A. The integrals over the source mass were carried out using cylindrical coordinates ($\varrho$, ${\theta }^{\prime }$, $z^{\prime }$) in the primed coordinate system, see Figure 3, centered in the middle of the source mass. In the primed coordinate system

$$\psi =\sqrt{{\varrho }^2-2\varrho {\varrho }_{s}cos({\theta }^{\prime }-{\theta }_s)+{\varrho }_s^2+{(z^{\prime }-z_s)}^2}$$

(4)

where $\varrho$ is the radial variable integrated between the inner and outer radius of the sample, ${\theta }^{\prime }$ is the angular extent of the sample, ${\varrho }_s$ is the radial distance to the sphere, ${\theta }_s$ is the angular position of the sphere, $z_s$ is the vertical position of the center of the sphere, and $z^{\prime }$ is the vertical coordinate integrated over the thickness of the sample.

The experiment is only sensitive to forces in the vertical direction. Normally, calculating the force in the z direction would be carried out by

$$F_z=-\frac{\partial }{\partial z^{\prime }}U$$

(5)

where $d{F}_z$ is the differential force in the vertical direction that needs to be integrated over the geometry of the source mass. However, since Equation (5) needs to be integrated along $z^{\prime }$, calculating the derivative explicitly can be avoided because the operations are the inverse of each other, meaning that the integral of Equation (5) over the source mass in z is simply the difference of Equation (3) evaluated at the $z^{\prime }$ integration limits of the source mass, $z_1$ and $z_2$.

$$F_z^{\left(n\right)}=\int \int (U^{\left(n\right)}\left(z_1\right)-U^{\left(n\right)}\left(z_2\right))d{A}_{sm}$$

(6)

where $d{A}_{sm}$ is the area element of the source mass that remains to be integrated. A polar coordinate system centered at the center of the sample, the primed coordinate system in Figure 3, is used for the last two integrals. The area element is expressed as $d{A}_{sm}=\varrho d\varrho d{\theta }^{\prime }$, where ($\varrho$, ${\theta }^{\prime }$) are the polar coordinates in the primed system. The integrals over $\varrho$ and ${\theta }^{\prime }$ were done numerically with Python code using SciPy version 1.11.4 packages [13].

In order to obtain the correct interaction from the layered geometry, the numerical integrations were carried out three times, once for each of the three different layers of the test mass. Each test mass layer was considered to be a solid sphere with corresponding radii of $R_{sap}=$ 149.30 $\mathsf{\mu }$m, $R_{Cr}=$ 149.31 $\mathsf{\mu }$m, and $R_{Au}=$ 149.56 $\mathsf{\mu }$m for sapphire, Cr, and Au, respectively. As an example, for n = 3 the integrations for each layer are

$$\begin{array}{c}\hfill F_{Au}^{\left(3\right)}=2\pi G{r}_0^2\int \int \left(\frac{R_{Au}(\psi \left(z_2\right)-\psi \left(z_1\right))}{\psi \left(z_1\right)\psi \left(z_2\right)}+ln\left(\frac{(\psi \left(z_1\right)-R_{Au})(\psi \left(z_2\right)+R_{Au})}{(\psi \left(z_1\right)+R_{Au})(\psi \left(z_2\right)-R_{Au})}\right)\right)d{A}_{sm}\end{array}$$

(7)

$$\begin{array}{c}\hfill F_{Cr}^{\left(3\right)}=-2\pi G{r}_0^2\int \int \left(\frac{R_{Cr}(\psi \left(z_2\right)-\psi \left(z_1\right))}{\psi \left(z_1\right)\psi \left(z_2\right)}+ln\left(\frac{(\psi \left(z_1\right)-R_{Cr})(\psi \left(z_2\right)+R_{Cr})}{(\psi \left(z_1\right)+R_{Cr})(\psi \left(z_2\right)-R_{Cr})}\right)\right)d{A}_{sm}\end{array}$$

(8)

$$\begin{array}{c}\hfill F_{Sap}^{\left(3\right)}=-2\pi G{r}_0^2\int \int \left(\frac{R_{Sap}(\psi \left(z_2\right)-\psi \left(z_1\right))}{\psi \left(z_1\right)\psi \left(z_2\right)}+ln\left(\frac{(\psi \left(z_1\right)-R_{Sap})(\psi \left(z_2\right)+R_{Sap})}{(\psi \left(z_1\right)+R_{Sap})(\psi \left(z_2\right)-R_{Sap})}\right)\right)d{A}_{sm}\end{array}$$

(9)

Equations (7)–(9) are the integrals over the source mass geometry carried out after the limit of Equation (3) was taken as $n\to$ 3, where $\psi \left(z_1\right)$ and $\psi \left(z_2\right)$ are Equation (4) evaluated at $z_1$ and $z_2$, respectively. The integrals for the other powers were carried out in the same manner. Once the integrals for each test mass layer are carried out, the total force due to the layered structure of the source mass and test mass (Figure 1) is

$$\begin{array}{cc}\hfill F_z^{\left(3\right)}={\Lambda }_3({\rho }_{Au}-& {\rho }_{Si})(({\rho }_{Sap}-{\rho }_{Cr})F_{Sap}^{\left(3\right)}+({\rho }_{Cr}-{\rho }_{Au})F_{Cr}^{\left(3\right)}+{\rho }_{Au}{F}_{Au}^{\left(3\right)})\hfill \end{array}$$

(10)

where ${\rho }_{Au}$, ${\rho }_{Cr}$, ${\rho }_{sap}$, and ${\rho }_{Si}$ are the densities of Au, Cr, sapphire, and Si, respectively, $F_z^{\left(3\right)}$ indicates the total force in the z-direction for the power $n=3$, and the factor out front, $({\rho }_{Au}-{\rho }_{Si})$, accounts for the difference when the sphere is over an Au or Si sector in the source mass. Furthermore, since the first two layers are a shared layer of Au and Cr, the contributions of these layers to the interaction are subtracted out.

The total force was calculated with the test mass at different angular positions (${\theta }_s^{\prime }$), Figure 4. The amplitude of the first harmonic for a particular power, $A_1^{\left(n\right)}$, of the force commensurate with the period, $\Theta$, of the sectors was equated to the error bars of the force measurements,

$$A_1^{\left(n\right)}=\frac{2}{\Theta }{\int }_0^{\Theta }{F}_z^{\left(n\right)}cos\left(\frac{2\pi {\theta }_s}{\Theta }\right)d{\theta }_s.$$

(11)

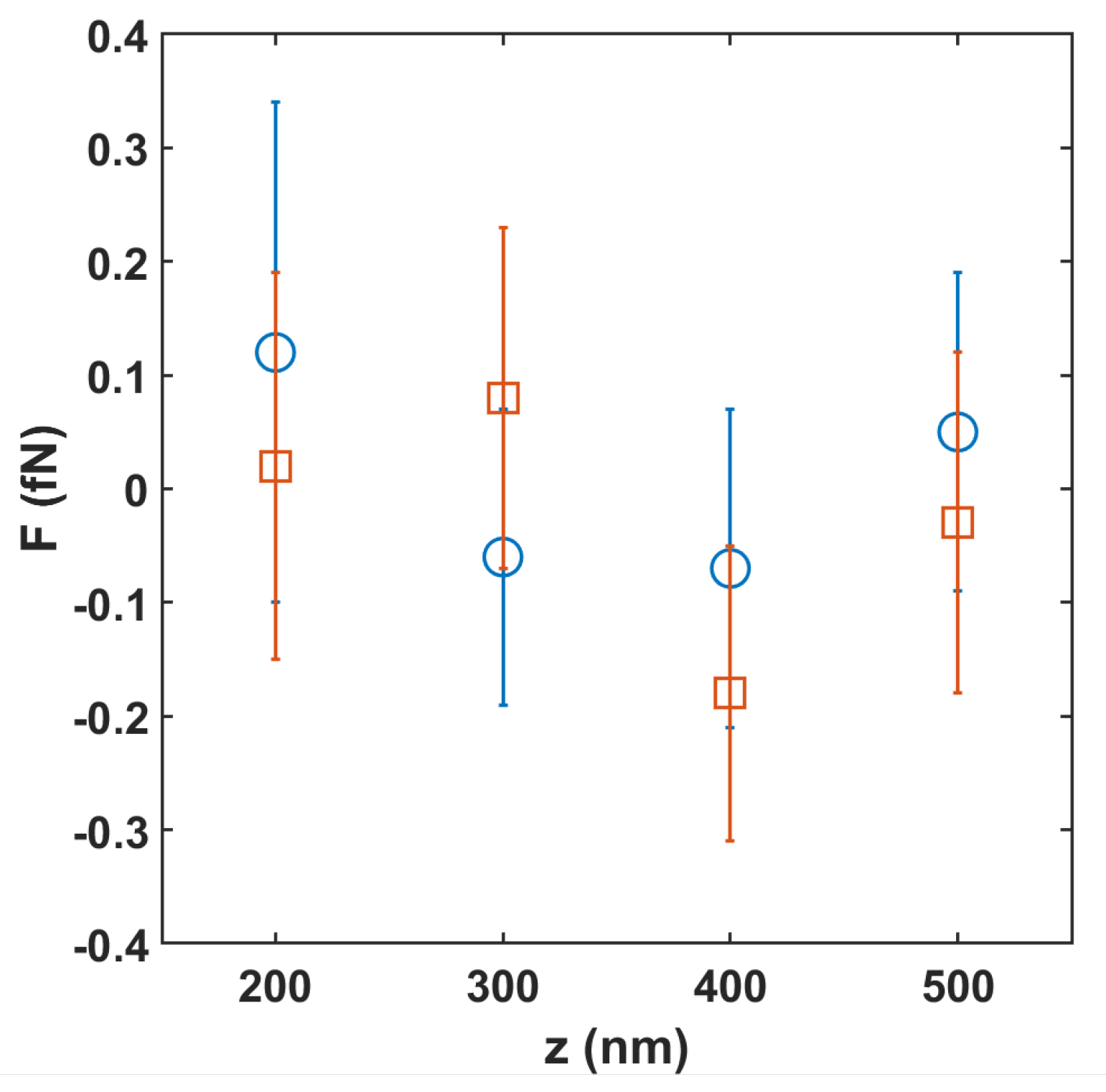
The error bar value is $f_{err}=0.13$ fN at a separation of 300 nm, as shown in Figure 5. Equating the calculated first harmonic of the force to the experimental error bar allows limits on ${\Lambda }_n$ to be extracted,

$${\Lambda }_n=\frac{f_{err}}{A_1^{\left(n\right)}}.$$

(12)

The same method to determine limits was used for the powers of $n=$ 1 to 5.

Table 1. The constraints placed on ${\Lambda }_n$ from our experiment compared to the limits reported in [8]. The two rightmost columns are the limits calculated using the data from the many-sector samples and the large-sector samples.

n	${\mathbf{\Lambda }}_{\mathit{n}}$ from [8]	${\mathbf{\Lambda }}_{\mathit{n}}$ Many-Sector Sample	${\mathbf{\Lambda }}_{\mathit{n}}$ Large-Sector Sample
1	$1\times {10}^{-9}$	$8.0\times {10}^8$	$3.0\times {10}^2$
2	$3.7\times {10}^8$	$6.1\times {10}^{15}$	$2.5\times {10}^{13}$
3	$7.5\times {10}^{19}$	$7.9\times {10}^{24}$	$8.8\times {10}^{23}$
4	$2.2\times {10}^{31}$	$1.2\times {10}^{34}$	$5.2\times {10}^{33}$
5	$6.7\times {10}^{42}$	$1.4\times {10}^{43}$	$1.0\times {10}^{43}$

shows the limits placed on ${\Lambda }_n$ from both the 300 sector source masses used in [11] and the 4 sector source mass with inner radius of 2.5 mm as depicted in Figure 2.

3. Results

The limits presented in Table 1 were calculated using results from an experiment that was not designed to probe power law extensions to the SM. The best limit on ${\Lambda }_n$ with this geometry came from the large-sector sample, but these limits are not better than the ones found in Ref. [8].

The reported values of ${\Lambda }_n$ in this work are large because those are the best limits that can be placed given these experimental data. As an example, for $n=5$, we are not claiming the value of ${\Lambda }_5={10}^{43}$, rather we assert that ${\Lambda }_5$ would need to be of that order for this experiment to have seen its effects, and likewise for the other values of n considered in this work. These are the values for ${\Lambda }_n$ that, for the used geometry, provide an interaction equal to our sensitivity.

4. Discussion

While the experiment did not yield better limits on ${\Lambda }_n$, changes could be made that would improve the system’s sensitivity. For example, if the source masses were designed with a power law in mind, the limits could be further improved. Figure 6 shows the potential limits for ${\Lambda }_5$ as a function of the sample’s radial extent ($\varrho$) for a thickness of 2 mm. The limits have a similar functional dependence with the other dimensions of the sample as the radius increases. The improvement is attributed to the larger volume of interaction improving the sensitivity of the measurement, yielding better limits. However, the improvement gained in the limits by increasing the source mass’s volume quickly diminishes.

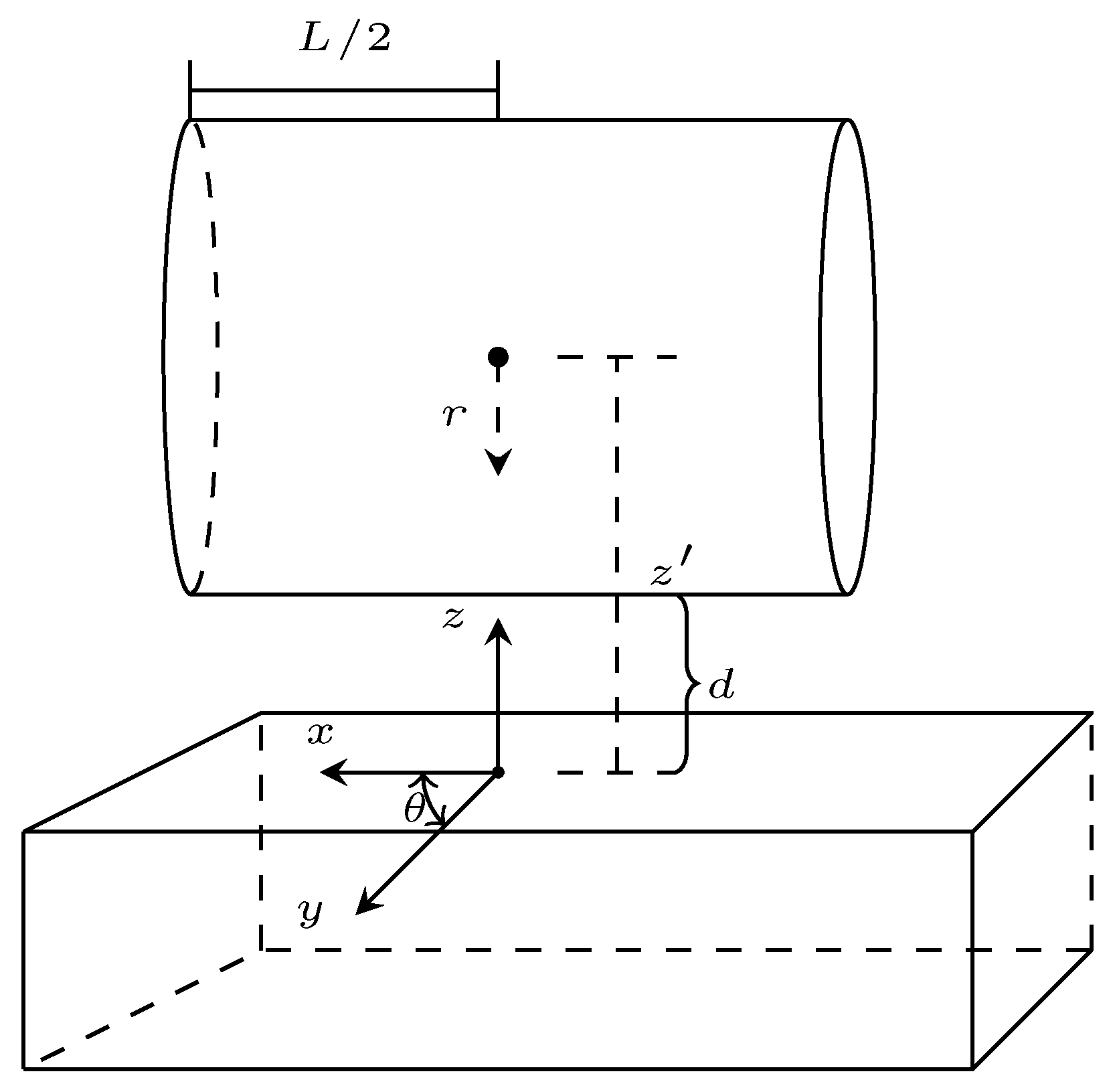
Altering the test mass to also have a larger volume of interaction could provide a small improvement on power law limits. Considering a system, like the one proposed in [14], where the test mass is cylindrical. If the spherical test mass is exchanged for a cylindrical one, and we carry out a similar analysis ot the one described above, the expected limits using the new test mass can be calculated. The details of the calculation can be found in Appendix B. The following is the expression of the force between a cylindrical test mass and an infinite slab with thickness $t=2$ mm along the vertical direction

$$\begin{array}{c}\hfill F_5=\frac{-{\pi }^{2}G{\rho }_1{\rho }_{2}L{\Lambda }_5{r}_0^4}{3}\left(\frac{A_2^2}{(R^2-A_2^2)\sqrt{A_2^2-R^2}}\right.-\left.\frac{A_1^2}{(R^2-A_1^2)\sqrt{A_1^2-R^2}}\right)\end{array}$$

(13)

where $A_1=d+R_{Au}$, $A_2=A_1+t$, and $L=500\mathsf{\mu }$m the length of the cylinder. The expected limits for the powers $n\in [1,5]$ using a cylindrical test mass are listed in

Table 2. The constraints that could be placed on ${\Lambda }_n$ if a cylindrical test mass were used with a source mass designed to probe power law potentials.

n	${\mathbf{\Lambda }}_{\mathit{n}}$ from [8]	Cylindrical Test Mass
1	$1\times {10}^{-9}$	$6.1\times {10}^{-2}$
2	$3.7\times {10}^8$	$4.4\times {10}^{10}$
3	$7.5\times {10}^{19}$	$2.0\times {10}^{22}$
4	$2.2\times {10}^{31}$	$1.1\times {10}^{32}$
5	$6.7\times {10}^{42}$	$2.8\times {10}^{41}$

, calculated using a separation $d=200$ nm. Only the power $n=5$ is expected to improve by about an order of magnitude using a cylinder. Using a cylinder with a length of 500 $\mathsf{\mu }$m and radius of curvature $R=150$ $\mathsf{\mu }$m optimizes the sensitivity given the constraints of the micromechanical oscillator and the lithographic fabrication method of the cylinder.

Other aspects of the experimental design could be change but would either have little impact or have fabrication limitations. One could conceivably use source mass materials that have larger difference in density since, as seen in Equation (10), the response is proportional to the difference in densities between the two materials. Changing the source materials, however, will not improve the signal by more than a factor of ∼2 if using Osmium and air. Certainly, our selection of Au and Si is not optimal, but as they are relatively easy to work with, we think it is a convenient selection. In regards to the test mass, Au is again a suitable choice. A thicker layer of Au would benefit the experiment; however, we started to observe a peel off of the Au from the sphere at 300 nm.

5. Conclusions

Improving the limits of power law extensions to gravity is a challenging endeavor, and new methods are needed to continue to make improvements on short-range interaction experiments. While these types of mechanical measurements have a high precision even at separations of ∼200 nm, this work shows that the size of the masses limit the sensitivity when probing power law interactions. The limit on ${\Lambda }_5$, reported here to be $1.0\times {10}^{43}$, is expected to improve about an order of magnitude the best current limits [8] if a cylindrical test mass were used. The cylinder has a larger interaction volume when compared to a sphere, which results in a higher sensitivity. Several experimental approaches are being developed to test gravity at short distances, seeking signs of deviations potentially due to dark matter effects [15,16,17,18,19,20,21,22]. The description of these new approaches does not explicitly state probing power law extensions to the SM. Researchers should evaluate whether these new techniques are equally suited to test power law models as well as Yukawa-like interactions, which could result in a larger selection of theories being ruled out.