Measurement Techniques for Three-Dimensional Metrology of High Aspect Ratio Internal Features—A Review
Abstract
1. Introduction
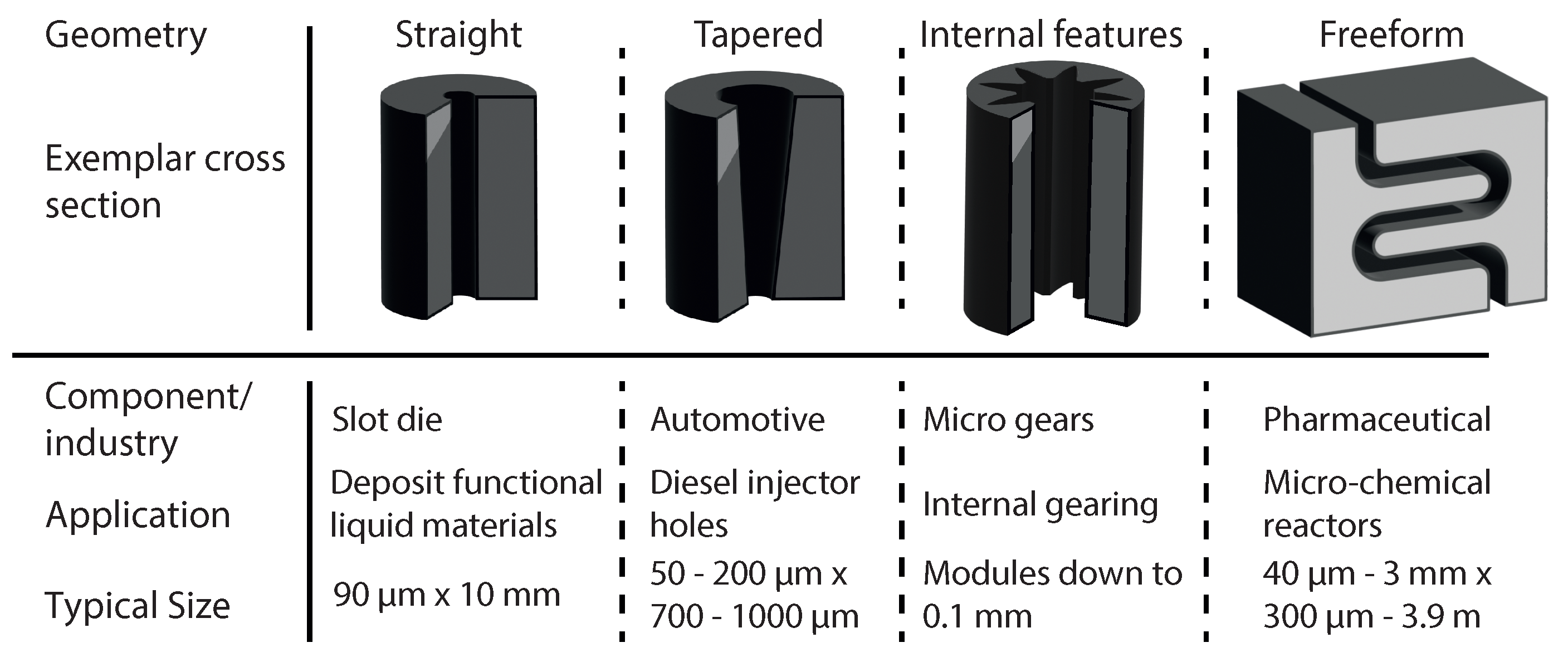
1.1. Exemplar Features
1.2. Measurement System Requirements
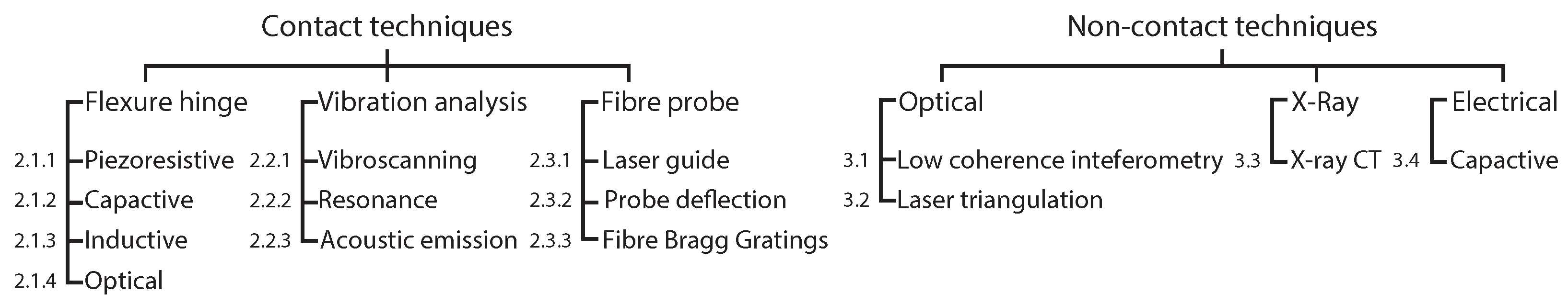
2. Contact Measurement Techniques
2.1. Elastic Mechanism-Based Flexure Probes
2.1.1. Piezoresistive
2.1.2. Capacitive
2.1.3. Inductive
2.1.4. Optical
QPD and PSD
QPD + Interferometric
Interferometric
2.2. Vibrating Probes
2.2.1. Vibroscanning—Electrical Contact
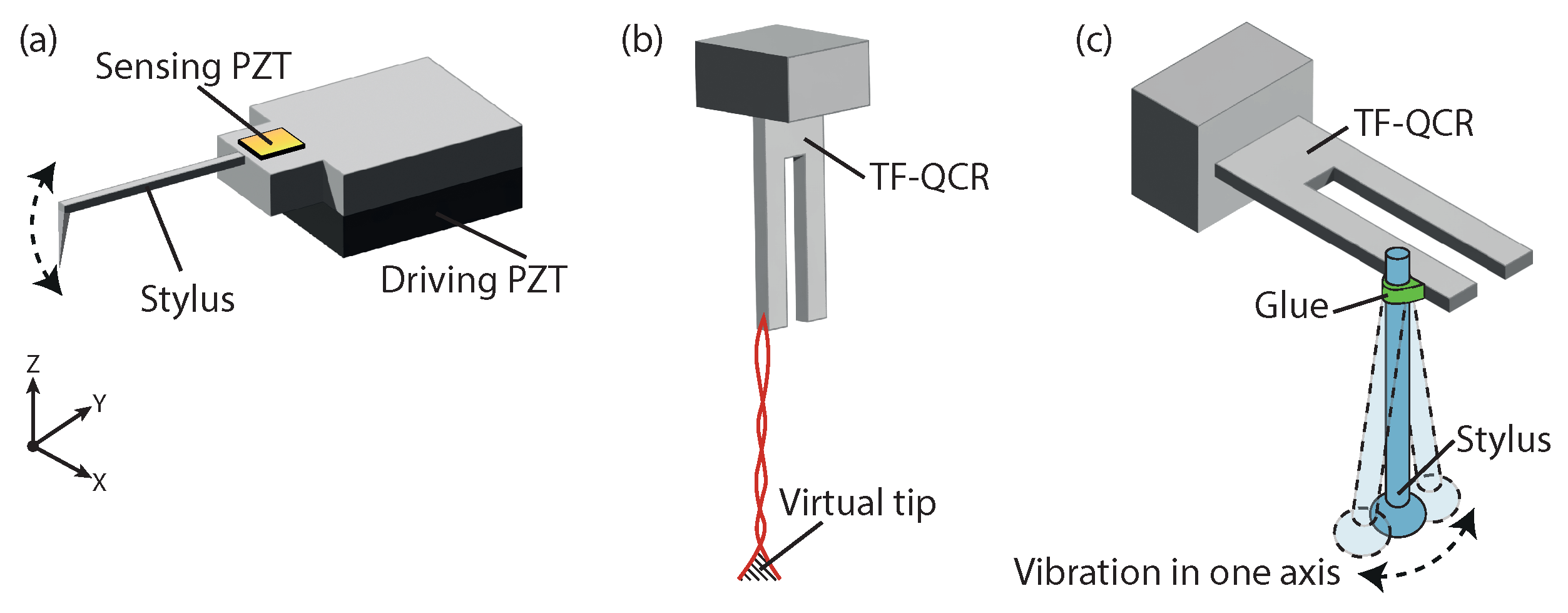
2.2.2. Resonant Vibration Probe
Piezoresistive Vibrating Probe
MEMS
Standing Wave
Shear Force
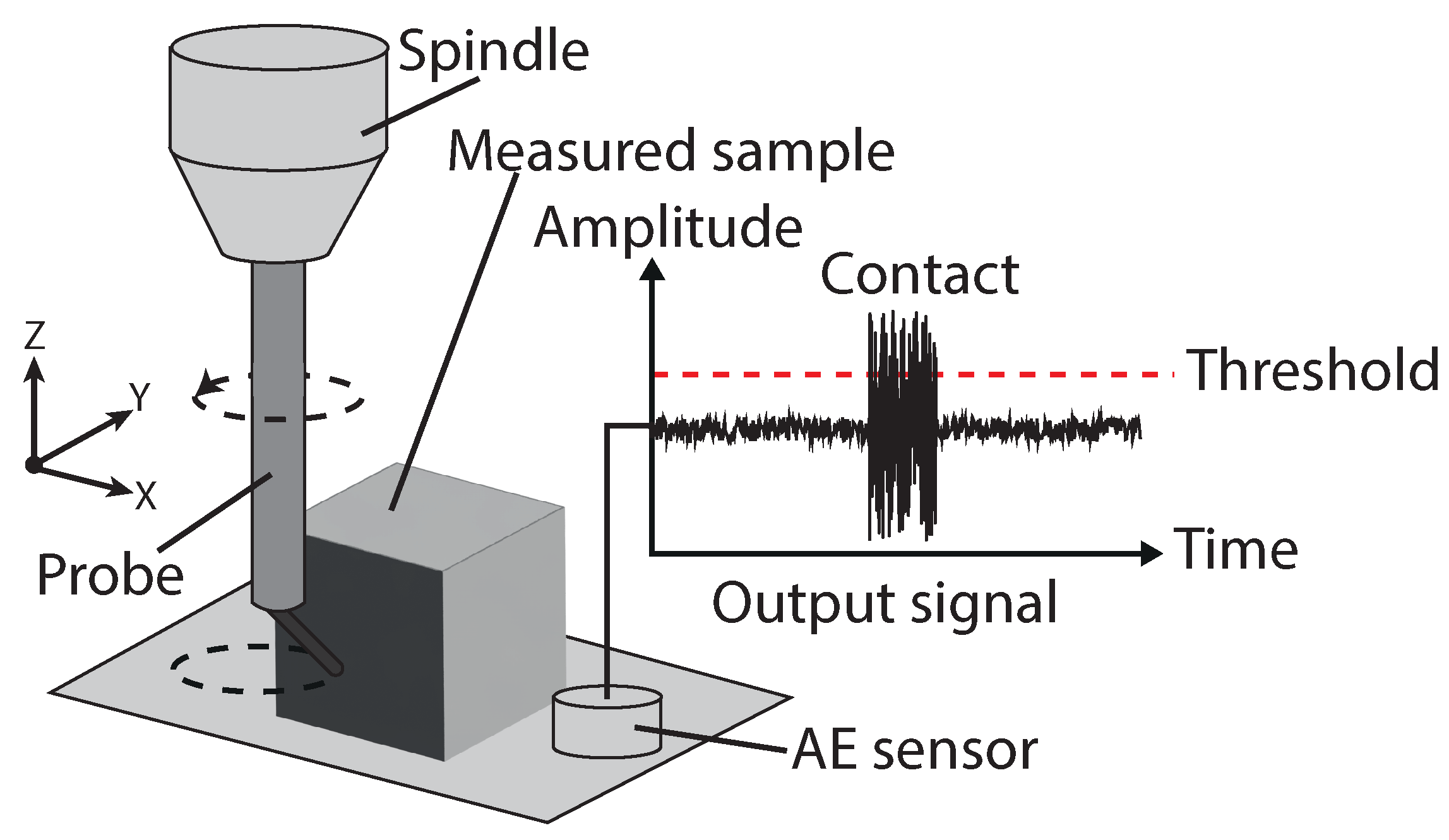
2.2.3. Acoustic Emission
2.3. Fibre-Probe Deflection
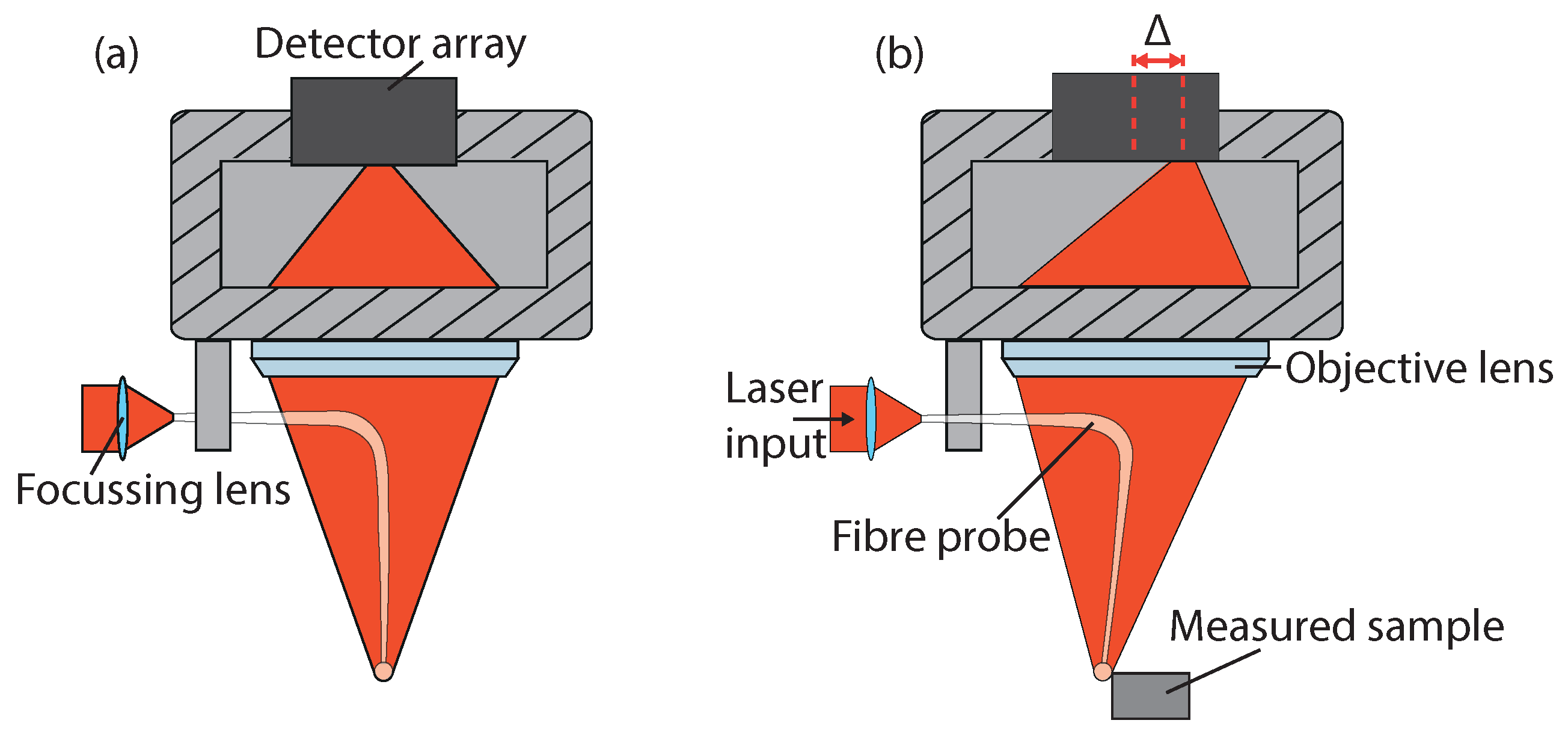
2.3.1. Laser Guidance/Back Reflection
2.3.2. Probe Deflection Detection
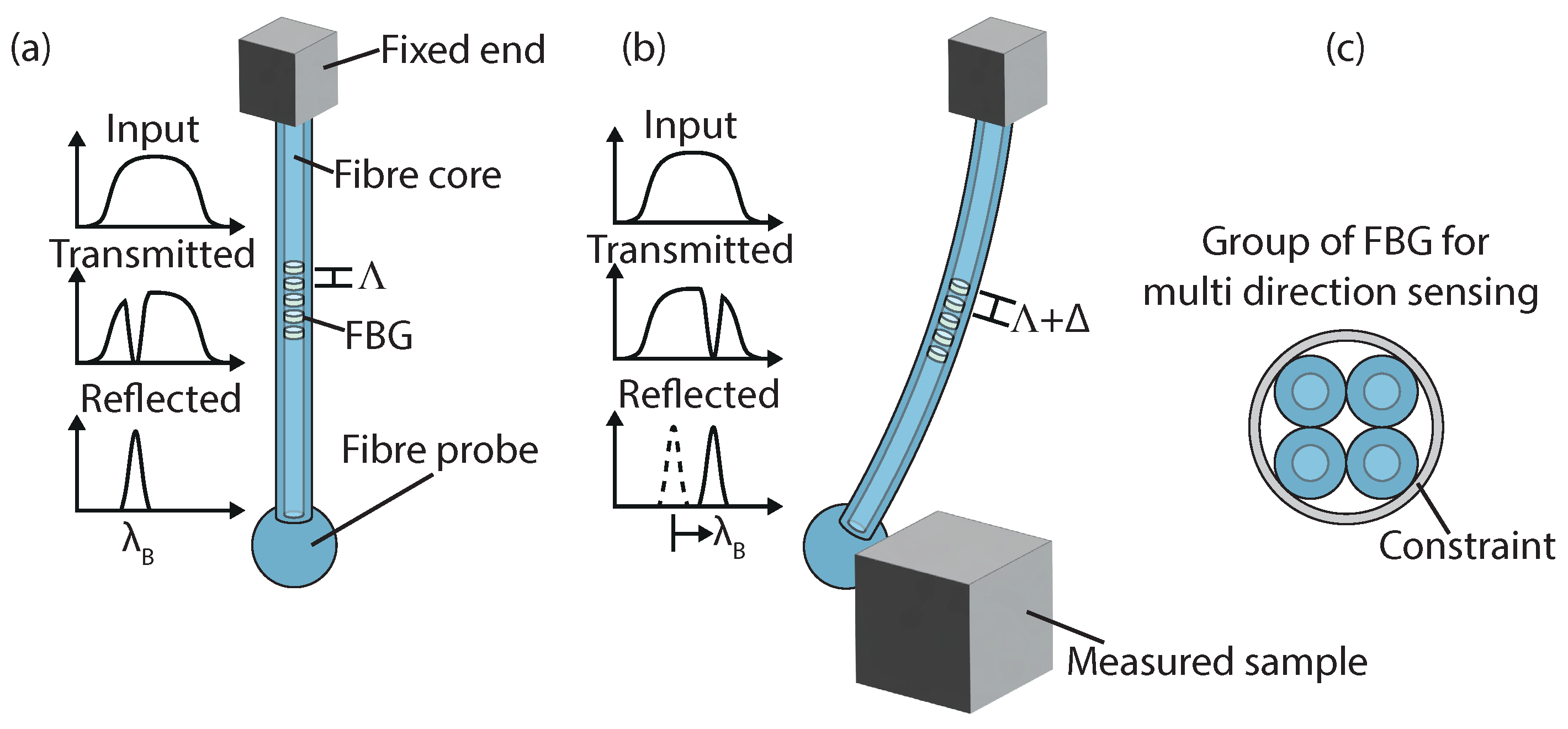
2.3.3. Fibre Bragg Gratings
2.4. Summary of Contact Measurements
3. Non-Contact Measurement Techniques
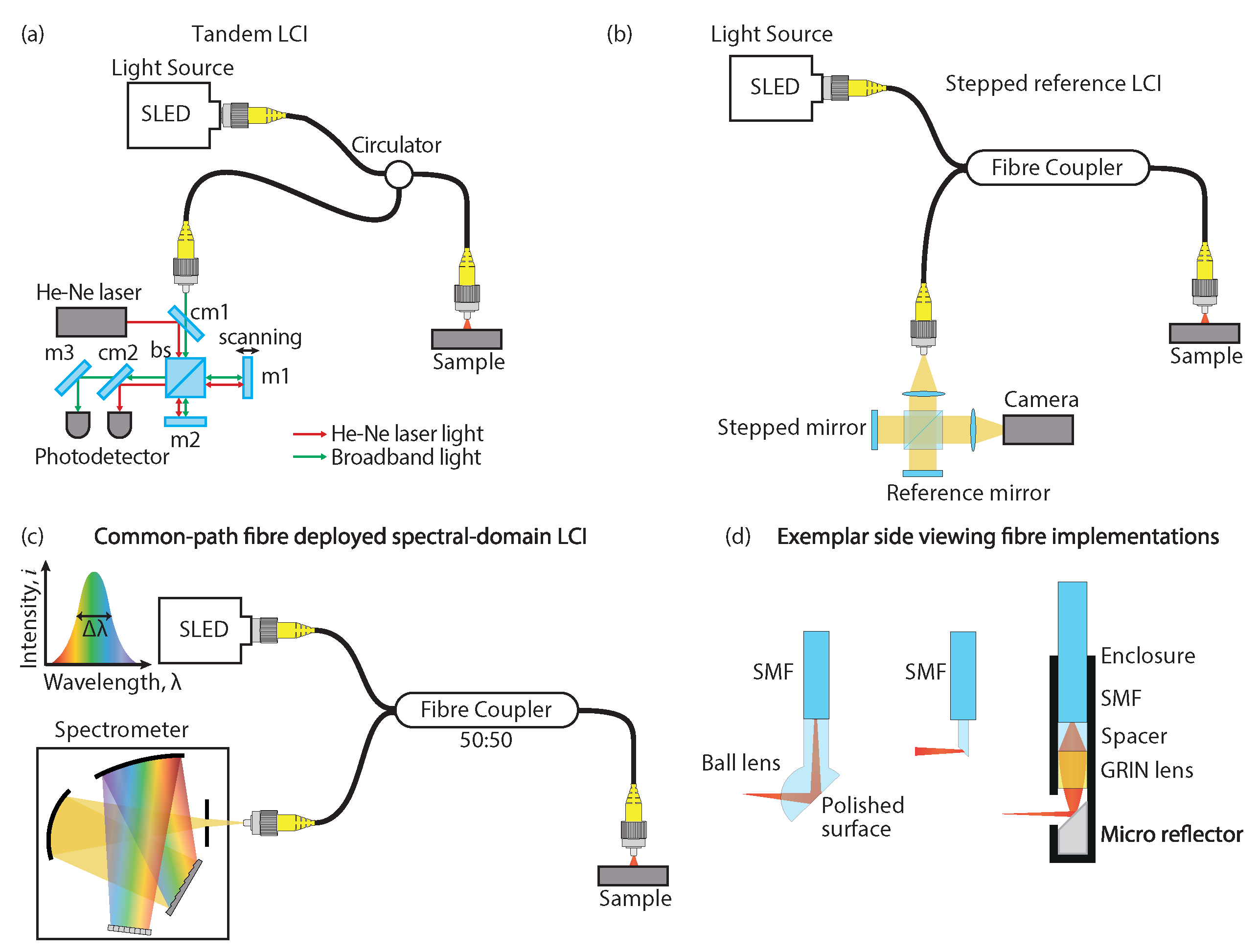
3.1. Low Coherence Interferometry
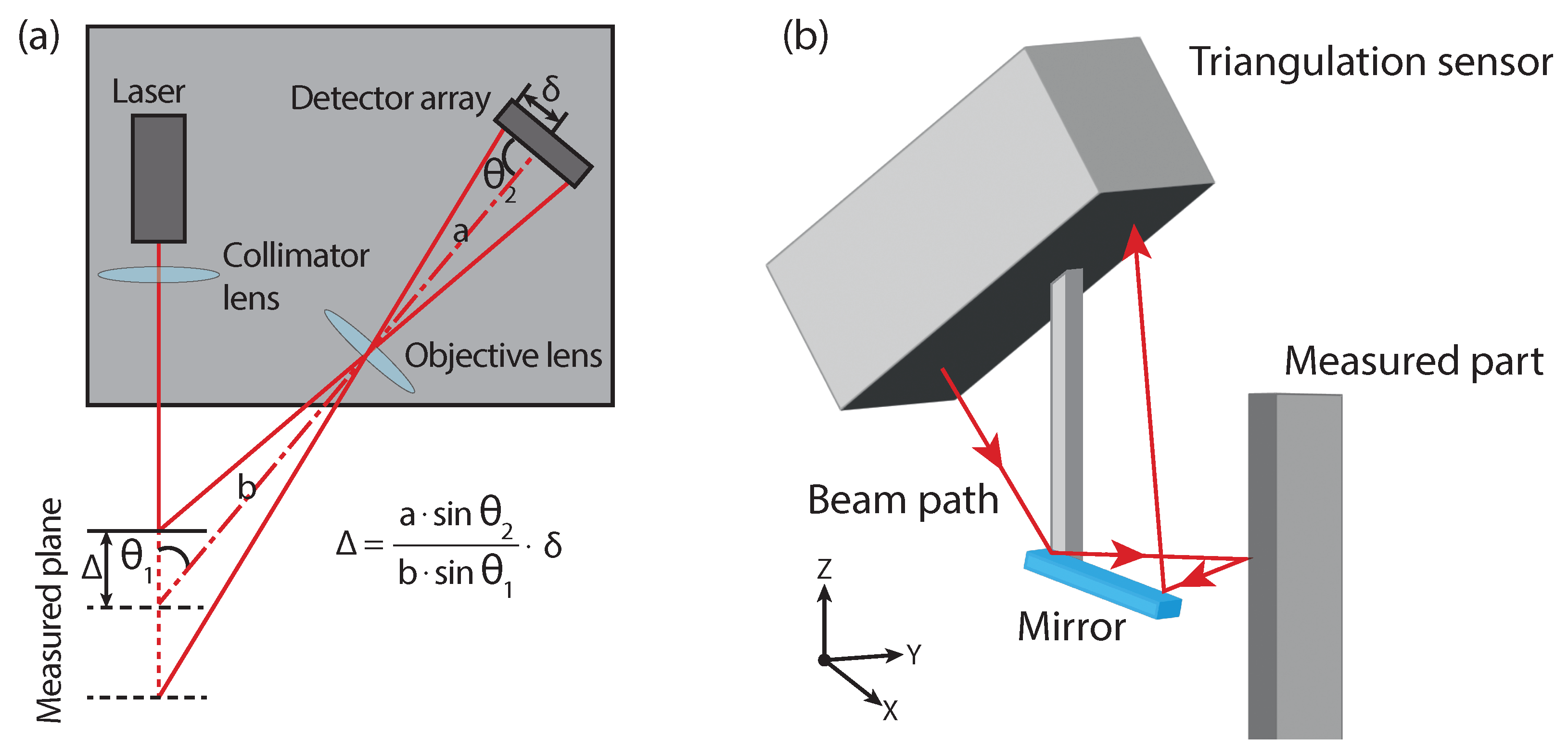
3.2. Laser Triangulation
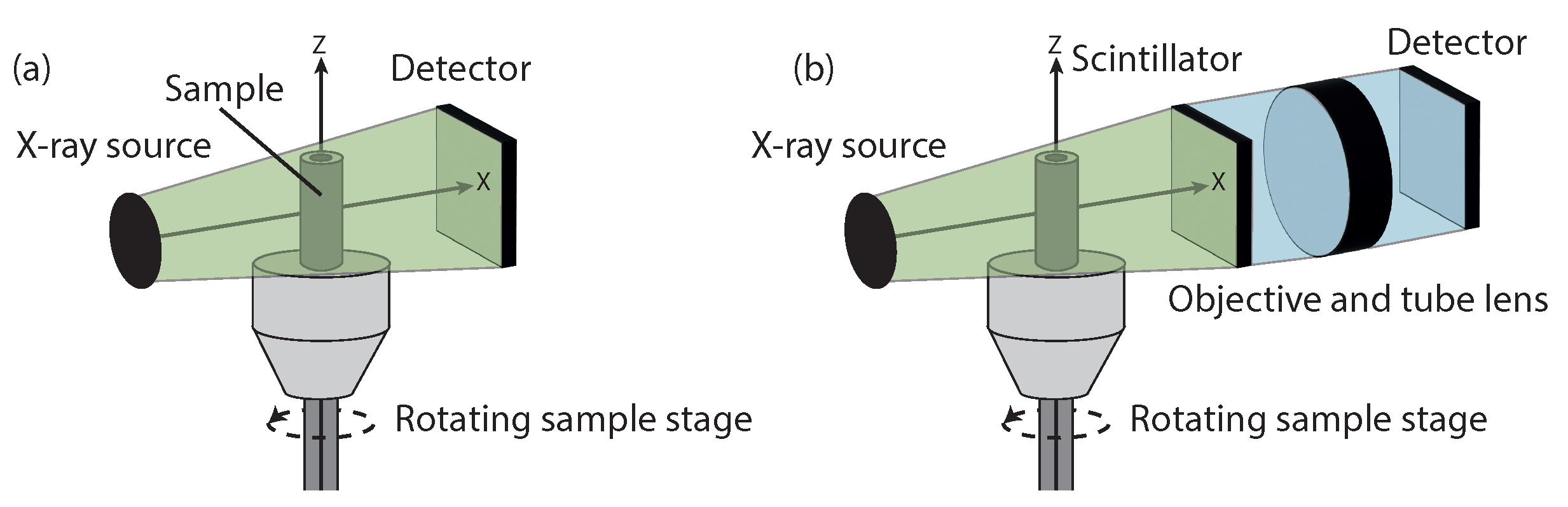
3.3. X-ray Computed Tomography
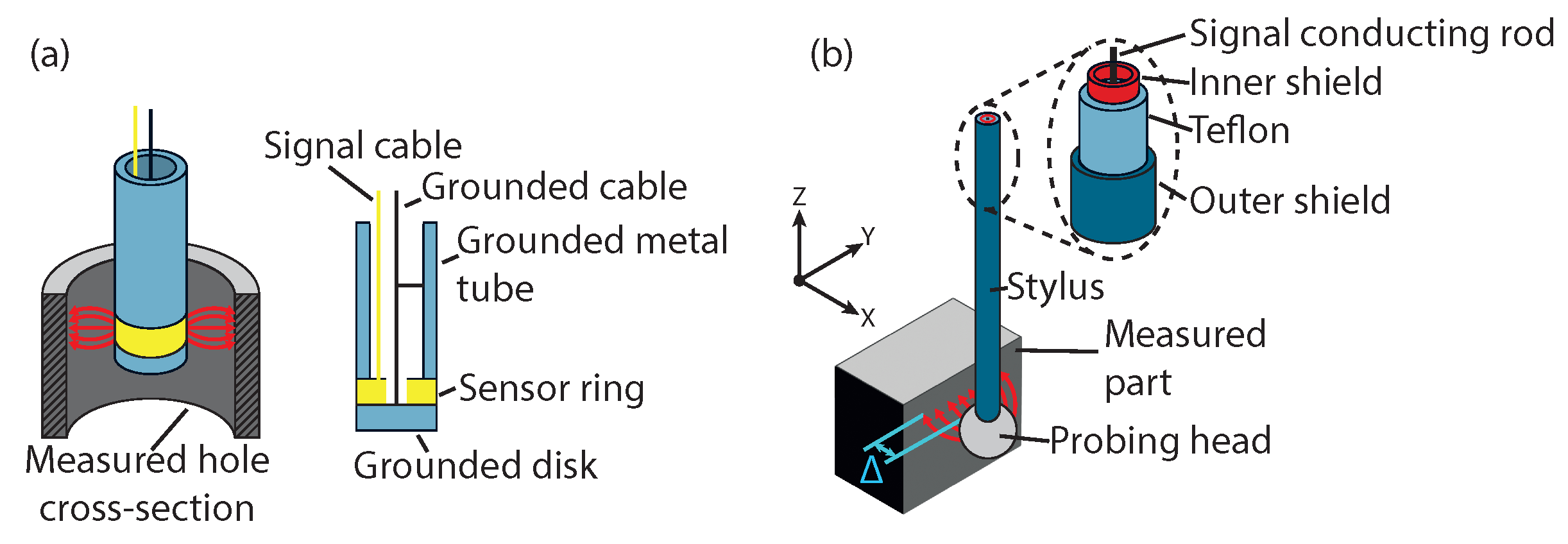
3.4. Capacitive Probes
3.5. Summary of Non-Contact Measurements
4. Challenges
5. Summary and Outlook
Author Contributions
Funding
Conflicts of Interest
References
- Tong, H.; Li, Y.; Zhang, L.; Li, B. Mechanism design and process control of micro EDM for drilling spray holes of diesel injector nozzles. Precis. Eng. 2013, 37, 213–221. [Google Scholar] [CrossRef]
- Yu, P.; Xu, J.; Hou, Y.; Yu, H. Mechanism design and process control of micro-EDM for drilling deep hole of bellows. J. Adv. Manuf. Technol 2021, 115, 2423–2432. [Google Scholar] [CrossRef]
- Aziz, M.; Ohnishi, O.; Onikura, H. Novel micro deep drilling using micro long flat drill with ultrasonic vibration. Precis. Eng. 2012, 36, 168–174. [Google Scholar] [CrossRef]
- Sun, X.Q.; Masuzawa, T.; Fujino, M. Micro ultrasonic machining and its applications in MEMS. Sens. Actuator A Phys. 1996, 57, 159–164. [Google Scholar] [CrossRef]
- Vala, M.; Homola, J. Flexible method based on four-beam interference lithography for fabrication of large areas of perfectly periodic plasmonic arrays. Opt. Express 2014, 22, 18778–18789. [Google Scholar] [CrossRef]
- Meijer, T.; Beardmore, J.; Fabrie, C.; van Lieshout, J.; Notermans, R.; Sang, R.; Vredenbregt, E.; Van Leeuwen, K. Structure formation in atom lithography using geometric collimation. Appl. Phys. B 2011, 105, 703–713. [Google Scholar] [CrossRef]
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Nanoimprint lithography. J. Vac. Sci. 1996, 14, 4129–4133. [Google Scholar] [CrossRef]
- Heckele, M.; Schomburg, W. Review on micro molding of thermoplastic polymers. J. Micromech. Microeng. 2003, 14, R1. [Google Scholar] [CrossRef]
- Pais, M.; Chow, L.; Mahefkey, E. Surface roughness and its effects on the heat transfer mechanism in spray cooling. J. Heat Transfer 1992, 114, 211–219. [Google Scholar] [CrossRef]
- Attinger, D.; Frankiewicz, C.; Betz, A.R.; Schutzius, T.M.; Ganguly, R.; Das, A.; Kim, C.J.; Megaridis, C.M. Surface engineering for phase change heat transfer: A review. MRS Energy Sustain. 2014, 1, E4. [Google Scholar] [CrossRef]
- Grabas, B. Vibration-assisted laser surface texturing of metals as a passive method for heat transfer enhancement. Exp. Therm. Fluid Sci. 2015, 68, 499–508. [Google Scholar] [CrossRef]
- Piasecka, M.; Strąk, K.; Grabas, B. Vibration-assisted laser surface texturing and electromachining for the intensification of boiling heat transfer in a minichannel. Arch. Metall. Mater. 2017, 62, 1983–1990. [Google Scholar] [CrossRef]
- Kumar, G.U.; Suresh, S.; Kumar, C.S.; Back, S.; Kang, B.; Lee, H.J. A review on the role of laser textured surfaces on boiling heat transfer. Appl. Therm. Eng. 2020, 174, 115274. [Google Scholar] [CrossRef]
- Dhadda, G.; Hamed, M.; Koshy, P. Electrical discharge surface texturing for enhanced pool boiling heat transfer. J. Mater. Process. Technol. 2021, 293, 117083. [Google Scholar] [CrossRef]
- Pettersson, U.; Jacobson, S. Influence of surface texture on boundary lubricated sliding contacts. Tribol. Int. 2003, 36, 857–864. [Google Scholar] [CrossRef]
- Hsu, S.M.; Jing, Y.; Zhao, F. Self-adaptive surface texture design for friction reduction across the lubrication regimes. Surf. Topogr. Metrol. Prop. 2015, 4, 014004. [Google Scholar] [CrossRef]
- Uddin, M.; Ibatan, T.; Shankar, S. Influence of surface texture shape, geometry and orientation on hydrodynamic lubrication performance of plane-to-plane slider surfaces. Lubr. Sci. 2017, 29, 153–181. [Google Scholar] [CrossRef]
- Edachery, V.; Shashank, R.; Kailas, S.V. Influence of surface texture directionality and roughness on wettability, sliding angle, contact angle hysteresis, and lubricant entrapment capability. Tribol. Int. 2021, 158, 106932. [Google Scholar] [CrossRef]
- Kubiak, K.; Wilson, M.; Mathia, T.; Carval, P. Wettability versus roughness of engineering surfaces. Wear 2011, 271, 523–528. [Google Scholar] [CrossRef]
- Misyura, S.; Kuznetsov, G.; Feoktistov, D.; Volkov, R.; Morozov, V.; Orlova, E. The influence of the surface microtexture on wettability properties and drop evaporation. Surf. Coat. Technol. 2019, 375, 458–467. [Google Scholar] [CrossRef]
- Hitchcock, S.; Carroll, N.; Nicholas, M. Some effects of substrate roughness on wettability. JMST 1981, 16, 714–732. [Google Scholar] [CrossRef]
- Ijaola, A.O.; Bamidele, E.A.; Akisin, C.J.; Bello, I.T.; Oyatobo, A.T.; Abdulkareem, A.; Farayibi, P.K.; Asmatulu, E. Wettability transition for laser textured surfaces: A comprehensive review. Surf. Interfaces 2020, 21, 100802. [Google Scholar] [CrossRef]
- Wassmann, T.; Kreis, S.; Behr, M.; Buergers, R. The influence of surface texture and wettability on initial bacterial adhesion on titanium and zirconium oxide dental implants. Int. J. Implant Dent 2017, 3, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Bico, J.; Thiele, U.; Quéré, D. Wetting of textured surfaces. Colloids Surf. A 2002, 206, 41–46. [Google Scholar] [CrossRef]
- Ito, S.; Kikuchi, H.; Chen, Y.; Shimizu, Y.; Gao, W.; Takahashi, K.; Kanayama, T.; Arakawa, K.; Hayashi, A. A micro-coordinate measurement machine (CMM) for large-scale dimensional measurement of micro-slits. Appl. Sci. 2016, 6, 156. [Google Scholar] [CrossRef]
- Nam, J.; Carvalho, M.S. Flow in tensioned-web-over-slot die coating: Effect of die lip design. Chem. Eng. Sci. 2010, 65, 3957–3971. [Google Scholar] [CrossRef]
- Pickett, L.M.; Siebers, D.L. An investigation of diesel soot formation processes using micro-orifices. Proc. Combust. Inst. 2002, 29, 655–662. [Google Scholar] [CrossRef]
- Kao, C.C.; Shih, A.J. Form measurements of micro-holes. Meas. Sci. Technol. 2007, 18, 3603. [Google Scholar] [CrossRef]
- Jantzen, S.; Neugebauer, M.; Meeß, R.; Wolpert, C.; Dietzel, A.; Stein, M.; Kniel, K. Novel measurement standard for internal involute microgears with modules down to 0.1 mm. Meas. Sci. Technol. 2018, 29, 125012. [Google Scholar] [CrossRef]
- Santos, R.J.; Sultan, M.A. State of the art of mini/micro jet reactors. Chem. Eng. Technol. 2013, 36, 937–949. [Google Scholar] [CrossRef]
- Yao, X.; Zhang, Y.; Du, L.; Liu, J.; Yao, J. Review of the applications of microreactors. Renew. Sustain. Energy Rev. 2015, 47, 519–539. [Google Scholar] [CrossRef]
- Murakami, H.; Katsuki, A.; Onikura, H.; Sajima, T.; Kawagoishi, N.; Kondo, E. Development of a system for measuring micro hole accuracy using an optical fiber probe. J. Adv. Mech. 2010, 4, 995–1004. [Google Scholar] [CrossRef]
- Bos, E.; Delbressine, F.; Haitjema, H. High-accuracy CMM metrology for micro systems. Vdi Berichte 2004, 1860, 511–522. [Google Scholar]
- Meli, F.; Küng, A. AFM investigation on surface damage caused by mechanical probing with small ruby spheres. Meas. Sci. Technol. 2007, 18, 496. [Google Scholar] [CrossRef]
- Meli, F.; Küng, A.; Thalmann, R. Ultra precision micro-CMM using a low force 3D touch probe. In Proceedings of the Optics and Photonics, San Diego, CA, USA, 24 August 2005; pp. 265–272. [Google Scholar]
- Weckenmann, A.; Estler, T.; Peggs, G.; McMurtry, D. Probing systems in dimensional metrology. CIRP Ann. 2004, 53, 657–684. [Google Scholar] [CrossRef]
- Van Vliet, W.; Schellekens, P. Accuracy limitations of fast mechanical probing. CIRP Ann. 1996, 45, 483–487. [Google Scholar] [CrossRef]
- Küng, A.; Meli, F.; Thalmann, R. Ultraprecision micro-CMM using a low force 3D touch probe. Meas. Sci. Technol. 2007, 18, 319. [Google Scholar] [CrossRef]
- Fang, H.; Xu, B.; Yin, D.; Zhao, S. A method to control dynamic errors of the stylus-based probing system for the surface form measurement of microstructures. J. Nanomater. 2016, 2016, 3727514. [Google Scholar] [CrossRef]
- Yin, Q.; Xu, B.; Yin, G.; Gui, P.; Xu, W.; Tang, B. Surface profile measurement and error compensation of triangular microstructures employing a stylus scanning system. J. Nanomater. 2018, 2018, 6396871. [Google Scholar] [CrossRef]
- Bos, E.J. Aspects of tactile probing on the micro scale. Precis. Eng. 2011, 35, 228–240. [Google Scholar] [CrossRef]
- Claverley, J.D.; Burisch, A.; Leach, R.K.; Raatz, A. Semi-automated assembly of a MEMS-based micro-scale CMM probe and future optimization of the process chain with a view to desktop factory automation. In Proceedings of the Precision Assembly Technologies and Systems; Springer: Berlin/Heidelberg, Germany, 2012; pp. 9–16. [Google Scholar]
- Li, R.; Chen, C.; Li, D.; Fan, K.C.; Cheng, Z.; Huang, Q.; Dang, X. Ball tips of micro/nano probing systems: A review. Chin. J. Mech. 2017, 30, 222–230. [Google Scholar] [CrossRef]
- Ferreira, N.; Brennecke, A.; Dietzel, A.; Büttgenbach, S.; Krah, T.; Metz, D.; Kniel, K.; Härtig, F. Reducing the probe ball diameters of 3D silicon-based microprobes for dimensional metrology. In Proceedings of the 7th International Conference on Sensing Technology, Wellington, New Zealand, 3–5 December 2013; pp. 301–306. [Google Scholar]
- Murakami, H.; Katsuki, A.; Sajima, T.; Uchiyama, K. Fabrication of ultra-small-diameter optical-fiber probe using acid-etch technique and CO2 laser for 3D-micro metrology. Int. J. Autom. 2017, 11, 699–706. [Google Scholar] [CrossRef]
- Sheu, D.Y. Multi-spherical probe machining by EDM: Combining WEDG technology with one-pulse electro-discharge. J. Mater. Process. Technol. 2004, 149, 597–603. [Google Scholar]
- Michihata, M. Surface-Sensing Principle of Microprobe System for Micro-Scale Coordinate Metrology: A Review. Metrology 2022, 2, 46–72. [Google Scholar] [CrossRef]
- Kinnell, P.; Habeb, R. An evaluation of cleaning methods for micro-CMM probes. Meas. Sci. Technol. 2013, 24, 085603. [Google Scholar] [CrossRef]
- Feng, X.; Pascal, J.; Lawes, S. A microscopy approach for in situ inspection of micro-coordinate measurement machine styli for contamination. Meas. Sci. Technol. 2017, 28, 095010. [Google Scholar] [CrossRef]
- Weckenmann, A.; Peggs, G.; Hoffmann, J. Probing systems for dimensional micro-and nano-metrology. Meas. Sci. Technol. 2006, 17, 504. [Google Scholar] [CrossRef]
- Fan, K.C.; Li, R.J.; Xu, P. Design and verification of micro/nano-probes for coordinate measuring machines. Nanomanuf. Metrol. 2019, 2, 1–15. [Google Scholar] [CrossRef]
- Kobayashi, M.; Michihata, M.; Hayashi, T.; Takaya, Y. Coordinate measurement of micro groove on MEMS device by optically controlled microprobe. In Proceedings of the International Symposium on Optomechatronic Technologies, Toronto, ON, Canada, 25–27 October 2010; pp. 1–5. [Google Scholar]
- Yacoot, A.; Koenders, L. Recent developments in dimensional nanometrology using AFMs. Meas. Sci. Technol. 2011, 22, 122001. [Google Scholar] [CrossRef]
- Nouira, H.; Bergmans, R.H.; Küng, A.; Piree, H.; Henselmans, R.; Spaan, H.A. Ultra-high precision CMMs and their associated tactile or/and optical scanning probes. Int. J. Metrol. Qual. Eng. 2014, 5, 204. [Google Scholar] [CrossRef]
- Feng, X.Y.; Xu, P.; Li, R.J.; Lei, Y.J.; Zhang, L.S.; Wang, B.; Huang, Q.X. Development of a High-Resolution Touch Trigger Probe Based on an Optical Lever for Measuring Micro Components. IEEE Sens. J. 2022, 22, 6466–6475. [Google Scholar] [CrossRef]
- Balzer, F.G.; Hausotte, T.; Dorozhovets, N.; Manske, E.; Jäger, G. Tactile 3D microprobe system with exchangeable styli. Meas. Sci. Technol. 2011, 22, 094018. [Google Scholar] [CrossRef]
- Alblalaihid, K.; Lawes, S.; Kinnell, P. Variable stiffness probing systems for micro-coordinate measuring machines. Precis. Eng. 2016, 43, 262–269. [Google Scholar] [CrossRef]
- Alblalaihid, K.; Kinnell, P.; Lawes, S.; Desgaches, D.; Leach, R. Performance assessment of a new variable stiffness probing system for micro-CMMs. Sensors 2016, 16, 492. [Google Scholar] [CrossRef]
- Wang, H.; Zhao, J.; Gao, R.; Yang, Y. A novel constant-force scanning probe incorporating mechanical-magnetic coupled structures. Rev. Sci. Instrum. 2011, 82, 075101. [Google Scholar] [CrossRef]
- Pril, W.; Struik, K.; Schellekens, P. Development of a 2D probing system with nanometer resolution. In Proceedings of the 2th Annual Meeting of the American Society for Precision Engineering, Monterey, CA, USA, 9–14 November 1996; pp. 438–442. [Google Scholar]
- Kleine-Besten, T.; Loheide, S.; Brand, U.; Bütefisch, S.; Büttgenbach, S. Development and characterisation of new probes for dimensional metrology on microsystem components. In Proceedings of the 1st Euspen International Conference; European Society for Precision Engineering and Nanotechnology: Cranfield, UK, 1999; Volume 2, pp. 387–390. [Google Scholar]
- Haitjema, H.; Pril, W.; Schellekens, P. Development of a silicon-based nanoprobe system for 3-D measurements. CIRP Ann. 2001, 50, 365–368. [Google Scholar] [CrossRef]
- Bos, E.; Delbressine, F.; Dietzel, A. Characterization of measurement effects in an MST based nano probe. In Proceedings of the 5th Euspen International Conference; European Society for Precision Engineering and Nanotechnology: Cranfield, UK, 2005; pp. 349–352. [Google Scholar]
- Bos, E.; Heldens, R.; Delbressine, F.; Schellekens, P.; Dietzel, A. Compensation of the anisotropic behavior of single crystalline silicon in a 3D tactile sensor. Sens. Actuator A Phys. 2007, 134, 374–381. [Google Scholar] [CrossRef]
- Bos, E. Tactile 3D Probing System for Measuring MEMS with Nanometer Uncertainty: Aspects of Probing, Design, Manufacturing and Assembly. Ph.D. Thesis, Technische Universiteit Eindhoven, Eindhoven, The Netherlands, 2008. [Google Scholar]
- Dai, G.; Bütefisch, S.; Pohlenz, F.; Danzebrink, H.U. A high precision micro/nano CMM using piezoresistive tactile probes. Meas. Sci. Technol. 2009, 20, 084001. [Google Scholar] [CrossRef]
- Metz, D.; Jantzen, S.; Wessel, D.; Mies, G.; Lüdenbach, J.; Stein, M.; Kniel, K.; Dietzel, A. Integration of an isotropic microprobe and a microenvironment into a conventional CMM. Meas. Sci. Technol. 2019, 30, 115007. [Google Scholar] [CrossRef]
- Peiner, E.; Balke, M.; Doering, L. Slender tactile sensor for contour and roughness measurements within deep and narrow holes. IEEE Sens. J. 2008, 8, 1960–1967. [Google Scholar] [CrossRef]
- Peiner, E.; Balke, M.; Doering, L.; Brand, U. Tactile probes for dimensional metrology with microcomponents at nanometre resolution. Meas. Sci. Technol. 2008, 19, 064001. [Google Scholar] [CrossRef]
- Xu, M.; Kirchhoff, J.; Brand, U. Development of a traceable profilometer for high-aspect-ratio microstructures metrology. Surf. Topogr. Metrol. Prop. 2014, 2, 024002. [Google Scholar] [CrossRef]
- Peggs, G.; Lewis, A.; Oldfield, S. Design for a compact high-accuracy CMM. CIRP Ann. 1999, 48, 417–420. [Google Scholar] [CrossRef]
- Liebrich, T.; Knapp, W. Improvements and experimental validation of a 3D-probing system for micro-components. CIRP Ann. 2012, 61, 475–478. [Google Scholar] [CrossRef]
- He, M.; Liu, R.; Li, Y.; Wang, H.; Lu, X.; Ding, G.; Wu, J.; Zhang, T.; Zhao, X. Tactile probing system based on micro-fabricated capacitive sensor. Sens. Actuators Phys. 2013, 194, 128–134. [Google Scholar] [CrossRef]
- Lei, L.H.; Li, Y.; Fan, G.F.; Wu, J.J.; Jian, L.; Cai, X.Y.; Li, T.B. Development of MEMS-based micro capacitive tactile probe. Chin. Phys. B 2014, 23, 118703. [Google Scholar] [CrossRef]
- Thalmann, R.; Meli, F.; Küng, A. State of the art of tactile micro coordinate metrology. Appl. Sci. 2016, 6, 150. [Google Scholar] [CrossRef]
- van Vliet, W.P.; Schellekens, P.H. Development of a fast mechanical probe for coordinate measuring machines. Precis. Eng. 1998, 22, 141–152. [Google Scholar] [CrossRef]
- Chu, C.L.; Chiu, C.Y. Development of a low-cost nanoscale touch trigger probe based on two commercial DVD pick-up heads. Meas. Sci. Technol. 2007, 18, 1831. [Google Scholar] [CrossRef]
- Li, R.J.; Xiang, M.; Fan, K.C.; Zhou, H.; Feng, J. Optimal design of a touch trigger probe. In Proceedings of the 9th International Symposium on Precision Engineering Measurement and Instrumentation, Changsha, China, 8–10 August 2014; pp. 462–468. [Google Scholar]
- Li, R.J.; Xiang, M.; He, Y.X.; Fan, K.C.; Cheng, Z.Y.; Huang, Q.X.; Zhou, B. Development of a high-precision touch-trigger probe using a single sensor. Appl. Sci. 2016, 6, 86. [Google Scholar] [CrossRef]
- Cui, J.; Bian, X.; He, Z.; Li, L.; Sun, T. A 3D nano-resolution scanning probe for measurement of small structures with high aspect ratio. Sens. Actuator A Phys. 2015, 235, 187–193. [Google Scholar] [CrossRef]
- Li, R.J.; Xu, P.; Wang, P.Y.; Fan, K.C.; Cheng, R.J.; Huang, Q.X. Development of a micro/nano probing system using double elastic mechanisms. Sensors 2018, 18, 4229. [Google Scholar] [CrossRef]
- Balzer, F.; Hofmann, N.; Dorozhovets, N.; Hausotte, T.; Manske, E.; Jäger, G. Investigation of the metrological properties of a 3-D microprobe with optical detection system. In Proceedings of the Instrumentation, Metrology, and Standards for Nanomanufacturing IV; SPIE: Paris, France, 2010; Volume 7767, pp. 168–177. [Google Scholar]
- Li, R.J.; Fan, K.C.; Miao, J.W.; Huang, Q.X.; Tao, S.; Gong, E.m. An analogue contact probe using a compact 3D optical sensor for micro/nano coordinate measuring machines. Meas. Sci. Technol. 2014, 25, 094008. [Google Scholar] [CrossRef]
- Li, R.J.; Fan, K.C.; Huang, Q.X.; Zhou, H.; Gong, E.M.; Xiang, M. A long-stroke 3D contact scanning probe for micro/nano coordinate measuring machine. Precis. Eng. 2016, 43, 220–229. [Google Scholar] [CrossRef]
- Liebrich, T.; Knapp, W. New concept of a 3D-probing system for micro-components. CIRP Ann. 2010, 59, 513–516. [Google Scholar] [CrossRef]
- Hidaka, K.; Saito, A.; Koga, S. Study of a micro-roughness probe with ultrasonic sensor. CIRP Ann. 2008, 57, 489–492. [Google Scholar] [CrossRef]
- Masuzawa, T.; Hamasaki, Y.; Fujino, M. Vibroscanning method for nondestructive measurement of small holes. CIRP Ann. 1993, 42, 589–592. [Google Scholar] [CrossRef]
- Kim, B.J.; Sawamoto, Y.; Masuzawa, T.; Fujino, M. Advanced vibroscanning method for micro-hole measurement, High speed and stability improvement of measurement technique. Int. J. Electr. Mach. 1996, 1, 41–44. [Google Scholar]
- Masuzawa, T.; Kim, B.; Bergaud, C.; Fujino, M. Twin-probe vibroscanning method for dimensional measurement of microholes. CIRP Ann. 1997, 46, 437–440. [Google Scholar] [CrossRef]
- Kim, B.; Masuzawa, T.; Fujita, H.; Tominaga, A. Dimensional measurement of microholes with silicon-based micro twin probes. In Proceedings of the 11th Annual International Workshop on Micro Electro Mechanical Systems: An Investigation of Micro Structures, Sensors, Actuators, Machines and Systems, Heidelberg, Germany, 25–29 January 1998; pp. 334–339. [Google Scholar]
- Kim, B.; Masuzawa, T.; Bourouina, T. The vibroscanning method for the measurement of micro-hole profiles. Meas. Sci. Technol. 1999, 10, 687. [Google Scholar] [CrossRef]
- Van Riel, M.; Bos, E.; Homburg, F. Analysis of the measurement sensitivity of multidimensional vibrating microprobes. Meas. Sci. Technol. 2014, 25, 075008. [Google Scholar] [CrossRef]
- Yamamoto, M.; Takeuchi, H.; Aoki, S. Dimensional measurement of high aspect ratio micro structures with a resonating micro cantilever probe. Microsyst. Technol. 2000, 6, 179–183. [Google Scholar] [CrossRef]
- Hidaka, K.; Schellekens, P. Study of a small-sized ultrasonic probe. CIRP Ann. 2006, 55, 567–570. [Google Scholar] [CrossRef]
- Claverley, J.D.; Leach, R.K. A vibrating micro-scale CMM probe for measuring high aspect ratio structures. Microsyst. Technol. 2010, 16, 1507–1512. [Google Scholar] [CrossRef]
- Claverley, J.D.; Leach, R.K. Development of a three-dimensional vibrating tactile probe for miniature CMMs. Precis. Eng. 2013, 37, 491–499. [Google Scholar] [CrossRef]
- Goj, B.; Dressler, L.; Hoffmann, M. Design and characterization of a resonant triaxial microprobe. J. Micromech. Microeng. 2015, 25, 125011. [Google Scholar] [CrossRef]
- Bauza, M.B.; Hocken, R.J.; Smith, S.T.; Woody, S.C. Development of a virtual probe tip with an application to high aspect ratio microscale features. Rev. Sci. Instrum. 2005, 76, 095112. [Google Scholar] [CrossRef]
- Bauza, M.B.; Woody, S.C.; Seugling, R.M.; Smith, S.T. Dimensional Measurements of Ultra Delicate Materials Using Micrometrology Tactile Sensing; Technical Report; Lawrence Livermore National Lab: Livermore, CA, USA, 2010.
- Bauza, M.; Woody, S.; Woody, B.; Smith, S. Surface profilometry of high aspect ratio features. Wear 2011, 271, 519–522. [Google Scholar] [CrossRef]
- Huang, Q.; Chen, C.; Wu, K.; Zhang, L.; Li, R.; Fan, K.C. A three-dimensional resonant triggering probe for micro-CMM. Appl. Sci. 2017, 7, 403. [Google Scholar] [CrossRef]
- Ito, S.; Kodama, I.; Gao, W. Development of a probing system for a micro-coordinate measuring machine by utilizing shear-force detection. Meas. Sci. Technol. 2014, 25, 064011. [Google Scholar] [CrossRef]
- Ito, S.; Chen, Y.L.; Shimizu, Y.; Kikuchi, H.; Gao, W.; Takahashi, K.; Kanayama, T.; Arakawa, K.; Hayashi, A. Uncertainty analysis of slot die coater gap width measurement by using a shear mode micro-probing system. Precis. Eng. 2016, 43, 525–529. [Google Scholar] [CrossRef]
- Ito, S.; Shima, Y.; Kato, D.; Matsumoto, K.; Kamiya, K. Development of a Microprobing System for Side Wall Detection Based on Local Surface Interaction Force Detection. Int. J. Autom. 2020, 14, 91–98. [Google Scholar] [CrossRef]
- Goo, C.S.; Jun, M.B.; Saito, A. Probing system for measurement of micro-scale components. J. Manuf. Process 2012, 14, 174–180. [Google Scholar] [CrossRef]
- Elfurjani, S.; Bayesteh, A.; Park, S.; Jun, M. Dimensional measurement based on rotating wire probe and acoustic emission. Measurement 2015, 59, 329–336. [Google Scholar] [CrossRef]
- Elfurjani, S.; Ko, J.; Jun, M.B. Micro-scale hole profile measurement using rotating wire probe and acoustic emission contact detection. Measurement 2016, 89, 215–222. [Google Scholar] [CrossRef]
- Schwenke, H.; Wäldele, F.; Weiskirch, C.; Kunzmann, H. Opto-tactile sensor for 2D and 3D measurement of small structures on coordinate measuring machines. CIRP Ann. 2001, 50, 361–364. [Google Scholar] [CrossRef]
- Tutsch, R.; Andras, M.; Neuschaefer-Rube, U.; Petz, M.; Wiedenhofer, T.; Wissmann, M. Three dimensional tactile-optical probing for the measurement of microparts. In Proceedings of the SENSOR + TEST; AMA: Seattle, WA, USA, 2009; pp. 139–144. [Google Scholar]
- Cui, J.; Li, L.; Tan, J. Optical fiber probe based on spherical coupling of light energy for inner-dimension measurement of microstructures with high aspect ratios. Opt. Lett. 2011, 36, 4689–4691. [Google Scholar] [CrossRef]
- Cui, J.; Li, L.; Tan, J. Opto-tactile probe based on spherical coupling for inner dimension measurement. Meas. Sci. Technol. 2012, 23, 085105. [Google Scholar] [CrossRef]
- Cui, J.; Li, L.; Li, J.; Tan, J.B. Fiber probe for micro-hole measurement based on detection of returning light energy. Sens. Actuators A Phys. 2013, 190, 13–18. [Google Scholar] [CrossRef]
- Cui, J.; Chen, Y.; Tan, J. Improvement of dimensional measurement accuracy of microstructures with high aspect ratio with a spherical coupling fiber probe. Meas. Sci. Technol. 2014, 25, 075902. [Google Scholar] [CrossRef]
- Chen, Y.; Cui, J.; Tan, J. Spherical coupling probe for measuring inner dimensions of microstructures. Opt. Express 2018, 26, 33523–33533. [Google Scholar] [CrossRef] [PubMed]
- Muralikrishnan, B.; Stone, J.; Vemuri, S.; Sahay, C.; Potluri, A.; Stoup, J. Fiber deflection probe for small hole measurements. In Proceedings of the ASPE Annual Meeting, New Orleans, LA, USA, 19–22 September 2004; pp. 24–27. [Google Scholar]
- Muralikrishnan, B.; Stone, J.; Stoup, J.R. Fiber deflection probe for small hole metrology. Precis. Eng. 2006, 30, 154–164. [Google Scholar] [CrossRef]
- Tan, J.; Wang, F.; Cui, J. Fiber deflection probing method based on micro focal-length collimation. Opt. Express 2010, 18, 2925–2933. [Google Scholar] [CrossRef] [PubMed]
- Murakami, H.; Katsuki, A.; Onikura, H.; Sajima, T.; Kawagoishi, N.; Kondo, E.; Honda, T. An Optical Fiber Probe for 3-D Micro Metrology. In Proceedings of the Key Engineering Materials; Trans Tech Publications Ltd.: Bach, Switzerland, 2010; Volume 447, pp. 524–528. [Google Scholar]
- Sajima, T.; Murakami, H.; Katsuki, A.; Tabuchi, D.; Ohnishi, O.; Kurokawa, S.; Onikura, H.; Doi, T.K. Precision profile measurement system for microholes using vibrating optical fiber. Sens. Mater. 2012, 24, 387–396. [Google Scholar]
- Murakami, H.; Katsuki, A.; Sajima, T. Analysis and evaluation of surface force effects in vibrating fiber probing system for 3-D micro structure measurements. In Proceedings of the Key Engineering Materials; Trans Tech Publications Ltd.: Bach, Switzerland, 2012; Volume 523, pp. 907–912. [Google Scholar]
- Murakami, H.; Katsuki, A.; Sajima, T.; Suematsu, T. Study of a vibrating fiber probing system for 3-D micro-structures: Performance improvement. Meas. Sci. Technol. 2014, 25, 094010. [Google Scholar] [CrossRef]
- Cui, J.; Li, J.; Feng, K.; Tan, J. Three-dimensional fiber probe based on orthogonal micro focal-length collimation for the measurement of micro parts. Opt. Express 2015, 23, 26386–26398. [Google Scholar] [CrossRef]
- Cui, J.; Li, J.; Feng, K.; Tan, J.; Zhang, J. A 3D fiber probe based on orthogonal micro focal-length collimation and fiber Bragg grating. Meas. Sci. Technol. 2016, 27, 074005. [Google Scholar] [CrossRef]
- Murakami, H.; Katsuki, A.; Sajima, T.; Uchiyama, K.; Yoshida, I.; Hamano, Y.; Honda, H. Development of measurement system for microstructures using an optical fiber probe: Improvement of measurable region and depth. Meas. Sci. Technol. 2020, 31, 075902. [Google Scholar] [CrossRef]
- Murakami, H.; Katsuki, A.; Sajima, T.; Uchiyama, K.; Yoshida, I.; Hamano, Y.; Honda, H. Investigation of factors affecting sensitivity enhancement of an optical fiber probe for microstructure measurement using oblique incident light. Appl. Sci. 2020, 10, 3191. [Google Scholar] [CrossRef]
- Murakami, H.; Katsuki, A.; Sajima, T.; Uchiyama, K.; Sata, Y. Development of the contact detection method in free-form surface of the optical fiber probe for microstructure measurement using long short term memory. Meas. Sens. 2021, 18, 100170. [Google Scholar] [CrossRef]
- Zou, L.; Ni, H.; Zhang, P.; Ding, X. Assembled Cantilever Fiber Touch Trigger Probe for Three-Dimensional Measurement of Microstructures. Sensors 2017, 17, 2652. [Google Scholar] [CrossRef]
- Ji, H.; Hsu, H.; Kong, L.; Wedding, A. Development of a contact probe incorporating a Bragg grating strain sensor for nano coordinate measuring machines. Meas. Sci. Technol. 2009, 20, 095304. [Google Scholar] [CrossRef]
- Liu, F.; Fei, Y.; Xia, H.; Chen, L. A new micro/nano displacement measurement method based on a double-fiber Bragg grating (FBG) sensing structure. Meas. Sci. Technol. 2012, 23, 054002. [Google Scholar] [CrossRef]
- Cui, J.; Feng, K.; Zhu, S.; Zhang, Y.; Hu, Y.; Tan, J. Development of FBG probes for dimensional metrology with micro parts of high aspect ratio. J. Mod. Opt. 2013, 60, 2001–2011. [Google Scholar] [CrossRef]
- Cui, J.; Feng, K.; Li, J.; Tan, J. Development of a double fiber probe with a single fiber Bragg grating for dimensional measurement of microholes with high aspect ratios. Opt. Lett. 2014, 39, 2868–2871. [Google Scholar] [CrossRef]
- Feng, K.; Cui, J.; Zhao, S.; Li, J.; Tan, J. A twin FBG probe and integration with a microhole-measuring machine for the measurement of microholes of high aspect ratios. IEEE-ASME Trans. Mechatron. 2016, 21, 1242–1251. [Google Scholar] [CrossRef]
- Feng, K.; Cui, J.; Dang, H.; Zhang, H.; Zhao, S.; Tan, J. Four-cores FBG probe based on capillary self-assembly fabrication technique for 3D measurement. IEEE Photon. Technol. Lett. 2016, 28, 2339–2342. [Google Scholar] [CrossRef]
- Liu, F.; Chen, L.; Wang, J.; Xia, H.; Li, R.; Yu, L.; Fei, Y. Modeling and prototyping of a fiber Bragg grating-based dynamic micro-coordinate measuring machine probe. Meas. Sci. Technol. 2016, 27, 025016. [Google Scholar] [CrossRef]
- Liu, F.; Wang, J.; Chen, L.; Li, R.; Xia, H.; Yu, L. Development and characterization of a high-sensitivity fiber Bragg grating-based vibrating nano-probe for 3D measurement. Sens. Rev. 2018, 39, 199–207. [Google Scholar] [CrossRef]
- Schmitt, R.; Pfeifer, T.; Depiereux, F.; König, N. Novel fiber-optical interferometer with miniaturized probe for in-hole measurements. Optoelectron Lett. 2008, 4, 140–142. [Google Scholar] [CrossRef]
- Zhao, W.; Tan, J.; Qiu, L.; Zou, L. A new laser heterodyne confocal probe for ultraprecision measurement of discontinuous contours. Meas. Sci. Technol. 2005, 16, 497. [Google Scholar] [CrossRef]
- Ehrig, W.; Neuschaefer-Rube, U.; Neugebauer, M.; Meeß, R. Traceable optical coordinate metrology applications for the micro range. In Proceedings of the Three-Dimensional Imaging Metrology; SPIE: Paris, France, 2009; Volume 7239, pp. 134–143. [Google Scholar]
- Fang, H.; Xu, B.; Chen, W.; Tang, H.; Zhao, S. A slope-adapted sample-tilting method for profile measurement of microstructures with steep surfaces. Nanomaterials 2015, 2015, 2. [Google Scholar] [CrossRef]
- Miliou, A. In-fiber interferometric-based sensors: Overview and recent advances. Photonics 2021, 8, 265. [Google Scholar] [CrossRef]
- Huang, D.; Swanson, E.A.; Lin, C.P.; Schuman, J.S.; Stinson, W.G.; Chang, W.; Hee, M.R.; Flotte, T.; Gregory, K.; Puliafito, C.A.; et al. Optical coherence tomography. Science 1991, 254, 1178–1181. [Google Scholar] [CrossRef] [PubMed]
- Pfeifer, T.; Schmitt, R.; Konig, N.; Mallmann, G.F. Interferometric measurement of injection nozzles using ultra-small fiber-optical probes. Chin. Opt. Lett. 2011, 9, 071202. [Google Scholar] [CrossRef]
- Matsui, K.; Matsumoto, H.; Takahashi, S.; Takamasu, K. New non-contact measurement of small inside-diameter using tandem low-coherence interferometer and optical fiber devices. In Proceedings of the Key Engineering Materials; Trans Tech Publications Ltd.: Bach, Switzerland, 2012; Volume 523, pp. 871–876. [Google Scholar]
- Hovell, T.; Petzing, J.; Justham, L.; Kinnell, P. From light to displacement: A design framework for Optimising spectral-domain low-coherence interferometric sensors for in situ measurement. Appl. Sci. 2020, 10, 8590. [Google Scholar] [CrossRef]
- Hovell, T.; Matharu, R.S.; Petzing, J.; Justham, L.; Kinnell, P. Lensless fiber-deployed low-coherence interferometer for in-situ measurements in nonideal environments. Opt. Eng. 2020, 59, 014113. [Google Scholar] [CrossRef]
- Hovell, T.; Petzing, J.; Justham, L.; Kinnell, P. In-situ measurement of electrochemical jet machining using low coherence interferometry. In Proceedings of the Euspen’s 20th International Conference & Exhibition; Euspen: Bedford, UK, 2020. [Google Scholar]
- Malak, M.; Marty, F.; Nouira, H.; Salgado, J.; Bourouina, T. All-silicon interferometric optical probe for non-contact dimensional measurements in confined environments. In Proceedings of the 25th International Conference on Micro Electro Mechanical Systems (MEMS), Paris, France, 29 January–2 February 2012; pp. 628–631. [Google Scholar]
- Hovell, T.; Petzing, J.; Justham, L.; Kinnell, P. Pragmatic micrometre to millimetre calibration using multiple methods for low-coherence interferometer in embedded metrology applications. Sensors 2021, 21, 5101. [Google Scholar] [CrossRef]
- Zhang, F.; Qu, X.; Ouyang, J. An automated inner dimensional measurement system based on a laser displacement sensor for long-stepped pipes. Sensors 2012, 12, 5824–5834. [Google Scholar] [CrossRef]
- Tong, Q.b.; Jiao, C.q.; Huang, H.; Li, G.b.; Ding, Z.l.; Yuan, F. An automatic measuring method and system using laser triangulation scanning for the parameters of a screw thread. Meas. Sci. Technol. 2014, 25, 035202. [Google Scholar] [CrossRef]
- Hošek, J.; Linduška, P. Simple Modification of a Commercial Laser Triangulation Sensor for Distance Measurement of Slot and Bore Side Surfaces. Sensors 2021, 21, 6911. [Google Scholar] [CrossRef]
- Li, X.Q.; Wang, Z.; Fu, L.H. A laser-based measuring system for online quality control of car engine block. Sensors 2016, 16, 1877. [Google Scholar] [CrossRef]
- Villarraga-Gómez, H.; Herazo, E.L.; Smith, S.T. X-ray computed tomography: From medical imaging to dimensional metrology. Precis. Eng. 2019, 60, 544–569. [Google Scholar] [CrossRef]
- Wang, S. Investigation of X-ray computed tomography for dimensional measurement. In Proceedings of the Euspen SIG: Advancing Precision in Additive Manufacturing; Euspen: Bedford, UK, 2019. [Google Scholar]
- Villarraga-Gómez, H.; Kotwal, N.; Parwani, R.; Weiß, D.; Krenkel, M.; Kimmig, W.; Vom Hagen, C.G. Improving the dimensional accuracy of 3D X-ray microscopy data. Meas. Sci. Technol. 2022, 33, 074002. [Google Scholar] [CrossRef]
- Ice, G.E.; Budai, J.D.; Pang, J.W. The race to x-ray microbeam and nanobeam science. Science 2011, 334, 1234–1239. [Google Scholar] [CrossRef]
- Wu, Z.j.; Li, Z.l.; Huang, W.d.; Gong, H.f.; Gao, Y.; Deng, J.; Hu, Z.j. Comparisons of nozzle orifice processing methods using synchrotron X-ray micro-tomography. J. Zhejiang Univ. Sci. A 2012, 13, 182–188. [Google Scholar] [CrossRef]
- Villarraga-Gómez, H.; Lee, C.; Smith, S.T. Dimensional metrology with X-ray CT: A comparison with CMM measurements on internal features and compliant structures. Precis. Eng. 2018, 51, 291–307. [Google Scholar] [CrossRef]
- Lee, N.K.; Chow, J.K.; Chan, A.C. Design of precision measurement system for metallic hole. J. Adv. Manuf. Technol 2009, 44, 539–547. [Google Scholar] [CrossRef]
- Tan, J.; Cui, J. Ultraprecision 3D probing system based on spherical capacitive plate. Sens. Actuator A Phys. 2010, 159, 1–6. [Google Scholar] [CrossRef]
- Chen, S.T.; Lin, S.m. Development of a capacitive sensing technology for the measurement of perpendicularity in the narrow, deep slot-walls of micromolds. Microelectron. Reliab. 2018, 83, 216–222. [Google Scholar] [CrossRef]
- Xingyuan, B.; Yesheng, L.; Yamin, Z.; Jiubin, T.; Junning, C. Processing circuits of weak capacitance signal for spherical scattering electrical-field probing sensor. In Proceedings of the 14th International Conference on Electronic Measurement & Instruments (ICEMI); IEEE: Piscataway, NJ, USA, 2019; pp. 692–697. [Google Scholar]
- Bian, X.; Cui, J.; Lu, Y.; Tan, J. Ultraprecision diameter measurement of small holes with large depth-to-diameter ratios based on spherical scattering electrical-field probing. Appl. Sci. 2019, 9, 242. [Google Scholar] [CrossRef]
- Bian, X.; Cui, J.; Lu, Y.; Zhao, Y.; Cheng, Z.; Tan, J. Quantitative investigation of surface charge distribution and point probing characteristics of spherical scattering electrical field probe for precision measurement of miniature internal structures with high aspect ratios. Appl. Sci. 2020, 10, 5268. [Google Scholar] [CrossRef]
- Pourciel, J.B.; Jalabert, L.; Masuzawa, T. Profile and surface measurement tool for high aspect-ratio microstructures. JSME Int. J. Ser. C 2003, 46, 916–922. [Google Scholar] [CrossRef]
- Kim, J.; de Mathelin, M.; Ikuta, K.; Kwon, D.S. Advancement of flexible robot technologies for endoluminal surgeries. Proc. IEEE 2022, 110, 909–931. [Google Scholar] [CrossRef]
- Hong, W.; Schmitz, A.; Bai, W.; Berthet-Rayne, P.; Xie, L.; Yang, G.Z. Design and compensation control of a flexible instrument for endoscopic surgery. In Proceedings of the International Conference on Robotics and Automation (ICRA), Paris, France, 31 May 2020–31 August 2020; pp. 1860–1866. [Google Scholar]



| Parameter | Piezoresistive [60,61,62,64,65,66,67,68,69,70] | Capacitive [71,72,73,74] | Inductive [35,38,75] | Optical [55,56,76,77,78,79,80,81,82,83,84,85] |
|---|---|---|---|---|
| Shaft length (mm) | 1.5–6.8 | 2.3–15.3 | - | 5–150 |
| Shaft diameter (m) | 30–500 | 200–1000 | - | 200–2000 |
| Probe head (m) | 22–300 | 300–1000 | 100–1000 | 150–4000 |
| Probing force (mN) | 0.001–15 | 0.02–11.6 | 0.5 | 58–5200 |
| Stiffness (N/m) | 10–3654 | 10–337.8 | 20 | 0.1–11 |
| Measurement range (m) | 30–400 | 1–20 | 200 | 0.5–100 |
| Resolution (nm) | 0.1–500 | 3–71 | - | 0.5–100 |
| Uncertainty (k = 1) (nm) | 10–100 | 11–110 | 50 | 0.14–250 |
| Repeatability (k = 1) (nm) | 1.3–4.4 | - | 5 | 1.7–95 |
| Probing speed (mm/s) | 0.02–1 | - | 0.5 | 0.005–70 |
| Parameter | Vibroscanning [87,88,89,90,91] | Resonant [25,93,94,95,96,97,98,99,100,101,102,103,104] | Acoustic Emission [105,106,107] |
|---|---|---|---|
| Shaft length (mm) | 0.11–3 | 0.9–5 | 10 |
| Shaft diameter (m) | 20–160 | 5–200 | - |
| Probe head (m) | 100–150 | 10–200 | 1.63 to 302.2 |
| Probing force (mN) | 0.0005–6 | 0.0001–0.05 | - |
| Stiffness (N/m) | 0.75 | 40–500 | - |
| Measurement range (m) | 4–10 | 0.1–50 | - |
| Resolution (nm) | - | 0.38–45 | 100 |
| Uncertainty (k = 1) (nm) | - | 39.2 | - |
| Repeatability (k = 1) (nm) | 0.5 | 56–2000 | 126–861 |
| Probing speed (mm/s) | 0.05–0.5 | 1.4 ×10–1 | 0.05–0.6 |
| Measurement speed (Hz) | 100 Hz | 5 to 500 | 100,000 |
| Parameter | Laser Guide [28,108,109,110,111,112,113,114] | Probe Deflection [32,115,116,117,118,119,120,121,122,123,124,125,126,127] | FBG [128,129,130,131,132,133,134,135] |
|---|---|---|---|
| Shaft length (mm) | 1–30 | 1–30 | 0.75–12.5 |
| Shaft diameter (m) | 15–200 | 0.4–125 | 2–125 |
| Probe head (m) | 10–300 | 1–200 | 102.5–280 |
| Probing force (mN) | 0.001–0.01 | 0.0002–26 | 0.001–2.94 |
| Stiffness (N/m) | 1 | 10 | 20 |
| Measurement range (m) | 10–40 | 0.18–20 | 2.6–10 |
| Resolution (nm) | 10–50 | 1–30 | 8–60 |
| Uncertainty (k = 1) (nm) | - | 22.5–55 | 200 |
| Repeatability (k = 1) (nm) | 50–300 | 20.6–54.4 | 19.9–256 |
| Probing speed (mm/s) | - | 0.0001 to 0.01 | - |
| Measurement speed (Hz) | - | 440 | - |
| Parameter | LCI [140,141,142,143,144,145,146,147,148] | Laser Triangulation [149,150,151,152] | XCT [153,154,155,156,157,158] | Capacative [159,160,161,162,163,164] |
|---|---|---|---|---|
| Shaft length (mm) | 4–50 | 12.5–550 | N/A | 100–150 |
| Shaft diameter (m) | 30–550 | 3700–18,000 | N/A | 30–2200 |
| Probe head (m) | 30–800 | 3700–18,000 | N/A | 1000–3000 |
| Measurement range (m) | 160–2100 | 1500–5000 | 500–65,000 | 0.2–200 |
| Resolution, XY (nm) | 7000–40,000 | 110,000–650,000 | 40–40,000 | - |
| Resolution, Z (nm) | 5500–46,000 | 30–1300 | 40–40,000 | 1–150 |
| Uncertainty (k = 1) (nm) | 16.9–89 | 1300 | 500–25,000 | 100 |
| Repeatability (k = 1) (nm) | 25.5–100 | 1100–5900 | 40–1400 | 1000–3000 |
| Linearity (k = 1) (nm) | 16.9–67.6 | 9 | - | 6–10 |
| Measurement speed (Hz) | Hz–kHz | Hz–kHz | 0.25–1.5 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hovell, T.; Petzing, J.; Guo, W.; Gill, C.; Justham, L.; Lohse, N.; Kinnell, P. Measurement Techniques for Three-Dimensional Metrology of High Aspect Ratio Internal Features—A Review. Metrology 2023, 3, 139-168. https://doi.org/10.3390/metrology3020009
Hovell T, Petzing J, Guo W, Gill C, Justham L, Lohse N, Kinnell P. Measurement Techniques for Three-Dimensional Metrology of High Aspect Ratio Internal Features—A Review. Metrology. 2023; 3(2):139-168. https://doi.org/10.3390/metrology3020009
Chicago/Turabian StyleHovell, Tom, Jon Petzing, Wen Guo, Connor Gill, Laura Justham, Niels Lohse, and Peter Kinnell. 2023. "Measurement Techniques for Three-Dimensional Metrology of High Aspect Ratio Internal Features—A Review" Metrology 3, no. 2: 139-168. https://doi.org/10.3390/metrology3020009
APA StyleHovell, T., Petzing, J., Guo, W., Gill, C., Justham, L., Lohse, N., & Kinnell, P. (2023). Measurement Techniques for Three-Dimensional Metrology of High Aspect Ratio Internal Features—A Review. Metrology, 3(2), 139-168. https://doi.org/10.3390/metrology3020009







