Evaluation of Thermodynamic and Kinetic Contributions to Over-Extraction of Extractables by Nonpolar Organic Solvents in Comparison to Lipids in Exhaustive and Exaggerated Extractions of Medical Devices Based on Abraham Solvation Model and Solvent–Material Interactions Using Low-Density Polyethylene as a Representative Material
Abstract
1. Introduction
- (1)
- Correlation of the partition constant between LDPE and lipids by the Abraham solvation model. The constructed (predictive) models are then used to predict the partition constant of extractables observed for LDPE over a wide hydrophobicity range.
- (2)
- Calculation of the partition constant between LDPE and hexane for the same LDPE extractables.
- (3)
- Assessment of the dependence of LDPE-lipid and LDPE-hexane partition constants on using extractables observed for LDPE.
- (4)
- Evaluation of the adequacy of the LDPE-lipid and LDPE-hexane predictive models.
- (5)
- Comparison of the range and difference of two sets of partition constants (LDPE-lipid and LDPE-hexane) for the observed LDPE extractables to understand the thermodynamic effect on over-extraction.
- (6)
- Study of LDPE-lipid interactions by the adsorption of lipids into LDPE in comparison to alkanes and other organic solvents.
- (7)
- Assessment of the range of solvent diffusion coefficients by both types of solvents.
- (8)
- Estimation of the extent of over-extractions by alkanes.
2. Methods
- Development of the Abraham solvation working models to correlate the partition constant () between LDPE and lipids using: (1) multiple linear regression (MLR) analysis of the measured partition constant (), and (2) a thermodynamic cycle method [55,56]. The solutes used in the model construction are directly taken from the ref [47]. These predictive models are used to predict the of experimentally observed LDPE extractables over a wide range of hydrophobicity.
- Establishment of empirical relationships between the partition constant and using experimentally observed LDPE extractables.
- Establishment of empirical relationships between the partition constant and using the same experimentally observed LDPE extractables for a comparison purpose.
- Comparison of the dependence of on (s: lipids or hexane) between hexane and lipids to assess the extractability of LDPE by hexane and lipids from a thermodynamic perspective.
- Justification of the suitability of the constructed solvation models in the prediction.
- Assessment of the practical implications of the difference between and values on over-extraction by hexane (or alkanes).
- Assessment of the material-solvent interactions between LDPE and alkanes/lipids and their influence on over-extraction.
- Compile and estimate the degree of over-extraction of LDPE material by alkane solvents relative to lipids/oils.
2.1. Abraham Solvation Model
2.2. Construction of Abraham Solvation Model for LDPE-Lipid Partition Constant
2.3. Construction of Abraham Solvation Model for LDPE-Hexane Partition Constant
2.4. Calculation of LDPE-Lipid and LDPE-Hexane Partition Constants of Observed LDPE Extractables
2.5. Evaluation of Dependence of and on of Observed LDPE Extractables
2.6. Suitability of Use of Constructed Models to Predict and for the Observed LDPE Extractables
2.7. Calculation of of Measured and LDPE Extractables Compounds
2.8. Differentiation between Thermodynamic and Kinetic Contributions
2.9. Estimation of Over-Extraction of LDPE by Alkanes Compared to Lipids
- (1)
- Direct calculation by:
- (2)
- Estimation by kinetic diffusion coefficients as follows:
3. Experimental
3.1. Collection of LDPE-Lipid Partition Constants
3.2. Collection of Observed LDPE Extractables
3.3. Compilation of Abraham Solvation Model Constants of LDPE-Water and Lipid-Water Partition Systems
3.4. Determination of Descriptors of Collected Compounds
3.5. Construction and Correlation of LDPE-Lipid Partition Constant by Abraham Solvation Model
3.6. Calculation of of Representative Extractables Compounds
3.7. Calculation of and for Observed Extractables Compounds
3.8. Correlation of and for Observed Extractables Compounds for Model Verification
3.9. Collection of Solvent Absorption and Swelling Data for LDPE
3.10. Estimation of Over-Extraction Data of LDPE by Alkanes
4. Results and Discussion
4.1. Correlation of LDPE-Lipid Partition Constant by Solvation Model
4.1.1. The Space of Abraham Solute Descriptors
4.1.2. Construction by the Multiple Linear Regression (MLR)
4.1.3. Construction by the Thermodynamic Cycle Conversion
4.2. Calculation of LDPE-Lipid and LDPE-Hexane Partition Constant Range for Collected LDPE Extractables
4.3. Dependence of on for LDPE Extractables
4.4. Model Validation
4.5. Comparison of MLR and Cycle Models in Predicting LDPE-Lipid Partition Constants for Observed LDPE Extractables
4.6. Comparison of LDPE-Hexane and LDPE-Lipid Partition Constants for LDPE Extractables
4.7. Thermodynamic Contribution to Over-Extraction of LDPE by Hexane
4.8. Determination of Over-Extraction of LDPE by Hexane over Lipid
4.8.1. Material-Solvent Interactions
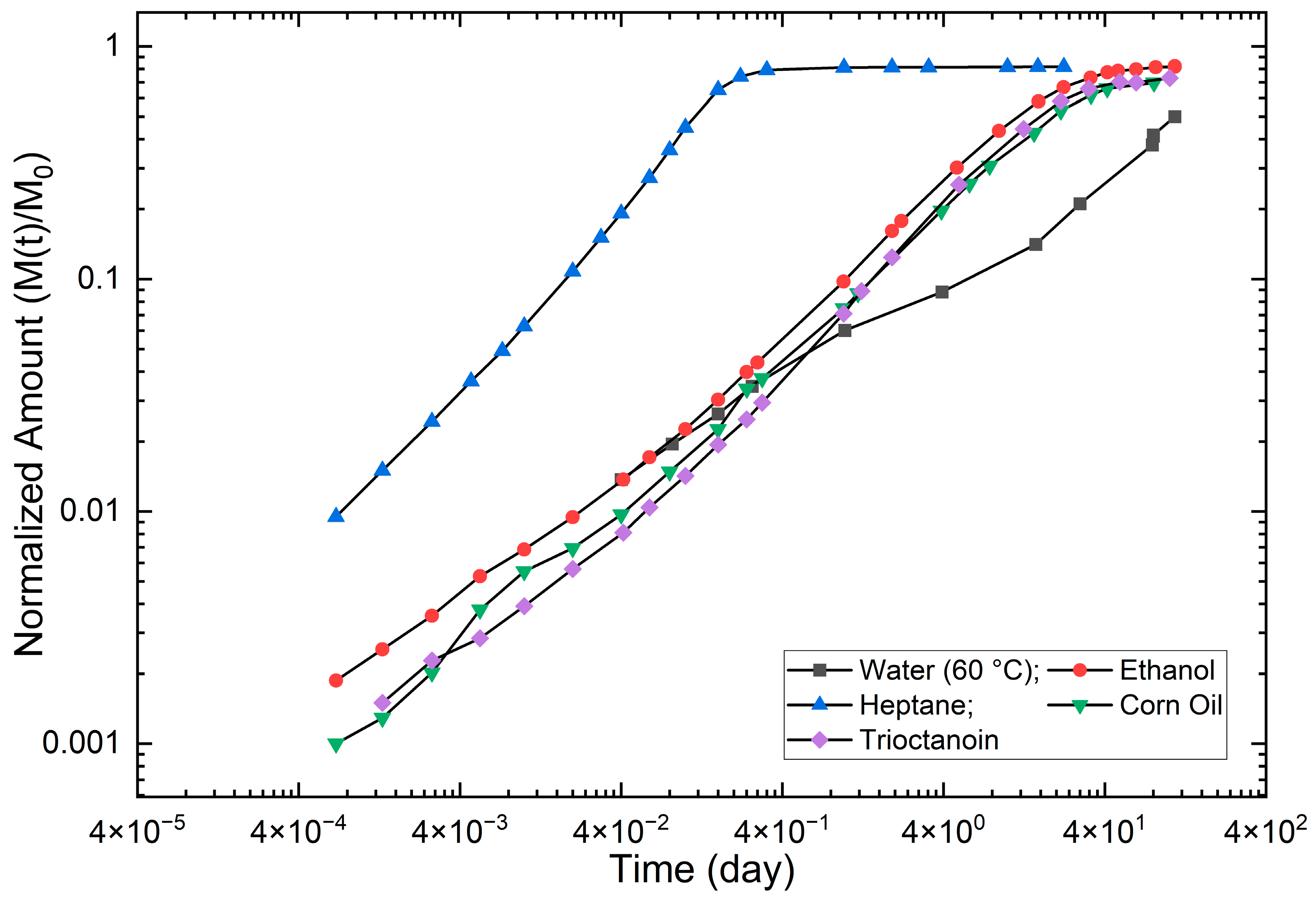
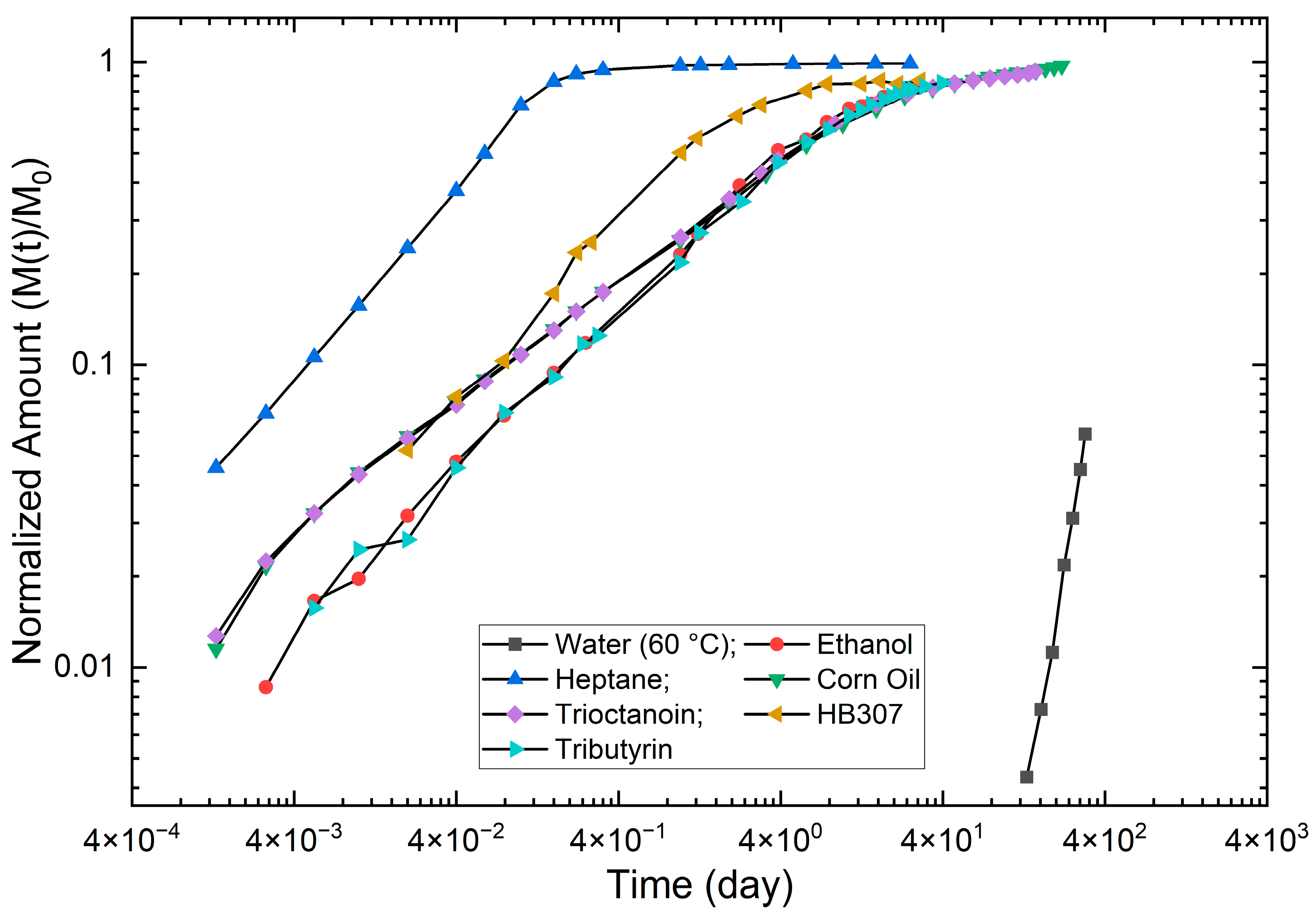
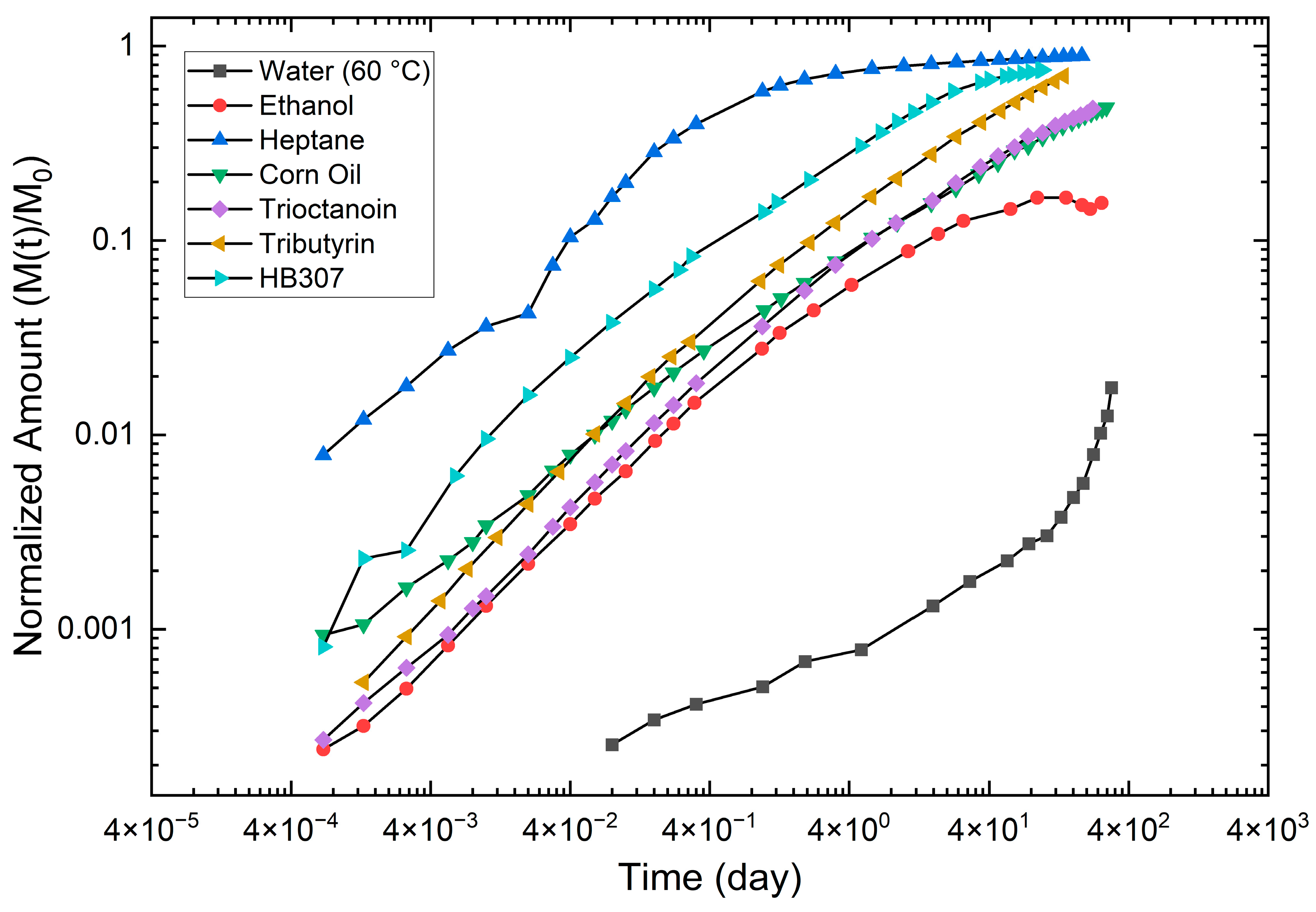
4.8.2. Time-Dependent LDPE Extractables Release Profiles
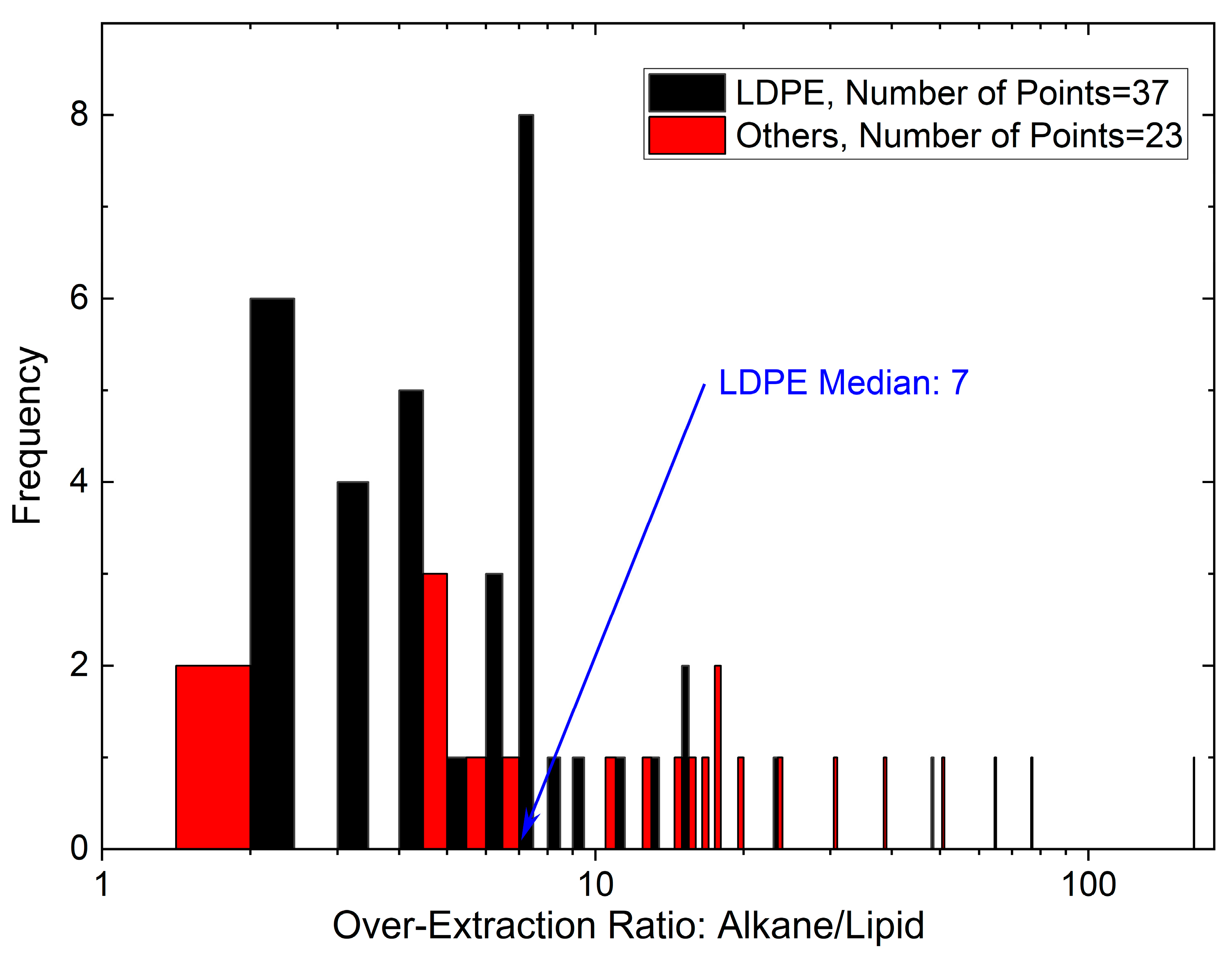
4.8.3. Over-Extraction by Alkanes
5. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Conflicts of Interest
References
- Reeve, L.; Baldrick, P. Biocompatibility assessments for medical devices—Evolving regulatory considerations. Expert Rev. Med. Devices 2017, 14, 161–167. [Google Scholar] [CrossRef] [PubMed]
- Cuadros-Rodríguez, L.; Lazúen-Muros, M.; Ruiz-Samblás, C.; Navas-Iglesias, N. Leachables from plastic materials in contact with drugs. State of the art and review of current analytical approaches. Int. J. Pharm. 2020, 583, 119332. [Google Scholar] [CrossRef] [PubMed]
- ISO10993-18:2020; Biological Evaluation of Medical Devices—Part 18: Chemical Characterization of Medical Device Materials within a Risk Management Process. Available online: https://www.iso.org/standard/64750.html (accessed on 27 November 2023).
- Kiradzhiyska, D.D.; Mantcheva, R.D. Overview of Biocompatible Materials and Their Use in Medicine. Folia Med. 2019, 61, 34–40. [Google Scholar] [CrossRef] [PubMed]
- Marturano, V.; Cerruti, P.; Ambrogi, V. Polymer additives. Phys. Sci. Rev. 2017, 2, 20160130. [Google Scholar] [CrossRef]
- Li, B.J. Exploring three-dimensional space of extractables and leachables in volatility, hydrophobicity, and molecular weight and assessment of roles of gas and liquid chromatographic methods in their comprehensive analysis. J. Pharm. Biomed. Anal. 2023, 223, 115142. [Google Scholar] [CrossRef] [PubMed]
- Geueke, B.; Groh, K.J.; Maffini, M.V.; Martin, O.V.; Boucher, J.M.; Chiang, Y.-T.; Gwosdz, F.; Jieh, P.; Kassotis, C.D.; Łańska, P.; et al. Systematic evidence on migrating and extractable food contact chemicals: Most chemicals detected in food contact materials are not listed for use. Crit. Rev. Food Sci. Nutr. 2022, 63, 9425–9435. [Google Scholar] [CrossRef] [PubMed]
- Finley, J.W.; deMan, J.M. Lipids. In Principles of Food Chemistry; Food Science Text Series; Springer: Cham, Switzerland, 2018. [Google Scholar] [CrossRef]
- Reis, A.; Rudnitskaya, A.; Blackburn, G.J.; Fauzi, N.M.; Pitt, A.R.; Spickett, C.M. A comparison of five lipid extraction solvent systems for lipidomic studies of human LDL. J. Lipid Res. 2013, 54, 1812–1824. [Google Scholar] [CrossRef] [PubMed]
- Muncke, J. Hazards of Food Contact Material: Food Packaging Contaminants. In Encyclopedia of Food Safety; Motarjemi, Y., Ed.; Academic Press: Cambridge, MA, USA, 2014; pp. 430–437. [Google Scholar] [CrossRef]
- Bhunia, K.; Sablani, S.S.; Tang, J.; Rasco, B. Migration of Chemical Compounds from Packaging Polymers during Microwave, Conventional Heat Treatment, and Storage. Compr. Rev. Food Sci. Food Saf. 2013, 12, 523–545. [Google Scholar] [CrossRef]
- Gavriil, G.; Kanavouras, A.; Coutelieris, F.A. Food-packaging migration models: A critical discussion. Crit. Rev. Food Sci. Nutr. 2018, 58, 2262–2272. [Google Scholar] [CrossRef]
- de Kruijf, N.; Rijk, R. The suitability of alternative fatty food simulants for overall migration testing under both low- and high-temperature test conditions. Food Addit. Contam. 1997, 14, 775–789. [Google Scholar] [CrossRef]
- Baner, A.; Bieber, W.; Figge, K.; Franz, R.; Piringer, O. Alternative fatty food simulants for migration testing of polymeric food contact materials. Food Addit. Contam. 1992, 9, 137–148. [Google Scholar] [CrossRef] [PubMed]
- Cooper, I.; Goodson, A.; O’Brien, A. Specific migration testing with alternative fatty food simulants. Food Addit. Contam. 1998, 15, 72–78. [Google Scholar] [CrossRef] [PubMed]
- De Kruijf, N.; Rijk, M.A.H. Iso-octane as fatty food simulant: Possibilities and limitations. Food Addit. Contam. 1988, 5, 467–483. [Google Scholar] [CrossRef]
- Guidance for Industry: Preparation of Premarket Submissions for Food Contact Substances (Chemistry Recommendations). Available online: https://www.fda.gov/regulatory-information/search-fda-guidance-documents/guidance-industry-preparation-premarket-submissions-food-contact-substances-chemistry (accessed on 27 November 2023).
- Fahy, E.; Subramaniam, S.; Brown, H.A.; Glass, C.K.; Merrill, A.H., Jr.; Murphy, R.C.; Raetz, C.R.H.; Russell, D.W.; Seyama, Y.; Shaw, W.; et al. A comprehensive classification system for lipids. J. Lipid Res. 2005, 46, 839–861. [Google Scholar] [CrossRef] [PubMed]
- ISO 10993-12:2021; Biological Evaluation of Medical Devices—Part 12: Sample Preparation and Reference Materials. Available online: https://www.iso.org/standard/75769.html (accessed on 7 January 2023).
- Chang, S.-S.; Pummer, W.J.; Maurey, J.R. Fat-simulating and accelerating solvents for polyolefins and MWD of solvent extracts of polyethylenes. Polymer 1983, 24, 1267–1272. [Google Scholar] [CrossRef]
- Limm, W.; Hollifield, H.C. Modelling of additive diffusion in polyolefins. Food Addit. Contam. 1996, 13, 949–967. [Google Scholar] [CrossRef] [PubMed]
- Koszinowski, J. Diffusion and solubility of n-alkanes in polyolefines. J. Appl. Polym. Sci. 1986, 32, 4765–4786. [Google Scholar] [CrossRef]
- Koszinowski, J. Diffusion and Solubility of Hydroxy Compounds in Polyolefines. J. Appl. Polym. Sci. 1986, 31, 2711–2720. [Google Scholar] [CrossRef]
- Poças, M.F.; Oliveira, J.C.; Oliveira, F.A.R.; Hogg, T. A critical survey of predictive mathematical models for migration from packaging. Crit. Rev. Food Sci. Nutr. 2008, 48, 913–928. [Google Scholar] [CrossRef] [PubMed]
- Wan, J.A.L.; Chatwin, P.C.; Katan, L.L. Migration from plastic packages into their contents. I. The role of mathematical models. Philos. Trans. R. Soc. Lond. Ser. A Phys. Eng. Sci. 1995, 350, 379–406. [Google Scholar] [CrossRef]
- Chatwin, P.C.; Katan, L.L. The role of mathematics and physics in migration predictions. Packag. Technol. Sci. Int. J. 1989, 2, 75–84. [Google Scholar] [CrossRef]
- Franz, R.; Stormer, A. Migration of Plastic Constituents. In Plastic Packaging—Interactions with Food and Pharmaceuticals, 2nd ed.; Piringer, O.G., Baner, A.L., Eds.; WILEY-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2008; Chapter 11; pp. 349–415. ISBN 978-3-527-31455-3. [Google Scholar]
- Franz, R. Migration of plastic constituents. In Plastic Packaging Materials for Food, Barrier Function, Mass Transport, Quality Assurance, and Legislation; Piringer, O.G., Baner, A.L., Eds.; WILEY-VCH: Weinheim, Germany, 2000; Chapter 10; pp. 287–357. [Google Scholar]
- Baner, A.L. Partition constant. In Plastic Packaging Materials for Food, Barrier Function, Mass Transport, Quality Assurance, and Legislation; Piringer, O.G., Baner, A.L., Eds.; WILEY-VCH: Weinheim, Germany, 2000; Chapter 4; pp. 78–123. [Google Scholar]
- Piringer, O.G.; Baner, A.L. Appendix II. In Plastic Packaging—Interactions with Food and Pharmaceuticals, 2nd ed.; WILEY-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2008; pp. 558–590. ISBN 978-3-527-31455-3. [Google Scholar]
- Piringer, O. Mathematical modeling of chemical migration from food contact materials. In Chemical Migration and Food Contact Materials; Barnes, K.A., Sinclair, C.R., Watson, D.H., Eds.; Woodhead: Cambridge, UK, 2007; pp. 180–202. [Google Scholar]
- Helmroth, E.; Rijk, R.; Dekker, M.; Jongen, W. Predictive modelling of migration from packaging materials into food products for regulatory purposes. Trends Food Sci. Technol. 2002, 13, 102–109. [Google Scholar] [CrossRef]
- Li, B.J. Correlation of Extractables Transfer from Low-Density Polyethylene into Extraction Solvents with Abraham Solvation Parameter Model and Assessment of Thermodynamic and Kinetic Effects in Achieving Exhaustive Extractions in Chemical Characterization of Medical Devices. J. Solut. Chem. 2023, 52, 967–1000. [Google Scholar] [CrossRef]
- Figge, K. Migration of additives from plastics films into edible oils and fat simulants. Food Cosmet. Toxicol. 1972, 10, 815–828. [Google Scholar] [CrossRef] [PubMed]
- Gramiccioni, L.; Di Prospero, P.; Milana, M.; Di Marzio, S.; Marcello, I. Global migration from plastic materials into olive oil and isooctane: An experimental comparison. Food Chem. Toxicol. 1986, 24, 23–26. [Google Scholar] [CrossRef] [PubMed]
- Garde, J.A.; Catalá, R.; Gavara, R.; Garde, R.C.J.A. Global and specific migration of antioxidants from polypropylene films into food simulants. J. Food Prot. 1998, 61, 1000–1006. [Google Scholar] [CrossRef] [PubMed]
- Hamdani, M.; Feigenbaum, A. Migration from plasticized poly(vinyl chloride) into fatty media: Importance of simulant selectivity for the choice of volatile fatty simulants. Food Addit. Contam. 1996, 13, 717–729. [Google Scholar] [CrossRef]
- Monteiro, M.; Nerín, C.; Reyes, F. Migration of Tinuvin P, a UV stabilizer, from PET bottles into fatty-food simulants. Packag. Technol. Sci. Int. J. 1999, 12, 241–248. [Google Scholar] [CrossRef]
- Reynier, A.; Dole, P.; Feigenbaum, A. Integrated approach of migration prediction using numerical modelling associated to experimental determination of key parameters. Food Addit. Contam. 2002, 19, 42–55. [Google Scholar] [CrossRef]
- Helmroth, I.E.; Dekker, M.; Hankemeier, T. Influence of solvent absorption on the migration of Irganox 1076 from LDPE. Food Addit. Contam. 2002, 19, 176–183. [Google Scholar] [CrossRef]
- Beldì, G.; Pastorelli, S.; Franchini, F.; Simoneau, C. Time- and temperature-dependent migration studies of Irganox 1076 from plastics into foods and food simulants. Food Addit. Contam. Part A Chem. Anal. Control Expo. Risk Assess. 2012, 29, 836–845. [Google Scholar] [CrossRef] [PubMed]
- Hernandez-Muñoz, P.; Catalá, R.; Gavara, R. Food aroma partition between packaging materials and fatty food simulants. Food Addit. Contam. 2001, 18, 673–682. [Google Scholar] [CrossRef] [PubMed]
- Paseiro-Cerrato, R.; Tongchat, C.; Franz, R. Study of the partition coefficients Kp/f of seven model migrants from LDPE polymer in contact with food simulants. Food Addit. Contam. Part A Chem. Anal. Control Expo. Risk Assess. 2016, 33, 885–892. [Google Scholar] [CrossRef] [PubMed]
- Seiler, A.; Bach, A.; Driffield, M.; Losada, P.P.; Mercea, P.; Tosa, V.; Franz, R. Correlation of foodstuffs with ethanol–water mixtures with regard to the solubility of migrants from food contact materials. Food Addit. Contam. Part A 2014, 31, 498–511. [Google Scholar] [CrossRef]
- Correlation of Partition Constants KPolymer/Food and KOctanol/Water for Potential Migrants in Food Contact Polymers. Available online: https://www.ivv.fraunhofer.de/content/dam/ivv/en/documents/Forschungsfelder/Produktsicherheit-und-analytik/Correlation_of_partition_coefficients.pdf (accessed on 27 November 2023).
- Migration of Low Molecular Weight Additives in Polyolefins and Copolymers, National Bureau of Standards (NBS), Final Project Report, NBSIR 82-2472. 1982. Available online: https://nvlpubs.nist.gov/nistpubs/Legacy/IR/nbsir82-2472.pdf (accessed on 27 November 2023).
- Smedes, F.; Rusina, T.P.; Beeltje, H.; Mayer, P. Partitioning of hydrophobic organic contaminants between polymer and lipids for two silicones and low density polyethylene. Chemosphere 2017, 186, 948–957. [Google Scholar] [CrossRef] [PubMed]
- Turner, P.; Elder, R.M.; Nahan, K.; Talley, A.; Shah, S.; Duncan, T.V.; Sussman, E.M.; Saylor, D.M. Leveraging Extraction Testing to Predict Patient Exposure to Polymeric Medical Device Leachables Using Physics-based Models. Toxicol. Sci. 2020, 178, 201–211. [Google Scholar] [CrossRef] [PubMed]
- Begley, T.; Castle, L.; Feigenbaum, A.; Franz, R.; Hinrichs, K.; Lickly, T.; Mercea, P.; Milana, M.; O’brien, A.; Rebre, S.; et al. Evaluation of migration models that might be used in support of regulations for food-contact plastics. Food Addit. Contam. 2005, 22, 73–90. [Google Scholar] [CrossRef] [PubMed]
- Jahnke, A.; McLachlan, M.S.; Mayer, P. Equilibrium sampling: Partitioning of organochlorine compounds from lipids into polydimethylsiloxane. Chemosphere 2008, 73, 1575–1581. [Google Scholar] [CrossRef]
- Geisler, A.; Endo, S.; Goss, K.-U. Partitioning of organic chemicals to storage lipids: Elucidating the dependence on fatty acid composition and temperature. Environ. Sci. Technol. 2012, 46, 9519–9524. [Google Scholar] [CrossRef]
- Rusina, T.P.; Smedes, F.; Klanova, J.; Booij, K.; Holoubek, I. Polymer selection for passive sampling: A comparison of critical properties. Chemosphere 2007, 68, 1344–1351. [Google Scholar] [CrossRef]
- Paxton, N.C.; Allenby, M.C.; Lewis, P.M.; Woodruff, M.A. Biomedical applications of polyethylene. Eur. Polym. J. 2019, 118, 412–428. [Google Scholar] [CrossRef]
- Lohmann, R. Critical Review of Low-Density Polyethylene’s Partitioning and Diffusion Coefficients for Trace Organic Contaminants and Implications for Its Use As a Passive Sampler. Environ. Sci. Technol. 2012, 46, 606–618. [Google Scholar] [CrossRef] [PubMed]
- Ulrich, N.; Böhme, A.; Strobel, A.B.; Egert, T. Predicting partitioning from low density polyethylene to blood and adipose tissue by linear solvation energy relationship models. J. Biomed. Mater. Res. B Appl. Biomater. 2023, 111, 2044–2054. [Google Scholar] [CrossRef] [PubMed]
- Egert, T.; Langowski, H.C. Linear solvation energy relationships (LSERs) for robust prediction of partition coefficients between low density polyethylene and water. Part II: Model evaluation and benchmarking. Eur. J. Pharm. Sci. 2022, 172, 106138. [Google Scholar] [CrossRef] [PubMed]
- Poole, C.F. Solvation parameter model: Tutorial on its application to separation systems for neutral compounds. J. Chromatogr. A 2021, 1645, 462108. [Google Scholar] [CrossRef] [PubMed]
- Poole, C.F. Partition constant database for totally organic biphasic systems. J. Chromatogr. A 2017, 1527, 18–32. [Google Scholar] [CrossRef]
- Abraham, M.H.; Acree, W.E., Jr. Descriptors for the Prediction of Partition Coefficients and Solubilities of Organophosphorus Compounds. Sep. Sci. Technol. 2013, 48, 884–897. [Google Scholar] [CrossRef]
- Jouyban, A.; Acree WMichael, H. Abraham and His Developed Parameters: Various Applications in Medicine, Chemistry and Biology. Pharm. Sci. 2022, 28, 170–173. [Google Scholar] [CrossRef]
- Khawar, M.I.; Nabi, D. Relook on the Linear Free Energy Relationships Describing the Partitioning Behavior of Diverse Chemicals for Polyethylene Water Passive Samplers. ACS Omega 2021, 6, 5221–5232. [Google Scholar] [CrossRef]
- Liu, Z.; Sun, X.; Xu, Y. Recalibrating polyparameter linear free energy relationships and reanalyzing mechanisms for partition of nonionic organic compounds to low-density polyethylene passive sampler. J. Chromatogr. A 2023, 1700, 464039. [Google Scholar] [CrossRef]
- Abraham, M.H.; Chadha, H.S.; Whiting, G.S.; Mitchell, R.C. Hydrogen bonding. 32. An analysis of water-octanol and water-alkane partitioning and the Δlog P parameter of seiler. J. Pharm. Sci. 1994, 83, 1085–1100. [Google Scholar] [CrossRef] [PubMed]
- Lickly, T.D.; Bell, C.D.; Lehr, K.M. The migration of Irganox 1010 antioxidant from high-density polyethylene and polypropylene into a series of potential fatty-food simulants. Food Addit. Contam. 1990, 7, 805–814. [Google Scholar] [CrossRef] [PubMed]
- Garde, J.A.; Catalá, R.; Gavara, R.; Hernandez, R.J. Characterizing the migration of antioxidants from polypropylene into fatty food simulants. Food Addit. Contam. 2001, 18, 750–762. [Google Scholar] [CrossRef] [PubMed]
- Li, B. Determination of the mass transport properties of chemical additives in polypropylene material/simulated food system. Food Addit. Contam. Part A 2019, 36, 625–637. [Google Scholar] [CrossRef] [PubMed]
- Zhu, T.; Jafvert, C.T.; Fu, D.; Hu, Y. A novel method for measuring polymer–water partition coefficients. Chemosphere 2015, 138, 973–979. [Google Scholar] [CrossRef] [PubMed]
- Booij, K.; Hofmans, H.E.; Fischer, C.V.; Van Weerlee, E.M. Temperature-dependent uptake rates of nonpolar organic compounds by semipermeable membrane devices and low-density polyethylene membranes. Environ. Sci. Technol. 2003, 37, 361–366. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Fahr, A.; Abraham, M.H.; Acree, W.E., Jr.; Tobin, D.J.; Liu, X. Comparison of lipid membrane–water partitioning with various organic solvent–water partitions of neutral species and ionic species: Uniqueness of cerasome as a model for the stratum corneum in partition processes. Int. J. Pharm. 2015, 494, 1–8. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Cao, Y.; Marra, M.; Anderson, B.D. Predictive relationships for the effects of triglyceride ester concentration and water uptake on solubility and partitioning of small molecules into lipid vehicles. J. Pharm. Sci. 2004, 93, 2768–2779. [Google Scholar] [CrossRef]
- Allan, I.J.; Vrana, B.; Ruus, A. Passive Sampling Helps the Appraisal of Contaminant Bioaccumulation in Norwegian Fish Used for Regulatory Chemical Monitoring. Environ. Sci. Technol. 2022, 56, 7945–7953. [Google Scholar] [CrossRef]
- Gargas, M.L.; Burgess, R.J.; Voisard, D.E.; Cason, G.H.; Andersen, M.E. Partition coefficients of low-molecular-weight volatile chemicals in various liquids and tissues. Toxicol. Appl. Pharmacol. 1989, 98, 87–99. [Google Scholar] [CrossRef]
- Ruus, A.; Allan, I.J.; Bæk, K.; Borgå, K. Partitioning of persistent hydrophobic contaminants to different storage lipid classes. Chemosphere 2021, 263, 127890. [Google Scholar] [CrossRef] [PubMed]
- Alan, D. Hendricker, Controlled Extraction Study on Low Density Polyethylene (LDPE). 2015. Available online: https://pqri.org/wp-content/uploads/2015/11/LDPE_Poster.pdf (accessed on 27 November 2023).
- Cozzi, A.C.; Briasco, B.; Salvarani, E.; Mannucci, B.; Fangarezzi, F.; Perugini, P. Evaluation of Mechanical Properties and Volatile Organic Extractable to Investigate LLDPE and LDPE Polymers on Final Packaging for Semisolid Formulation. Pharmaceutics 2018, 10, 113. [Google Scholar] [CrossRef] [PubMed]
- Jenke, D.; Castner, J.; Egert, T.; Feinberg, T.; Hendricker, A.; Houston, C.; Hunt, D.G.; Lynch, M.; Shaw, A.; Nicholas, K.; et al. Extractables characterization for five materials of construction representative of packaging systems used for parenteral and ophthalmic drug products. PDA J. Pharm. Sci. Technol. 2013, 67, 448–511. [Google Scholar] [CrossRef] [PubMed]
- Vera, P.; Canellas, E.; Barknowitz, G.; Goshawk, J.; Nerín, C. Ion-Mobility Quadrupole Time-of-Flight Mass Spectrometry: A Novel Technique Applied to Migration of Nonintentionally Added Substances from Polyethylene Films Intended for Use as Food Packaging. Anal. Chem. 2019, 91, 12741–12751. [Google Scholar] [CrossRef] [PubMed]
- Schwope, A.; Till, D.; Ehntholt, D.; Sidman, K.; Whelan, R.; Schwartz, P.; Reid, R. Migration of BHT and Irganox 1010 from low-density polyethylene (LDPE) to foods and food-simulating liquids. Food Chem. Toxicol. 1987, 25, 317–326. [Google Scholar] [CrossRef]
- Chapke, K.; Gandhi, K.; Lata, K.; Sharma, R.; Mann, B.; Singh, N. Migration study of chemical additives from low density polyethylene (LDPE) into dahi. J. Food Sci. Technol. 2022, 59, 3283–3295. [Google Scholar] [CrossRef] [PubMed]
- Wessling, C.; Nielsen, T.; Leufvén, A.; Jägerstad, M. Mobility of α-tocopherol and BHT in LDPE in contact with fatty food simulants. Food Addit. Contam. 1998, 15, 709–715. [Google Scholar] [CrossRef] [PubMed]
- RMG: Group Contribution Method for Abraham Solute Parameter Prediction. Available online: https://rmg.mit.edu/database/solvation/search/ (accessed on 27 November 2023).
- Poole, C.F. Wayne State University experimental descriptor database for use with the solvation parameter model. J. Chromatogr. A 2020, 1617, 460841. [Google Scholar] [CrossRef]
- Li, J.; Sobańtka, A. A Systematic Analysis of the Effect of Extraction Solvents on the Chemical Composition of Extraction Solutions and the Analytical Implications in Extractables and Leachables Studies. J. Pharm. Biomed. Anal. 2023, 222, 115081. [Google Scholar] [CrossRef]
- Igwe, I.O. Uptake of aromatic solvents by polyethylene films. J. Appl. Polym. Sci. 2007, 104, 3849–3854. [Google Scholar] [CrossRef]
- Riquet, A.-M.; Scholler, D.; Feigenbaum, A. Tailoring fatty food simulants made from solvent mixtures (2): Determining the equivalent migration behaviour of olive oil and of solvents in the case of polyolefins. Food Addit. Contam. 2002, 19, 582–593. [Google Scholar] [CrossRef] [PubMed]
- Fava, L.P.P. Study of diffusion through LDPE film of Di-n-butyl phthalate. Food Addit. Contam. 1999, 16, 353–359. [Google Scholar] [CrossRef] [PubMed]
- Hedenqvist, M.; Gedde, U. Parameters affecting the determination of transport kinetics data in highly swelling polymers above Tg. Polymer 1999, 40, 2381–2393. [Google Scholar] [CrossRef]
- Harding, S.G.; Johns, M.L.; Pugh, S.R.; Fryer, P.J.; Gladden, L.F. Magnetic resonance imaging studies of diffusion in polymers. Food Addit. Contam. 1997, 14, 583–589. [Google Scholar] [CrossRef] [PubMed]
- Riquet, A.M.; Wolff, N.; Laoubi, S.; Vergnaud, J.M.; Feigenbaum, A. Food and packaging interactions: Determination of the kinetic parameters of olive oil diffusion in polypropylene using concentration profiles. Food Addit. Contam. 1998, 15, 690–700. [Google Scholar] [CrossRef] [PubMed]
- Nasiri, A.; Peyron, S.; Gastaldi, E.; Gontard, N. Effect of nanoclay on the transfer properties of immanent additives in food packages. J. Mater. Sci. 2016, 51, 9732–9748. [Google Scholar] [CrossRef]
- Rusina, T.P.; Smedes, F.; Klanova, J. Diffusion Coefficients of Polychlorinated Biphenyls and Polycyclic Aromatic Hydrocarbons in Polydimethylsiloxane and Low-Density Polyethylene Polymers. J. Appl. Polym. Sci. 2010, 116, 1803–1810. [Google Scholar] [CrossRef]
- Randová, A.; Bartovská, L.; Hovorka, Š.; Bartovský, T.; Izák, P.; Poloncarzová, M.; Fries, K. Diffusion coefficients in systems LDPE+cyclohexane and LDPE+benzene. e-Polymers 2010, 10, 742–747. [Google Scholar] [CrossRef][Green Version]
- Mauricio-Iglesias, M.; Guillard, V.; Gontard, N.; Peyron, S. Application of FTIR and Raman microspectroscopy to the study of food/packaging interactions. Food Addit. Contam. Part A Chem. Anal. Control Expo. Risk Assess. 2009, 26, 1515–1523. [Google Scholar] [CrossRef]
- Helmroth, I.E.; Dekker, M.; Hankemeier, T. Additive Diffusion from LDPE Slabs into Contacting Solvents as a Function of Solvent Absorption. J. Appl. Polym. Sci. 2003, 90, 1609–1617. [Google Scholar] [CrossRef]
- Ibarra, V.G.; Sendón, R.; García-Fonte, X.; Losada, P.P.; de Quirós, A.R.B. Migration studies of butylated hydroxytoluene, tributyl acetylcitrate and dibutyl phthalate into food simulants. J. Sci. Food Agric. 2019, 99, 1586–1595. [Google Scholar] [CrossRef] [PubMed]
- de Kruijf, N.; Rijk, M.; Soetardhi, L.; Rossi, L. Selection and application of a new volatile solvent as a fatty food simulant for determining the global migration of constituents of plastics materials. Food Chem. Toxicol. 1983, 21, 187–191. [Google Scholar] [CrossRef] [PubMed]
- Hernández-Muñoz, P.; Catalá, R.; Gavara, R. Simple method for the selection of the appropriate food simulant for the evaluation of a specific food/packaging interaction. Food Addit. Contam. 2002, 19, 192–200. [Google Scholar] [CrossRef] [PubMed]
- Kirchkeszner, C.; Petrovics, N.; Tábi, T.; Magyar, N.; Kovács, J.; Szabó, B.S.; Nyiri, Z.; Eke, Z. Swelling as a promoter of migration of plastic additives in the interaction of fatty food simulants with polylactic acid- and polypropylene-based plastics. Food Control 2022, 132, 108354. [Google Scholar] [CrossRef]
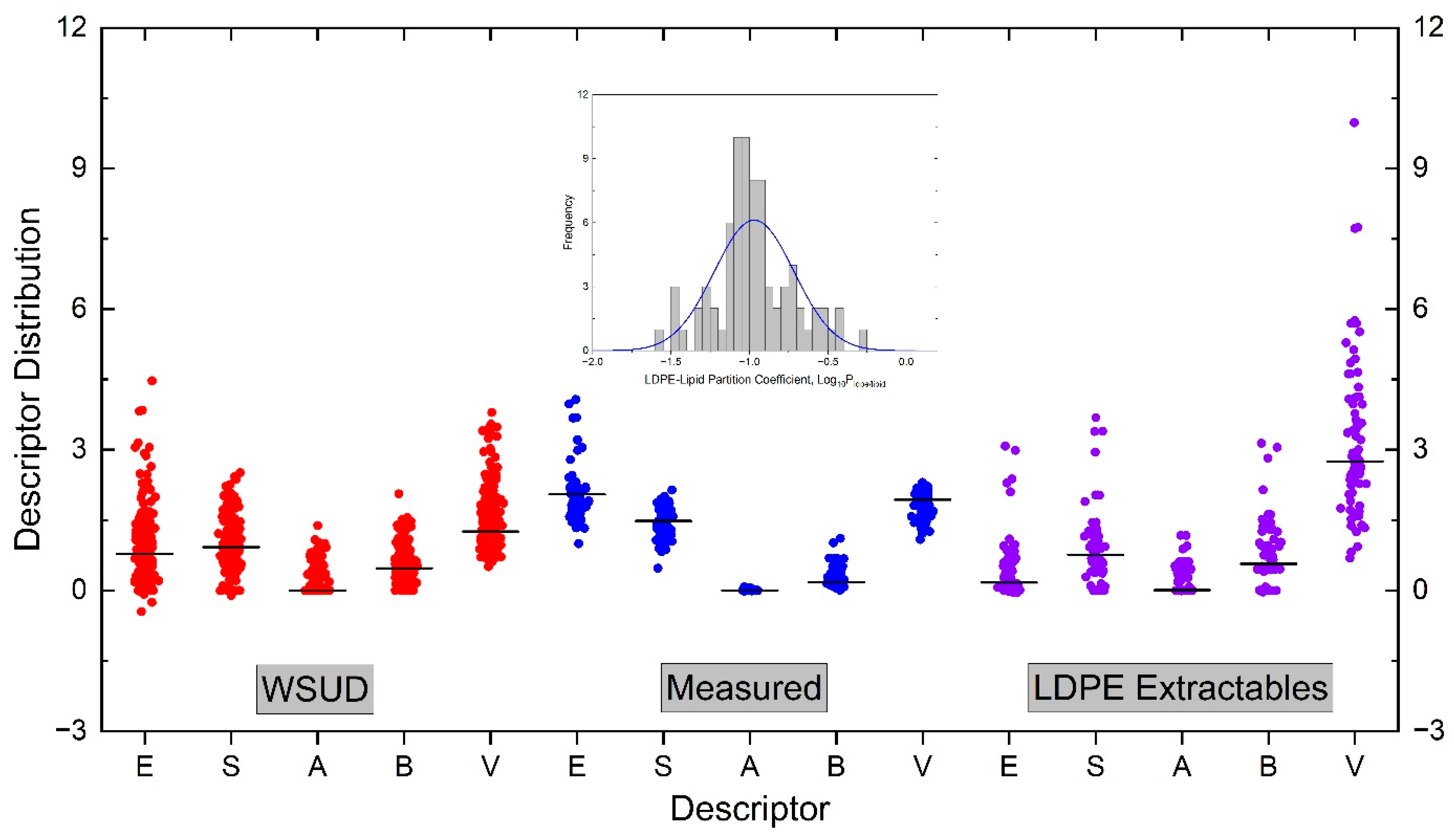


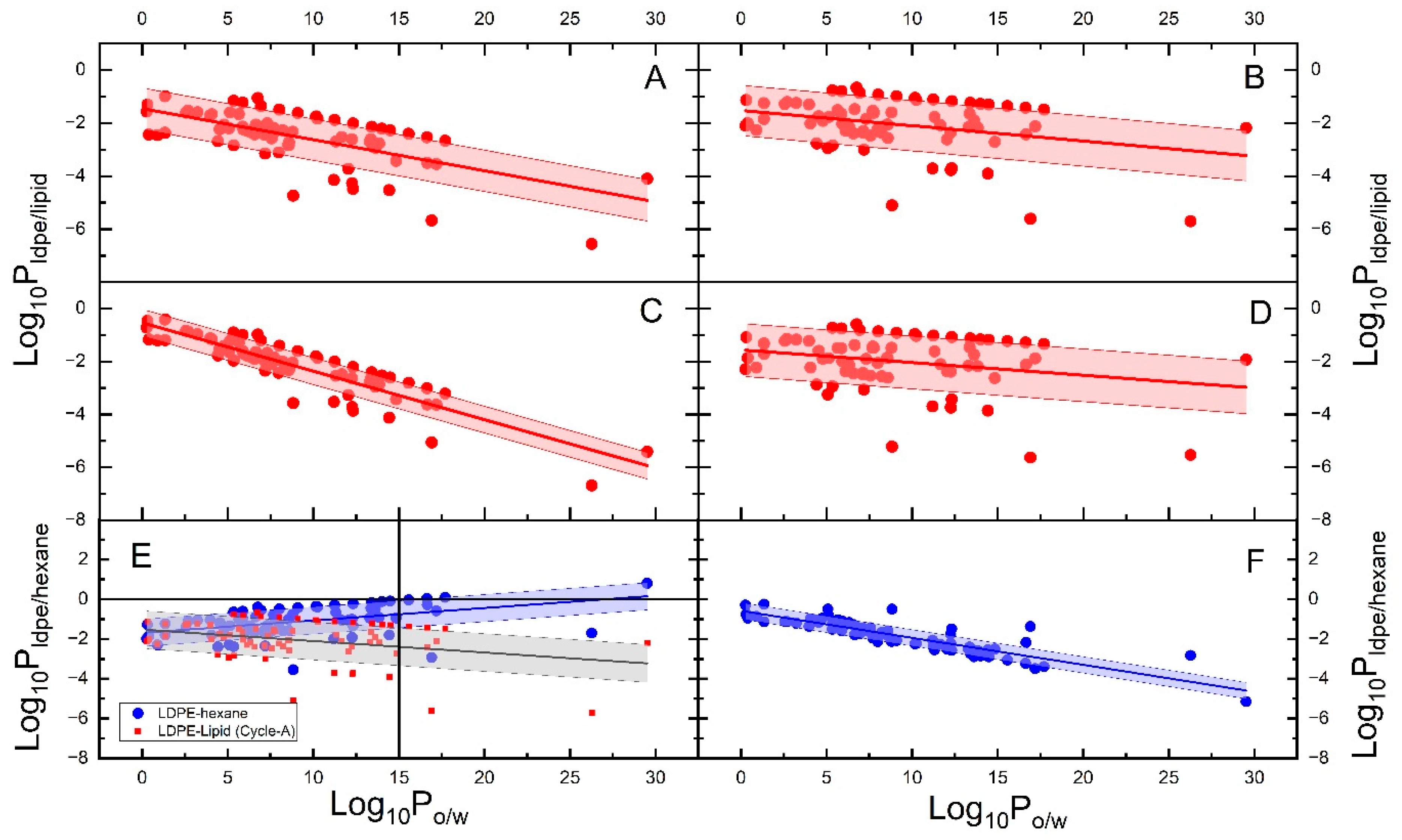

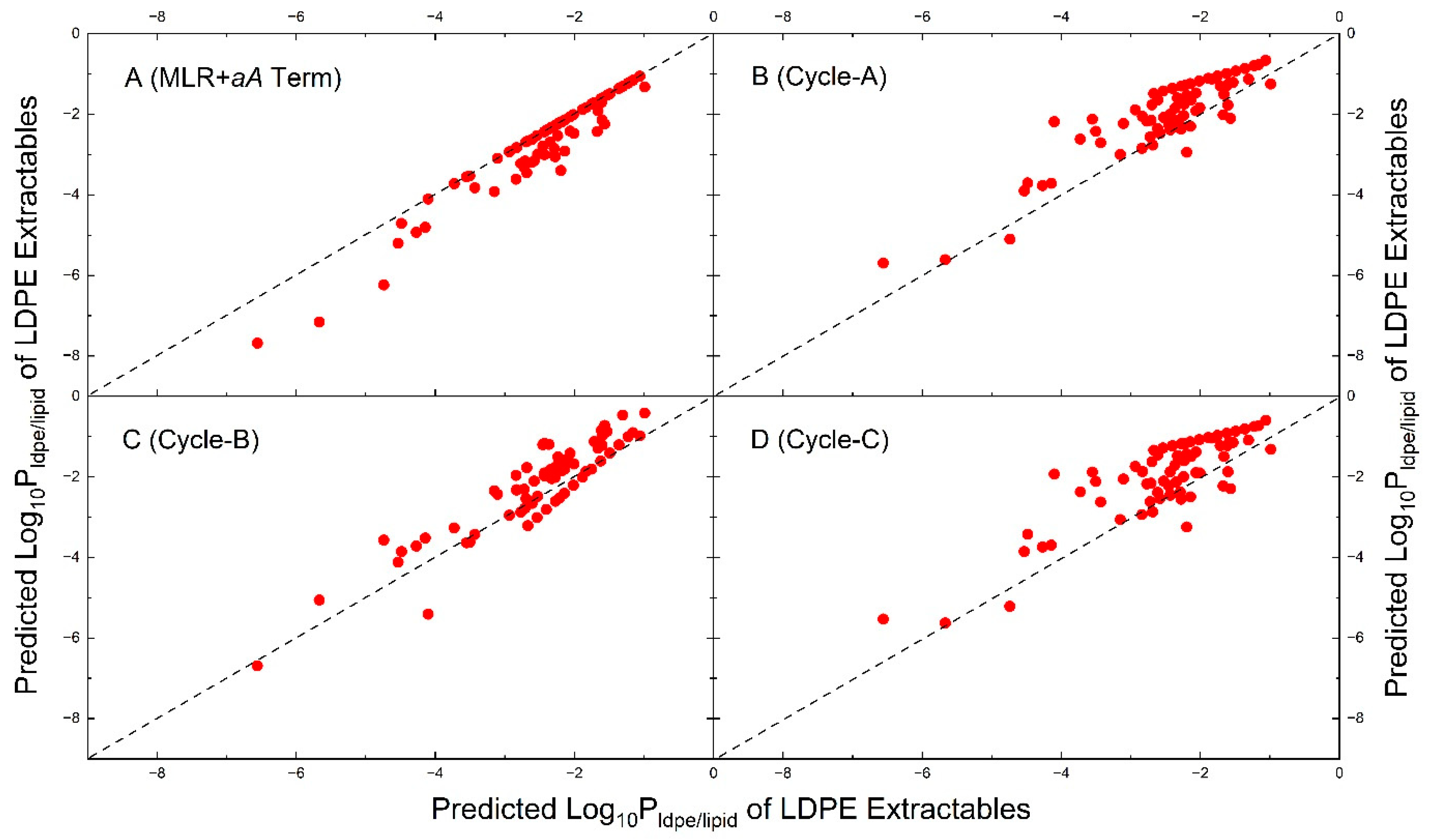
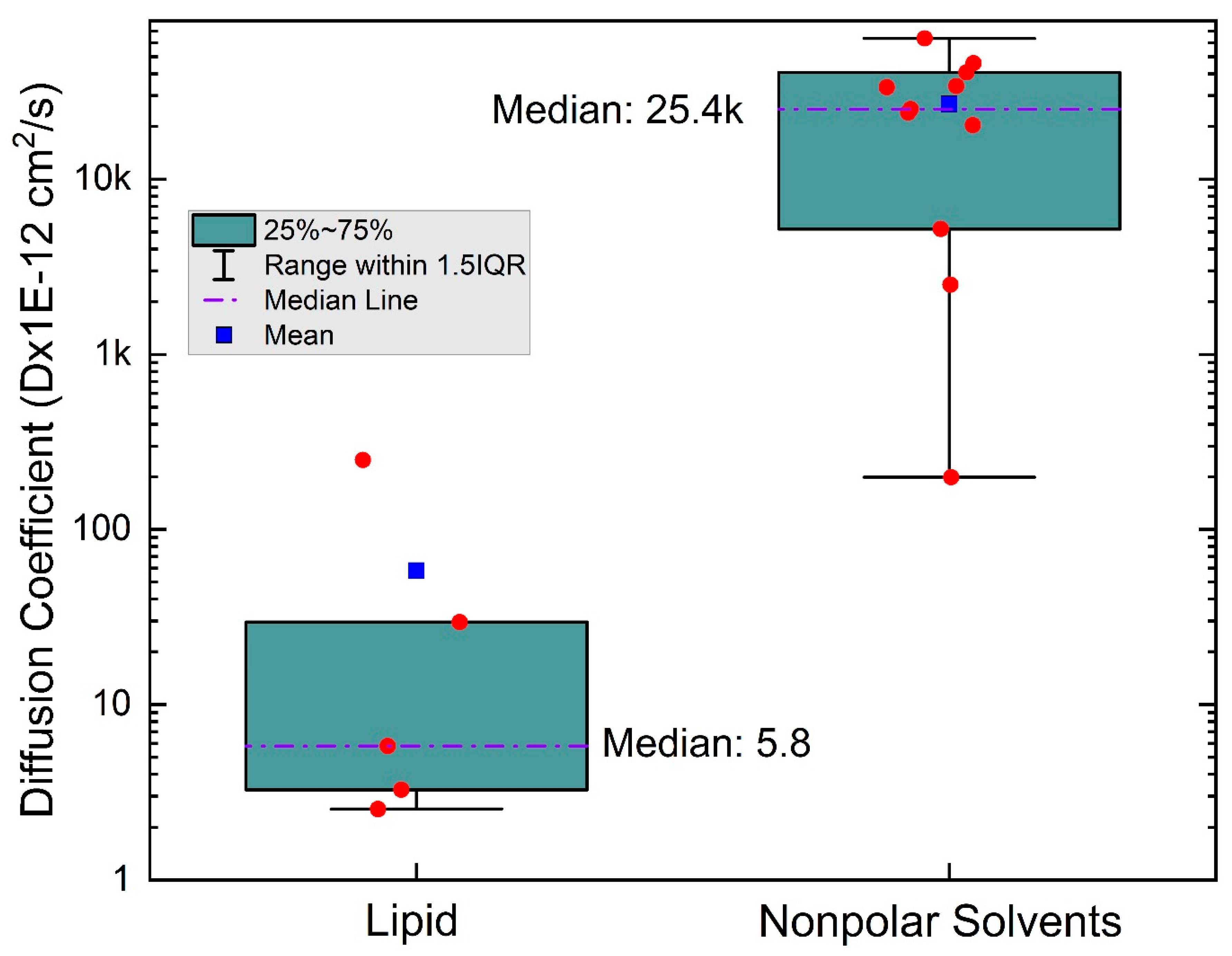
| Method | Partition System | Solvation Model System Constants a | Descriptive Statistics b | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| System Label c | System Description d | c | e | s | a | b | v | R | SE | F | Range | SE/Range (%) | AR (AAR) | |
| MLR by Equation (1) (Section 2.2 and Section 3.5) | A e | LDPE-Lipid (4–20 °C) | −0.529 (0.089) | 0.526 (0.043) | −0.368 (0.095) | 1.475 h (1.089) | −0.640 (0.082) | −0.455 (0.073) | 0.893 | 0.118 | 57 | 1.265 (−1.55 to −0.287) | 9.4 | 0.00 (0.08) |
| B f (MLR) | LDPE-Lipid (4–20 °C) | −0.524 (0.089) | 0.506 (0.041) | −0.329 (0.091) | NA | −0.619 (0.081) | −0.465 (0.073) | 0.890 | 0.119 | 69 | 9.4 | 0.00 (0.08) | ||
| C g | LDPE-Lipid (4–20 °C) | −0.997 (0.151) | 0.005 h (0.026) | 0.021 | 0.256 | 0.04 | 20.2 | 0.00 (0.19) | ||||||
| Compilation from Literatures and Thermodynamic Cycle Conversion (Section 2.2 and Section 3.5) | D i | LDPE-Water (25 °C) | −0.529 (0.077) | 1.098 (0.047) | −1.557 (0.081) | −2.991 (0.116) | −4.617 (0.110) | 3.886 (0.058) | ||||||
| E j | LDPE-Water (20–25 °C) | 1.002 (0.075) | −1.296 (0.128) | −1.820 (0.472) | −4.037 (0.153) | 3.399 (0.072) | ||||||||
| F k | LDPE-Water (20–25 °C) | −0.54 (0.05) | 1.13 (0.03) | −1.58 (0.06) | −3.47 (0.08) | −4.53 (0.07) | 3.92 (0.04) | |||||||
| G l | Lipid-Water (37 °C) | −0.07 (0.07) | 0.7 (0.06) | −1.08 (0.08) | −1.72 (0.13) | −4.14 (0.09) | 4.11 (0.06) | |||||||
| H m | Hexane-Water (w/d, 25 °C) | 0.333 | 0.56 | −1.71 | −3.578 | −4.939 | 4.463 | |||||||
| I n (Cycle-A) | LDPE-lipid (25–37 °C) | −0.459 (0.104) | 0.398 (0.076) | −0.477 (0.114) | −1.271 (0.174) | −0.477 (0.142) | −0.224 (0.084) | |||||||
| J o (Cycle-B) | LDPE-lipid (20–37 °C) | 0.070 (0.07) | 0.302 (0.096) | −0.216 (0.151) | −0.100 (0.490) | 0.103 (0.178) | −0.711 (0.094) | |||||||
| K p (Cycle-C) | LDPE-lipid (20–37 °C) | −0.470 (0.086) | 0.430 (0.067) | −0.500 (0.100) | −1.750 (0.153) | −0.390 (0.114) | −0.190 (0.072) | |||||||
| L q (Equation (3)) | LDPE-Hexane (23 °C) | −1.104 s (0.153) | 0.329 (0.109) | −0.033 (0.136) | −1.315 (0.242) | −0.977 (0.176) | 0.229 (0.087) | |||||||
| M r | LDPE-Hexane (25–37 °C) | −0.862 s | 0.538 | 0.153 | 0.587 | 0.322 | −0.577 | |||||||
| Partition System a | Regression Results b | Descriptive Statistics c | |||||
|---|---|---|---|---|---|---|---|
| Intercept | Slope | R | Adj. R | SE | F | n | |
| LDPE-hexane (L) | −1.685 (0.152) | 0.062 (0.014) | 0.452 | 0.193 | 0.682 | 19 | 76 |
| LDPE-hexane d (M) | −0.576 (0.092) | −0.136 (0.009) | 0.878 | 0.768 | 0.411 | 249 | 76 |
| LDPE-lipid (B) | −1.453 (0.174) | −0.117 (0.016) | 0.640 | 0.402 | 0.779 | 51 | 76 |
| LDPE-lipid (I) | −1.521 (0.211) | −0.058 (0.020) | 0.319 | 0.090 | 0.949 | 8 | 76 |
| LDPE-lipid (J) | −0.537 (0.113) | −0.183 (0.011) | 0.895 | 0.798 | 0.506 | 297 | 76 |
| LDPE-lipid (K) | −1.561 (0.222) | −0.048 (0.021) | 0.258 | 0.054 | 0.995 | 5 | 76 |
| (Equation (4), B) | −0.231 (0.080) | 0.179 (0.008) | 0.941 | 0.884 | 0.357 | 573 | 76 |
| (Equation (4), I) | −0.164 (0.072) | 0.120 (0.007) | 0.899 | 0.806 | 0.323 | 313 | 76 |
| (Equation (4), J) | −1.148 (0.071) | 0.245 (0.007) | 0.974 | 0.947 | 0.319 | 1345 | 76 |
| (Equation (4), K) | −0.124 (0.077) | 0.110 (0.007) | 0.870 | 0.754 | 0.346 | 230 | 76 |
| LDPE-Hexane a | LDPE-Lipid b (B) | LDPE-Lipid c (I) | LDPE-Lipid d (K) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Fit | Fit + SE | Fit − SE | Fit | Fit + SE | Fit | Fit + SE | Fit | Fit + SE | |
| 0 | 0.02 | 0.10 | 0.004 | 0.0354 | 0.2122 | 0.0302 | 0.268 | 0.0276 | 0.272 |
| 2.5 | 0.03 | 0.14 | 0.006 | 0.0180 | 0.1083 | 0.0217 | 0.192 | 0.0209 | 0.206 |
| 5 | 0.04 | 0.20 | 0.009 | 0.0092 | 0.0553 | 0.0155 | 0.138 | 0.0159 | 0.157 |
| 7.5 | 0.06 | 0.29 | 0.013 | 0.0047 | 0.0282 | 0.0111 | 0.099 | 0.0121 | 0.119 |
| 10 | 0.09 | 0.41 | 0.018 | 0.0024 | 0.0144 | 0.0080 | 0.071 | 0.0091 | 0.090 |
| 12.5 | 0.12 | 0.59 | 0.026 | 0.0012 | 0.0073 | 0.0057 | 0.051 | 0.0069 | 0.068 |
| 15 | 0.18 | 0.85 | 0.037 | 0.0006 | 0.0037 | 0.0041 | 0.036 | 0.0053 | 0.052 |
| 17.5 | 0.25 | 1.21 | 0.052 | 0.0003 | 0.0019 | 0.0029 | 0.026 | 0.0040 | 0.039 |
| 20 | 0.36 | 1.72 | 0.075 | 0.0002 | 0.0010 | 0.0021 | 0.019 | 0.0030 | 0.030 |
| 22.5 | 0.51 | 2.46 | 0.107 | 0.0001 | 0.0005 | 0.0015 | 0.013 | 0.0023 | 0.023 |
| 25 | 0.73 | 3.52 | 0.153 | 0.00004 | 0.0003 | 0.0011 | 0.010 | 0.0017 | 0.017 |
| 27.5 | 1.05 | 5.03 | 0.218 | 0.00002 | 0.0001 | 0.0008 | 0.007 | 0.0013 | 0.013 |
| 30 | 1.50 | 7.18 | 0.312 | 0.00001 | 0.0001 | 0.0006 | 0.005 | 0.0010 | 0.010 |
| Material | Solvent | Temperature (°C) | Solvent Absorption (w/w%) a | Diffusion Coefficient (D × 10−12 cm2/s) |
|---|---|---|---|---|
| LDPE | Fish Oil | 20 | 1.55 | 2.53 |
| 47 | 3.26 | |||
| Triolein | 20 | 2.58 | 5.79 | |
| 47 | 29.6 | |||
| Olive oil | 40 | 1.6–2 | 250–300 | |
| Olive oil b | 40 | <2 (~1.5) | ||
| Ethanol b | ~0.2 | |||
| Isopropanol b | <0.4 | |||
| Ethyl acetate b | 3 | |||
| Isooctane b | 15 | |||
| Cyclohexane b | 25 | |||
| Tributyrin b | <1 | |||
| Tricaprylin b | <2 | |||
| Cyclohexane | 40 | 29 | 34,000 | |
| Isooctane | 12 | 5200 | ||
| n-Heptane | 14 | 24,000 | ||
| Isooctane | 25 | 199 | ||
| Ethanol d | 25 | 8.66 | ||
| Olive oil | 121 | 10 | ||
| Isooctane | 40 | 4 | ||
| 60 | 10 | |||
| 80 | 19 | |||
| Cyclohexane | 10 | 10.88 | 20,300 | |
| 15 | 12.67 | 25,100 | ||
| 25 | 18.14 | 33,400 | ||
| Benzene | 10 | 9.09 | 40,600 | |
| 15 | 10.74 | 45,900 | ||
| 25 | 13.07 | 63,600 | ||
| n-Hexane | 25 | 11 | 2500 | |
| n-Heptane | NA | 5–15 | ||
| Ethanol d | <1 | |||
| Corn oil or Triglycerides | 2–5 | |||
| LLDPE c | Olive oil | 40 | 1.3 | 690 |
| Isooctane | 40 | 10.65 | ||
| 95% Ethanol d | 40 | 0.45 | ||
| PP c | Olive oil | 40 | ~2% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J. Evaluation of Thermodynamic and Kinetic Contributions to Over-Extraction of Extractables by Nonpolar Organic Solvents in Comparison to Lipids in Exhaustive and Exaggerated Extractions of Medical Devices Based on Abraham Solvation Model and Solvent–Material Interactions Using Low-Density Polyethylene as a Representative Material. Liquids 2024, 4, 117-147. https://doi.org/10.3390/liquids4010006
Li J. Evaluation of Thermodynamic and Kinetic Contributions to Over-Extraction of Extractables by Nonpolar Organic Solvents in Comparison to Lipids in Exhaustive and Exaggerated Extractions of Medical Devices Based on Abraham Solvation Model and Solvent–Material Interactions Using Low-Density Polyethylene as a Representative Material. Liquids. 2024; 4(1):117-147. https://doi.org/10.3390/liquids4010006
Chicago/Turabian StyleLi, Jianwei. 2024. "Evaluation of Thermodynamic and Kinetic Contributions to Over-Extraction of Extractables by Nonpolar Organic Solvents in Comparison to Lipids in Exhaustive and Exaggerated Extractions of Medical Devices Based on Abraham Solvation Model and Solvent–Material Interactions Using Low-Density Polyethylene as a Representative Material" Liquids 4, no. 1: 117-147. https://doi.org/10.3390/liquids4010006
APA StyleLi, J. (2024). Evaluation of Thermodynamic and Kinetic Contributions to Over-Extraction of Extractables by Nonpolar Organic Solvents in Comparison to Lipids in Exhaustive and Exaggerated Extractions of Medical Devices Based on Abraham Solvation Model and Solvent–Material Interactions Using Low-Density Polyethylene as a Representative Material. Liquids, 4(1), 117-147. https://doi.org/10.3390/liquids4010006








