Partial Denaturation of Double-Stranded DNA on Pristine Graphene under Physiological-like Conditions
Abstract
1. Introduction
2. Models and Methodology
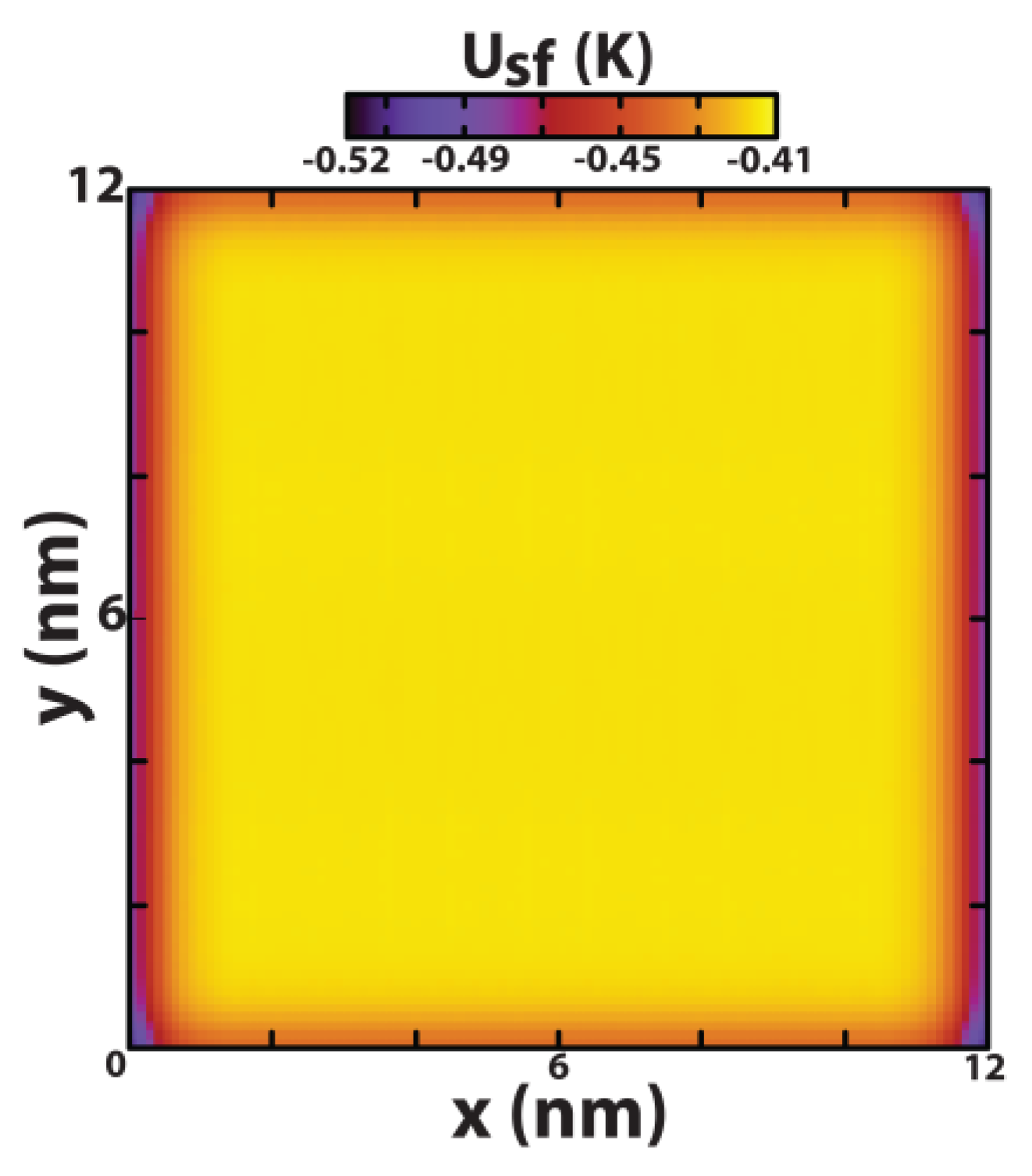
2.1. Molecular Models and System Setup
2.2. Classical Molecular Dynamics (CMD) and Well-Tempered Metadynamics
3. Results
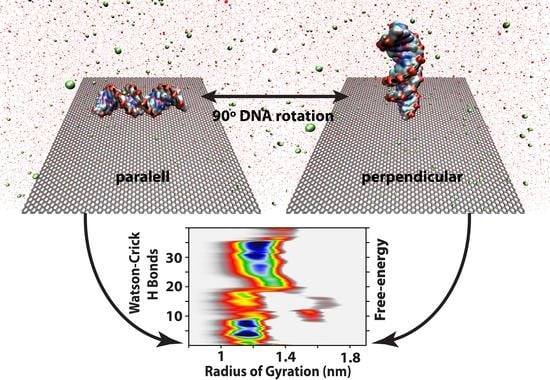
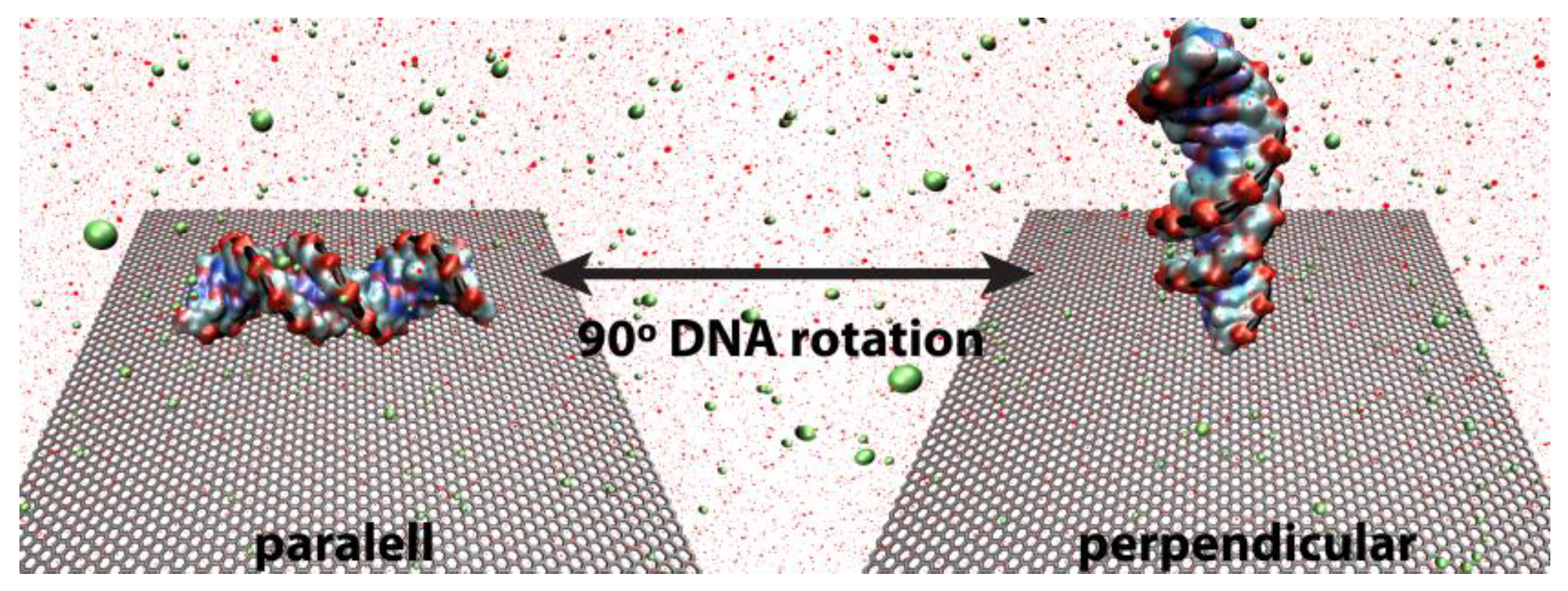
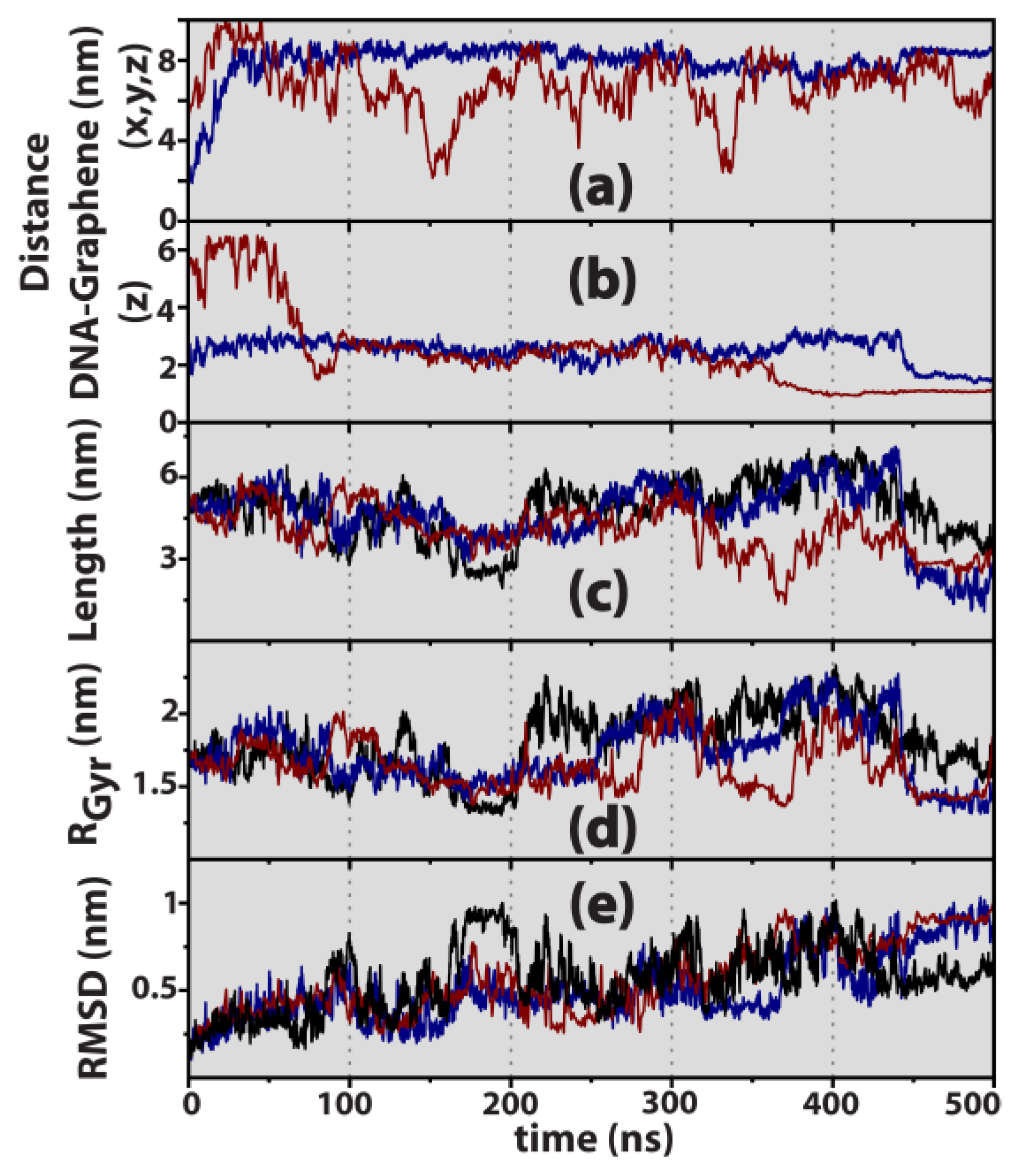
3.1. Structural and Geometrical Features
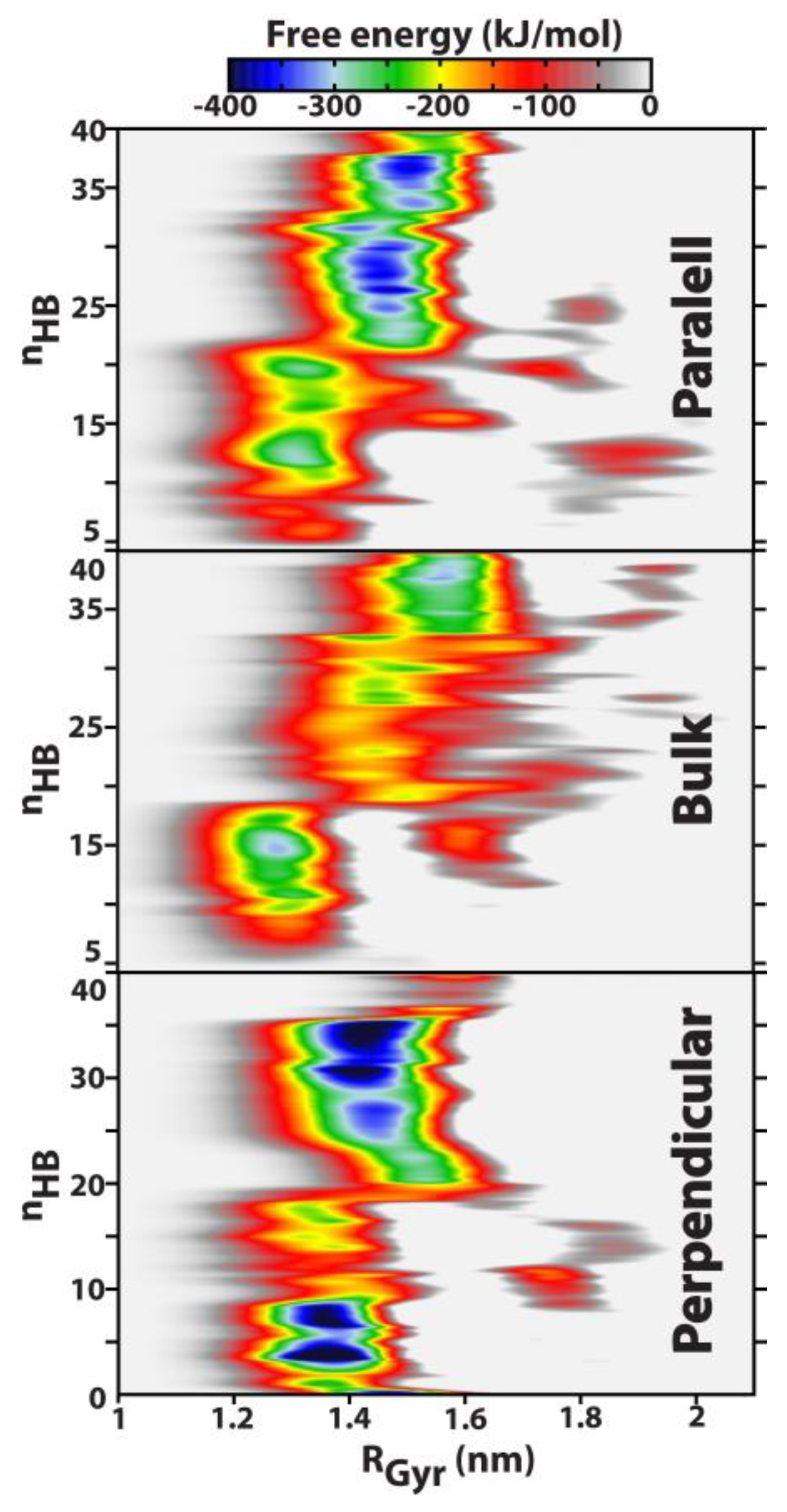
3.2. Molecular Thermodynamics
3.3. Canonical Watson–Crick H-Bonds
3.4. Intrastrand Hybridization
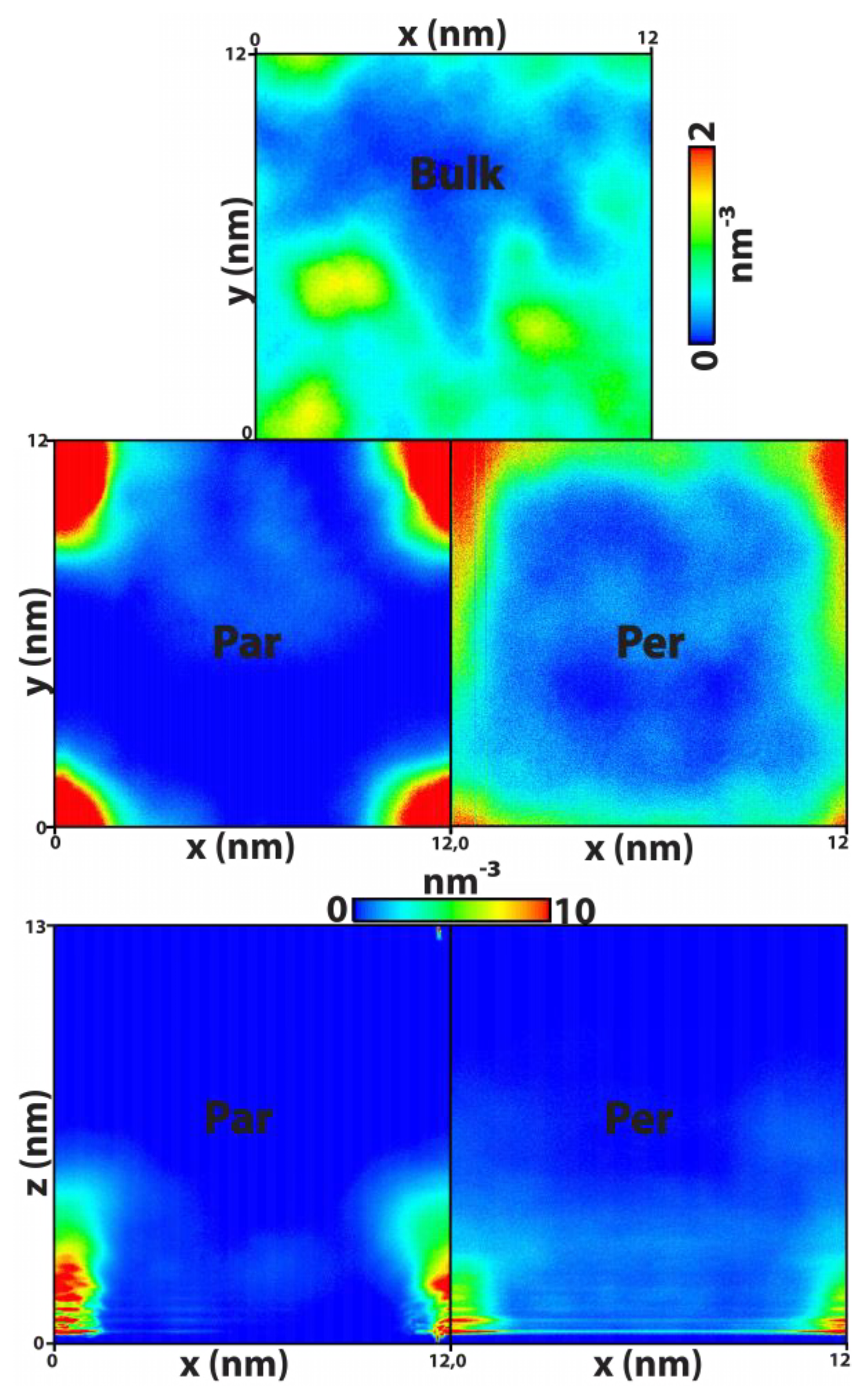
3.5. Molecular Density Maps
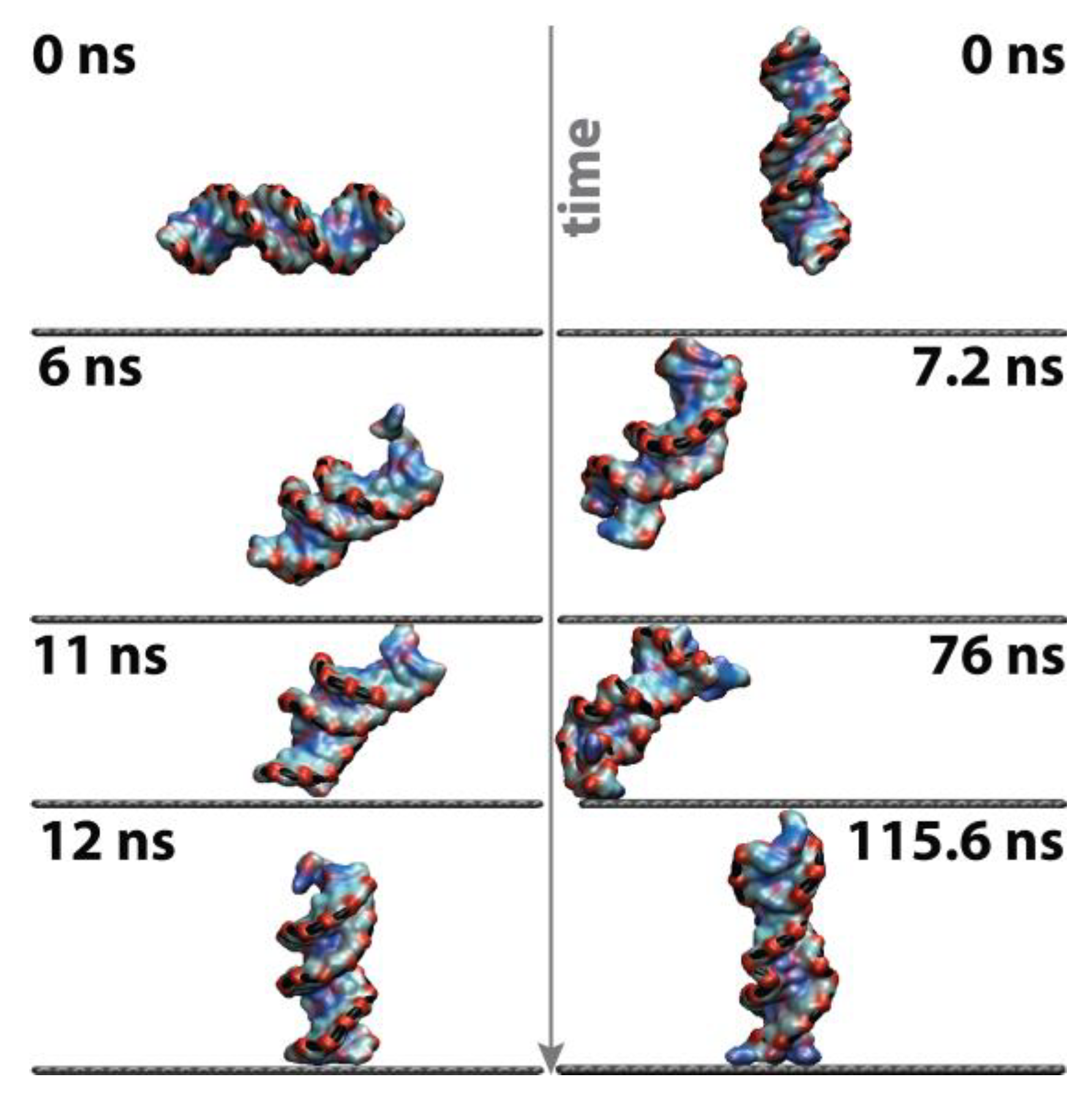
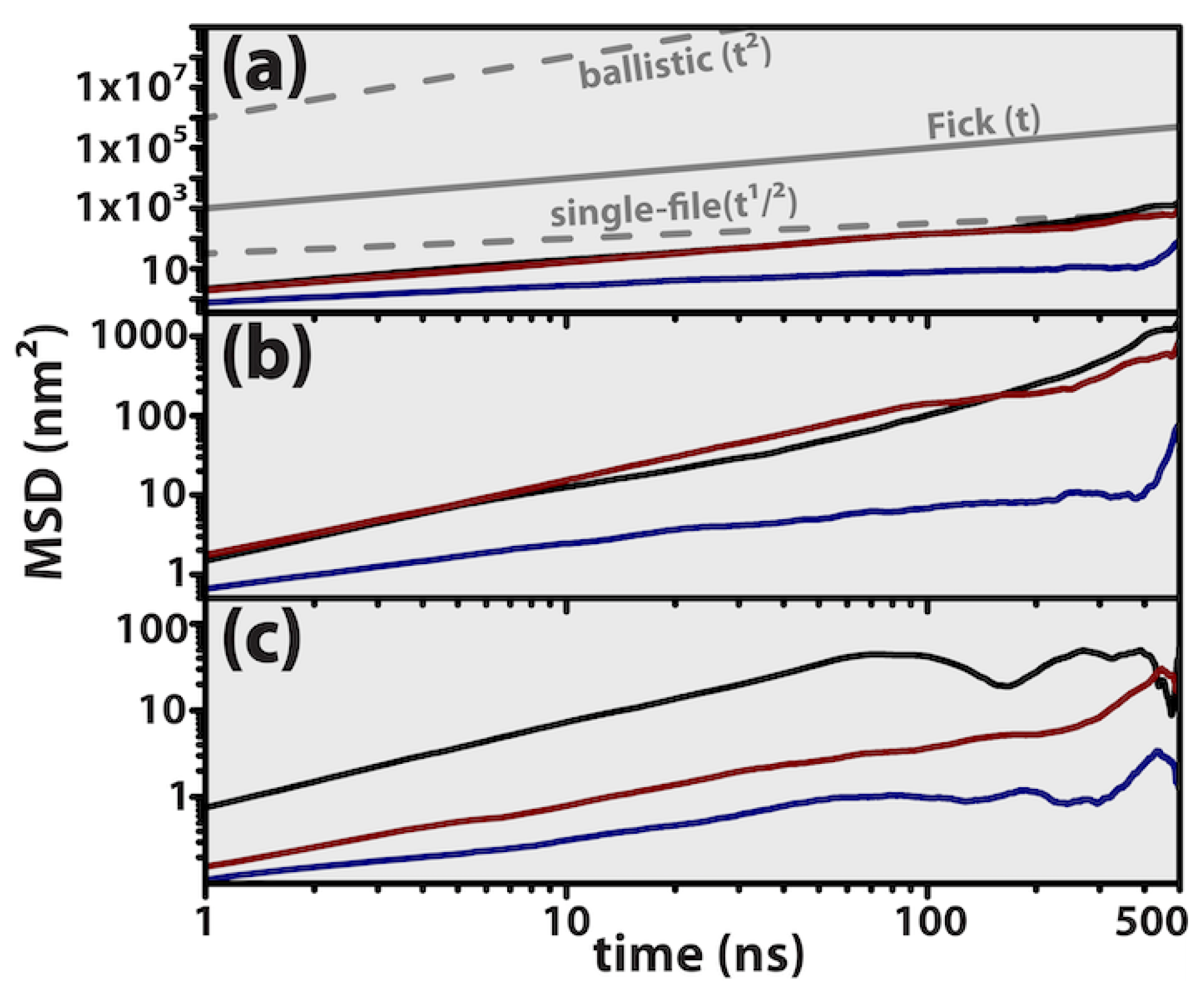
3.6. Dynamics
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Jiang, D.; Schedin, F.; Booth, T.J.; Khotkevich, V.V.; Morozov, S.V.; Geim, A.K. Two-dimensional atomic crystals. Proc. Natl. Acad. Sci. USA 2005, 102, 10451–10453. [Google Scholar] [CrossRef]
- Reina, G.; González-Domínguez, J.M.; Criado, A.; Vázquez, E.; Bianco, A.; Prato, M. Promises, facts and challenges for graphene in biomedical applications. Chem. Soc. Rev. 2017, 46, 4400–4416. [Google Scholar] [CrossRef] [PubMed]
- Pykal, M.; Jurecka, P.; Karlicky, F.; Otyepka, M. Modelling of graphene functionalization. Phys. Chem. Chem. Phys. 2016, 18, 6351–6372. [Google Scholar] [CrossRef]
- Sun, Z.; Fang, S.; Hu, Y.H. 3D Graphene Materials: From Understanding to Design and Synthesis Control. Chem. Rev. 2020, 120, 10336–10453. [Google Scholar] [CrossRef] [PubMed]
- Shankla, M.; Aksimentiev, A. Step-defect guided delivery of DNA to a graphene nanopore. Nat. Nanotechnol. 2019, 14, 858–865. [Google Scholar] [CrossRef]
- Kamińska, I.; Bohlen, J.; Yaadav, R.; Schüler, P.; Raab, M.; Schröder, T.; Zähringer, J.; Zielonka, K.; Krause, S.; Tinnefeld, P. Graphene Energy Transfer for Single-Molecule Biophysics, Biosensing, and Super-Resolution Microscopy. Adv. Mater. 2021, 33, 2101099. [Google Scholar] [CrossRef]
- Fadeel, B.; Bussy, C.; Merino, S.; Vázquez, E.; Flahaut, E.; Mouchet, F.; Evariste, L.; Gauthier, L.; Koivisto, A.J.; Vogel, U.; et al. Safety Assessment of Graphene-Based Materials: Focus on Human Health and the Environment. ACS Nano 2018, 12, 10582–10620. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mat. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Meyyappan, M. (Ed.) Carbon Nanotubes: Science and Applications; CRC Press: London, UK, 2005. [Google Scholar]
- Salmon, L.; Yang, S.; Al-Hashimi, H.M. Advances in the Determination of Nucleic Acid Conformational Ensembles. Ann. Rev. Phys. Chem. 2014, 65, 293–316. [Google Scholar] [CrossRef]
- Cervantes-Salguero, K.; Biaggne, A.; Youngsman, J.M.; Ward, B.M.; Kim, Y.C.; Li, L.; Hall, J.A.; Knowlton, W.B.; Graugnard, E.; Kuang, W. Strategies for Controlling the Spatial Orientation of Single Molecules Tethered on DNA Origami Templates Physisorbed on Glass Substrates: Intercalation and Stretching. Int. J. Mol. Sci. 2022, 23, 7690. [Google Scholar] [CrossRef] [PubMed]
- Bascom, G.; Andricioaei, I. Single-Walled Carbon Nanotubes Modulate the B- to A-DNA Transition. J. Phys. Chem. C 2014, 118, 29441–29447. [Google Scholar] [CrossRef] [PubMed]
- Zhao, X.; Johnson, J.K. Simulation of Adsorption of DNA on Carbon Nanotubes. J. Am. Chem. Soc. 2007, 129, 10438–10445. [Google Scholar] [CrossRef] [PubMed]
- Cruz, F.J.A.L.; De Pablo, J.J.; Mota, J.P.B. Endohedral confinement of a DNA dodecamer onto pristine carbon nanotubes and the stability of the canonical B form. J. Chem. Phys. 2014, 140, 225103. [Google Scholar] [CrossRef]
- Zhao, X. Self-Assembly of DNA Segments on Graphene and Carbon Nanotube Arrays in Aqueous Solution: A Molecular Simulation Study. J. Phys. Chem. C 2011, 115, 6181–6189. [Google Scholar] [CrossRef]
- Cruz, F.J.A.L.; De Pablo, J.J.; Mota, J.P.B. Free energy landscapes of the encapsulation mechanism of DNA nucleobases onto carbon nanotubes. RSC Adv. 2014, 4, 1310–1321. [Google Scholar] [CrossRef]
- Kode, V.R.; Hinkle, K.R.; Ao, G. Interaction of DNA-Complexed Boron Nitride Nanotubes and Cosolvents Impacts Dispersion and Length Characteristics. Langmuir 2021, 37, 10934–10944. [Google Scholar] [CrossRef]
- Iliafar, S.; Mittal, J.; Vezenov, D.; Jagota, A. Interaction of single-stranded DNA with curved carbon nanotube is much stronger than with flat graphite. J. Am. Chem. Soc. 2014, 136, 12947–12957. [Google Scholar] [CrossRef]
- Kode, V.R.; Thompson, M.E.; McDonald, C.; Weicherding, J.; Dobrila, T.D.; Fodor, P.S.; Ao, G. Purification and assembly of DNA-stabilized boron nitride nanotubes into aligned films. ACS Appl. Nano Mat. 2019, 2, 2099–2105. [Google Scholar] [CrossRef]
- Zheng, S.; Sajib, M.S.J.; Wei, Y.; Wei, T. Discontinuous Molecular Dynamics Simulations of Biomolecule Interfacial Behavior: Study of Ovispirin-1 Adsorption on a Graphene Surface. J. Chem. Theory Comput. 2021, 17, 1874–1882. [Google Scholar] [CrossRef]
- Jia, Q.; Yang, C.; Venton, B.J.; DuBay, K.H. Atomistic simulations of dopamine diffusion dynamics on a pristine graphene surface. Chem. Phys. Chem. 2022, 23, e202100783. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, S.; Chakrabarti, R. Unzipping of Double-Stranded Ribonucleic Acids by Graphene and Single-Walled Carbon Nanotube: Helix Geometry versus Surface Curvature. J. Phys. Chem. C 2016, 120, 22681–22693. [Google Scholar] [CrossRef]
- Gao, D.; Li, B.; Yang, Y.; Qu, Y.; Li, Y.-Q.; Zhao, M.; Liu, Y.; Liu, X.; Li, W. Defect-Induced Double-Stranded DNA Unwinding on Graphene. J. Phys. Chem. B 2021, 125, 2833–2840. [Google Scholar] [CrossRef] [PubMed]
- Wilson, J.; Aksimentiev, A. Water-Compression Gating of Nanopore Transport. Phys. Rev. Lett. 2018, 120, 268101. [Google Scholar] [CrossRef] [PubMed]
- Gu, Z.; Zhao, L.; Ge, C.; Liu, S.; Fang, G.; Chen, S.S.; Yang, Z.; Zhou, R. Facet-regulated adhesion of double-stranded DNA on palladium surfaces. Nanoscale 2019, 11, 1827–1836. [Google Scholar] [CrossRef] [PubMed]
- Gu, Z.; Zhao, L.; Liu, S.; Duan, G.; Perez-Aguilar, J.M.; Luo, J.; Li, W.; Zhou, R. Orientational Binding of DNA Guided by the C2N Template. ACS Nano 2017, 11, 3198–3206. [Google Scholar] [CrossRef]
- Zhao, L.; Gu, Z. Potential Unwinding of Double-Stranded DNA upon Binding to a Carbon Nitride Polyaniline (C3N) Nanosheet. J. Phys. Chem. B 2021, 125, 2258–2265. [Google Scholar] [CrossRef]
- Li, B.; Xie, X.; Duan, G.; Chen, S.H.; Meng, X.-Y.; Zhou, R. Binding patterns and dynamics of double-stranded DNA on the phosphorene surface. Nanoscale 2020, 12, 9430–9439. [Google Scholar] [CrossRef]
- Deng, Y.; Wang, F.; Liu, Y.; Yang, Y.; Qu, Y.; Zhao, M.; Mu, Y.; Li, W. Orientational DNA binding and directed transport on nanomaterial heterojunctions. Nanoscale 2020, 12, 5217–5226. [Google Scholar] [CrossRef]
- Zhou, H.; Xie, Z.-X.; Liang, L.; Zhang, P.; Ma, X.; Kong, Z.; Shen, J.-W.; Hu, W. Theoretical investigation on the adsorption orientation of DNA on two-dimensional MoSe2. Chem. Phys. 2021, 551, 111329. [Google Scholar] [CrossRef]
- Steele, W.A. Molecular Interactions for Physical Adsorption. Chem. Rev. 1993, 93, 2355–2378. [Google Scholar] [CrossRef]
- Girifalco, L.A.; Hodak, M.; Lee, R.S. Carbon nanotubes, buckyballs, ropes, and a universal graphitic potential. Phys. Rev. B 2000, 62, 13104–13110. [Google Scholar] [CrossRef]
- Macke, T.J.; Case, D.A. Modeling Unusual Nucleic Acid Structures. In Molecular Modeling of Nucleic Acids; Leontis, N.B., SantaLucia, J., Eds.; American Chemical Society: Washington, DC, USA, 1998; pp. 379–393. [Google Scholar]
- Wang, J.; Cieplak, P.; Kollman, P.A. How Well Does a Restrained Electrostatic Potential (RESP) Model Perform in Calculating Conformational Energies of Organic and Biological Molecules? J. Comput. Chem. 2000, 21, 1049–1074. [Google Scholar] [CrossRef]
- Lindorff-Larsen, K.; Piana, S.; Palmo, K.; Maragakis, P.; Klepeis, J.L.; Dror, R.O.; Shaw, D.E. Improved side-chain torsion potentials for the Amber ff99SB protein force field. Proteins 2010, 78, 1950–1958. [Google Scholar] [CrossRef] [PubMed]
- Jorgensen, W.L.; Chandrasekhar, J.; Madura, J.D.; Impey, R.W.; Klein, M.L. Comparison of Simple Potential Functions for Simulating Liquid Water. J. Chem. Phys. 1983, 79, 926–935. [Google Scholar] [CrossRef]
- Jorgensen, W.L. Transferable Intermolecular Potential Functions for Water, Alcohols, and Ethers. Application to Liquid Water. J. Am. Chem. Soc. 1981, 103, 335–340. [Google Scholar] [CrossRef]
- Noy, A.; Soteras, I.; Luque, F.J.; Orozco, M. The Impact of Monovalent Ion Force Field Model in Nucleic Acids Simulations. Phys. Chem. Chem. Phys. 2009, 11, 10596–10607. [Google Scholar] [CrossRef]
- Hess, B.; Kutzner, C.; Van Der Spoel, D.; Lindahl, E. GROMACS 4: Algorithms for Highly Efficient, Load-Balanced, and Scalable Molecular Simulation. J. Chem. Theory Comp. 2008, 4, 435–447. [Google Scholar] [CrossRef]
- Humphrey, W.; Dalke, A.; Schulten, K. VMD—Visual Molecular Dynamics. J. Molec. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef]
- Nosé, S. A Unified Formulation of the Constant Temperature Molecular Dynamics Methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical Dynamics: Equilibrium Phase-space Distributions. Phys. Rev. A 1985, 31, 1695–1697. [Google Scholar] [CrossRef]
- Parrinello, M.; Rahman, A. Polymorphic Transitions in Single Crystals: A New Molecular Dynamics Method. J. Appl. Phys. 1981, 52, 7182–7190. [Google Scholar] [CrossRef]
- Darden, T.; York, D.; Pedersen, L. Particle Mesh Ewald: An N log(N) Method for Ewald Sums in Large Systems. J. Chem. Phys. 1993, 98, 10089–10092. [Google Scholar] [CrossRef]
- Essmann, U.; Perera, L.; Berkowitz, M.L.; Darden, T.; Lee, H.; Pedersen, L.G. A Smooth Particle Mesh Ewald Potential. J. Chem. Phys. 1995, 103, 8577–8592. [Google Scholar] [CrossRef]
- Haile, J.M. Molecular Dynamics Simulation: Elementary Methods; Wiley: New York, NY, USA, 1992. [Google Scholar]
- Allen, M.P.; Tildesley, D.J. Computer Simulation of Liquids; Clarendon Press: Oxford, UK, 1990. [Google Scholar]
- Barducci, A.; Bussi, G.; Parrinello, M. Well-Tempered Metadynamics: A Smoothly Converging and Tunable Free-Energy Method. Phys. Rev. Lett. 2008, 100, 020603. [Google Scholar] [CrossRef] [PubMed]
- Sutto, L.; Marsili, S.; Gervasio, F.L. New Advances in Metadynamics. WIREs Comput. Mol. Sci. 2012, 2, 771–779. [Google Scholar] [CrossRef]
- Valsson, O.; Tiwary, P.; Parrinello, M. Enhancing Important Fluctuations: Rare Events and Metadynamics from a Conceptual Viewpoint. Ann. Rev. Phys. Chem. 2016, 67, 159–184. [Google Scholar] [CrossRef]
- Elder, R.M.; Pfaendtner, J.; Jayaraman, A. Effect of Hydrophobic and Hydrophilic Surfaces on the Stability of Double-Stranded DNA. Biomacromolecules 2015, 16, 1862–1869. [Google Scholar] [CrossRef]
- Deighan, M.; Bonomi, M.; Pfaendtner, J. Efficient Simulation of Explicitly Solvated Proteins in the Well-Tempered Ensemble. J. Chem. Theory Comput. 2012, 8, 2189–2219. [Google Scholar] [CrossRef]
- Laio, A.; Gervasio, F.L. Metadynamics: A Method to Simulate Rare Events and Reconstruct the Free Energy in Biophysics, Chemistry and Material Science. Rep. Prog. Phys. 2008, 71, 126601. [Google Scholar] [CrossRef]
- Cruz, F.J.A.L.; Mota, J.P.B. Conformational Thermodynamics of DNA Strands in Hydrophilic Nanopores. J. Phys. Chem. C 2016, 120, 20357–20367. [Google Scholar] [CrossRef]
- Tribello, G.A.; Bonomi, M.; Branduardi, D.; Camilloni, C.; Bussi, G. PLUMED 2: New feathers for an old bird. Comp. Phys. Comm. 2014, 185, 604–613. [Google Scholar] [CrossRef]
- Consortium, T.P. Promoting transparency and reproducibility in enhanced molecular simulations. Nat. Methods 2019, 16, 670–673. [Google Scholar] [CrossRef] [PubMed]
- Ricci, C.G.; de Andrade, A.S.; Mottin, M.; Netz, P.A. Molecular Dynamics of DNA: Comparison of Force Fields and Terminal Nucleotide Definitions. J. Phys. Chem. B 2010, 114, 9882–9893. [Google Scholar] [CrossRef] [PubMed]
- Naskar, S.; Gosika, M.; Joshi, H.; Maiti, P.K. Tuning the Stability of DNA Nanotubes with Salt. J. Phys. Chem. C 2019, 123, 9461–9470. [Google Scholar] [CrossRef]
- Shiraki, T.; Tsuzuki, A.; Toshimitsu, F.; Nakashima, N. Thermodynamics for the Formation of Double-Stranded DNA–Single-Walled Carbon Nanotube Hybrids. Chem. Eur. J. 2016, 22, 4774–4779. [Google Scholar] [CrossRef]
- Alegret, N.; Santos, E.; Rodriguez-Fortea, A.; Rius, F.X.; Poblet, J.M. Disruption of small double stranded DNA molecules on carbon nanotubes: A molecular dynamics study. Chem. Phys. Lett. 2012, 525, 120–124. [Google Scholar] [CrossRef]
- Franklin, R.E.; Gosling, R.G. Molecular Configuration in Sodium Thymonucleate. Nature 1953, 171, 740–741. [Google Scholar] [CrossRef]
- Santosh, M.; Panigrahi, S.; Bhattacharyya, D.; Sood, A.K. Unzipping and Binding of Small Interfering RNA with Single Walled Carbon Nanotube: A platform for Small Interfering RNA Delivery. J. Chem. Phys. 2012, 136, 65106. [Google Scholar] [CrossRef]
- Agnihotri, S.; Zheng, Y.; Mota, J.P.B.; Ivanov, I.; Kim, P.C. Practical Modeling of Heterogeneous Bundles of Single-Walled Carbon Nanotubes for Adsorption Applications: Estimating the Fraction of Open-Ended Nanotubes in Samples. J. Phys. Chem. C 2007, 111, 13747–13755. [Google Scholar] [CrossRef]
- Karger, J. Transport Phenomena in Nanoporous Materials. Chem. Phys. Chem. 2015, 16, 24–51. [Google Scholar] [CrossRef] [PubMed]
- Cruz, F.J.A.L.; Mota, J.P.B. Dynamics of B-DNA in Electrically Charged Solid Nanopores. J. Phys. Chem. C 2017, 121, 16568–16575. [Google Scholar] [CrossRef]
- Balducci, A.; Mao, P.; Han, J.; Doyle, P.S. Double-Stranded DNA Diffusion in Slitlike Nanochannels. Macromolecules 2006, 39, 6273–6281. [Google Scholar] [CrossRef]
- Krause, S.; Ploetz, E.; Bohlen, J.; Schuler, P.; Yaadav, R.; Selbach, F.; Steiner, F.; Kaminśka, I.; Tinnefeld, P. Graphene-on-Glass Preparation and Cleaning Methods Characterized by Single-Molecule DNA Origami Fluorescent Probes and Raman Spectroscopy. ACS Nano 2021, 15, 6430–6438. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cruz, F.J.A.L.; Mota, J.P.B. Partial Denaturation of Double-Stranded DNA on Pristine Graphene under Physiological-like Conditions. Liquids 2023, 3, 168-186. https://doi.org/10.3390/liquids3020013
Cruz FJAL, Mota JPB. Partial Denaturation of Double-Stranded DNA on Pristine Graphene under Physiological-like Conditions. Liquids. 2023; 3(2):168-186. https://doi.org/10.3390/liquids3020013
Chicago/Turabian StyleCruz, Fernando J. A. L., and José P. B. Mota. 2023. "Partial Denaturation of Double-Stranded DNA on Pristine Graphene under Physiological-like Conditions" Liquids 3, no. 2: 168-186. https://doi.org/10.3390/liquids3020013
APA StyleCruz, F. J. A. L., & Mota, J. P. B. (2023). Partial Denaturation of Double-Stranded DNA on Pristine Graphene under Physiological-like Conditions. Liquids, 3(2), 168-186. https://doi.org/10.3390/liquids3020013