Solvent and H/D Isotopic Substitution Effects on the Krichevskii Parameter of Solutes: A Novel Approach to Their Accurate Determination
Abstract
:1. Introduction
2. Fundamentals from Molecular Thermodynamics
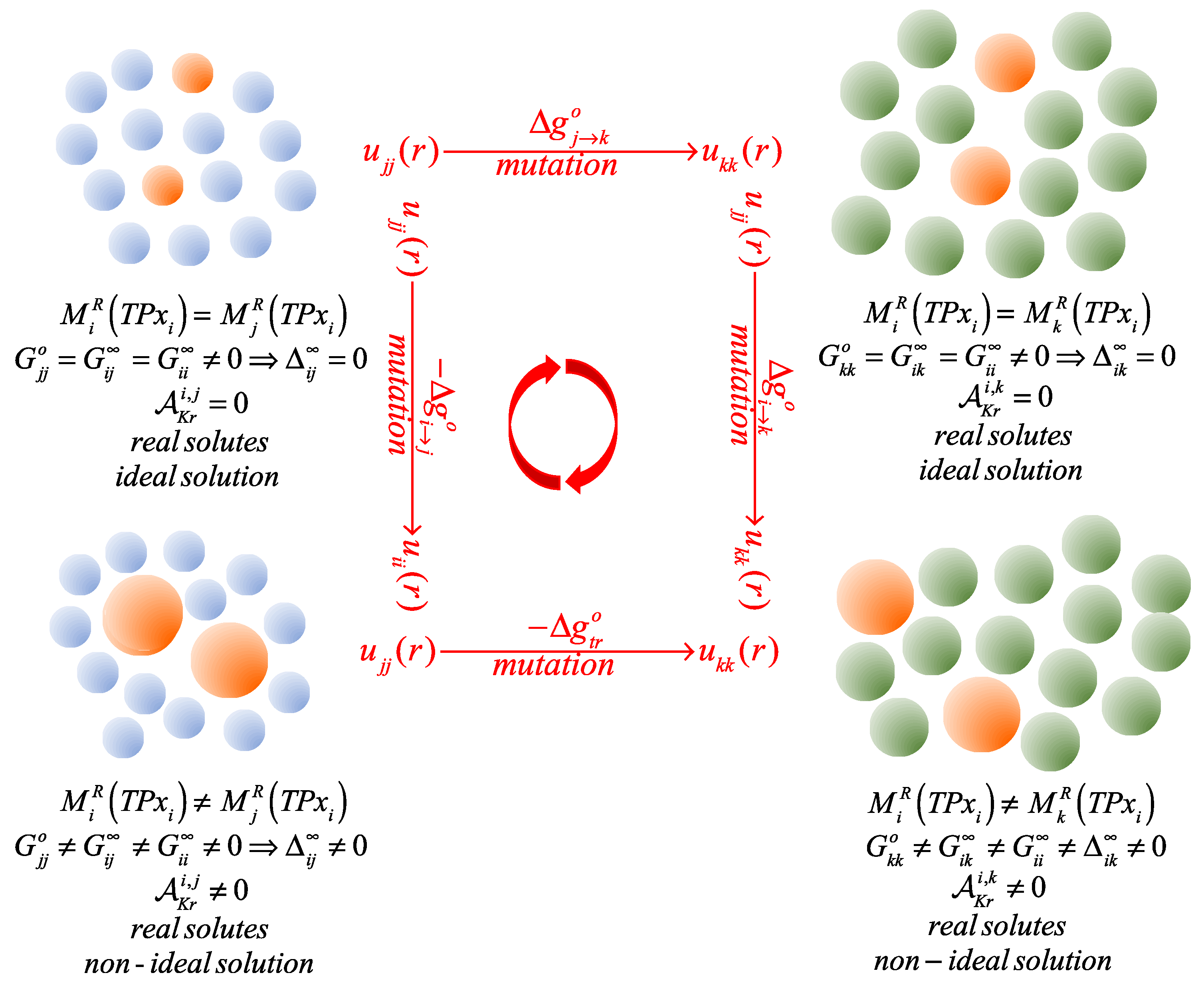
2.1. Molecular-Based Description of the Solvent Effect on the Solvation Behavior of a Solute
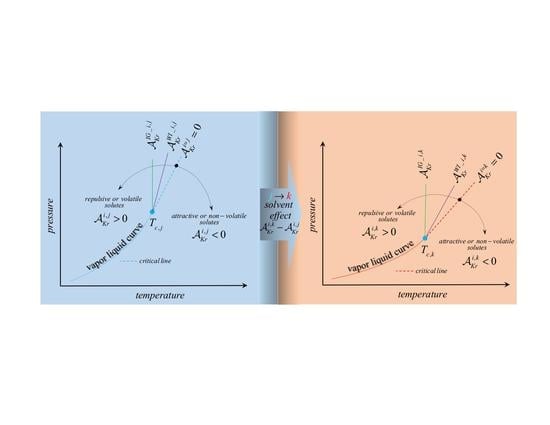
2.2. Link between the Solvent Effect on the Solute’s Solvation and Its Krichevskii Parameter
3. Experimental Evidence of the Solvent H/D−Isotope Substitution Effects and Solvation Interpretation
3.1. Identity of the Aqueous Solute Species and the Sources of Their Experimental Data
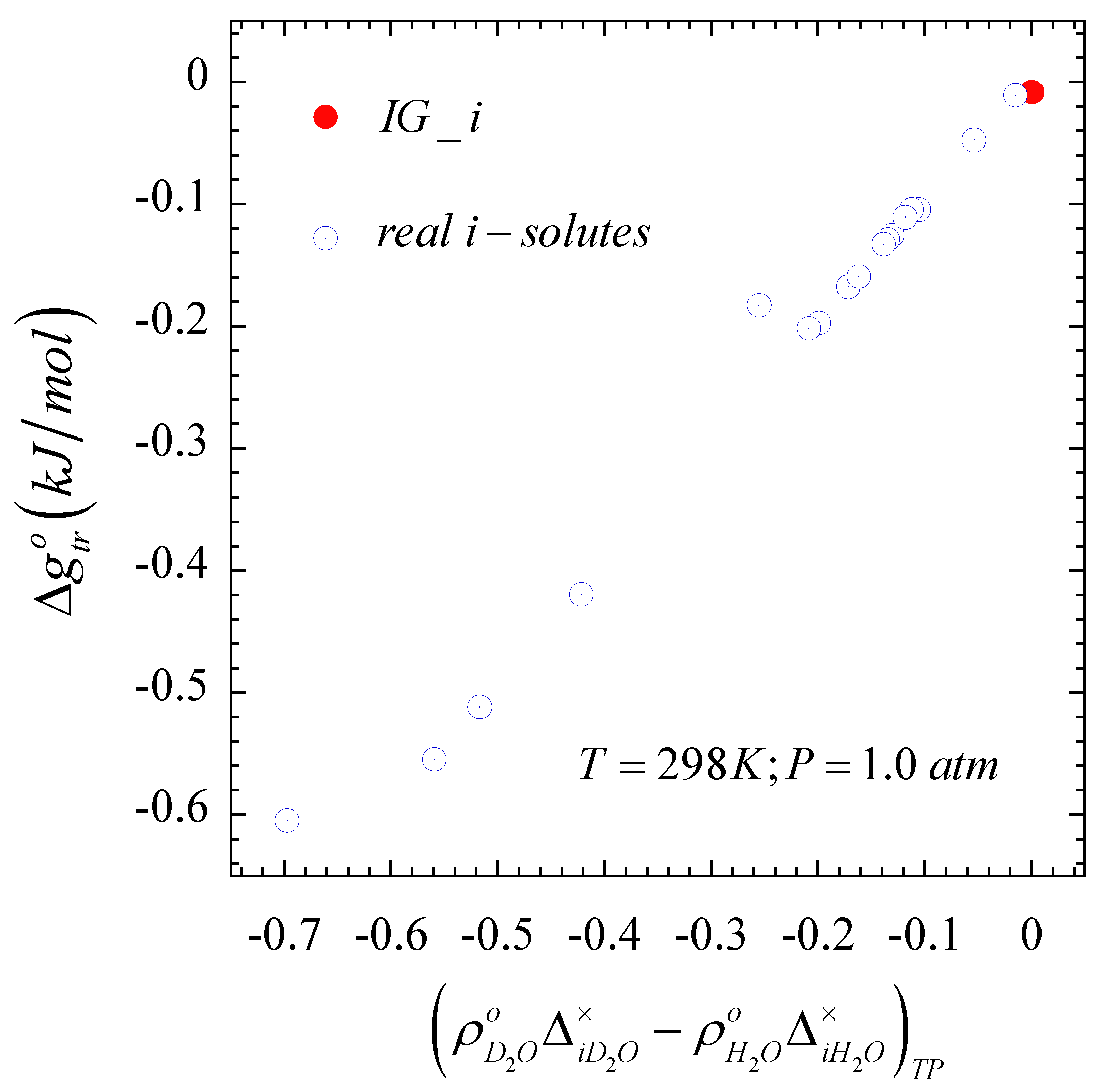
3.2. Brute-Force Difference Approach to the Solvent Effect on the Krichevskii Parameter of a Solute
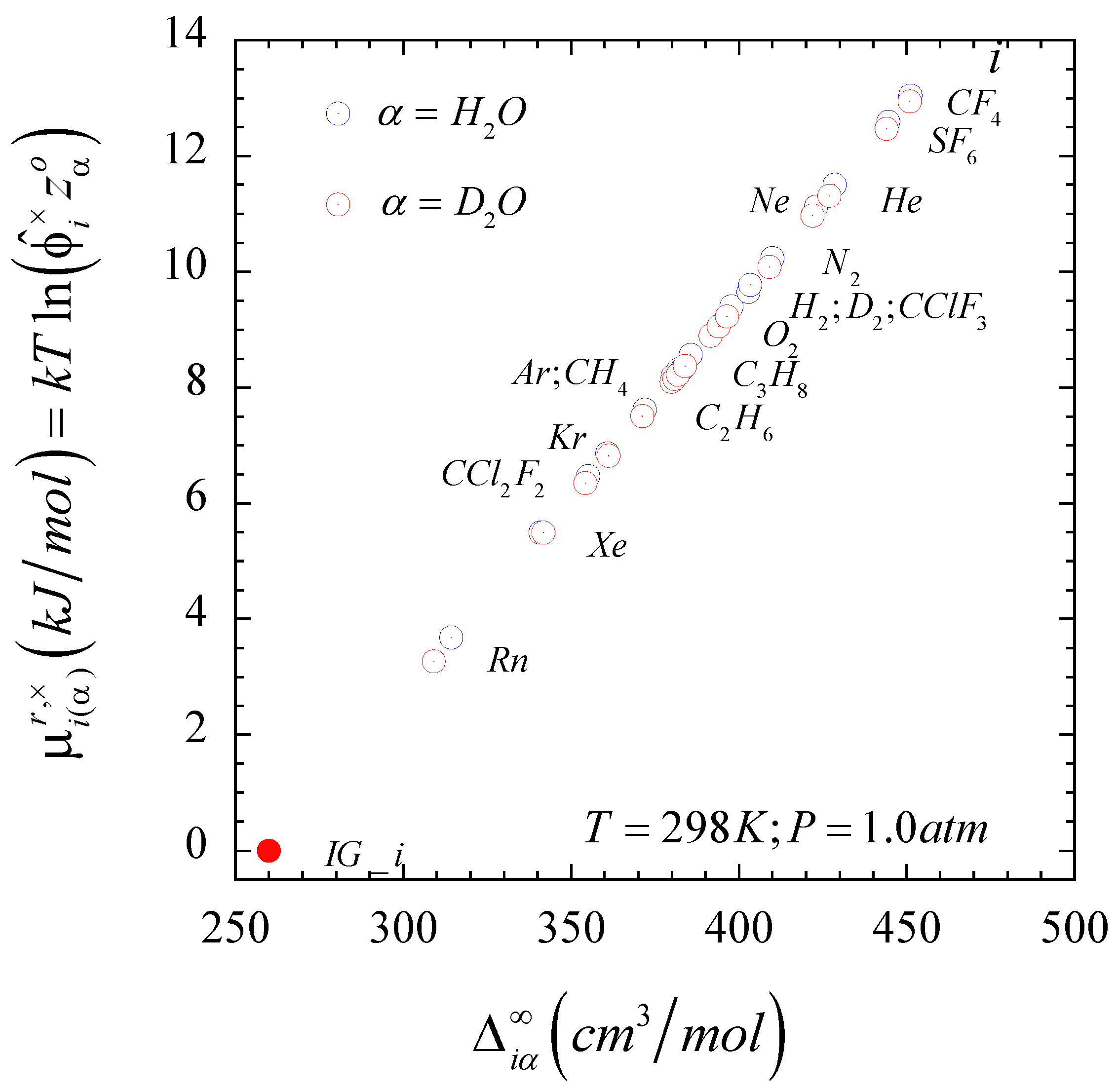
3.3. Required Solvation Properties in the Molecular-Based Approach to the Solvent H/D−Effect on the Krichevskii Parameter
3.4. Resulting Linear Representation for the Krichevskii Parameter
3.5. Link between the Solvent H/D−Effect on the Krichevskii Parameter and Solute–Solvent Intermolecular Interaction Asymmetries
3.6. Solvent H/D−Effect on the Krichevskii Parameter of the Emblematic Ideal Gas Solute
3.7. Solvent H/D−Effect on the Krichevskii Parameter of the Emblematic Case of Lewis-Randall’s Quasi-Ideal Solutions
4. Discussion and Relevant Observations
5. Final Remarks and Outlook
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols | |
| Krichevskii parameter of an solute in a solvent, i.e., | |
| direct correlation function integral, aka DCFI, for the interactions at the ⊗ conditions, either infinite dilution ∞ or pure component o | |
| direct correlation function integral | |
| fugacity of the species | |
| isobaric-isothermal function defined by Equation (6) | |
| Kirkwood-Buff integral for the interactions at the ⊗ conditions, either infinite dilution ∞ or pure component o | |
| Henry’s law constant of an species in a solvent given by | |
| Boltzmann constant | |
| Kirkwood-Buff | |
| isothermal compressibility of the pure solvent | |
| vapor-liquid solute distribution coefficient | |
| molar weight of an species | |
| reference molality of an solute | |
| number of molecules of an species | |
| system pressure | |
| generic isobaric-isothermal residual property of an infinitely dilute species in an solvent | |
| generic partial molar property of an infinitely dilute species | |
| structure making/breaking parameter of an infinitely dilute species in a solvent | |
| short- and long-range contributions to the Kirkwood-Buff integral according to the Ornstein-Zernike equation | |
| total correlation function integral, aka Kirkwood-Buff integral | |
| state conditions defined by the system temperature and pressure | |
| state conditions defined by the system temperature and density | |
| system volume at the specified state conditions and composition | |
| partial molar volume of the species | |
| liquid phase composition defined by the mole fraction of the species | |
| compressibility factor for the pure solvent | |
| standard solvation Gibbs free energy of the solute in the solvent | |
| solvation Gibbs free energy of transfer of an infinitely dilute solute between two solvent environments | |
| solvation Gibbs free energy according to Ben-Naim’s definition | |
| linear combination of Kirkwood-Buff integrals related to the non-ideality of the dilute solution, i.e., | |
| partial molar fugacity coefficient of the species | |
| a general function | |
| Lewis-Randall’s activity coefficient of the species, i.e., | |
| isobaric-isothermal residual chemical potential of the species at the specified state conditions and composition | |
| isochoric-isothermal residual chemical potential of the species at the specified state conditions and composition | |
| molar density of the system at the specified state conditions and composition | |
| Sub- and super-scripts | |
| critical condition for the pure solvent | |
| pure component | |
| infinite dilution | |
| solute species | |
| ideal solution | |
| solvent species | |
| Lewis-Randall | |
| ideal gas condition | |
| special case of solute as an ideal gas species | |
| special case of Lewis-Randall ideality when | |
| residual property at constant | |
| residual property at constant | |
Appendix A. Relation among Solvation Gibbs Free Energy Expressions
Appendix B. Krichevskii Parameter of Solutes in Quasi-Ideal Solutions
Appendix C. Relation between the Krichevskii Parameter and the Structure Making/Breaking Parameter
Appendix D. The Standard Hydration Gibbs Free Energy of Water Isotopomers and Their Link to the Corresponding Krichevskii Parameters
References
- Chialvo, A.A. Solvation Phenomena in Dilute Solutions: Formal, Experimental Evidence, and Modeling Implications. In Fluctuation Theory of Solutions: Applications in Chemistry, Chemical Engineering and Biophysics; Matteoli, E., O’Connell, J.P., Smith, P.E., Eds.; CRC Press: Boca Raton, FL, USA, 2013; pp. 191–224. [Google Scholar]
- Sengers, J.M.L. Solubility Near the Solvent’s Critical Point. J. Supercrit. Fluids 1991, 4, 215–222. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Cummings, P.T. Solute-induced Effects on the Structure and the Thermodynamics of Infinitely Dilute Mixtures. AlChE J. 1994, 40, 1558–1573. [Google Scholar] [CrossRef]
- Chialvo, A.A. On the Krichevskii Parameter of Solutes in Dilute Solutions: Formal Links between its Magnitude, the Solute-solvent Intermolecular Asymmetry, and the Precise Description of Solution Thermodynamics. Fluid Phase Equilibria 2020, 513, 112546. [Google Scholar] [CrossRef]
- Fisher, M.E. Correlation Functions and the Critical Region of Simple Fluids. J. Math. Phys. 1964, 5, 944–962. [Google Scholar] [CrossRef]
- Munster, A. Critical Fluctuations. In Fluctuation Phenomena in Solids; Burgess, R.E., Ed.; Academic Press: New York, NY, USA, 1965; pp. 180–264. [Google Scholar]
- Japas, M.L.; Sengers, J.M.H.L. Gas Solubility and Henry’s Law Near the Solvent’s Critical Point. AlChE J. 1989, 35, 705–713. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Cummings, P.T. Comments on “Near Critical Phase Behavior of Dilute Mixtures”. Mol. Phys. 1995, 84, 41–48. [Google Scholar] [CrossRef]
- Akinfiev, N.N.; Diamond, L.W. Thermodynamic description of aqueous nonelectrolytes at infinite dilution over a wide range of state parameters. Geochim. Cosmochim. Acta 2003, 67, 613–629. [Google Scholar] [CrossRef]
- Plyasunov, A.V.; Shock, E.L. Prediction of the vapor-liquid distribution constants for volatile nonelectrolytes in water up to its critical temperature. Geochim. Cosmochim. Acta 2003, 67, 4981–5009. [Google Scholar] [CrossRef]
- Anisimov, M.A.; Sengers, J.V.; Sengers, J.M.H.L. Chapter 2—Near-critical behavior of aqueous systems. In Aqueous Systems at Elevated Temperatures and Pressures; Palmer, D.A., Fernández-Prini, R., Harvey, A.H., Eds.; Academic Press: London, UK, 2004; pp. 29–71. [Google Scholar]
- Orakova, S.M.; Rasulov, S.M.; Abdulagatov, I.M. Experimental study of the isomorphism behavior of weakly (CVX) and strongly (CPX, KTX) singular properties of 0.082 n-hexane+0.918 water mixtures near the upper critical point. J. Mol. Liq. 2013, 187, 7–19. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Crisalle, O.D. On density-based modeling of dilute non-electrolyte solutions involving wide ranges of state conditions and intermolecular asymmetries: Formal results, fundamental constraints, and the rationale for its molecular thermodynamic foundations. Fluid Phase Equilibria 2021, 535, 112969. [Google Scholar] [CrossRef]
- Harvey, A.H.; Crovetto, R.; Sengers, J.M.H.L. Limiting vs. Apparent Critical Behavior of Henry’s Constant and K Factors. AlChE J. 1990, 36, 1901–1904. [Google Scholar] [CrossRef]
- Abdulagatov, A.I.; Stepanov, G.V.; Abdulagatov, I.M. The critical properties of binary mixtures containing carbon dioxide: Krichevskii parameter and related thermodynamic properties. High Temp. 2007, 45, 408–424. [Google Scholar] [CrossRef]
- Plyasunov, A.V. Values of the Krichevskii Parameter, AKr, of Aqueous Nonelectrolytes Evaluated from Relevant Experimental Data. J. Phys. Chem. Ref. Data 2012, 41, 033104. [Google Scholar] [CrossRef]
- Abdulagatov, A.I.; Abdulagatov, I.M.; Stepanov, G.V. Crossover equation of state and microstructural properties of infinitely dilute solutions near the critical point of a pure solvent. J. Struct. Chem. 2001, 42, 412–422. [Google Scholar] [CrossRef]
- Blanco, S.T.; Gil, L.; Garcia-Gimenez, P.; Artal, M.; Otin, S.; Velasco, I. Critical Properties and High-Pressure Volumetric Behavior of the Carbon Dioxide plus Propane System at T = 308.15 K. Krichevskii Function and Related Thermodynamic Properties. J. Phys. Chem. B 2009, 113, 7243–7256. [Google Scholar] [CrossRef]
- Gil, L.; Martinez-Lopez, J.F.; Artal, M.; Blanco, S.T.; Embid, J.M.; Fernandez, J.; Otin, S.; Velasco, I. Volumetric Behavior of the {CO2 (1) + C2H6 (2)} System in the Subcritical (T = 293.15 K), Critical, and Supercritical (T = 308.15 K) Regions. J. Phys. Chem. B 2010, 114, 5447–5469. [Google Scholar] [CrossRef] [PubMed]
- Rivas, C.; Blanco, S.T.; Fernandez, J.; Artal, M.; Velasco, I. Influence of methane and carbon monoxide in the volumetric behaviour of the anthropogenic CO2: Experimental data and modelling in the critical region. Int. J. Greenh. Gas Control. 2013, 18, 264–276. [Google Scholar] [CrossRef]
- Plyasunov, A.V. Empirical evaluation of the Krichevskii parameter for aqueous solutes. J. Mol. Liq. 2017, 239, 92–95. [Google Scholar] [CrossRef]
- Wilhelm, E. Solubilities, Fugacities and All That in Solution Chemistry. J. Solut. Chem. 2015, 44, 1004–1061. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Crisalle, O.D. On the Linear Orthobaric-density Representation of Near-critical Solvation Quantities: What Can We Conclude about the Accuracy of this Paradigm? Fluid Phase Equilibria 2020, 514, 112535. [Google Scholar] [CrossRef]
- Fernández-Prini, R.; Alvarez, J.L.; Harvey, A.H. Henry’s constants and vapor-liquid distribution constants for gaseous solutes in H2O and D2O at high temperatures. J. Phys. Chem. Ref. Data 2003, 32, 903–916. [Google Scholar] [CrossRef] [Green Version]
- Hansen, J.P.; McDonald, I.R. Theory of Simple Liquids, 3rd ed.; Academic Press: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Haile, J.M. On the Use of Computer Simulation to Determine the Excess Free Energy in Fluid Mixtures. Fluid Phase Equilibria 1986, 26, 103–127. [Google Scholar] [CrossRef]
- Kirkwood, J.G. Statistical mechanics of liquid solutions. Chem. Rev. 1936, 19, 275–307. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Cummings, P.T.; Simonson, J.M.; Mesmer, R.E. Solvation in High-Temperature Electrolyte Solutions. II. Some Formal Results. J. Chem. Phys. 1999, 110, 1075–1086. [Google Scholar] [CrossRef] [Green Version]
- Ben-Naim, A. Solvation Thermodynamics; Plenum Press: New York, NY, USA, 1987. [Google Scholar]
- Chialvo, A.A.; Crisalle, O.D. On the behavior of the osmotic second virial coefficients of gases in aqueous solutions: Rigorous results, accurate approximations, and experimental evidence. J. Chem. Phys. 2019, 150, 124503. [Google Scholar] [CrossRef] [PubMed]
- Plyasunov, A.V.; Shock, E.L. Estimation of the Krichevskii parameter for aqueous nonelectrolytes. J. Supercrit. Fluids 2001, 20, 91–103. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Cummings, P.T.; Kalyuzhnyi, Y.V. Solvation effect on kinetic rate constant of reactions in supercritical solvents. AlChE J. 1998, 44, 667–680. [Google Scholar] [CrossRef]
- Abbott, M.M.; Nass, K.K. Equations of State and Classical Solution Thermodynamics—Survey of the Connections. ACS Symp. Ser. 1986, 300, 2–40. [Google Scholar]
- Conrad, J.K.; Tremaine, P.R. A study of the deuterium isotope effect on zinc(II) hydrolysis and solubility under hydrothermal conditions using density functional theory. Chem. Eng. Sci. 2022, 254, 117596. [Google Scholar] [CrossRef]
- Plumridge, J.; Arcis, H.; Tremaine, P.R. Limiting Conductivities of Univalent Cations and the Chloride Ion in H2O and D2O Under Hydrothermal Conditions. J. Solut. Chem. 2015, 44, 1062–1089. [Google Scholar] [CrossRef]
- Trevani, L.N.; Balodis, E.C.; Tremaine, P.R. Apparent and standard partial molar volumes of NaCl, NaOH, and HCl in water and heavy water at T = 523 K and 573 K at p = 14 MPa. J. Phys. Chem. B 2007, 111, 2015–2024. [Google Scholar] [CrossRef] [PubMed]
- Dohnal, V.; Fenclova, D.; Vrbka, P. Temperature dependences of limiting activity coefficients, Henry’s law constants, and derivative infinite dilution properties of lower (C-1-C-5) 1-alkanols in water. Critical compilation, correlation, and recommended data. J. Phys. Chem. Ref. Data 2006, 35, 1621–1651. [Google Scholar] [CrossRef]
- Majer, V.; Sedlbauer, J.; Wood, R.H. Chapter 4—Calculation of standard thermodynamic properties of aqueous electrolytes and nonelectrolytes. In Aqueous Systems at Elevated Temperatures and Pressures; Palmer, D.A., Fernández-Prini, R., Harvey, A.H., Eds.; Academic Press: London, UK, 2004; pp. 99–147. [Google Scholar]
- Wilhelm, E.; Battino, R.; Wilcock, R.J. Low-Pressure Solubility of Gases in Liquid Water. Chem. Rev. 1977, 77, 219–262. [Google Scholar] [CrossRef]
- Moine, E.; Privat, R.; Sirjean, B.; Jaubert, J.N. Jaubert, Estimation of Solvation Quantities from Experimental Thermodynamic Data: Development of the Comprehensive CompSol Databank for Pure and Mixed Solutes. J. Phys. Chem. Ref. Data 2017, 46, 033102. [Google Scholar] [CrossRef]
- Scharlin, P.; Battino, R. Solubility of 13 Nonpolar Gases in Deuterium-Oxide at 15-Degrees-C-45-Degrees-C and 101.325-Kpa—Thermodynamics of Transfer of Nonpolar Gases from H2O to D2O. J. Solution Chem. 1992, 21, 67–91. [Google Scholar] [CrossRef]
- Scharlin, P.; Battino, R. Solubility of CCl2F2,CClF3,CF4, and c-C4F8 in H2O and D2O at 298 K to 318 K and 101.325 KPa. Thermodynamics of Transfer of Gases from H2O to D2O. Fluid Phase Equilibria 1994, 95, 137–147. [Google Scholar] [CrossRef]
- Taylor, J.R. An Introduction to Error Analysis: The Study of Uncertainties in Physical Measurements; University Science Books: Sausalito, CA, USA, 2022. [Google Scholar]
- Chialvo, A.A.; Crisalle, O.D. Linear Orthobaric-density Approach to the Krichevskii Parameter of a Solute from the Vapor-Liquid Distribution Coefficient: What can we learn about its accuracy from systems whose precise behavior is known? Fluid Phase Equilibria 2023, 565, 113651. [Google Scholar] [CrossRef]
- Ben-Naim, A.; Marcus, Y. Solvation Thermodynamics Of Nonionic Solutes. J. Chem. Phys. 1984, 81, 2016–2027. [Google Scholar] [CrossRef]
- Chialvo, A.A. Gas solubility in dilute solutions: A novel molecular thermodynamic perspective. J. Chem. Phys. 2018, 148, 174502. [Google Scholar] [CrossRef]
- Chialvo, A.A.; Crisalle, O.D. Solvation behavior of solutes in dilute solutions novel formal results, rules of thumb, and potential modeling pitfalls. Fluid Phase Equilibria 2019, 496, 17–30. [Google Scholar] [CrossRef]
- Wagner, W.; Pruss, A. The IAPWS formulation 1995 for the thermodynamic properties of ordinary water substance for general and scientific use. J. Phys. Chem. Ref. Data 2002, 31, 387–535. [Google Scholar] [CrossRef]
- Hill, P.G.; Macmillan, R.D.C.; Lee, V. A Fundamental Equation of State of Heavy Water. J. Phys. Chem. Ref. Data 1982, 11, 1–14. [Google Scholar] [CrossRef]
- Herrig, S.; Thol, M.; Harvey, A.H.; Lemmon, E.W. A Reference Equation of State for Heavy Water. J. Phys. Chem. Ref. Data 2018, 47, 043102. [Google Scholar] [CrossRef] [Green Version]
- Chialvo, A.A. On the Solvation Thermodynamics Involving Species with Large Intermolecular Asymmetries: A Rigorous Molecular-Based Approach to Simple Systems with Unconventionally Complex Behaviors. J. Phys. Chem. B 2020, 124, 7879–7896. [Google Scholar] [CrossRef] [PubMed]
- Ben-Naim, A. Molecular Theory of Solutions; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Jancsó, G.; Rebelo, L.P.N.; Van Hook, W.A. Non-ideality in Isotopic Mixtures. J. Chem. Soc. 1994, 23, 257–264. [Google Scholar] [CrossRef]
- Japas, M.L.; Prini, R.F.; Horita, J.; Wesolowski, D.J. Fractionation of Isotopic-species between Coexistening Liquid and Vapor: Complete Range, including the Asymptotic Critical Behavior. J. Phys. Chem. 1995, 99, 5171–5175. [Google Scholar] [CrossRef]
- Jancsó, G. Are isotopic mixtures ideal? Pure Appl. Chem. 2004, 76, 11–17. [Google Scholar] [CrossRef] [Green Version]
- Jancso, G.; Rebelo, L.P.N.; Van Hook, W.A. Isotope Effects in Solution Thermodynamics: Excess Properties in Solutions of Isotopomers. Chem. Rev. 1993, 93, 2645–2666. [Google Scholar] [CrossRef]
- Abdulkadirova, K.S.; Wyczalkowska, A.K.; Anisimov, M.A.; Sengers, J.V. Thermodynamic properties of mixtures of H2O and D2O in the critical region. J. Chem. Phys. 2002, 116, 4597–4610. [Google Scholar] [CrossRef]
- Bazaev, A.R.; Abdulagatov, I.M.; Magee, J.W.; Bazaev, E.A.; Ramazanova, A.E. PVTx measurements for H2O+D2O mixtures in the near-critical and supercritical regions. J. Supercrit. Fluids 2003, 26, 115–128. [Google Scholar] [CrossRef]
- Mazo, R.M. Statistical Mechanical Theory of Solutions. J. Chem. Phys. 1958, 29, 1122–1128. [Google Scholar] [CrossRef]
- Chialvo, A.A. Alternative Approach to Modeling Excess Gibbs Free Energy in Terms of Kirkwood-Buff Integrals. In Advances in Thermodynamics; Matteoli, E., Mansoori, G.A., Eds.; Taylor & Francis: New York, NY, USA, 1990; pp. 131–173. [Google Scholar]
- Sengers, J.M.H.L. Critical Behavior of Fluids: Concepts and Applications. In Supercritical Fluids; Kiran, E., Sengers, J.M.H.L., Eds.; Fundamentals for Applications, Kluwer Academic Publishers: Dordrecht, The Netherlands, 1994; pp. 3–38. [Google Scholar]
- Chialvo, A.A. On the Solute-Induced Structure-Making/Breaking Effect: Rigorous Links among Microscopic Behavior, Solvation Properties, and Solution Non-Ideality. J. Phys. Chem. B 2019, 123, 2930–2947. [Google Scholar] [CrossRef] [PubMed]
- Chialvo, A.A.; Crisalle, O.D. Can Jones-Dole’s B-coefficient Be a Consistent Structure Making/Breaking Marker?. Rigorous molecular-based analysis and critical assessment of its marker uniqueness. J. Phys. Chem. B 2021, 125, 12028–12041. [Google Scholar] [CrossRef] [PubMed]
- Debenedetti, P.G.; Mohamed, R.S. Attractive, Weakly Attractive and Repulsive Near-Critical Systems. J. Chem. Phys. 1989, 90, 4528–4536. [Google Scholar] [CrossRef]
- Petsche, I.B.; Debenedetti, P.G. On the Influence of Solute-Solvent Asymmetry upon the Behavior of Dilute Supercritical Mixtures. J. Phys. Chem. 1991, 95, 386–399. [Google Scholar] [CrossRef]
- Chialvo, A.A. Solute-Solute and Solute-Solvent Correlations in Dilute Near-Critical Ternary Mixtures: Mixed Solute and Entrainer Effects. J. Phys. Chem. 1993, 97, 2740–2744. [Google Scholar] [CrossRef]
- Wilhelm, E.; Battino, R. Thermodynamic Functions of Solubilities of Gases in Liquids at 25 degrees C. Chem. Rev. 1973, 73, 1–9. [Google Scholar] [CrossRef]
- Crovetto, R.; Fernandez-Prini, R.; Japas, M.L. Solubility of Inert Gases and Methane in H2O and D2O in the Temperature Range of 300 to 600K. J. Chem. Phys. 1982, 76, 1077. [Google Scholar] [CrossRef]
- Alvarez, J.; Fernandez-Prini, R. A semiempirical Procedure to Describe the Thermodynamics of Dissolution of Non-Polar Gases in Water. Fluid Phase Equilibria 1991, 66, 309–326. [Google Scholar] [CrossRef]
- Van Ness, H.C.; Abbott, M.M. Classical Thermodynamics of Nonelectrolyte Solutions; McGraw Hill: New York, NY, USA, 1982. [Google Scholar]
- Kirkwood, J.G.; Buff, F.P. The Statistical Mechanical Theory of Solution. I. J. Chem. Phys. 1951, 19, 774–777. [Google Scholar] [CrossRef]

| Solute | (a) | (a) | (b) | (b) |
|---|---|---|---|---|
| 1661 | 1673 | 19.418 | 19.482 | |
| 1836 | 1780 | 19.038 | 19.132 | |
| 1692 | 1656 | 16.242 | 16.321 | |
| 1668 | 1642 | 14.773 | 14.987 | |
| 1482 | 1487 | 13.404 | 13.655 | |
| --- | --- | 11.590 | 11.432 | |
| 1675 | --- | 17.687 | 17.394 (c) | |
| --- | 1562 | 17.565 (c) | 17.222 (c) | |
| 1750 | --- | 18.149 | 18.251 | |
| 1688 | --- | 16.479 | 16.539 | |
| 1623 | 1617 | 16.231 | 16.388 | |
| 1570 | --- | 15.535 | 15.671 | |
| --- | --- | 16.108 | 16.265 | |
| 2103 | --- | 20.510 | 20.643 | |
| --- | --- | 14.383 | 14.512 | |
| --- | --- | 17.310 | 17.060 | |
| --- | --- | 20.960 | 21.111 |
| Solute | (a) | (b) | (c) | (d) | |
|---|---|---|---|---|---|
| 1661 | ±52 | 1673 | ±40 | 12 ± 92 | |
| 1836 | ±108 | 1780 | ±32 | −56 ± 140 | |
| 1692 | ±37 | 1656 | ±67 | −36 ± 104 | |
| 1668 | ±52 | 1642 | ±11 | −26 ± 63 | |
| 1675 | ±76 | 1562 (e) | ±101 | −113 ± 177 | |
| 1623 | ±56 | 1617 | ±15 | −6 ± 71 |
| Solute | (a) | (b) | (c) |
|---|---|---|---|
| −198.0 | 428.5 | 427.0 | |
| −168.0 | 423.0 | 421.9 | |
| −183.0 | 383.2 | 380.7 | |
| −48.0 | 360.8 | 361.2 | |
| −11.0 | 340.8 | 341.7 | |
| −420.0 | 314.3 | 309.1 | |
| −555.0 | 403.3 | 396.4 | |
| −605.0 | 402.8 | 393.9 | |
| −160.0 | 410.0 | 408.9 | |
| −202.0 | 385.7 | 383.9 | |
| −105.0 | 382.0 | 383.4 | |
| −126.0 | 371.9 | 371.2 | |
| −105.0 | 380.3 | 379.9 | |
| −129.0 | 444.5 | 444.0 | |
| −133.0 | 355.1 | 354.2 | |
| −512.0 | 397.8 | 391.5 | |
| −111.0 | 451.1 | 450.8 |
| Solute | (a) |
|---|---|
| −25.50 | |
| −24.14 | |
| −22.42 | |
| −26.97 | |
| −36.60 | |
| −38.26 | |
| −23.21 | |
| −23.15 | |
| −19.68 | |
| −19.85 | |
| −19.58 | |
| −23.96 | |
| −19.17 | |
| −34.80 | |
| −23.69 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chialvo, A.A.; Crisalle, O.D. Solvent and H/D Isotopic Substitution Effects on the Krichevskii Parameter of Solutes: A Novel Approach to Their Accurate Determination. Liquids 2022, 2, 474-503. https://doi.org/10.3390/liquids2040028
Chialvo AA, Crisalle OD. Solvent and H/D Isotopic Substitution Effects on the Krichevskii Parameter of Solutes: A Novel Approach to Their Accurate Determination. Liquids. 2022; 2(4):474-503. https://doi.org/10.3390/liquids2040028
Chicago/Turabian StyleChialvo, Ariel A., and Oscar D. Crisalle. 2022. "Solvent and H/D Isotopic Substitution Effects on the Krichevskii Parameter of Solutes: A Novel Approach to Their Accurate Determination" Liquids 2, no. 4: 474-503. https://doi.org/10.3390/liquids2040028
APA StyleChialvo, A. A., & Crisalle, O. D. (2022). Solvent and H/D Isotopic Substitution Effects on the Krichevskii Parameter of Solutes: A Novel Approach to Their Accurate Determination. Liquids, 2(4), 474-503. https://doi.org/10.3390/liquids2040028