Understanding Separation Mechanisms of Monoatomic Gases, Such as Kr and Xe, via DD3R Zeolite Membrane Using Molecular Dynamics
Abstract
:1. Introduction
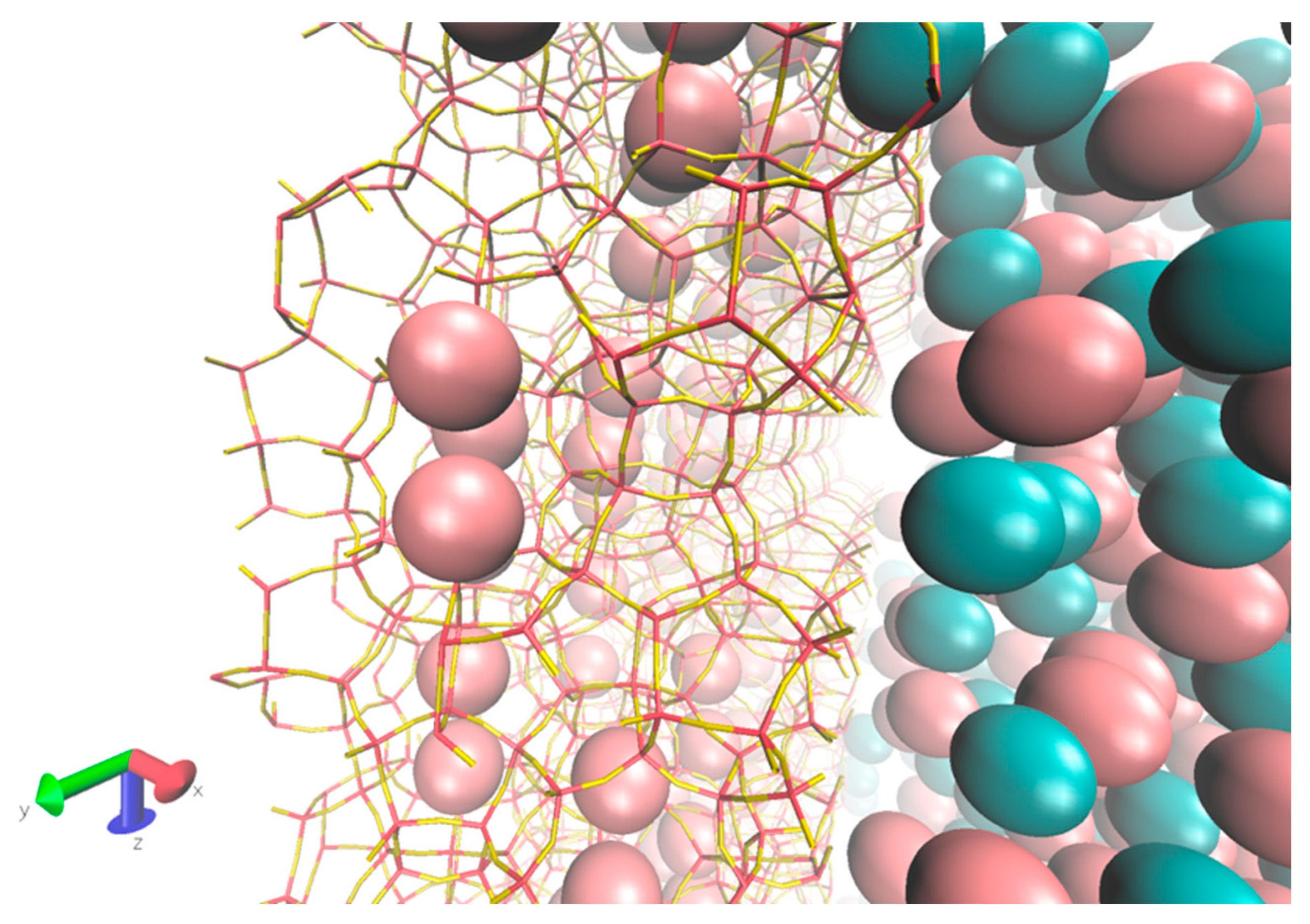
2. Materials and Methods
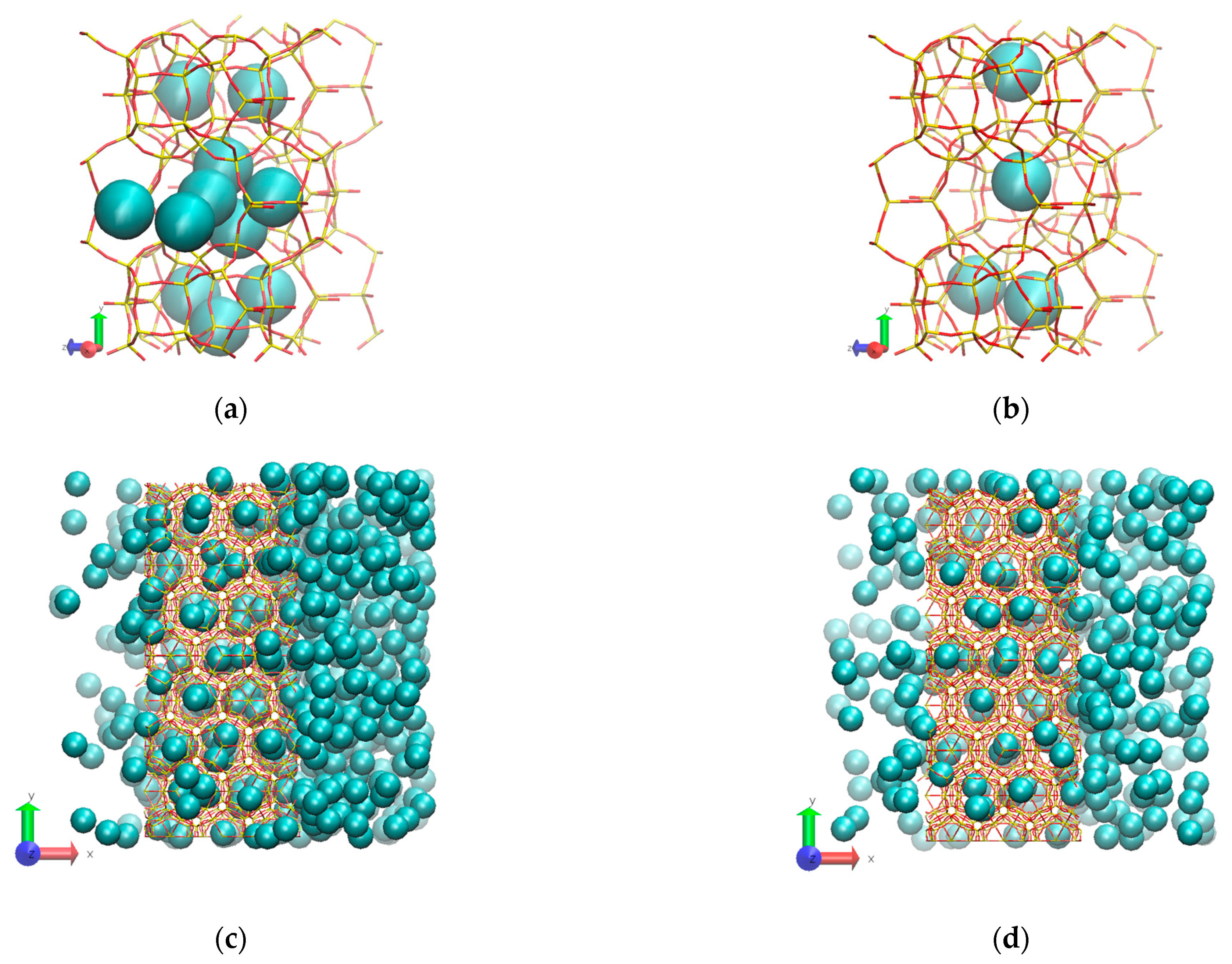
3. Results
3.1. Pure Gases
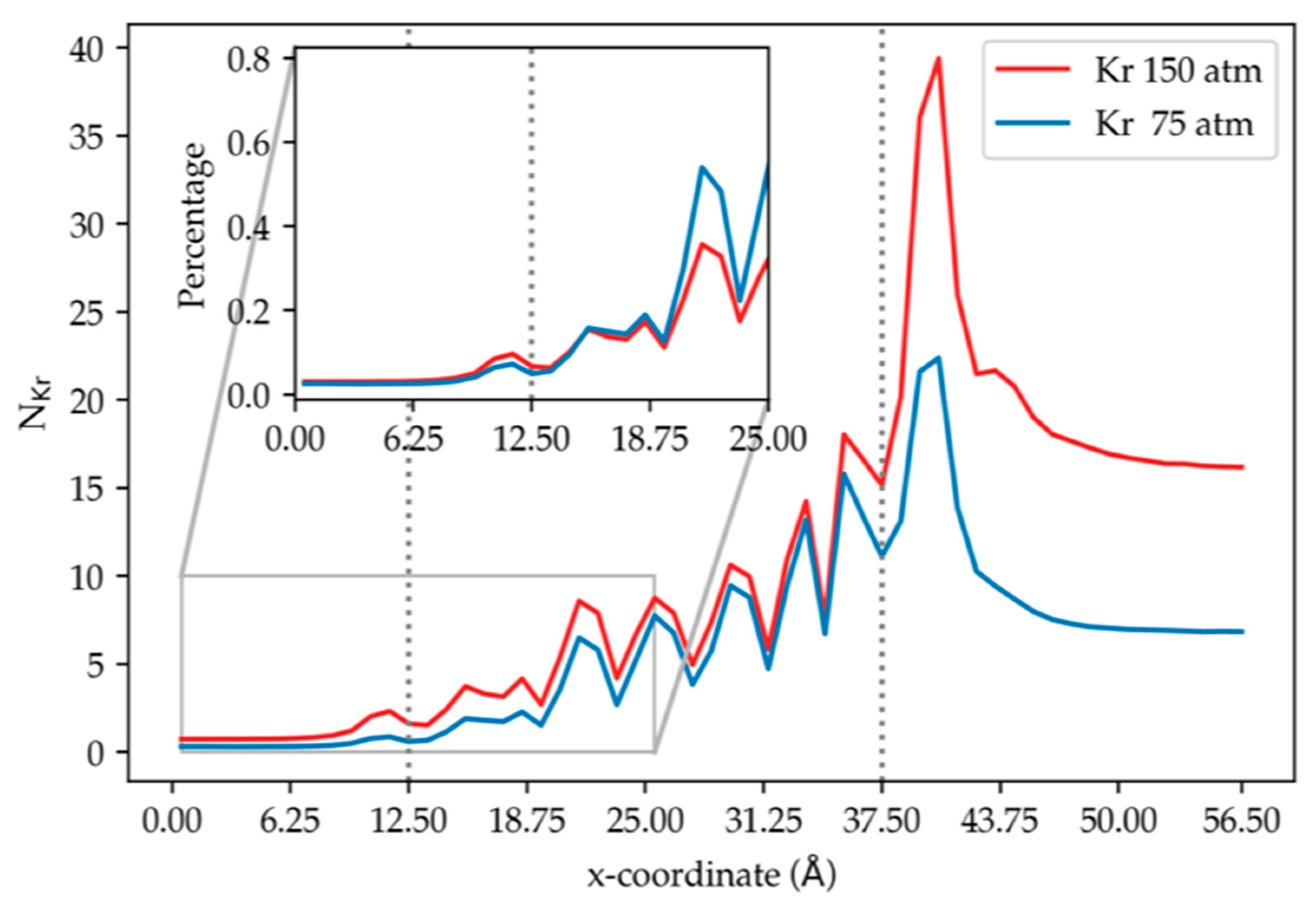
3.1.1. Effect of Pressure on Pure Kr Permeation
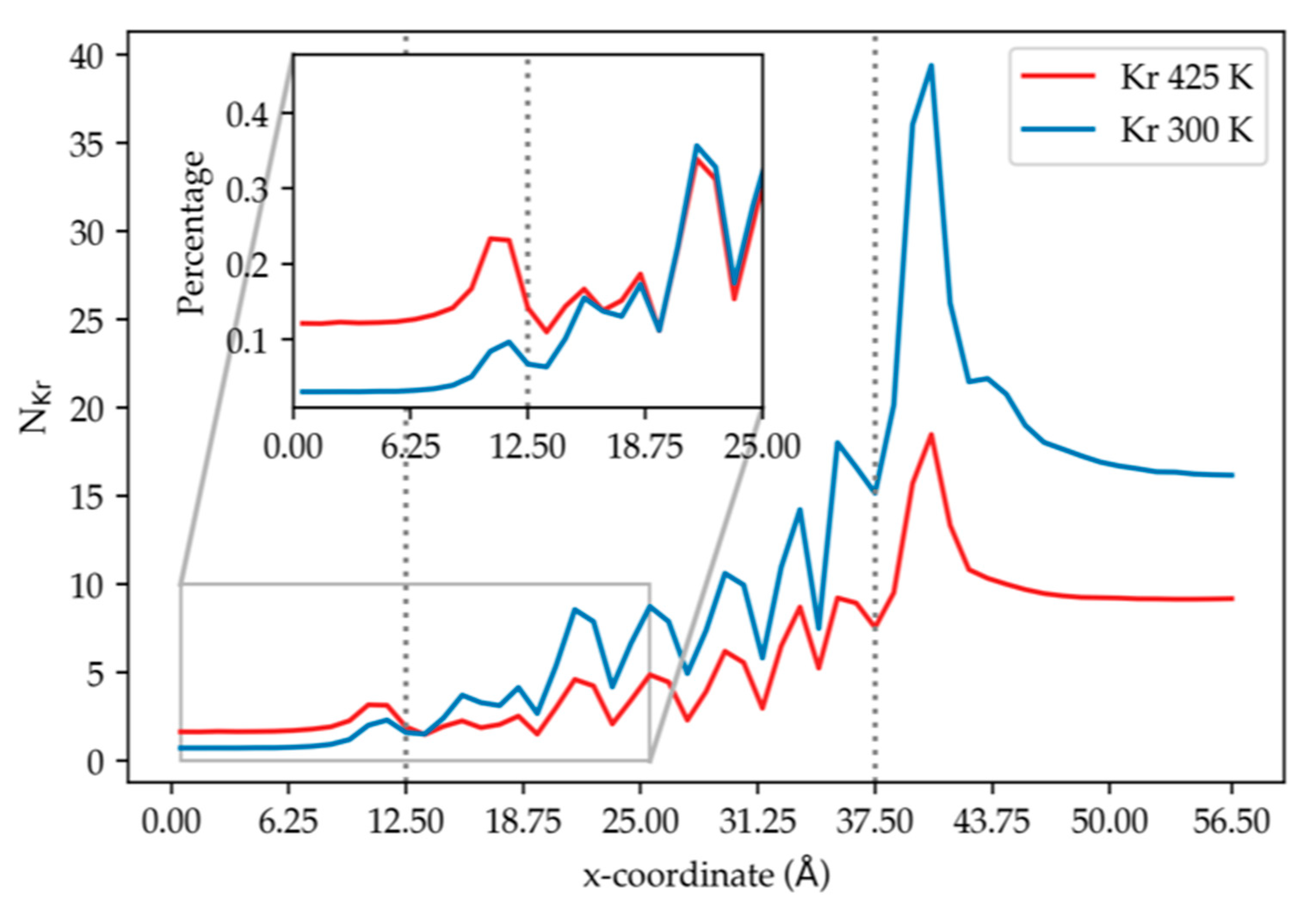
3.1.2. Effect of Temperature on Pure Kr Permeation
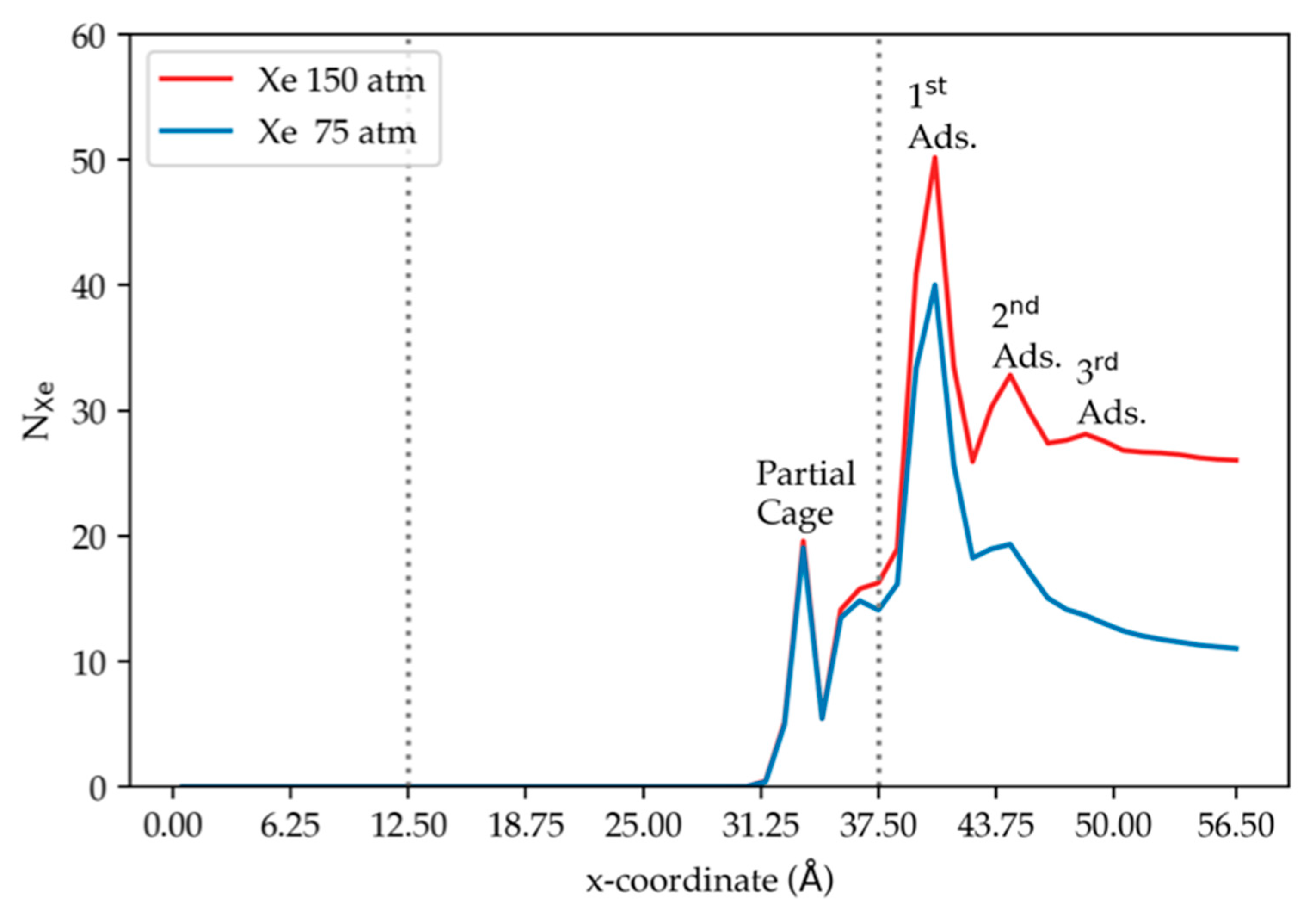
3.1.3. Effect of Pressure on Pure Xe Permeation
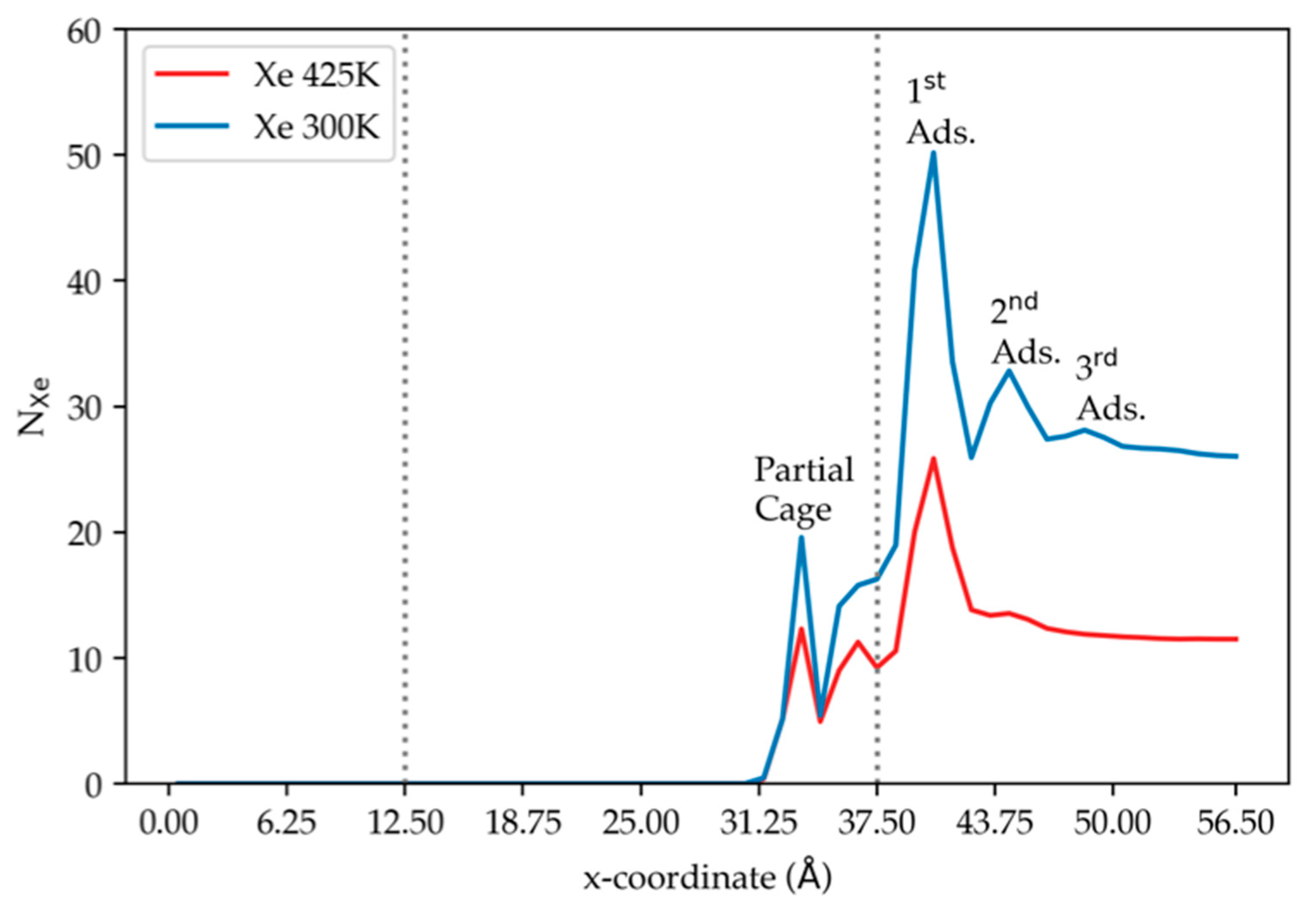
3.1.4. Effect of Temperature on Pure Xe Permeation
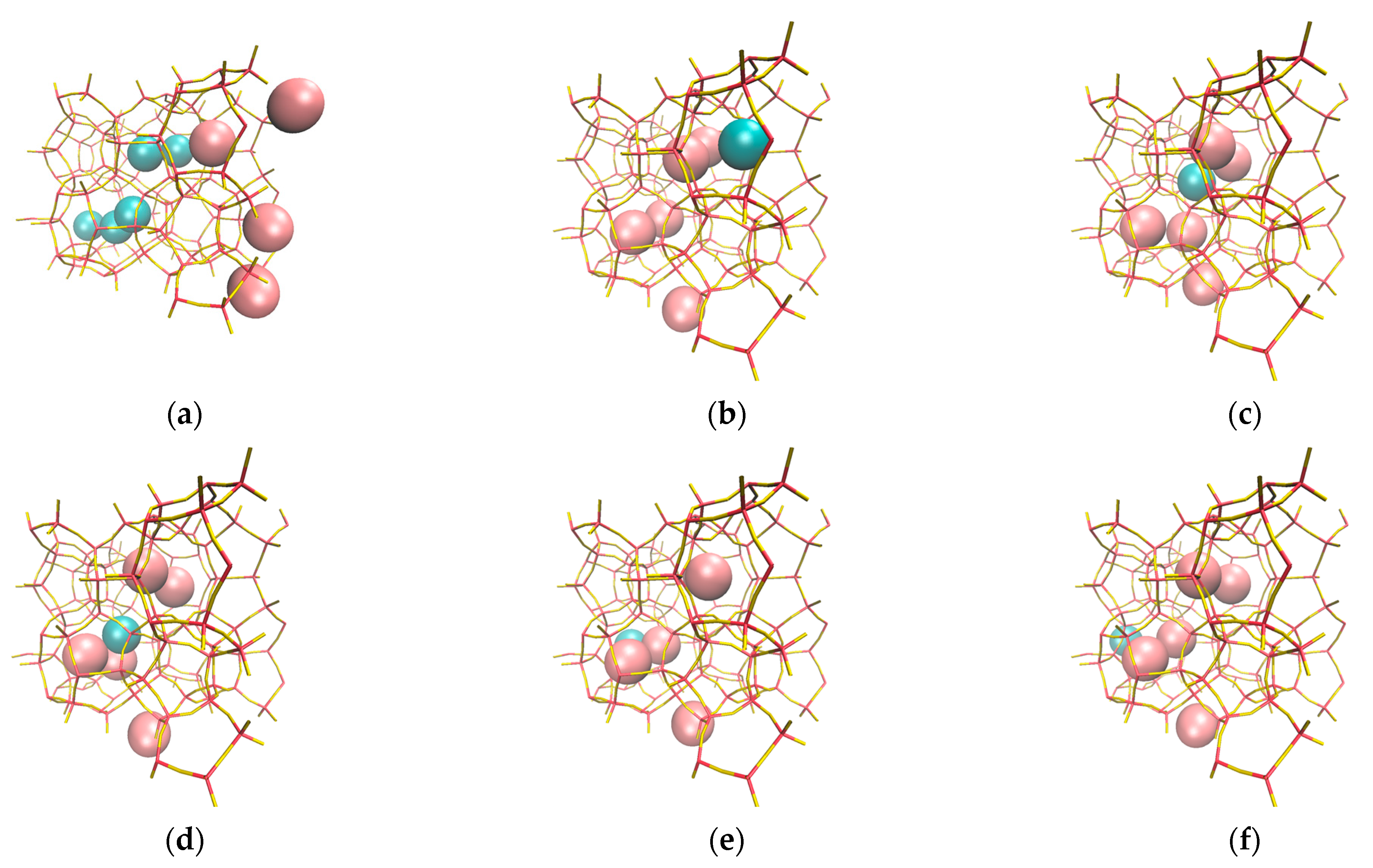
3.2. Mixture Gases
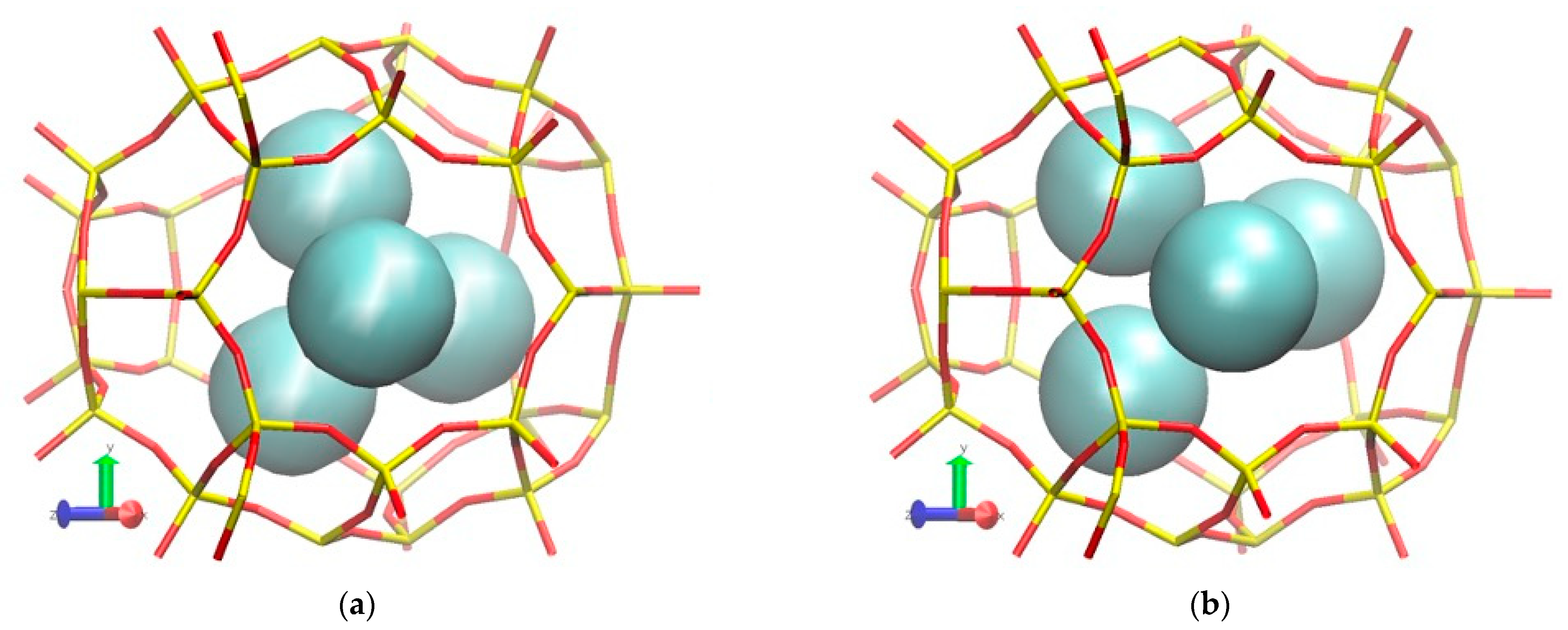
3.2.1. Kr Permeation in Kr–Xe Mixture
3.2.2. Xe Permeation in Kr–Xe Mixture
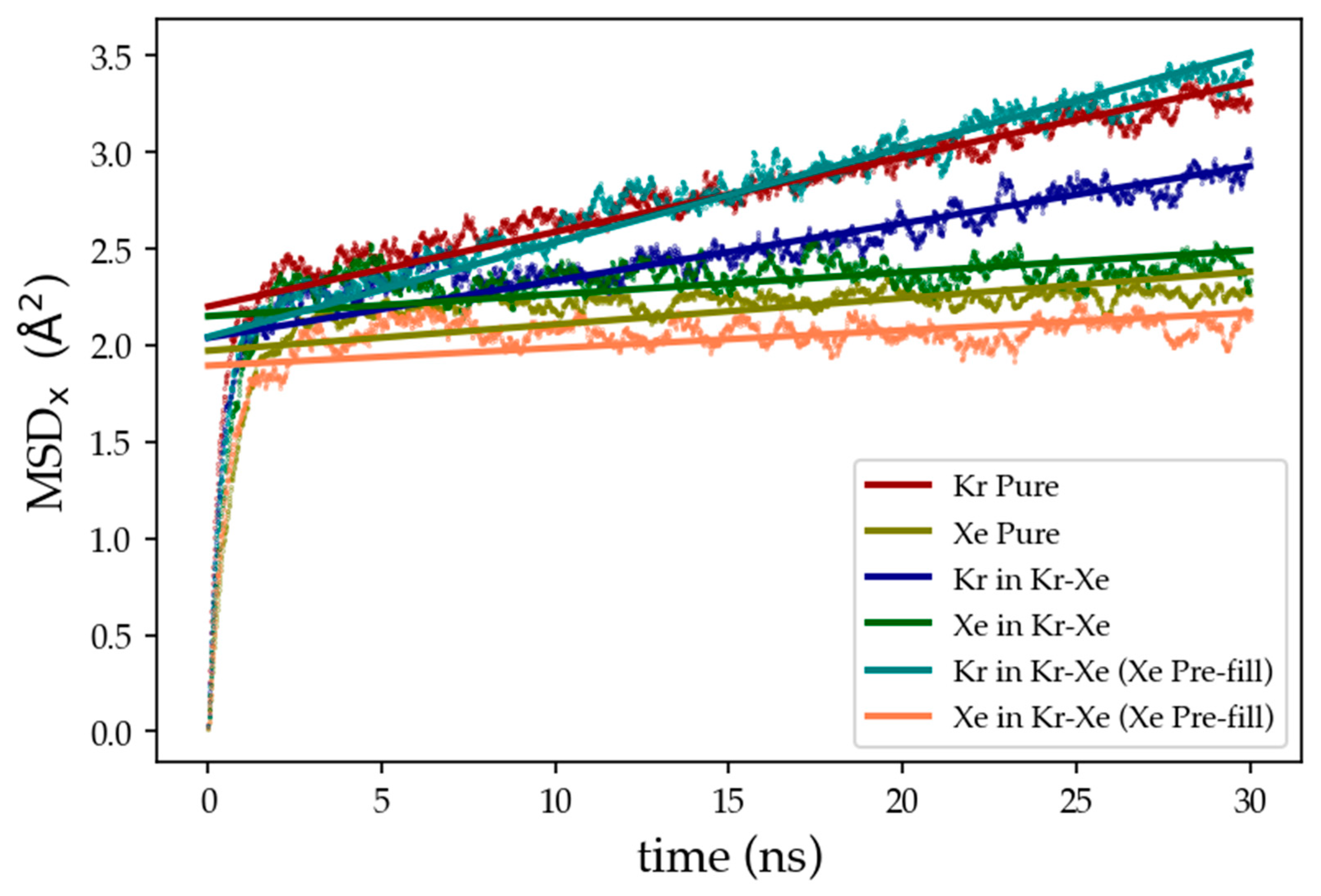
3.3. MSD Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jameson, C.J.; Jameson, A.K.; Lim, H.-M. Competitive Adsorption of Xenon and Krypton in Zeolite NaA: 129Xe Nuclear Magnetic Resonance Studies and Grand Canonical Monte Carlo Simulations. J. Chem. Phys. 1997, 107, 4364–4372. [Google Scholar] [CrossRef]
- Wang, X.; Zhou, T.; Zhang, P.; Yan, W.; Li, Y.; Peng, L.; Veerman, D.; Shi, M.; Gu, X.; Kapteijn, F. High-Silica CHA Zeolite Membrane with Ultra-High Selectivity and Irradiation Stability for Krypton/Xenon Separation. Angew. Chem. Int. Ed. 2021, 60, 9032–9037. [Google Scholar] [CrossRef]
- Wang, X.; (Nanjing Tech University, Nanjing, China). Personal communication, 2020.
- Wang, X.; Zhang, Y.; Wang, X.; Andres-Garcia, E.; Du, P.; Giordano, L.; Wang, L.; Hong, Z.; Gu, X.; Murad, S.; et al. Xenon Recovery by DD3R Zeolite Membranes: Application in Anaesthetics. Angew. Chem. Int. Ed. 2019, 58, 15518–15525. [Google Scholar] [CrossRef] [PubMed]
- van de Graaf, J.M.; Kapteijn, F.; Moulijn, J.A. Methodological and Operational Aspects of Permeation Measurements on Silicalite-1 Membranes. J. Membr. Sci. 1998, 144, 87–104. [Google Scholar] [CrossRef]
- van den Bergh, J.; Zhu, W.; Gascon, J.; Moulijn, J.A.; Kapteijn, F. Separation and Permeation Characteristics of a DD3R Zeolite Membrane. J. Membr. Sci. 2008, 316, 35–45. [Google Scholar] [CrossRef]
- Martínez, L.; Andrade, R.; Birgin, E.G.; Martínez, J.M. PACKMOL: A package for building initial configurations for molecular dynamics simulations. J. Comput. Chem. 2009, 30, 2157–2164. [Google Scholar] [CrossRef] [PubMed]
- Baerlocher, C.; McCusker, L.B. Database of Zeolite Structures. Available online: http://www.iza-structure.org/databases/ (accessed on 8 October 2021).
- Murad, S.; Jia, W.; Krishnamurthy, M. Ion-Exchange of Monovalent and Bivalent Cations with NaA Zeolite Membranes: A Molecular Dynamics Study. Mol. Phys. 2004, 102, 2103–2112. [Google Scholar] [CrossRef]
- Qu, F.; Shi, R.; Peng, L.; Zhang, Y.; Gu, X.; Wang, X.; Murad, S. Understanding the Effect of Zeolite Crystal Expansion/Contraction on Separation Performance of NaA Zeolite Membrane: A Combined Experimental and Molecular Simulation Study. J. Membr. Sci. 2017, 539, 14–23. [Google Scholar] [CrossRef]
- Hinkle, K.R.; Wang, X.; Gu, X.; Jameson, C.J.; Murad, S. Computational Molecular Modeling of Transport Processes in Nanoporous Membranes. Processes 2018, 6, 124. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.; Cheng, Y.; Peng, L.; Zhang, C.; Wu, Z.; Gu, X.; Wang, X.; Murad, S. Fabrication and Stability Exploration of Hollow Fiber Mordenite Zeolite Membranes for Isopropanol/Water Mixture Separation. Microporous Mesoporous Mater. 2019, 274, 347–355. [Google Scholar] [CrossRef]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Michael Brown, W.; Crozier, P.S.; in’t Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS—A Flexible Simulation Tool for Particle-Based Materials Modeling at the Atomic, Meso, and Continuum Scales. Comput. Phys. Commun. 2021, 271, 108171. [Google Scholar] [CrossRef]
- Momma, K.; Izumi, F. VESTA: A Three-Dimensional Visualization System for Electronic and Structural Analysis. J. Appl. Crystallogr. 2008, 41, 653–658. [Google Scholar] [CrossRef]
- Humphrey, W.; Dalke, A.; Schulten, K. VMD: Visual Molecular Dynamics. J. Mol. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef]
- Parkes, M.V.; Demir, H.; Teich-McGoldrick, S.L.; Sholl, D.S.; Greathouse, J.A.; Allendorf, M.D. Molecular Dynamics Simulation of Framework Flexibility Effects on Noble Gas Diffusion in HKUST-1 and ZIF-8. Microporous Mesoporous Mater. 2014, 194, 190–199. [Google Scholar] [CrossRef]
- Kitani, S.; Takada, J. Adsorption of Krypton and Xenon on Various Adsorbents. J. Nucl. Sci. Technol. 1965, 2, 51–56. [Google Scholar] [CrossRef]
- Guillot, B.; Sator, N. Noble Gases in High-Pressure Silicate Liquids: A Computer Simulation Study. Geochim. Cosmochim. Acta 2012, 80, 51–69. [Google Scholar] [CrossRef] [Green Version]
- Fatriansyah, J.F.; Dhaneswara, D.; Kuskendrianto, F.R.; Abdurrahman, M.H.; Yusuf, M.B.; Abdillah, F.A. The Effect of Temperature and Pressure on Nitrogen Adsorption in Amorphous Silica. J. Phys. Conf. Ser. 2021, 2070, 012045. [Google Scholar] [CrossRef]
- Tomita, T.; Nakayama, K.; Sakai, H. Gas Separation Characteristics of DDR Type Zeolite Membrane. Microporous Mesoporous Mater. 2004, 68, 71–75. [Google Scholar] [CrossRef]
- Auerbach, S.M.; Carrado, K.A.; Dutta, P.K. Handbook of Zeolite Science and Technology; CRC Press: Boca Raton, FL, USA, 2003; ISBN 978-0-203-91116-7. [Google Scholar]
- Shang, J.; Li, G.; Singh, R.; Xiao, P.; Liu, J.Z.; Webley, P.A. Determination of Composition Range for “Molecular Trapdoor” Effect in Chabazite Zeolite. J. Phys. Chem. C 2013, 117, 12841–12847. [Google Scholar] [CrossRef]
- Lito, P.F.; Santiago, A.S.; Cardoso, S.P.; Figueiredo, B.R.; Silva, C.M. New Expressions for Single and Binary Permeation through Zeolite Membranes for Different Isotherm Models. J. Membr. Sci. 2011, 367, 21–32. [Google Scholar] [CrossRef]
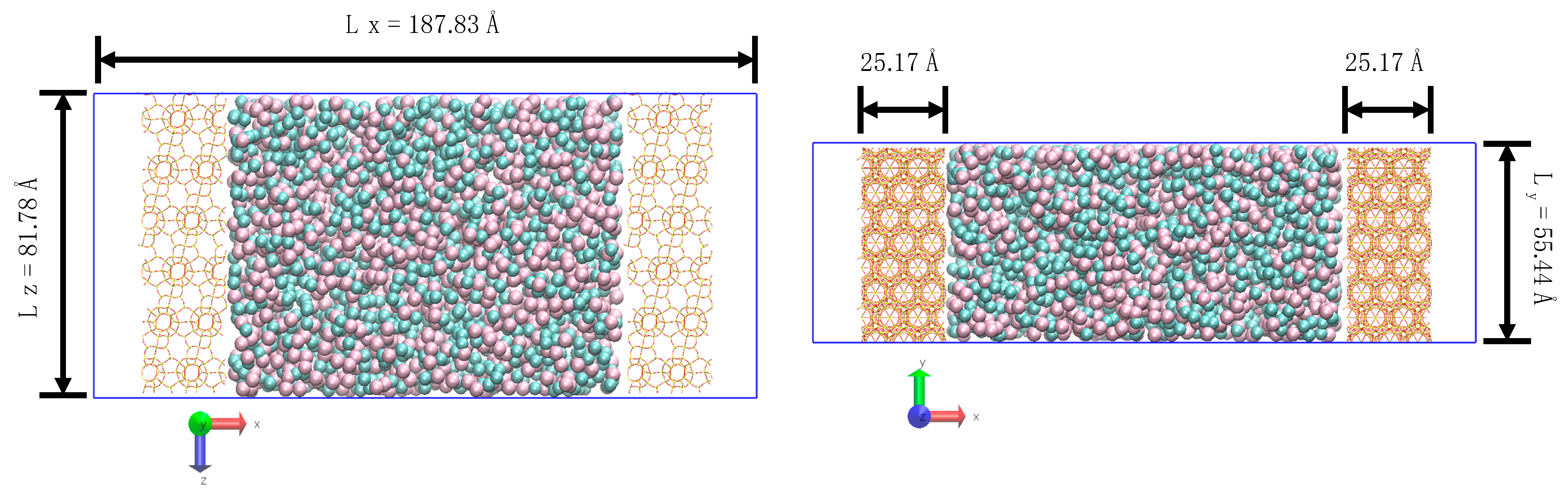
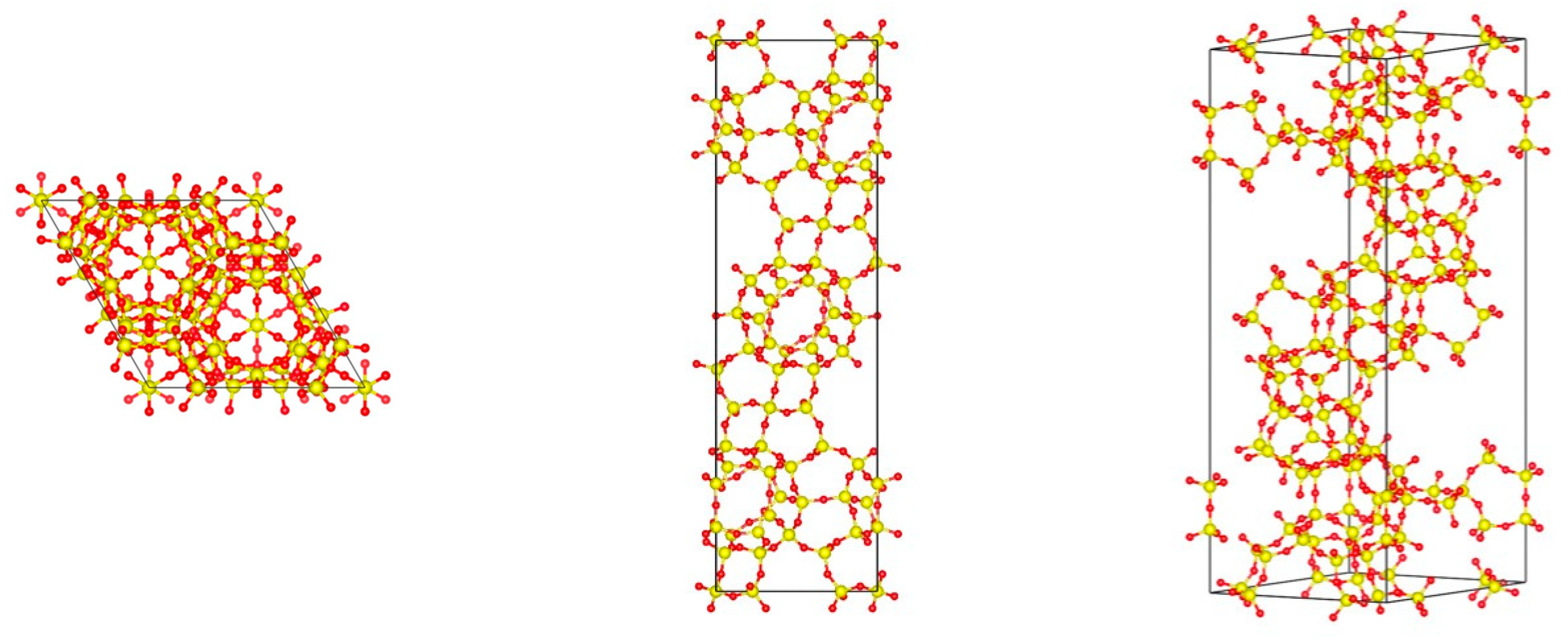




| Pair | ε (kcal/mol) | σ (Å) | Reference |
|---|---|---|---|
| Si–Si (zeolite) | 0.0010 | 1.000 | [4] |
| O–O (zeolite) | 0.1898 | 3.000 | [4] |
| Kr–Kr (gas) | 0.3380 | 3.690 | [16] |
| Xe–Xe (gas) | 0.4190 | 4.100 | [16] |
| Gas Composition | No. | Pressure | Temperature | Feed Region | Membrane Region | Complete Permeation |
|---|---|---|---|---|---|---|
| Pure Kr | (1) | 75 atm | 300 K | 0.7302 (878) | 0.2595 (312) | 0.0138 (17) |
| (2) | 150 atm | 300 K | 0.8225 (1977) | 0.1650 (396) | 0.0116 (28) | |
| (3) | 150 atm | 425 K | 0.7940 (1081) | 0.1684 (229) | 0.0369 (50) | |
| Pure Xe | (4) | 75 atm | 300 K | 0.9478 (1442) | 0.0518 (155) | |
| (5) | 150 atm | 300 K | 0.9019 (3030) | 0.0972 (166) | ||
| (6) | 150 atm | 425 K | 0.9232 (1364) | 0.0762 (113) | ||
| Kr in Kr–Xe Mixture | (7) | 150 atm | 300 K | 0.8512 (1198) | 0.1394 (196) | 0.0088 (12) |
| Xe in Kr–Xe Mixture | (7) | 150 atm | 300 K | 0.9188 (1293) | 0.0804 (113) | |
| Kr in Kr–Xe Mixture (Xe Pre-filled DD3R) | (8) | 150 atm | 300 K | 0.8916 (1255) | 0.0838 (118) | 0.0240 (34) |
| Xe in Kr–Xe Mixture (Xe Pre-filled DD3R) | (8) | 150 atm | 300 K | 0.9189 (1294) | 0.0803 (113) |
| Simulation | DD3R Cages | Diffusion Coefficient |
|---|---|---|
| Pure Kr | Empty | 1.932 × 10−13 m2 s−1 |
| Kr in Kr–Xe Mixture | Empty | 1.481 × 10−13 m2 s−1 |
| Kr in Kr–Xe Mixture | Xe Pre-filled | 2.450 × 10−13 m2 s−1 |
| Pure Xe | Empty | 6.809 × 10−14 m2 s−1 |
| Xe in Kr–Xe Mixture | Empty | 5.674 × 10−14 m2 s−1 |
| Xe in Kr–Xe Mixture | Xe Pre-filled | 4.567 × 10−14 m2 s−1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bashmmakh, B.J.; Wang, X.; Jameson, C.J.; Murad, S. Understanding Separation Mechanisms of Monoatomic Gases, Such as Kr and Xe, via DD3R Zeolite Membrane Using Molecular Dynamics. Thermo 2022, 2, 56-73. https://doi.org/10.3390/thermo2010005
Bashmmakh BJ, Wang X, Jameson CJ, Murad S. Understanding Separation Mechanisms of Monoatomic Gases, Such as Kr and Xe, via DD3R Zeolite Membrane Using Molecular Dynamics. Thermo. 2022; 2(1):56-73. https://doi.org/10.3390/thermo2010005
Chicago/Turabian StyleBashmmakh, Bandar J., Xiaoyu Wang, Cynthia J. Jameson, and Sohail Murad. 2022. "Understanding Separation Mechanisms of Monoatomic Gases, Such as Kr and Xe, via DD3R Zeolite Membrane Using Molecular Dynamics" Thermo 2, no. 1: 56-73. https://doi.org/10.3390/thermo2010005
APA StyleBashmmakh, B. J., Wang, X., Jameson, C. J., & Murad, S. (2022). Understanding Separation Mechanisms of Monoatomic Gases, Such as Kr and Xe, via DD3R Zeolite Membrane Using Molecular Dynamics. Thermo, 2(1), 56-73. https://doi.org/10.3390/thermo2010005






