Spectral Optical Properties of Rabbit Brain Cortex between 200 and 1000 nm
Abstract
1. Introduction
2. Materials and Methods
2.1. Tissue Collection and Sample Preparation
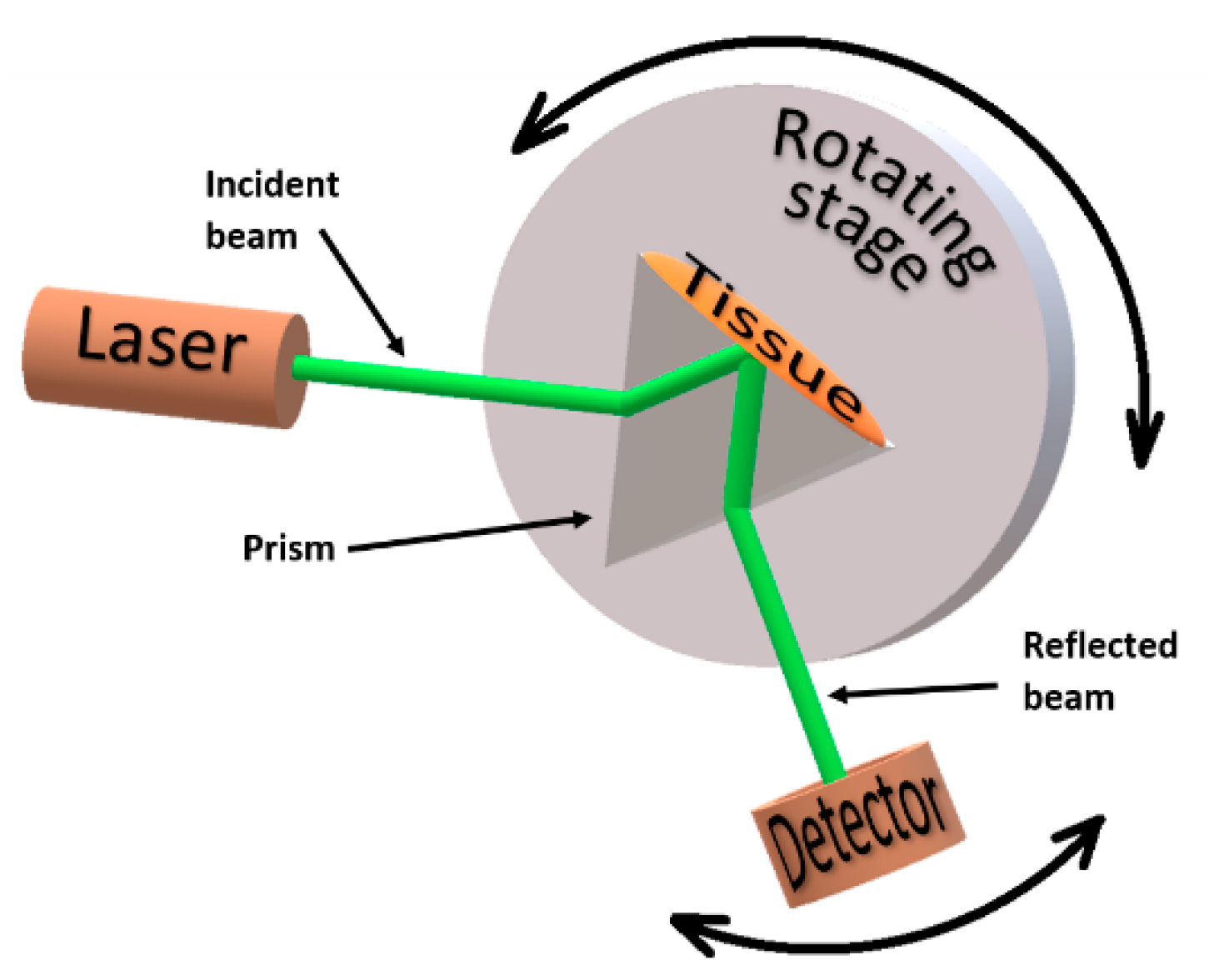
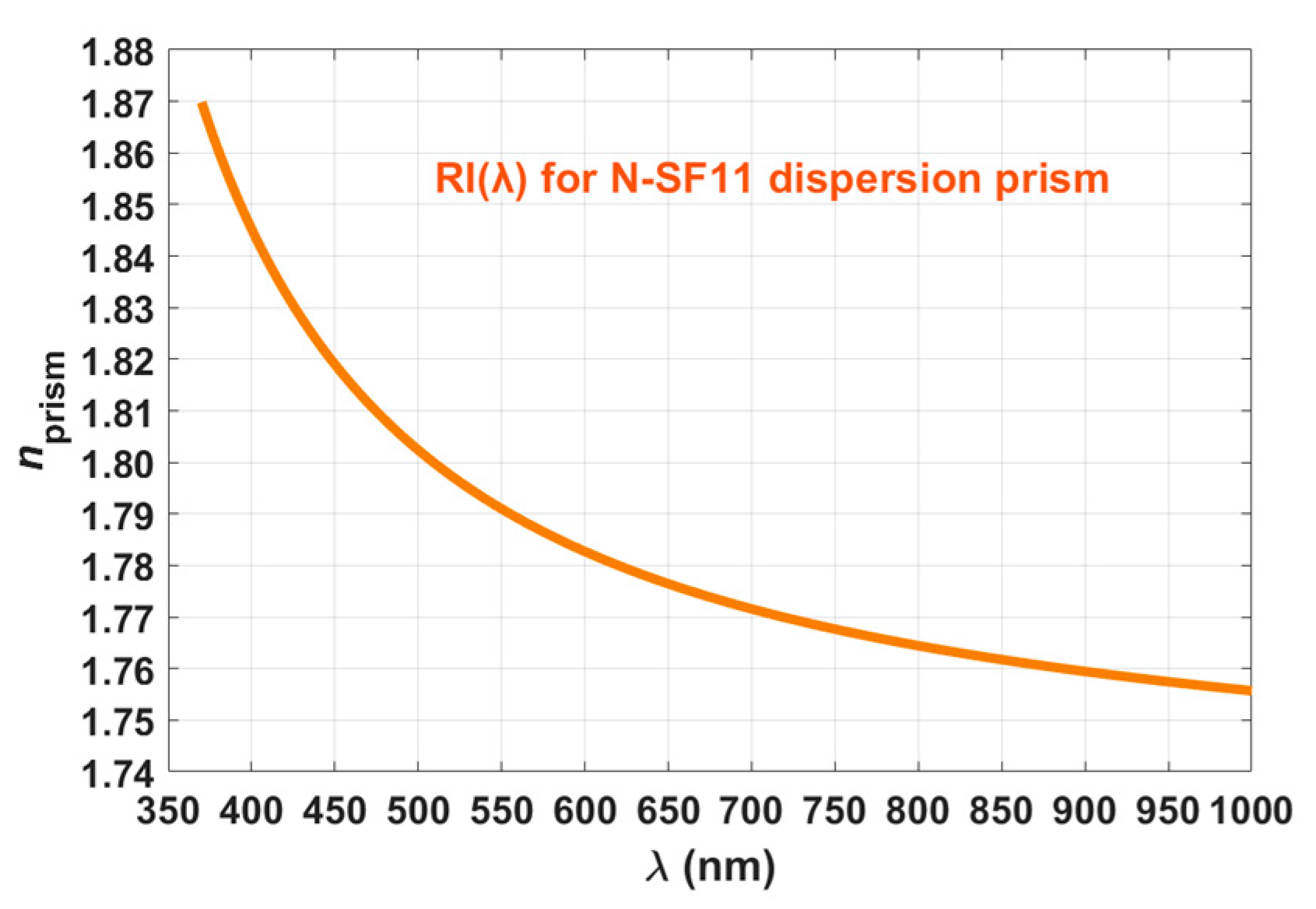
2.2. RI Measurements
- The sample was placed in perfect contact with the base of the prism (see Figure 1).
- Illumination of the setup was made with the laser beam through one side of the prism.
- The reflected beam was collected with a photocell (a laser power meter from Coherent with spectral resolution from 0.15 μm to 11 μm), connected to a voltmeter (from Wavetek Meterman) to read the electrical potential.
- This measuring procedure was repeated for several incidence angles (α) between the incident laser beam and the normal to the air/prism interface. The angular resolution for these measurements was 1°.
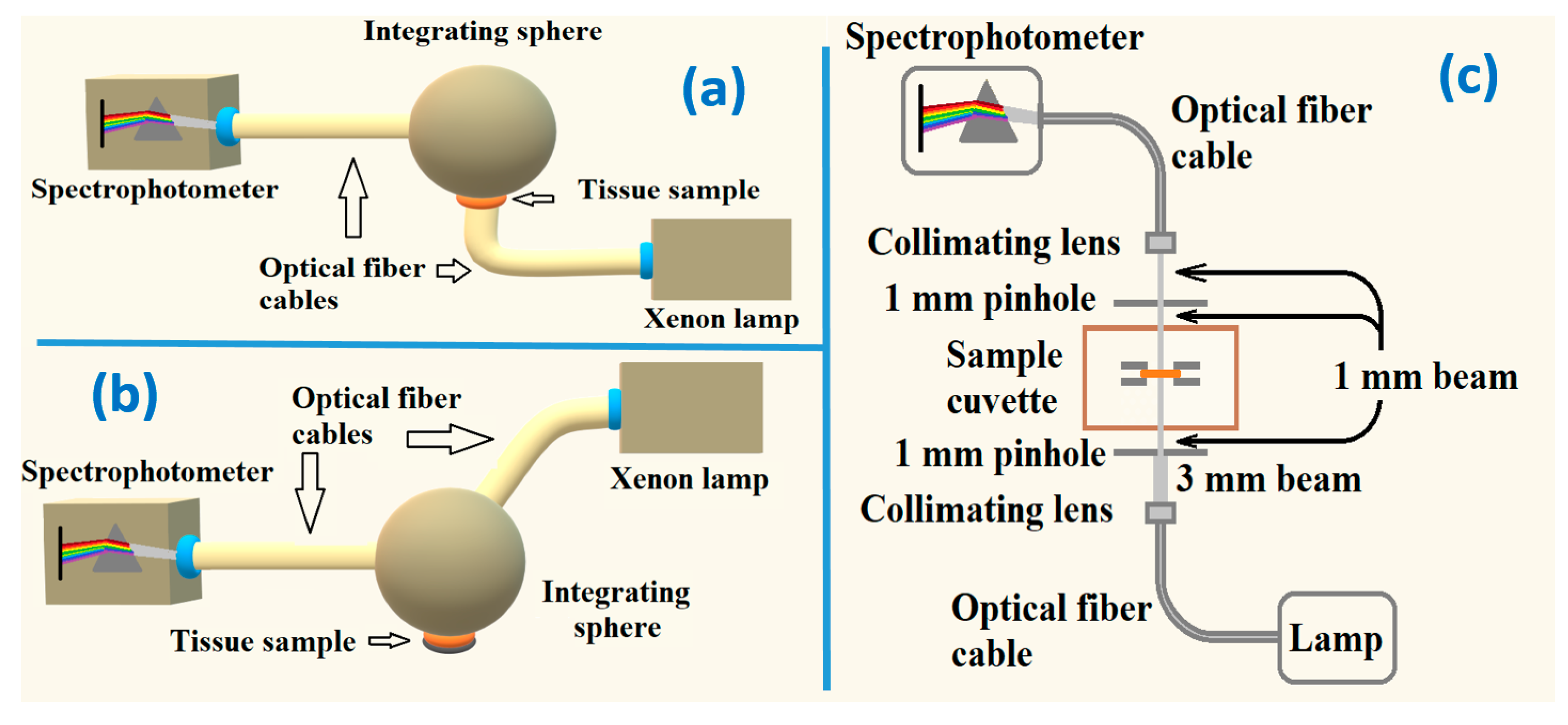
2.3. Spectral Measurements
2.4. Calculations
3. Results
3.1. RI Measurements
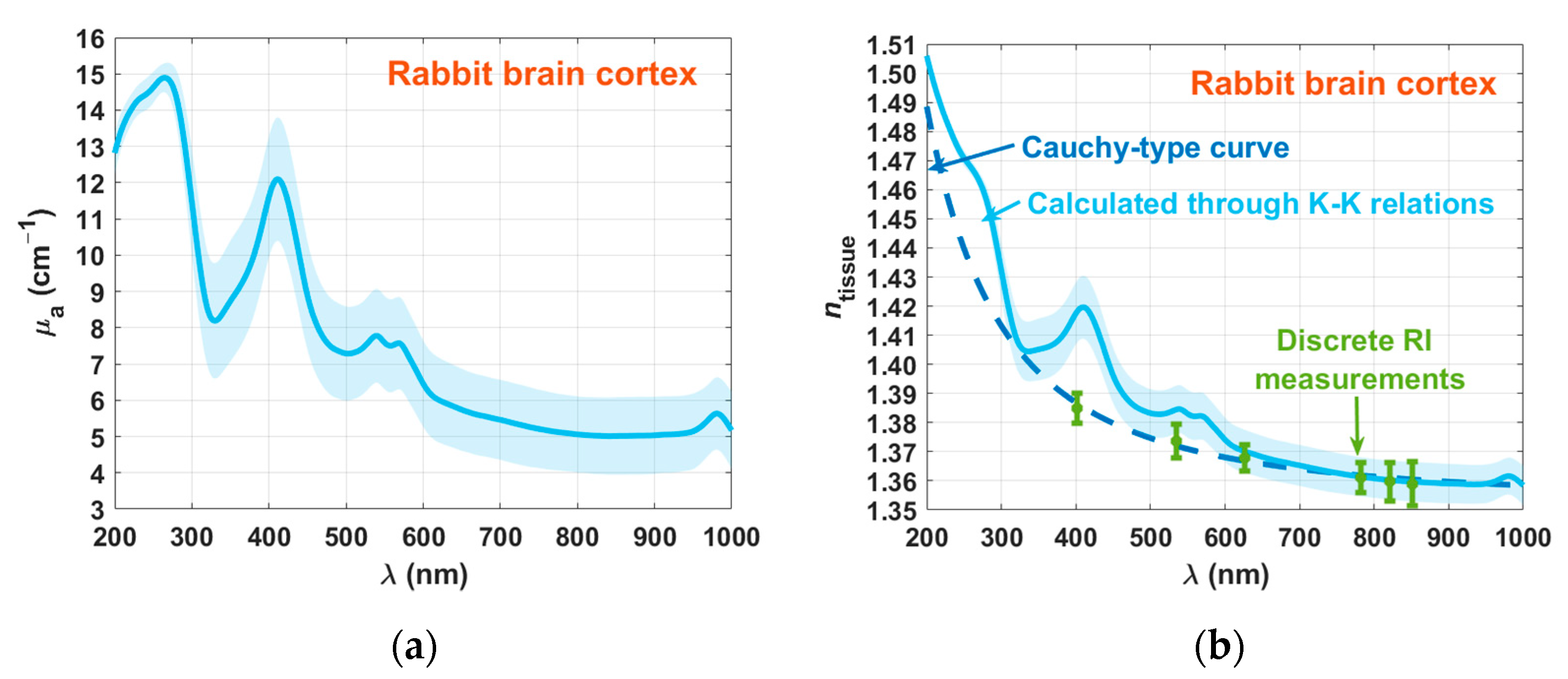
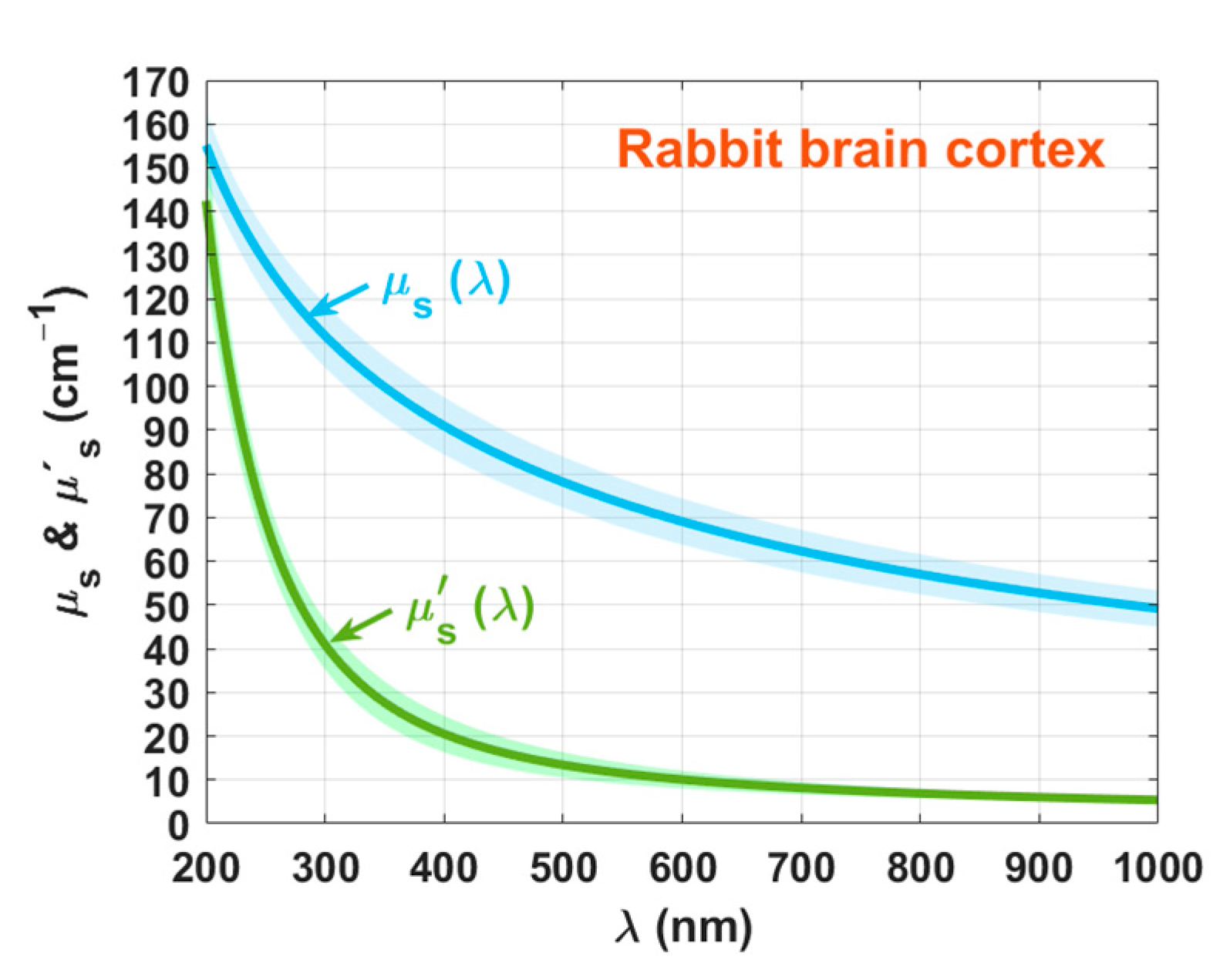
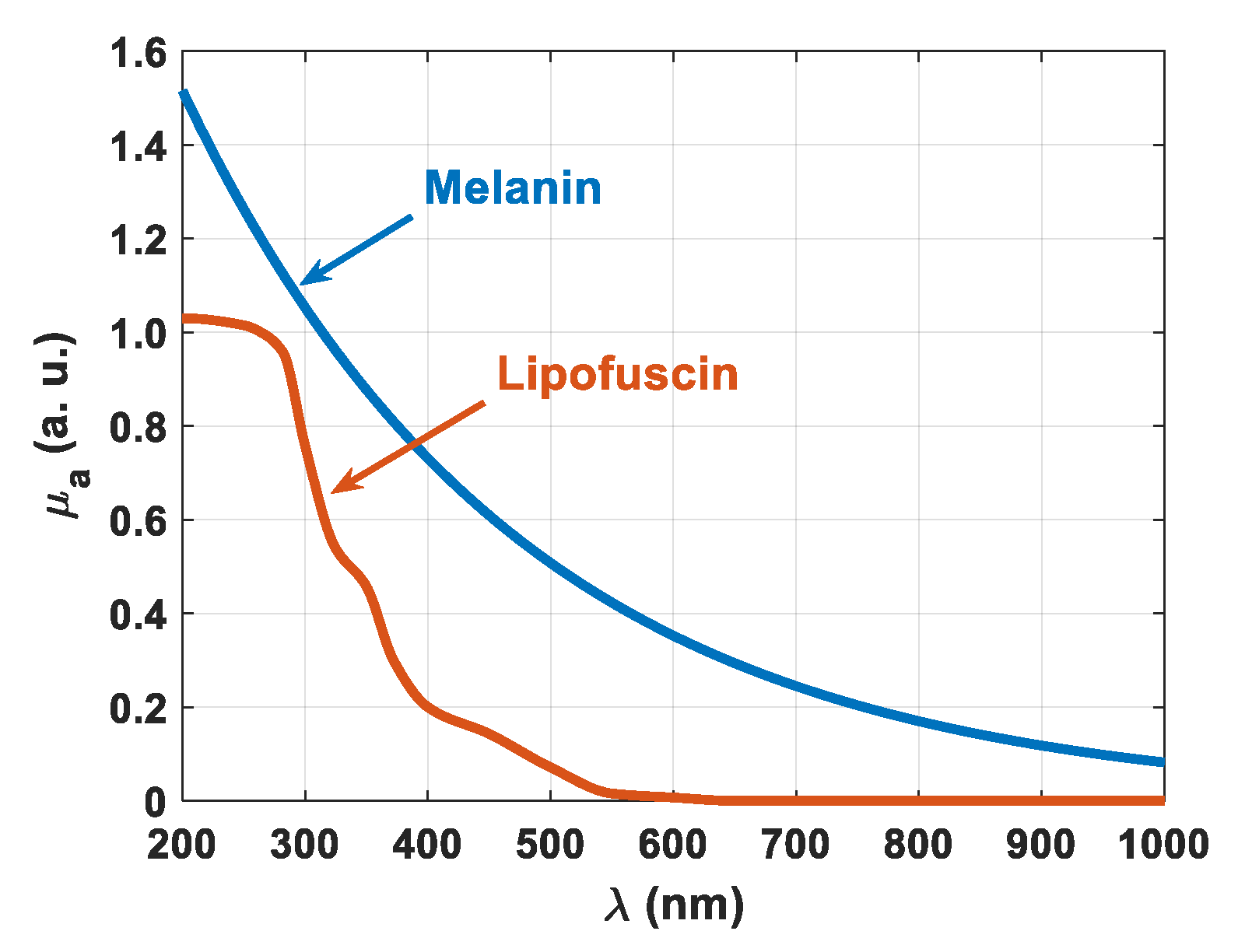
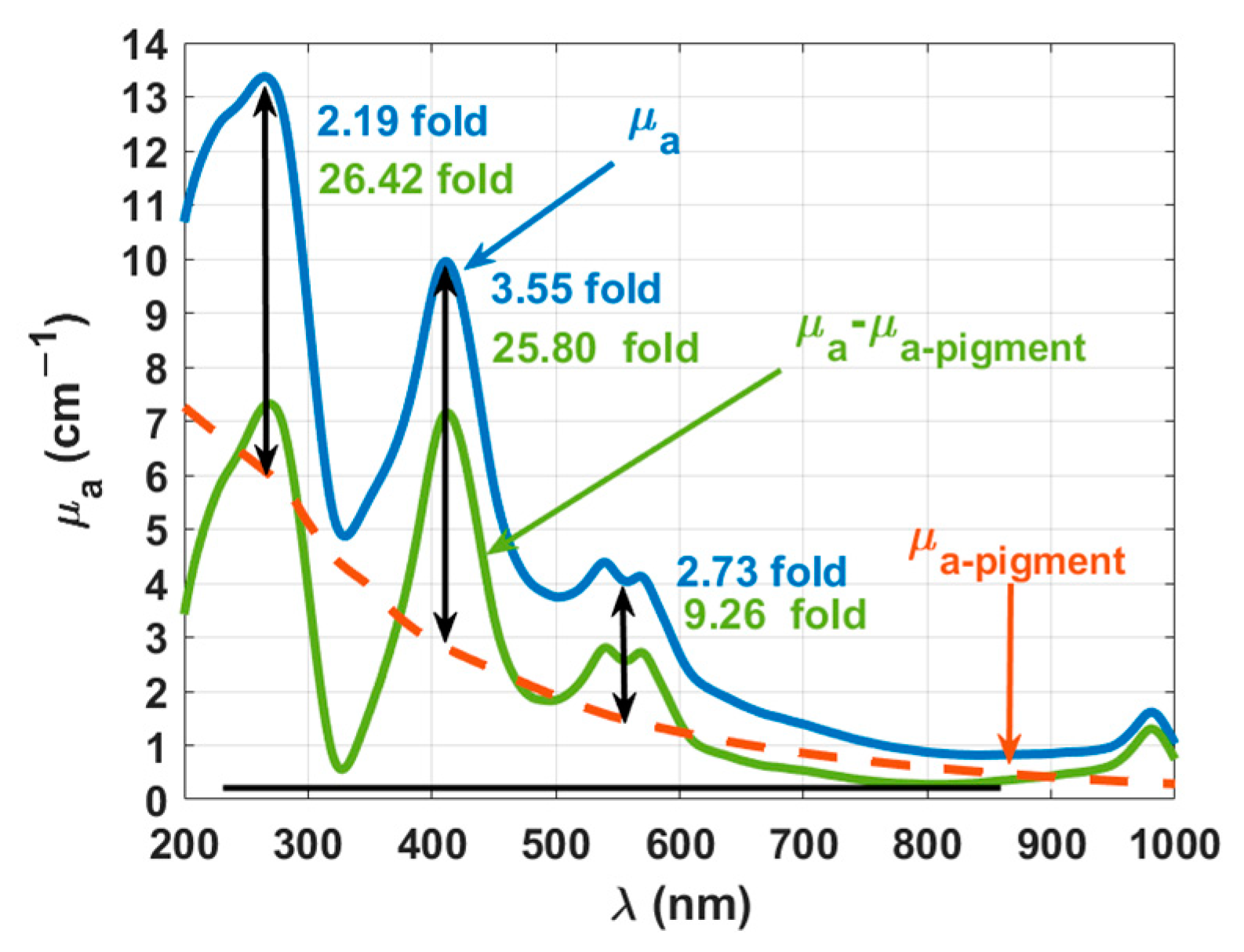
3.2. Spectral Measurements and Calculated Spectral Optical Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Carvalho, S.; Carneiro, I.; Henrique, R.; Tuchin, V.V.; Oliveira, L. Lipofuscin-type pigment as a marker of colorectal cancer. Electronics 2020, 9, 1805. [Google Scholar] [CrossRef]
- Oliveira, L.M.; Tuchin, V.V. The Optical Clearing Method—A New Tool for Clinical Practice and Biomedical Engineering; Springer: Cham, Switzerland, 2019; pp. 1–106. [Google Scholar]
- Tuchin, V.V. Tissue Optics: Light Scattering Methods and Instruments for Medical Diagnostics, 3rd ed.; SPIE Press: Bellingham, WA, USA, 2015; pp. 245–358. [Google Scholar]
- Wang, L.; Jacques, S.L.; Zheng, L. MCML-Monte Carlo modeling of light transport in multi-layered tissues. Comp. Method Prog. Biomed. 1995, 47, 131–146. [Google Scholar] [CrossRef]
- Prahl, S.A.; van Gemert, M.J.C.; Welch, A.J. Determining the optical properties of turbid media by using the adding-doubling method. Appl. Opt. 1993, 32, 559–568. [Google Scholar] [CrossRef]
- Sordillo, D.C.; Sordillo, L.A.; Sordillo, P.P.; Shi, L.; Alfano, R.R. Short wavelength infrared optical windows for evaluation of benign and malignant tissues. J. Biomed. Opt. 2017, 22, 45002. [Google Scholar] [CrossRef]
- Shi, L.; Sordillo, L.A.; Rodríguez-Contreras, A.; Alfano, R. Transmission in near-infrared optical Windows for deep-brain imaging. J. Biophotonics 2016, 9, 38–43. [Google Scholar] [CrossRef]
- Oliveira, L.M.; Zaytsev, K.I.; Tuchin, V.V. Improved biomedical imaging over a wide spectral range from UV to THz towards multimodality. In Proceedings of the Third International Conference on Biophotonics Riga 2020, Riga, Latvia, 24–25 August 2020; Spigulis, J., Ed.; SPIE: Bellingham, WA, USA, 2020; Volume 11585, p. 11585. [Google Scholar]
- Carneiro, I.; Carvalho, S.; Henrique, R.; Oliveira, L.; Tuchin, V. Measurement of optical properties of normal and pathological human liver tissue from deep-UV to NIR. In Proceedings of the Photonics Europe: Tissue Optics and Photonics, Online Conference, 2 April 2020; Tuchin, V.V., Blondel, W., Zalevsky, Z., Eds.; SPIE: Bellingham, WA, USA, 2020; Volume 11363, p. 113630G. [Google Scholar]
- Backman, B.; Wax, A.; Zhang, H. A Laboratory Manual in Biophotonics; CRC Press: Boca Raton, FL, USA, 2018; p. 100. [Google Scholar]
- Jacques, S.L. Optical properties of biological tissues: A review. Phys. Med. Biol. 2013, 58, R37–R61. [Google Scholar] [CrossRef]
- Yanina, I.Y.; Lazareva, E.N.; Tuchin, V.V. Refractive index of adipose tissue and lipid droplet measured in wide spectral and temperature ranges. Appl. Opt. 2018, 57, 4839. [Google Scholar] [CrossRef]
- Martins, I.; Silva, H.; Tuchin, V.V.; Oliveira, L. Estimation of rabbit pancreas dispersion between 400 and 1000 nm. J. Biomed. Phot. Eng. 2021, 7, 020303. [Google Scholar]
- Lazareva, E.N.; Oliveira, L.; Yanina, I.Y.; Chernomyrdin, N.V.; Musina, G.R.; Tuchina, D.K.; Bashkatov, A.N.; Zaytsev, K.I.; Tuchin, V.V. Refractive index measurements of tissue and blood and OCAs in a wide spectral range. In Tissue Optical Clearing: New Prospects in Optical Imaging; Zhu, D., Genina, E., Tuchin, V., Eds.; CRC Press: Boca Raton, FL, USA, to be published.
- Carneiro, I.; Carvalho, S.; Henrique, R.; Oliveira, L.; Tuchin, V.V. Simple multimodal optical technique for evaluation of free/bound water and dispersion of human liver tissue. J. Biomed. Opt. 2017, 22, 125002. [Google Scholar] [CrossRef]
- Sydoruk, O.; Zhernovaya, O.; Tuchin, V.V.; Douplik, A. Refractive index of solutions of human hemoglobin from the near-infrared to the ultraviolet range: Kramers-Kronig analysis. J. Biomed. Opt. 2012, 17, 115002. [Google Scholar] [CrossRef]
- Gienger, J.; Groβ, H.; Neukammer, J.; Bär, M. Determining the refractive index of human hemoglobin solutions by Kramers-Kronig relations with an improved absorption model. Appl. Opt. 2016, 55, 8951–8961. [Google Scholar] [CrossRef]
- Bashkatov, A.N.; Genina, E.A.; Kozintseva, M.D.; Kochubei, V.I.; Gorodkov, S.Y.; Tuchin, V.V. Optical properties of peritoneal biological tissues in the spectral range of 350–2500 nm. Opt. Spectrosc. 2016, 120, 6–14. [Google Scholar] [CrossRef]
- Carvalho, S.; Gueiral, N.; Nogueira, E.; Henrique, R.; Oliveira, L.; Tuchin, V. Comparative study of the optical properties of colon mucosa and colon precancerous polyps between 400 and 1000 nm. In Proceedings of the BIOS-Photonics West 2017: Dynamics and Fluctuations in Biomedical Photonics, San Francisco, CA, USA, 28 January–2 February 2017; Tuchin, V.V., Larin, K.V., Leahy, M.J., Wang, R.K., Eds.; SPIE: Bellingham, WA, USA, 2017; Volume 10063, p. 100631L. [Google Scholar]
- Fernandes, L.; Carvalho, S.; Carneiro, I.; Henrique, R.; Tuchin, V.V.; Oliveira, H.; Oliveira, L. Diffuse reflectance and machine learning techniques to differentiate colorectal cancer ex vivo. Chaos 2021, 31, 053118. [Google Scholar] [CrossRef] [PubMed]
- GBD 2016 Neurology Collaborators. Global, regional, and national burden of neurological disorders, 1990–2016: A systematic analysis for the global burden of disease study 2016. Lancet Neurol. 2019, 18, 459–480. [Google Scholar] [CrossRef]
- Mattson, M.P.; Duan, W.; Pedersen, W.A.; Culmsee, C. Neurodegenerative disorders and ischemic brain diseases. Apoptosis 2001, 6, 69–81. [Google Scholar] [CrossRef] [PubMed]
- Double, K.L.; Dedov, V.N.; Fedorow, H.; Kettle, E.; Halliday, G.M.; Garner, B.; Brunk, U.T. The comparative biology of neuromelanin and lipofuscin in the brain. Cell Mol. Life Sci. 2008, 65, 1669–1682. [Google Scholar] [CrossRef] [PubMed]
- Moreno-García, A.; Kun, A.; Calero, M.; Calero, O. The neuromelanin paradox and its role in oxidative stress and neurodegeneration. Antioxidants 2021, 10, 124. [Google Scholar] [CrossRef]
- Tuchin, V.V. Optical Clearing of Tissues and Blood; SPIE Press: Bellingham, WA, USA, 2005; p. 79. [Google Scholar]
- Sdobnov, A.Y.; Darvin, M.; Genina, E.A.; Bashkatov, A.N.; Lademann, J.; Tuchin, V.V. Recent progress in tissue optical clearing for spectroscopic application. Spectrochim. Acta Part A Biomol. Spectrosc. 2018, 197, 216–229. [Google Scholar] [CrossRef]
- Li, H.; Xie, S. Measurement method of the refractive index of biotissue by total internal reflection. Appl. Opt. 1996, 35, 1793–1975. [Google Scholar] [CrossRef]
- Ding, H.; Lu, J.Q.; Jacobs, K.M.; Hu, X.H. Determination of refractive indices of porcine skin tissues and intralipid at eight wavelengths between 325 and 1557 nm. J. Opt. Soc. Am. A 2005, 22, 1151–1157. [Google Scholar] [CrossRef]
- Deng., Z.; Wang, J.; Ye, Q.; Sun, T.; Zhou, W.Y.; Mei, J.; Zhang, C.; Tian, J. Determination of continuous complex refractive index dispersion of biotissue based on internal reflection. J. Biomed. Opt. 2016, 21, 015004. [Google Scholar] [CrossRef][Green Version]
- Refractive Index Database. Available online: https://refractiveindex.info/ (accessed on 1 June 2021).
- Song, Q.W.; Ku, C.Y.; Zhang, C.; Gross, R.B.; Birge, R.R.; Michalak, R. Modified critical angle method for measuring the refractive index of bio-optical materials and its application to bacteriorhodopsin. J. Opt. Soc. Am. B 1995, 12, 797–803. [Google Scholar] [CrossRef]
- Carneiro, I.; Carvalho, S.; Henrique, R.; Oliveira, L.; Tuchin, V.V. Water content and scatterers dispersion evaluation in colorectal tissues. J. Biomed. Phot. Eng. 2017, 3, 040301. [Google Scholar] [CrossRef]
- Carvalho, S.; Gueiral, N.; Nogueira, E.; Henrique, R.; Oliveira, L.; Tuchin, V.V. Wavelength dependence of the refractive index of human colorectal tissues: Comparison between healthy mucosa and cancer. J. Biomed. Phot. Eng. 2016, 2, 040307. [Google Scholar] [CrossRef][Green Version]
- Bashkatov, A.N.; Genina, E.A.; Kochubey, V.I.; Tuchin, V.V. Optical properties of the subcutaneous adipose tissue in the spectral range 400–2500 nm. Opt. Spectrosc. 2005, 99, 836–842. [Google Scholar] [CrossRef]
- Roggan, A.; Dörschel, K.; Minet, O.; Wolff, D.; Müller, G. The optical properties of biological tissue in the near infrared wavelength range—Review and measurements. In Laser-Induced Interstitial Thermotherapy; Müller, G., Roggan, A., Eds.; SPIE Press: Bellingham, WA, USA, 1995; pp. 10–44. [Google Scholar]
- Binding, J.; Arous, J.B.; Léger, J.F.; Gigan, S.; Boccara, C.; Bourdieu, L. Brain refractive index measured in vivo with high-NA defocus-corrected full-field OCT and consequences for two-photon microscopy. Opt. Express 2011, 19, 4833–4847. [Google Scholar] [CrossRef]
- Sun, J.; Lee, S.J.; Wu, L.; Sarntinoranont, M.; Xie, H. Refractive index measurement of acute rat brain tissue slices using optical coherence tomography. Opt. Express 2012, 20, 1084–1095. [Google Scholar] [CrossRef]
- Fasman, G.D. Ultraviolet spectra of derivatives of cysteine, cysteine, histidine, phenylalanine, tyrosine, and tryptophan. In Handbook of Biochemistry and Molecular Biology, 3rd ed.; Fasman, G.D., Ed.; CRC Press: Boca Raton, FL, USA, 2018; Volume 1, Chapter 17. [Google Scholar]
- Wetlaufer, D.B. Ultraviolet spectra of proteins and amino acids. In Advances in Protein Chemistry; Afinsen, C.B., Jr., Ed.; Academic Press: London, UK, 1962; Chapter 6; Volume 17. [Google Scholar]
- Narayan, R.K.; Heydon, W.E.; Creed, G.J.; Jacobowitz, D.M. Identification of major proteins in human cerebral cortex and brain tumors. J. Protein Chem. 1985, 4, 375–389. [Google Scholar] [CrossRef]
- Zhou, Y.; Yao, J.; Wang, L.V. Tutorial on photoacoustic tomography. J. Biomed. Opt. 2016, 21, 061007. [Google Scholar] [CrossRef]
- Pitzschke, A.; Lovisa, B.; Seydoux, O.; Haenggi, M.; Oertel, M.F.; Zellweger, M.; Tardy, Y.; Wagnières, G. Optical properties of rabbit brain in the red and near-infrared: Changes observed under in vivo, postmortem, frozen, and formalin-fixated conditions. J. Biomed. Opt. 2015, 20, 025006. [Google Scholar] [CrossRef] [PubMed]
- Koeppen, A.H.; Dentinger, M.P. Brain hemosiderin and superficial siderosis of the central nervous system. J. Neuropathol. Exp. Neurol. 1988, 47, 249–270. [Google Scholar] [CrossRef]
- Granick, S. Ferritin: Its properties and significance for iron metabolism. Chem. Rev. 1946, 38, 379–403. [Google Scholar] [CrossRef] [PubMed]
- Maoka, T. Carotenoids as natural functional pigments. J. Nat. Med. 2020, 74, 1–16. [Google Scholar] [CrossRef]
- Johansson, J. Spectroscopic method for determination of the absorption coefficient in brain tissue. J. Biomed. Opt. 2010, 15, 057005. [Google Scholar] [CrossRef] [PubMed]
- Wakamatsu, K.; Murase, T.; Zucca, F.A.; Zecca, L.; Ito, S. Biosynthetic pathway to measure neuromelanin and its aging process. Pigment. Cell Melanoma Res. 2012, 25, 792–803. [Google Scholar] [CrossRef] [PubMed]
- Gilissen, E.P.; Staneva-Dobrovski, L. Distinct types of lipofuscin pigment in the hippocampus and cerebellum of aged cheirogaleid primates. Anat. Rec. 2013, 296, 1895–1906. [Google Scholar] [CrossRef]
- Heinsen, H. Lipofuscin in the cerebellar cortex of albino rats: An electron microscopic study. Anat. Embryol. 1979, 115, 333–345. [Google Scholar] [CrossRef]
- Ivy, G.O.; Kanai, S.; Ohta, M.; Smith, G.; Sato, Y.; Kobayashi, M.; Kitani, K. Lipofucin-like substances accumulate rapidly in brain, retina and internal organs with cysteine protease inhibition. Adv. Exp. Med. Biol. 1989, 266, 31–45. [Google Scholar]
- Johansson, J.D.; Wårdell, K. Intracerebral quantitative chromophore estimation from reflectance spectra captured during deep brain stimulation implantation. J. Biophot. 2013, 6, 435–445. [Google Scholar] [CrossRef] [PubMed]
- Różanowska, M.B.; Pawlak, A.; Różanowski, B. Products of docosahexaenoate oxidation as contributors to photosensitizing properties of retinal lipofuscin. Int. J. Mol. Sci. 2021, 22, 3525. [Google Scholar] [CrossRef]
- Zonios, G.; Dimou, A.; Bassukas, I.; Galaris, D.; Tsolakidis, A.; Kaxiras, E. Melanin absorption spectroscopy: New method for noninvasive skin investigation and melanoma detection. J. Biomed. Opt. 2008, 13, 014017. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Zou, X.; Zhang, B.; Cui, L.; Zhang, J.; Mao, Y.; Chen, L.; Ji, M. Label-free imaging of hemoglobin degradation and hemosiderin formation in brain tissues with femtosecond pump-probe microscopy. Theranostics 2018, 8, 4129–4140. [Google Scholar] [CrossRef] [PubMed]
- Zaghdoudi, K.; Ngomo, O.; Vanderesse, R.; Anoux, P.; Myrzakhmetov, B.; Frochot, C.; Guiavarch, Y. Extraction, identification and photo-physical characterization of persimmon (Diospyros kaki L.) carotenoids. Foods 2017, 6, 4. [Google Scholar] [CrossRef] [PubMed]
- Raja, A.S.; Sathiyabama, J.; Venkatesan, R.; Prathipa, V. Corrosion control of carbon steel by eco-friendly inhibitor L-Cysteine-Zn2+ system in acqueous medium. J. Chem. Biol. Phys. Sci. 2014, 4, 3182–3189. [Google Scholar]
- Hazra, C.; Samanta, T.; Mahalingam, V. A resonance energy transfer approach for the selective detection of aromatic amino acids. J. Mater. Chem. C 2014, 2, 10157–10163. [Google Scholar] [CrossRef]
- Lin, J.-H.; Yu, C.-J.; Yang, Y.-C.; Tseng, W.-L. Formation of fluorescence polydopamine dots from hydroxyl radical-induced degradation of polydopamine nanoparticles. Phys. Chem. Chem. Phys. 2015, 17, 15124. [Google Scholar] [CrossRef]
- Feng, S.; Harayama, T.; Montessuit, S.; David, F.P.; Winssinger, N.; Martinou, J.-C.; Riezman, H. Mitochondria-specific photoactivation to monitor local sphingosine metabolism and function. Elife 2018, 7, e34555. [Google Scholar] [CrossRef]
- Jung, T.; Bader, N.; Grune, T. Lipofuscin: Formation, distribution, and metabolic consequences. Ann. N. Y. Acad. Sci. 2007, 1119, 97–111. [Google Scholar] [CrossRef]
- Katz, M.L.; Robinson, W.G., Jr. What is lipofuscin? Defining characteristics and differentiation from other autofluorescent lysosomal storage bodies. Arch. Gerontol. Geriatr. 2002, 34, 169–184. [Google Scholar] [CrossRef]
- Höhn, A.; Jung, T.; Grimm, S.; Grune, T. Lipofuscin-bound iron is a major intracellular source of oxidants: Role in senescent cells. Free Rad. Biol. Med. 2010, 48, 1100–1108. [Google Scholar] [CrossRef]
- Hunter, J.J.; Morgan, J.I.W.; Merigan, W.H.; Sliney, D.H.; Sparrow, J.R.; Williams, D.R. The susceptibility of the retina to photochemical damage from visible light. Prog. Retin Eye Res. 2012, 31, 28–42. [Google Scholar] [CrossRef]
- Gosnell, M.E.; Anwer, A.G.; Cassano, J.C.; Sue, C.M.; Goldys, E.M. Functional hyperspectral imaging captures subtle details of cell metabolism in olfactory neurosphere cells, disease-specific models of neurodegenerative disorders. Biochim. Biophys. Acta 2016, 1863, 56–63. [Google Scholar] [CrossRef]





| Laser | ntissue | Mean | SD |
|---|---|---|---|
| 1.3883 | |||
| 401.4 nm | 1.3877 | 1.3850 | 0.0053 |
| 1.3789 | |||
| 1.3679 | |||
| 534.6 nm | 1.3735 | 1.3736 | 0.0058 |
| 1.3794 | |||
| 1.3632 | |||
| 626.6 nm | 1.3686 | 1.3680 | 0.0045 |
| 1.3721 | |||
| 1.3562 | |||
| 782.1 nm | 1.3609 | 1.3611 | 0.0050 |
| 1.3662 | |||
| 1.3552 | |||
| 820.8 nm | 1.3566 | 1.3597 | 0.0066 |
| 1.3673 | |||
| 1.3516 | |||
| 850 nm | 1.3583 | 1.3589 | 0.0076 |
| 1.3667 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gonçalves, T.M.; Martins, I.S.; Silva, H.F.; Tuchin, V.V.; Oliveira, L.M. Spectral Optical Properties of Rabbit Brain Cortex between 200 and 1000 nm. Photochem 2021, 1, 190-208. https://doi.org/10.3390/photochem1020011
Gonçalves TM, Martins IS, Silva HF, Tuchin VV, Oliveira LM. Spectral Optical Properties of Rabbit Brain Cortex between 200 and 1000 nm. Photochem. 2021; 1(2):190-208. https://doi.org/10.3390/photochem1020011
Chicago/Turabian StyleGonçalves, Tânia M., Inês S. Martins, Hugo F. Silva, Valery V. Tuchin, and Luís M. Oliveira. 2021. "Spectral Optical Properties of Rabbit Brain Cortex between 200 and 1000 nm" Photochem 1, no. 2: 190-208. https://doi.org/10.3390/photochem1020011
APA StyleGonçalves, T. M., Martins, I. S., Silva, H. F., Tuchin, V. V., & Oliveira, L. M. (2021). Spectral Optical Properties of Rabbit Brain Cortex between 200 and 1000 nm. Photochem, 1(2), 190-208. https://doi.org/10.3390/photochem1020011








