Shear Strength of Strain-Hardening Cementitious Materials
Abstract
:1. Introduction
2. Background for the Model of the Failure Envelope of SHCC
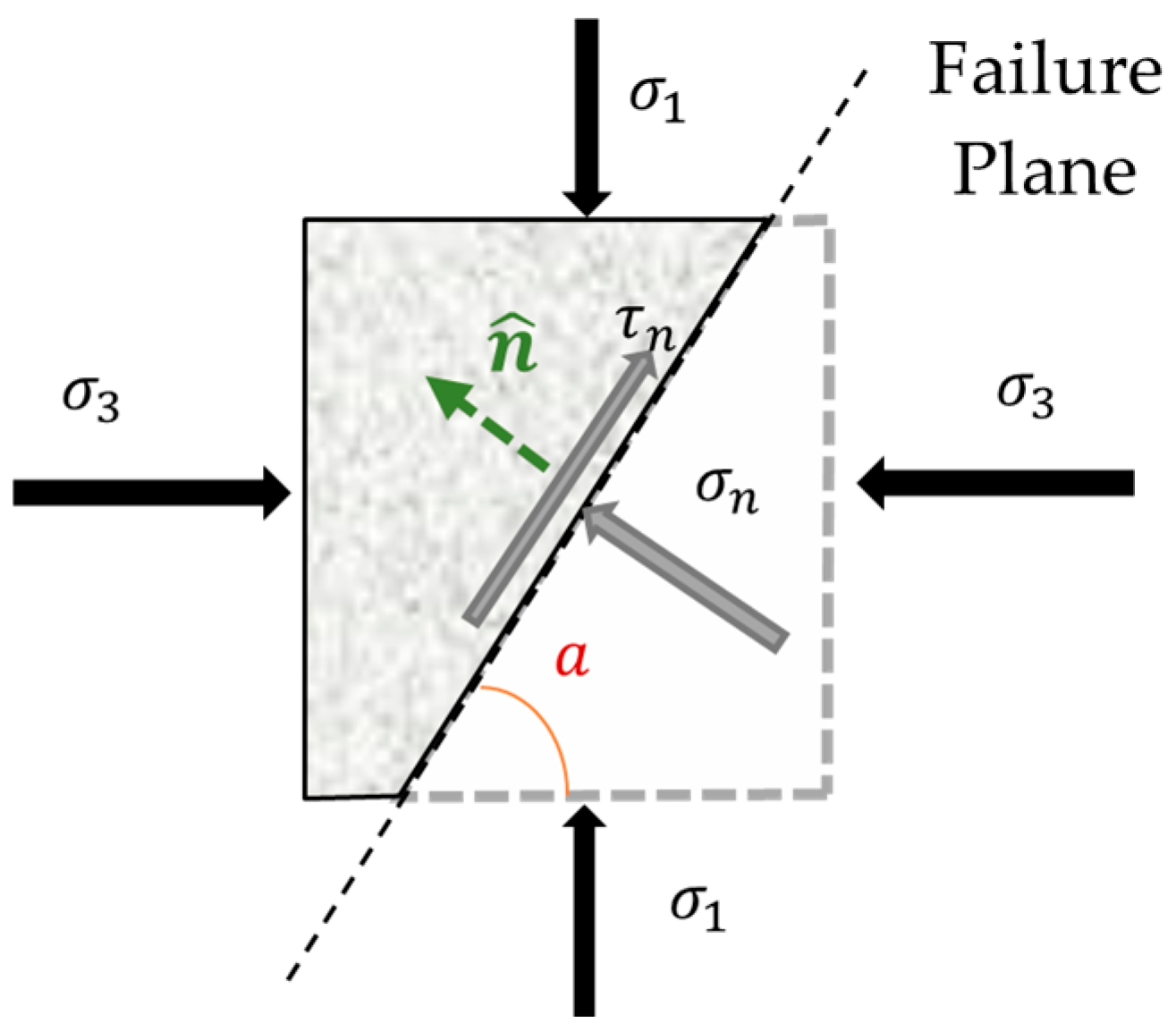
2.1. Three-Parameter Failure Model of Concrete
2.2. Internal Confinement of SHCC—Effect on the State of Stress
3. Materials and Methods
3.1. Experimental Methodology
3.2. Mix Design and Materials
4. Experimental Results
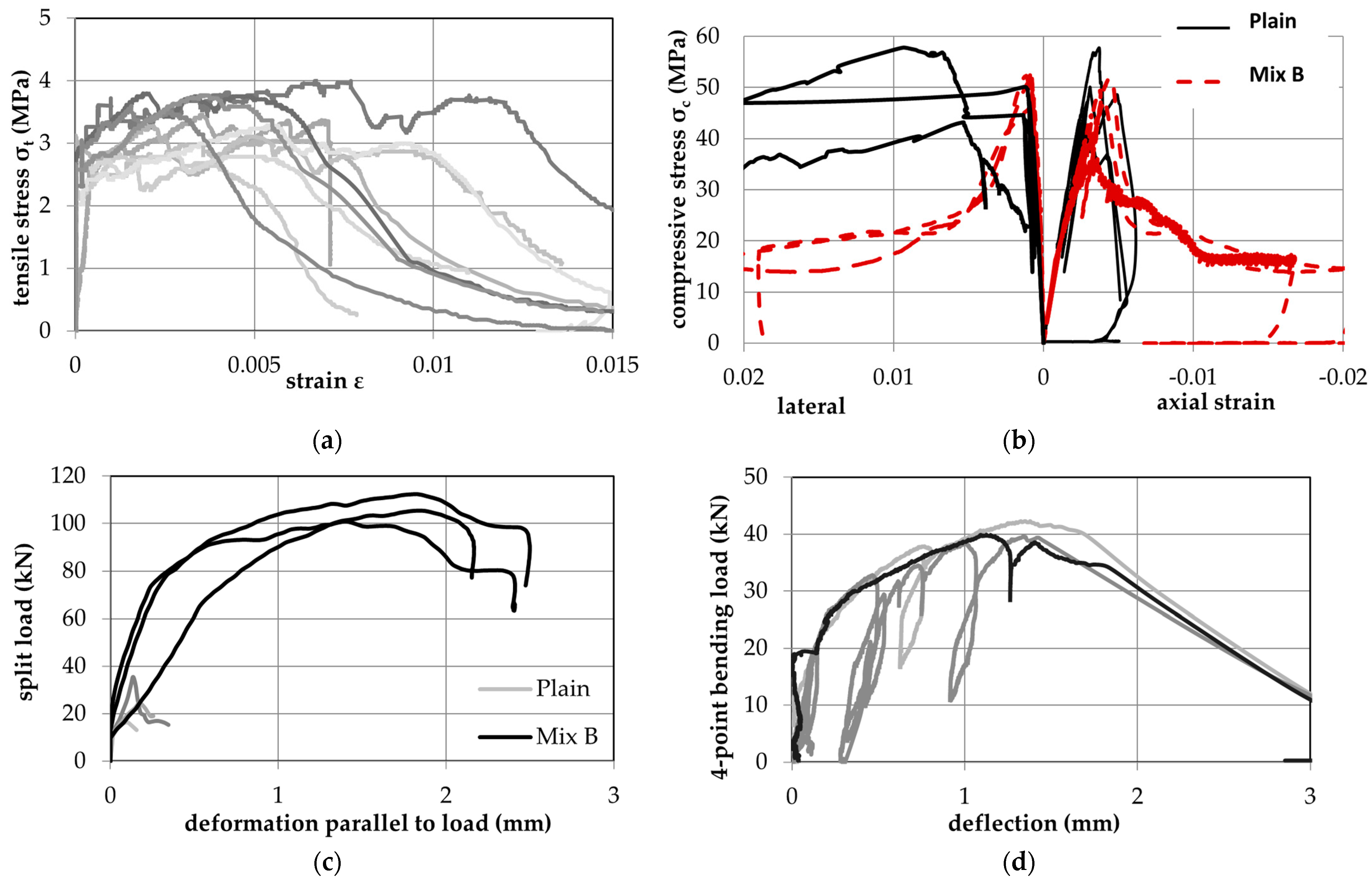
4.1. Material Characterization Test Results
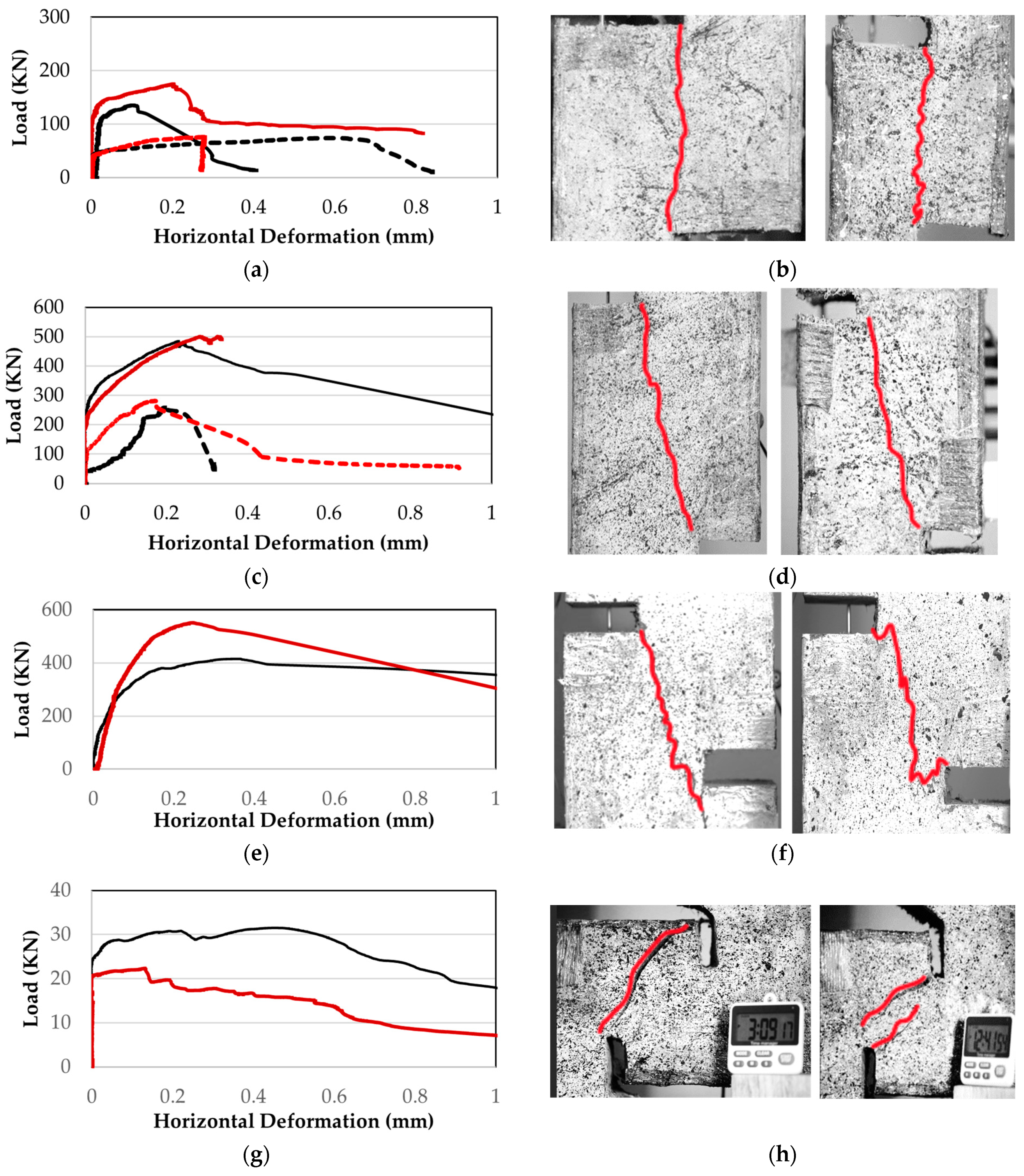
4.2. Push-off Test Results
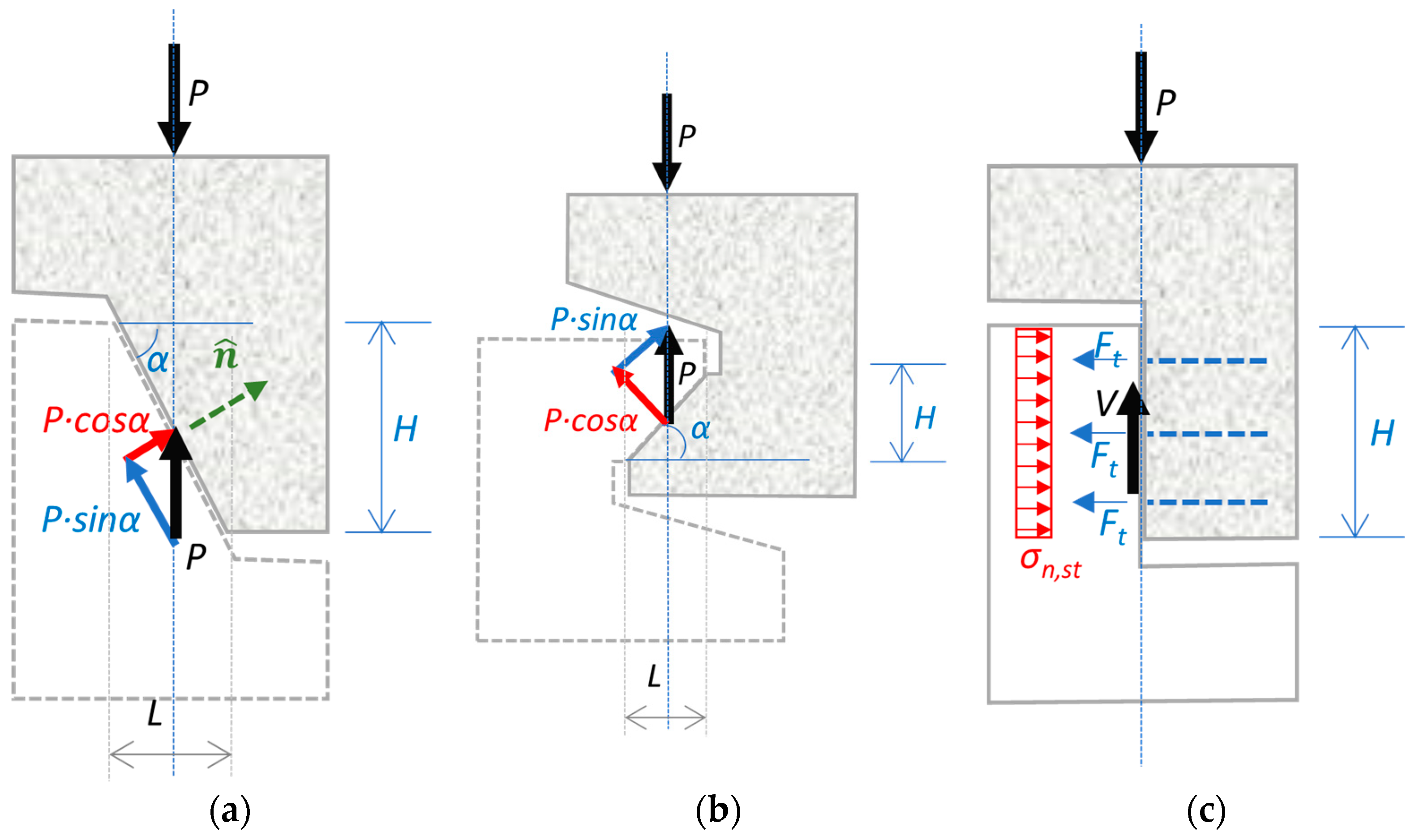
4.2.1. Variation in Shear Plane
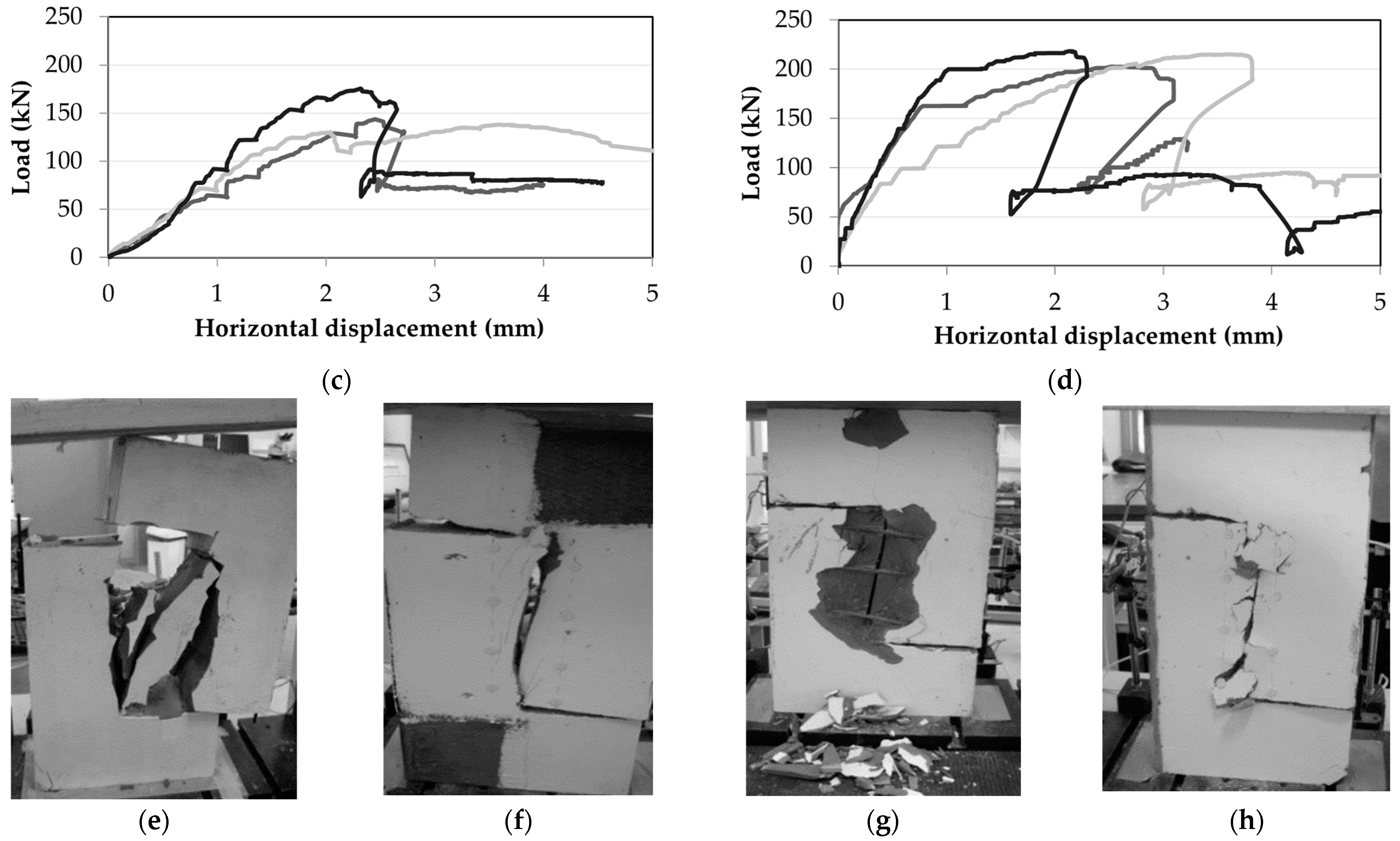
4.2.2. Effect of Reinforcement Crossing the Failure Plane (Fibres and Stirrups)
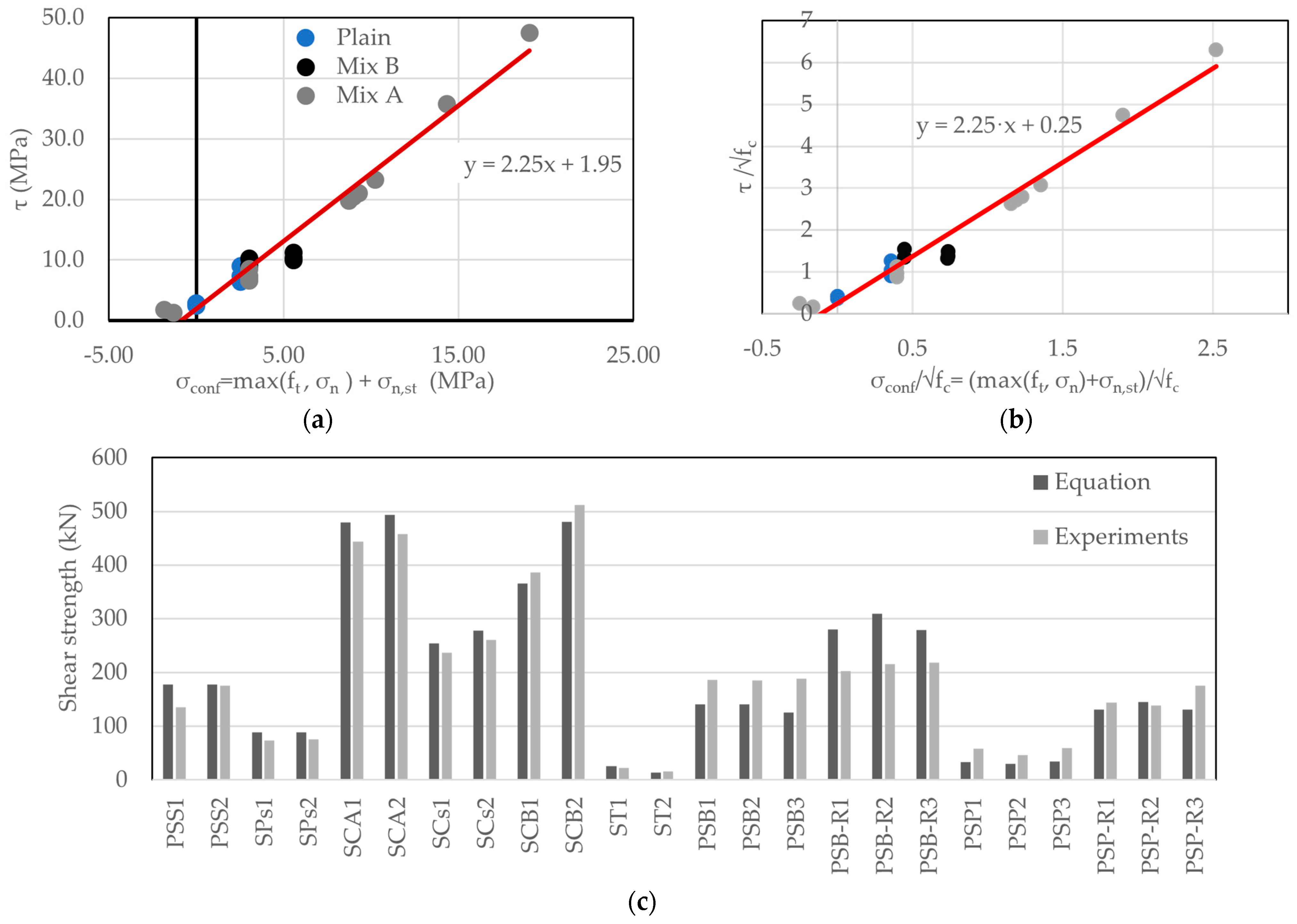
5. Calculation and Analysis of the Shear Failure Stress
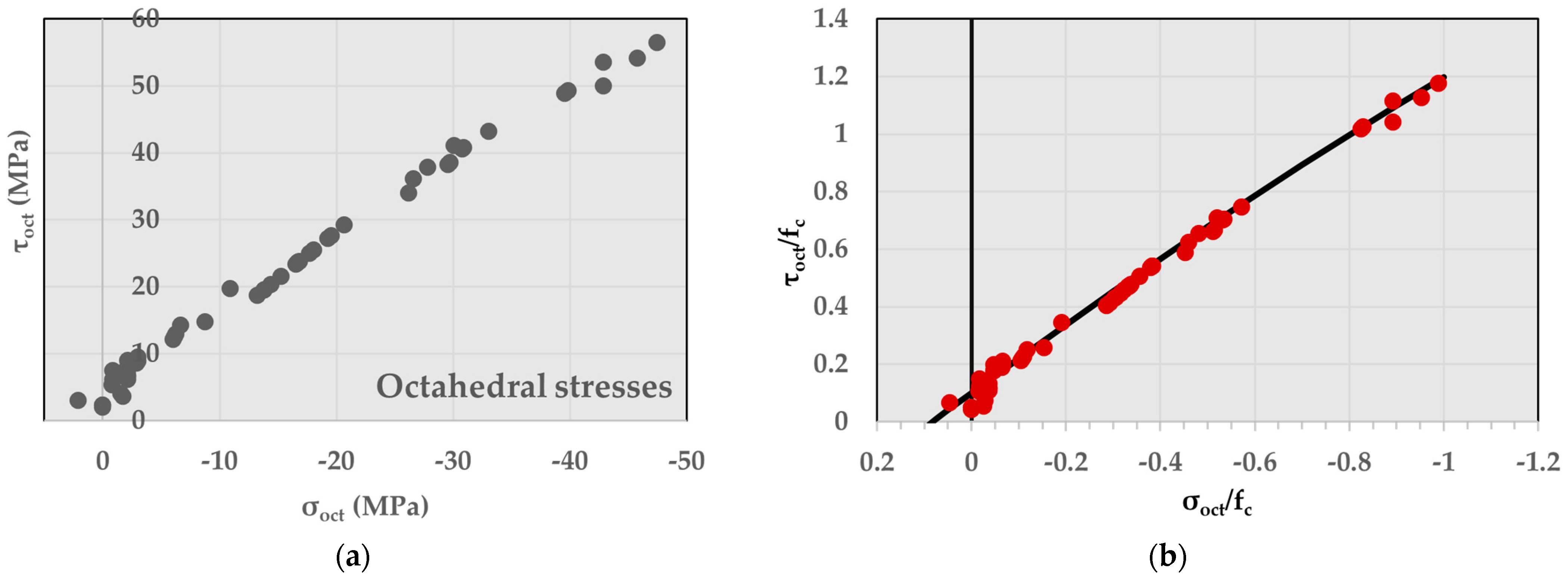
6. Discussion: A Three-Parameter Failure Criterion for SHCC
7. Conclusions
- SHCC with tension-hardening behaviour exhibits a nearly linear increase in shear resistance with increasing normal compressive stress acting on the plane of sliding.
- Damage in the form of dilation and crack opening is limited by the fibres and the fine and dense aggregate structure of the SHCC material. Also, it reduces with an increasing volumetric ratio of fibres, which also prolongs the range of tension-hardening behaviour of the composite.
- The pressure sensitivity which is inherent to all cementitious materials is also observed in SHCC. This refers to the dependence of the shear strength of the material to the normal clamping pressures that are acting on the shear plane by means of externally applied load, reinforcement, and by the internal confinement of the material which is owing to the distributed fibres embedded in the matrix.
- In the present work, the mechanisms of shear resistance of SHCC were studied using a range of different tests intended to produce tension-related shear failure under different normal stress states.
- The results were used to calibrate a Mohr–Coulomb-type shear-normal stress failure model that predicts the contribution of SHCC to the shear strength of a structural component (Equation (10)). Without the use of steel reinforcement or external load, this comprises the contributions of the matrix concrete and the fibres, as follows: where A is the shear area.
- The internal angle of friction was estimated at slightly over 60°, which is needed in structural components to define the crack plane with respect to the transverse axis of a member failing in shear and normal compression (e.g., in a column, this corresponds to an angle of 30° with respect to the longitudinal axis).
- The failure envelope, expressed in terms of stress invariants, was also derived to be used as an experimentally calibrated generalized failure criterion for SHCC materials in numerical modelling. The new failure criterion, which accounts for the internal confinement imparted by the fibres, shows an almost negligible contribution of the nonlinear term which is characteristic of conventional concrete. This underscores the damage control imparted by the fibres, thereby producing an almost linear meridian between octahedral stresses, and it is an exceptional feature of SHCC that sets it apart from plain concrete.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vecchio, F.J.; Collins, M.P. Compression Response of Cracked Reinforced Concrete. J. Struct. Eng. 1993, 119, 29–41. [Google Scholar] [CrossRef]
- Balaguru, P.N.; Shah, S.P. Fiber Reinforced Cement Composites; McGraw-Hill: New York, NY, USA, 1992. [Google Scholar]
- Georgiou, A.V.; Pantazopoulou, S.J. Effect of Fiber Length and Surface Characteristics on the Mechanical Properties of Cementitious Composites. Constr. Build. Mater. 2016, 125, 1216–1228. [Google Scholar] [CrossRef]
- NF P 18-710; French Standard (AFNOR): National Addition to Eurocode 2, Design of Concrete Structures: Specific Rules for Ultra-High Performance FRC. AFNOR: Paris, France, 2016.
- CSA International. Canadian Highway Bridge Design Code, Section 16: Fibre-Reinforced Structures, Annex 8; CSA International: Toronto, ON, Canada, 2020. [Google Scholar]
- Li, V.; Kanda, T. Innovations Forum: Engineered Cementitious Composites for Structural Applications. Concr. Int. 1998, 10, 66–69. [Google Scholar] [CrossRef]
- Paegle, I.; Fischer, G. Evaluation of Standardized Test Methods to Characterize Fiber Reinforced Cement Composites. In Proceedings of the 8th RILEM International Symposium on Fiber Reinforced Concrete: Challenges and Opportunities (BEFIB 2012), Guimarães, Portugal, 19–21 September 2012; Barros, J., Ed.; RILEM Publications S.A.R.L.: Bagneux Cedex, France, 2012; pp. 9–16. [Google Scholar]
- ACI Committee 318. Building Code Requirements for Reinforced Concrete (ACI 318-71); American Concrete Institute: Detroit, MI, USA, 1971. [Google Scholar]
- EN 1992-1-2; Eurocode 2: Design of Concrete Structures—Part 1–2: General Rules—Structural Fire Design. European Committee for Standardization: Brussels, Belgium, 2004.
- Jiang, X.; Li, Q.; Yin, X.; Xu, S. Investigation on Triaxial Compressive Mechanical Properties of Ultra High Toughness Cementitious Composites with High Strain Capacity. Cem. Concr. Res. 2023, 170, 107185. [Google Scholar] [CrossRef]
- Jang, H.O.; Lee, H.S.; Cho, K.; Kim, J. Numerical and Experimental Analysis of the Shear Behavior of Ultrahigh-Performance Concrete Construction Joints. Adv. Mater. Sci. Eng. 2018, 2018, 6429767. [Google Scholar] [CrossRef]
- Wu, P.; Xu, S.; Li, Q.; Zhou, F.; Al-Mansour, A.; Zhao, X. A Plasticity-Based Dynamic Constitutive Model for Ultra High Toughness Cementitious Composites. Int. J. Impact Eng. 2022, 161, 104086. [Google Scholar] [CrossRef]
- Liao, Q.; Su, Y.; Yu, J.; Yu, K. Compression-Shear Performance and Failure Criteria of Seawater Sea-Sand Engineered Cementitious Composites with Polyethylene Fibers. Constr. Build. Mater. 2022, 345, 128386. [Google Scholar] [CrossRef]
- Krahl, P.A.; Carrazedo, R.; El Debs, M.K. Mechanical Damage Evolution in UHPFRC: Experimental and Numerical Investigation. Eng. Struct. 2018, 170, 63–77. [Google Scholar] [CrossRef]
- Hung, C.C.; Li, S.H. Three-Dimensional Model for Analysis of High Performance Fiber Reinforced Cement-Based Composites. Compos. Part B Eng. 2013, 45, 1441–1447. [Google Scholar] [CrossRef]
- Chen, M.; Pei, C. Influence of Different Kinds of Steel Fibers on Flexural Behavior of Ultra-High Performance Concrete Beam by AnSYS. In Proceedings of the 5th International Conference on Machinery, Materials and Computing Technology (ICMMCT 2017), Taiyuan, China, 14–16 April 2017; pp. 1–6. [Google Scholar]
- Menetrey, P.; Willam, K.J. Triaxial Failure Criterion for Concrete and Its Generalization. ACI Struct. J. 1995, 92, 311–318. [Google Scholar]
- Ralli, Z.G.; Genikomsou, A.S.; Pantazopoulou, S.J. Comparative Evaluation of Nonlinear F.E.A. Inverse Analysis of Tensile Properties of UHPFRC. In Proceedings of the Fib Symposium 2021, Lisbon, Portugal, 14–16 June 2021; pp. 563–573. [Google Scholar]
- Riedel, W.; Mayrhofer, C.; Thoma, K.; Stolz, A. Engineering and Numerical Tools for Explosion Protection of Reinforced Concrete. Int. J. Prot. Struct. 2010, 1, 85–102. [Google Scholar] [CrossRef]
- Riedel, W.; Thoma, K.; Hiermaier, S. Penetration of Reinforced Concrete by BETA-B-500, Numerical Analysis Using a New Macroscopic Concrete Model for Hydrocodes. In Proceedings of the International Symposium on the Interaction of the Effects of Munitions with Structures (ISIEMS), Berlin, Germany, 17–20 May 1999; pp. 315–322. [Google Scholar]
- Riedel, W. 10 Years RHT: A Review of Concrete Modelling and Hydrocode Applications. In Predictive Modeling of Dynamic Processes: A Tribute to Professor Klaus Thoma; Hiermaier, S., Ed.; Springer: New York, NY, USA, 2009; pp. 143–165. [Google Scholar]
- Wilson, W.; O’Flaherty, T. Finite Element Analysis of Ultra High Performance Fibre Reinforced Concrete Beams Using Microplane Modelling. In Proceedings of the Fibre Reinforced Concrete: Improvements and Innovations, RILEM-Fib International Symposium on FRC, Valencia, Spain, 18–20 November 2020; pp. 1–10. [Google Scholar]
- ANSYS. ANSYS® AUTODYN 2022 R2 Release 2022; ANSYS: Canonsburg, PA, USA, 2022. [Google Scholar]
- Smith, M. ABAQUS/Standard User’s Manual; Dassault Systèmes: Vélizy-Villacoublay, France, 2009. [Google Scholar]
- Ferraris, C.F.; Obla, K.H.; Hill, R. The Influence of Mineral Admixtures on the Rheology of Cement Paste and Concrete. Cem. Concr. Res. 2001, 31, 245–255. [Google Scholar] [CrossRef]
- Thomas, M.D.A. Optimizing the Use of Fly Ash in Concrete; Portland Cement Association: Skokie, IL, USA, 2007. [Google Scholar]
- Georgiou, A.V. Characterization of the Structural Performance of Strain Hardening Fiber Reinforced Cementitious Composites. Ph.D. Thesis, University of Cyprus, Nicosia, Cyprus, 2017. [Google Scholar]
- Bresler, B.; Pister, K.S. Strength of Concrete under Combined Stresses. ACI J. Proc. 1958, 551, 321–345. [Google Scholar]
- Chen, W.F. Plasticity in Reinforced Concrete; McGraw-Hill: New York, NY, USA, 1982. [Google Scholar]
- CSA International. Canadian Highway Bridge Design Code—Annex 8.1 Fibre Reinforced Concrete (New Edition); CSA International: Toronto, ON, Canada, 2018. [Google Scholar]
- Georgiou, A.V.; Pantazopoulou, S.J. Experimental Investigation on the Confining Effect of Fibers in SHFRCC. Compos. Struct. 2018, 202, 29–37. [Google Scholar] [CrossRef]
- Mattock, A.H.; Hawkins, N.M. Shear Transfer in Reinforced Concrete. ACI J Proc. 1969, 66, 735–744. [Google Scholar]
- Walraven, J.C.; Reinhardt, H.W. Theory and Experiments on the Mechanical Behaviour of Cracks in Plain and Reinforced Concrete Subjected to Shear Loading; Delft University Press: Delft, The Netherlands, 1981; Volume 26, pp. 1–64. [Google Scholar]
- Foster, R.M.; Morley, C.T.; Lees, J.M. Modified Push-Off Testing of an Inclined Shear Plane in Reinforced Concrete Strengthened with CFRP Fabric. J. Compos. Constr. 2016, 20, 04015061. [Google Scholar] [CrossRef]
- ASTM C496/C496M; Standard Test Method for Splitting Tensile Strength of Cylindrical Concrete Specimens. ASTM International: West Conshohocken, PA, USA, 2006; Volume 06, pp. 1–5.
- ASTM C618; Standard Specification for Coal Fly Ash and Raw or Calcined Natural Pozzolan for Use in Concrete. ASTM International: West Conshohocken, PA, USA, 2008; Volume 04, pp. 1–6.
- Cucuzza, R.; Aloisio, A.; Accornero, F.; Marinelli, A.; Bassoli, E.; Marano, G.C. Size-Scale Effects and Modelling Issues of Fibre-Reinforced Concrete Beams. Constr. Build. Mater. 2023, 392, 131727. [Google Scholar] [CrossRef]
- Minelli, F.; Conforti, A.; Cuenca, E.; Plizzari, G. Are Steel Fibres Able to Mitigate or Eliminate Size Effect in Shear? Mater. Struct. 2014, 47, 459–473. [Google Scholar] [CrossRef]
- Georgiou, A.V.; Pantazopoulou, S.J. Experimental and Analytical Investigation of the Shear Behavior of Strain Hardening Cementitious Composites. Struct. Eng. Mech. 2019, 72, 781–792. [Google Scholar]
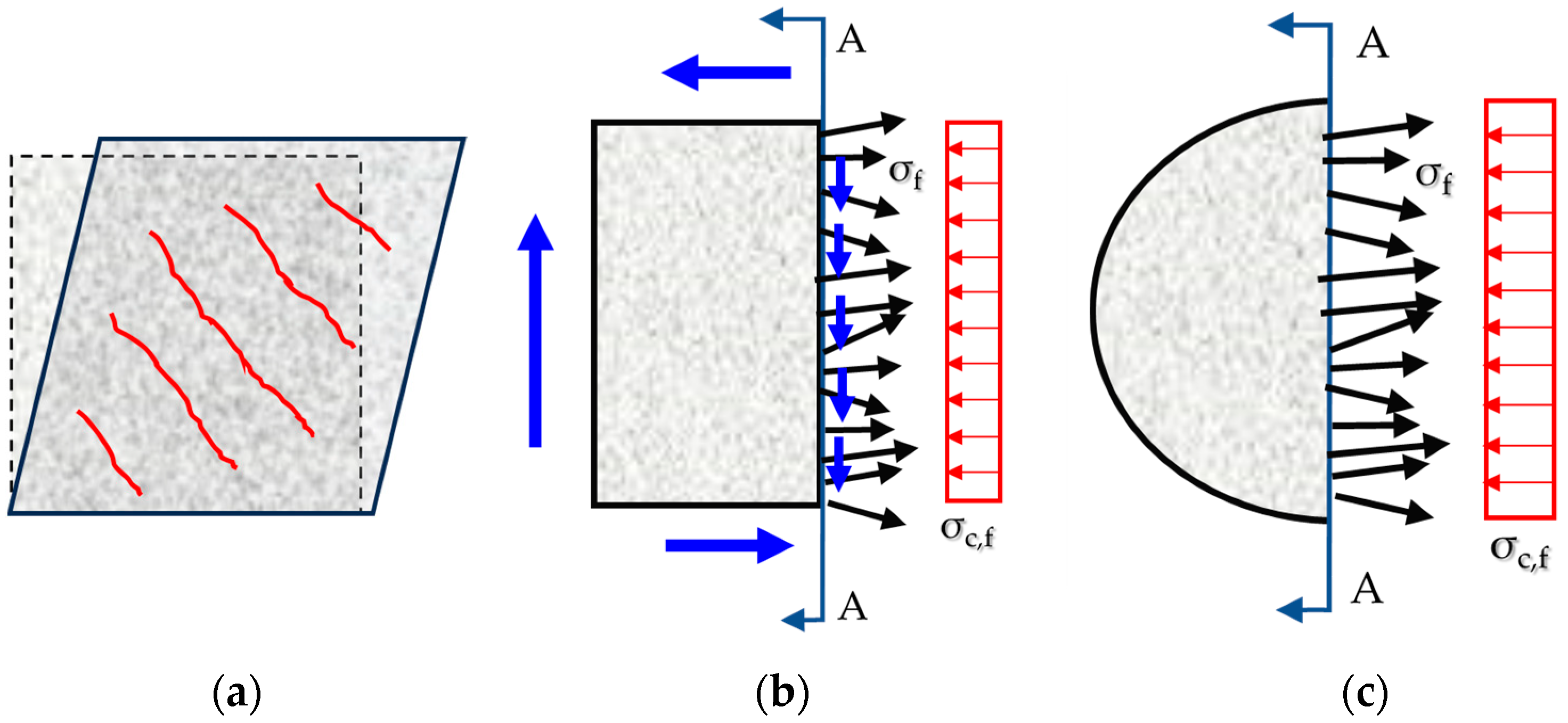



| ID | Mix | Rebar Rein. (mm2) | α (°) | Height (mm) | Shear Plane R (mm) | Scale | No. of Specimens |
|---|---|---|---|---|---|---|---|
| PSS | A | - | 90° | 480 | 205 | - | 2 |
| PSs | A | - | 90° | 240 | 102.5 | 1:2 | 2 |
| CSA | A | - | 66.3° | 480 | 269.6 | - | 2 |
| CSs | A | - | 66.3° | 240 | 134.8 | 1:2 | 2 |
| CSB | A | - | 68.2° | 480 | 167.1 | - | 2 |
| ST | A | - | −45° | 480 | 101 | - | 2 |
| PS-B | B | - | 90° | 480 | 200 | - | 3 |
| PS-BR | B | 180 | 90° | 480 | 200 | - | 3 |
| PS-P | Plain | - | 90° | 480 | 200 | - | 3 |
| PS-PR | Plain | 180 | 90° | 480 | 200 | - | 3 |
| Mix | Cement | Fly Ash | Silica Fume | Slag | Sand | Water | SP | PVA Fibres (%v) |
|---|---|---|---|---|---|---|---|---|
| Mix A | 1.00 | 1.70 | 0.28 | 0.1 | 1.10 | 0.78 | 0.025 | 2.3 (df = 0.1 mm) |
| Mix B | 1.00 | 1.20 | - | - | 0.80 | 0.55 | 0.012 | 2 (df = 0.039 mm) |
| Plain | 1.00 | 1.20 | - | - | 0.80 | 0.60 | 0.017 | - |
| Fibre Type | Diameter (μm) | Length (mm) | Density (kg/m3) | Tensile Strength (MPa) | Young’s Modulus (GPa) | Strain Capacity (%) |
|---|---|---|---|---|---|---|
| PVA39 | 39 | 12 | 1300 | 1600 | 40 | 6.5 |
| PVA100 | 100 | 12 | 1100 | 1235 | 29 | 12.5 |
| Rebar | εsy | εsyy | εsu | fsy (MPa) | fsyy (MPa) | fsu (MPa) |
|---|---|---|---|---|---|---|
| Φ6 | 0.0026 | 0.026 | 0.2 | 312 | 320 | 376 |
| Mix | Uniaxial Tension ft (MPa) | Uniaxial Compr. fc (MPa) | Split Tension ft,spl (MPa) | Flex. Tension ft,fl (MPa) (a/d = 1) | Flexure Tension ft,fl (MPa) (a/d = 2) |
|---|---|---|---|---|---|
| Mix A | - | 60.75 | - | 10.98 | 9.96 |
| Mix B | 3.00 | 48.30 | 5.73 | 12.07 | 9.86 |
| Plain | - | 52.80 | 1.35 | 3.35 | - |
| Spec. ID | ρ % (Stirrup Reinforc.) | Push-off Max Load (kN) | H (mm) | L (mm) | α | R (mm) | τn (MPa) | σc,f (MPa) | σconf = max(ft,u,σc,f) + σn,st (MPa) |
|---|---|---|---|---|---|---|---|---|---|
| PSS1 | 0 | 135.4 | 205 | 0 | 90 | 205 | 6.60 | 0.00 | 3.00 |
| PSS2 | 0 | 175.1 | 205 | 0 | 90 | 205 | 8.54 | 0.00 | 3.00 |
| SPs1 | 0 | 73.97 | 102.5 | 0 | 90 | 102.5 | 7.22 | 0.00 | 3.00 |
| SPs2 | 0 | 75.85 | 102.5 | 0 | 90 | 102.5 | 7.40 | 0.00 | 3.00 |
| SCA1 | 0 | 484.7 | 205 | 90 | 66.30 | 223.89 | 19.82 | 8.70 | 8.70 |
| SCA2 | 0 | 500 | 205 | 90 | 66.30 | 223.89 | 20.45 | 8.98 | 8.98 |
| SCs1 | 0 | 257.98 | 102.5 | 45 | 66.30 | 111.94 | 21.10 | 9.26 | 9.26 |
| SCs2 | 0 | 284.23 | 102.5 | 45 | 66.30 | 111.94 | 23.25 | 10.21 | 10.21 |
| SCB1 | 0 | 415.4 | 100 | 40 | 68.20 | 107.70 | 35.81 | 14.32 | 14.32 |
| SCB2 | 0 | 551.85 | 100 | 40 | 68.20 | 107.70 | 47.57 | 19.03 | 19.03 |
| ST1 | 0 | −31.54 | 85 | 85 | 45 | 120.21 | 1.86 | −1.86 | −1.86 |
| ST2 | 0 | −22.34 | 85 | 85 | 45 | 120.21 | 1.31 | −1.31 | −1.31 |
| PSB1 | 0 | 186.21 | 206.05 | 0 | 90 | 206.05 | 9.04 | 0.00 | 3.00 |
| PSB2 | 0 | 185.47 | 205.56 | 0 | 90 | 205.56 | 9.02 | 0.00 | 3.00 |
| PSB3 | 0 | 188.79 | 182.8 | 0 | 90 | 182.8 | 10.33 | 0.00 | 3.00 |
| PSB-R1 | 0.00848 | 202.7 | 194.81 | 0 | 90 | 194.81 | 10.40 | 0.00 | 5.54 |
| PSB-R2 | 0.00848 | 215.16 | 215.32 | 0 | 90 | 215.32 | 9.99 | 0.00 | 5.54 |
| PSB-R3 | 0.00848 | 218.35 | 194.76 | 0 | 90 | 194.76 | 11.21 | 0.00 | 5.54 |
| PSP1 | 0 | 57.78 | 201.31 | 0 | 90 | 201.31 | 2.87 | 0.00 | 0.00 |
| PSP2 | 0 | 46.10 | 186 | 0 | 90 | 186 | 2.48 | 0.00 | 0.00 |
| PSP3 | 0 | 59.60 | 208.41 | 0 | 90 | 208.41 | 2.86 | 0.00 | 0.00 |
| PSP-R1 | 0.008482 | 143.65 | 194.81 | 0 | 90 | 194.81 | 7.37 | 0.00 | 2.54 |
| PSP-R2 | 0.008482 | 138.10 | 215.32 | 0 | 90 | 215.32 | 6.41 | 0.00 | 2.54 |
| PSP-R3 | 0.008482 | 175.47 | 194.76 | 0 | 90 | 194.76 | 9.01 | 0.00 | 2.54 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Georgiou, A.; Eshghi, N.; Pantazopoulou, S. Shear Strength of Strain-Hardening Cementitious Materials. Constr. Mater. 2023, 3, 509-528. https://doi.org/10.3390/constrmater3040032
Georgiou A, Eshghi N, Pantazopoulou S. Shear Strength of Strain-Hardening Cementitious Materials. Construction Materials. 2023; 3(4):509-528. https://doi.org/10.3390/constrmater3040032
Chicago/Turabian StyleGeorgiou, Antroula, Najmeh Eshghi, and Stavroula Pantazopoulou. 2023. "Shear Strength of Strain-Hardening Cementitious Materials" Construction Materials 3, no. 4: 509-528. https://doi.org/10.3390/constrmater3040032
APA StyleGeorgiou, A., Eshghi, N., & Pantazopoulou, S. (2023). Shear Strength of Strain-Hardening Cementitious Materials. Construction Materials, 3(4), 509-528. https://doi.org/10.3390/constrmater3040032






