Calculation Method for Traffic Load-Induced Permanent Deformation in Soils under Flexible Pavements
Abstract
:1. Introduction
2. Explicit Calculation Method and HCA Model
2.1. Explicit and Implicit Methods
2.2. HCA Model
2.3. Direction of Accumulation
2.4. Determination and Calibration of the HCA Parameters
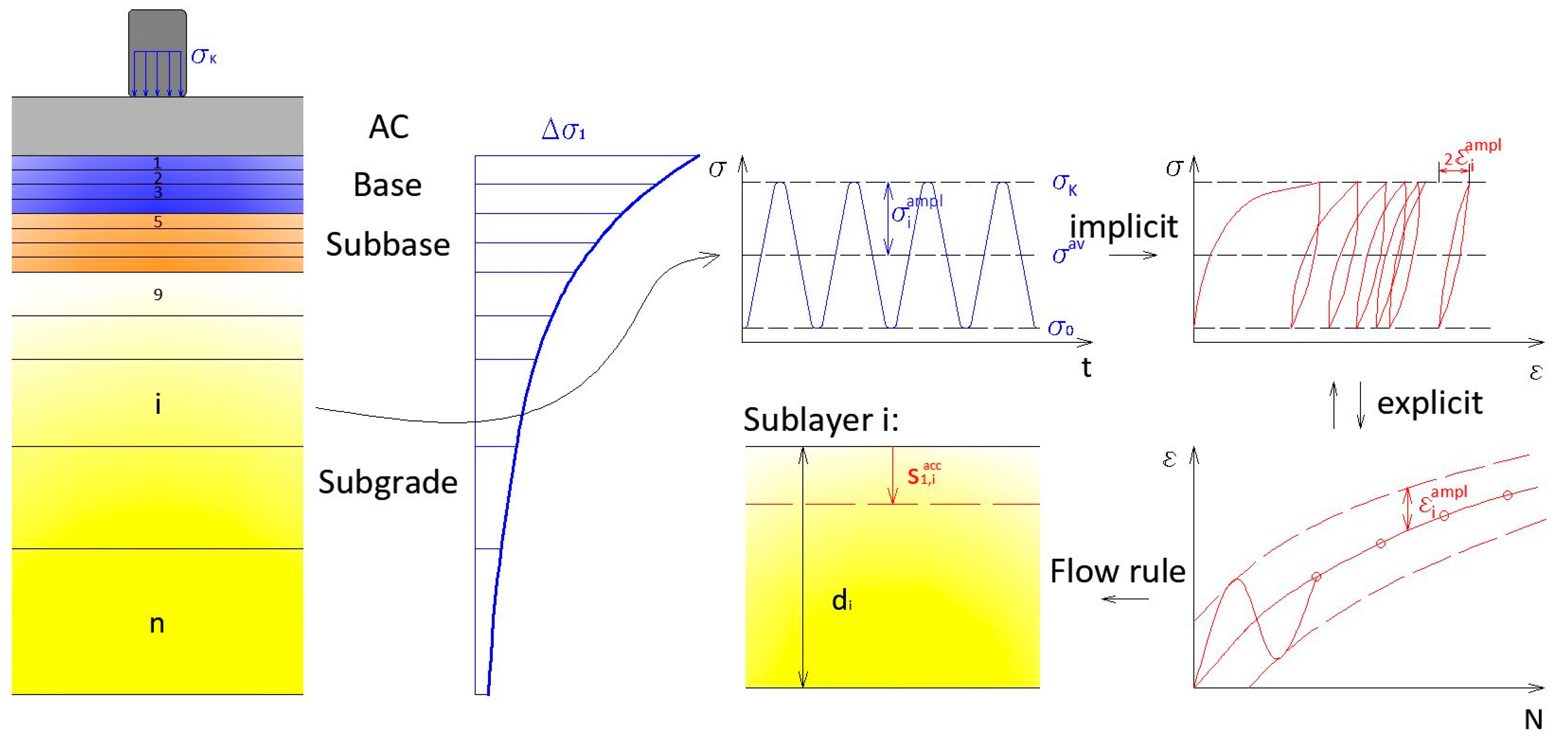
3. The Developed Layered Calculation Method
3.1. Basic Principles
3.2. Calculation Steps
- The vertical and horizontal stresses due to the own weight of the soil are determined;
- ;
- , where is the coefficient of earth pressure at rest by also considering overconsolidation;
- The additional vertical load in each sublayer due to the wheel load is determined as the function of the geometry and stiffness of the sublayer;
- The additional horizontal load is determined from the additional vertical load , where is the coefficient of earth pressure considering overconsolidation and the incremental loading;
- The additional vertical strain is calculated from the additional stresses, mean normal stress, relative density and strain-dependent stiffness: . Because the incremental strain is the function of stiffness, and stiffness is the function of strain, iteration shall be used to determine the strain increment;
- The total vertical strain and strain amplitude are calculated as the sum of increments in each sublayer: ;
- The total horizontal strain and strain amplitude are calculated from the vertical strain and the ratio of horizontal strains in each sublayer: = ;
- Then, the elastic strain amplitude is obtained using Equation (1);
- For the base and subbase courses, the above implicit calculation method is simplified to the extent that the stiffness is taken into account with the MR resilient modulus, which is independent of the void ratio and strain level.
- 11.
- I mean normal stress and stress state () is determined in the center line of sublayer “i” and then the factors independent of the number of cycles and are calculated;
- 12.
- In the next step, factors of Equation (4) that depend on the number of cycles are obtained: from the actual , from the actual and from the actual e void ratio;
- 13.
- The accumulated strain rate is calculated using Equation (4). In the case of drained conditions, Equation (2) becomes ;
- 14.
- The strain increment due to cycles is determined by , then ;
- 15.
- Calculating the rate of the preloading variable with the actual value of ;
- 16.
- The actual value of is updated due to load cycles by ;
- 17.
- Vertical strains are calculated using the flow rule , then compression of each sublayer by ;
- 18.
- Volumetric strain increment is determined due to cycles, then the new void ratio is obtained by , ;
- 19.
- The characterizing a denser state results in a higher stiffness , thus points 6.–9. of the implicit calculation are repeated;
- 20.
- Steps 12.–18. of the explicit calculation are repeated until the goal value of a number of cycles.
4. Analysed Pavement Structures and Material Models
4.1. Analysed Pavement Structures
4.2. Materials and Their Properties
4.3. Material Model of the Implicit Calculation
5. Implicit Calculation Model and Comparative Study
5.1. Vertical Stress Distribution
5.2. Horizontal Strain Ratio
5.3. Coefficient of Earth Pressure
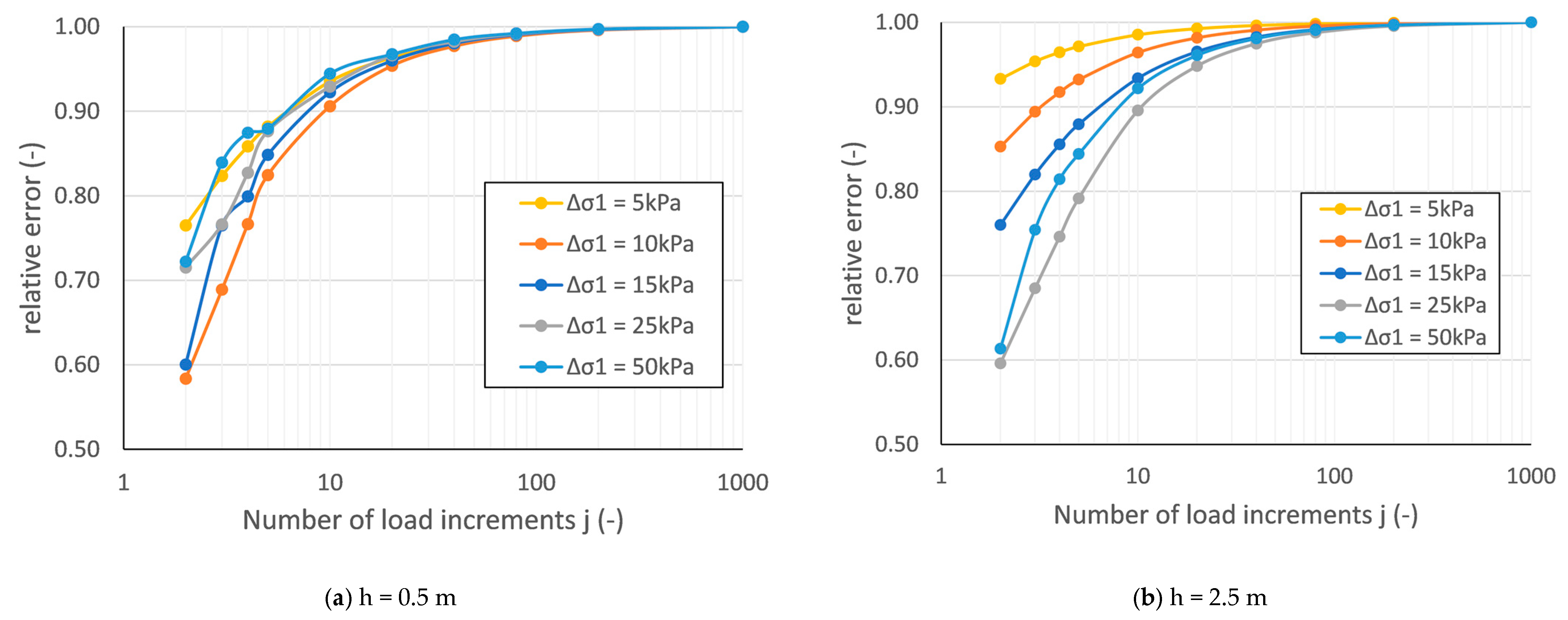
5.4. Number of Load Increments
5.5. Number and Arrangement of Layers
6. Discussion of Layered Model Results
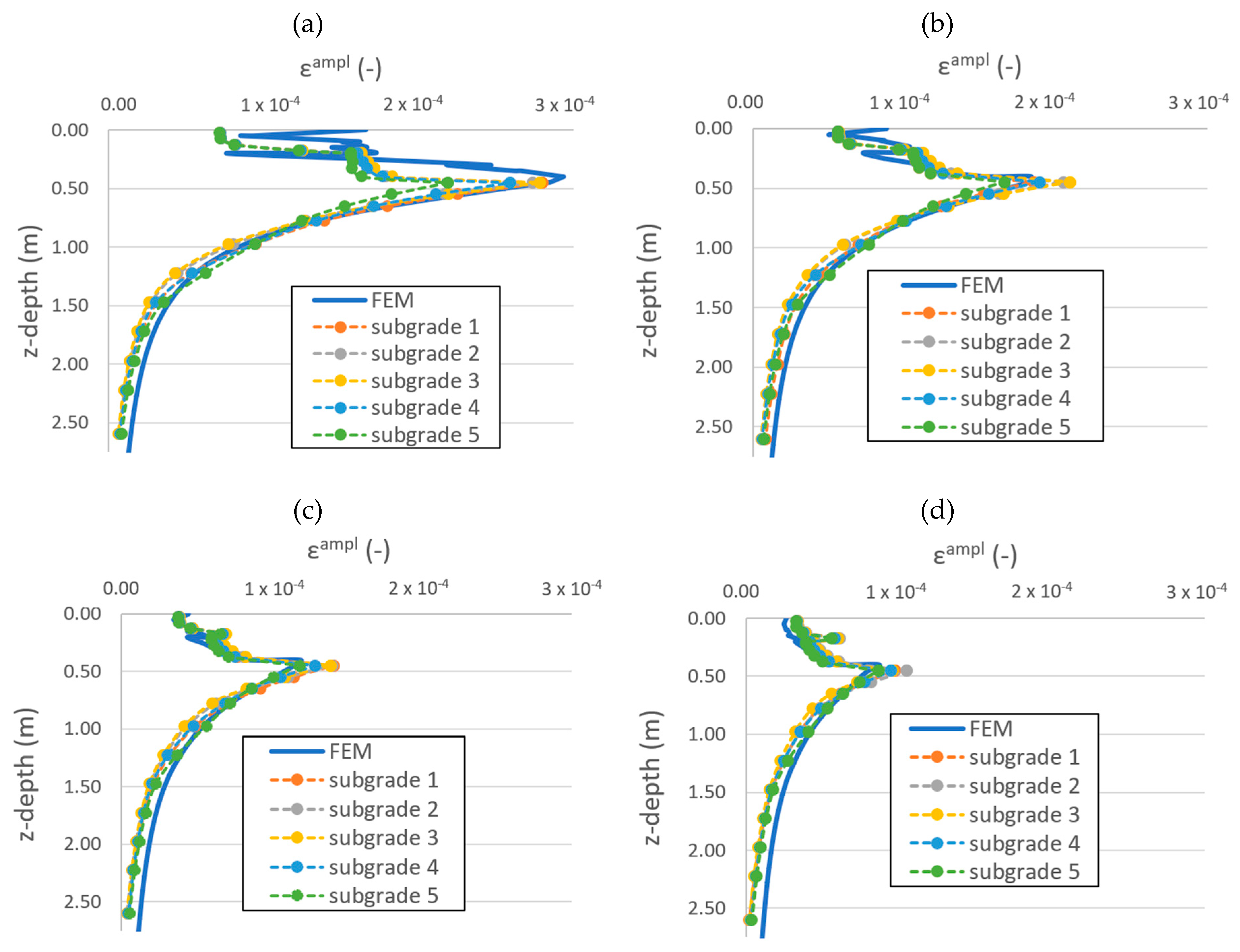
6.1. Implicit Calculation Results
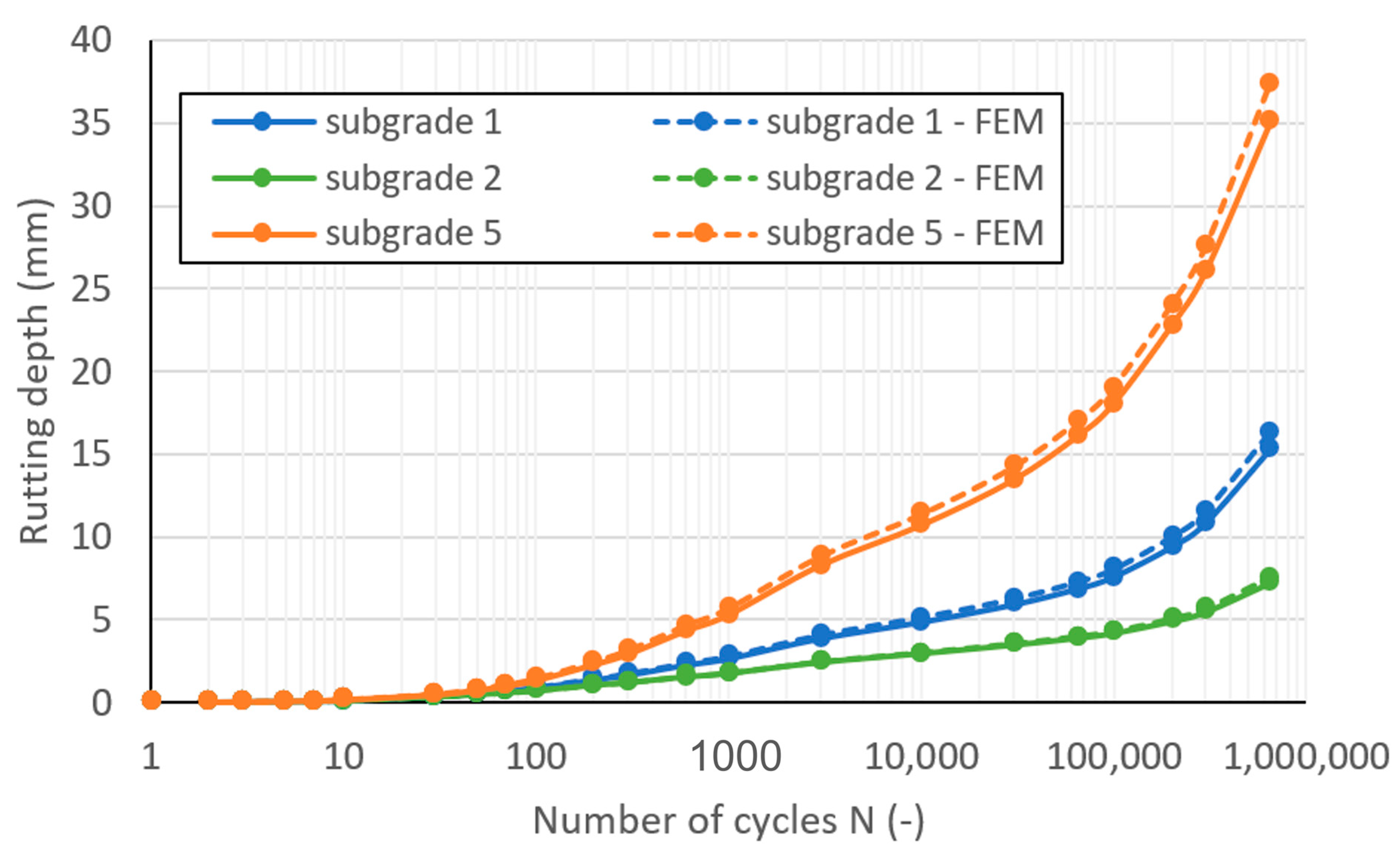
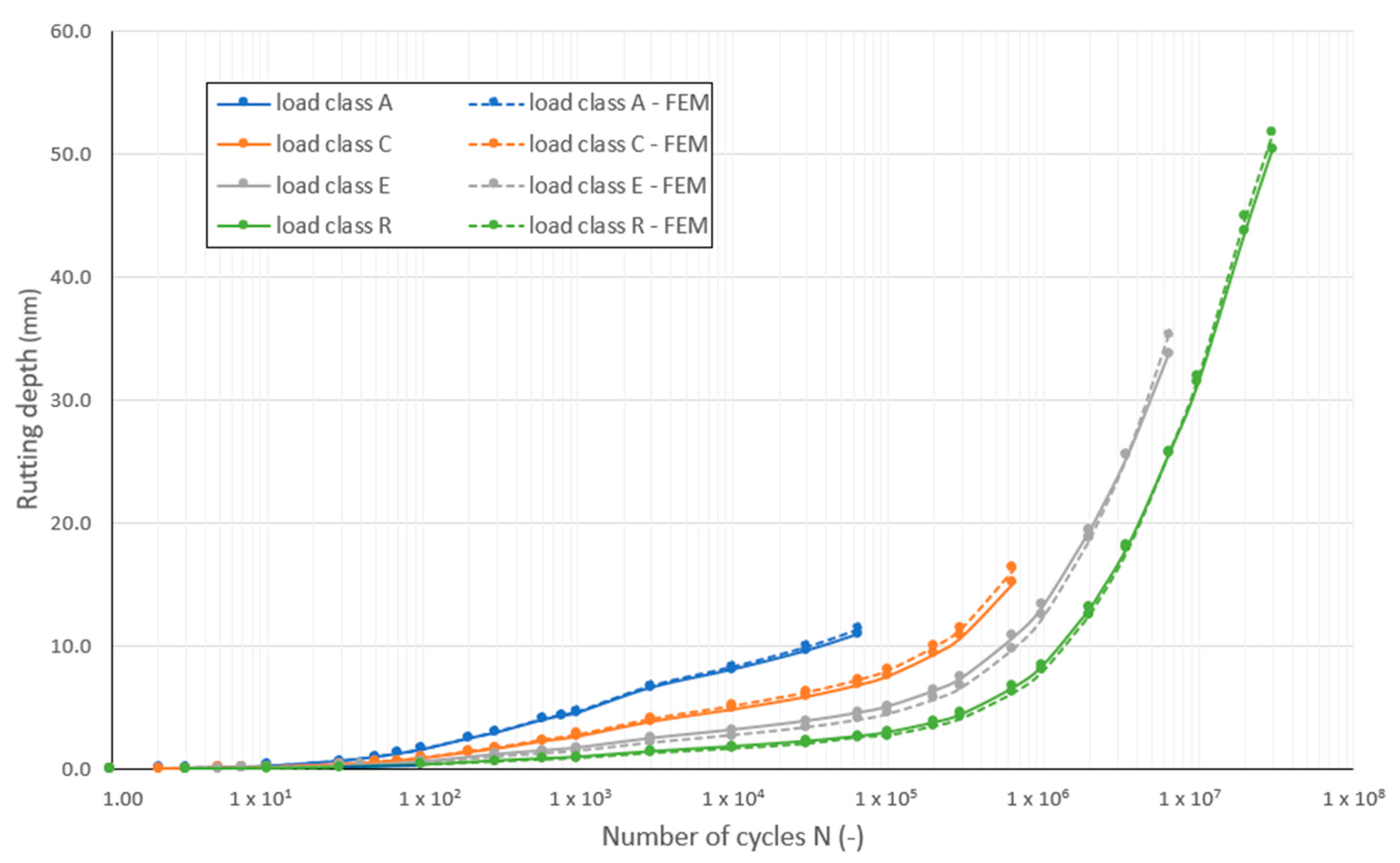
6.2. Comparison of Permanent Deformation Results between Layered and FE Model
6.3. Effect of Traffic Load Class on Permanent Deformations
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- e-UT06.03.13 (ÚT 2-1.202); Aszfaltburkolatú Útpályaszerkezetek Méretezése És Megerősítése (Design of Road Pavement Structures and Overlay Design with Asphalt Surfacing). Útügyi Műszaki Előírás (Road Design and Specification Standard): Budapest, Hungary, 2017.
- FGSV Verlag. RStO Richtlinie für die Standardisierung des Oberbaues von Verkehrsflächen; FGSV Verlag: Köln, Germany, 2001. [Google Scholar]
- Powell, W.D.; Potter, J.F.; Mayhew, H.C.; Nunn, M.E. The structural design of bituminous roads. In Trrl Laboratory Report; Transport and Road Research Laboratory: Wokingham, UK, 1984. [Google Scholar]
- Shell. SPDM-PC User Manual. Shell Pavement Design Method for Use in a Personal Computer (Version 1994, Release 2.0); Shell International Petroleum: London, UK, 1994. [Google Scholar]
- Little, P.H. The Design of Unsurfaced Roads Using Geosynthetics. Ph.D. Dissertation, The University of Nottingham, Nottingham, UK, 1993. [Google Scholar]
- Gidel, G.; Hornych, P.; Chauvin, J.; Breysse, D.; Denis, A. A New Approach for Investigating the Permanent Deformation Behaviour of Unbound Granular Material Using the Repeated Loading Triaxial Apparatus. Bull. Lab. Ponts Chaussees 2001, 1, 5–21. [Google Scholar]
- Uzan, J. Permanent Deformation in Flexible Pavements. J. Transp. Eng. 2004, 130, 6–13. [Google Scholar] [CrossRef]
- Werkmeister, S. Permanent Deformation Behaviour of Unbound Granular Materials in Pavement Constructions. Ph.D. Értekezés, TU Dresden, Drezda, Germany, 2003. [Google Scholar]
- Niemunis, A.; Wichtmann, T.; Triantafyllidis, T. A High-Cycle Accumulation Model for Sand. Comput. Geotech. 2005, 32, 245–263. [Google Scholar] [CrossRef]
- Wichtmann, T. Explicit Accumulation Model for Non-Cohesive Soils under Cyclic Loading; Schriftenreihe des Institutes für Bodenmechanik der Ruhr-Universität Bochum: Bochum, Germany, 2005. [Google Scholar]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Flow Rule in a High-Cycle Accumulation Model Backed by Cyclic Test Data of 22 Sands. Acta Geotech. 2014, 9, 695–709. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. On the Determination of a Set of Material Constants for a High-cycle Accumulation Model for Non-Cohesive Soils. Int. J. Numer. Anal. Methods Geomech. 2010, 34, 409–440. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Simplified Calibration Procedure for a High-Cycle Accumulation Model Based on Cyclic Triaxial Tests on 22 Sands. In Frontiers in Offshore Geotechnics II; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Strain Accumulation in Sand Due to Cyclic Loading: Drained Cyclic Tests with Triaxial Extension. Soil Dyn. Earthq. Eng. 2007, 27, 42–48. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Experimental Evidence of a Unique Flow Rule of Non-Cohesive Soils under High-Cyclic Loading. Acta Geotech. 2006, 1, 59–73. [Google Scholar] [CrossRef]
- Wichtmann, T.; Triantafyllidis, T. An Experimental Database for the Development, Calibration and Verification of Constitutive Models for Sand with Focus to Cyclic Loading: Part I—Tests with Monotonic Loading and Stress Cycles. Acta Geotech. 2016, 11, 739–761. [Google Scholar] [CrossRef]
- Niemunis, A. Extended Hypoplastic Models for Soils. Habilitáció; Institutes für Grundbau und Bodenmechanik der Ruhr-Universität Bochum: Bochum, Germany, 2003. [Google Scholar]
- Wichtmann, T. Soil Behaviour under Cyclic Loading—Experimental Observations, Constitutive Description and Applications. Habilitation Thesis; Veröffentlichungen des Institutes für Bodenmechanik und Felsmechanik am Karlsruher Institut für Technologie (KIT); Karlsruher Institut für Technologie (KIT): Karlsruhe, Germany, 2016; ISBN 0453-3267. [Google Scholar]
- Chang, C.S.; Whitman, R.V. Drained Permanent Deformation of Sand Due to Cyclic Loading. J. Geotech. Eng. 1988, 114, 1164–1180. [Google Scholar] [CrossRef]
- Wichtmann, T.; Rondón, H.; Niemunis, A.; Triantafyllidis, T.; Lizcano, A. Prediction of Permanent Deformations in Pavements Using a High-Cycle Accumulation Model. J. Geotech. Geoenviron. Eng. 2010, 136, 728–740. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Improved Simplified Calibration Procedure for a High-Cycle Accumulation Model. Soil Dyn. Earthq. Eng. 2015, 70, 118–132. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T.H. Validation and Calibration of a High-Cycle Accumulation Model Based on Cyclic Triaxial Tests on Eight Sands. Soils Found. 2009, 49, 711–728. [Google Scholar] [CrossRef]
- Wichtmann, T.; Triantafyllidis, T. Inspection of a High-Cycle Accumulation Model for Large Numbers of Cycles (N = 2 Million). Soil Dyn. Earthq. Eng. 2015, 75, 199–210. [Google Scholar] [CrossRef]
- Lukkezen, T. Implementation and Inspection of a High-Cycle Accumulation Model; TU Delft: Delft, The Netherlands, 2016. [Google Scholar]
- Häcker, A. Erweiterung eines Lamellenmodells für Zyklisch Belastete Flachgründungen (Extension of a Laminal Modell for Cyclic Loaded Foundations). Graduation Thesis, Karlsruher Institute für Technologie, Institute für Bodenmechanik und Felsmechanik, Karlsruhe, Germany, 2013. [Google Scholar]
- Wöhrle, T. Überprüfung und Entwicklung Einfaher Ingenieurmodelle für Offshore-Windenerigealagen auf Bass Eines Akkumulationsmodells (Examination and Development of Simplified Engineering Oriented Model for Offshore Wind Turbins on the Basis of an Accumulation Modell). Graduation Thesis, Karlsruher Institute für Technologie, Institute für Bodenmechanik und Felsmechanik, Karlsruhe, Germany, 2012. [Google Scholar]
- Zachert, H. Zur Gebrauchstauglichkeit von Gründungen für Offshore-Windenergieanlagen (On the Usability of Foundations for Offshore Wind Turbins). Ph.D. Thesis, Veröffentlichungen des Institutes für Bodenmechanik und Felsmechanik am Karlsruher Institut für Technologie (KIT); Karlsruher Institut für Technologie (KIT), Karlsruhe, Germany, 2015. [Google Scholar]
- Káli, A. Ipari Padlók Ágyazatként és Útépítési Védőrétegként Használt Durvaszemcsés Talaj Sajátmodulusának Numerikus Modellezése (Numerical Analysis of the Bearing Capacity Modulus of Course Soils Used in Pavement and Industrial Slab Layers). BSc Thesis, Budapest University of Technology and Economics, Budapest, Hungary, 2020. (In Hungarian). [Google Scholar]
- Rondón, H.A.; Wichtmann, T.; Triantafyllidis, T.; Lizcano, A. Hypoplastic Material Constants for a Well-Graded Granular Material for Base and Subbase Layers of Flexible Pavements. Acta Geotech. 2007, 2, 113–126. [Google Scholar] [CrossRef]
- Útügyi Lapok, Kutatási Jelentés: Tervezési Útmutató: Aszfaltburkolatú Útpályaszerkezetek Méretezésének Alternatív Módszere; (Research Report: Design Guide: Alternative Design Method of Asphalt Pavement Structures). 2016. Available online: https://utugyilapok.hu/wp-content/uploads/2015/11/Analitikus-TU.pdf (accessed on 30 August 2023).
- Vámos, M.J.; Szendefy, J. Overconsolidated Stress and Strain Condition of Pavement Layers as a Result of Preloading during Construction. Period. Polytech. Civil Eng. 2023, 67, 1273–1283. [Google Scholar] [CrossRef]
- Wichtmann, T.; Triantafyllidis, T. Effect of Uniformity Coefficient on G/Gmax and Damping Ratio of Uniform to Well-Graded Quartz Sands. J. Geotech. Geoenviron. Eng. 2013, 139, 59–72. [Google Scholar] [CrossRef]
- Wichtmann, T.; Triantafyllidis, T. Influence of the Grain-Size Distribution Curve of Quartz Sand on the Small Strain Shear Modulus Gmax. J. Geotech. Geoenviron. Eng. 2009, 135, 1404–1418. [Google Scholar] [CrossRef]
- Triantafyllidis, T.; Niemunis, A.; Kudella, P.; Wichtmann, T.; Solf, O.; Wienbroer, H.; Zahert, H.; Chrisopoulos, S. Abschlussbericht 0327618 zum Verbundprojekt Geotechnische Robustheit und Selbsteilung bei der Gründung von Offshore-Windenergieanlagen, Technischer Bericht; Karlsruher Institut für Technologie (KIT): Karlsruhe, Germany, 2011. [Google Scholar]
- AASHTO. AASHTO Guide for Design of Pavement Structures; American Association of State Highway and Transportation Officials: Washington, DC, USA, 1993; ISBN 978-1-56051-055-0. [Google Scholar]
- Nübel, K.; Karcher, C.; Herle, I. Ein Einfaches Konzept Zur Abschätzung von Setzungen. Geotechnik 1999, 4, 251–258. [Google Scholar]





| Influencing Parameter | Function | Material Constants |
|---|---|---|
| Strain amplitude | ||
| Cyclic preloading | ||
| Average mean pressure | ||
| Average stress ratio | ||
| Void ratio |
| Traffic Load Class | Design Traffic (Million Axles) | Thickness of Base Course (cm) | Thickness of AC-Layer (cm) |
|---|---|---|---|
| A | 0.03–0.1 | 20 | 10 |
| B | 0.1–0.3 | 20 | 12 |
| C | 0.3–1.0 | 20 | 15 |
| D | 1.0–3.0 | 20 | 18 |
| E | 3.0–10.0 | 20 | 22 |
| K | 10.0–30.0 | 20 | 25 |
| R | Over 30 | 20 | 29 |
| Layer | d50 (mm) | CU (-) | emin (-) | emax (-) | ρdmax (g/cm3) | e0 (-) | υ (-) |
|---|---|---|---|---|---|---|---|
| Subgrade 1 | 0.2 | 3.0 | 0.540 | 0.920 | 1.75 | 0.627 | 0.33–0.36 |
| Subgrade 2 | 2.0 | 3.0 | 0.491 | 0.783 | 1.81 | 0.577 | 0.33–0.36 |
| Subgrade 3 | 0.6 | 3.0 | 0.474 | 0.829 | 1.83 | 0.559 | 0.33–0.36 |
| Subgrade 4 | 0.6 | 5.0 | 0.394 | 0.749 | 1.93 | 0.478 | 0.35–0.38 |
| Subgrade 5 | 0.6 | 8.0 | 0.356 | 0.673 | 1.98 | 0.439 | 0.36–0.40 |
| Subbase | 2.0 | 11.9 | 0.364 | 0.513 | 2.06 | 0.340 | 0.40 |
| Base | 6.3 | 100.0 | 0.230 | 0.440 | 2.30 | 0.188 | 0.40 |
| Layer | Campl | Ce | Cp | CY | CN1 | CN2 | CN3 | fcc |
|---|---|---|---|---|---|---|---|---|
| Subgrade 1 (L26) | 1.70 | 0.513 | 0.47 | 2.26 | 5.49·10−3 | 1.30·10−2 | 2.38·10−5 | 32.76° |
| Subgrade 2 (L19) | 1.70 | 0.466 | 0.21 | 2.98 | 2.11·10−3 | 2.77·10−2 | 1.22·10−5 | 34.73° |
| Subgrade 3 (L12) | 1.70 | 0.450 | 0.41 | 2.60 | 3.88·10−3 | 1.54·10−2 | 2.05·10−5 | 33.20° |
| Subgrade 4 (L14) | 1.70 | 0.374 | 0.41 | 2.60 | 8.44·10−3 | 6.72·10−3 | 3.21·10−5 | 33.20° |
| Subgrade 5 (L16) | 1.70 | 0.338 | 0.41 | 2.60 | 1.53·10−2 | 5.67·10−3 | 4.53·10−5 | 33.20° |
| Base | 1.10 | 0.070 | −0.22 | 1.80 | 5.20·10−4 | 0.03 | 1.30·10−5 | 44° |
| Subbase | 1.10 | 0.204 | −0.22 | 1.80 | 5.20·10−4 | 0.03 | 1.30·10−5 | 42° |
| Layer | k1 (psi) | k1 (MPa) | k2 (-) |
|---|---|---|---|
| Granular base course | 3000–8000 | 20.6–55.2 | 0.5–0.7 |
| Granular subbase course | 2500–7000 | 17.2–48.3 | 0.4–0.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vamos, M.J.; Szendefy, J. Calculation Method for Traffic Load-Induced Permanent Deformation in Soils under Flexible Pavements. Geotechnics 2023, 3, 955-974. https://doi.org/10.3390/geotechnics3030051
Vamos MJ, Szendefy J. Calculation Method for Traffic Load-Induced Permanent Deformation in Soils under Flexible Pavements. Geotechnics. 2023; 3(3):955-974. https://doi.org/10.3390/geotechnics3030051
Chicago/Turabian StyleVamos, Mate Janos, and Janos Szendefy. 2023. "Calculation Method for Traffic Load-Induced Permanent Deformation in Soils under Flexible Pavements" Geotechnics 3, no. 3: 955-974. https://doi.org/10.3390/geotechnics3030051
APA StyleVamos, M. J., & Szendefy, J. (2023). Calculation Method for Traffic Load-Induced Permanent Deformation in Soils under Flexible Pavements. Geotechnics, 3(3), 955-974. https://doi.org/10.3390/geotechnics3030051





