Analysis of the Mobilization of an Unsaturated Infinite Natural Slope Due to Strength Reduction
Abstract
1. Introduction
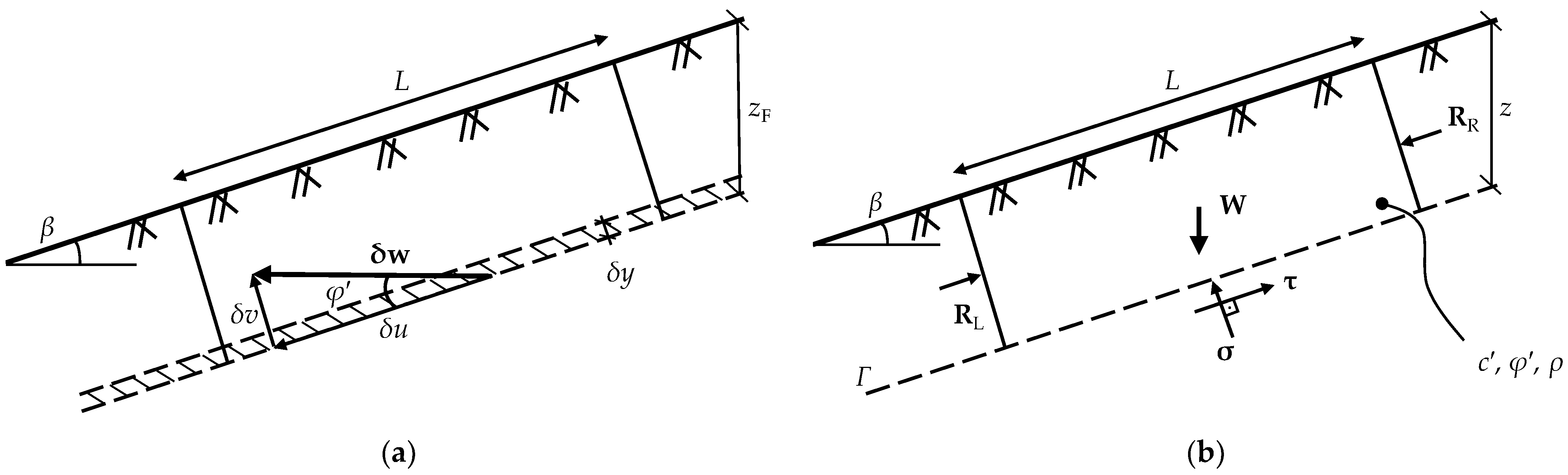
2. System Analysis and Conceptual Framework Adopted
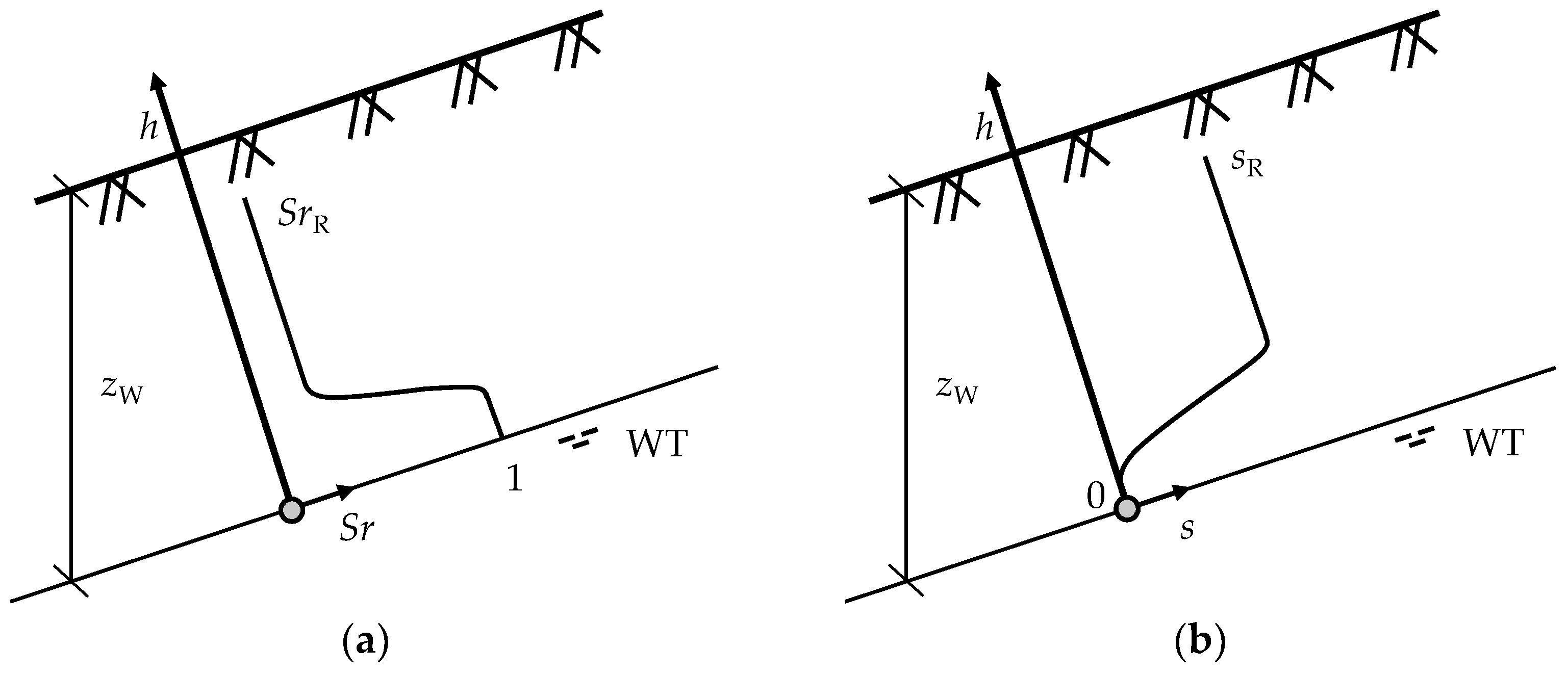
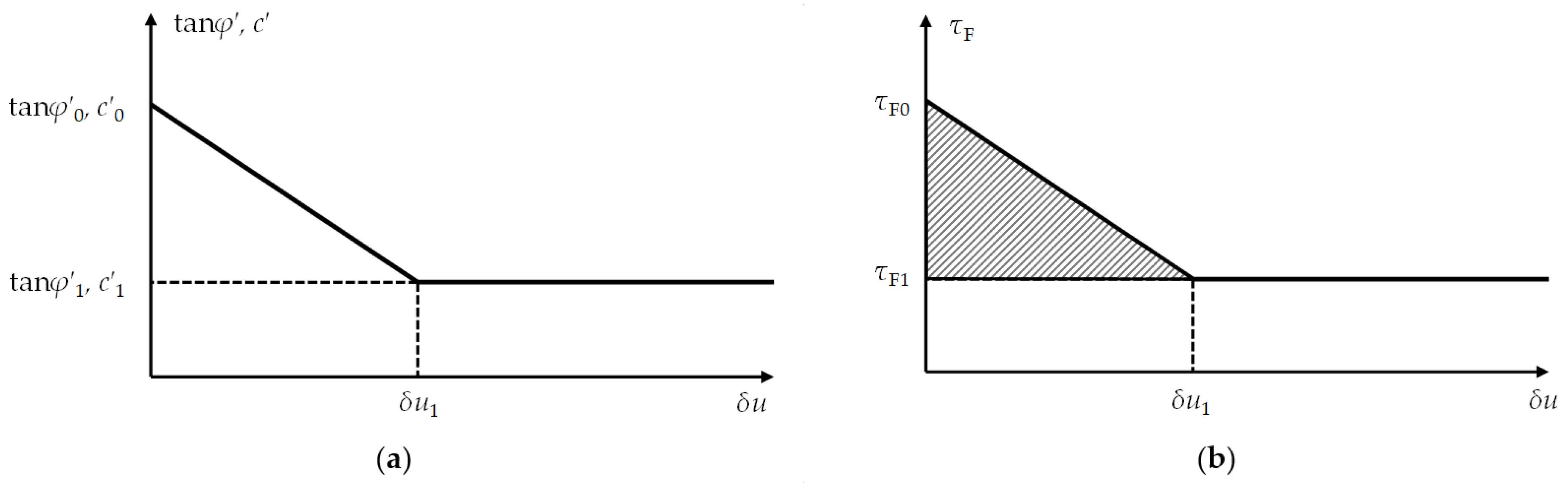
3. Failure and Mobilization
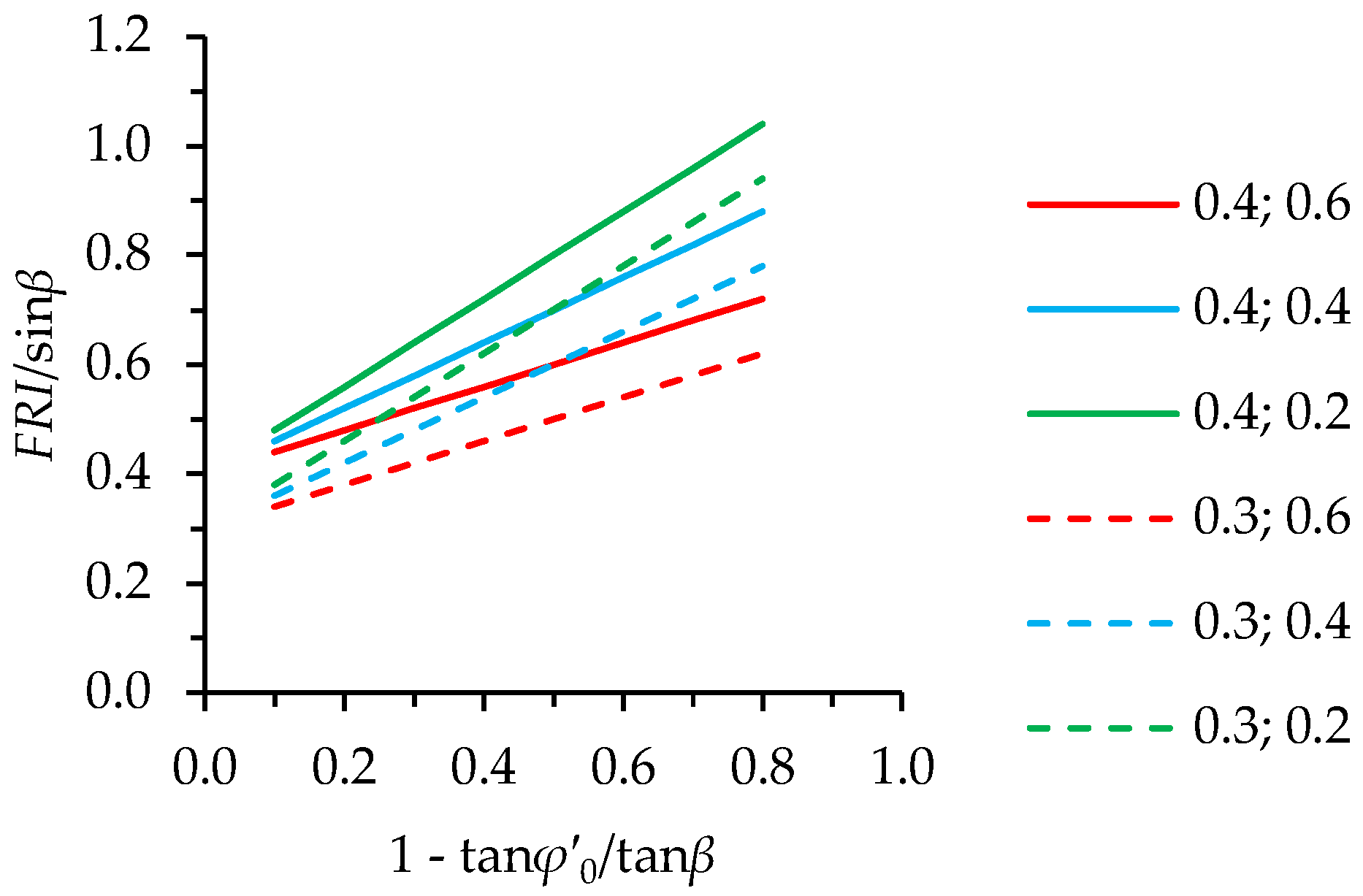
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lacasse, S.; Nadim, F.; Kalsnes, B. Living with Landslide Risk. Geotech. Eng. J. SEAGS AGSSEA 2010, 41, 4. [Google Scholar]
- Shanmugam, G.; Wang, Y. The Landslide Problem. J. Palaeogeogr. 2015, 4, 109–166. [Google Scholar] [CrossRef]
- Gariano, S.L.; Guzzetti, F. Landslides in a Changing Climate. Earth Sci. Rev. 2016, 162, 227–252. [Google Scholar] [CrossRef]
- Puzrin, A.M. Editorial: Modern Trends in Landslide Mechanics. Géotechnique 2016, 66, 173–174. [Google Scholar] [CrossRef]
- Taylor, D.W. Fundamentals of Soil Mechanics; John Willey & Sons: New York, NY, USA, 1948. [Google Scholar]
- Skempton, A.W.; Delory, F.A. Stability of Natural Slopes in London Clay. In Proceedings of the 4th International Conference on Soil Mechanics and Foundation Engineering; Butterworths Scientific Publications: London, UK, 1957; pp. 378–381. [Google Scholar]
- Duncan, J.M.; Wright, S.G.; Brandon, T.L. Soil Strength and Slope Stability; John Willey & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Ng, C.W.W.; Liu, H.W.; Feng, S. Analytical Solutions for Calculating Pore-Water Pressure in an Infinite Unsaturated Slope with Different Root Architectures. Can. Geotech. J. 2015, 52, 1981–1992. [Google Scholar] [CrossRef]
- Dey, R.; Hawlader, B.; Phillips, R.; Soga, K. Modeling of Large-Deformation Behaviour of Marine Sensitive Clays and Its Application to Submarine Slope Stability Analysis. Can. Geotech. J. 2016, 53, 1138–1155. [Google Scholar] [CrossRef]
- Huang, W.; Leong, E.-C.; Rahardjo, H. Upper-Bound Limit Analysis of Unsaturated Soil Slopes under Rainfall. J. Geotech. Geoenvironmental Eng. 2018, 144, 04018066. [Google Scholar] [CrossRef]
- Zhang, L.L.; Zhang, J.; Zhang, L.M.; Tang, W.H. Stability Analysis of Rainfall-Induced Slope Failure: A Review. Proc. Inst. Civ. Eng.-Geotech. Eng. 2011, 164, 299–316. [Google Scholar] [CrossRef]
- Chen, W.-F. Limit Analysis and Soil Plasticity; Elsevier Science: Amsterdam, The Netherlands, 1975. [Google Scholar]
- Atkinson, J. The Mechanics of Soils and Foundations; Taylor & Francis: New York, NY, USA, 2007. [Google Scholar]
- Lim, T.T.; Rahardjo, H.; Chang, M.F.; Fredlund, D.G. Effect of Rainfall on Matric Suctions in a Residual Soil Slope. Can. Geotech. J. 1996, 33, 618–628. [Google Scholar] [CrossRef]
- Hungr, O.; Corominas, J.; Eberhardt, E. Estimating Landslide Motion Mechanism, Travel Distance and Velocity. In Landslide Risk Management; Hungr, O., Fell, R., Couture, R., Eberhardt, E., Eds.; Taylor & Francis Group: London, UK, 2005; pp. 99–128. [Google Scholar]
- Rahardjo, H.; Lee, T.T.; Leong, E.C.; Rezaur, R.B. Response of a Residual Soil Slope to Rainfall. Can. Geotech. J. 2005, 42, 340–351. [Google Scholar] [CrossRef]
- Kim, J.; Salgado, R.; Lee, J. Stability Analysis of Complex Soil Slopes Using Limit Analysis. J. Geotech. Geoenvironmental Eng. 2002, 128, 546–557. [Google Scholar] [CrossRef]
- Iverson, R.M. Regulation of Landslide Motion by Dilatancy and Pore Pressure Feedback. J. Geophys. Res. 2005, 110, F02015. [Google Scholar] [CrossRef]
- Alonso, E.E.; Pereira, J.-M.; Vaunat, J.; Olivella, S. A Microstructurally Based Effective Stress for Unsaturated Soils. Géotechnique 2010, 60, 913–925. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Morgenstern, N.R.; Widger, R.A. The Shear Strength of Unsaturated Soils. Can. Geotech. J. 1978, 15, 313–321. [Google Scholar] [CrossRef]
- Sheng, D. Review of Fundamental Principles in Modelling Unsaturated Soil Behaviour. Comput. Geotech. 2011, 38, 757–776. [Google Scholar] [CrossRef]
- Sheng, D.; Zhou, A.; Fredlund, D.G. Shear Strength Criteria for Unsaturated Soils. Geotech. Geol. Eng. 2011, 29, 145–159. [Google Scholar] [CrossRef]
- Terzaghi, K. Theoretical Soil Mechanics; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1943. [Google Scholar]
- Potts, D.M.; Dounias, G.T.; Vaughan, P.R. Finite Element Analysis of Progressive Failure of Carsington Embankment. Géotechnique 1990, 40, 79–101. [Google Scholar] [CrossRef]
- Chen, X.; Zhu, H.; Huang, J.; Liu, D. Stability Analysis of an Ancient Landslide Considering Shear Strength Reduction Behavior of Slip Zone Soil. Landslides 2016, 13, 173–181. [Google Scholar] [CrossRef]
- Iverson, R.M. Comment on “The Reduction of Friction in Long-Runout Landslides as an Emergent Phenomenon” by Brandon C. Johnson et Al. J. Geophys Res. Earth Surf. 2016, 121, 2238–2242. [Google Scholar] [CrossRef]
- Hungr, O. Dynamics of Rapid Landslides. In Progress in Landslide Science; Springer: Berlin/Heidelberg, Germany, 2007; pp. 47–57. [Google Scholar]

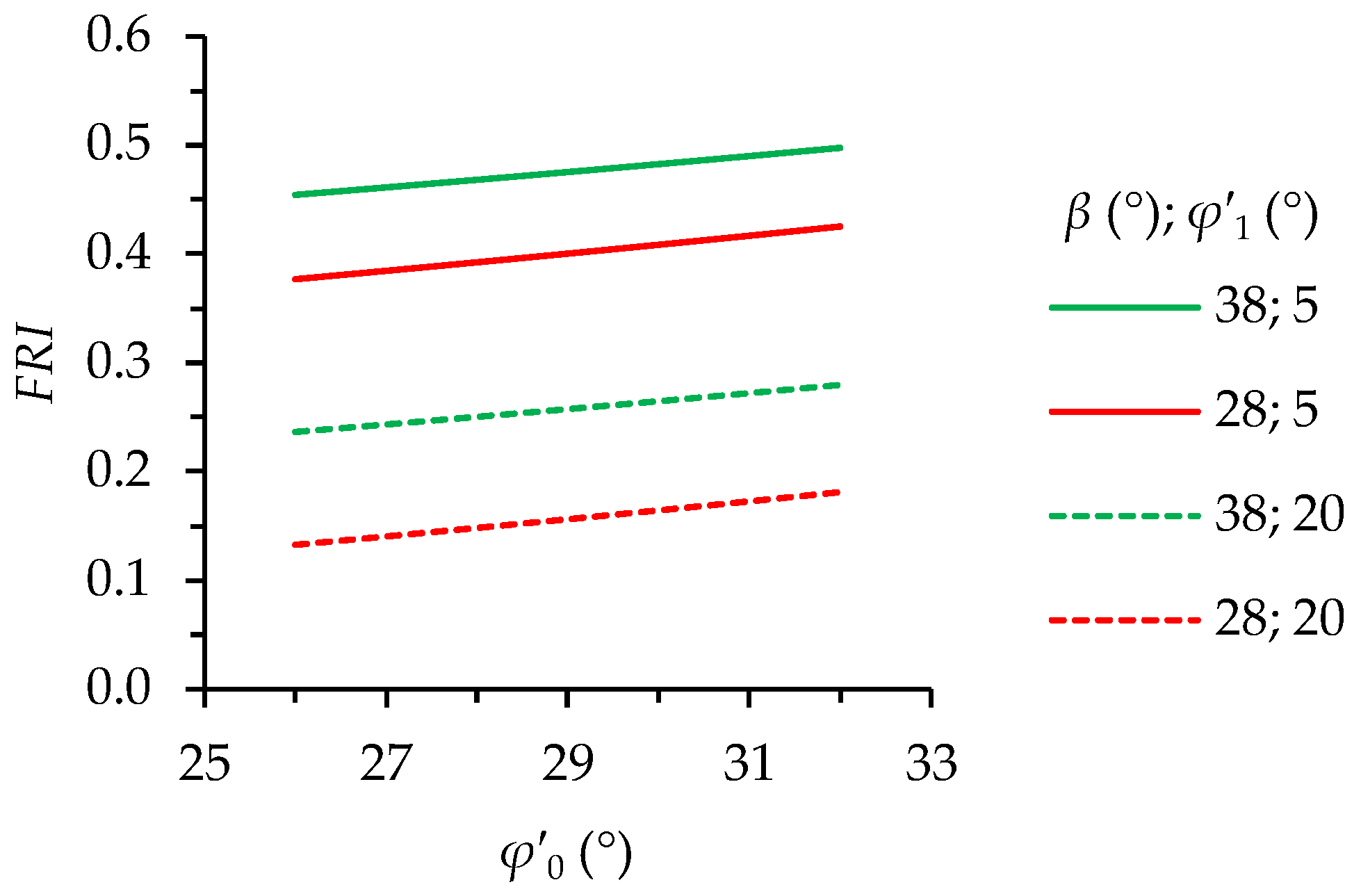

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Navarro, V.; De la Morena, G.; Torres-Serra, J.; Ruiz, E.; Asensio, L. Analysis of the Mobilization of an Unsaturated Infinite Natural Slope Due to Strength Reduction. Geotechnics 2022, 2, 1027-1034. https://doi.org/10.3390/geotechnics2040048
Navarro V, De la Morena G, Torres-Serra J, Ruiz E, Asensio L. Analysis of the Mobilization of an Unsaturated Infinite Natural Slope Due to Strength Reduction. Geotechnics. 2022; 2(4):1027-1034. https://doi.org/10.3390/geotechnics2040048
Chicago/Turabian StyleNavarro, Vicente, Gema De la Morena, Joel Torres-Serra, Emilio Ruiz, and Laura Asensio. 2022. "Analysis of the Mobilization of an Unsaturated Infinite Natural Slope Due to Strength Reduction" Geotechnics 2, no. 4: 1027-1034. https://doi.org/10.3390/geotechnics2040048
APA StyleNavarro, V., De la Morena, G., Torres-Serra, J., Ruiz, E., & Asensio, L. (2022). Analysis of the Mobilization of an Unsaturated Infinite Natural Slope Due to Strength Reduction. Geotechnics, 2(4), 1027-1034. https://doi.org/10.3390/geotechnics2040048






