Effects of Different Target Distances on the Kinematics of Hip, Knee, and Ankle Joints in the Fencing Lunge
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Trial Conditions
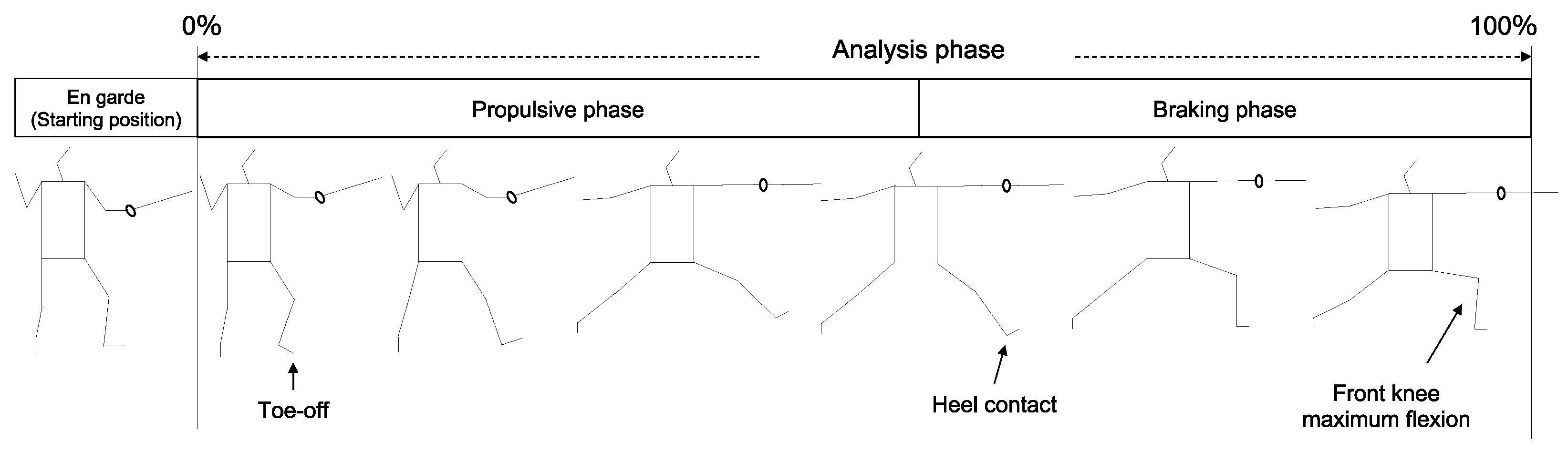
2.3. Procedure
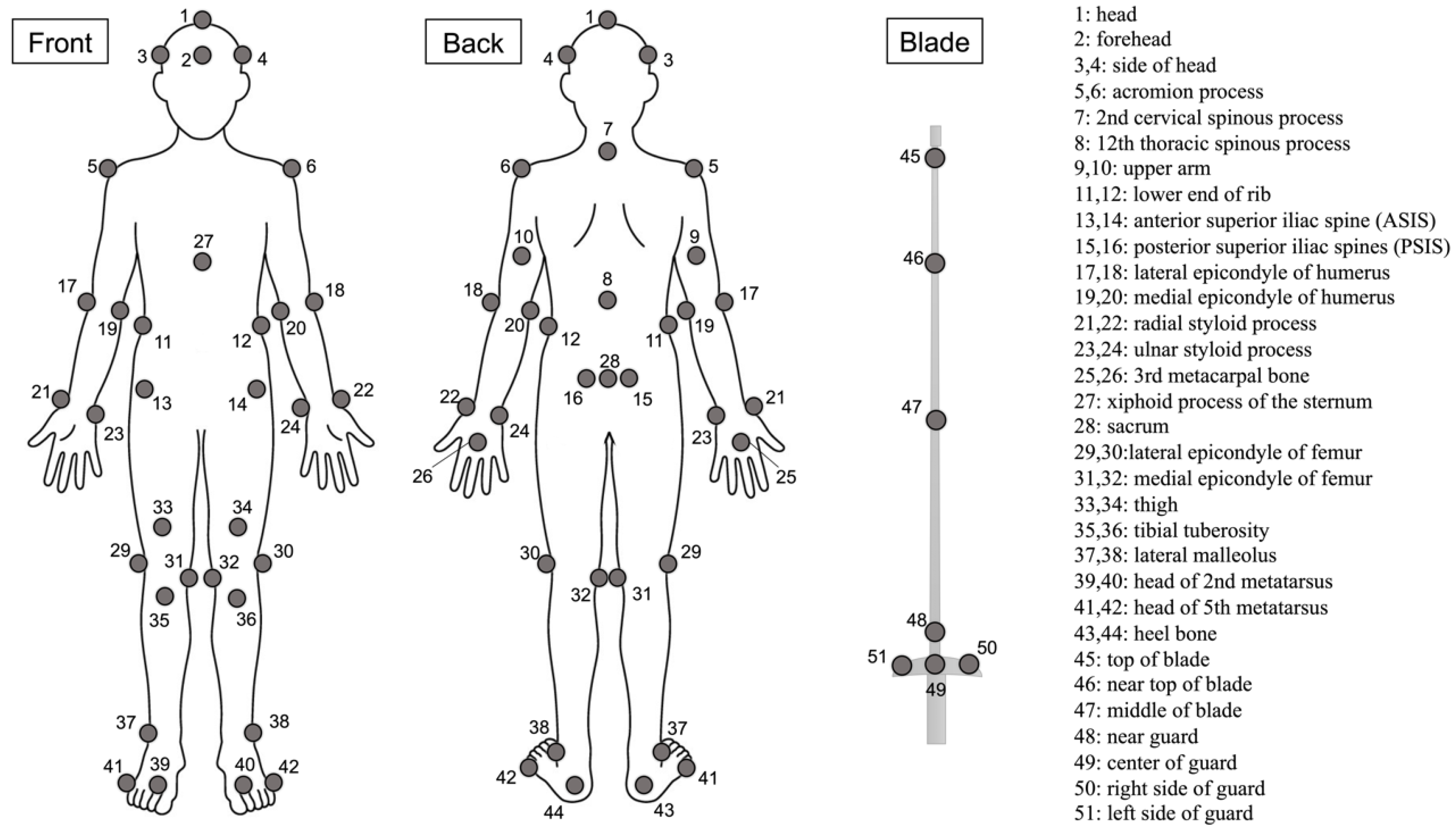
2.4. Data Collection
2.5. Data Analysis
2.6. Statistical Analyses
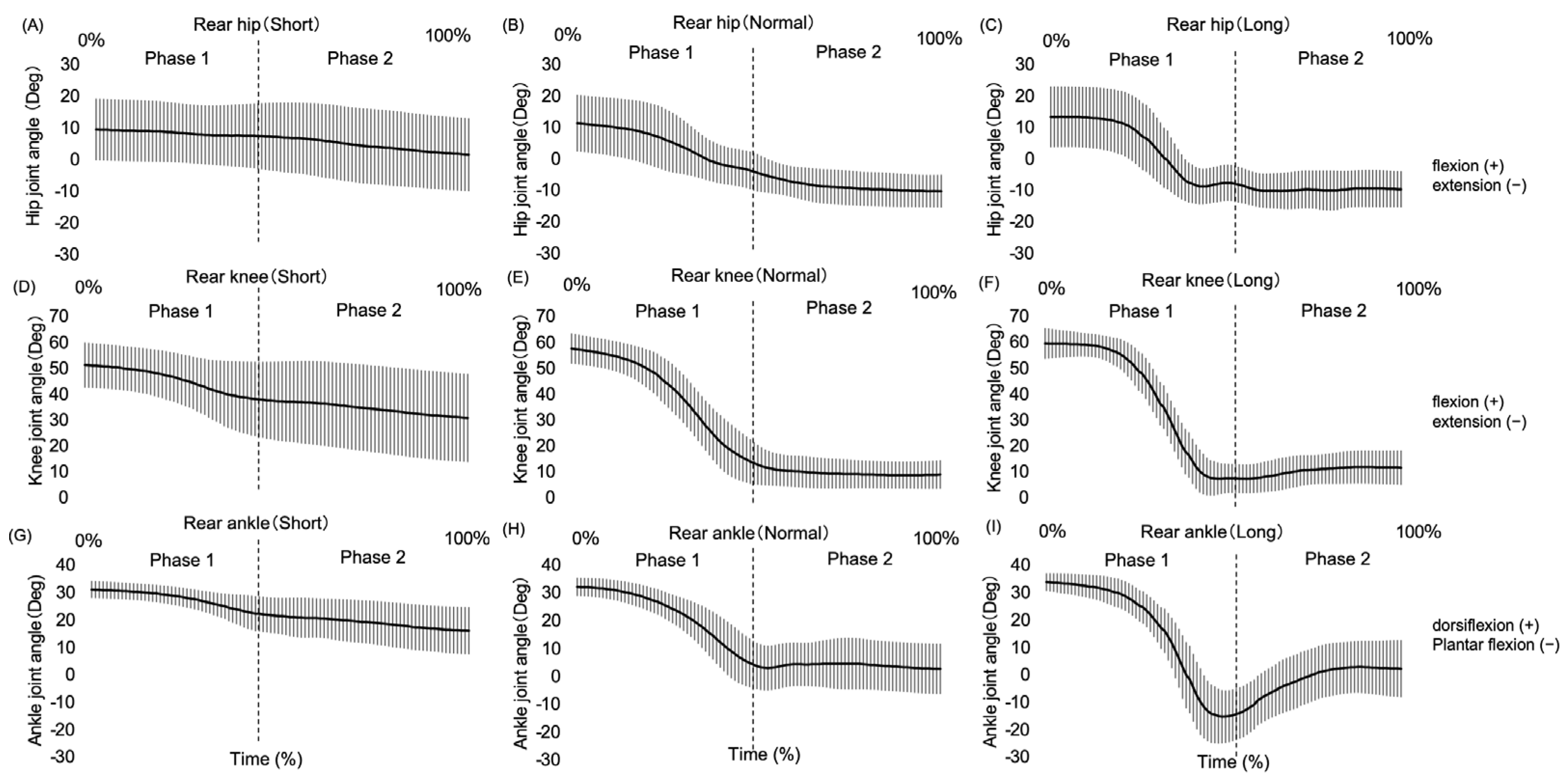
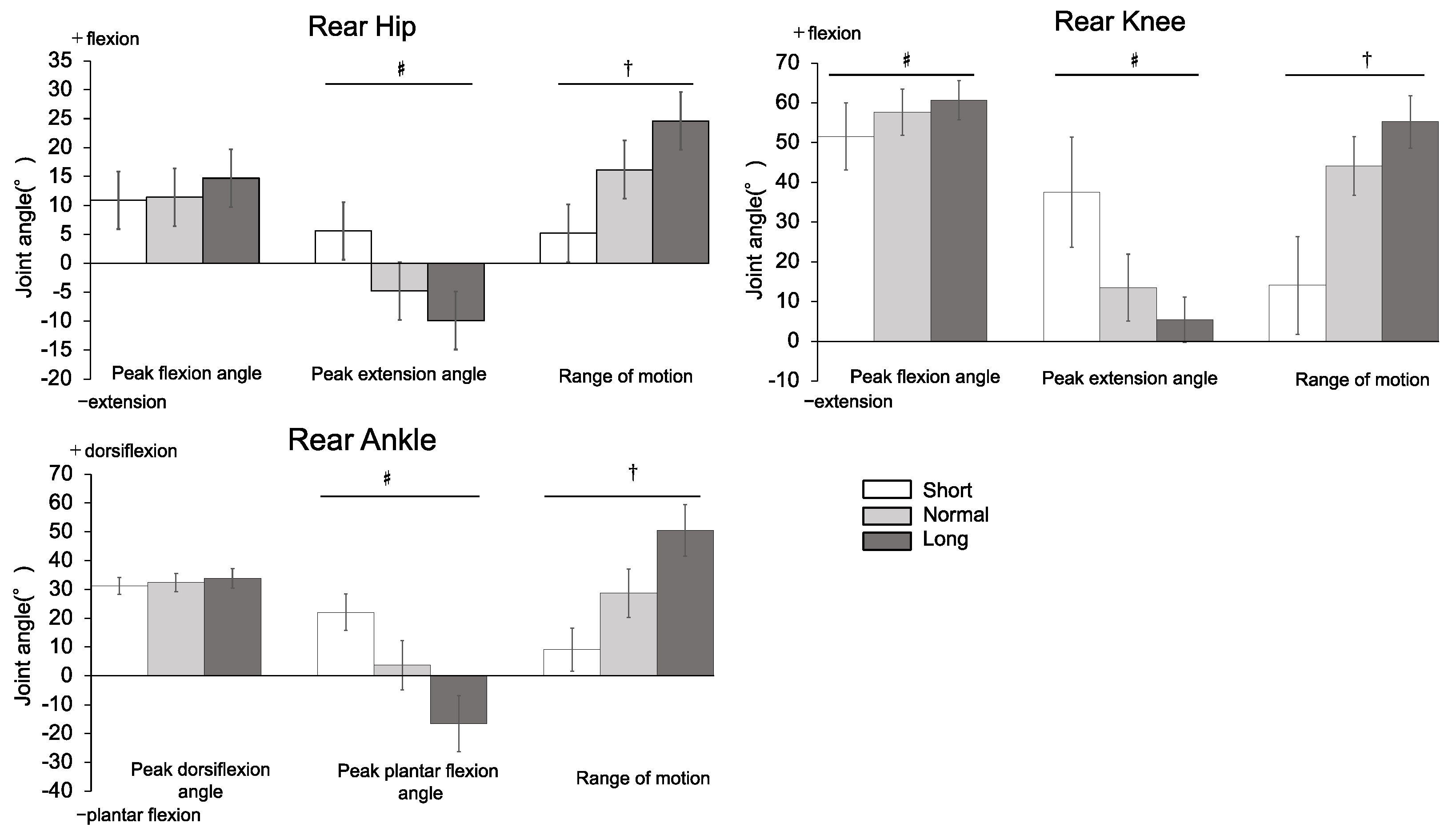
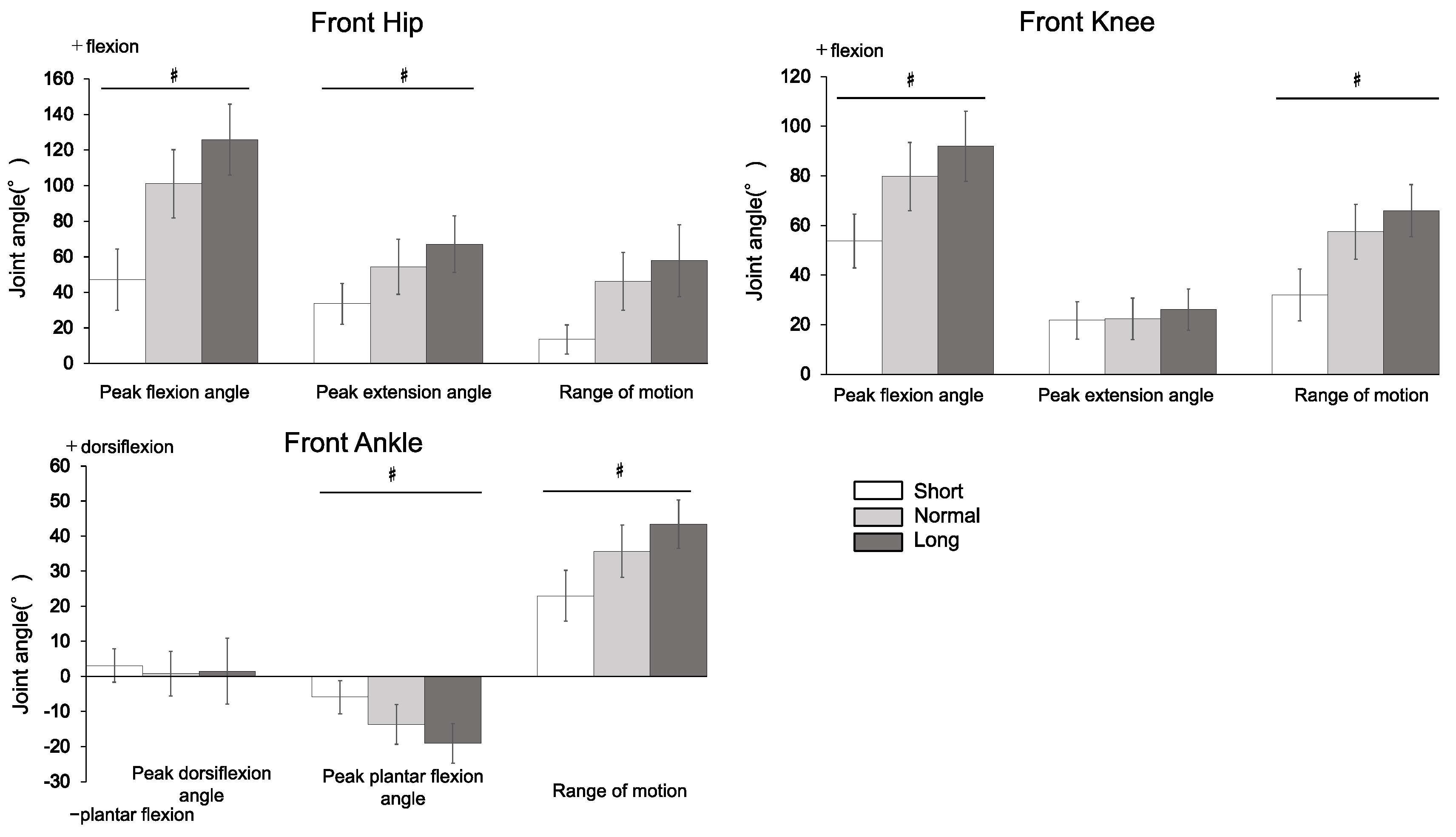
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barth, B.; Beck, E. Performance structure, performance capacity and performance development (Chapter 2). In The Complete Guide to Fencing; Barth, B., Beck, E., Eds.; Meyer & Meyer Verlag: Aachen, Germany, 2007. [Google Scholar]
- Aquili, A.; Tancredi, V.; Triossi, T.; De Sanctis, D.; Padua, E.; D’Arcangelo, G.; Melchiorri, G. Performance analysis in sabre. J. Strength Cond. Res. 2013, 27, 624–630. [Google Scholar] [CrossRef]
- Turner, A.; Bishop, C.; Chavda, S.; Edwards, M.; Brazier, J.; Kilduff, L.P. Physical Characteristics Underpinning Lunging and Change of Direction Speed in Fencing. J. Strength Cond. Res. 2016, 30, 2235–2241. [Google Scholar] [CrossRef] [PubMed]
- Wojciechowski, Z. Principles of coaching (Chapter 2). In This is Fencing! Advanced Training and Performance Principles for Foil; Wojciechowski, Z., Ed.; Crowood: Marlborough, UK, 2019. [Google Scholar]
- Gholipour, M.; Tabrizi, A.; Farahmand, F. Kinematics analysis of lunge fencing using stereophotogrametry. World J. Sport Sci. 2008, 1, 32–37. [Google Scholar]
- Bottoms, L.; Greenhalgh, A.; Sinclair, J. Kinematic determinants of weapon velocity during the fencing lunge in experienced épée fencers. Acta Bioeng. Biomech. 2013, 15, 109–113. [Google Scholar] [PubMed]
- Chida, K.; Inami, T.; Yamaguchi, S.; Yoshida, Y.; Kohtake, N. Relationship between Body Center of Mass Velocity and Lower Limb Joint Angles during Advance Lunge in Skilled Male University Fencers. Biomechanics 2023, 3, 377–388. [Google Scholar] [CrossRef]
- Gresham-Fiegel, C.N.; House, P.D.; Zupan, M.F. The effect of nonleading foot placement on power and velocity in the fencing lunge. J. Strength Cond. Res. 2013, 27, 57–63. [Google Scholar] [CrossRef] [PubMed]
- Guan, Y.; Guo, L.; Wu, N.; Zhang, L.; Warburton, D.E.R. Biomechanical insights into the determinants of speed in the fencing lunge. Eur. J. Sport Sci. 2018, 18, 201–208. [Google Scholar] [CrossRef]
- Guilhem, G.; Giroux, C.C.; Couturier, A.; Chollet, D.; Rabita, G. Mechanical and muscular coordination patterns during a high-level fencing assault. Med. Sci. Sports Exerc. 2014, 46, 341–350. [Google Scholar] [CrossRef] [PubMed]
- Morris, N.; Farnsworth, M.; Robertson, D.G. Kinetic analyses of two fencing attacks—Lunge and fleche. Port. J. Sport Sci. 2011, 11, 343–346. [Google Scholar]
- Mulloy, F.; Mullineaux, D.R.; Irwin, G. Use of the kinematic chain in the fencing attacking lunge. In Proceedings of the International Conference on Biomechanics in Sports, Poitiers, France, 28 June–2 July 2016; pp. 1114–1117. [Google Scholar]
- Chida, K.; Inami, T.; Yamaguchi, S.; Nishioka, T.; Yoshida, Y.; Kohtake, N. Assessing the validity of two-dimensional video analysis for measuring lower limb joint angles during fencing lunge. Front. Sports Act. Living 2024, 6, 1335272. [Google Scholar] [CrossRef] [PubMed] [PubMed Central]
- Chen, T.L.; Wong, D.W.; Wang, Y.; Ren, S.; Yan, F.; Zhang, M. Biomechanics of fencing sport: A scoping review. PLoS ONE 2017, 12, e0171578. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.W.; Kwon, M.S.; Yenuga, S.S.; Kwon, Y.H. The effects of target distance on pivot hip, trunk, pelvis, and kicking leg kinematics in Taekwondo roundhouse kicks. Sports Biomech. 2010, 9, 98–114. [Google Scholar] [CrossRef] [PubMed]
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences, 2nd ed.; Lawrence Erlbaum: Hillsdale, NJ, USA, 1988. [Google Scholar]
- Gutiérrez-Dávila, M.; Rojas, F.J.; Antonio, R.; Navarro, E. Response timing in the lunge and target change in elite versus medium-level fencers. Eur. J. Sport Sci. 2013, 13, 364–371. [Google Scholar] [CrossRef] [PubMed]
- Williams, L.R.; Walmsley, A. Response amendment in fencing: Differences between elite and novice subjects. Percept. Mot. Ski. 2000, 91, 131–142. [Google Scholar] [CrossRef] [PubMed]
- Dempster, W.T. Space requirements of the seated operator. Geometrical kinematic and mechanical aspects of the body with special reference to the limbs. Tech. Rep. 1955, 55/159, 1–254. [Google Scholar]
- Bell, A.L.; Brand, R.A.; Pedersen, D.R. Prediction of hip joint centre location from external landmarks. Hum. Mov. Sci. 1989, 8, 3–16. [Google Scholar] [CrossRef]
- Błażkiewicz, M.; Borysiuk, Z.; Gzik, M. Determination of loading in the lower limb joints during step-forward lunge in fencing. Acta Bioeng. Biomech. 2018, 20, 3–8. [Google Scholar] [PubMed]
- Thompson, K.; Chang, G.; Alaia, M.; Jazrawi, L.; Gonzalez-Lomas, G. Lower extremity injuries in U.S. national fencing team members and U.S. fencing Olympians. Phys. Sportsmed. 2022, 50, 212–217. [Google Scholar] [CrossRef] [PubMed]


| Target Distance Condition | p Values | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Variable | Short | Neutral | Long | F | ANOVA | Pairwise Comparisons | ηp2 | ||||||
| Horizontal displacement of CoM (cm) | 33.8 | ± | 10.5 | 67.3 | ± | 8.3 | 99.5 | ± | 8.3 | 283.366 | <0.001 | S-N: <0.001 | 0.953 |
| S-L: <0.001 | |||||||||||||
| N-L: <0.001 | |||||||||||||
| Peak velocity of CoM (m/s) | 1.03 | ± | 0.24 | 1.91 | ± | 0.18 | 2.47 | ± | 0.20 | 247.360 | <0.001 | S-N: <0.001 | 0.946 |
| S-L: <0.001 | |||||||||||||
| N-L: <0.001 | |||||||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chida, K.; Inami, T.; Yamaguchi, S.; Yoshida, Y.; Kohtake, N. Effects of Different Target Distances on the Kinematics of Hip, Knee, and Ankle Joints in the Fencing Lunge. Biomechanics 2024, 4, 309-318. https://doi.org/10.3390/biomechanics4020020
Chida K, Inami T, Yamaguchi S, Yoshida Y, Kohtake N. Effects of Different Target Distances on the Kinematics of Hip, Knee, and Ankle Joints in the Fencing Lunge. Biomechanics. 2024; 4(2):309-318. https://doi.org/10.3390/biomechanics4020020
Chicago/Turabian StyleChida, Kenta, Takayuki Inami, Shota Yamaguchi, Yasumasa Yoshida, and Naohiko Kohtake. 2024. "Effects of Different Target Distances on the Kinematics of Hip, Knee, and Ankle Joints in the Fencing Lunge" Biomechanics 4, no. 2: 309-318. https://doi.org/10.3390/biomechanics4020020
APA StyleChida, K., Inami, T., Yamaguchi, S., Yoshida, Y., & Kohtake, N. (2024). Effects of Different Target Distances on the Kinematics of Hip, Knee, and Ankle Joints in the Fencing Lunge. Biomechanics, 4(2), 309-318. https://doi.org/10.3390/biomechanics4020020






