Lower Extremity Joint Kinetics during Walk-to-Run and Run-to-Walk Transitions

Abstract

Lower extremity joint kinetic factors are thought to modulate walk-to-run transition (WRT) and run-to-walk transition (RWT). This study aimed to investigate joint stiffness and energetic patterns during the WRT and RWT processes and identify whether gait transitions occur within a single step or not. Ten healthy subjects participated in treadmill WRT (1.8–2.4 m/s) and RWT (2.4–1.8 m/s) tests. We investigated two steps before transition (S–2, S–1), two steps after transition (S1, S2) and the transition step (S0). We found significant differences between S2 and S–2 for ankle joint stiffness during WRT and RWT (p < 0.001); for hip joint stiffness, we found significant differences between S1 and S–1 during WRT and RWT (p ≤ 0.001). Additionally, stance phase mechanical energy generation was observed to transfer from proximal to distal joints at S0 during WRT, and from distal to proximal at S0 during RWT. Transition step ankle kinematic and kinetic patterns were similar to the target locomotion task gait format in both WRT and RWT. Moreover, RWT required longer adaptation time compared with WRT. These findings indicate that WRT and RWT were modulated before and after the actual transitions, not within a single step. Redistribution of joint mechanical work generation was related to gait transition triggers, which modulate the WRT and RWT processes.

1. Introduction

Walking and running have different gait characteristics [1] and whole-body center of mass (COM) dynamic patterns [1,2,3]. The transition between the walking inverted pendulum and running spring mass paradigm is primarily determined by stance phase lower extremity kinetic factors [4,5]. When walking at a constantly increasing speed or running at a constantly decreasing speed, walk-to-run transition (WRT) or run-to-walk transition (RWT) dependably occurs at a preferred transition speed (PTS) [6]. The magnitude of acceleration and deceleration affects the speed at which gait transition occurs [7].

Previous studies have investigated different factors to explain gait transition mechanisms. Generally, there are four proposed gait transition triggers: metabolic energy cost, mechanical cost, mechanical load, cognitive and perceptual modulation [8]. From a biomechanical perspective, mechanical cost and mechanical load may be the more important factors contributing to gait transition [5,8]. With regard to mechanical cost, muscle properties [9,10] and power generation efficiency [9] are related to mechanical energy expenditure [11]. Specifically, when walking compared with running at speeds above the PTS, or running compared with walking at speeds below PTS, more muscle fiber work is required, respectively [11]. There seems to be a feedback system associated with the neuromusculoskeletal system [9] to help minimize mechanical cost of locomotion [3,8,12,13], which triggers gait transition. Mechanical load trigger [5] is known as a protective muscle stress reduction and injury prevention mechanism [8,14,15]. Specifically, when walking above PTS or running below PTS, the protective mechanism minimizes peak loads to reduce injury risk [5,16,17], which leads to gait transition. Other studies have suggested that gait transition is an active, non-spontaneous reorganization process, based on the non-linear behavior of the vertical ground reaction force, joint kinetics and muscle activity patterns before the gait transition [18,19,20].

Lower extremity joint level kinetic patterns are closely related to musculoskeletal system mechanical efficiency and mechanical load mechanisms, which help to modulate gait transition. Previous studies have investigated lower extremity joint mechanics in walking and running across different speeds [21,22], the joint kinetic characteristics around the PTS [5,9] and gait kinematics and kinetics during the transition [2,16,17,23,24,25]. It was reported that different locomotion speeds or tasks result in the changes in joint level stiffness, mechanical work and power quantities [21], and that ankle and hip joint mechanics contribute to the WRT [5]. Specifically, lower extremity joint power generation tends to shift from hip to ankle joint when running above PTS compared with walking, as it is beneficial for positive mechanical work and power generation, as well as improving gait mechanical efficiency by switching gait patterns [5,9]. Additionally, a switch from walking to running near PTS has been reported to reduce the effort of ankle and hip muscles [5]. These findings indicate that the joint power transfer phenomenon between the ankle and hip is related to the gait transition trigger mechanisms [5]. Whether lower extremity joint kinetics are different within each step and whether the mechanical work and power transfer between each joint still exists during both WRT and RWT processes remains unknown and needs further investigation.

Previous studies which focused on incremental locomotion protocols around PTS reported that gait transition happens instantaneously at the transition step, and the change in joint kinetic quantities is regarded as a mechanical trigger component to modulate gait transition [5,17]. Another study focused on continuous protocols suggested that gait transition is an active reorganization process rather than an instantaneous event due to the non-linear patterns in some joint kinematic and kinetic quantities among steps before WRT [19]. Most previous studies were focused either on joint mechanics among the steps before WRT or the comparisons between different locomotion tasks around PTS. With these contradictions and gaps, if we regard the time before, during and after transition as a whole process, no studies have investigated joint kinetics during both WRT and RWT processes. From the joint kinetics aspect, it remains unknown whether WRT and RWT occur instantaneously at the transition step only or are modulated before and after transition.

The change in locomotion tasks or speeds is known to affect changes in joint mechanics: faster locomotion speeds required higher mechanical work and power generation [5,9,21,26], depending on modulation of lower extremity joint stiffness and moment angular impulse. Joint stiffness reflects joint elastic spring-like behavior under loading [21,27], and moment angular impulse represents the joint moment executed over time [28]. Both quantities reflect dynamic loading and response, and they are regarded as the end-effect of muscle functions during locomotion [5,21]. However, it remains unknown how continuously accelerated or decelerated locomotion speed influences the joint stiffness and moment angular impulse during transitions between walking and running. Further investigation can be helpful to identify whether the modulation of joint kinetics is an active reorganization process before and after transition or whether it occurs solely within the transition step. Moreover, lower extremity joints play different functional roles between stance and swing phase in both walking and running across different speeds [21]. Whether WRT and RWT require different functional roles for lower extremity joints and the differences between joint kinetic patterns remains unknown, and both need further investigation.

In this study, we aimed to investigate lower extremity joint stance phase dynamic loading and response, as well as stance and swing phase joint energetics during WRT and RWT processes. Further investigation of these patterns during WRT and RWT will benefit future lower extremity assistive device development, which might be designed to meet multiple locomotion tasks and be better suited for gait transition. Since lower extremity joint kinetic quantities increase with locomotion speeds [21] and the distal end joint generates more mechanical work when running above PTS [5,9], we hypothesized that (1) lower extremity joint stiffness increases during the WRT and decreases during the RWT; (2) joint work and extensor moment angular impulse increase during the WRT and decrease during the RWT process; (3) distal end joint generate more mechanical energy in stance phase during WRT and decrease during the RWT process.

2. Materials and Methods

2.1. Participants

Ten middle-aged healthy subjects (5 males, 5 females, 50.7 $\pm$ 6.0 years, 173.4 $\pm$ 11.4 cm, 69.7 $\pm$ 14.9 kg) participated in the study. All subjects signed informed written consent approved by the university’s institutional review board (RCS #07302015.030) before participation. All subjects self–reported to be free of lower extremity musculoskeletal injuries which would affect walking and running for the past 6 months.

2.2. Experimental Protocol and Data Collection

Fifty-five retro-reflective markers were placed on the skin surface of the subjects based on a previously published whole-body marker set [29]. Before the formal test, subjects were provided with a 10-min treadmill acceleration and deceleration practice. Then, the subjects were asked to complete the WRT protocol: they walked on a force-instrumented treadmill (Bertec, Inc., Columbus, OH, USA) at 1.8 m/s for 30 s, and then the treadmill was constantly accelerated at 0.1 m/s² up to 2.4 m/s. The subjects were asked to transition to a running gait whenever they felt ready during the acceleration process. After transitioning to a running gait, they ran at 2.4 m/s for another 30 s, before the treadmill was slowed down and stopped to allow a short break. Next, the subjects completed the RWT protocol: they ran at 2.4 m/s for 30 s, and then the treadmill was constantly decelerated at −0.1 m/s² down to 1.8 m/s. The subjects were asked to transition to a walking gait whenever they felt ready during the deceleration process. Once they transitioned to a walking gait, they walked at 1.8 m/s for another 30 s. Treadmill acceleration and deceleration magnitude for the WRT and RWT protocols were chosen based on previous work [25]. The subjects were required to complete at least two WRT and RWT trials. Segmental kinematic data were collected at 120 Hz using an 8-camera motion capture system (Motion Analysis Corp., Santa Rosa, CA, USA). Ground reaction force data were collected at 1200 Hz using the force-instrumented treadmill. Kinematic and kinetic data were filtered with a low-pass fourth-order Butterworth filter at 6 Hz and 50 Hz, respectively, based on previous studies [21,22,30,31,32].

2.3. Data Analysis

The transition step (S0) was determined based on the number of swing phases in vertical ground reaction force (GRF) data [18,19,20]. Specifically, the first step with dual swing phases was regarded as S0 during WRT, and the first step with a single swing phase was regarded as S0 during RWT [18,19,20]. Vertical GRF threshold was set at 20 N to identify the stance phase between heel strike and toe off. All outcome variable calculations and analyses were focused on two steps before gait transition (S–2, S–1), the transition step (S0) and two steps after transition (S1, S2) for both WRT and RWT. Lower extremity joint angles, moments and net joint powers were calculated using an inverse dynamics model in Visual 3D (C–Motion, Inc., Germantown, MD, USA). Joint stiffness ($K_{joint}$) was calculated as the change in sagittal plane joint moment (${∆M}_{joint}$) divided by sagittal plane joint angular displacement (${∆\theta }_{joint}$) in the braking phase of ground contact [27,33], expressed as

$$K_{joint}=\frac{{∆M}_{joint}}{{∆\theta }_{joint}}$$

(1)

Stance and swing phase joint positive work ($W_{joint}^{+}$) and negative work ($W_{joint}^{-}$) were calculated as the sum of all positive or negative net joint power integrated over time, respectively [26]. Stance phase joint extensor moment angular impulse ($I_{joint}$) was calculated as the sum of all stance phase extensor joint moment integrated over time [34,35]. Total lower extremity joint support moment impulse ($I_{total}$) was calculated as the sum of ankle, knee and hip joint stance phase extensor moment angular impulse [34,35], expressed as

$$I_{total}=I_{ankle}+I_{knee}+I_{hip}.$$

(2)

Group average net joint power curves were plotted for each of the five steps analyzed for WRT and RWT trials. Stance phase sagittal plane ankle joint angle–moment curves were averaged for further analysis.

Joint stiffness ($K_{joint}$), joint work ($W_{joint}$) and moment impulse ($I_{joint}$) were examined for differences between joints and steps before, during and after the transition using 2-way ANOVAs (joint $\times$ step) for WRT and RWT in SPSS (V22.0, IBM, Armonk, NY, USA). Total joint support moment impulse ($I_{total}$) was examined using a 1-way ANOVA to compare between the five steps tested during WRT and RWT, respectively. Initial alpha level was set to 0.05. When a main effect or interaction effects were detected, Bonferroni adjustments were used for pairwise comparison. The post hoc alpha level was adjusted based on the comparison numbers. Joint level main effect pairwise comparison adjusted $\alpha$ was set at 0.0167, step main effect pairwise comparison adjusted $\alpha$ was set at 0.005. Joint $\times$ step interaction effect pairwise comparison $\alpha$ was set at 0.0011. Additionally, to identify whether each transition step is the walking or running pattern, paired t-test was conducted using peak ankle angle and moment during each transition step and the actual walking and running conditions between 1.8 m/s and 2.2 m/s from the previous studies with the same subjects [22].

3. Results

3.1. Joint Stiffness

Joint stiffness ($K_{joint}$) mean values are presented in

Table 1. Joint stiffness (Nm/kg/deg) across WRT and RWT steps. Sample mean (SD); n = 10.

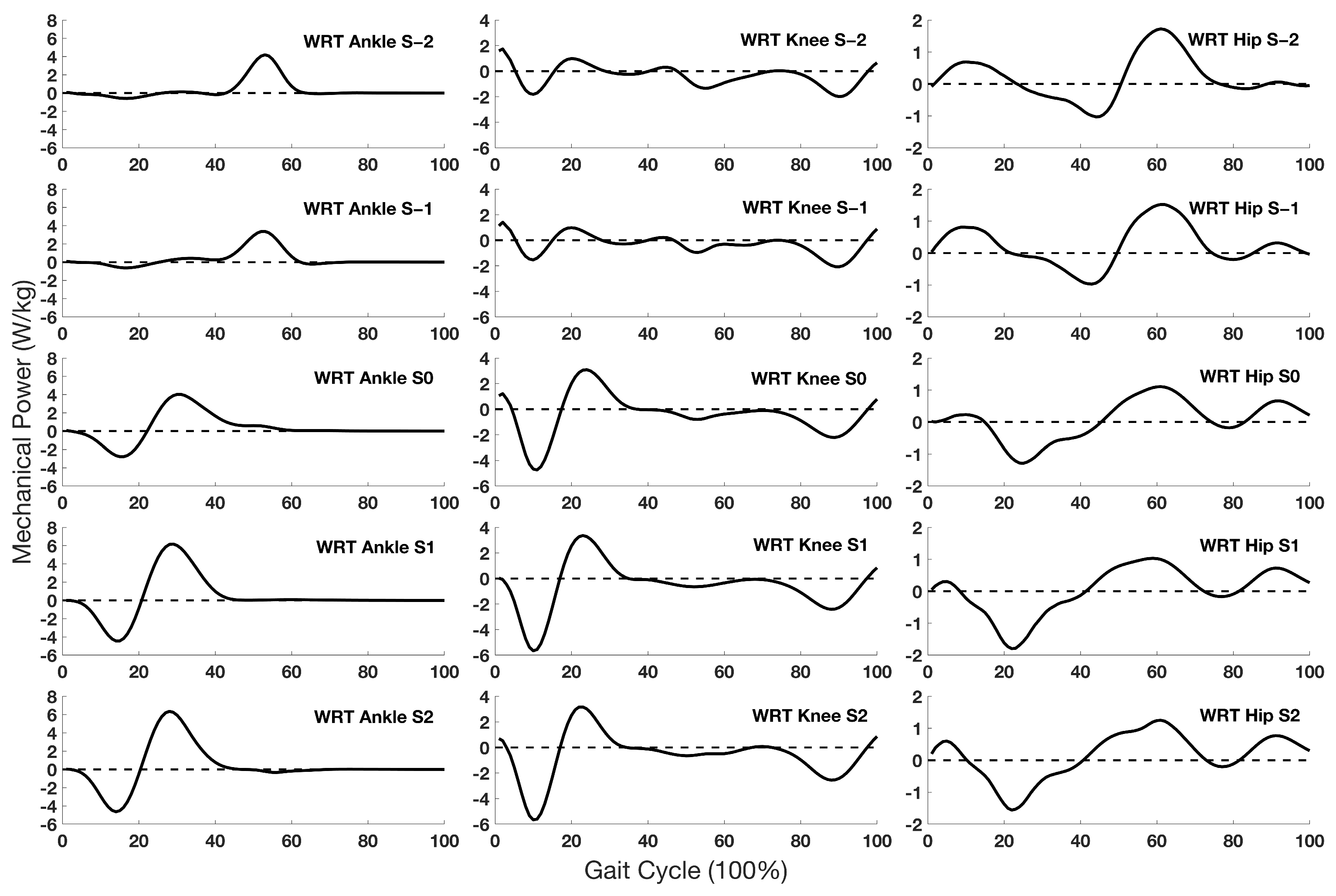
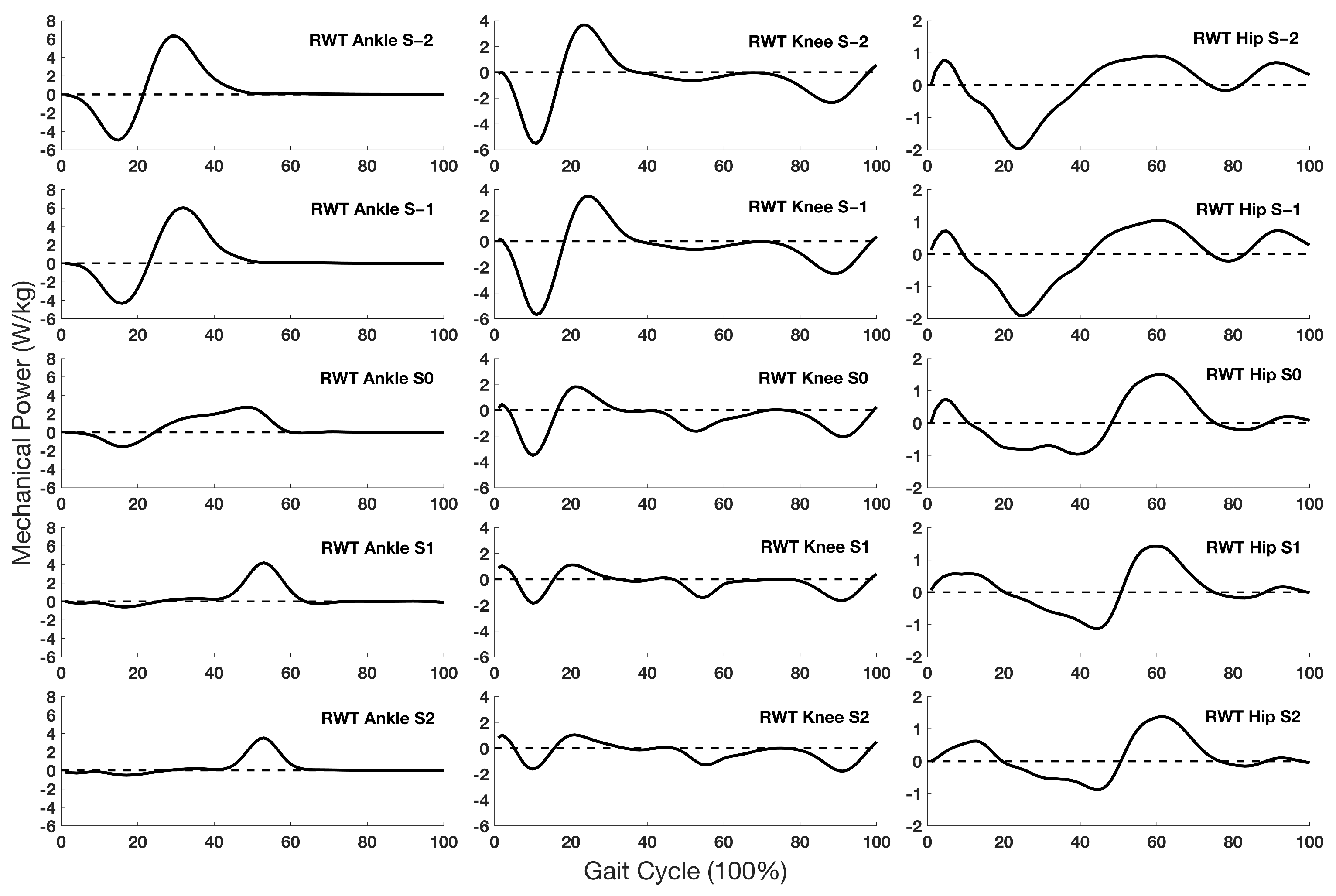
Joint Stiffness (Nm/kg/deg)	Steps
	S–2	S–1	S0	S1	S2
WRT
Ankle	0.13 (0.05) c	0.12 (0.04) c	0.16 (0.09) c	0.23 (0.11) b	0.24 (0.10) b,c
Knee	0.11 (0.05)	0.10 (0.03)	0.11 (0.06)	0.11 (0.05) a,b	0.11 (0.06) b
Hip	0.08 (0.03) d	0.08 (0.02) d	0.17 (0.09)	0.20 (0.06) a,d	0.24 (0.12)
RWT
Ankle	0.21 (0.09) e,g	0.18 (0.07) e,g	0.19 (0.10)	0.15 (0.06)	0.13 (0.06) g
Knee	0.12 (0.08) e,f	0.11 (0.07) e,f	0.12 (0.07)	0.12 (0.07)	0.09 (0.04)
Hip	0.21 (0.12) f	0.18 (0.07) f,h	0.12 (0.05)	0.11 (0.05) h	0.08 (0.05) h

^a: Statistically significant differences between $K_{hip}$ and $K_{knee}$ at S1 during WRT (p < 0.001); ^b: differences between $K_{ankle}$ and $K_{knee}$ at S1 and S2 (p $\le$ 0.001) during WRT; ^c: differences between S2 and S–2, S2 and S–1, S2 and S0 (p < 0.001) during WRT within $K_{ankle}$; ^d: differences between S1 and S–2, S1 and S–1 (p < 0.001) during WRT, within $K_{hip}$; ^e: differences between $K_{ankle}$ and $K_{knee}$ at S–2 and S–1 (p < 0.001) during RWT; ^f: differences between $K_{hip}$ and $K_{knee}$ at S–2 and S–1 (p < 0.001) during RWT; ^g: differences between S2 and S–2, S2 and S–1 (p < 0.001) during RWT, within $K_{ankle}$; ^h: differences between S–1 and S1, S–1 and S2 (p $\le$ 0.001) during RWT, within $K_{hip}$.

. During WRT, $K_{hip}$ was higher than $K_{knee}$ at S1 (p < 0.001), and $K_{ankle}$ was higher than $K_{knee}$ at S1 and S2 (p $\le$ 0.001). Within $K_{ankle}$, S2 was higher than S–2, S–1 and S0 (p < 0.001); within $K_{hip}$, S1 was higher than S–2 and S–1 (p < 0.001). During RWT, $K_{knee}$ was lower than $K_{ankle}$ (p < 0.001) and $K_{hip}$ (p < 0.001) at both S–2 and S–1. Within $K_{ankle}$, S2 was lower than S–2 and S–1 (p < 0.001); S–1 was higher than S1 and S2 (p $\le$ 0.001) within $K_{hip}$.

3.2. Joint Mechanical Work

WRT $W_{joint}$ mean values are presented in

Table 2. Joint work (J/kg) across WRT steps. Sample mean (SD); n = 10.

Joint Work (J/kg)	Steps
	S–2	S–1	S0	S1	S2
Stance Phase Positive Work
Ankle	0.40 (0.16) a	0.39 (0.13) a	0.55 (0.25)	0.63 (0.29) b	0.61 (0.23) a,b
Knee	0.21 (0.08) a	0.20 (0.08) a	0.37 (0.19)	0.31 (0.15) c	0.28 (0.14) a,c
Hip	0.20 (0.07) d	0.24 (0.08) e	0.09 (0.09) e	0.05 (0.05) b,c,d,e	0.06 (0.03) b,c,d,e
Stance Phase Negative Work
Ankle	−0.10 (0.04) h	−0.11 (0.06) f,i	−0.28 (0.13)	−0.36 (0.12) h,i	−0.36 (0.08) h,i
Knee	−0.30 (0.21)	−0.24 (0.06) f	−0.41 (0.32)	−0.43 (0.18) g	−0.41 (0.21) g
Hip	−0.17 (0.12)	−0.15 (0.08)	−0.22 (0.18)	−0.24 (0.19) g	−0.19 (0.19) g
Swing Phase Positive Work
Ankle j	0.01 (0.00)	0.01 (0.00)	0.01 (0.00)	0.01 (0.00)	0.01 (0.00)
Knee k	0.02 (0.02)	0.02 (0.01)	0.01 (0.01)	0.01 (0.01)	0.03 (0.04)
Hip j,k	0.14 (0.03) l	0.13 (0.06)	0.21 (0.07)	0.23 (0.07) l	0.26 (0.11)
Swing Phase Negative Work
Ankle m	−0.01 (0.03)	<−0.01 (0.00) o	<−0.01 (0.00) o	<−0.01 (0.00) o	−0.03 (0.10)
Knee m,n	−0.23 (0.03) p	−0.24 (0.04) q	−0.31 (0.05) p,q	–0.34 (0.06) p,q	−0.35 (0.06) p,q
Hip n	−0.03 (0.02)	−0.02 (0.01) o	−0.02 (0.01) o	–0.02 (0.01) o	−0.02 (0.01)

Note: $W_{joint}$ < 0.01 indicates a negligible value. ^a: Statistically significant differences between $W_{ankle}^{+}$ and $W_{knee}^{+}$ at S–2, S–1 and S2 (p < 0.001) during stance; ^b: differences between $W_{ankle}^{+}$ and $W_{hip}^{+}$ at S1 and S2 (p < 0.001) during stance; ^c: differences between $W_{knee}^{+}$ and $W_{hip}^{+}$ at S1 and S2 (p $\le$ 0.001) during stance; ^d: differences between S–2 and S1, S–2 and S2 (p < 0.001) during stance within $W_{hip}^{+}$; ^e: differences between S–1 and S0, S–1 and S1, S–1 and S2 (p < 0.001) during stance within $W_{hip}^{+}$; ^f: differences between $W_{ankle}^{-}$ and $W_{knee}^{-}$ at S–1 during stance (p < 0.001); ^g: differences between $W_{knee}^{-}$ and $W_{hip}^{-}$ at S1 and S2 (p $\le$ 0.001) during stance; ^h: differences between S–2 and S1, S–2 and S2 (p < 0.001) during stance within $W_{ankle}^{-}$; ⁱ: differences between S–1 and S1, S–1 and S2 (p < 0.001) during stance within $W_{ankle}^{-}$; ^j: differences between $W_{hip}^{+}$ and $W_{ankle}^{+}$ during swing for all steps (p < 0.001); ^k: differences between $W_{hip}^{+}$ and $W_{knee}^{+}$ during swing for all steps (p < 0.001); ^l: differences between S–2 and S1 during swing within $W_{hip}^{+}$ (p < 0.001); ^m: differences between $W_{knee}^{-}$ and $W_{ankle}^{-}$ during swing for all steps (p < 0.001); ⁿ: differences between $W_{knee}^{-}$ and $W_{hip}^{-}$ during swing for all steps (p < 0.001); ^o: differences between $W_{ankle}^{-}$ and $W_{hip}^{-}$ during swing for all steps between S–1 and S1 (p < 0.001); ^p: differences between S–2 and all steps between S0 and S2 during swing within $W_{knee}^{-}$, (p < 0.001); ^q: differences between S–1 and all steps between S0 and S2 during swing within $W_{knee}^{-}$, (p < 0.001).

. During the WRT trials, stance phase $W_{ankle}^{+}$ was higher than $W_{knee}^{+}$ at S–2, S–1 and S2 (p < 0.001), $W_{ankle}^{+}$ was also higher than $W_{hip}^{+}$ at S1 and S2 (p < 0.001), $W_{knee}^{+}$ was higher than $W_{hip}^{+}$ at S1 and S2 (p $\le$ 0.001). Within $W_{hip}^{+}$ in stance, S–2 was higher than S1 and S2 (p < 0.001), S–1 was higher than S0, S1 and S2 (p < 0.001). Stance phase $W_{knee}^{-}$ was higher than $W_{ankle}^{-}$ at S–1 (p < 0.001), $W_{knee}^{-}$ was also higher than $W_{hip}^{-}$ at S1 and S2 (p $\le$ 0.001). Within $W_{ankle}^{-}$ in stance, S–2 was lower than S1 and S2 (p < 0.001), S–1 was lower than S1 and S2 (p < 0.001). During WRT in the swing phase, $W_{hip}^{+}$ was higher than $W_{ankle}^{+}$ and $W_{knee}^{+}$ at all steps between S–2 and S2 (p < 0.001); within $W_{hip}^{+}$, S–2 was lower than S1 (p < 0.001). Swing phase $W_{knee}^{-}$ was higher than $W_{ankle}^{-}$ and $W_{hip}^{-}$ at all steps (p < 0.001); among steps between S–1 and S1, $W_{hip}^{-}$ was higher than $W_{ankle}^{-}$ (p < 0.001); within $W_{knee}^{-}$, S–2 and S–1 were lower than all steps between S0 and S2, respectively (p < 0.001).

For the RWT trials, $W_{joint}$ mean values are presented in

Table 3. Joint work (J/kg) across RWT steps. Sample mean (SD); n = 10.

Joint Work (J/kg)	Steps
	S–2	S–1	S0	S1	S2
Stance Phase Positive Work
Ankle	0.67 (0.31) a,b,d	0.66 (0.33) a,b	0.50 (0.21) a,b	0.48 (0.34)	0.37 (0.20) d
Knee	0.33 (0.16) a,c	0.32 (0.15) a,c,e	0.21 (0.14) a	0.23 (0.17) e	0.19 (0.11)
Hip	0.06 (0.04) b,c,f	0.07 (0.05) b,c,g	0.16 (0.08) b	0.20 (0.06) f,g	0.18 (0.04) f,g
Stance Phase Negative Work
Ankle	−0.41 (0.19) i	−0.38 (0.18) j	−0.17 (0.12) i,j	−0.12 (0.08) h,i,j	−0.10 (0.05) h,i,j
Knee	−0.41 (0.21)	−0.45 (0.24)	−0.42 (0.22)	−0.28 (0.10) h	−0.26 (0.06) h
Hip	−0.25 (0.22)	−0.26 (0.18)	−0.23 (0.18)	−0.22 (0.15)	−0.18 (0.11)
Swing Phase Positive Work
Ankle l	0.01 (0.00)	0.01 (0.00) m	0.01 (0.00)	0.01 (0.01)	0.01 (0.00)
Knee k	0.01 (0.01)	<0.01 (0.00) m	0.01 (0.01)	0.02 (0.04)	0.01 (0.01)
Hip k,l	0.22 (0.09)	0.22 (0.09)	0.13 (0.04)	0.12 (0.06)	0.12 (0.03)
Swing Phase Negative Work
Ankle n	<−0.01 (0.00)	<−0.01 (0.00) p	<−0.01 (0.00) p	<−0.01 (0.00) p	<−0.01 (0.00) p
Knee n,o	−0.33 (0.09) q	−0.34 (0.08) q,r	−0.23 (0.05) r	−0.21 (0.08)	−0.21 (0.04) q
Hip o	−0.01 (0.01)	−0.02 (0.01) p	−0.02 (0.01) p	−0.02 (0.01) p	−0.02 (0.01) p

Note: $W_{joint}$ < 0.01 indicates a negligible value. ^a: Statistically significant differences between $W_{ankle}^{+}$ and $W_{knee}^{+}$ during stance for all steps between S–2 and S0 (p $\le$ 0.001); ^b: differences between $W_{ankle}^{+}$ and $W_{hip}^{+}$ during stance for all steps between S–2 and S0 (p $\le$ 0.001); ^c: differences between $W_{knee}^{+}$ and $W_{hip}^{+}$ at S–2 and S–1 during stance (p $\le$ 0.001); ^d: differences between S–2 and S2 during stance within $W_{ankle}^{+}$ (p < 0.001); ^e: differences between S–1 and S1 during stance within $W_{knee}^{+}$ (p = 0.001); ^f: differences between S–2 and steps between S1 and S2 during stance within $W_{hip}^{+}$ (p $\le$ 0.001); ^g: differences between S–1 and steps between S1 and S2 during stance within $W_{hip}^{+}$ (p $\le$ 0.001); ^h: differences between $W_{ankle}^{-}$ and $W_{knee}^{-}$ at S1 and S2 (p < 0.001) during stance; ⁱ: differences between S–2 and steps between S0 and S2 during stance within $W_{ankle}^{-}$ (p $\le$ 0.001); ^j: differences between S–1 and steps between S0 and S2 during stance within $W_{ankle}^{-}$ (p $\le$ 0.001); ^k: differences between $W_{hip}^{+}$ and $W_{knee}^{+}$ during swing for all steps except for $W_{knee}^{+}$ at S1 (p < 0.001); ^l: differences between $W_{hip}^{+}$ and $W_{ankle}^{+}$ during swing for all steps (p < 0.001); ^m: differences between $W_{ankle}^{+}$ and $W_{knee}^{+}$ at S–1 during swing (p < 0.001); ⁿ: differences between $W_{knee}^{-}$ and $W_{ankle}^{-}$ for all steps during swing (p < 0.001); ^o: differences between $W_{knee}^{-}$ and $W_{hip}^{-}$ for all steps during swing (p < 0.001); ^p: differences between $W_{ankle}^{-}$ and $W_{hip}^{-}$ for steps between S–1 and S2 during swing (p $\le$ 0.001); ^q: differences between S2 and S–2, S2 and S–1 (p < 0.001) during swing within $W_{knee}^{-}$; ^r: differences between S–1 and S0 during swing within $W_{knee}^{-}$, (p < 0.001).

. Stance phase $W_{ankle}^{+}$ was higher than $W_{knee}^{+}$ and $W_{hip}^{+}$ at all steps between S–2 and S0, respectively (p $\le$ 0.001); $W_{knee}^{+}$ was higher than $W_{hip}^{+}$ at S–2 and S–1 (p $\le$ 0.001). Within $W_{ankle}^{+}$ in stance, S–2 was higher than S2 (p < 0.001); within $W_{knee}^{+}$, S–1 was higher than S1 (p = 0.001); within $W_{hip}^{+}$, S–2 and S–1 were lower than steps between S1 and S2 (p $\le$ 0.001). Stance phase $W_{ankle}^{-}$ was lower than $W_{knee}^{-}$ at S1 and S2 (p < 0.001); within $W_{ankle}^{-}$, S–2 and S–1 were higher than all steps between S0 and S2 (p $\le$ 0.001). During RWT in the swing phase, for positive work, many significant differences were detected. Except for $W_{knee}^{+}$ at S1, $W_{hip}^{+}$ was higher than $W_{ankle}^{+}$ and $W_{knee}^{+}$ among all other steps between S–2 and S2 (p < 0.001); $W_{ankle}^{+}$ was higher than $W_{knee}^{+}$ at S–1 (p < 0.001). Swing phase $W_{knee}^{-}$ was higher than $W_{ankle}^{-}$ and $W_{hip}^{-}$ at all steps, respectively (p < 0.001); $W_{hip}^{-}$ was also higher than $W_{ankle}^{-}$ at all steps between S–1 and S2 (p $\le$ 0.001); within $W_{knee}^{-}$, S2 was lower than S–2 and S–1 (p < 0.001), and S0 was lower than S–1 (p < 0.001).

3.3. Joint Moment Angular Impulse

During WRT in the stance phase (

Table 4. Joint stance phase extensor moment angular impulse (Nm$·$s/kg) and total joint support moment impulse (Nm$·$s/kg) across WRT and RWT steps. Sample mean (SD); n = 10.

Impulse (Nm·s/kg)	Steps
	S–2	S–1	S0	S1	S2
WRT
Ankle a	0.40 (0.12) b	0.36 (0.07) b	0.38 (0.12)	0.41 (0.15)	0.40 (0.11)
Knee	0.16 (0.12) b,d	0.14 (0.08) b,e	0.29 (0.19)	0.30 (0.14) c,d,e	0.26 (0.15) d
Hip a	0.12 (0.03) f	0.12 (0.02) g	0.10 (0.04) h	0.05 (0.03) c,f,g,h	0.07 (0.02) f,g
Total	0.68 (0.23)	0.62 (0.12)	0.77 (0.28)	0.76 (0.28)	0.73 (0.24)
RWT
Ankle i	0.46 (0.22)	0.44 (0.21)	0.41 (0.19)	0.44 (0.24)	0.37 (0.15)
Knee	0.30 (0.15) j,k	0.32 (0.15) j,k	0.25 (0.13)	0.18 (0.10) k	0.16 (0.08)
Hip i	0.06 (0.03) j,l	0.06 (0.04) j,m	0.08 (0.05)	0.12 (0.04) l	0.11 (0.04) l,m
Total	0.82 (0.36)	0.82 (0.36)	0.73 (0.33)	0.75 (0.37)	0.65 (0.24)

^a: Statistically significant differences between $I_{ankle}$ and $I_{hip}$ for all steps during WRT (p $\le$ 0.001); ^b: differences between $I_{ankle}$ and $I_{knee}$ at S–2 and S–1 (p < 0.001) during WRT; ^c: differences between $I_{knee}$ and $I_{hip}$ at S1 during WRT (p < 0.001); ^d: differences between S–2 and S1, S–2 and S2 (p < 0.001) during WRT within $I_{knee}$; ^e: differences between S–1 and S1 during WRT within $I_{knee}$ (p < 0.001); ^f: differences between S–2 and S1, S–2 and S2 during WRT within $I_{hip}$ (p < 0.001); ^g: differences between S–1 and S1, S–1 and S2 during WRT within $I_{hip}$ (p < 0.001); ^h: differences between S0 and S1 during WRT within $I_{hip}$ (p < 0.001); ⁱ: differences between $I_{ankle}$ and $I_{hip}$ for all steps during RWT (p < 0.001); ^j: differences between $I_{knee}$ and $I_{hip}$ at S–2 and S–1 (p < 0.001) during RWT; ^k: differences between S1 and S–2, S1 and S–1 (p < 0.001) during RWT within $I_{knee}$; ^l: differences between S–2 and S1, S–2 and S2 (p < 0.001) during RWT within $I_{hip}$; ^m: differences between S–1 and S2 during RWT within $I_{hip}$ (p < 0.001).

), $I_{ankle}$ was higher than $I_{hip}$ at all steps, respectively (p $\le$ 0.001), $I_{ankle}$ was higher than $I_{knee}$ at S–2 and S–1 (p < 0.001), and $I_{knee}$ was higher than $I_{hip}$ at S1 (p < 0.001); within $I_{knee}$, S–2 was lower than S1 and S2 (p < 0.001), and S–1 was lower than S1 (p < 0.001); S–2 and S–1 were higher than all steps between S1 and S2 (p < 0.001), and S0 was higher than S1 (p < 0.001) within $I_{hip}$. During RWT in the stance phase (Table 4), $I_{ankle}$ was higher than $I_{hip}$ at all steps (p < 0.001), and $I_{knee}$ was higher than $I_{hip}$ at S–2 and S–1 (p < 0.001); within $I_{knee}$, S1 was lower than S–2 and S–1 (p < 0.001); within $I_{hip}$, S–2 was lower than S1 and S2 (p < 0.001), S–1 was lower than S2 (p < 0.001).

3.4. Joint Mechanical Power

During WRT trials, net joint power characteristics in all three joints at S0 were observed to be similar to running gait patterns (Figure 1). Stance phase peak power increased and time to peak power reduced at S0 compared with the previous walking stride. Specifically, peak negative ankle and knee joint power were 123% and 84% higher, respectively, at S0 than at S–1. The time to peak positive ankle and peak negative hip power were 52% and 59% lower, respectively, at S0 than S–1. At S0 for the RWT process, joint power patterns tended to be a combination of both walking and running (Figure 2). Specifically, stance phase peak negative ankle and knee joint power were 94% and 41% lower, respectively, at S0 than at S–1. The peak negative ankle and knee joint power were 79% and 51% higher, respectively, at S0 than at S1.

4. Discussion

This study aimed to investigate lower extremity joint stance phase loading and response patterns and functional roles of lower extremity joints in stance and swing phase during WRT and RWT processes. The hypothesis that $K_{joint}$ increases during WRT and decreases during RWT was partially supported. Specifically, $K_{knee}$ tended to remain unchanged across transition steps, $K_{ankle}$ and $K_{hip}$ tended to increase from S0 to S2 during WRT and decrease from S0 to S2 during RWT (Table 1). This indicates that the transition between walking and running has greater influence on ankle and hip joint dynamic loading and response rather than the knee. A previous study reported higher $K_{ankle}$ and $K_{hip}$ in running compared with walking at the same speed [21], which supports the current gait transition findings. Additionally, $K_{ankle}$ and $K_{hip}$ were both significantly different between steps before and after transition. This indicates that both WRT and RWT were modulated over more than a single transition step. It also supports and expands the previous finding that ankle and hip mechanics contribute not only to the WRT, but also the RWT at the transition step [5].

The hypothesis that $W_{joint}^{+}$, $W_{joint}^{-}$ and $I_{joint}$ increase during WRT and decrease during RWT was also partially supported. Specifically, only $W_{ankle}^{+}$ and $W_{knee}^{+}$ increased during WRT and decreased during RWT, which supported the hypothesis. During the WRT stance phase, $W_{ankle}^{+}$ and $W_{knee}^{+}$ were 34% and 60% higher, respectively, at S0 compared with S–1, while for $W_{hip}^{+},$ there was a significant decrease at S0 compared with S–1 (Table 2). This indicates $W_{joint}^{+}$ did not gradually change among the five steps during WRT, but rather changed instantaneously at the transition step and continued to modulate in the following steps. Furthermore, $W_{ankle}^{+}$ and $W_{knee}^{+}$ were higher compared with $W_{hip}^{+}$ within steps between S1 and S2, indicating that when switching from walking to running gait, the ankle and knee joint played more dominant roles in stance phase energy generation at S0 and the following steps (S1, S2). This observation agrees with previous reports [24]. Decreasing $W_{hip}^{+}$ while increasing $W_{ankle}^{+}$ within the steps from S0 to S2 compared with previous steps before the transition indicated that during the WRT process, stance phase energy generation tended to transfer from proximal to distal. The redistribution of $W_{joint}^{+}$ among lower extremity joints at S0 was related to the mechanical load and efficiency trigger mechanisms which help modulate WRT. It would be favorable for hip muscles to reduce hip peak power and work at S0 during WRT [5,11,36] and it would be more efficient for ankle plantar flexor power generation between S0 and S2 during WRT [5]. All these factors were related to switching gait patterns from walking to running and should contribute to improving gait mechanical efficiency during the WRT [5,9].

During the RWT stance phase, the ankle and knee played more dominant roles in energy generation within the pre-transition steps (S–2, S–1) (Table 3). The $W_{ankle}^{+}$ and $W_{knee}^{+}$ at S0 were 28% and 42% lower than at S–1, respectively, while $W_{hip}^{+}$ at S0 was 78% higher than at S–1 (Table 3). This indicates that during the RWT stance phase, energy generation tended to transfer from distal to proximal, and that $W_{ankle}^{+}$ and $W_{knee}^{+}$ decreased while $W_{hip}^{+}$ increased during and after transition. This may be due to a reduced requirement of ankle plantar flexor power and force generation efficiency in walking compared with running at S0 during RWT [5,9]. A previous study investigated walking and running across different speeds, reporting that the percentage and amount of $W_{joint}^{+}$ contributed to the total lower extremity positive work at fast walking speeds (1.8 and 2.0 m/s) were around 45% for $W_{ankle}^{+}$ and 24% for $W_{hip}^{+}$ [21]; at slow running speeds (1.8 and 2.2 m/s) $W_{ankle}^{+}$ contributed to 65% while $W_{hip}^{+}$ accounted for only 6% of total work, which also supports the current finding that $W_{joint}^{+}$ transfers between lower extremity joints when locomotion tasks change between walking and running, even within the similar locomotion speed range [21]. The non-linear change in $W_{joint}^{+}$ generation at S0 to S2 compared with the pre–transition steps (S–2, S–1) also implied that RWT occurred at the transition step and then continued to modulate during the following steps.

The energy generation transfer phenomenon among lower extremity joints at S0 during both WRT and RWT may be attributed to lower extremity distal joints having higher energy generation efficiency or less required effort when running at speeds above PTS [5,9], and vice versa for the proximal joints when walking at speeds below PTS. Stance phase energy generation transfer was sensitive at the transition step (S0) for both WRT and RWT. The redistribution of $W_{joint}^{+}$ and the transfer mechanism can be attributed to the combined choice of gait transition trigger mechanisms: optimization of mechanical work efficiency and minimization of musculoskeletal system effort at the transition step [5,9], both of which help to modulate gait transitions. In the present study, the knee joint played an important function during both WRT and RWT stance phase energy absorption. In the swing phase for both transition types, the hip and knee joints played dominant roles in energy generation and absorption.

During both WRT and RWT, $I_{ankle}$ accounted for more than 57% of $I_{total}$ at S–2 and S–1 during WRT, as well as at S1 and S2 during RWT (Table 4). This observation suggests that walking stance phase relies more on ankle plantar flexor moment for providing total body support and forward propulsion during both transitions. Additionally, $I_{ankle}$ was determined by ankle plantar flexor muscle moment and stance time. Stance time was changeable due to the continuous changing speed protocol in this study, while $I_{ankle}$ consistently contributed to nearly 50% of $I_{total}$ among all steps. This indicates that ankle plantar flexor muscle force and moment compensate for the changeable stance time length, and they help to maintain a relatively consistent $I_{ankle}$ output to support and move the body forward efficiently during the transitions between different locomotion tasks. During WRT, $I_{knee}$ at S0 was 70% higher than at S–1, indicating that additional knee joint extensor muscle work is needed in terminal stance phase at S0 to drive the body transitioning from walking to running gait, especially in preparation for the first flight phase immediately after the stance phase of S0.

Since ankle joint kinetics contribute to and help modulate both WRT and RWT, we further examined the stance phase sagittal plane ankle angle–moment relationship during both transitions (Figure 3). The ankle angle–moment relationship displayed a clockwise hysteresis curve [22,27,37,38]. The ascending phase can be regarded as a dynamic loading period and the descending phase considered as an energy generation period [37].

During WRT trials, S–2 was similar to a standard walking gait pattern; however, S1 and S2 exhibited a typical running gait pattern (Figure 3) [21,22]. No statistically significant difference (p > 0.05) between WRT peak ankle angle and moment at S–2, S–1 with actual walking at 1.8 m/s and 2.0 m/s, as well as the comparisons between S1, S2 during WRT with actual running at 2.2 m/s from previous study [22] confirm the above assumption. At S–1, the ankle started to plantar flex in the mid-stance phase and the early plantar flexion movement resulted in peak ankle moment decreasing from 1.9 Nm/kg at S–2 to 1.6 Nm/kg at S–1 in the terminal stance phase. This indicates that the ankle joint started to prepare for gait transition at S–1 due to the constantly accelerated speed. At S0, the curve was similar to a typical running gait pattern. Specifically, after initial contact, the ankle dorsiflexed to around –13°. Compared with S1 and S2, a relatively wide-open area between the ascending and descending phase at S0 indicated additional energy generation was needed at S0 to modulate and prepare for the following running strides during WRT. A significant difference (p = 0.002) between WRT peak ankle angle at S0 and actual walking at 2.0 m/s, as well as no significant difference between S0 at WRT and actual running at 1.8 m/s and 2.2 m/s (p > 0.05) from previous study [22], also validate the finding that S0 at WRT is close to a running pattern.

During the RWT process, S–2 and S–1 exhibited a typical running kinematic and kinetic pattern, while S1 and S2 were similar to a walking pattern (Figure 3). No statistically significant difference (p > 0.05) between S–2, S–1 and actual running at 2.2 m/s, as well as between S1, S2 and actual walking at 1.8 m/s from previous [22] study further confirm this observation. A four-phase pattern was observed at S0. After initial contact, the ankle dorsiflexed to a smaller angle, around –5° in the ascending phase, then plantar flexed with a slight increase in the ankle moment. This may be attributed to the constantly decelerating speed, along with an increase in stance time. Compared with a typical walking condition, the energy generation period during S0 was observed to be earlier. The ankle kinematic and kinetic pattern at S0 were both closer to the walking gait pattern. The absence of statistically significant difference (p > 0.05) of peak ankle angle and moment between S0 at RWT and actual walking at 2.0 m/s, as well as significant difference (p < 0.05) between S0 and actual running at 1.8 m/s or 2.2 m/s from previous study [22], also validate it.

A unique ankle angle–moment curve pattern at S0 was observed in both WRT and RWT. During WRT, it was similar to a running gait pattern; however, it appeared to be a combination of both walking and running conditions during RWT, with the kinematic and kinetic values closer to the walking gait pattern. This observation reveals that during RWT, a longer time was needed to adjust and modulate the motor response compared with the WRT. For both WRT and RWT, we recorded the treadmill instantaneous speed at S0 as the PTS. During WRT, the calculated PTS was 2.06 $\pm$ 0.09 m/s, and transition time was about 2.6 s (from 1.80–2.06 m/s). For the RWT, the calculated PTS was 1.97 $\pm$ 0.10 m/s, and transition time was about 4.3 s (from 2.40–1.97 m/s). These results confirm that subjects needed more time to modulate the RWT process compared with the WRT.

One limitation of this study is the singularity of acceleration and deceleration used for transitions. Different acceleration and deceleration magnitudes would likely affect the PTS and possibly the gait patterns of the transition step [7]. Additionally, this study has a relatively small sample size. Individual differences such as age and health status could lead to varying gait transition characteristics. Moreover, this study was conducted on a treadmill, walking on which may differ from actual walking and running on the ground. These factors may potentially affect the interpretation of the results and limit the generalizability of our findings.

5. Conclusions

In conclusion, the reorganization processes of WRT and RWT were modulated before and after transitions, and gait transition is not a single-step event. Both WRT and RWT exhibited unique ankle and hip joint stiffness characteristics at the transition step and then continued to modulate those patterns in subsequent steps. Stance phase energy generation tended to transfer from proximal to distal joints during WRT, and vice versa during the RWT process. Redistribution of joint mechanical work generation suggests that mechanical efficiency and load triggers were the key factors modulating the gait transition. Ankle kinematic and kinetic patterns at transition step were similar to the target locomotion task format in both WRT and RWT. With the same continuously accelerated or decelerated speed, longer times were required to modulate and finish RWT than WRT. Overall, gait transitions appeared to distinctively affect lower extremity joint kinetic patterns.