Definition of a Global Coordinate System in the Foot for the Surgical Planning of Forefoot Corrections
Abstract
:1. Introduction
2. Materials and Methods
2.1. Requirements to Define a Global Coordinate System
- Be well defined. A well-defined coordinate system includes the definition of two axes and the position of the origin;
- Be robust. A robust coordinate system constructs the coordinate system consistently using the same definition, regardless of anatomical variations amongst patients (i.e., accessory ossicles);
- Be highly repeatable. A highly repeatable coordinate system implies the construction of exactly the same coordinate system within an individual foot if the protocol is repeated. This will enable the same foot orientation in the preoperative planning and independent analysis, regardless of the operator;
- Be clinically relevant with recognizable anatomical planes. This is necessary for the clinical interpretation of the deformity. When the virtual AP and lateral views of the coordinate system correspond with the corresponding radiographic images, a coordinate system is clinically relevant and has recognizable anatomical planes;
- Be compatible with CT scans of the foot. This will make it possible to construct the coordinate system regardless of the scanned section of the tibia and fibula;
- Not be sensitive to the ankle joint angle. This will enable the forefoot to be positioned clinically relevantly in the coordinate system, regardless of the ankle joint angle;
- Not include the shape and orientation of the bones in the forefoot by fitting an object since these bones might be deformed.
2.2. Study Design and Subjects
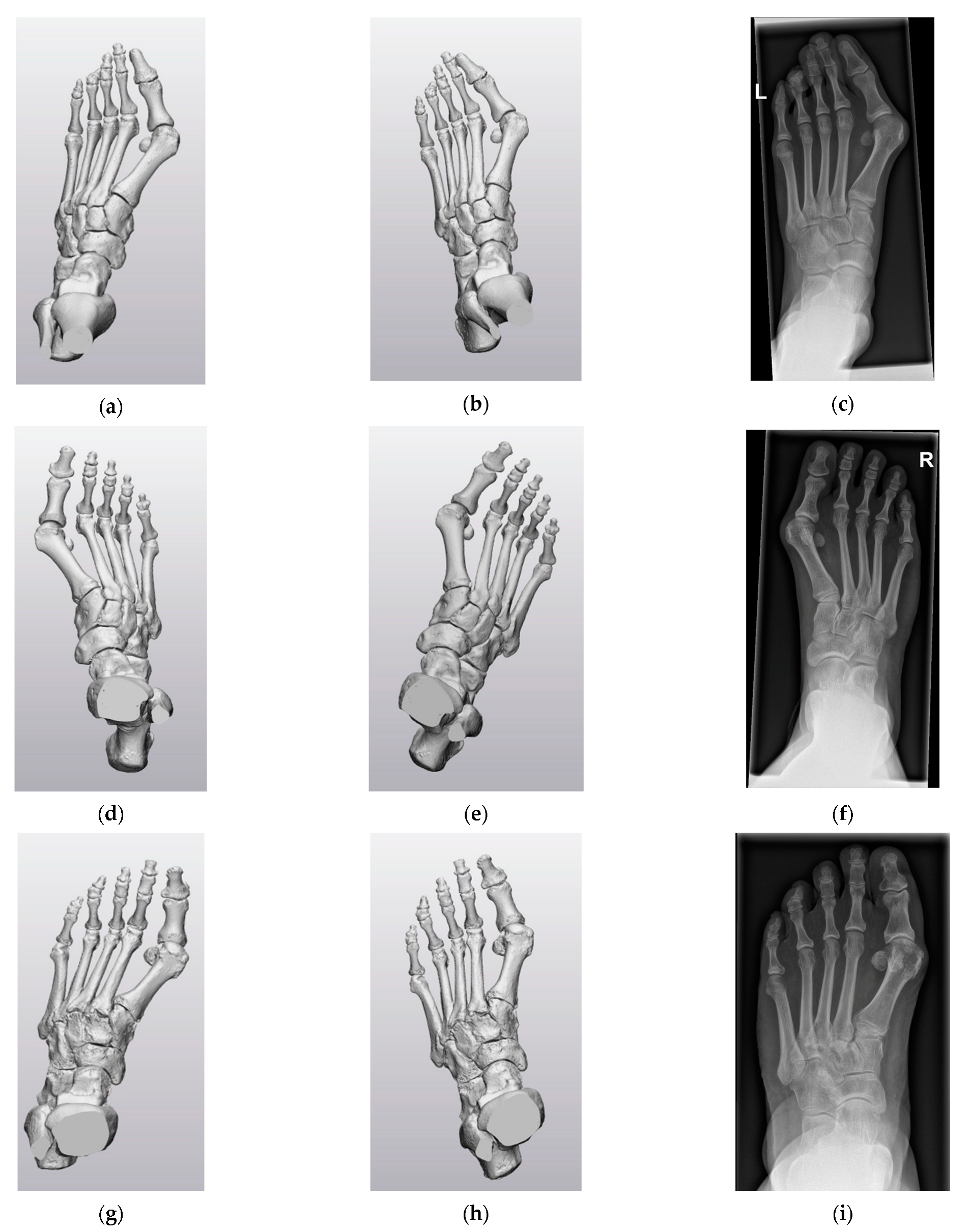
2.3. Data Acquisition
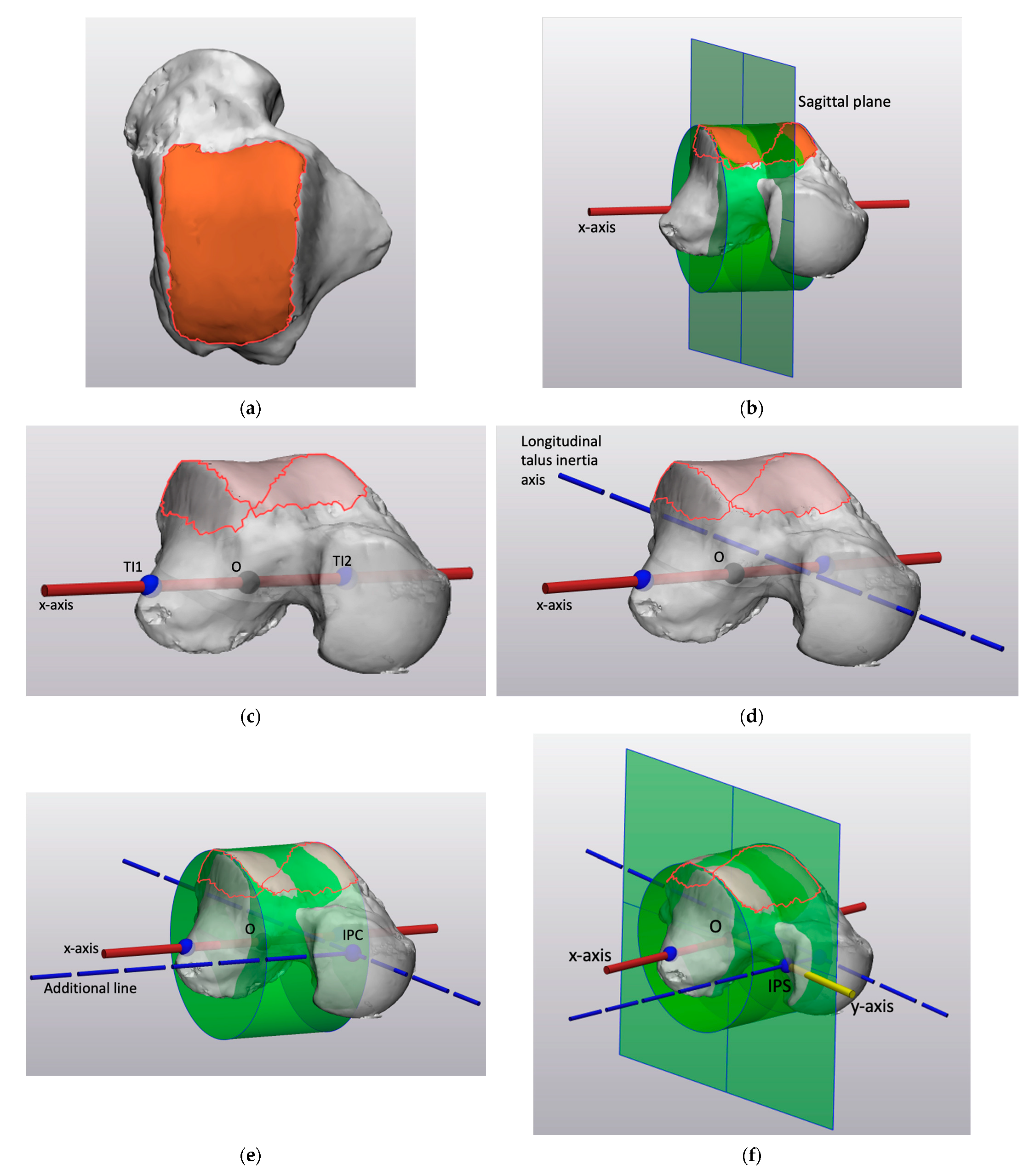
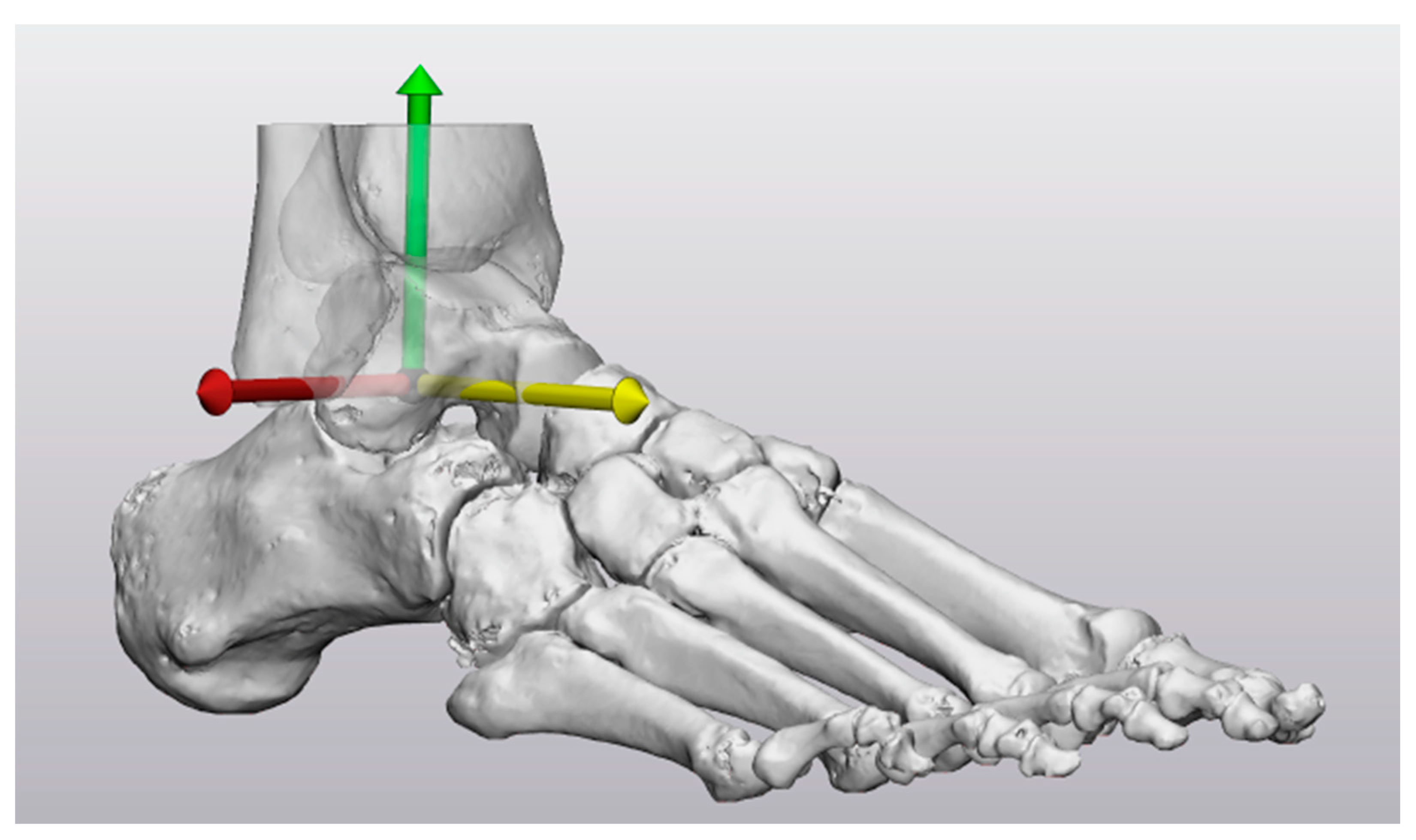
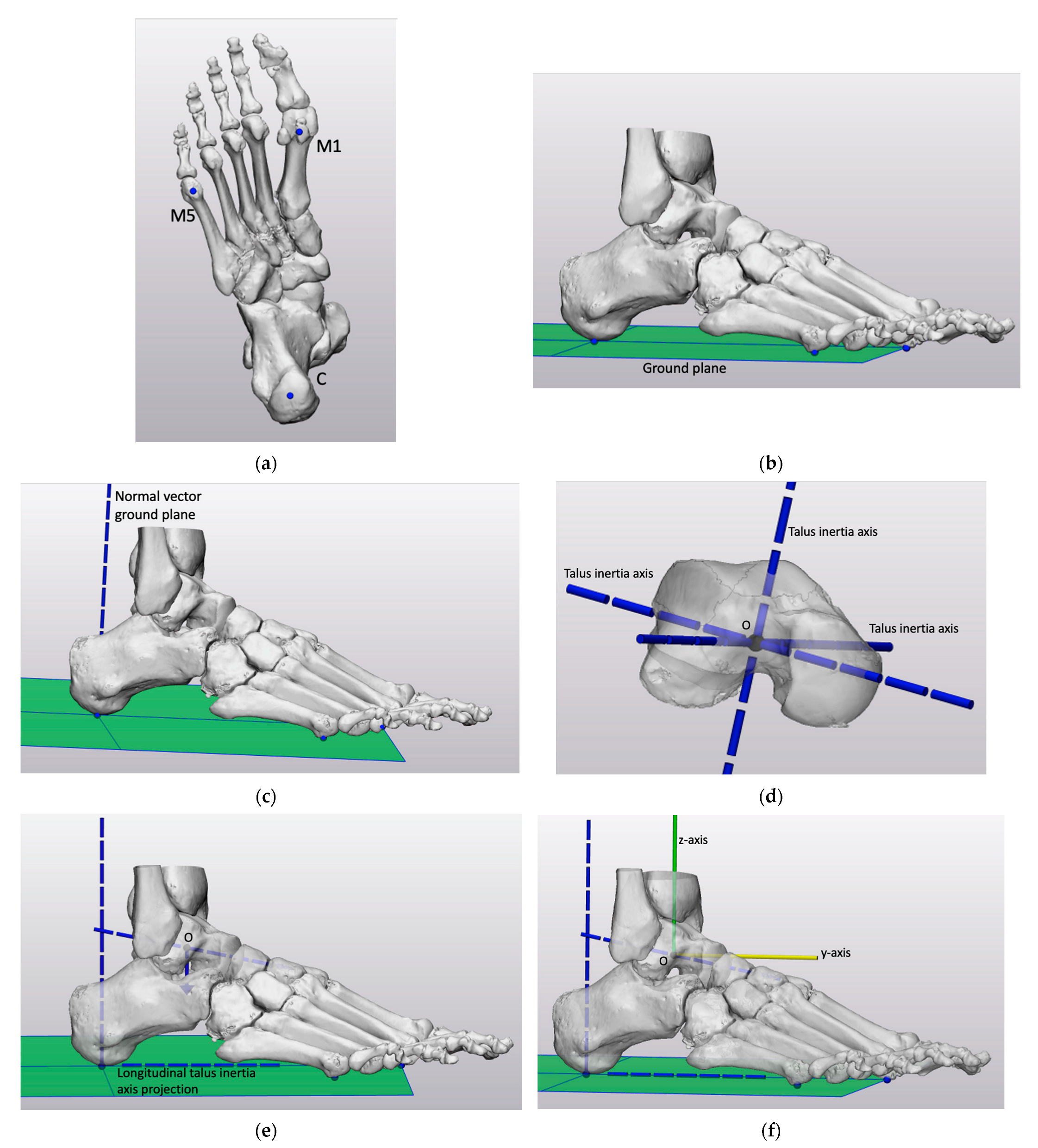
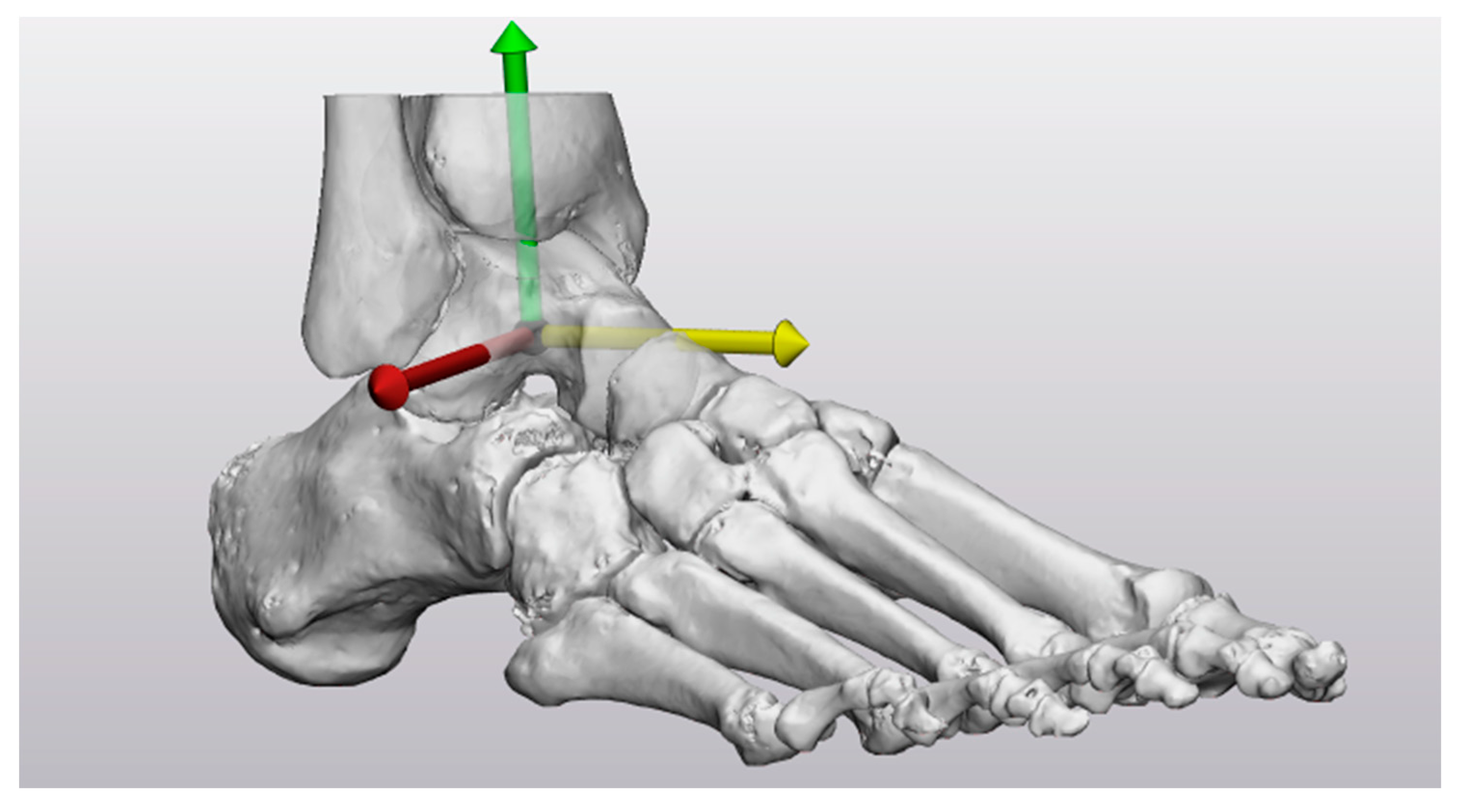
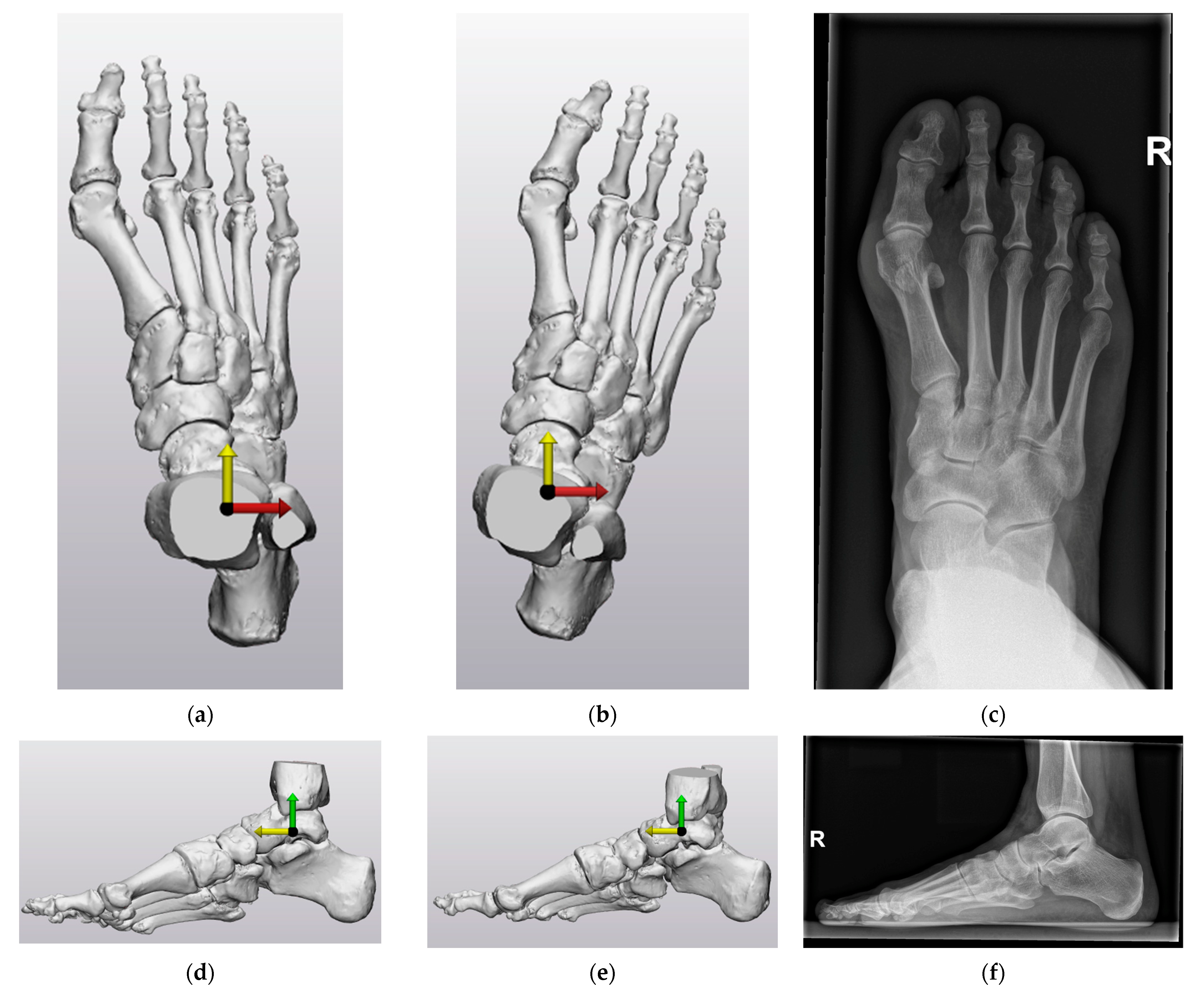
2.4. Coordinate System Definitions
2.5. Coordinate System Evaluation
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Zelen, C.M. Advances in Forefoot Surgery. Clin. Podiatr. Med. Surg. 2013, 30, 13–14. [Google Scholar] [CrossRef] [PubMed]
- Femino, J.E.; Mueller, K. Complications of Lesser Toe Surgery. Clin. Orthop. Relat. Res. 2001, 391, 72–88. [Google Scholar] [CrossRef] [PubMed]
- Sammarco, G.J.; Idusuyi, O.B. Complications After Surgery of the Hallux. Clin. Orthop. Relat. Res. 2001, 391, 59–71. [Google Scholar] [CrossRef] [PubMed]
- Green, C.; Fitzpatrick, C.; FitzPatrick, D.; Stephens, M.; Quinlan, W.; Flavin, R. Definition of Coordinate System for Three-Dimensional Data Analysis in the Foot and Ankle. Foot Ankle Int. 2011, 32, 193–199. [Google Scholar] [CrossRef]
- Kuhn, J.; Alvi, F. Hallux Valgus, StatPearls. Treasure Island (FL). Available online: https://www.ncbi.nlm.nih.gov/books/NBK553092/ (accessed on 30 June 2023).
- Gutekunst, D.J.; Liu, L.; Ju, T.; Prior, F.W.; Sinacore, D.R. Reliability of clinically relevant 3D foot bone angles from quantitative computed tomography. J. Foot Ankle Res. 2013, 6, 38. [Google Scholar] [CrossRef]
- Welck, M.J.; Al-Khudairi, N. Imaging of Hallux Valgus How to Approach the Deformity. Foot Ankle Clin. 2018, 23, 183–192. [Google Scholar] [CrossRef]
- Schweizer, A.; Fürnstahl, P.; Harders, M.; Székely, G.; Nagy, L. Complex Radius Shaft Malunion: Osteotomy with Computer-Assisted Planning. Hand 2010, 5, 171–178. [Google Scholar] [CrossRef]
- Maestro, M.; Besse, J.-L.; Ragusa, M.; Berthonnaud, E. Forefoot morphotype study and planning method for forefoot osteotomy. Foot Ankle Clin. 2003, 8, 695–710. [Google Scholar] [CrossRef]
- Conconi, M.; Pompili, A.; Sancisi, N.; Leardini, A.; Durante, S.; Belvedere, C. New anatomical reference systems for the bones of the foot and ankle complex: Definitions and exploitation on clinical conditions. J. Foot Ankle Res. 2021, 14, 66. [Google Scholar] [CrossRef]
- Ozturk, A.M.; Suer, O.; Coban, I.; Ozer, M.A.; Govsa, F. Three-Dimensional Printed Anatomical Models Help in Correcting Foot Alignment in Hallux Valgus Deformities. Indian J. Orthop. 2020, 54, 199–209. [Google Scholar] [CrossRef]
- Wu, G.; Siegler, S.; Allard, P.; Kirtley, C.; Leardini, A.; Rosenbaum, D.; Whittle, M.; D’Lima, D.D.; Cristofolini, L.; Witte, H.; et al. ISB recommendation on definitions of joint coordinate system of various joints for the reporting of human joint motion—Part I: Ankle, hip, and spine. J. Biomech. 2002, 35, 543–548. [Google Scholar] [CrossRef] [PubMed]
- Cappozzo, A.; Catani, F.; Della Croce, U.; Leardini, A. Position and orientation in space of bones during movement: Anatomical frame definition and determination. Clin. Biomech. 1995, 10, 171–178. [Google Scholar] [CrossRef] [PubMed]
- Geng, X.; Wang, C.; Ma, X.; Wang, X.; Huang, J.; Zhang, C.; Xu, J.; Yang, J. Mobility of the first metatarsal-cuneiform joint in patients with and without hallux valgus: In vivo three-dimensional analysis using computerized tomography scan. J. Orthop. Surg. Res. 2015, 10, 140. [Google Scholar] [CrossRef] [PubMed]
- Ortolani, M.; Leardini, A.; Pavani, C.; Scicolone, S.; Girolami, M.; Bevoni, R.; Lullini, G.; Durante, S.; Berti, L.; Belvedere, C. Angular and linear measurements of adult flexible flatfoot via weight-bearing CT scans and 3D bone reconstruction tools. Sci. Rep. 2021, 11, 16139. [Google Scholar] [CrossRef]
- Yoshioka, N.; Ikoma, K.; Kido, M.; Imai, K.; Maki, M.; Arai, Y.; Fujiwara, H.; Tokunaga, D.; Inoue, N.; Kubo, T. Weight-bearing three-dimensional computed tomography analysis of the forefoot in patients with flatfoot deformity. J. Orthop. Sci. 2016, 21, 154–158. [Google Scholar] [CrossRef]
- Modenese, L.; Renault, J.-B. Automatic generation of personalised skeletal models of the lower limb from three-dimensional bone geometries. J. Biomech. 2021, 116, 110186. [Google Scholar] [CrossRef]
- Brown, K.M.; Bursey, D.E.; Arneson, L.J.; Andrews, C.A.; Ludewig, P.M.; Glasoe, W.M. Consideration of digitization precision when building local coordinate axes for a foot model. J. Biomech. 2009, 42, 1263–1269. [Google Scholar] [CrossRef]
- Moerenhout, B.A.; Gelaude, F.; Swennen, G.R.; Casselman, J.W.; Van Der Sloten, J.; Mommaerts, M.Y. Accuracy and repeatability of cone-beam computed tomography (CBCT) measurements used in the determination of facial indices in the laboratory setup. J. Cranio-Maxillofac. Surg. 2009, 37, 18–23. [Google Scholar] [CrossRef]
- Broeck, J.V.D.; Vereecke, E.; Wirix-Speetjens, R.; Sloten, J.V. Segmentation accuracy of long bones. Med. Eng. Phys. 2014, 36, 949–953. [Google Scholar] [CrossRef]
- Lo Giudice, A.; Ronsivalle, V.; Grippaudo, C.; Lucchese, A.; Muraglie, S.; Lagravère, M.O.; Isola, G. One Step before 3D Printing—Evaluation of Imaging Software Accuracy for 3-Dimensional Analysis of the Mandible: A Comparative Study Using a Surface-to-Surface Matching Technique. Materials 2020, 13, 2798. [Google Scholar] [CrossRef]
- Mandolini, M.; Brunzini, A.; Facco, G.; Mazzoli, A.; Forcellese, A.; Gigante, A. Comparison of Three 3D Segmentation Software Tools for Hip Surgical Planning. Sensors 2022, 22, 5242. [Google Scholar] [CrossRef] [PubMed]
- Bertolini, M.; Luraghi, G.; Belicchi, I.; Migliavacca, F.; Colombo, G. Evaluation of segmentation accuracy and its impact on patient-specific CFD analysis. Int. J. Interact. Des. Manuf. 2022, 16, 545–556. [Google Scholar] [CrossRef]
- Abdullah, J.Y.; Abdullah, A.M.; Hadi, H.; Husein, A.; Rajion, Z.A. Comparison of STL skull models produced using open-source software versus commercial software. Rapid Prototyp. J. 2019, 25, 1585–1591. [Google Scholar] [CrossRef]
- van der Woude, P.; Keizer, S.B.; Wever-Korevaar, M.; Thomassen, B.J. Intra- and Interobserver Agreement in Hallux Valgus Angle Measurements on Weightbearing and Non-Weightbearing Radiographs. J. Foot Ankle Surg. 2019, 58, 706–712. [Google Scholar] [CrossRef]
- Fuhrmann, R.A.; Layher, F.; Wetzel, W.D. Radiographic Changes in Forefoot Geometry with Weightbearing. Foot Ankle Int. 2003, 24, 326–331. [Google Scholar] [CrossRef]
- Tanaka, Y.; Takakura, Y.; Takaoka, T.; Akiyama, K.; Fujii, T.; Tamai, S. Radiographic Analysis of Hallux Valgus in Women on Weightbearing and Nonweightbearing. Clin. Orthop. Relat. Res. 1997, 336, 186–194. [Google Scholar] [CrossRef]
- Boszczyk, A.; Kwapisz, S.; Kiciński, M.; Kordasiewicz, B.; Liszka, H. Non-weightbearing compared with weightbearing x-rays in hallux valgus decision-making. Skelet. Radiol. 2020, 49, 1441–1447. [Google Scholar] [CrossRef]
- Godoy-Santos, A.L.; Bernasconi, A.; Bordalo-Rodrigues, M.; Lintz, F.; Lôbo, C.F.T.; Netto, C.d.C. Weight-bearing cone-beam computed tomography in the foot and ankle specialty: Where we are and where we are going—An update. Radiol. Bras. 2021, 54, 177–184. [Google Scholar] [CrossRef]
- Lintz, F.; Beaudet, P.; Richardi, G.; Brilhault, J. Weight-bearing CT in foot and ankle pathology. Orthop. Traumatol. Surg. Res. 2021, 107, 102772. [Google Scholar] [CrossRef]
- Maken, P.; Gupta, A. 2D-to-3D: A Review for Computational 3D Image Reconstruction from X-ray Images. Arch. Comput. Methods Eng. 2023, 30, 85–114. [Google Scholar] [CrossRef]
- Rohan, P.-Y.; Perrier, A.; Ramanoudjame, M.; Hausselle, J.; Lelièvre, H.; Seringe, R.; Skalli, W.; Wicart, P. Three-Dimensional Reconstruction of Foot in the Weightbearing Position From Biplanar Radiographs: Evaluation of Accuracy and Reliability. J. Foot Ankle Surg. 2018, 57, 931–937. [Google Scholar] [CrossRef] [PubMed]


| Study | Limitations |
|---|---|
| Cappozo et al. [13] | Operator-dependent accuracy and repeatability |
| Green et al. [4] | Dependent on the scanned section of the fibula |
| Geng et al. [14] | Origin not explicitly defined |
| Ortolani et al. [15] | Origin not explicitly defined |
| Yoshioka et al. [16] | The ankle joint angle determines the location of the forefoot in the global coordinate system |
| Modenese et al. [17] | No definition of how axes intersect the origin |
| Helical Region of Interest | Just above the tibiotalar joint through to the carpal–metacarpal joints, dependent on the region of interest |
| Collimation | Slice thickness: 1.25 mm or smaller Slice increment: 0.625 mm (50% overlap) |
| kVp | 120 |
| mAs | As given by the automatic system |
| Pitch | Use 1 or smaller |
| Field of View (FOV) | Fit the whole foot |
| Matrix | Use a 512 × 512 matrix |
| Kernel/Algorithm | Moderate/soft tissue |
| Absolute Angle of Rotation | Patient 1 | Patient 2 | Patient 3 | Patient 4 | Patient 5 | Patient 6 | Mean (SD) |
|---|---|---|---|---|---|---|---|
| CS1 | |||||||
| TP1–TP2 | 1.66° | 0.48° | 0.86° | 1.48° | 2.12° | 1.75° | 1.39° (0.61°) |
| TP1–OS | 2.10° | 1.30° | 0.92° | 1.35° | 4.43° | 5.86° | 2.66° (2.01°) |
| CS2 | |||||||
| TP1–TP2 | 0° | 0° | 0° | 0° | 0° | 0° | 0° (0°) |
| TP1–OS | 0° | 0° | 0° | 0° | 0° | 0° | 0° (0°) |
| Axis with Angle Magnitude | Patient 1 | Patient 2 | Patient 3 | Patient 4 | Patient 5 | Patient 6 | |
|---|---|---|---|---|---|---|---|
| CS1 | |||||||
| x-axis | 0.28° | 0.10° | 0.40° | 1.26° | −0.56° | −0.12° | |
| TP1–TP2 | y-axis | −0.34° | −0.44° | −0.42° | 0.69° | −2.00° | −1.59° |
| z-axis | 1.60° | −0.16° | 0.63° | −0.36° | −0.46° | 0.72° | |
| x-axis | 0.50° | −1.1° | −0.40° | 0.82° | 0.36° | 0.88° | |
| TP1–OS | y-axis | −2.00° | −0.08° | −0.23° | 0.75° | −4.03° | −5.69° |
| z-axis | 0.38° | −0.72° | −0.79° | 0.76° | −1.81° | 1.12° | |
| CS2 | |||||||
| x-axis | 0° | 0° | 0° | 0° | 0° | 0° | |
| TP1–TP2 | y-axis | 0° | 0° | 0° | 0° | 0° | 0° |
| z-axis | 0° | 0° | 0° | 0° | 0° | 0° | |
| x-axis | 0° | 0° | 0° | 0° | 0° | 0° | |
| TP1–OS | y-axis | 0° | 0° | 0° | 0° | 0° | 0° |
| z-axis | 0° | 0° | 0° | 0° | 0° | 0° | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krakers, S.; Peters, A.; Homan, S.; olde Heuvel, J.; Tuijthof, G. Definition of a Global Coordinate System in the Foot for the Surgical Planning of Forefoot Corrections. Biomechanics 2023, 3, 523-538. https://doi.org/10.3390/biomechanics3040042
Krakers S, Peters A, Homan S, olde Heuvel J, Tuijthof G. Definition of a Global Coordinate System in the Foot for the Surgical Planning of Forefoot Corrections. Biomechanics. 2023; 3(4):523-538. https://doi.org/10.3390/biomechanics3040042
Chicago/Turabian StyleKrakers, Sanne, Anil Peters, Sybrand Homan, Judith olde Heuvel, and Gabriëlle Tuijthof. 2023. "Definition of a Global Coordinate System in the Foot for the Surgical Planning of Forefoot Corrections" Biomechanics 3, no. 4: 523-538. https://doi.org/10.3390/biomechanics3040042
APA StyleKrakers, S., Peters, A., Homan, S., olde Heuvel, J., & Tuijthof, G. (2023). Definition of a Global Coordinate System in the Foot for the Surgical Planning of Forefoot Corrections. Biomechanics, 3(4), 523-538. https://doi.org/10.3390/biomechanics3040042








