Relationship between Swimming Velocity and Trunk Twist Motion in Short-Distance Crawl Swimming
Abstract
1. Introduction
2. Materials and Methods
2.1. Analysis
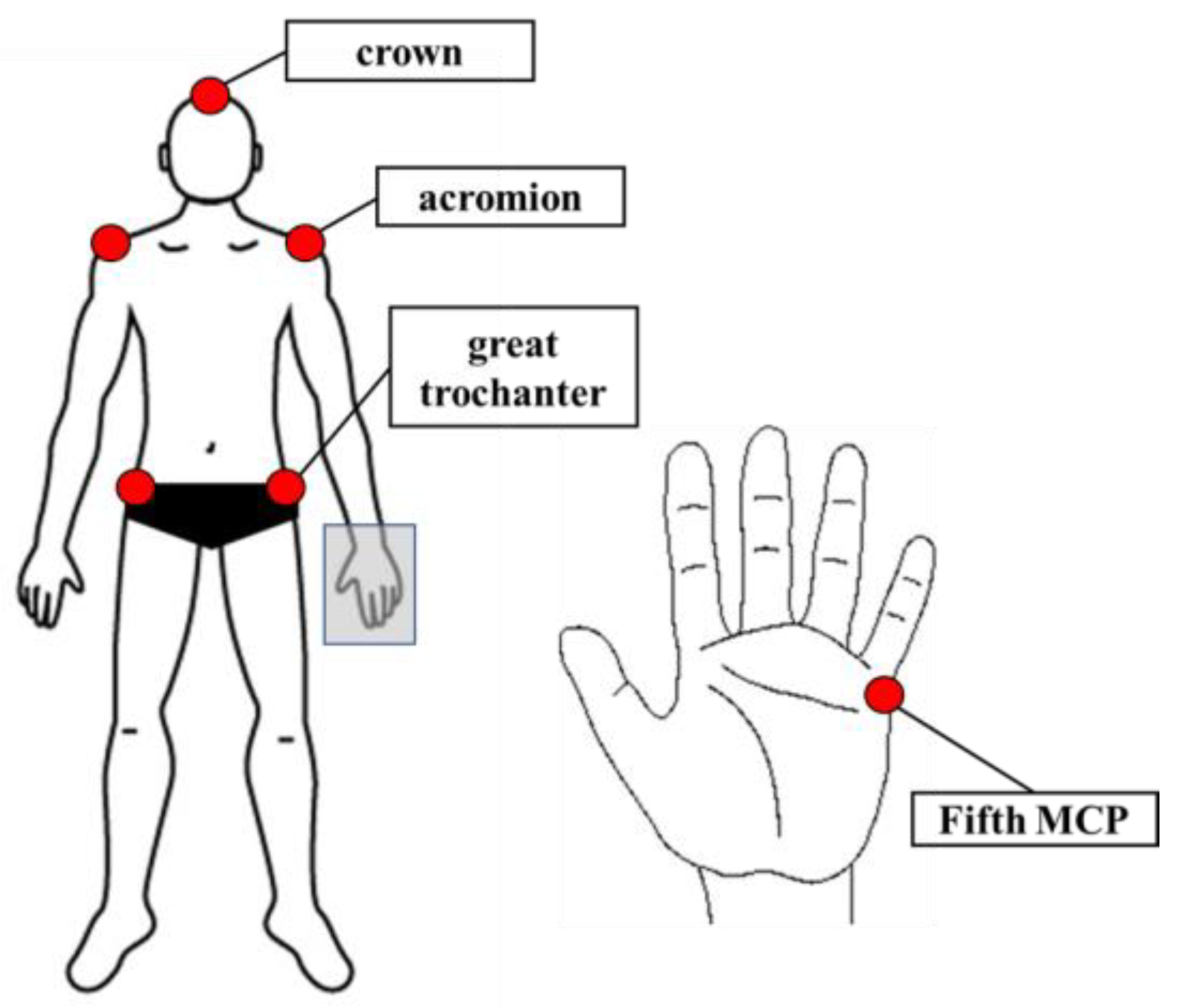
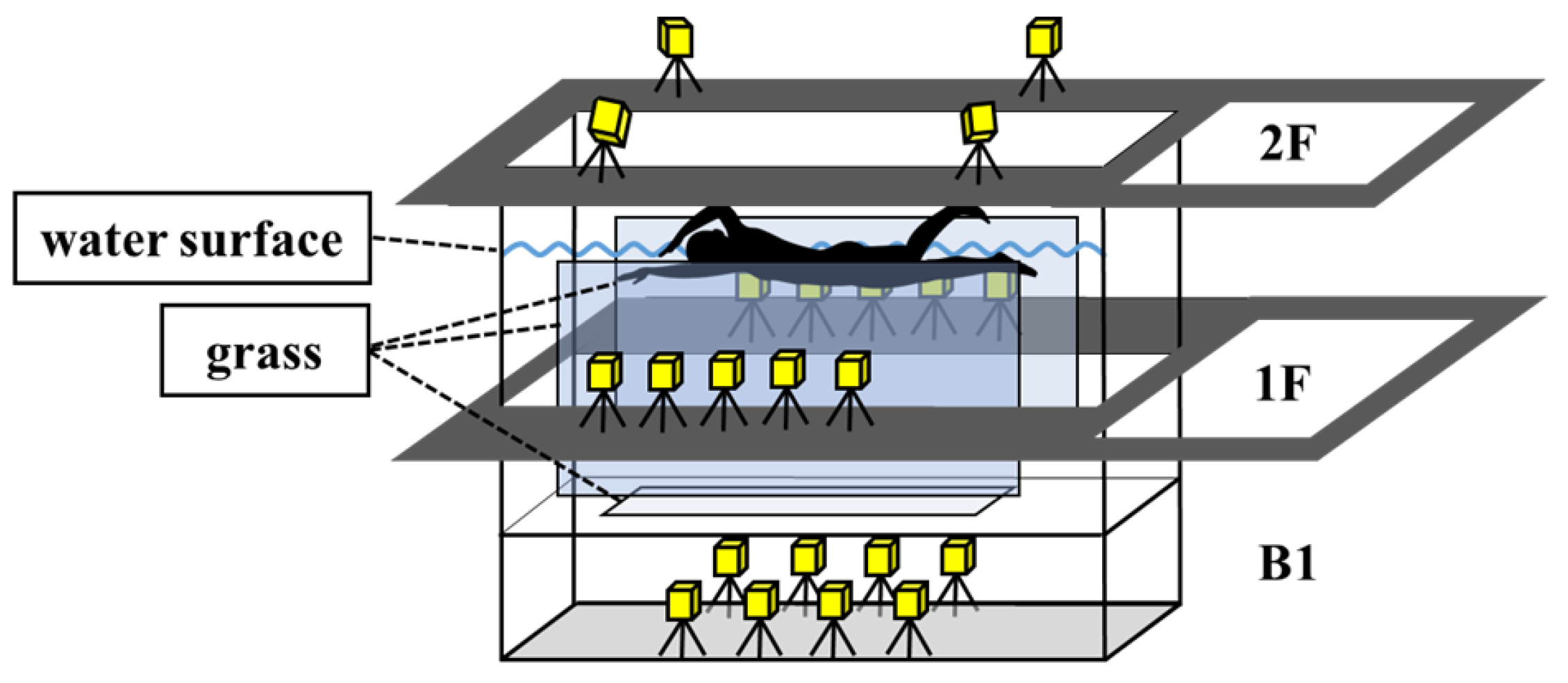
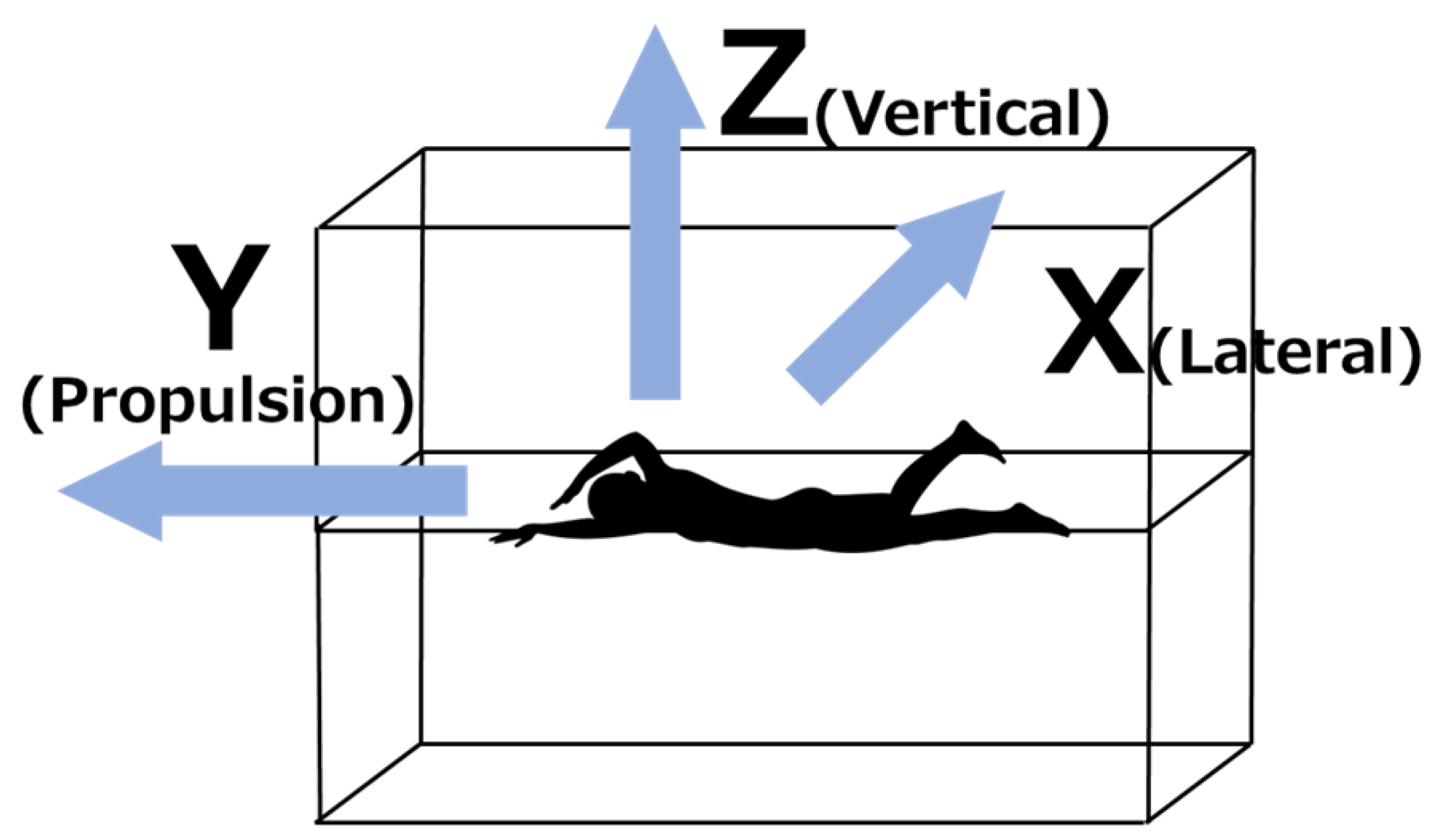
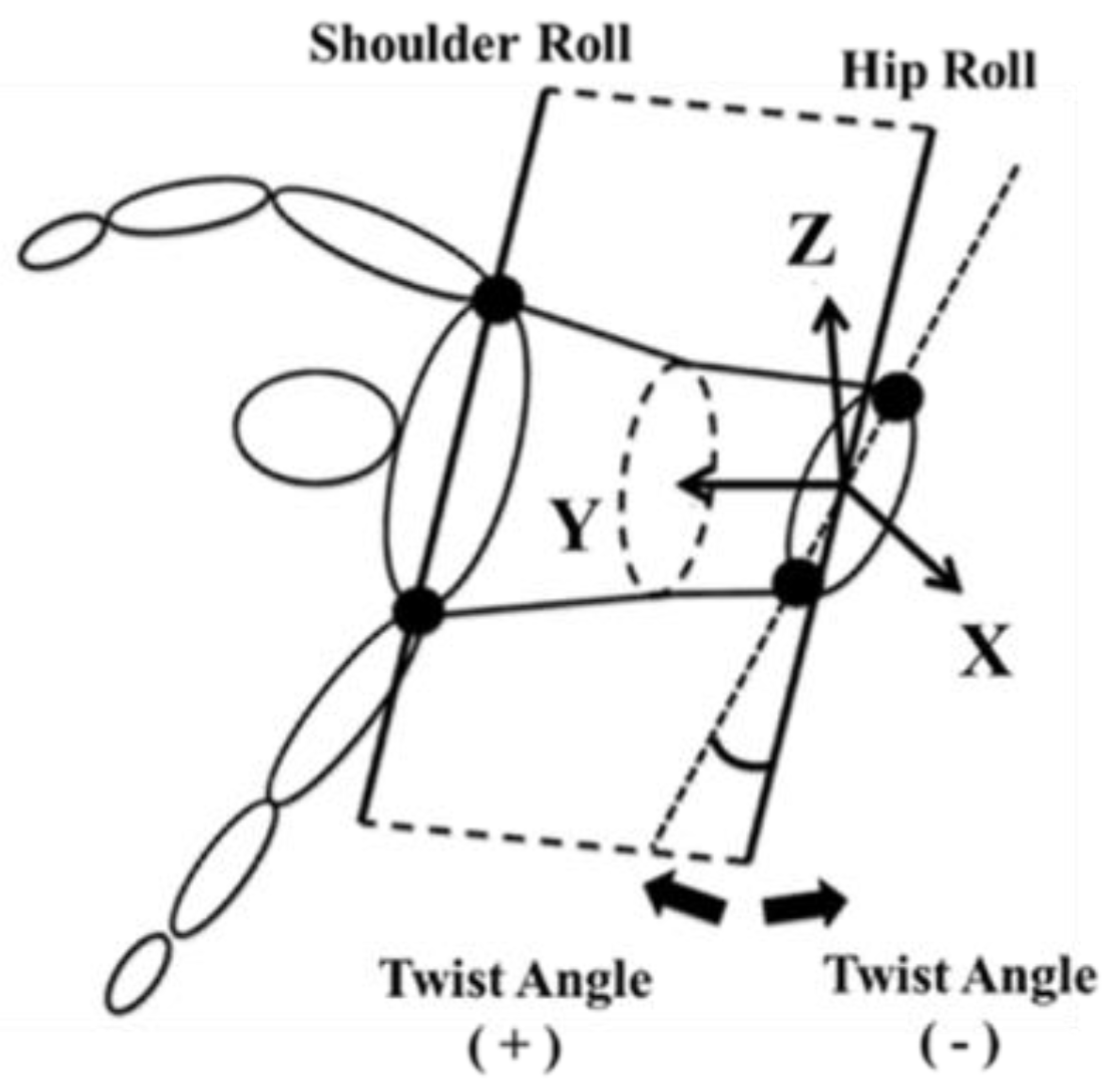
2.2. Measurement Method
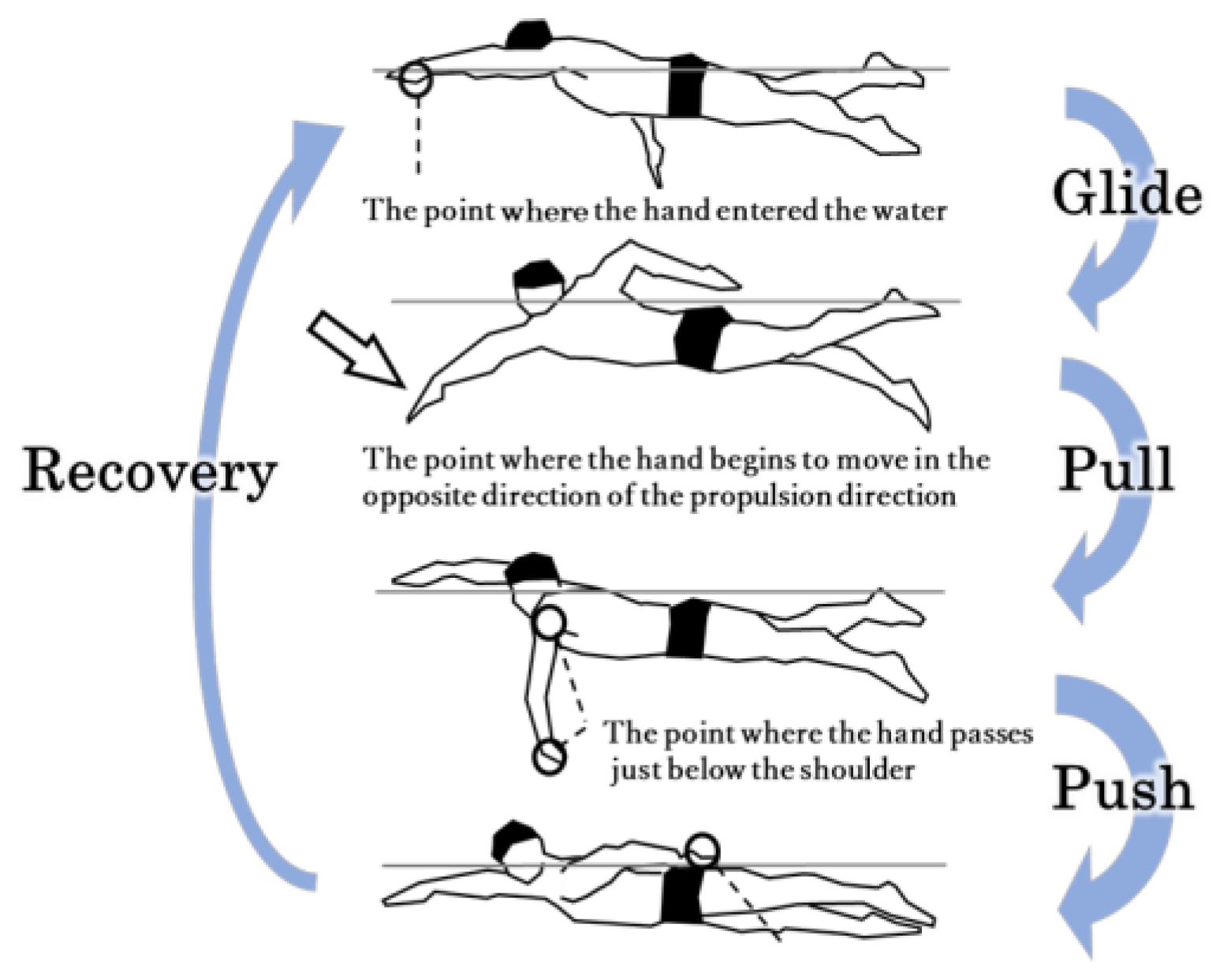
2.3. Phasing of Upper Limb Strokes
2.4. Three-Dimensional Motion Analysis and Measurement of Upper Limb Strokes
2.5. Statistical Analysis
3. Results
3.1. Set Swim Speed (SV), SF, and SL for Each Trial
3.2. Comparison of Different Swimming Speeds
3.3. Relationship between Rotation Angular Velocity and TA
4. Discussion
4.1. Comparison of ShR, HiR, and TA between Trials
4.2. Comparison of Rotation Angular Velocity between Trials
4.3. Relationship between the Rate of Change in TA and the Rate of Change in Rotation Angular Velocity between Trials
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hollander, A.P.; De Groot, G.; van Ingen Schenau, G.J.; Toussaint, H.M.; De Best, H.; Peeters, W.; Schreurs, A.W. Measurement of active drag during crawl arm stroke swimming. J. Sport. Sci. 1986, 4, 21–30. [Google Scholar] [CrossRef]
- Narita, K.; Nakashima, M.; Takagi, H. Effect of leg kick on active drag in front-crawl swimming: Comparison of whole stroke and arms-only stroke during front-crawl and the streamlined position. J. Biomech. 2018, 76, 197–203. [Google Scholar] [CrossRef]
- Watkins, J.; Gordon, A.T. The effects of leg action on performance in the sprint front crawl stroke. Int. Symp. Biomech. Med. Swim. 1983, 14, 310–314. [Google Scholar]
- Deschodt, V.J.; Arsac, L.M.; Rouard, A.H. Relative contribution of arms and legs in humans to propulsion in 25-m sprint front-crawl swimming. Eur. J. Appl. Physiol. Occup. Physiol. 1999, 80, 192–199. [Google Scholar] [CrossRef]
- Gourgoulis, V.; Boli, A.; Aggeloussis, N.; Toubekis, A.; Antoniou, P.; Kasimatis, P.; Mavromatis, G. The effect of leg kick on sprint front crawl swimming. J. Sport. Sci. 2014, 32, 278–289. [Google Scholar] [CrossRef] [PubMed]
- Narita, K.; Nakashima, M.; Takagi, H. Developing a methodology for estimating the drag in front-crawl swimming at various velocities. J. Biomech. 2017, 54, 123–128. [Google Scholar] [CrossRef]
- Tsunokawa, T.; Mankyu, H.; Ogita, F. Relationship between swimming velocity and stroke parameters during the front-crawl. Jpn. Soc. Phys. Educ. Health Sport Sci. 2019, 64, 385–400. [Google Scholar] [CrossRef]
- Yanai, T. Stroke frequency in front crawl: Its mechanical link to the fluid forces required in non-propulsive directions. J. Biomech. 2003, 36, 53–62. [Google Scholar] [CrossRef]
- Schleihauf, R.E. Three-Dimensional Analysis of Hand Propulsion in the Sprint Front Crawl Stroke, Biomechanics and Medicine in Swimming; Human Kinetics Publishers: Champaign, IL, USA, 1983; pp. 173–184. [Google Scholar]
- Grimston, S.K.; Hay, J.G. Relationships among anthropometric and stroking characteristics of college swimmers. Med. Sci. Sport. Exerc. 1986, 18, 60–68. [Google Scholar] [CrossRef]
- Payton, C.; Baltzopoulos, V.; Bartlett, R. Contributions of rotations of the trunk and upper extremity to hand velocity during front crawl swimming. J. Appl. Biomech. 2002, 18, 243–256. [Google Scholar] [CrossRef]
- Kudo, S.; Vennell, R.; Wilson, B. The effect of unsteady flow due to acceleration on hydrodynamic forces acting on the hand in swimming. J. Biomech. 2013, 46, 1697–1704. [Google Scholar] [CrossRef] [PubMed]
- Hyodo, H.; Koga, D.; Wada, T.; Sengoku, Y. A study of shoulder and hip rotation in short-distance front crawl swimming. Jpn. Soc. Phys. Educ. Health Sport Sci. 2021, 66, 841–852. [Google Scholar] [CrossRef]
- Koga, D.; Gonjo, T.; Kawai, E.; Tsunokawa, T.; Sakai, S.; Sengoku, Y.; Takagi, H. Effects of exceeding stroke frequency of maximal effort on hand kinematics and hand propulsive force in front crawl. Sport. Biomech. 2020, 29, 1–13. [Google Scholar] [CrossRef]
- Kennedy, P.; Brown, P.; Chengalur, S.N.; Nelson, R.C. Analysis of male and female Olympic swimmers in the 100-meter events. J. Appl. Biomech. 1990, 6, 187–197. [Google Scholar] [CrossRef]
- Wada, S.; Ae, T.; Endo, T.; Tanaka, M. Biomechanical analysis of the torso twisting during the spiking motion in volleyball. Jpn. Soc. Volleyb. 2003, 5, 1–5. [Google Scholar] [CrossRef]
- Hopkins, W.; Marshall, S.; Batterham, A.; Hanin, J. Progressive statistics for studies in sports medicine and exercise science. Med.+ Sci. Sport.+ Exerc. 2009, 41, 3. [Google Scholar] [CrossRef]
- Welch, C.M.; Banks, S.A.; Cook, F.F.; Draovitch, P. Hitting a baseball: A biomechanical description. J. Orthop. Sport. Phys. Ther. 1995, 22, 193–201. [Google Scholar] [CrossRef] [PubMed]
- Psycharakis, S.G.; Sanders, R.H. Shoulder and hip roll changes during 200-m front crawl swimming. Med. Sci. Sport. Exerc. 2008, 40, 2129–2136. [Google Scholar] [CrossRef]
- Takahashi, K.; Kariyama, H.; Yoshida, T.; Hayashi, R.; Asai, T. Characteristics of force and power outputs during the trunk-twist exercise accompanying stretch-shortening cycle movement. Jpn. Soc. Phys. Educ. Health Sport Sci. 2018, 63, 641–657. [Google Scholar] [CrossRef]
- Kageyama, M.; Iwamoto, M.; Sugiyama, T.; Mizutani, M.; Kanehisa, H.; Maeda, A. Effect of stretch-shortening cycle and pitching movements elicited by trunk rotation on ball velocity in university baseball pitchers. Jpn. Soc. Phys. Educ. Health Sport Sci. 2014, 59, 189–201. [Google Scholar] [CrossRef]
- Kudo, S.; Sakurai, Y.; Miwa, T.; Matsuda, Y. Relationship between shoulder roll and hand propulsion in the front crawl stroke. J. Sport. Sci. 2017, 35, 945–952. [Google Scholar] [CrossRef] [PubMed]
| Subject | Age (years) | Height (cm) | Weight (kg) | Best Record of 50 m FreeStyle (s″) | Fina Point | Best Record of 100 m FreeStyle (s″) | Fina Point |
|---|---|---|---|---|---|---|---|
| A | 21 | 189 | 80 | 23.37 | 716.3 | 51.74 | 745.3 |
| B | 20 | 169 | 71 | 23.94 | 666.3 | 53.04 | 691.8 |
| C | 23 | 180 | 83 | 23.41 | 712.6 | 51.34 | 762.8 |
| D | 18 | 180 | 76 | 24.06 | 656.4 | 51.72 | 746.1 |
| E | 22 | 180 | 74 | 23.59 | 696.4 | 51.91 | 738.0 |
| F | 18 | 185 | 72 | 23.40 | 713.5 | 52.11 | 729.5 |
| G | 21 | 169 | 72 | 23.87 | 672.2 | 50.95 | 780.5 |
| H | 20 | 183 | 75 | 24.26 | 640.3 | 51.43 | 758.8 |
| I | 20 | 175 | 79 | 23.95 | 665.5 | 51.33 | 763.3 |
| J | 27 | 188 | 91 | 21.67 | 898.4 | 48.35 | 913.3 |
| mean | 21.0 | 179.8 | 77.3 | 23.55 | 51.39 | ||
| SD | 2.5 | 6.7 | 5.9 | 0.69 | 1.15 |
| Subject | SV (m/s) | SF (Stroke/min) | SL (m/Stroke) | |||||
|---|---|---|---|---|---|---|---|---|
| V50 m | V100 m | V50 m | V100 m | V50 m | V100 m | |||
| A | 1.97 | 1.85 | 65.22 | 58.82 | 1.81 | 1.89 | ||
| B | 1.94 | 1.81 | 62.50 | 53.57 | 1.86 | 2.03 | ||
| C | 1.97 | 1.86 | 59.41 | 54.55 | 1.99 | 2.05 | ||
| D | 1.92 | 1.86 | 65.22 | 59.41 | 1.77 | 1.88 | ||
| E | 1.98 | 1.85 | 67.42 | 58.25 | 1.76 | 1.91 | ||
| F | 1.99 | 1.84 | 61.86 | 51.72 | 1.93 | 2.13 | ||
| G | 1.93 | 1.88 | 69.77 | 60.61 | 1.66 | 1.86 | ||
| H | 1.90 | 1.86 | 55.56 | 52.63 | 2.05 | 2.12 | ||
| I | 1.94 | 1.88 | 63.83 | 61.22 | 1.82 | 1.84 | ||
| J | 2.14 | 1.98 | 60.00 | 56.07 | 2.14 | 2.12 | ||
| mean | 1.97 ** | 1.87 | 63.08 ** | 56.69 | 1.88 ** | 1.98 | ||
| SD | 0.06 | 0.04 | 3.93 | 3.26 | 0.14 | 0.11 | ||
| Variables | Unit | V50 m | V100m | Difference | Rate of Change (%) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Mean | ± | SD | Mean | ± | SD | |||||||
| ShR | deg | 52.9 | 12.9 | 55.8 | 14.0 | −2.9 | 94.8 | |||||
| HiR | deg | 29.0 | 8.8 | 30.8 | 8.1 | −1.8 | 94.2 | |||||
| TA | deg | 34.0 | 7.2 | 30.6 | 8.9 | 3.3 * | 110.9 | |||||
| ShRAV | Pull Roll | rad/s | −3.5 | 1.0 | −3.8 | 1.0 | 0.3 | 92.7 | ||||
| Pull Roll Back | rad/s | 2.7 | 1.8 | 2.7 | 1.6 | 0.0 | 99.7 | |||||
| Push | rad/s | 4.9 | 1.0 | 4.5 | 0.8 | 0.4 | 108.5 | |||||
| HiRAV | Pull Roll | rad/s | −2.3 | 0.6 | −2.4 | 0.7 | 0.0 | 98.5 | ||||
| Pull Roll Back | rad/s | 2.3 | 1.0 | 2.4 | 0.9 | −0.1 | 97.7 | |||||
| Push | rad/s | 2.5 | 0.7 | 2.4 | 0.7 | 0.1 | 104.3 | |||||
| ShR Peak Time | % | 35.3 | 1.0 | 37.4 | 10.4 | −2.1 | 94.4 | |||||
| HiR Peak Time | % | 29.0 | 12.4 | 33.9 | 12.0 | −4.9 ** | 85.5 | |||||
| Variables | Rate of Change (%) | Correlation Coefficient (r) | ||
|---|---|---|---|---|
| vs. TA | ||||
| TA | 110.9 | |||
| ShRAV | Pull Roll | 92.7 | 0.080 | |
| Pull Roll Back | 99.7 | 0.575 * | ||
| Push | 105.7 | 0.722 ** | ||
| HiRAV | Pull Roll | 97.6 | −0.317 | |
| Pull Roll Back | 97.7 | 0.117 | ||
| Push | 104.3 | 0.748 ** | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hyodo, H.; Koga, D.; Sengoku, Y.; Wada, T. Relationship between Swimming Velocity and Trunk Twist Motion in Short-Distance Crawl Swimming. Biomechanics 2023, 3, 193-203. https://doi.org/10.3390/biomechanics3020017
Hyodo H, Koga D, Sengoku Y, Wada T. Relationship between Swimming Velocity and Trunk Twist Motion in Short-Distance Crawl Swimming. Biomechanics. 2023; 3(2):193-203. https://doi.org/10.3390/biomechanics3020017
Chicago/Turabian StyleHyodo, Hiroki, Daiki Koga, Yasuo Sengoku, and Tadashi Wada. 2023. "Relationship between Swimming Velocity and Trunk Twist Motion in Short-Distance Crawl Swimming" Biomechanics 3, no. 2: 193-203. https://doi.org/10.3390/biomechanics3020017
APA StyleHyodo, H., Koga, D., Sengoku, Y., & Wada, T. (2023). Relationship between Swimming Velocity and Trunk Twist Motion in Short-Distance Crawl Swimming. Biomechanics, 3(2), 193-203. https://doi.org/10.3390/biomechanics3020017






