Biomechanical Modeling of Cerebral Aneurysm Progression to Estimate Rupture Risk
Abstract
1. Introduction
2. Materials and Methods
2.1. Geometrical Modeling
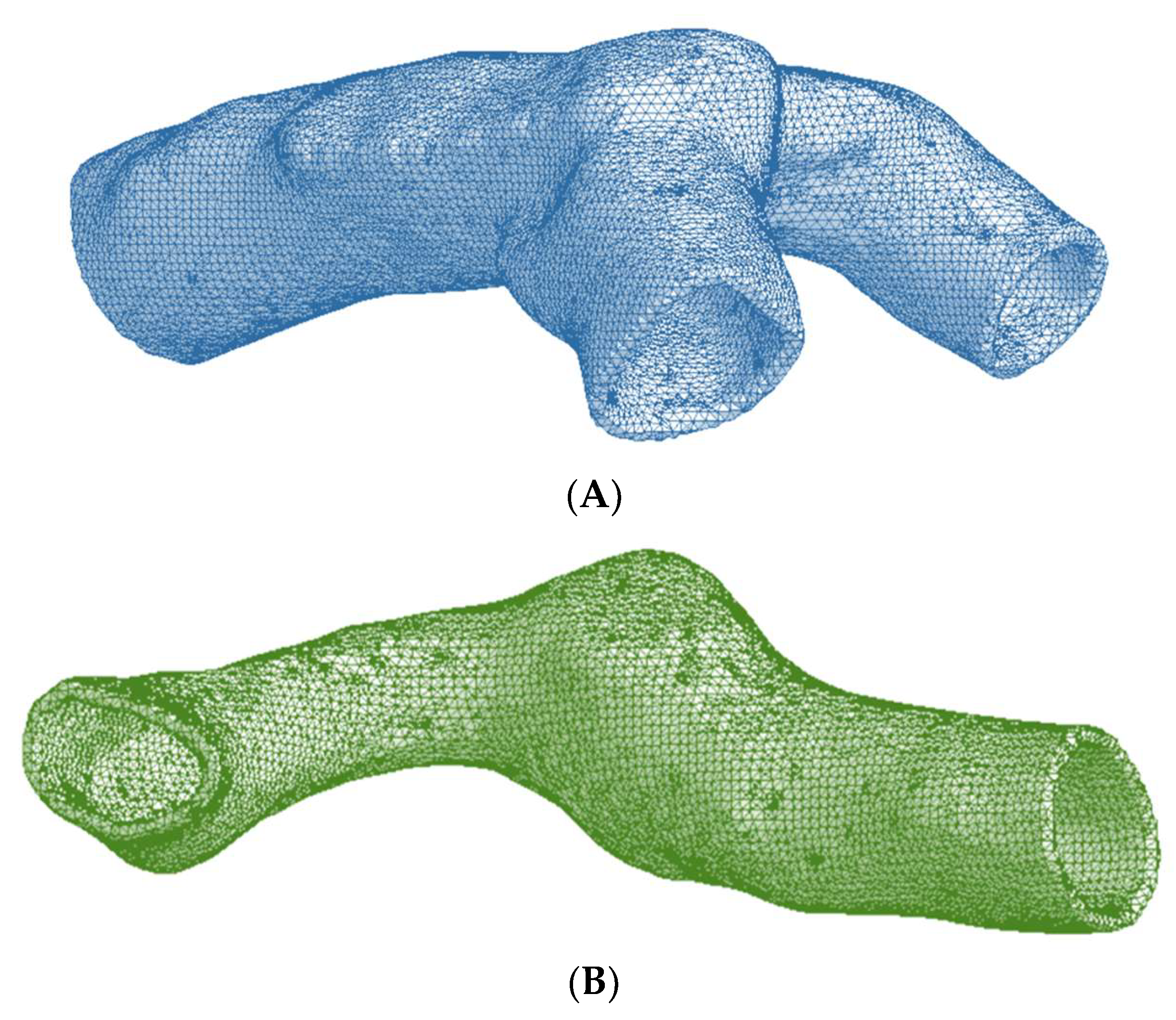
2.1.1. Arterial Segmentation and Modeling
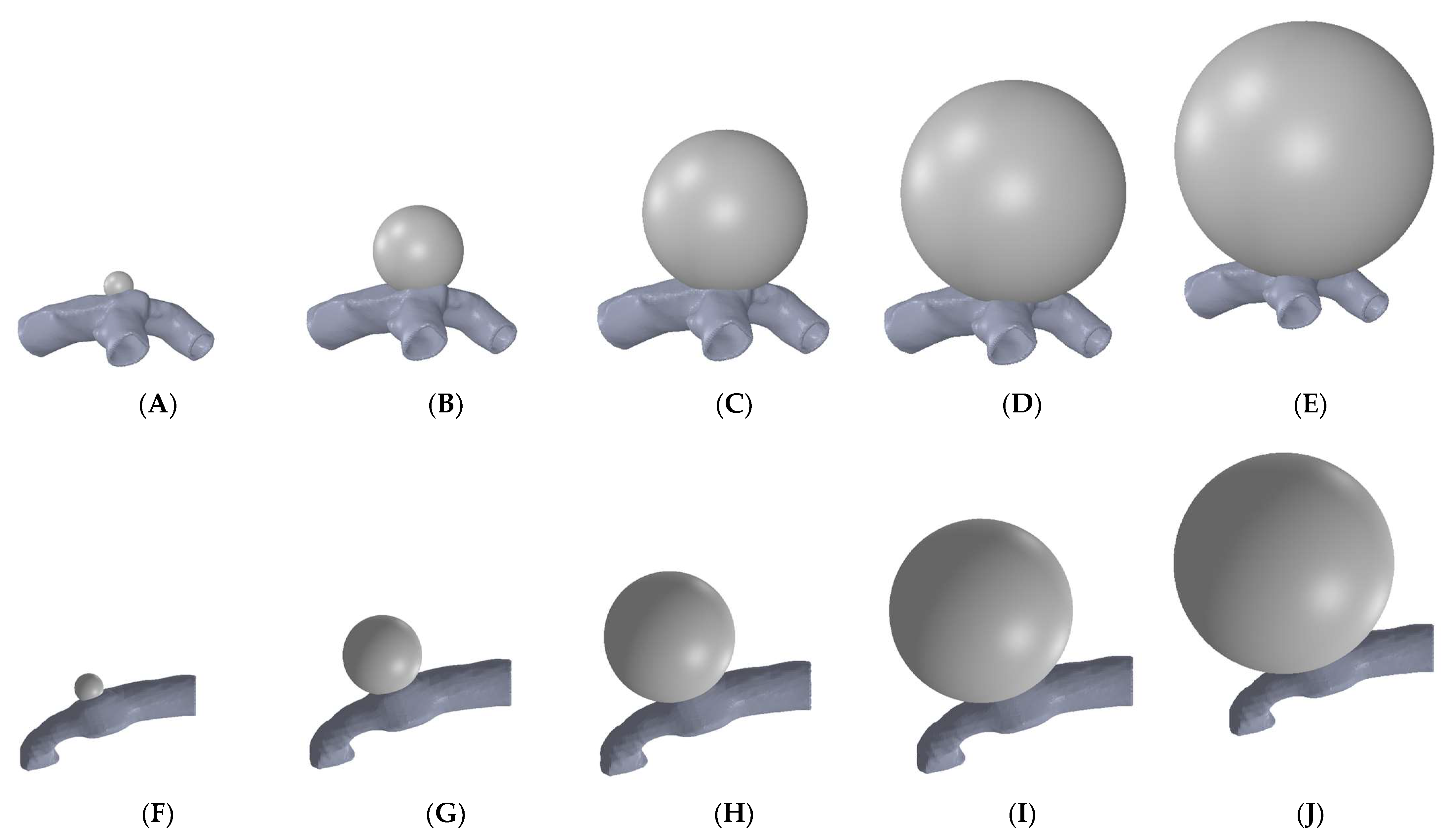
2.1.2. Modeling of Different Grades of Aneurysm
2.2. Finite Element Modeling
2.2.1. Meshing and Mesh Convergence Study
2.2.2. Material Properties
2.2.3. Loads and Boundary Conditions
3. Results and Discussion
3.1. Mesh Convergence Results
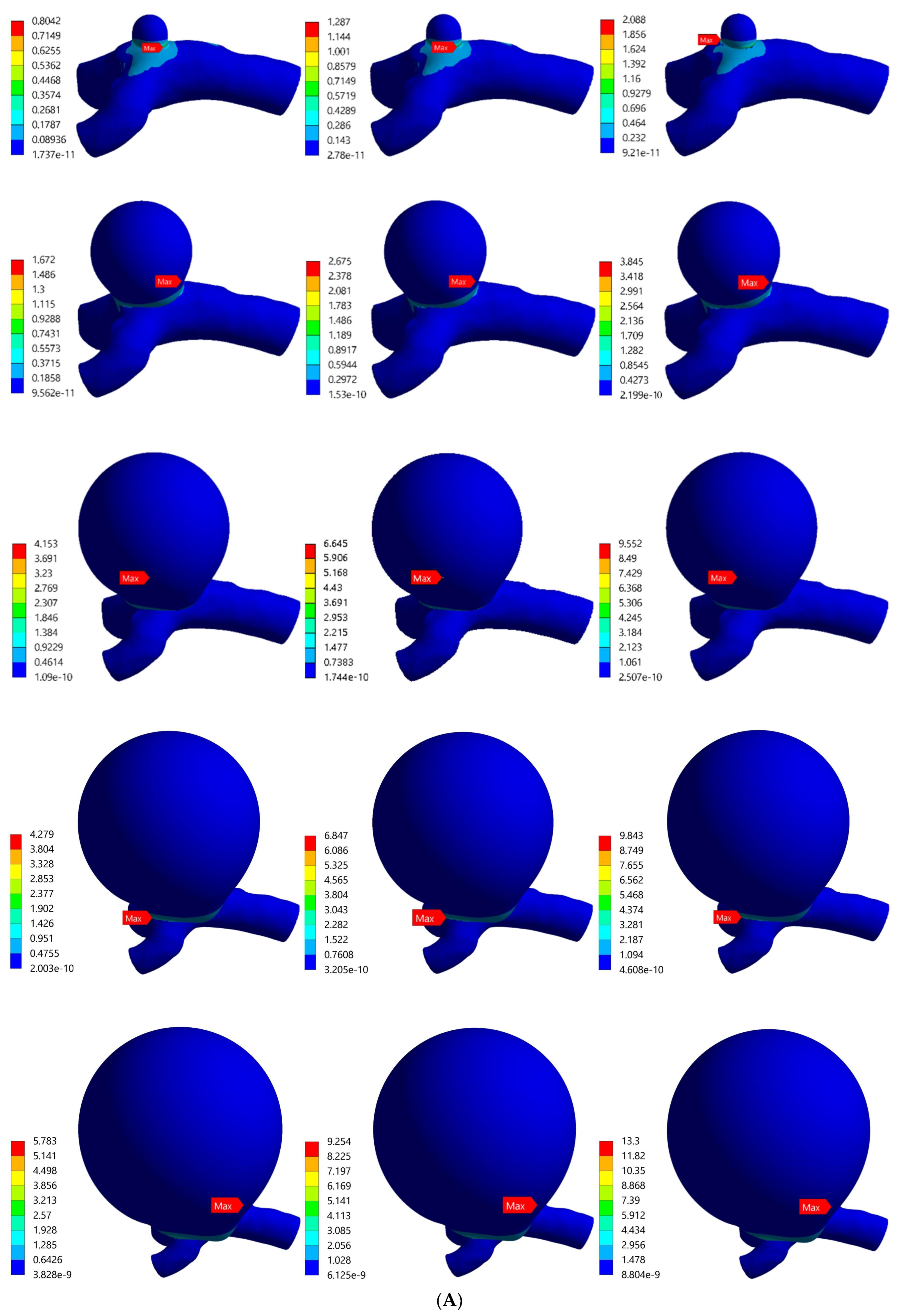
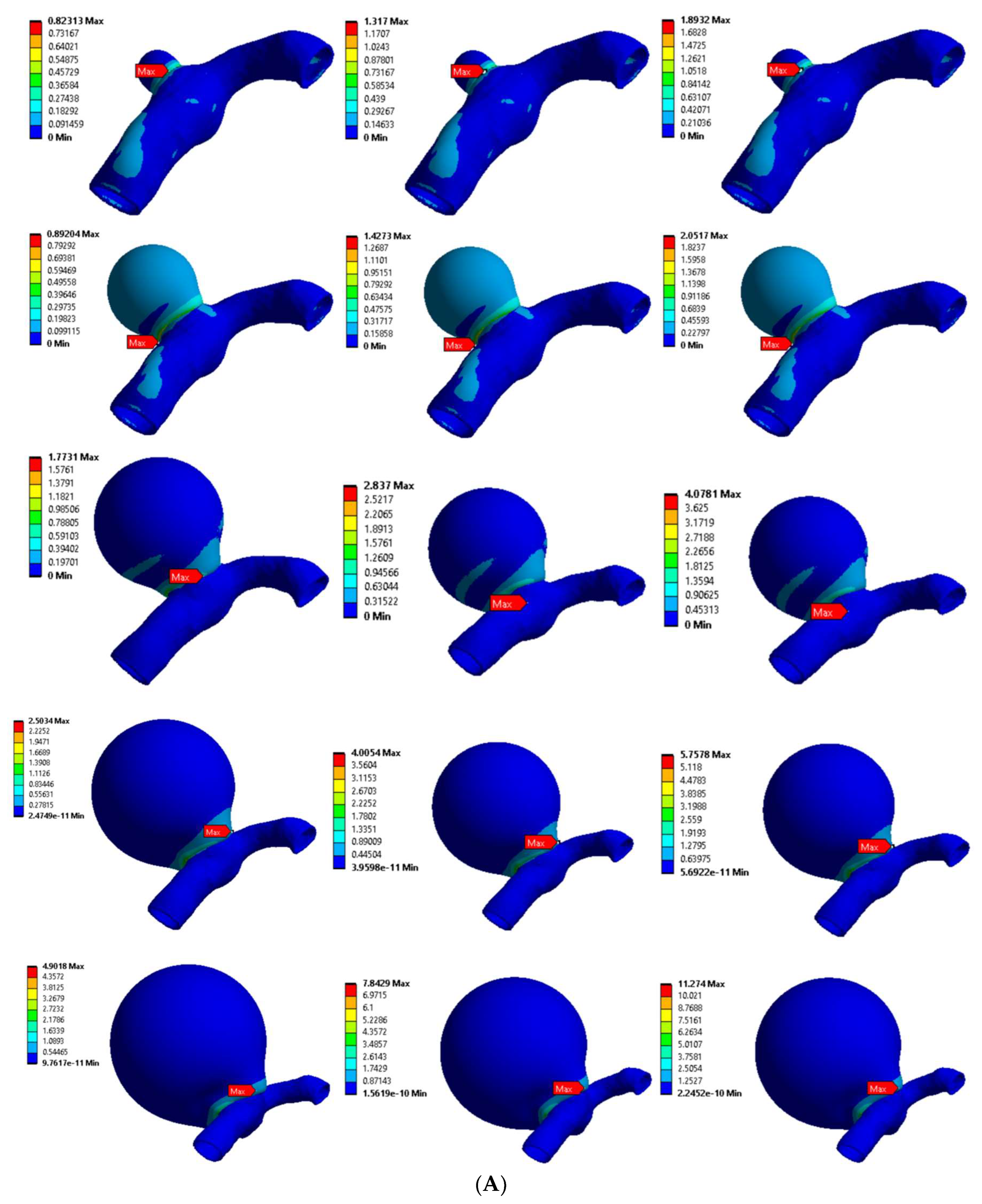
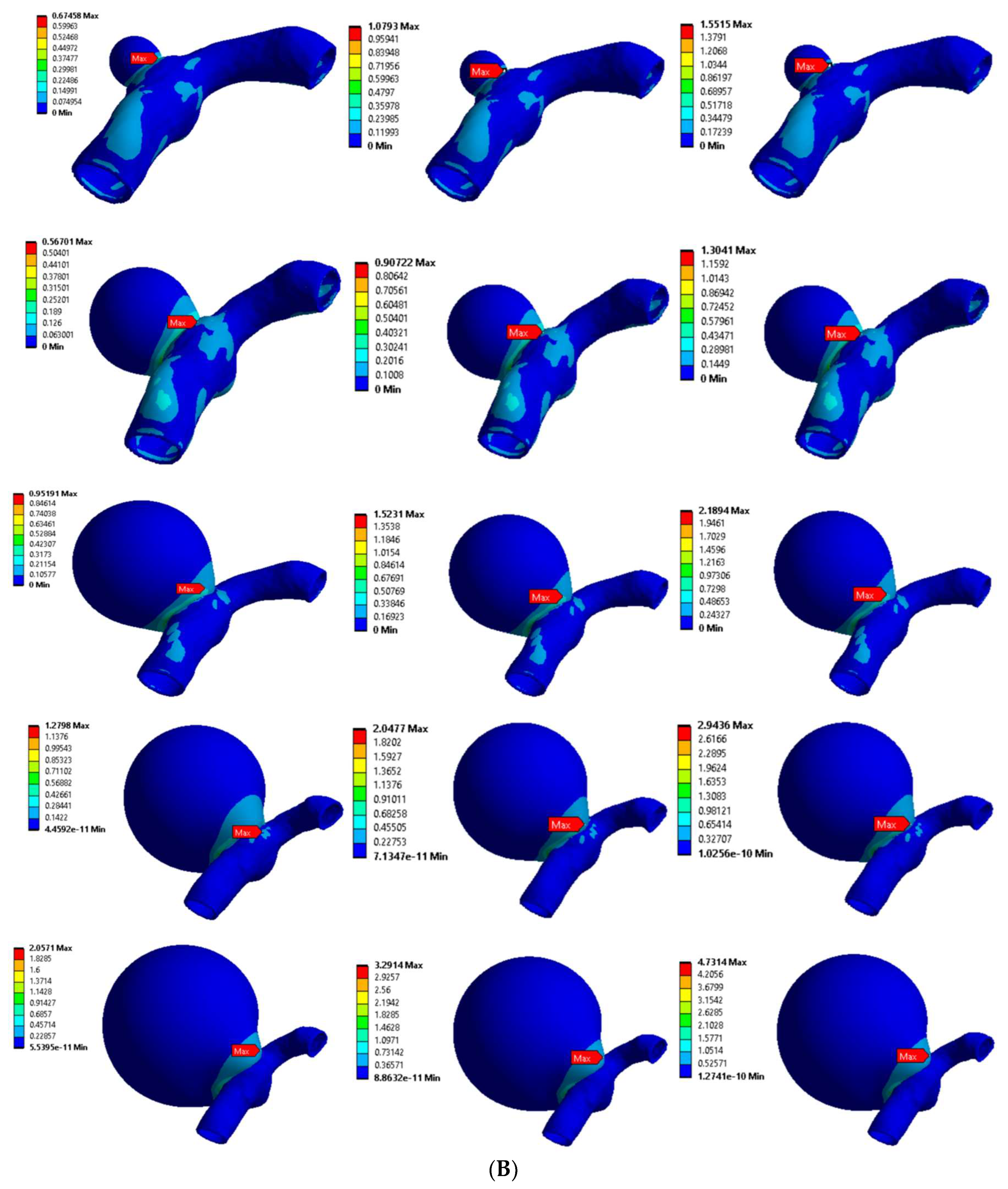
3.2. Stress Distribution with Aneurysm Growth
3.2.1. Bifurcated MCA Section
3.2.2. Curved MCA Section
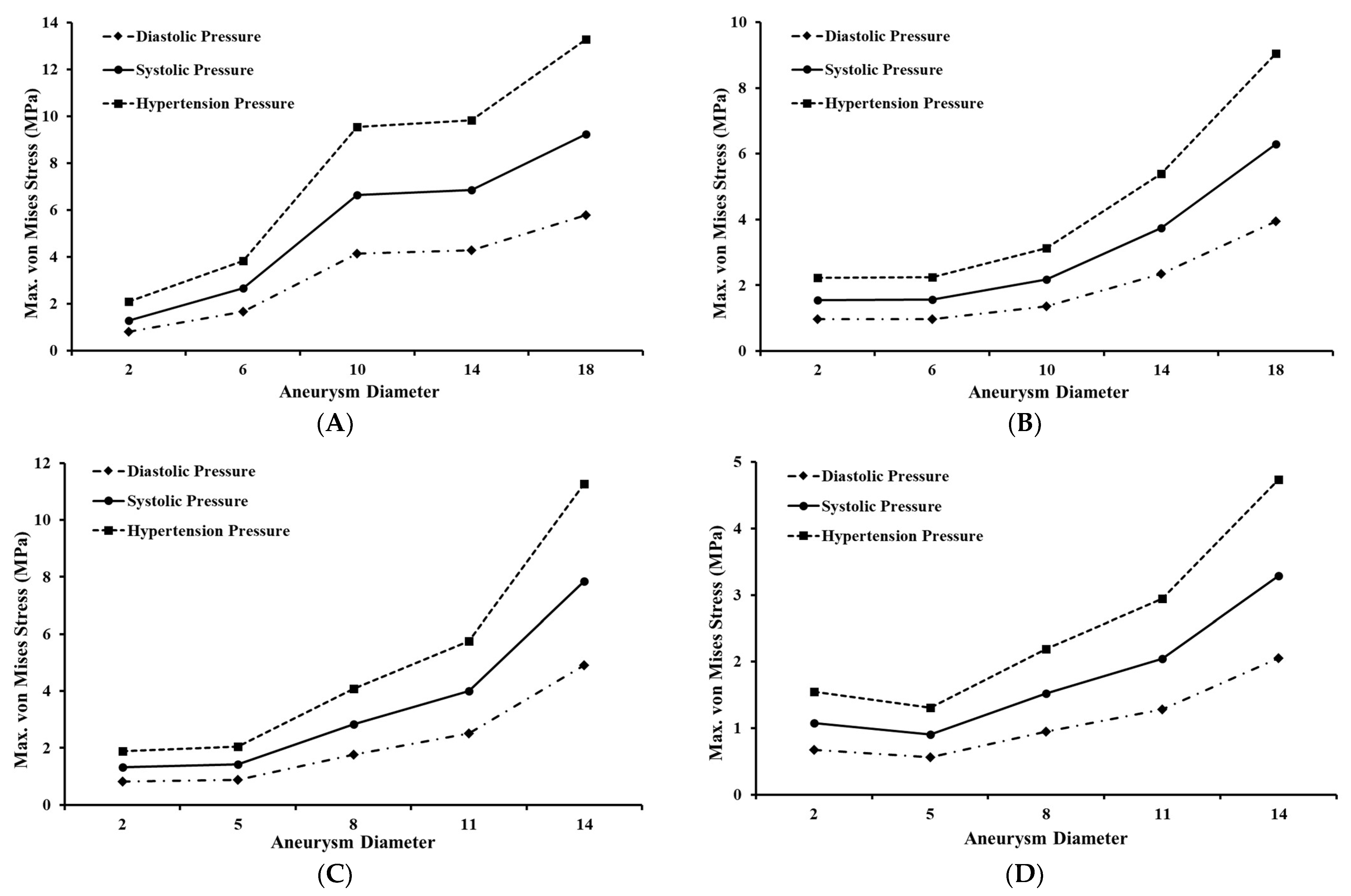
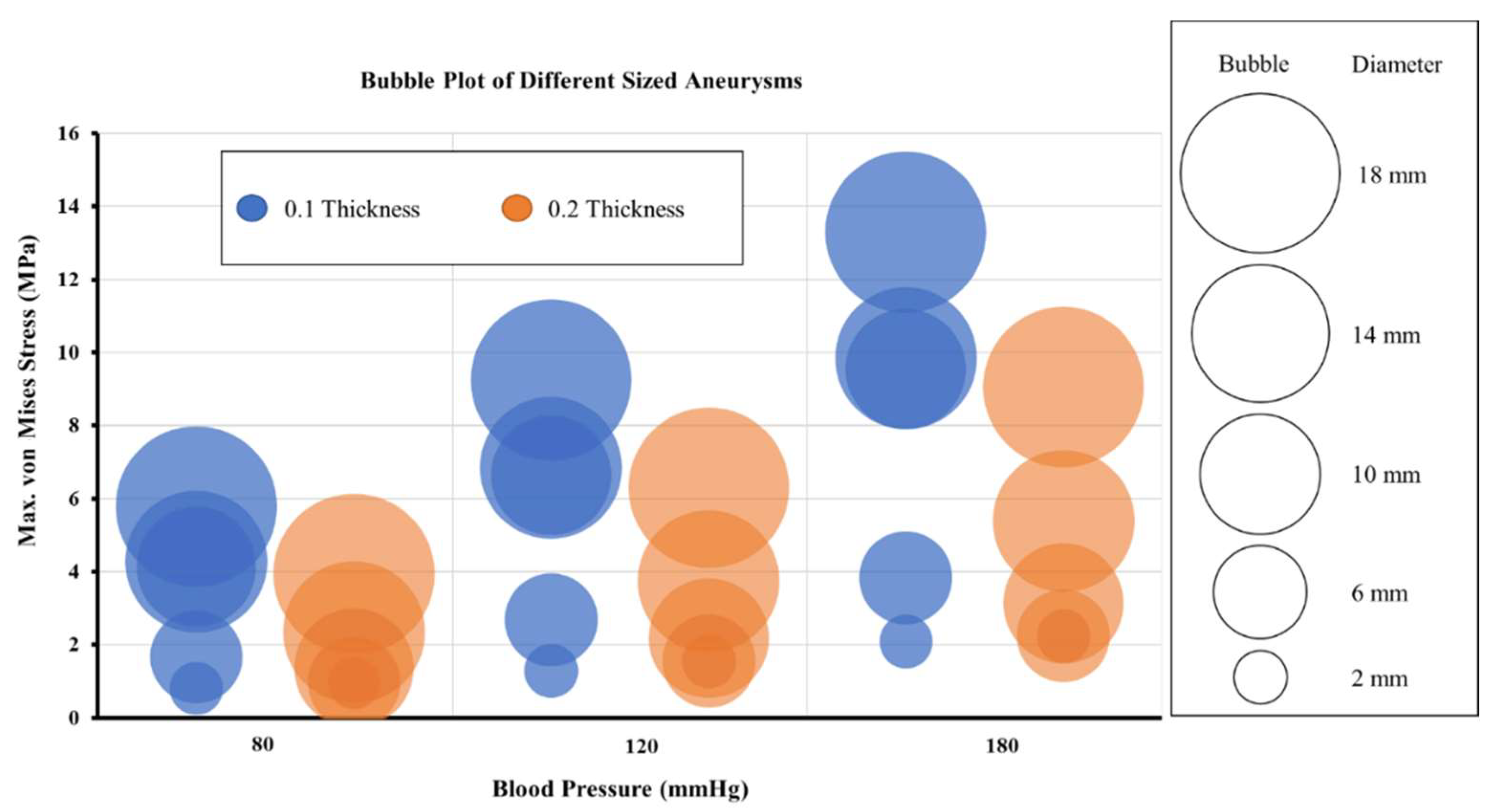
3.3. Effect of Structural Parameters on the Induced Stresses in Aneurysm Wall
3.4. Effect of Varying Blood Pressure on Aneurysm Wall Stresses
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ferrari, G. Cerebral Aneurysm and Complications: Diagnosis and Treatment. Riv. Di Neuroradiol. 2002, 15, 501–506. [Google Scholar] [CrossRef]
- Seok, J.H.; Seok, C.J.H. Current Science Management of Cerebral Aneurysm, Comment. J. Mol. Pathophysiol. 2021, 10, 1–2. [Google Scholar]
- Fennell, V.S.; Kalani, M.Y.S.; Atwal, G.; Martirosyan, N.L.; Spetzler, R.F. Biology of Saccular Cerebral Aneurysms: A Review of Current Understanding and Future Directions. Front. Surg. 2016, 3, 43. [Google Scholar] [CrossRef]
- Li, D.; Robertson, A.M. A structural multi-mechanism damage model for cerebral arterial tissue. J. Biomech. Eng. 2009, 14–15, 2920–2928. [Google Scholar] [CrossRef] [PubMed]
- Miyata, H.; Koseki, H.; Takizawa, K.; Kasuya, H.; Nozaki, K.; Narumiya, S.; Aoki, T. T cell function is dispensable for intracranial aneurysm formation and progression. PLoS ONE 2017, 12, e0175421. [Google Scholar] [CrossRef]
- Revilla-Pacheco, F.; Escalante-Seyffert, M.C.; Herrada-Pineda, T.; Manrique-Guzman, S.; Perez-Zuniga, I.; Rangel-Suarez, S.; Rubalcava-Ortega, J.; Loyo-Varela, M. Prevalence of Incidental Clinoid Segment Saccular Aneurysms. World Neurosurg. 2018, 115, e244–e251. [Google Scholar] [CrossRef]
- Chalouhi, N.; Hoh, B.L.; Hasan, D. Review of cerebral aneurysm formation, growth, and rupture. Stroke 2013, 44, 3613–3622. [Google Scholar] [CrossRef]
- Rinkel, G.J.E.; Djibuti, M.; Algra, A.; Van Gijn, J. Prevalence and Risk of Rupture of Intracranial Aneurysms. Stroke 1998, 29, 251–256. [Google Scholar] [CrossRef]
- Kroon, M. Simulation of cerebral aneurysm growth and prediction of evolving rupture risk. Model. Simul. Eng. 2011, 2011, 289523. [Google Scholar] [CrossRef]
- Jiang, B.; Paff, M.; Colby, G.P.; Coon, A.L.; Lin, L.-M. Cerebral aneurysm treatment: Modern neurovascular techniques. Stroke Vasc. Neurol. 2016, 1, 93–100. [Google Scholar] [CrossRef]
- Vol, M.-J.; Drake, C.G. Progress in cerebrovascular disease. Management of cerebral aneurysm. Stroke 1981, 12, 273–283. [Google Scholar] [CrossRef]
- De Gelidi, S.; Bucchi, A. Comparative finite element modelling of aneurysm formation and physiologic inflation in the descending aorta. Comput. Methods Biomech. Biomed. Eng. 2019, 22, 1197–1208. [Google Scholar] [CrossRef] [PubMed]
- Cebral, J.R.; Castro, M.A.; Burgess, J.E.; Pergolizzi, R.S.; Sheridan, M.J.; Putman, C.M. Characterization of cerebral aneurysms for assessing risk of rupture by using patient-specific computational hemodynamics models. Am. J. Neuroradiol. 2005, 26, 2550–2559. [Google Scholar] [CrossRef] [PubMed]
- John, E.; Ziema-Davis, M.; Edwards, G.; Brom, G.; Shah, K. Anterior communicating artery complex aneurysms: Anatomic characteristics as predictors of surgical outcome in 300 cases. World Neurosurg. 2019, 122, e896–e906. [Google Scholar]
- Jeong, W.; Rhee, K. Hemodynamics of cerebral aneurysms: Computational analyses of aneurysm progress and treatment. Comput. Math. Methods Med. 2012, 2012, 782801. [Google Scholar] [CrossRef] [PubMed]
- Valencia, A.; Burdiles, P.; Ignat, M.; Mura, J.; Bravo, E.; Rivera, R.; Sordo, J. Fluid structural analysis of human cerebral aneurysm using their own wall mechanical properties. Comput. Math. Methods Med. 2013, 2013, 293128. [Google Scholar] [CrossRef] [PubMed]
- You, S.H.; Kong, D.S.; Kim, J.S.; Jeon, P.; Kim, K.H.; Roh, H.K.; Kim, G.M.; Lee, K.H.; Hong, S.C. Characteristic features of unruptured intracranial aneurysms: Predictive risk factors for aneurysm rupture. J. Neurol. Neurosurg. Psychiatry 2010, 81, 479–484. [Google Scholar] [CrossRef]
- Korja, M.; Kivisaari, R.; Jahromi, B.R.; Lehto, H. Size and location of ruptured intracranial aneurysms: Consecutive series of 1993 hospital-admitted patients. J. Neurosurg. 2017, 127, 748–753. [Google Scholar] [CrossRef]
- Macdonald, D.J.; Finlay, H.M.; Canham, P.B. Directional Wall Strength in Saccular Brain Aneurysms from Polarized Light Microscopy. Ann. Biomed. Eng. 2000, 28, 533–542. [Google Scholar] [CrossRef]
- Parlea, L.; Fahrig, R.; Holdsworth, D.W.; Lownie, S.P. An analysis of the geometry of saccular intracranial aneurysms. Am. J. Neuroradiol. 1999, 20, 1079–1089. [Google Scholar]
- Paz, C.; Suarez, E.; Cabarcos, A.; Pinto, S.I.S. FSI modeling on the effect of artery-aneurysm thickness and coil embolization in patient cases. Comp. Meth. Prog. Biomed. 2021, 206, 106148. [Google Scholar] [CrossRef] [PubMed]
- Muhib, F.; Islam, M.D.; Arafat, M.T. A study on the computational hemodynamic and mechanical parameters for understanding intracranial aneurysms of patients with hypertension and atrial fibrillation. Inform. Med. Unlocked 2022, 32, 101031. [Google Scholar] [CrossRef]
- Xu, L.; Liang, F.; Zhao, B.; Wan, J.; Liu, H. Influence of aging-induced flow waveform variation on hemodynamics in aneurysms present at the internal carotid artery: A computational model-based study. Comp. Biol. Med. 2018, 101, 51–60. [Google Scholar] [CrossRef] [PubMed]
- Dubey, A.; Vasu, B.; Beg, O.A.; Gorla, R.S.R. Finite element computation of magneto-hemodynamic flow and heat transfer in a bifurcated artery with saccular aneurysm using the Carreau-Yasuda biorheological model. Microvasc. Res. 2021, 138, 104221. [Google Scholar] [CrossRef]
- Liu, J.; Chen, Y.; Xiong, Y.; Chen, X.; Duan, Y.; Yang, Y. Computational hemodynamics study of anterior communicating artery aneurysm associated with fenestration: A case report. Interdiscip. Neurosurg. 2020, 19, 100563. [Google Scholar] [CrossRef]
- Ebrahimi, A.P. Mechanical properties of normal and diseased cerebrovascular system. J. Vasc. Interv. Neurol. 2009, 2, 155–162. [Google Scholar]
- Isaksen, J.G.; Bazilevs, Y.; Kvamsdal, T.; Zhang, Y.; Kaspersen, J.H.; Waterloo, K.; Romner, B.; Ingebrigtsen, T. Determination of wall tension in cerebral artery aneurysms by numerical simulation. Stroke 2008, 39, 3172–3178. [Google Scholar] [CrossRef]
- Frolov, S.V.; Potlov, A.Y.; Proskurin, S.G.; Frolova, T.A. Young’s Modulus Evaluation of the Walls of Cerebral Arteries with Aneurysms. In Proceedings of the 2020 Medical Technologies Congress (TIPTEKNO), Antalya, Turkey, 19–20 November 2020; pp. 31–34. [Google Scholar] [CrossRef]
- Humphrey, J.D.; Canham, P.B. Structure, Mechanical Properties, and Mechanics of Intracranial Saccular Aneurysms. J. Elast. 2000, 61, 49–81. [Google Scholar] [CrossRef]




| MCA Section | Aneurysm Diameter (mm) | ||||
|---|---|---|---|---|---|
| Bifurcated | 2 | 6 | 10 | 14 | 18 |
| Curvature | 2 | 5 | 8 | 11 | 14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yadav, P.N.; Singh, G.; Gupta, S.; Chanda, A. Biomechanical Modeling of Cerebral Aneurysm Progression to Estimate Rupture Risk. Biomechanics 2023, 3, 13-28. https://doi.org/10.3390/biomechanics3010002
Yadav PN, Singh G, Gupta S, Chanda A. Biomechanical Modeling of Cerebral Aneurysm Progression to Estimate Rupture Risk. Biomechanics. 2023; 3(1):13-28. https://doi.org/10.3390/biomechanics3010002
Chicago/Turabian StyleYadav, Prem Nath, Gurpreet Singh, Shubham Gupta, and Arnab Chanda. 2023. "Biomechanical Modeling of Cerebral Aneurysm Progression to Estimate Rupture Risk" Biomechanics 3, no. 1: 13-28. https://doi.org/10.3390/biomechanics3010002
APA StyleYadav, P. N., Singh, G., Gupta, S., & Chanda, A. (2023). Biomechanical Modeling of Cerebral Aneurysm Progression to Estimate Rupture Risk. Biomechanics, 3(1), 13-28. https://doi.org/10.3390/biomechanics3010002








