On the Behavior of Bauxite Tailings under a Wide Range of Stresses
Abstract
1. Introduction
2. Materials and Methods
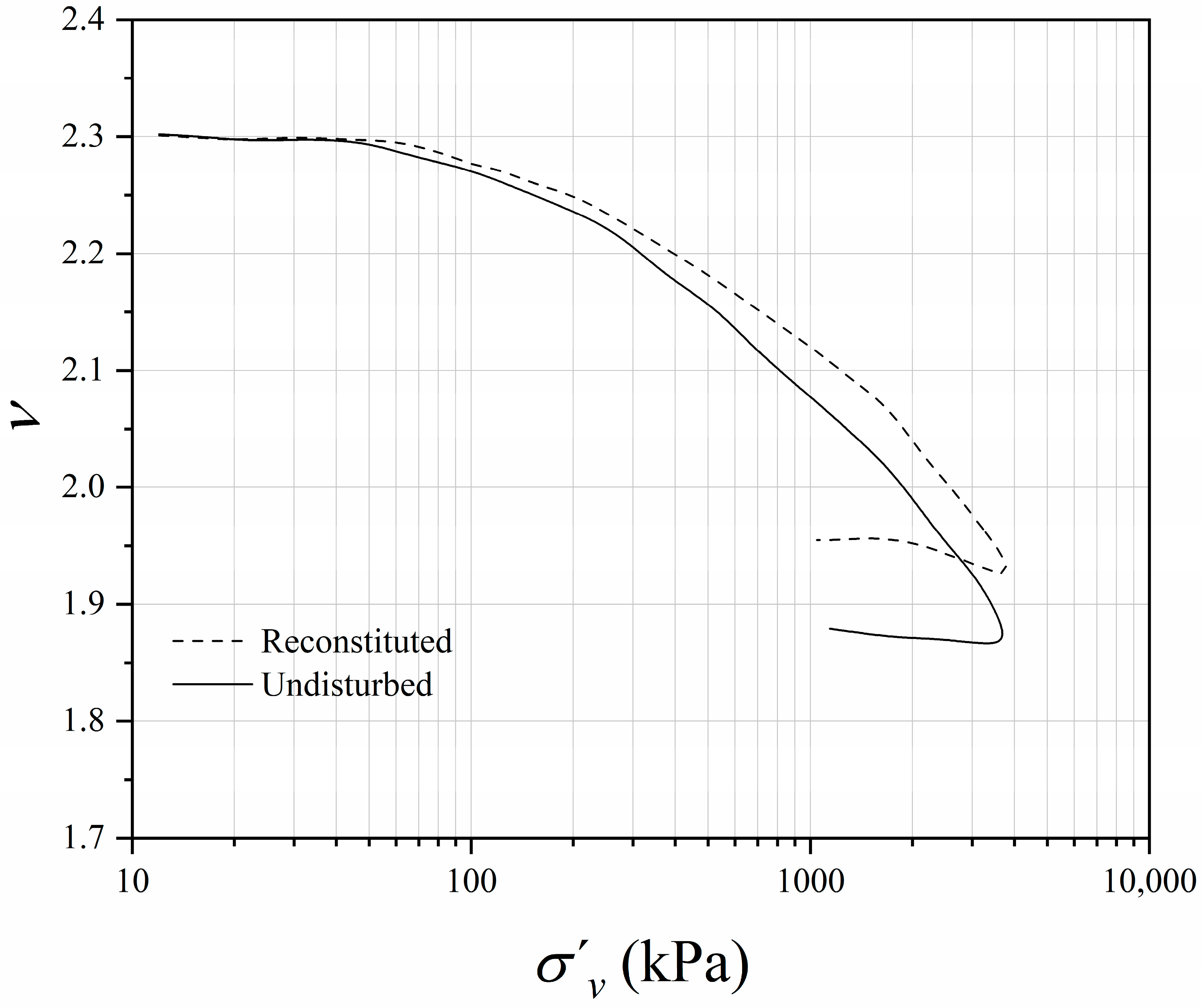
3. One-Dimensional Compression Tests
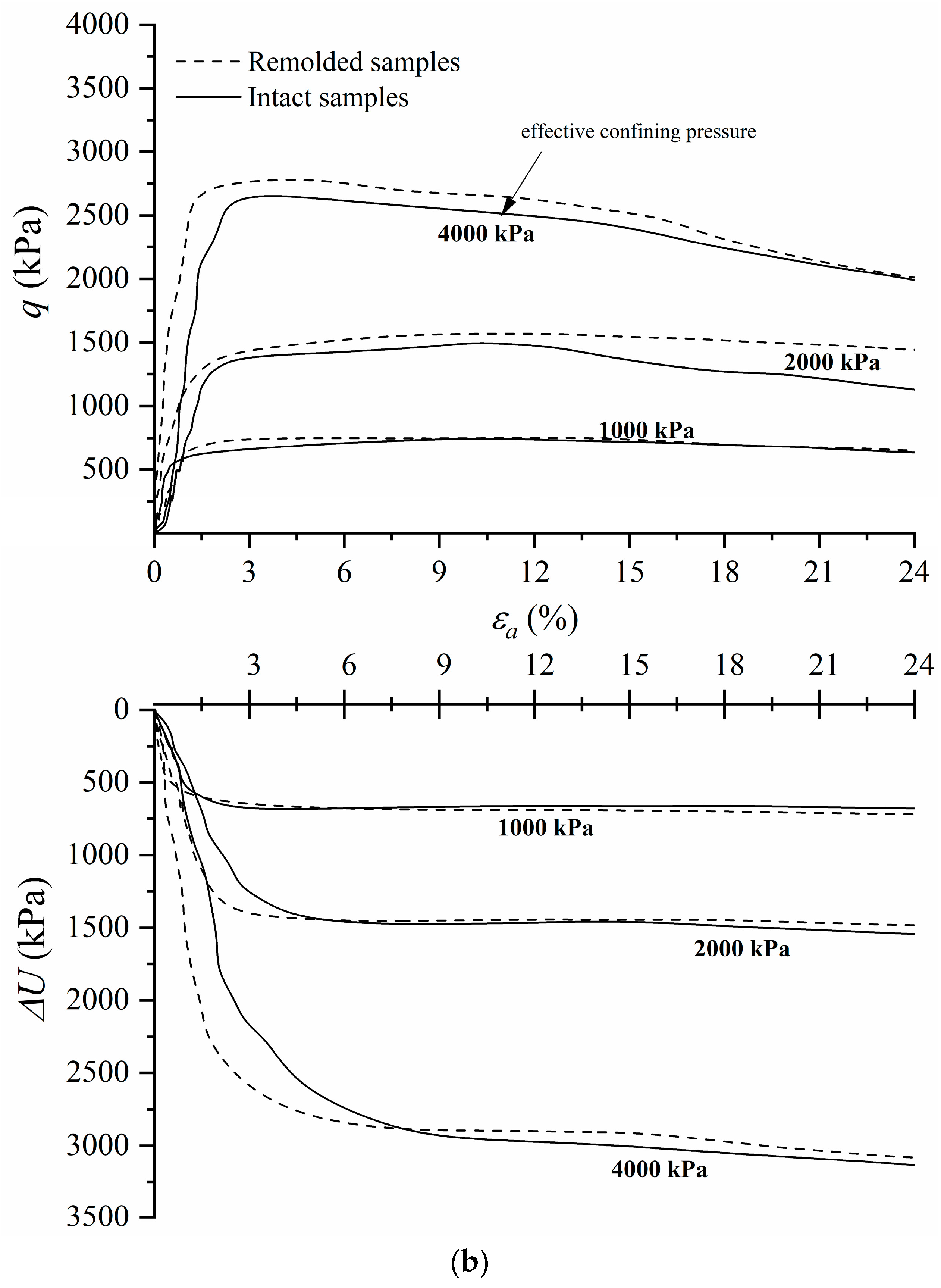
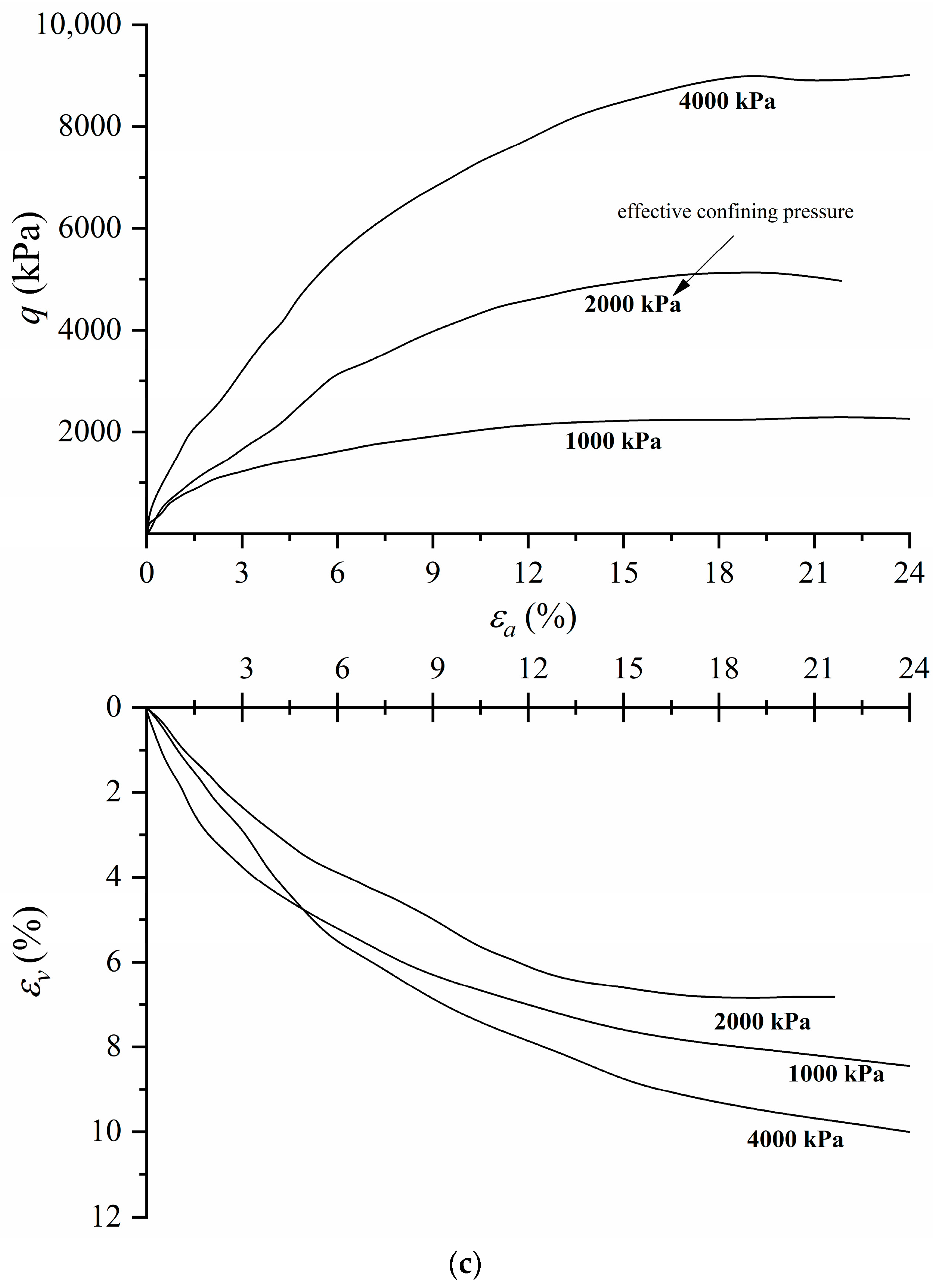
4. Triaxial Tests
5. Results
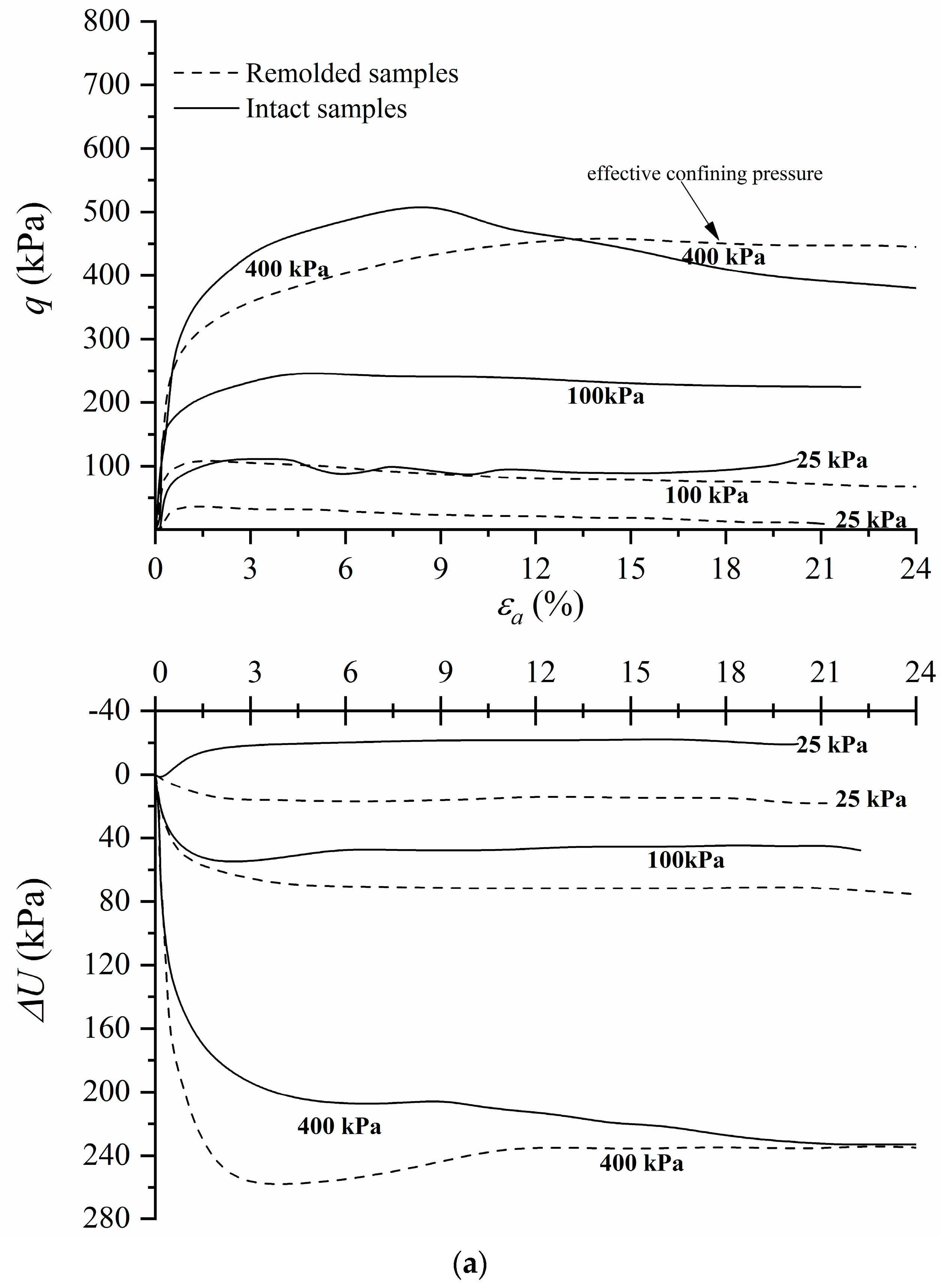
6. Shearing Behavior in the Triaxial Tests
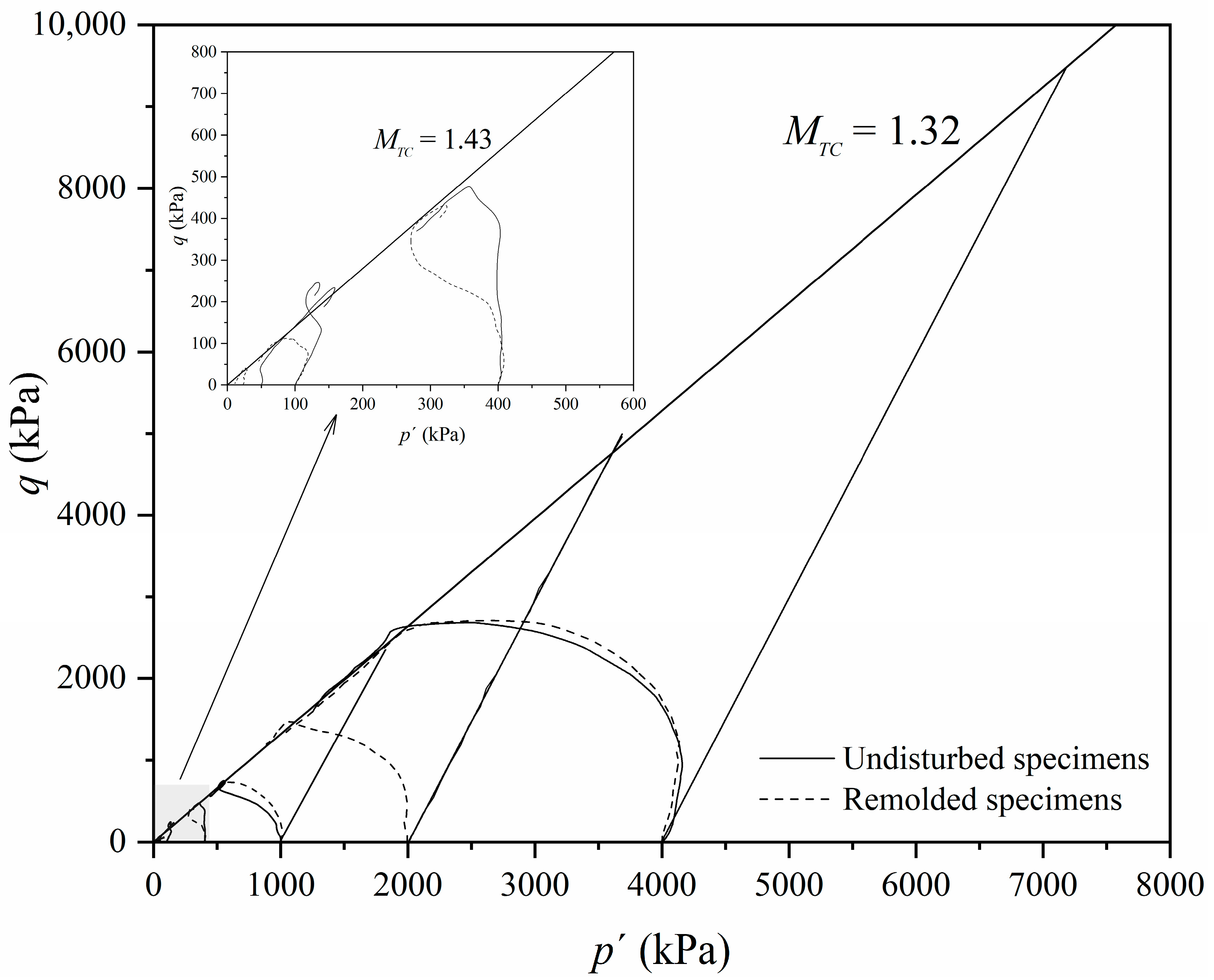
7. Stress Paths and Critical States
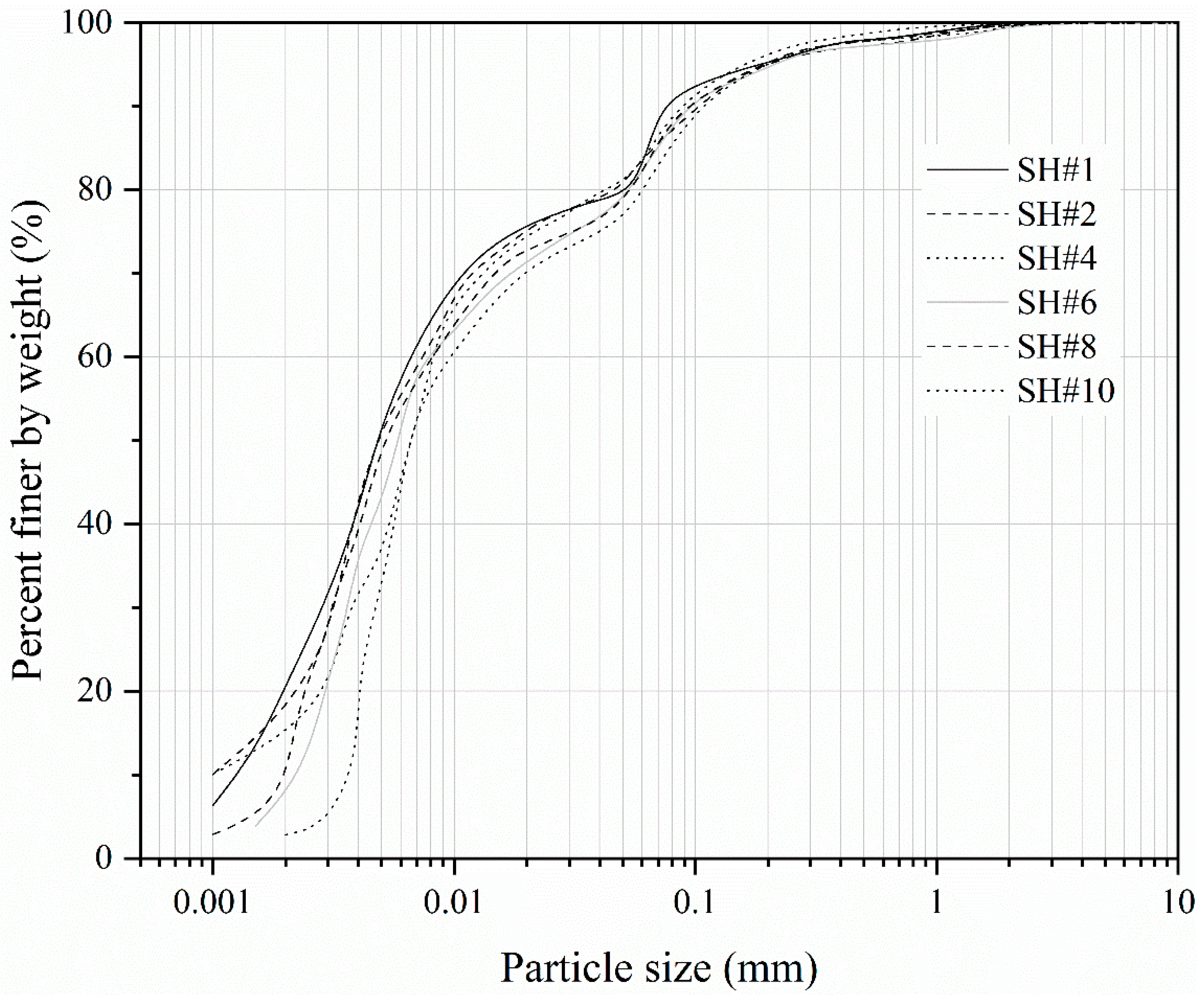
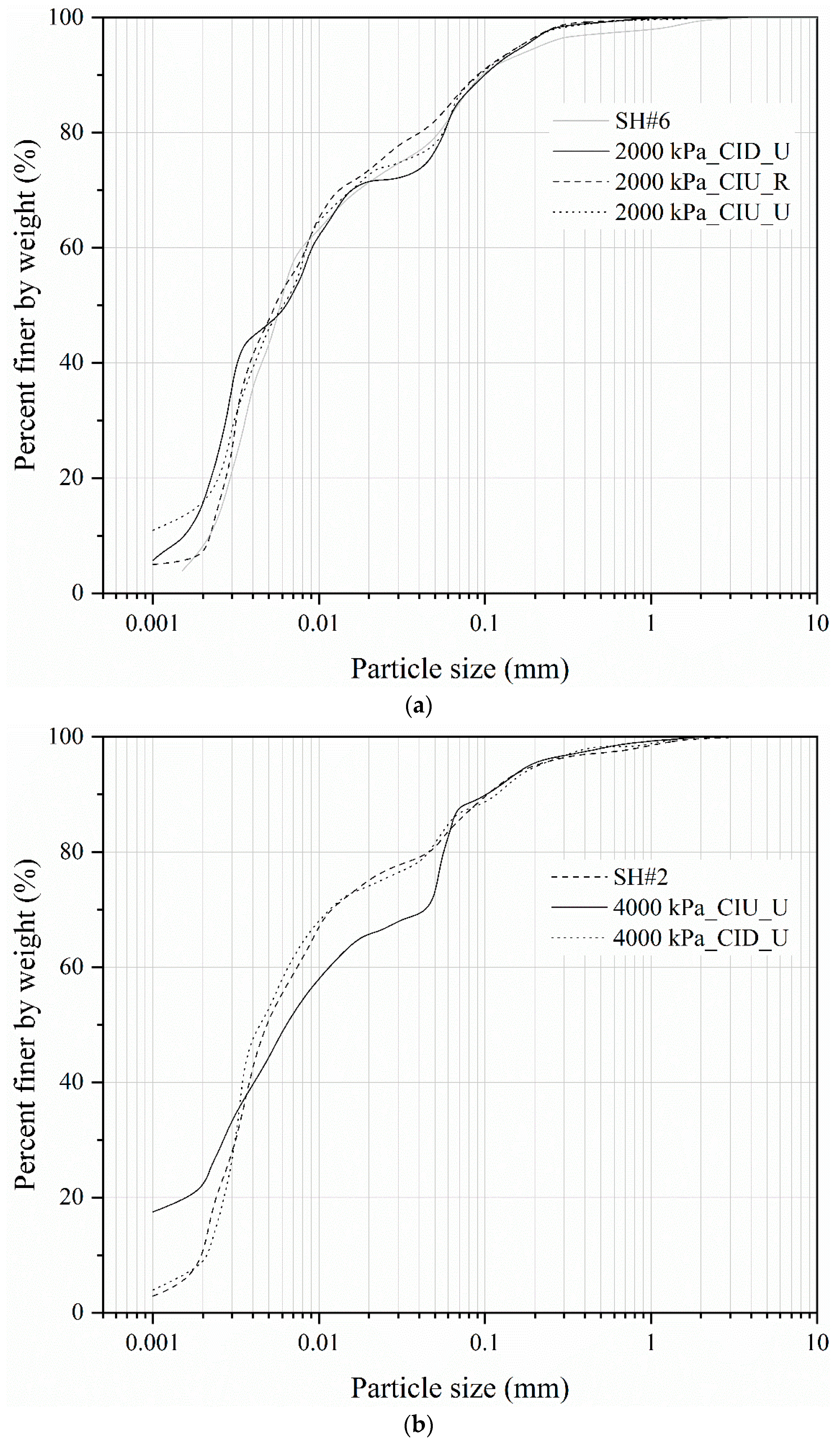
8. Grain Size Analysis
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
List of Notations
| Bf | breakage factor |
| e | void ratio; void ratio for undisturbed sample |
| emax | maximum void ratio |
| emin | minimum void ratio |
| p’ | mean effective stress |
| q | deviatoric stress |
| CSL | critical state line |
| LL | liquid limit |
| NCL | normal compression line |
| ICL | isotropic compression line |
| PI | plasticity index |
| PL | plasticity limit |
| εa | axial strain |
| SG | specific gravity of solids |
| ν | specific volume |
| σ’v | effective vertical stress |
| ϕ’cs | critical state angle of friction |
| R | remolded |
| U | undisturbed |
References
- IBRAM—Instituto Brasileiro de Mineração. Gestão E Manejo de Rejeitos de Mineração; IBRAM: Brasilia, Brazil, 2016; ISBN 978-85-61993-10-8. [Google Scholar]
- Nkuna, R.; Ijoma, G.N.; Matambo, T.S.; Chimwani, N. Accessing Metals from Low-Grade Ores and the Environmental Impact Considerations: A Review of the Perspectives of Conventional versus Bioleaching Strategies. Minerals 2022, 12, 506. [Google Scholar] [CrossRef]
- Kossoff, D.; Dubbin, W.E.; Alfredsson, M.; Edwards, S.J.; Macklin, M.G.; Hudson-Edwards, K.A. Mine Tailings Dams: Characteristics, Failure, Environmental Impacts, and Remediation. Appl. Geochem. 2014, 51, 229–245. [Google Scholar] [CrossRef]
- Silva, J.P.S.; Rissoli, A.L.C.; Cacciari, P.P.; da Fonseca, A.J.P.V.; Filho, H.C.S.; Wagner, A.C.; Carvalho, J.V.d.A.; Festugato, L.; Consoli, N.C. Triaxial Testing Response of Compacted Iron Ore Tailings Considering a Broad Spectrum of Confining Pressures. Soils Found. 2024, 64, 101438. [Google Scholar] [CrossRef]
- ANM—Agência Nacional de Mineiração Cadastro Nacional. Sistema de Gestão de Segurança de Barragens de Mineração—SIGBM; ANM: Brasília, Brazil, 2021. [Google Scholar]
- Coop, M.R.; Lee, I.K.; Houlsby, G.T.; Schofield, A.N. The Behaviour of Granular Soils at Elevated Stresses. In Proceedings of the Wroth Memorial Symposium Held at St Catherine’s College, Oxford, UK, 27–29 July 1992; Thomas Telford: Oxford, UK, 1992. [Google Scholar]
- Bedin, J.; Schnaid, F.; Da Fonseca, A.V.; Costa Filho, L.D.M. Gold Tailings Liquefaction under Critical State Soil Mechanics. Géotechnique 2012, 62, 263–267. [Google Scholar] [CrossRef]
- Li, W.; Coop, M.R.; Senetakis, K.; Schnaid, F. The Mechanics of a Silt-Sized Gold Tailing. Eng. Geol. 2018, 241, 97–108. [Google Scholar] [CrossRef]
- De Azevedo, A.R.G.; Marvila, M.T.; De Oliveira, M.A.B.; Umbuzeiro, C.E.M.; Huaman, N.R.C.; Monteiro, S.N. Perspectives for the Application of Bauxite Wastes in the Development of Alternative Building Materials. J. Mater. Res. Technol. 2022, 20, 3114–3125. [Google Scholar] [CrossRef]
- Nascimento, R.S.; Corrêa, J.A.M.; Figueira, B.A.M.; Pinheiro, P.A.; Silva, J.H.; Freire, P.T.C.; Quaranta, S. From Mining Waste to Environmetal Remediation: A Nanoadsorbent from Amazon Bauxite Tailings for the Removal of Erythrosine B Dye. Appl. Clay Sci. 2022, 222, 106482. [Google Scholar] [CrossRef]
- Ou, X.; Chen, S.; Jiang, J.; Qin, J.; Zhang, L. Reuse of Red Mud and Bauxite Tailings Mud as Subgrade Materials from the Perspective of Mechanical Properties. Materials 2022, 15, 1123. [Google Scholar] [CrossRef]
- Singh, V.; Bano, S.; Chauhan, V.B.; Pal, P.; Kumar, A.; Srivastava, J.B. Red Mud as a Sustainable Road Construction Material: An Experimental Investigation. Constr. Build. Mater. 2024, 411, 134549. [Google Scholar] [CrossRef]
- Bruschi, G.J.; dos Santos, C.P.; Tonini de Araújo, M.; Ferrazzo, S.T.; Marques, S.F.V.; Consoli, N.C. Green Stabilization of Bauxite Tailings: Mechanical Study on Alkali-Activated Materials. J. Mater. Civ. Eng. 2021, 33, 06021007. [Google Scholar] [CrossRef]
- Bruschi, G.J.; Dos Santos, C.P.; Levandoski, W.M.K.; Ferrazzo, S.T.; Korf, E.P.; Saldanha, R.B.; Consoli, N.C. Leaching Assessment of Cemented Bauxite Tailings through Wetting and Drying Cycles of Durability Test. Environ. Sci. Pollut. Res. 2022, 29, 59247–59262. [Google Scholar] [CrossRef] [PubMed]
- Atkinson, J. An Introduction to the Mechanics of Soils and Foundations: Through Critical State Soil Mechanics; McGraw-Hill International Series in Civil Engineering; McGraw-Hill Book Co.: London, UK; New York, NY, USA, 1993; ISBN 978-0-07-707713-6. [Google Scholar]
- Atkinson, J.H.; Bransby, P.L. The Mechanics of Soils: An Introduction to Critical State Soil Mechanics; Indo American Books: Delhi, India, 2012; ISBN 978-93-82661-06-1. [Google Scholar]
- ASTM D854; ASTM Test Methods for Specific Gravity of Soil Solids by Water Pycnometer. ASTM International: West Conshohocken, PA, USA, 2014.
- ASTM D7928; ASTM Test Method for Particle-Size Distribution (Gradation) of Fine-Grained Soils Using the Sedimentation (Hydrometer) Analysis. ASTM International: West Conshohocken, PA, USA, 2021.
- ASTM D4318; ASTM Test Methods for Liquid Limit, Plastic Limit, and Plasticity Index of Soils. ASTM International: West Conshohocken, PA, USA, 2017.
- ASTM D2487; ASTM Practice for Classification of Soils for Engineering Purposes (Unified Soil Classification System). ASTM International: West Conshohocken, PA, USA, 2017.
- De Villiers, J.P.R.; Lu, L. XRD Analysis and Evaluation of Iron Ores and Sinters. In Iron Ore; Elsevier: Amsterdam, The Netherlands, 2015; pp. 85–100. ISBN 978-1-78242-156-6. [Google Scholar]
- Buhrke, V.E.; Jenkins, R.; Smith, D.K. (Eds.) A Practical Guide for the Preparation of Specimens for X-ray Fluorescence and X-ray Diffraction Analysis; Wiley-VCH: New York, NY, USA, 1999; ISBN 978-0-471-19458-3. [Google Scholar]
- ASTM D2435; ASTM Test Methods for One-Dimensional Consolidation Properties of Soils Using Incremental Loading. ASTM International: West Conshohocken, PA, USA, 2011.
- ASTM D7181; ASTM Test Method for Consolidated Drained Triaxial Compression Test for Soils. ASTM International: West Conshohocken, PA, USA, 2020.
- ASTM D4767; ASTM Test Method for Consolidated Undrained Triaxial Compression Test for Cohesive Soils. ASTM International: West Conshohocken, PA, USA, 2020.
- David Suits, L.; Sheahan, T.; Frost, J.; Park, J.-Y. A Critical Assessment of the Moist Tamping Technique. Geotech. Test. J. 2003, 26, 9850. [Google Scholar] [CrossRef]
- Clayton, C.R.I.; Khatrush, S.A. A New Device for Measuring Local Axial Strains on Triaxial Specimens. Géotechnique 1986, 36, 593–597. [Google Scholar] [CrossRef]
- Hong, Z.-S.; Yin, J.; Cui, Y.-J. Compression Behaviour of Reconstituted Soils at High Initial Water Contents. Géotechnique 2010, 60, 691–700. [Google Scholar] [CrossRef]
- Carrion, M.A.; Coop, M.R.; Nocilla, A. Assessment of the Effects of the Structure on the Compression Behaviour of a Young Alluvial Silty Soil. Soils Found. 2019, 59, 1024–1036. [Google Scholar] [CrossRef]
- Rotta, G.V.; Consoli, N.C.; Prietto, P.D.M.; Coop, M.R.; Graham, J. Isotropic Yielding in an Artificially Cemented Soil Cured under Stress. Géotechnique 2003, 53, 493–501. [Google Scholar] [CrossRef]
- Zhang, F.; Jiang, M. Do the Normal Compression Lines of Cemented and Uncemented Geomaterials Run Parallel or Converge to Each Other after Yielding? Eur. J. Environ. Civ. Eng. 2021, 25, 368–386. [Google Scholar] [CrossRef]
- Leroueil, S.; Vaughan, P.R. The General and Congruent Effects of Structure in Natural Soils and Weak Rocks. Géotechnique 1990, 40, 467–488. [Google Scholar] [CrossRef]
- Cuccovillo, T.; Coop, M.R. Yielding and Pre-Failure Deformation of Structured Sands. Géotechnique 1997, 47, 491–508. [Google Scholar] [CrossRef]
- Bandini, V.; Coop, M.R. The Influence of Particle Breakage on the Location of the Critical State Line of Sands. Soils Found. 2011, 51, 591–600. [Google Scholar] [CrossRef]
- Ghafghazi, M.; Shuttle, D.A.; DeJong, J.T. Particle Breakage and the Critical State of Sand. Soils Found. 2014, 54, 451–461. [Google Scholar] [CrossRef]
- Tong, C.-X.; Zhai, M.-Y.; Li, H.-C.; Zhang, S.; Sheng, D. Particle Breakage of Granular Soils: Changing Critical State Line and Constitutive Modelling. Acta Geotech. 2022, 17, 755–768. [Google Scholar] [CrossRef]
- Jefferies, M.; Been, K. Soil Liquefaction: A Critical State Approach, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2015; ISBN 978-0-429-15391-4. [Google Scholar]
- Schnaid, F.; Prietto, P.D.M.; Consoli, N.C. Characterization of Cemented Sand in Triaxial Compression. J. Geotech. Geoenviron. Eng. 2001, 127, 857–868. [Google Scholar] [CrossRef]
- Lade, P.V.; Overton, D.D. Cementation Effects in Frictional Materials. J. Geotech. Engrg. 1989, 115, 1373–1387. [Google Scholar] [CrossRef]
- Lade, P.V.; Trads, N. The Role of Cementation in the Behaviour of Cemented Soils. Geotech. Res. 2014, 1, 111–132. [Google Scholar] [CrossRef]
- Nakata, A.F.L.; Hyde, M.; Hyodo, H.; Murata. A Probabilistic Approach to Sand Particle Crushing in the Triaxial Test. Géotechnique 1999, 49, 567–583. [Google Scholar] [CrossRef]

| Physical Properties | Mean Value | Test Method |
|---|---|---|
| Specific gravity 1 | 3.01 | ASTM D854 |
| Plastic limit—PL (%) | 23 | ASTM D4318 |
| Liquid limit—LL (%) | 32 | |
| Plastic index—PI (%) | 9 | |
| Coarse Sand 1 (2.00 mm < diameter < 4.75 mm) (%) | 0 | ASTM D7928 |
| Medium Sand 1 (0.425 mm < diameter < 2.00 mm) (%) | 0 | |
| Fine Sand 1 (0.075 mm < diameter < 0.425 mm) (%) | 9 | |
| Silt 1 (0.002 < diameter < 0.075 mm) (%) | 73 | |
| Clay 1 (diameter < 0.002 mm) (%) | 18 |
| Tests | Shear Condition | Sample | Shelby | p’0 | |
|---|---|---|---|---|---|
| 1 | Triaxial Test | CIU | R | SH #1 | 25 kPa |
| 2 | U | ||||
| 3 | U | SH #8 | 50 kPa | ||
| 4 | R | 100 kPa | |||
| 5 | U | ||||
| 6 | U | 400 kPa | |||
| 7 | R | SH #5 | |||
| 8 | R | SH #8 | 1000 kPa | ||
| 9 | U | SH #4 | |||
| 10 | R | SH #6 | 2000 kPa | ||
| 11 | U | ||||
| 12 | R | SH #2 | 4000 kPa | ||
| 13 | U | ||||
| 14 | CID | U | SH #4 | 1000 kPa | |
| 15 | U | SH #6 | 2000 kPa | ||
| 16 | U | SH #2 | 4000 kPa | ||
| 1 | One-Dimensional Compression | U | SH #1 | 4000 kPa | |
| 2 | R | SH #8 | 4000 kPa | ||
| P’0 (kPa) | Shearing | Sample | Bf |
|---|---|---|---|
| 2000 | U | REM | 0.02 |
| 2000 | U | UND | 0.07 |
| 2000 | D | UND | 0.05 |
| 4000 | U | UND | 0.03 |
| 4000 | D | UND | 0.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gonçalves, R.R.S.M.; de Rezende Dutra, M.; Hoch, B.Z.; Scheuermann Filho, H.C.; Schnaid, F.; Festugato, L. On the Behavior of Bauxite Tailings under a Wide Range of Stresses. Mining 2024, 4, 629-641. https://doi.org/10.3390/mining4030035
Gonçalves RRSM, de Rezende Dutra M, Hoch BZ, Scheuermann Filho HC, Schnaid F, Festugato L. On the Behavior of Bauxite Tailings under a Wide Range of Stresses. Mining. 2024; 4(3):629-641. https://doi.org/10.3390/mining4030035
Chicago/Turabian StyleGonçalves, Rosanne Rodrigues Santos Maciel, Matheus de Rezende Dutra, Bruna Zakharia Hoch, Hugo Carlos Scheuermann Filho, Fernando Schnaid, and Lucas Festugato. 2024. "On the Behavior of Bauxite Tailings under a Wide Range of Stresses" Mining 4, no. 3: 629-641. https://doi.org/10.3390/mining4030035
APA StyleGonçalves, R. R. S. M., de Rezende Dutra, M., Hoch, B. Z., Scheuermann Filho, H. C., Schnaid, F., & Festugato, L. (2024). On the Behavior of Bauxite Tailings under a Wide Range of Stresses. Mining, 4(3), 629-641. https://doi.org/10.3390/mining4030035




