Evaluation of Flow Liquefaction Susceptibility in Non-Plastic Silty Soils Using the Seismic Cone
Abstract
1. Introduction
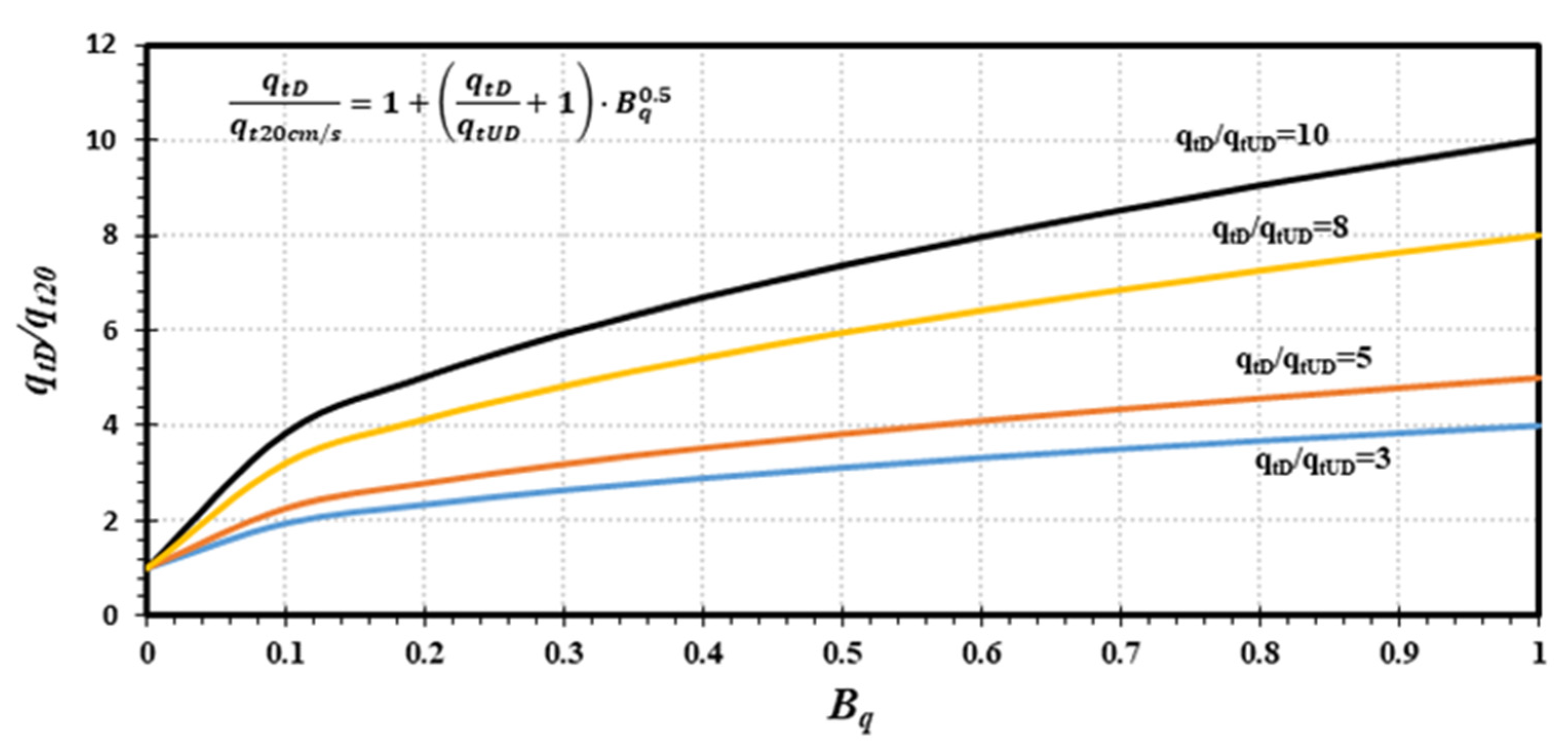
2. Drainage Conditions on CPTu Tests
3. Classification and Flow Liquefaction Evaluation for Non-Plastic Silty Soils Based on Seismic Cone Tests
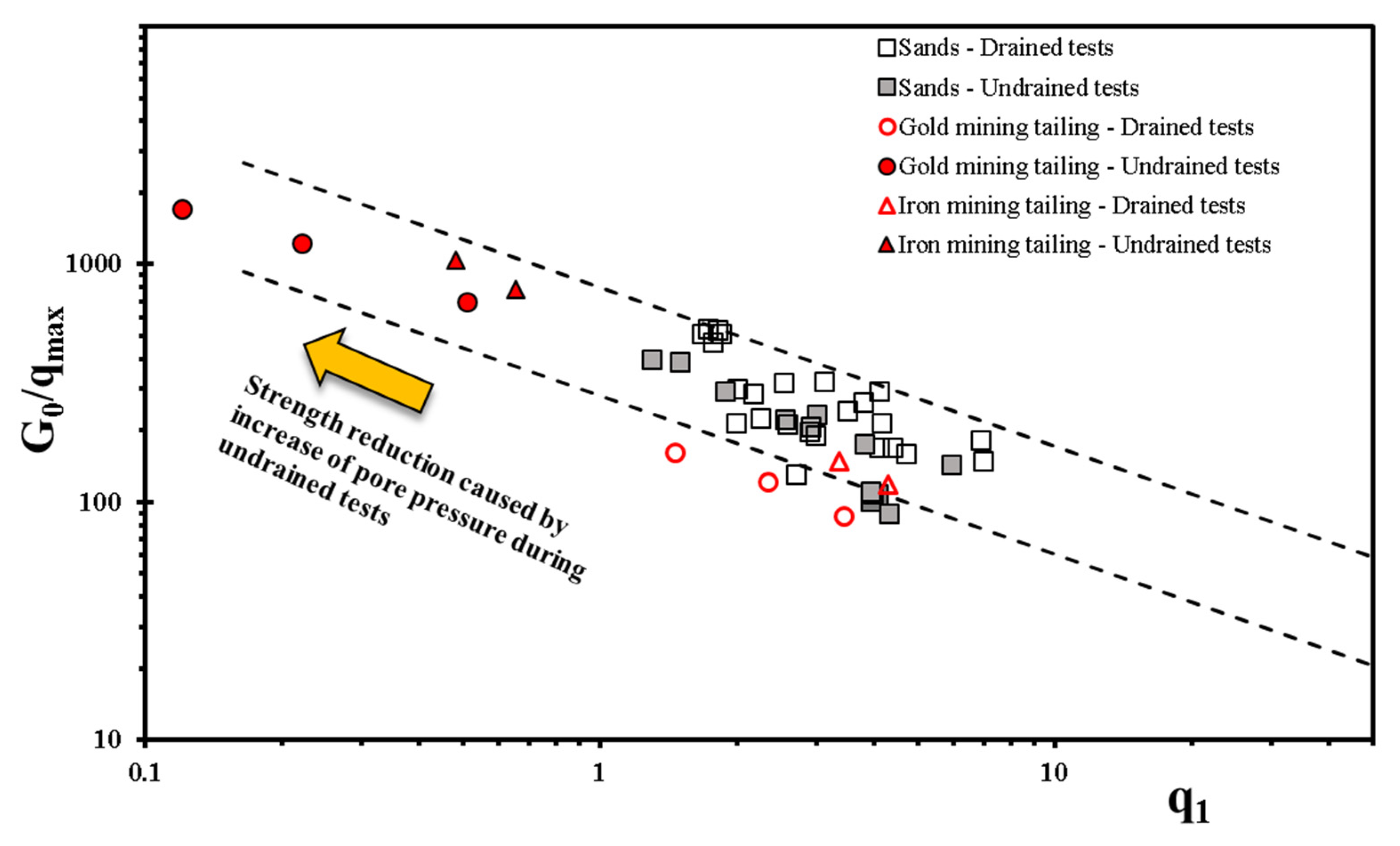
3.1. Laboratory Test Results Analysis
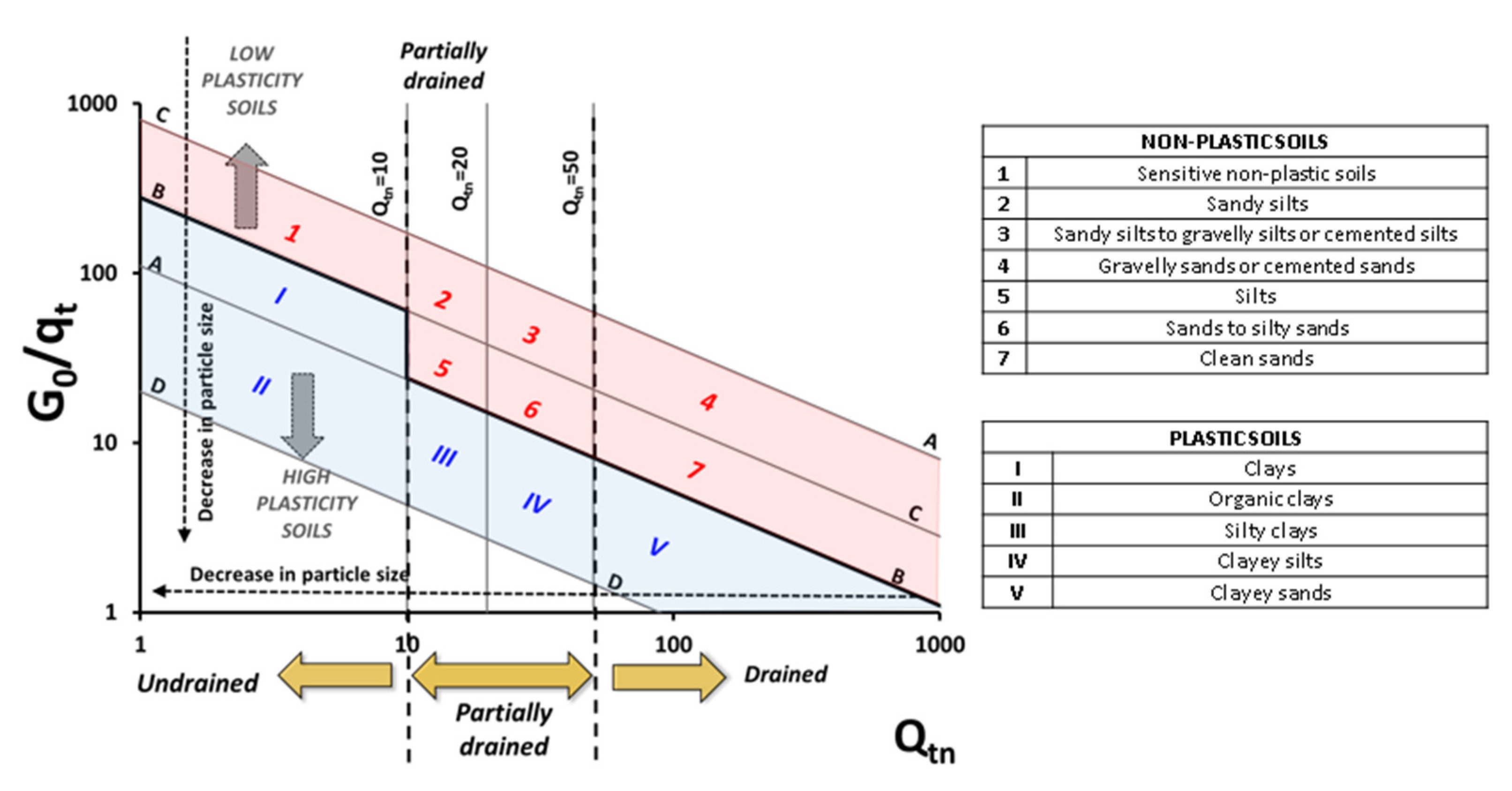
3.2. Developed Expansion on the Classification System
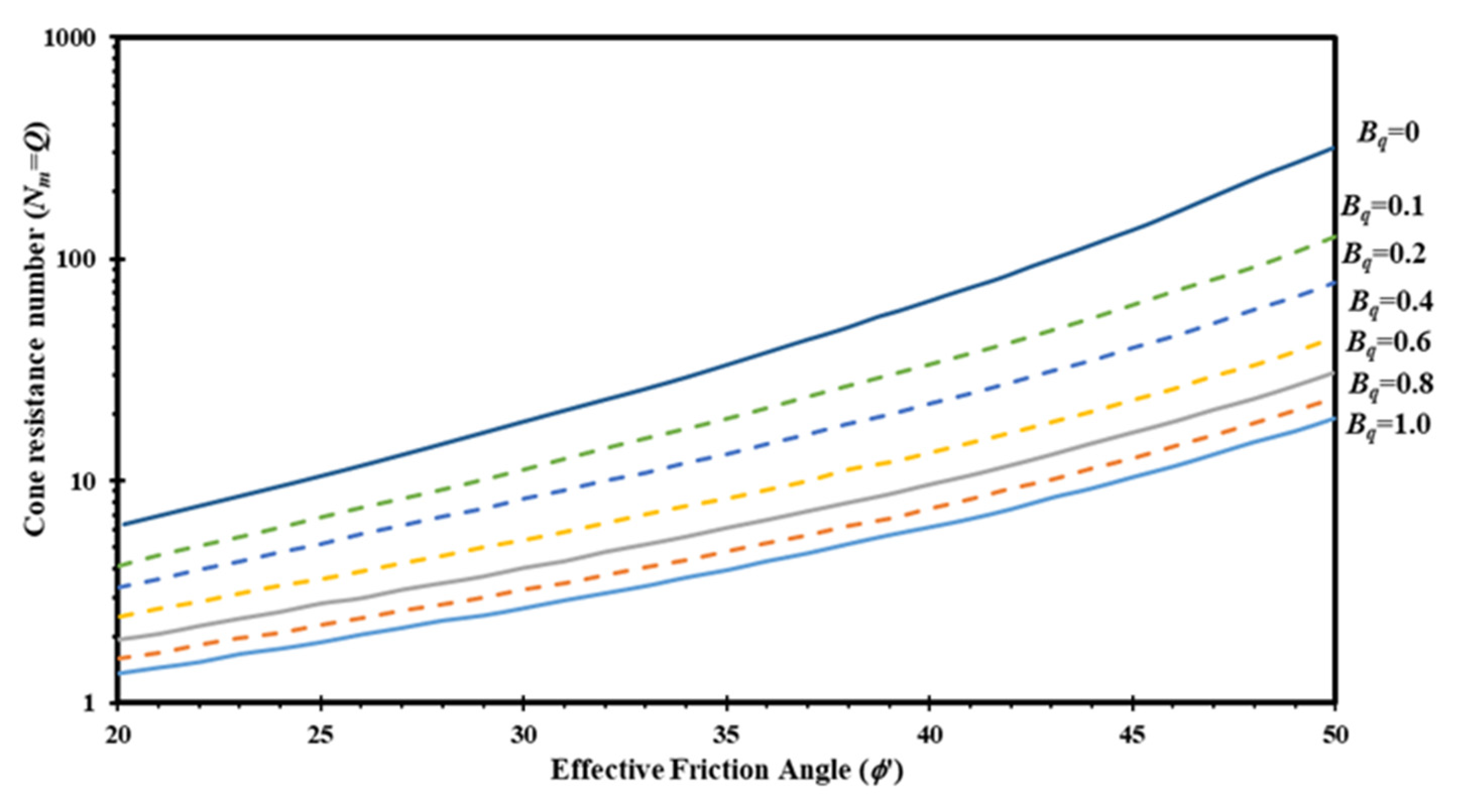
3.3. State Parameter Assessment for Non-Plastic Silty Soils and Flow Liquefaction Evaluation
3.4. Validation and Calibration
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nierwinski, H.P.; Schnaid, F.; Odebrecht, E. In-situ state parameter assessment of non-plastic silty soils using the seismic cone. In Proceedings of the 6th International Conference on Geotechnical and Geophysical Site Characterization, Online, 26–29 September 2021. [Google Scholar]
- Robertson, P.K.; Fear, C.E. Liquefaction of sands and its evaluation. In Proceedings of the IS-Tokyo ’95: The First International Conference on Earthquake Geotechnical Engineering, Tokyo, Japan, 14–16 November 1995; Ishihara, K., Ed.; Balkema: Rotterdam, The Netherlands, 1995; pp. 1253–1289. [Google Scholar]
- Seed, H.B. Soil liquefaction and cyclic mobility evaluation for level ground during earthquakes. J. Geotech. Eng. Div. ASCE 1979, 105, 201–255. [Google Scholar] [CrossRef]
- Castro, G. Liquefaction and cyclic mobility of saturated sands. J. Geotech. Eng. Div. ASCE 1975, 101, 551–569. [Google Scholar] [CrossRef]
- Finn, W.D.L. Liquefaction potential: Developments since 1976. In Proceedings of the 1st International Conference on Recent Advances in Geotechnical Earthquake Engineering and Soil Dynamics, St. Louis, MO, USA, 28 April 1981; Prakash, S., Ed.; University of Missouri-Rolla: Rolla, MO, USA, May 1981; Volume 2, pp. 655–681. [Google Scholar]
- Ishihara, K. Liquefaction and flow failure during earthquakes. Géotechnique 1993, 43, 351–415. [Google Scholar] [CrossRef]
- Seed, H.B.; Idriss, I.M. Simplified procedure for evaluating soil liquefaction potential. J. Geotech. Eng. ASCE 1971, 97, 1249–1273. [Google Scholar] [CrossRef]
- Robertson, P.K.; Wride, C.E. Evaluating cyclic liquefaction potential using the CPT. Can. Geotech. J. 1998, 35, 442–459. [Google Scholar] [CrossRef]
- Davies, M.P.; McRoberts, E.C.; Martin, T.E. Static Liquefaction of Tailings–Fundamentals and Case Histories. In Proceedings Tailings Dams ASDSO/USCOLD; Association of State Dam Safett Official: Las Vegas, NV, USA, 2002. [Google Scholar]
- Jefferies, M.G.; Been, K. Soil Liquefaction: A Critical State Approach; CRC Press, Taylor & Francis: Boca Raton, FL, USA, 2016; 690p, ISBN 978-1-4822-1368-3. [Google Scholar]
- Morgenstern, N.R.; Vick, S.G.; Viotti, C.B.; Watts, B.D. Fundão Tailings Dam Review Panel. Report on the Immediate Causes of the Failure of the Fundão Dam. August 2016. Available online: https://www.resolutionmineeis.us/documents/fundao-2016 (accessed on 15 April 2022).
- Robertson, P.K. Evaluation of flow liquefaction and liquefied strength using the Cone Penetration Test. J. Geotech. Geoenviron. Eng. 2010, 136, 842–853. [Google Scholar] [CrossRef]
- Yang, J.; Liang, L.B.; Chen, Y. Instability and liquefaction flow slide of granular soils: The role of initial shear stress. Acta Geotech. 2022, 17, 65–79. [Google Scholar] [CrossRef]
- Zhu, Z.; Cheng, W. Parameter Evaluation of Exponential-Form Critical State Line of a State-Dependent Sand Constitutive Model. Appl. Sci. 2020, 10, 328. [Google Scholar] [CrossRef]
- Been, K.; Jefferies, M.G. A state parameter for sands. Géotechnique 1985, 35, 99–112. [Google Scholar] [CrossRef]
- Shuttle, D.A.; Cunning, J. Liquefaction potential of silts from CPTu. Can. Geotech. J. 2007, 44, 1–19. [Google Scholar] [CrossRef]
- Ledesma, O.; Manzanal, D.; Sfriso, A. Formulation and numerical implementation of a state parameter-based generalized plasticity model for mine tailings. Comput. Geotech. 2021, 135, 104158. [Google Scholar] [CrossRef]
- Robertson, P.K. Estimating in situ state parameter and friction angle in sandy soils from CPT. In Proceedings of the 2nd International Symposium on Cone Penetration Testing, Huntington Beach, CA, USA, 9–11 May 2010. [Google Scholar]
- Been, K.; Crooks, J.H.A.; Becker, D.E.; Jefferies, M.G. The cone penetration test in sands: Part I, State parameter interpretation. Géotechnique 1986, 36, 239–249. [Google Scholar] [CrossRef]
- Schnaid, F.; Yu, H.S. Interpretation of the seismic cone test in granular soils. Géotechnique 2007, 57, 265–272. [Google Scholar] [CrossRef]
- Yu, H.; Schnaid, F.; Collins, I. Analysis of Cone Pressuremeter Tests in Sands. J. Geotech. Eng. 1996, 122, 623–632. [Google Scholar] [CrossRef]
- Schnaid, F. Geocharacterisation and properties of natural soils by in situ tests. In Proceedings of the International Conference on Soil Mechanics and Geotechnical Engineering; AA Balkema Publishers: Rotterdam, The Netherlands, 2005; Volume 16, p. 3. [Google Scholar]
- Schneider, J.A.; Moss, R.E.S. Linking cyclic stress and cyclic strain based methods for assessment of cyclic liquefaction triggering in sands. Géotechnique Lett. 2011, 1, 31–36. [Google Scholar] [CrossRef]
- Robertson, P.K. Cone Penetration Test (CPT)-Based Soil Behaviour Type (SBT) Classification System–An Update. Can. Geotech. J. 2016, 53, 1910–1927. [Google Scholar] [CrossRef]
- Schnaid, F.; Nierwinski, H.P.; Odebrecht, E. Classification and state parameter assessment of granular soils using the seismic cone. J. Geotech. Geoenviron. Eng. ASCE 2020, 146, 06020009. [Google Scholar] [CrossRef]
- Holmsgaard, R.; Nielsen, B.N.; Ibsen, L.B. Interpretation of Cone Penetration Testing in Silty Soils Conducted under Partially Drained Conditions. J. Geotech. Geoenviron. Eng. 2015, 14, 204015064. [Google Scholar] [CrossRef]
- Dienstmann, G.; Schnaid, F.; Maghous, S.; Dejong, J. Piezocone Penetration Rate Effects in Transient Gold Tailings. J. Geotech. Geoenviron. Eng. 2018, 144, 04017116. [Google Scholar] [CrossRef]
- DeJong, J.T.; Randolph, M.F. Influence of partial consolidation during cone penetration on estimated soil behavior type and pore pressure dissipation measurements. J. Geotech. Geoenviron. Eng. 2012, 138, 777–788. [Google Scholar] [CrossRef]
- Randolph, M.F.; Hope, S.N. Effect of cone velocity on cone resistance and excess pore pressure. In Proceedings of the IS Osaka–Engineering Practice and Performance of Soft Deposits; Yodogawa Kogisha Co. Ltd.: Osaka, Japan, 2004; pp. 147–152. [Google Scholar]
- Chung, S.F.; Randolph, M.F.; Schneider, J.A. Effect of penetration rate on penetrometer resistance in clay. J. Geotech. Geoenviron. Eng. 2006, 132, 1188–1196. [Google Scholar] [CrossRef]
- Kim, K.; Prezzi, M.; Salgado, R.; Lee, W. Effect of penetration rate on cone penetration resistance in saturated clayey soils. J. Geotech. Geoenviron. Eng. 2008, 134, 1142–1153. [Google Scholar] [CrossRef]
- Paniagua, P.; Carroll, R.; L’Heureux, J.-S.; Nordal, S. Monotonic and Dilatory Excess Pore Water Dissipations in Silt Following CPTU at Variable Penetration Rate. In Proceedings of the 5th Intern. Conf. on Geotechn. and Geophys. Site Characterisation, ISC’5, Queensland, Australia, 5–10 September 2016; pp. 509–514. [Google Scholar]
- Suzuki, Y.; Lehane, B.M. Rate dependence of qc in two clayey sands. In Proceedings of the 3rd International Symposium on Cone Penetration Testing, Madison, WI, USA, 13–14 May 2014; Volume 1. [Google Scholar]
- Schnaid, F. In Situ Testing in Geomechanics: The Main Tests; Taylor e Francis: London, UK, 2009; 329p. [Google Scholar]
- Hight, D.W.; Georgiannou, V.N.; Ford, C.J. Characterization of clayey sand. In Proceedings of the 7th International Conference on Behavior of Offshore Structures, Cambridge, MA, USA, 12–15 July 1994; Volume 1, pp. 321–340. [Google Scholar]
- Santos, J.A.; Gomes, R.C.; Lourenço, J.C.; Marquer, F.; Coelho, P.; Azeiteiro, R.; Santos, L.A.; Marques, V.; Viana da Fonsceca, A.; Soares, M.; et al. Coimbra Sand Round Robin Tests to Evaluate Liquefaction Resistance. In Proceedings of the 15th World Conference on Earthquake Engineering 15 WCEE, Lisbon, Portugal, 24–28 September 2012. [Google Scholar]
- Sharma, R.; Baxter, C.; Jander, M. Relationship between shear wave velocity and stresses at failure for weakly cemented sands during drained triaxial compression. Soils Found. 2011, 51, 761–771. [Google Scholar] [CrossRef]
- Carraro, J.A.H.; Prezzi, M.; Salgado, R. Shear Strength and Stiffness of Sands Containing Plastic or Nonplastic Fines. J. Geotech. Geoenviron. Eng. 2009, 135, 1167–1178. [Google Scholar] [CrossRef]
- Salgado, R.; Bandini, P.; Karim, A. Shear strength and stiffness of silty sand. J. Geotech. Geoenviron. Eng. 2000, 126, 451–462. [Google Scholar] [CrossRef]
- Huang, Y.T.; Huang, A.B.; Kuo, Y.C.; Tsai, M.D. A laboratory study on the undrained strength of a silty sand from Central Western Taiwan. Soil Dyn. Earthq. Eng. 2004, 24, 733–743. [Google Scholar] [CrossRef]
- Nyunt, T.T.; Leong, E.C.; Rahardjo, H. Strength and Small-Strain Stiffness Characteristics of Unsaturated Sand. Geotech. Test. J. 2011, 34, 551–561. [Google Scholar]
- Prashant, A.; Bhattacharya, D.; Gundlapalli, S. Stress-state dependency of small-strain shear modulus in silty sand and sandy silt of Ganga. Géotechnique 2018, 69, 42–56. [Google Scholar] [CrossRef]
- Bedin, J. Study of the Geomechanical Behavior of Tailings. Ph.D. Thesis, Federal University of Rio Grande do Sul, Porto Alegre, Brazil, 2010. (In Portuguese). [Google Scholar]
- Zhu, Z.; Zhang, F.; Dupla, J.-C.; Canou, J.; Foerster, E. Investigation on the undrained shear strength of loose sand with added materials at various mean diameter ratios. Soil Dyn. Earthq. Eng. 2020, 137, 106276. [Google Scholar] [CrossRef]
- Senneset, K.; Sandven, R.; Janbu, N. Evaluation of Soil Parameters from Piezocone Tests; Transportation Research Record 1235; Geotechnical Division, The Norwegian Institute of Technology: Trondheim, Norway, 1989. [Google Scholar]
- Sandven, R. Strength and Deformation Properties Obtained from Piezocone Tests. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 1990; 342p. [Google Scholar]
- Lehane, B.M.; O’Loughlin, C.D.; Gaudin, C.; Randolph, M.F. Rate effects on penetrometer resistance in kaolin. Geotechnique 2009, 59, 41–52. [Google Scholar] [CrossRef]
- Ouyang, Z.; Mayne, P.W. Effective friction angle of clays and silts from piezocone penetration tests. Can. Geotech. J. 2019, 1, 1230–1247. [Google Scholar] [CrossRef]




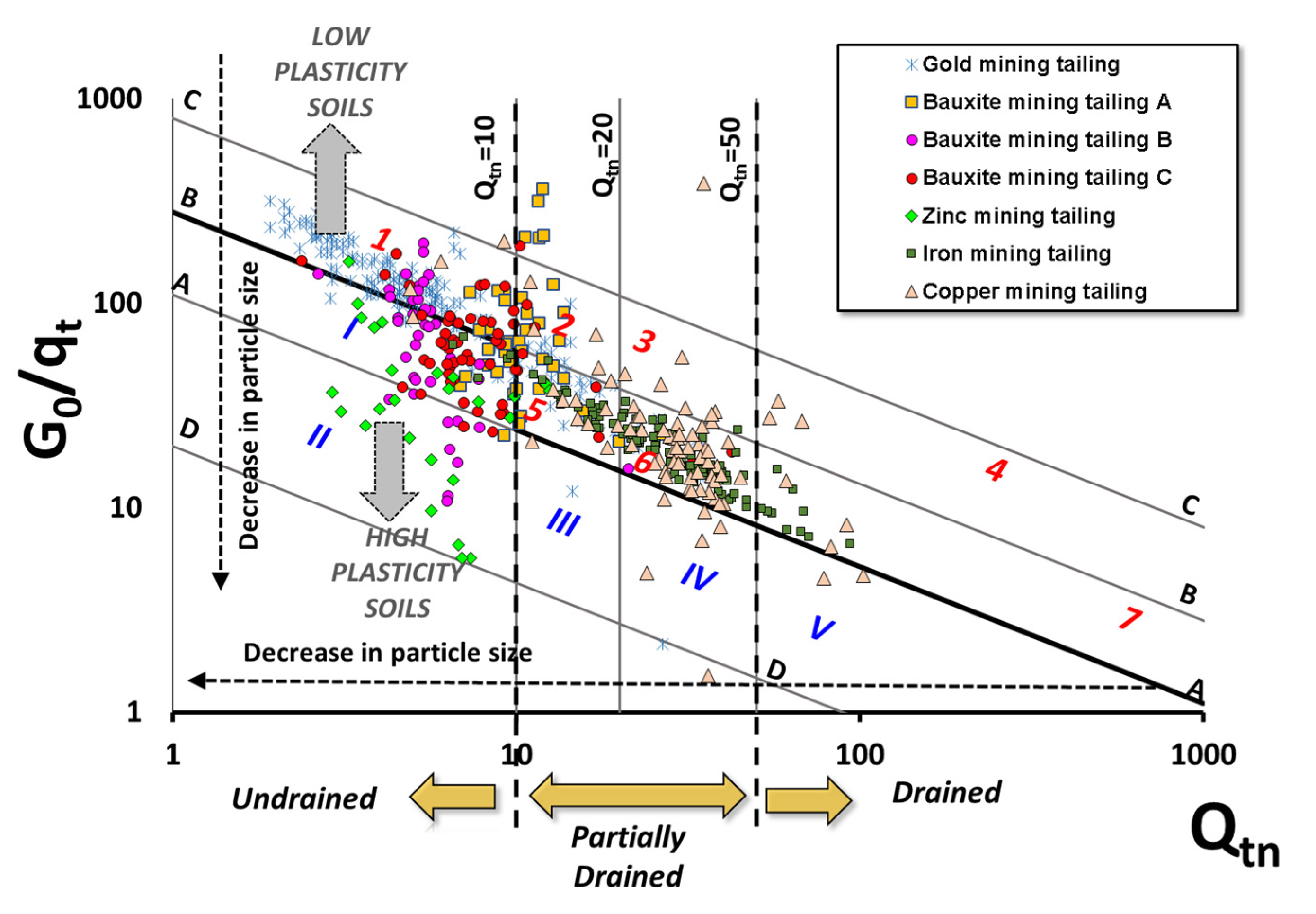
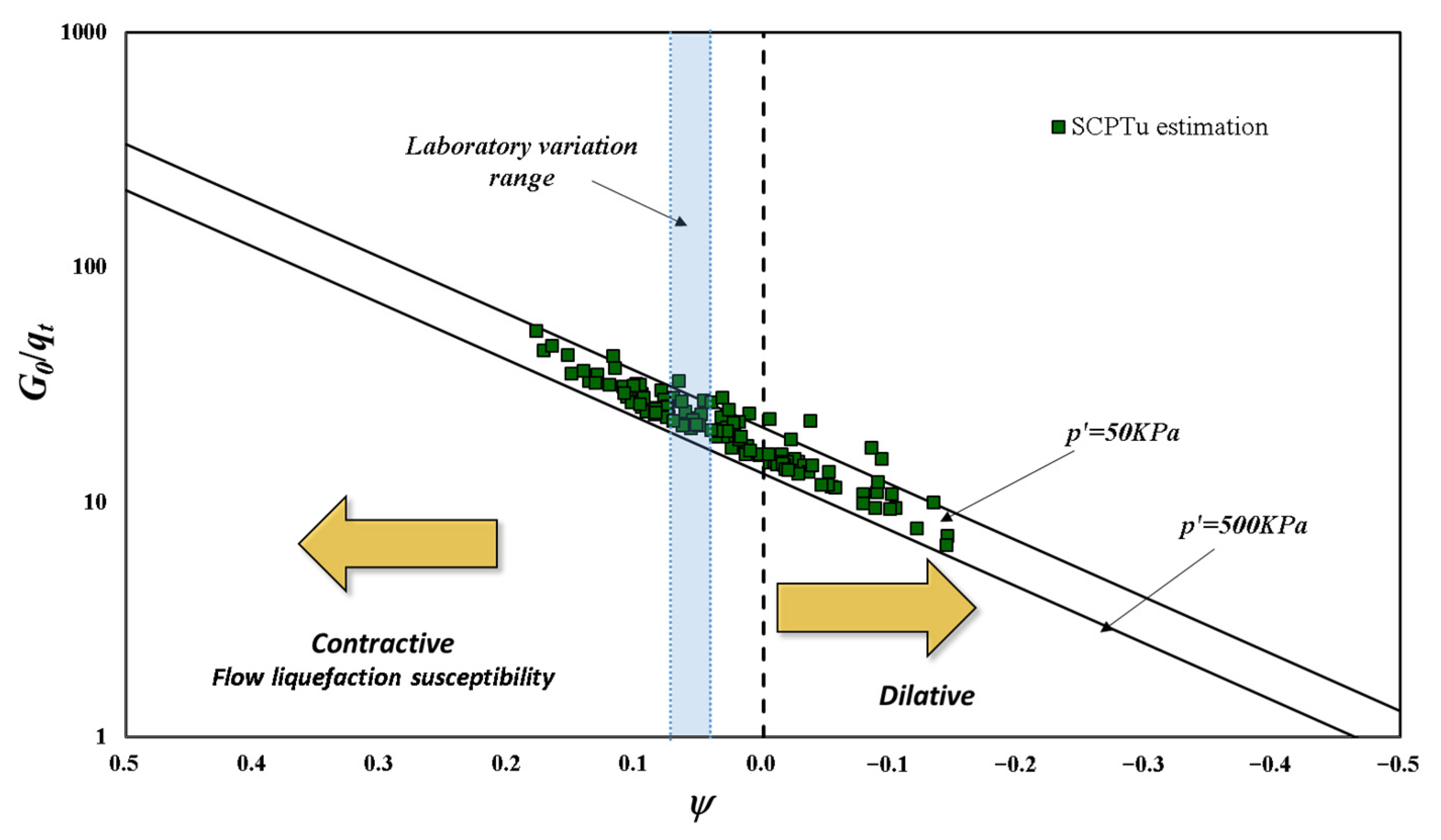
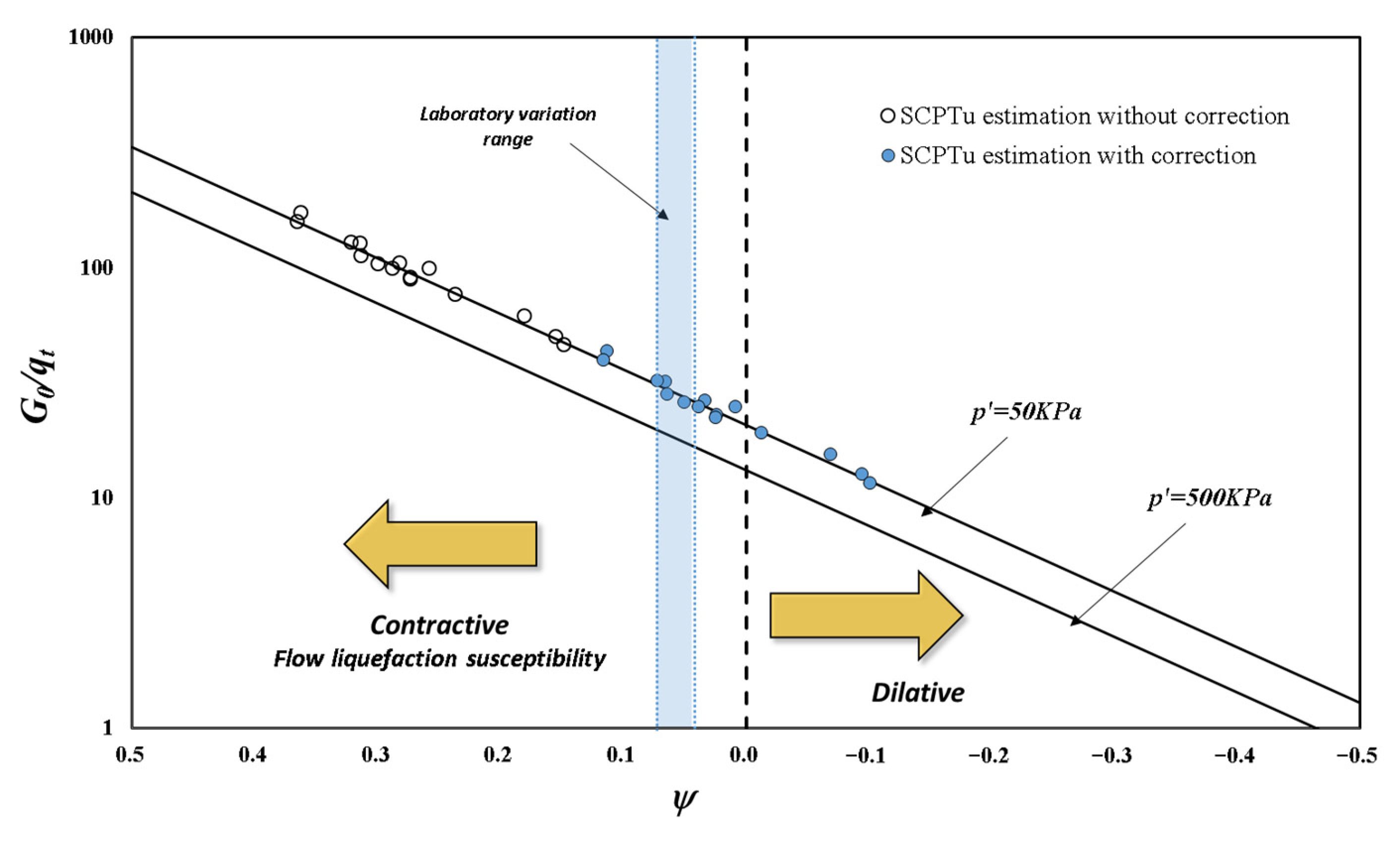
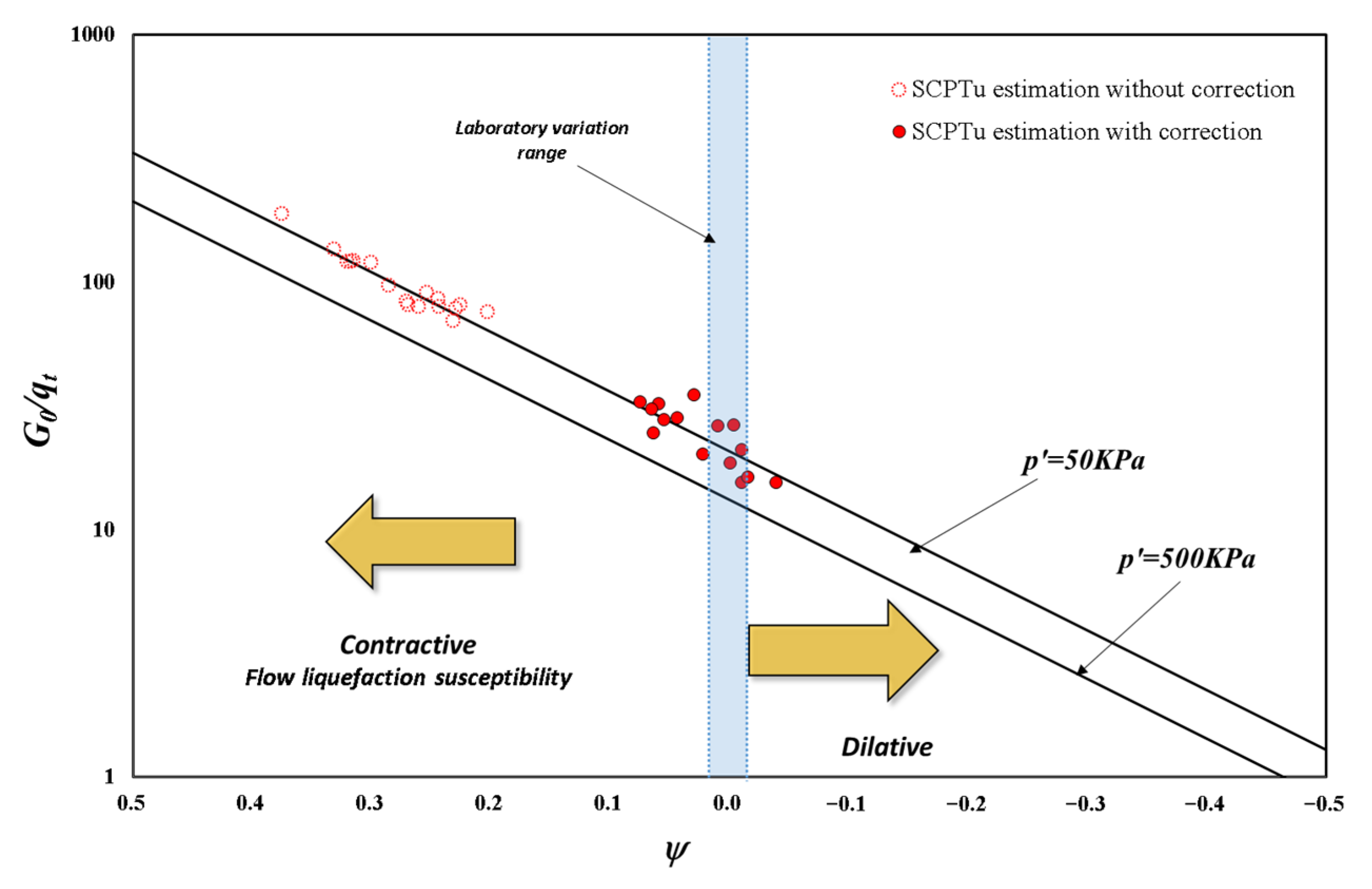
| Mining Tailing | D50 (mm) | γn (kN/m³) | wnat (%) | G | Liquid Limit (LL) | Plastc Index (IP) |
|---|---|---|---|---|---|---|
| Gold | 0.032 | 18.6–20.5 | 31–39 | 2.86–3.15 | - | NP |
| Bauxite A | 0.0023 | 15.9–20.1 | 23–89 | 2.72–3.27 | 34–53 | 1–17.3 |
| Bauxite B | 0.03 | 15.8–17.8 | 55–70 | 3.0–3.15 | 31–39 | 5–14 |
| Bauxite C | 0.003 | 17.5–18.8 | 40–47 | 3.01–3.07 | 38 | 3 |
| Zinc | 0.015 | 11.3–14.9 | 90–210 | 3.28–3.37 | 61–101 | 25–54 |
| Iron | 0.075 | 15.7–19.0 | 6–15 | 2.92–3.06 | - | NP |
| Copper | 0.075 | 14.2–16.5 | 50–63 | 2.82–2.85 | - | NP |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nierwinski, H.P.; Schnaid, F.; Odebrecht, E. Evaluation of Flow Liquefaction Susceptibility in Non-Plastic Silty Soils Using the Seismic Cone. Mining 2024, 4, 21-36. https://doi.org/10.3390/mining4010003
Nierwinski HP, Schnaid F, Odebrecht E. Evaluation of Flow Liquefaction Susceptibility in Non-Plastic Silty Soils Using the Seismic Cone. Mining. 2024; 4(1):21-36. https://doi.org/10.3390/mining4010003
Chicago/Turabian StyleNierwinski, Helena Paula, Fernando Schnaid, and Edgar Odebrecht. 2024. "Evaluation of Flow Liquefaction Susceptibility in Non-Plastic Silty Soils Using the Seismic Cone" Mining 4, no. 1: 21-36. https://doi.org/10.3390/mining4010003
APA StyleNierwinski, H. P., Schnaid, F., & Odebrecht, E. (2024). Evaluation of Flow Liquefaction Susceptibility in Non-Plastic Silty Soils Using the Seismic Cone. Mining, 4(1), 21-36. https://doi.org/10.3390/mining4010003






