Required Plug Strength for Continuously Poured Cemented Paste Backfill in Longhole Stopes
Abstract
1. Introduction
2. Predictive Model Development, Validation, and Calibration
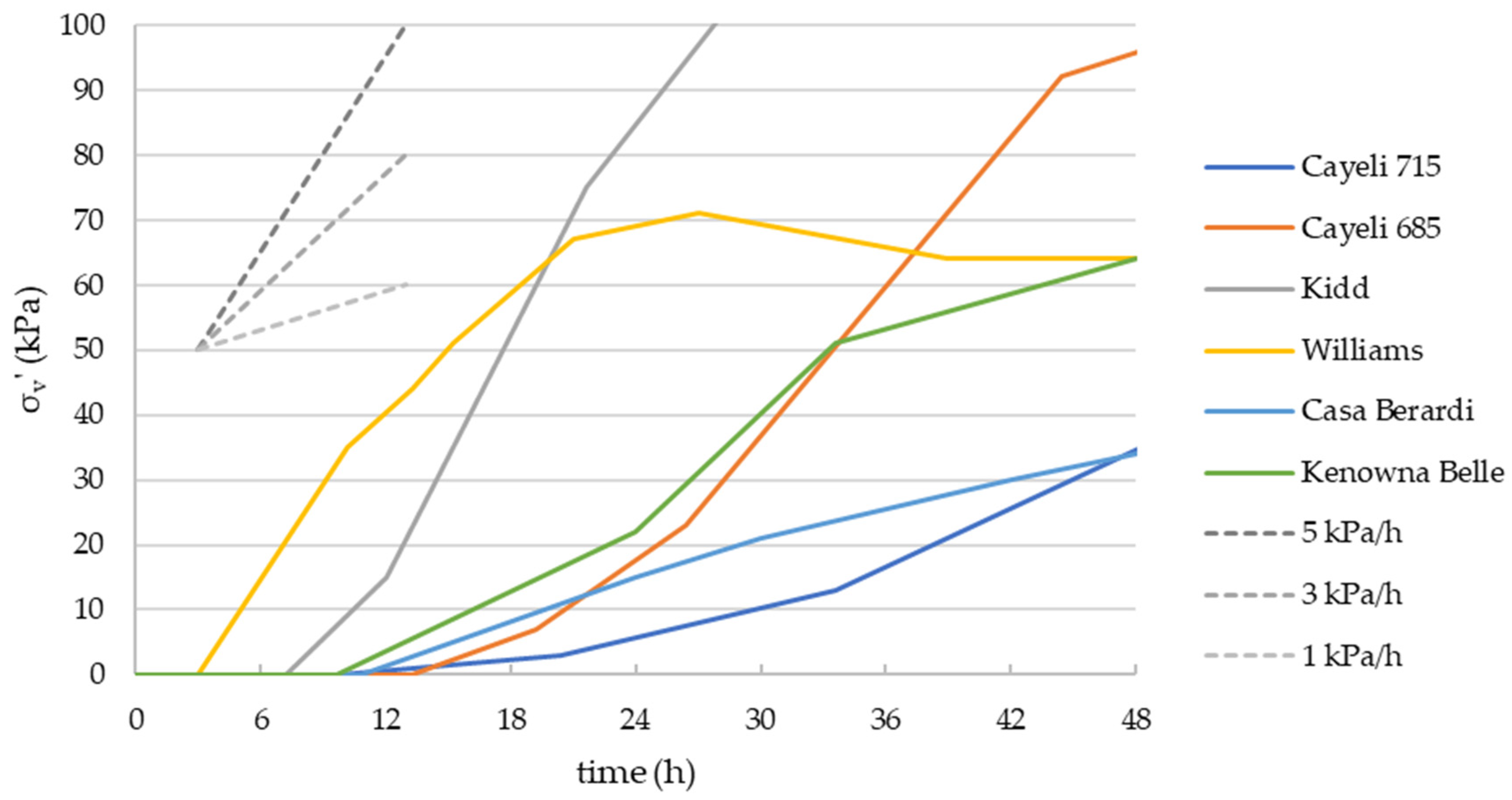
2.1. Effective Stress Development during Field Pours
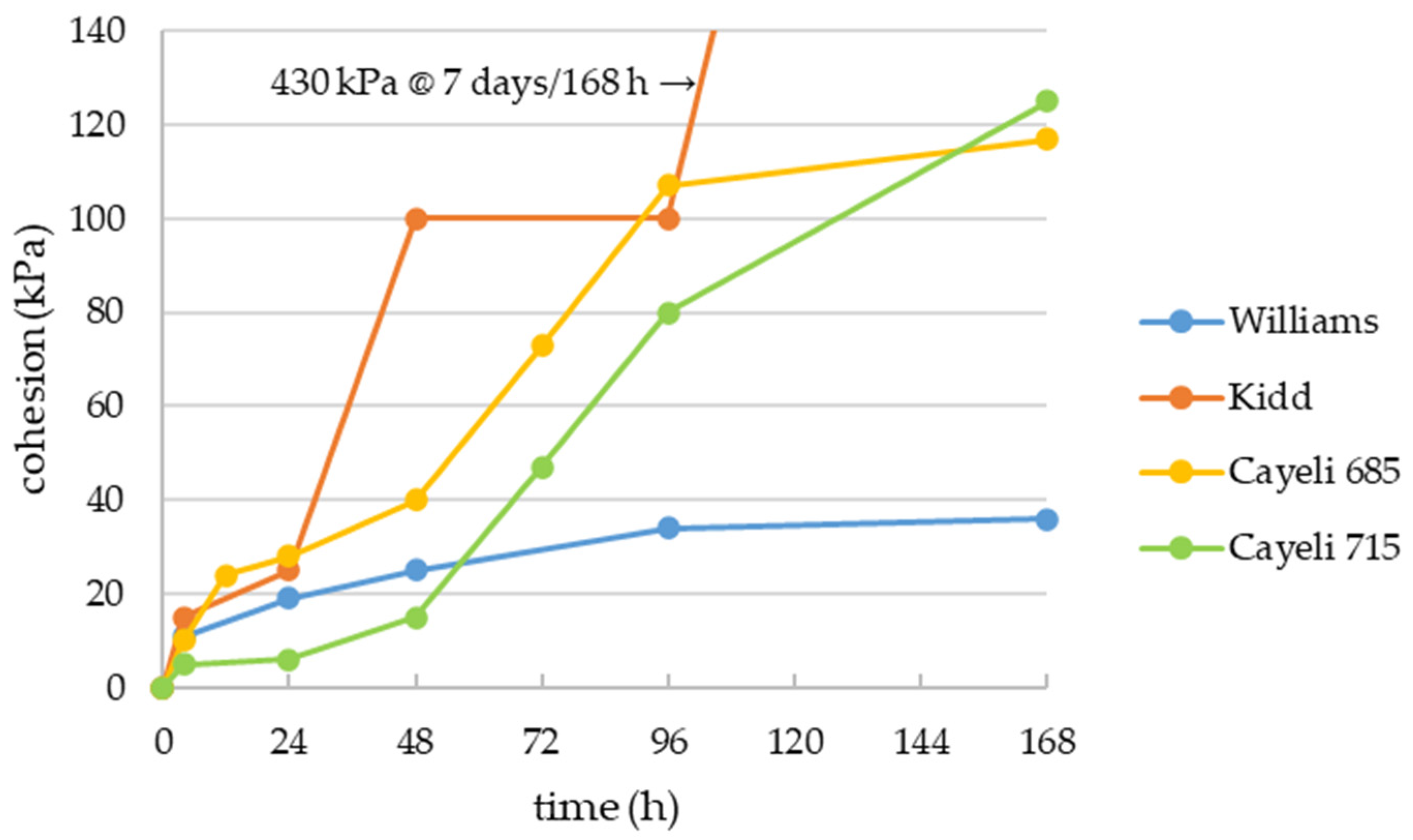
2.2. Strength Development during Field Pours
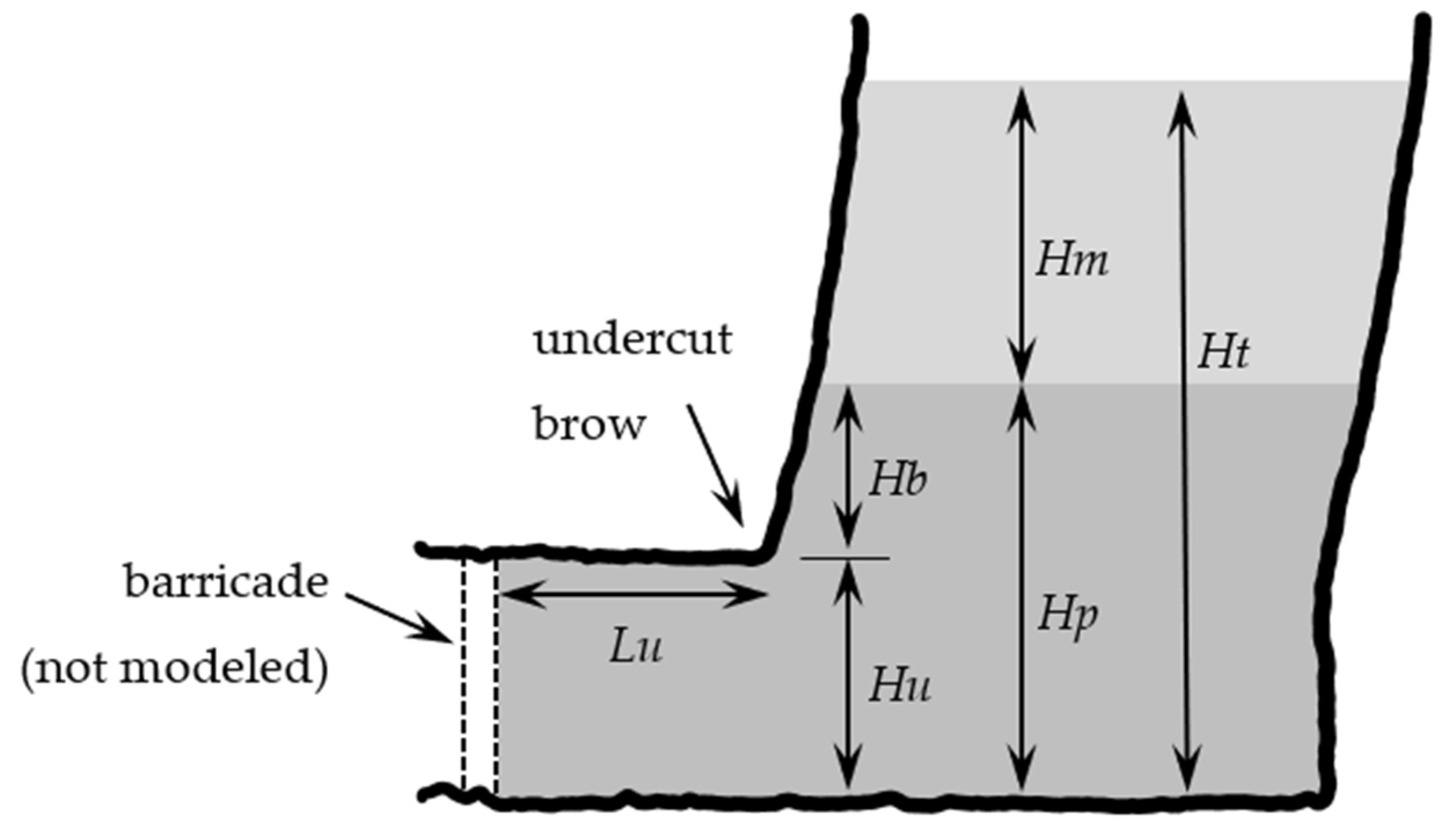
2.3. Preliminary Analytical Model for CPB Plug Strength
- The undercut has square cross section with height , and the distance between the backfill barricade and the undercut brow is ;
- The undercut walls are rough so that shear occurs through the CPB across asperities, rather than along the CPB-rock interface;
- The CPB plug has height with height above the undercut brow ;
- The height of main pour at any given time is above the plug, and the main pour exerts fluid-like pressure on the plug where is the backfill’s unit weight;
- The total backfill height at any time is ;
- The average CPB plug undrained cohesion at the end of the plug pour must be sufficient to create a “self-supporting” condition, such that the CPB plug no longer relies on the barricade for stability; and
- The average CPB plug undrained cohesion must continue to increase sufficiently during the main pour to support the surcharge and prevent failure through the CPB plug which would otherwise then exert further pressure on the barricade and potentially fail it.
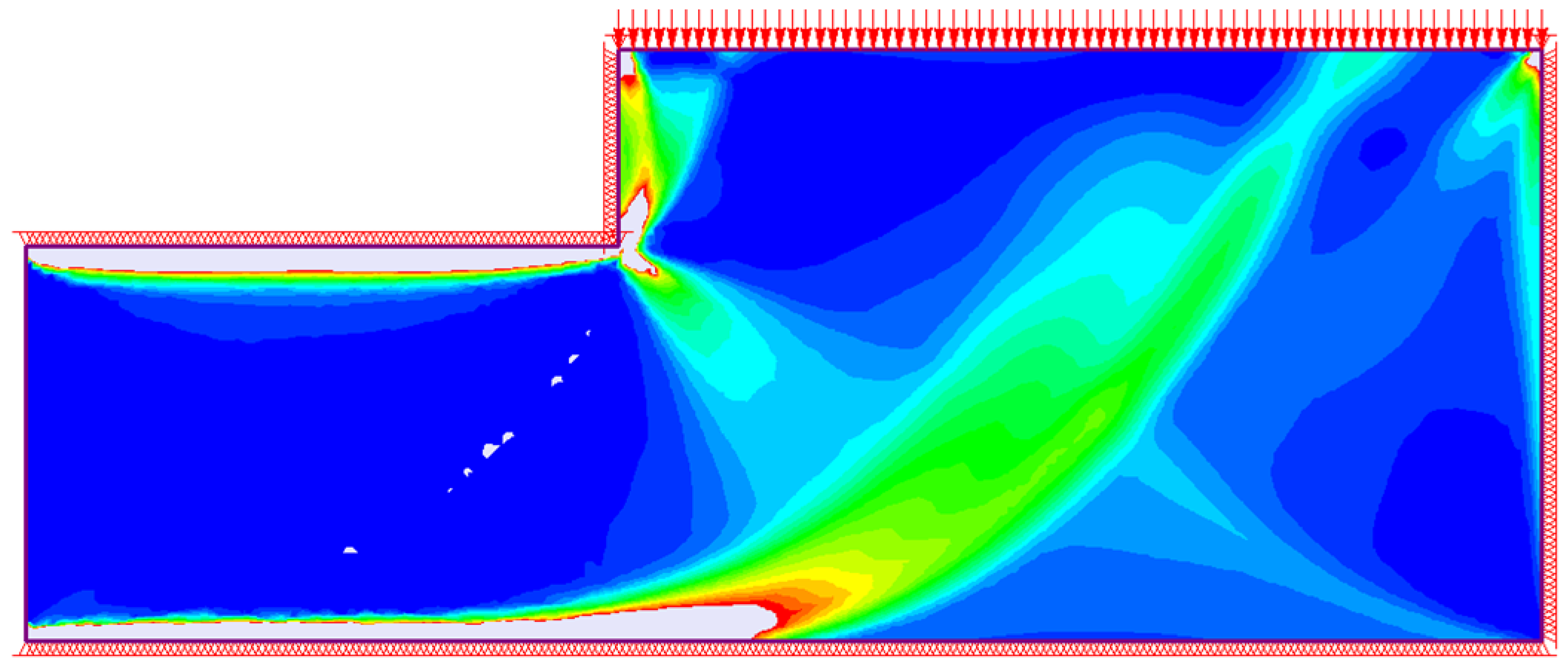
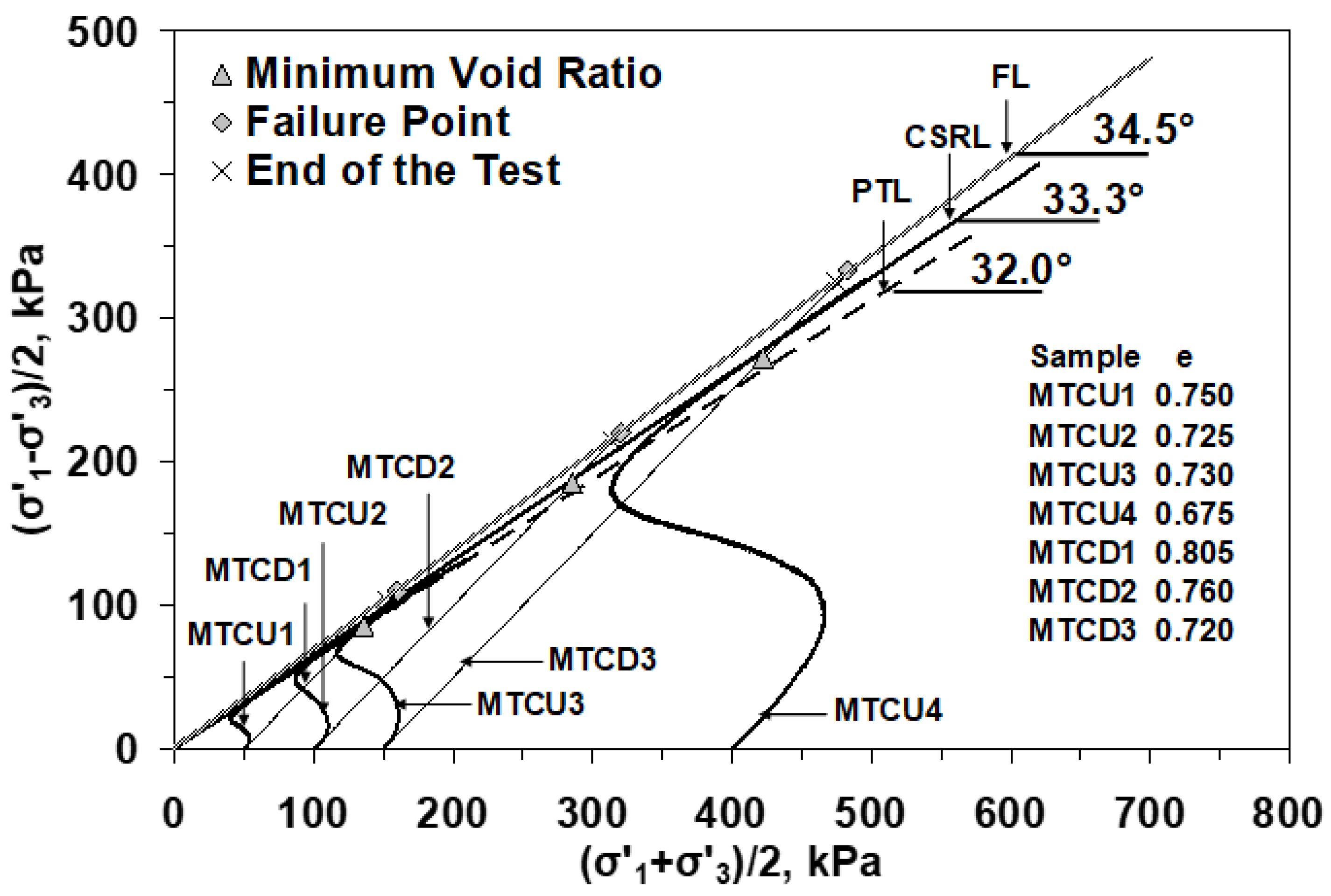
2.4. Numerical Validation/Calibration
2.5. Non-Uniform Strength Effects
2.6. Recommended Analysis Procedure
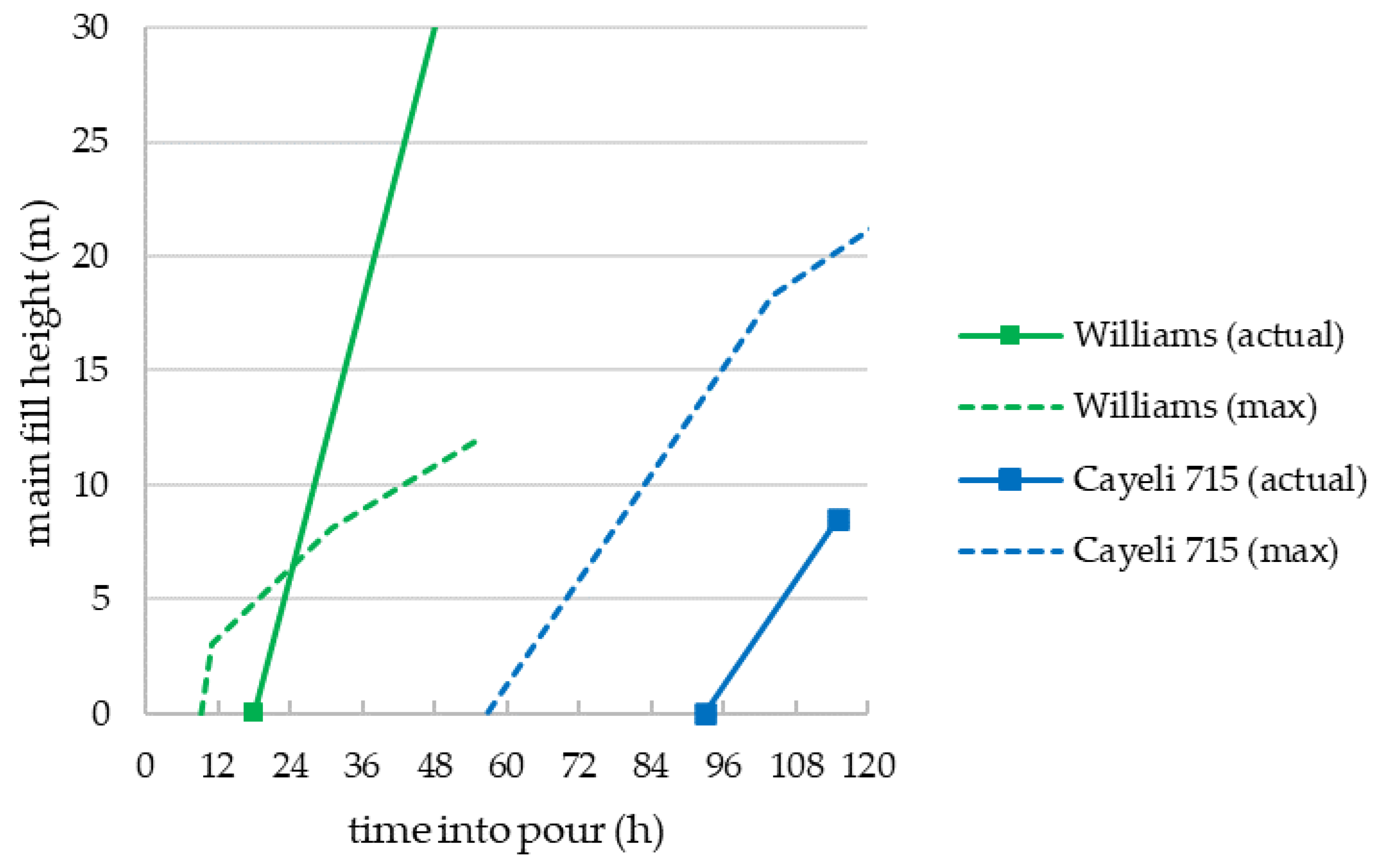
3. Back-Analyses of Field Case Histories
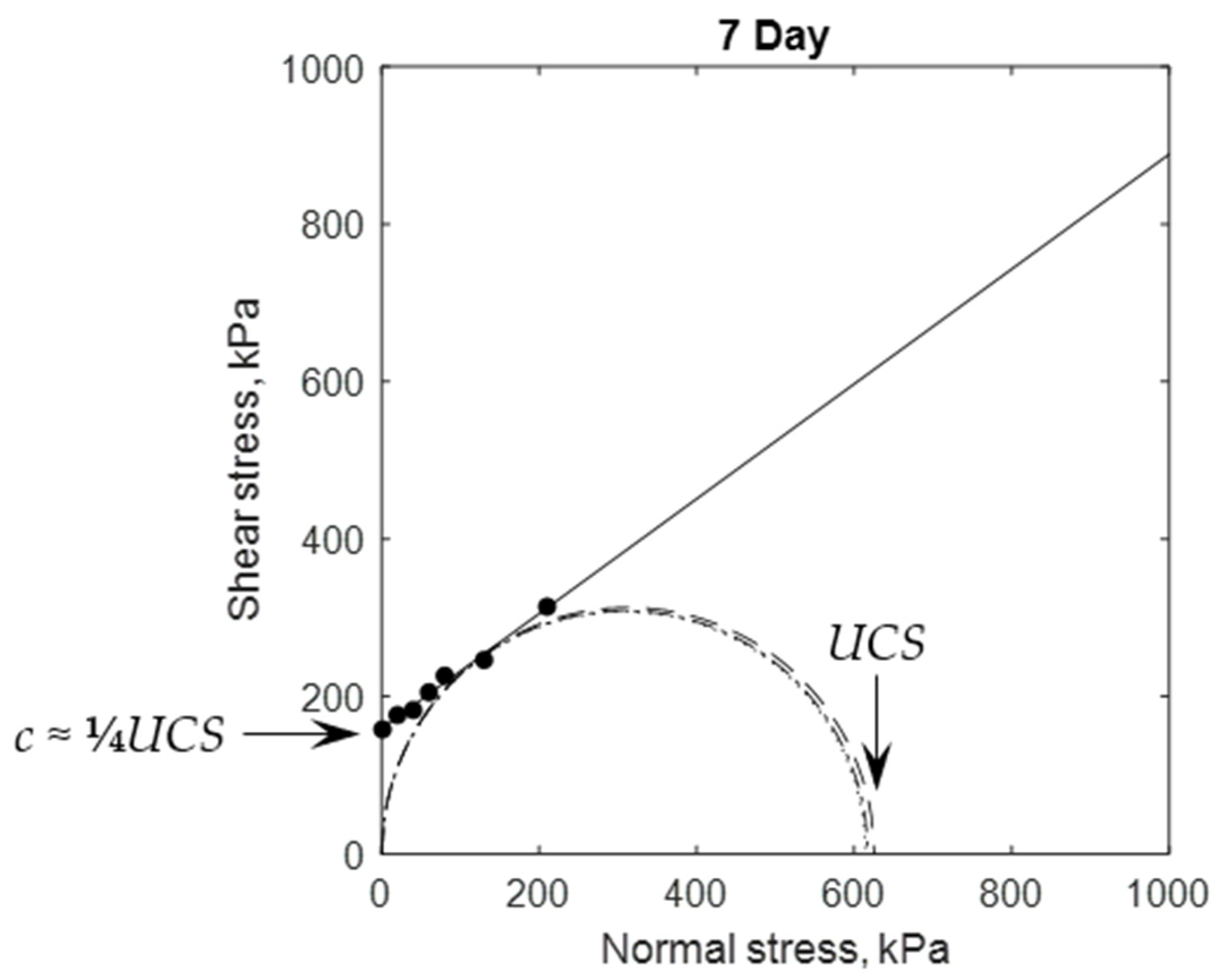
3.1. Case History Analysis Parameters
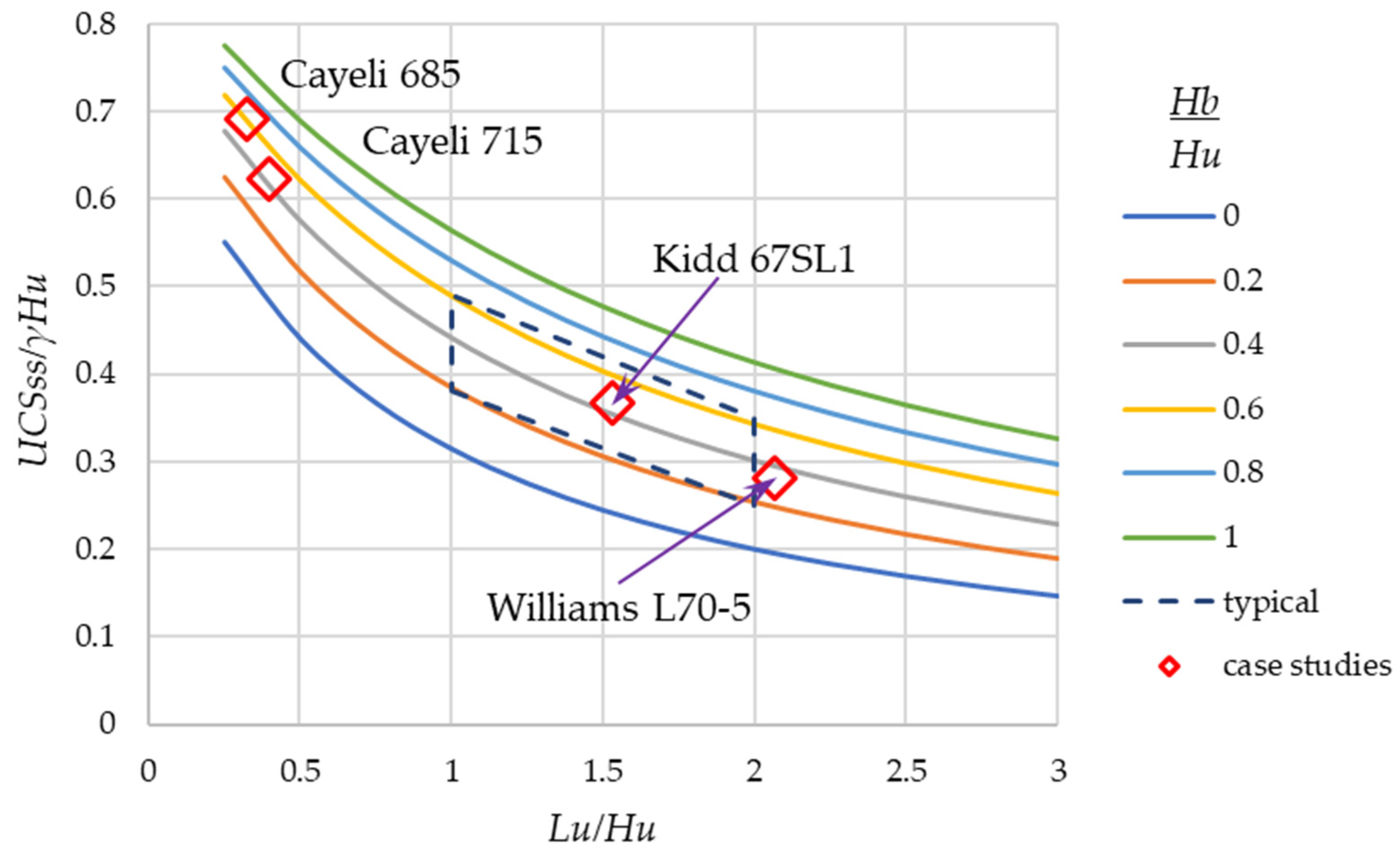
3.2. Self-Supporting CPB Plug Strength Requirements
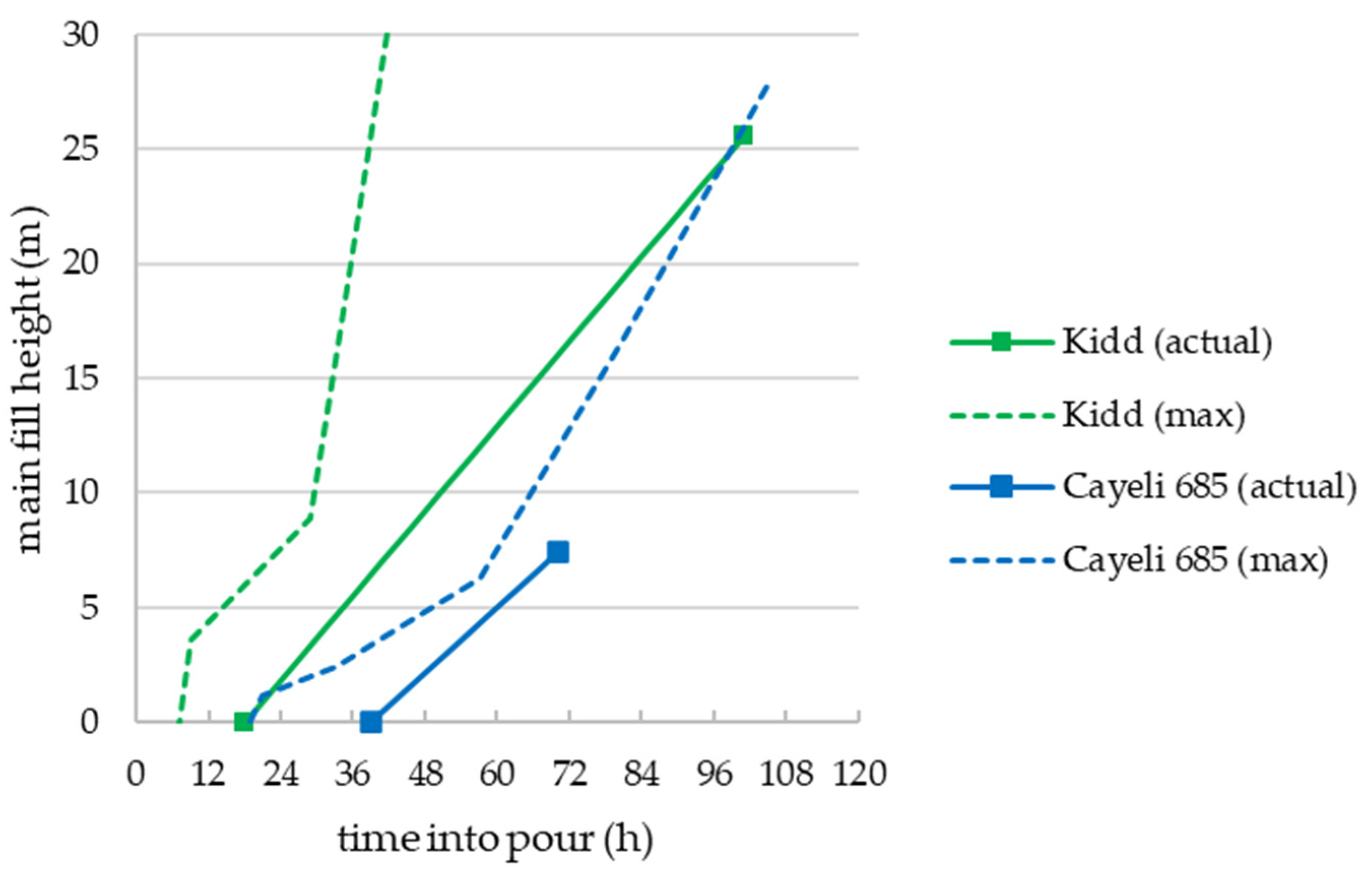
3.3. Main Pour Back Analyses to Determine Continuous Pour Viability
3.4. Final CPB Plug Strength Requirement
4. Discussion: Application at Other Mine Sites
“In bulk mining stopes, the paste fill should be placed approximately 1 m above the draw point brow and permitted to cure to approximately 150 kPa strength before filling the remainder of the stope.”([2], p.92)
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, J.; Ferreira, J.; Le Lievre, T. Transition from discontinuous to continuous paste filling at Cannington Mine. In Proceedings of the 11th International Symposium on Mining with Backfill, Perth, Australia, 20–22 May 2014; Australian Center for Geomechanics: Perth, Australia, 2014. [Google Scholar]
- Potvin, Y.; Thomas, E.; Fourie, A. Handbook on Mine Fill; Australian Centre for Geomechanics: Perth, Australia, 2005; ISBN 0-9756756-2-1. [Google Scholar]
- Yumlu, M.; Guresci, M. Paste backfill bulkhead failures and pressure monitoring at Cayeli mine. CIM Bull. 2007, 100, 1001–1010. [Google Scholar]
- Revell, M.; Sainsbury, D. Paste bulkhead failures. In Proceedings of the 9th International Symposium on Mining with Backfill, Montreal, QC, Canada, 29 April–2 May 2007; Canadian Institute of Mining: Montreal, QC, Canada, 2007; p. 10. [Google Scholar]
- Starfield, A.; Cundall, P. Towards a methodology for rock mechanics modelling. Int. J. Rock Mech. Min. Sci. Geomecahnics Abstr. 1988, 25, 99–106. [Google Scholar] [CrossRef]
- Hassani, F.; Fotoohi, K.; Doucet, C. Instrumentation and backfill performance in a narrow vein gold mine. Int. J. Rock Mech. Min. Sci. 1998, 35, 106. [Google Scholar] [CrossRef]
- Hassani, F.; Ouellet, J.; Servant, S. In situ measurements in a paste backfill: Backfill and rock mass response in the context of rockbursts. In Proceedings of the 17th International Mining Congress and Exhibition of Turkey, IMCET 2001, Ankara, Turkey, 19–22 June 2001; Chamber of Mining Engineers of Turkey: Ankara, Turkey, 2001. [Google Scholar]
- Belem, T.; Harvey, A.; Simon, R.; Aubertin, M. Measurement and prediction of internal stresses in an underground opening during its filling with cemented fill. In Proceedings of the 5th International Symposium on Ground Support in Mining and Underground Construction, Perth, Australia, 28–30 September 2004; Taylor & Francis Group: London, UK, 2004; pp. 619–630. [Google Scholar]
- Doherty, J.; Hasan, A.; Suazo, G.; Fourie, A. Investigation of some controllable factors that impact the stress state in cemented paste backfill. Can. Geotech. J. 2015, 52, 1901–1912. [Google Scholar] [CrossRef]
- Seymour, J.B.; Raffaldi, M.J.; Abraham, H.; Johnson, J.C.; Zahl, E.G. Monitoring the in situ performance of cemented paste backfill at the Lucky Friday mine. In Proceedings of the 12th International Symposium on Mining with Backfill, Minefill 2017, Denver, CO, USA, 19–22 February 2017; Society for Mining, Metallurgy, and Exploration, Inc.: Englewood, CO, USA, 2017; pp. 19–22. [Google Scholar]
- Thompson, B.; Bawden, W.F.; Grabinsky, M. In situ measurements of cemented paste backfill at the Cayeli Mine. Can. Geotech. J. 2012, 49, 755–772. [Google Scholar] [CrossRef]
- Thompson, B.; Grabinsky, M.; Bawden, W. In situ monitoring of cemented paste backfill pressure to increase backfilling efficiency. CIM J. 2011, 2, 199–209. [Google Scholar]
- Alcott, J.; Dallaire, D.; Belem, T. Pastefill optimization at Hecla Quebec’s Casa Berardi mine. In Proceedings of the 53rd US Rock Mechanics/Geomechanics Symposium, New York, NY, USA, 23–26 June 2019. [Google Scholar]
- Helinski, M.; Fahey, M.; Fourie, A. Numerical modeling of cemented mine backfill deposition. J. Geotech. Geoenviron. Eng. 2007, 133, 1308–1319. [Google Scholar] [CrossRef]
- Veenstra, R.L. A Design Procedure for Determining the In Situ Stresses of Early Age Cemented Paste Backfill. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2013. Available online: http://hdl.handle.net/1807/36027 (accessed on 19 December 2018).
- Yilmaz, E.; Belem, T.; Benzaazoua, M. Effects of curing and stress conditions on hydromechanical, geotechnical and geochemical properties of cemented paste backfill. Eng. Geol. 2014, 168, 23–37. [Google Scholar] [CrossRef]
- Yilmaz, E.; Benzaazoua, M.; Belem, T.; Bussière, B. Effect of curing under pressure on compressive strength development of cemented paste backfill. Miner. Eng. 2009, 22, 772–785. [Google Scholar] [CrossRef]
- Fahey, M.; Helinski, M.; Fourie, A. Development of specimen curing procedures that account for the influence of effective stress during curing on the strength of cemented mine backfill. Geotech. Geol. Eng. 2011, 29, 709–723. [Google Scholar] [CrossRef]
- Walske, M.; McWilliam, H.; Doherty, J.; Fourie, A. Influence of curing temperature and stress conditions on mechanical properties of cementing paste backfill. Can. Geotech. J. 2015, 53, 148–161. [Google Scholar] [CrossRef]
- Cui, L.; Fall, M. Mechanical and thermal properties of cemented tailings materials at early ages: Influence of initial temperature, curing stress and drainage conditions. Constr. Build. Mater. 2016, 125, 553–563. [Google Scholar] [CrossRef]
- Grabinsky, M.; Bawden, W.F.; Simon, D.; Thompson, B.T.; Veenstra, R.L. In situ properties of Cemented Paste Backfill from three mines. In Proceedings of the 66th Canadian Geotechnical Conference, GeoMontreal 2013, Montreal, QC, Canada, 29 September–3 October 2013; p. 8. [Google Scholar]
- Grabinsky, M.; Simon, D.; Thompson, B.D.; Bawden, W.F.; Veenstra, R.L. Interpretation of as-placed cemented paste backfill properties from three mines. In Proceedings of the Mine Fill 2014, Perth, Australia, 20–22 May 2014; Australian Centre for Geomechanics: Perth, Australia, 2014. Available online: https://papers.acg.uwa.edu.au/p/1404_28_Grabinsky/ (accessed on 20 March 2020).
- Skempton, A.W. The bearing capacity of clays. In Proceedings of the Building Research Congress, London, UK, 11–20 September 1951; Institution of Civil Engineers: London, UK, 1951; pp. 180–189. [Google Scholar] [CrossRef]
- Grabinsky, M.; Thompson, B.; Bawden, W. Evaluating Cemented Paste Backfill Plug Strength and the Potential for Continuous Pouring; Minefill 2020–2021; Taylor & Francis OA: Katowice, Poland, 2021. [Google Scholar]
- Pan, A. Mechanical Properties of Cemented Paste Backfill under Low Confining Stress. Master’s Thesis, University of Toronto, Toronto, ON, Canada, 2019. Available online: http://hdl.handle.net/1807/98310 (accessed on 9 April 2021).
- Helinski, M.; Fahey, M.; Fourie, A. Behavior of cemented paste backfill in two mine stopes: Measurements and modeling. J. Geotech. Geoenviron. Eng. 2010, 137, 171–182. [Google Scholar] [CrossRef]
- El Mkadmi, N.; Aubertin, M.; Li, L. Effect of drainage and sequential filling on the behavior of backfill in mine stopes. Can. Geotech. J. 2013, 51, 1–15. [Google Scholar] [CrossRef]
- Cui, L.; Fall, M. Multiphysics modeling of arching effects in fill mass. Comput. Geotech. 2017, 83, 114–131. [Google Scholar] [CrossRef]
- Witteman, M.; Simms, P. Unsaturated flow in hydrating porous media with application to cemented mine backfill. Can. Geotech. J. 2017, 54, 835–845. [Google Scholar] [CrossRef]
- Shahsavari Goughari, M. Effects of Transient Hydro-mechanical Cemented Paste Tailings Properties on One-dimensional Deposition Behaviour. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2020. Available online: http://hdl.handle.net/1807/103748 (accessed on 9 April 2021).
- Le Roux, K.; Bawden, W.; Grabinsky, M. Liquefaction analysis of early age Cemented Paste Backfill. In Proceedings of the Eighth International Symposia on Mining with Backfill, Minefill 2004, Beijing, China, 19–21 September 2004; The Nonferrous Metals Society of China: Beijing, China, 2004; pp. 233–241. [Google Scholar]
- Le Roux, K. In Situ Properties and Liquefaction Potential of Cemented Paste Backfill. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2004. [Google Scholar]
- Abdelaal, A.G. Early Age Mechanical Behavior and Stiffness Development of Cemented Paste Backfill with Sand. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2011. Available online: http://hdl.handle.net/1807/31668 (accessed on 9 April 2021).
- Simms, P.; Grabinsky, M. Direct measurement of matric suction in triaxial tests on early-age cemented paste backfill. Can. Geotech. J. 2009, 46, 93–101. [Google Scholar] [CrossRef]
- Saebimoghaddam, A. Liquefaction of early age cemented paste backfill. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2010. Available online: http://hdl.handle.net/1807/24868 (accessed on 13 March 2020).
- Belem, T.; Fourie, A.B.; Fahey, M. Time-dependent failure criterion for cemented paste backfills. In Proceedings of the 13th International Seminar on Paste and Thickened Tailings, Paste 2010, Toronto, ON, Canada, 3–6 May 2010; Australian Center for Geomechanics: Perth, Australia, 2010; pp. 147–162. [Google Scholar] [CrossRef]
- Rankine, R.M.; Sivakugan, N. Geotechnical properties of cemented paste backfill from Cannington Mine, Australia. Geotech. Geol. Eng. 2007, 25, 383–393. [Google Scholar] [CrossRef]
- De Araujo, E.E.B.; Simon, D.; de Franca, F.A.N.; de Freitas Neto, O.; dos Santos Junior, O.F. Shear strength of a Cemented Paste Backfill submitted to high confining pressure. Appl. Mech. Mater. 2017, 858, 219–224. [Google Scholar] [CrossRef]
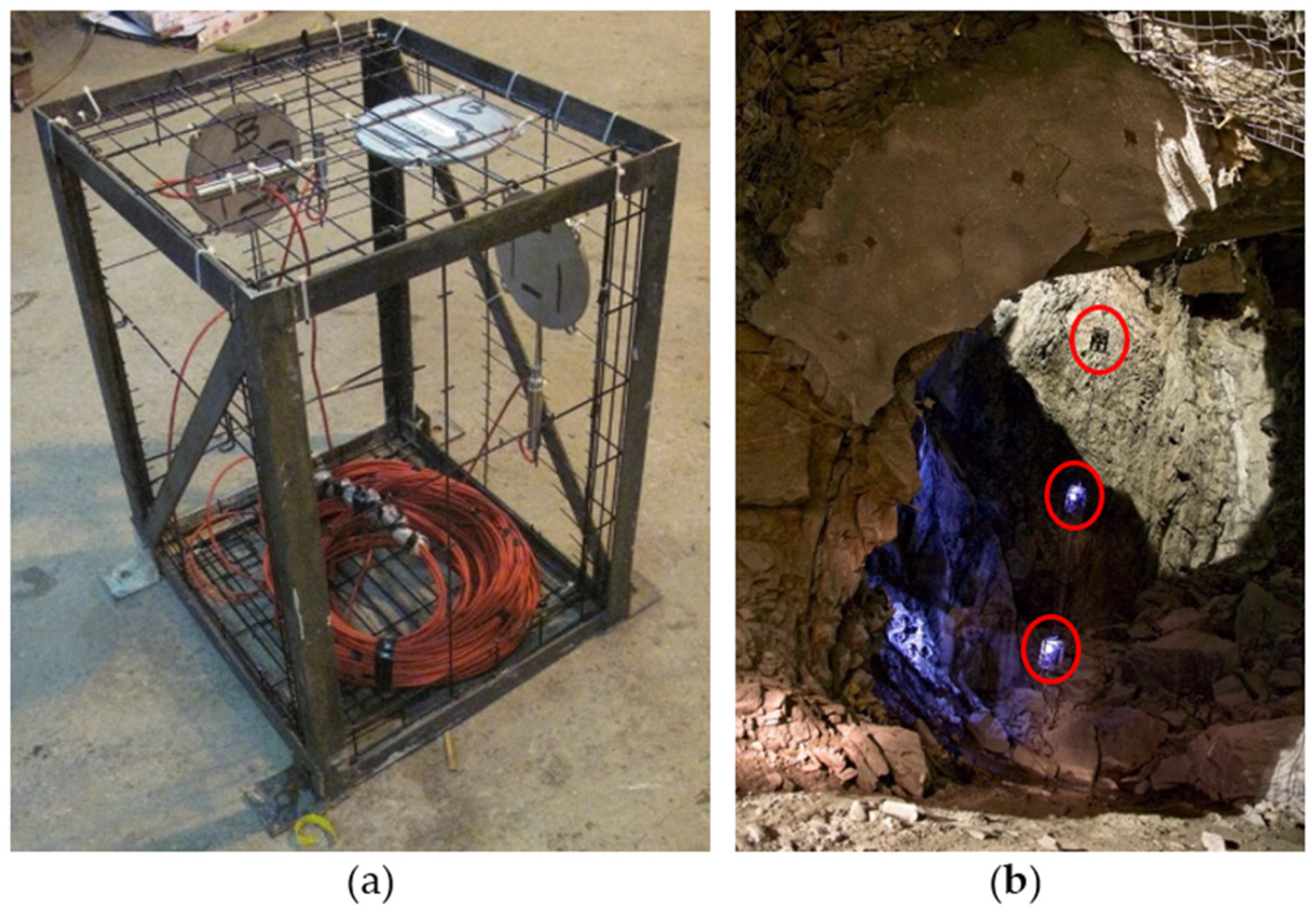


| Zone | Driving Effect | Resisting Effect |
|---|---|---|
| plug’s top surface | n/a | |
| above brow, Zone 1 | ~4 | |
| ½-Prandtl, Zones 2 and 3 | ~0.65 | ~3 |
| undercut, Zone 4 | n/a | 4 |
| Mine/Stope | ||||||
|---|---|---|---|---|---|---|
| Cayeli 685 | 1.8 | 5.5 | 3.5 | 9.0 | 7.4 | 16.4 |
| Cayeli 715 | 1.8 | 4.5 | 2.0 | 6.5 | 8.5 | 15.0 |
| Kidd 67-SL1 | 6.75 | 4.4 | 2.0 | 6.4 | 25.6 | 32.0 |
| Williams L70-5 | 9.3 | 4.5 | 1.5 | 6.0 | 49.0 | 55.0 |
| Mine/Stope | |||||||
|---|---|---|---|---|---|---|---|
| (kN/m3) | (m/h) | (h) | (h) | (h) | (m/h) | (h) | |
| Cayeli 685 | 21.4 | 0.23 | 9 | 39 | 39 | 0.24 | 70 |
| Cayeli 715 | 22.4 | 0.33 | 8 | 20 | 93 | 0.39 | 115 |
| Kidd 67-SL1 | 20.6 | 0.36 | 5 | 18 | 18 | 0.31 | 101 |
| Williams L70-5 | 19.8 | 0.33 | 7 | 18 | 18 | 1.00 | 67 |
| Mine/Stope | SF | ||||
|---|---|---|---|---|---|
| (h) | (kPa) | (kPa) | (kPa ϕ = 36°) | ||
| Cayeli 685 | 30 | 31 | 20 | 1.6 | 80 |
| Cayeli 715 | 12 | 5 | 16 | 0.3 | 64 |
| Kidd 67-SL1 | 13 | 20 | 8 | 2.5 | 32 |
| Williams L70-5 | 11 | 14 | 6 | 2.3 | 24 |
| Mine/Stope | |||||
|---|---|---|---|---|---|
| (m) | (kPa) | (kPa, ϕ = 27°) | (kPa, ϕ = 36°) | (h) | |
| Cayeli 685 | 7.4 | 43.5 | 141 | 174 | 61 |
| Cayeli 715 | 8.5 | 45.6 | 148 | 182 | 107 |
| Kidd 67-SL1 | 25.6 | 56.5 | 184 | 226 | 96 |
| Williams L70-5 | 49.0 | 83.2 | n/a | 333 | 60 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grabinsky, M.; Bawden, W.; Thompson, B. Required Plug Strength for Continuously Poured Cemented Paste Backfill in Longhole Stopes. Mining 2021, 1, 80-99. https://doi.org/10.3390/mining1010006
Grabinsky M, Bawden W, Thompson B. Required Plug Strength for Continuously Poured Cemented Paste Backfill in Longhole Stopes. Mining. 2021; 1(1):80-99. https://doi.org/10.3390/mining1010006
Chicago/Turabian StyleGrabinsky, Murray, Will Bawden, and Ben Thompson. 2021. "Required Plug Strength for Continuously Poured Cemented Paste Backfill in Longhole Stopes" Mining 1, no. 1: 80-99. https://doi.org/10.3390/mining1010006
APA StyleGrabinsky, M., Bawden, W., & Thompson, B. (2021). Required Plug Strength for Continuously Poured Cemented Paste Backfill in Longhole Stopes. Mining, 1(1), 80-99. https://doi.org/10.3390/mining1010006





