An Investigation of the Dynamics Leading to the Emergence of Jets and Rossby Waves from a Background of Homogeneous Turbulence in a Baroclinic Atmosphere ^†

Abstract

Turbulence is a dominant feature of atmospheric motions. Despite its chaotic nature, it is observed to create and maintain coherent structures such as zonal jets and large-scale waves in the atmosphere. Previous studies on the self-organization of turbulence in a baroclinic two-layered fluid have shown that large-scale coherent structures emerge out of a homogeneous turbulent field through a collective type of instability with a preference for barotropic flows. That is, the barotropic coherent structures reorganize the turbulence in such a way as to reinforce themselves through a positive feedback and emerge in the flow. In this work, a statistical framework (S3T) is utilized in order to study the vorticity and thermal flux feedbacks underlying this instability. It is found that the feedbacks produced by the organization of incoherent barotropic and baroclinic eddies by the coherent emergent structures differ. For large stratification, the feedback that results from the organization of barotropic eddies by baroclinic coherent structures is negative and completely cancels out the positive feedback produced by the organization of the baroclinic eddies. On the contrary, the feedback resulting from the organization of the barotropic and the baroclinic turbulent eddies by the barotropic coherent structures do not cancel each other for most scales and lead to the emergence of these structures in the flow. The spatial features of the emerging coherent structures depend on the value of the non-dimensional planetary vorticity gradient. For small values of the gradient, the maximum value of the feedback is found for zonal jets, while for large values of the gradient, the maximum value of the feedback is found for Rossby waves.

1. Introduction

A common feature of atmospheric turbulence is its self-organization in large-scale coherent structures such as zonal jets and Rossby waves. In order to investigate the dynamics of the formation and maintenance of these large-scale structures, simplified models of atmospheric macro-turbulence have been utilized [1,2]. One such model of the baroclinic stably stratified atmosphere is the Phillips two-layer model on a beta-plane with turbulence sustained by random stirring. Numerical simulations of this model have shown that coherent zonal jets and westward-propagating Rossby waves suddenly emerge from the background of the homogeneous turbulent field when a threshold of the energy rate of the stochastic forcing is surpassed [3]. Interestingly, the emerging structures were found to be barotropic for a strong enough stratification, even in cases of mainly exciting baroclinic eddies.

The breaking of the homogeneity of the turbulent flow has been attributed to a new type of collective instability between the emerging large-scale structures and the small-scale turbulent eddies. Since this instability involves the feedback between the statistical mean of the turbulent fluxes and the organization of the turbulent eddies by the emerging flows, it can only be addressed within a framework that describes the statistical state dynamics of the turbulent flow. One such framework that addresses the dynamics of the statistics of the flow through a second-order closure is the Stochastic Structural Stability Theory (S3T) [4]. By utilizing the S3T framework, we investigate in this work the eddy–mean flow feedback underlying the instability of the statistical state dynamics that forms the large-scale flows. The goal is to explain the characteristics of the instability and especially the preference for the emergence of barotropic flows.

2. Formulation of S3T for the Two-Layer Model

Consider a quasi-geostrophic, stably stratified, two-layered fluid on a β-plane. The equations that determine the evolution of the streamfunction ${\psi }_1$ and ${\psi }_2$ of the flow in the two layers can be described in terms of the barotropic $\psi =({\psi }_1+{\psi }_2)/2$ and the baroclinic $\theta =({\psi }_1-{\psi }_2)/2$ streamfunctions [5]:

$${\partial }_t\zeta +\mathcal{J}\left(\psi ,\zeta \right)+\mathcal{J}\left(\theta ,{\nabla }^2\theta \right)+\beta {\partial }_x\psi =-r\zeta +{\xi }_{\psi },$$

(1)

$${\partial }_t\eta +\mathcal{J}\left(\psi ,\eta \right)+\mathcal{J}\left(\theta ,\zeta \right)+\beta {\partial }_x\theta =-r\eta +{\xi }_{\theta },$$

(2)

where $\zeta ={\nabla }^2\psi$ is the barotropic relative vorticity, $\eta =\left({\nabla }^2-2{\lambda }^2\right)\theta$ is its baroclinic counterpart, ${\nabla }^2={\partial }_x^2{+\partial }_y^2$ is the horizontal Laplacian, $\mathcal{J}\left(A,B\right)={\partial }_{x}A{\partial }_{y}B{-\partial }_{y}B{\partial }_{x}A$ is the Jacobian and $\beta$ is the gradient of planetary vorticity. The stratification of the flow is taken into account in the inverse radius of deformation $\lambda =f_0/\sqrt{g^{\prime }H/2}$, where $H/2$ is the depth of each layer, $f_0$ is the Coriolis parameter in the center of the plane, g′ = $2g\left({\rho }_2-{\rho }_1\right)/\left({\rho }_1+{\rho }_2\right)$ is the reduced gravity and ${\rho }_1$, ${\rho }_2$ are the densities in the two layers. Turbulence is sustained by the random stirring ${\xi }_i$ representing vorticity sources in the two layers. The excitation is assumed to be temporally delta-correlated and spatially homogeneous and isotropic injecting energy at a rate $\epsilon$ in a delta ring of radius $K_f$ in wavenumber space. The forcing in the two layers is also assumed to be uncorrelated so that there is the same tendency for the excitation of both barotropic and baroclinic eddies. In addition, there is a sink of energy in the form of the linear dissipation of potential vorticity in both layers with the same coefficient $r$.

In order to investigate analytically the dynamics that lead to the spontaneous emergence of the large-scale structures, we utilize the statistical framework of Stochastic Structural Stability Theory (S3T), which describes the evolution of the first two cumulants of the dynamics [4]. The first-order cumulants are the ensemble means $Z=〈\zeta 〉$, $H=〈\eta 〉$ where the brackets denote an ensemble average over different realizations of the forcing. These can be written as the compact vector $\mathit{\Omega }={\left[〈\zeta 〉 〈\eta 〉\right]}^{{\rm T}}$, where $T$ denotes the transpose. Defining the vorticity deviations from the mean as ${\zeta }_a^{\prime }=\zeta \left({\mathit{x}}_a,t\right)-Z\left({\mathit{x}}_a,t\right)$, where the subscript denotes its evaluation at point ${\mathit{x}}_a$, yields the second-order cumulants as the two-point correlation functions of the vorticity deviations that can be written in compact form as the matrix:

$${\mathit{C}}_{ab}=\mathit{C}\left({\mathit{x}}_a,{\mathit{x}}_b\right)=\left(\begin{array}{cc}〈{\zeta }_a^{\prime }{\zeta }_b^{\prime }〉& 〈{\zeta }_a^{\prime }{\eta }_b^{\prime }〉\\ 〈{\eta }_a^{\prime }{\zeta }_b^{\prime }〉& 〈{\eta }_a^{\prime }{\eta }_b^{\prime }〉\end{array}\right).$$

(3)

The corresponding correlation functions of the random stirring are given by

$$〈{\xi }_i\left({\mathit{x}}_a,t_a\right){\xi }_i\left({\mathit{x}}_b,t_b\right)〉=\delta \left(t_a-t_b\right)\Xi \left(\left|{\mathit{x}}_a-{\mathit{x}}_b\right|\right),$$

(4)

where $\Xi$ is the spatial correlation function with the properties described above. Assuming that the ensemble average is a Reynolds average over the turbulent eddy motions and ignoring higher order cumulants that describe eddy–eddy interactions, it has been shown that the dynamics close in second order [6]. That is, the dynamical system evolving the first two cumulants is self-contained and given by:

$${\partial }_t\mathit{\Omega }=\mathit{{\rm N}}\left(\mathit{\Omega }\right)+\mathit{R}\left(\mathit{C}\right),$$

(5)

$${\partial }_t{\mathit{C}}_{ab}={\mathit{A}}_a\left(\mathit{\Omega }\right){\mathit{C}}_{ab}+{\left({\mathit{A}}_b\left(\mathit{\Omega }\right){\mathit{C}}_{ab}^T\right)}^T+\Xi \mathit{I},$$

(6)

where $\mathit{N}$ is the function expressing the non-linear dynamics of Equations (1) and (2) in terms of the mean flow vector $\mathit{\Omega }$, $\mathit{R}$ is a matrix expressing the turbulent momentum and heat fluxes forcing the barotropic and the baroclinic mean flow, $\mathit{A}\left(\mathit{\Omega }\right)$ is the matrix describing the influence of the mean flow on the turbulent eddies and $\mathit{I}$ is the identity matrix. This dynamical system describes the co-evolution of the coherent large-scale structures $\mathit{\Omega }$ and the statistics of the small-scale turbulent eddies ${\mathit{C}}_{ab}$.

3. Investigation of the Eddy–Mean Flow Feedback

The state $\mathit{\Omega }=0$, ${\mathit{C}}_{ab}=\Xi \mathit{I}/2$, is an equilibrium of the S3T dynamics of (5)–(6). This state represents a homogeneous turbulent field with no mean flow. The stability of this equilibrium is addressed by introducing harmonic perturbations $\delta \mathit{\Omega }=\mathit{m}{e}^{i\mathit{n}·\mathit{x}+\sigma t}$ and $\delta \mathit{C}=e^{i\mathit{n}·\left({\mathit{x}}_a+{\mathit{x}}_b\right)/2+\sigma t}{\tilde{\mathit{C}}}_h({\mathit{x}}_a-{\mathit{x}}_b)$, where $\mathit{n}=\left(n_x{,n}_y\right)$ is the wave vector of the perturbations and $\sigma$ the eigenvalue of the harmonic modes. In case the real part of the eigenvalue is positive, the perturbations grow and coherent structures of wavenumber $\mathit{n}$ emerge. The manifestation of this instability is the emergence of coherent structures in the turbulent flow. It has been shown that the eigenvectors separate into purely barotropic modes with $H=0$ and baroclinic modes with $Z=0$ [3]. It was also shown in the same work that the eigenvalues for the barotropic (${\sigma }_{\psi }$) and the baroclinic (${\sigma }_{\theta }$) modes, respectively, satisfy the equations:

$${\sigma }_{\psi }-i{n}_x\beta /{\left|\mathit{n}\right|}^2+r=f_{\psi }^{\psi }\left({\sigma }_{\psi },\mathit{n},\beta ,\lambda \right)+f_{\psi }^{\theta }\left({\sigma }_{\psi },\mathit{n},\beta ,\lambda \right),$$

(7)

$${\sigma }_{\theta }-i{n}_x\beta /\left({2\lambda }^2+{\left|\mathit{n}\right|}^2\right)+r=f_{\theta }^{\psi }\left({\sigma }_{\theta },\mathit{n},\beta ,\lambda \right)+f_{\theta }^{\psi }\left({\sigma }_{\theta },\mathit{n},\beta ,\lambda \right),$$

(8)

where $f_{\psi }^{\psi } \mathrm{a}\mathrm{n}\mathrm{d} f_{\psi }^{\theta }$ are the momentum and heat fluxes produced by the reorganization of the barotropic ($f_{\psi }^{\psi }$) and the baroclinic ($f_{\psi }^{\theta }$) turbulent eddies by the barotropic flow, respectively. Similarly, $f_{\theta }^{\psi }$ and $f_{\theta }^{\theta }$ are the momentum and heat fluxes produced by the reorganization of the barotropic and the baroclinic turbulent eddies by the baroclinic flow. As it can be seen from (7)–(8), the mean flow tendency is solely driven by the turbulent fluxes. If the fluxes are upgradient, they tend to reinforce the mean flow perturbations, leading to instability. It is worth noting that there can be positive or negative interference between the two flux terms. That is, the barotropic and the baroclinic eddies can be organized in different ways by the large-scale flow and can produce fluxes that are either mutually reinforcing (both upgradient or downgradient) or negating (one upgradient and one downgradient) each other. The flow will respond to their sum.

In order to investigate the fluxes driving the instability, we focus on the parameter regime very close to the stability boundary, that is, when $real\left({\sigma }_{\psi }\right)=real\left({\sigma }_{\theta }\right)=0$. In this limit, it was shown that the imaginary part of the eigenvalues follow the barotropic and baroclinic Rossby wave dispersion relations, respectively [3], that is: $imag\left({\sigma }_{\psi }\right)={\omega }_R^{\psi }=n_x\beta /{\left|\mathit{n}\right|}^2$ and $imag\left({\sigma }_{\theta }\right)={\omega }_R^{\theta }=n_x\beta /\left({2\lambda }^2+{\left|\mathit{n}\right|}^2\right)$. We therefore calculate the real part of the fluxes in this limit

$$f_t^t=real\left[f_{\psi }^{\psi }\left(i{\omega }_R^{\psi },\mathit{n},\beta ,\lambda \right)\right], f_t^c=real\left[f_{\psi }^{\theta }\left(i{\omega }_R^{\psi },\mathit{n},\beta ,\lambda \right)\right],$$

(9)

$$f_c^t=real\left[f_{\theta }^{\psi }\left(i{\omega }_R^{\theta },\mathit{n},\beta ,\lambda \right)\right], f_c^c=real\left[f_{\theta }^{\theta }\left(i{\omega }_R^{\theta },\mathit{n},\beta ,\lambda \right)\right],$$

(10)

and term these as barotropic (denoted with the subscript t) and baroclinic (denoted with the subscript c) flux feedbacks as they describe how the mean flow can reorganize the turbulent eddies so that the turbulent fluxes can enhance it (if the feedback is positive) or weaken it (if the feedback is negative).

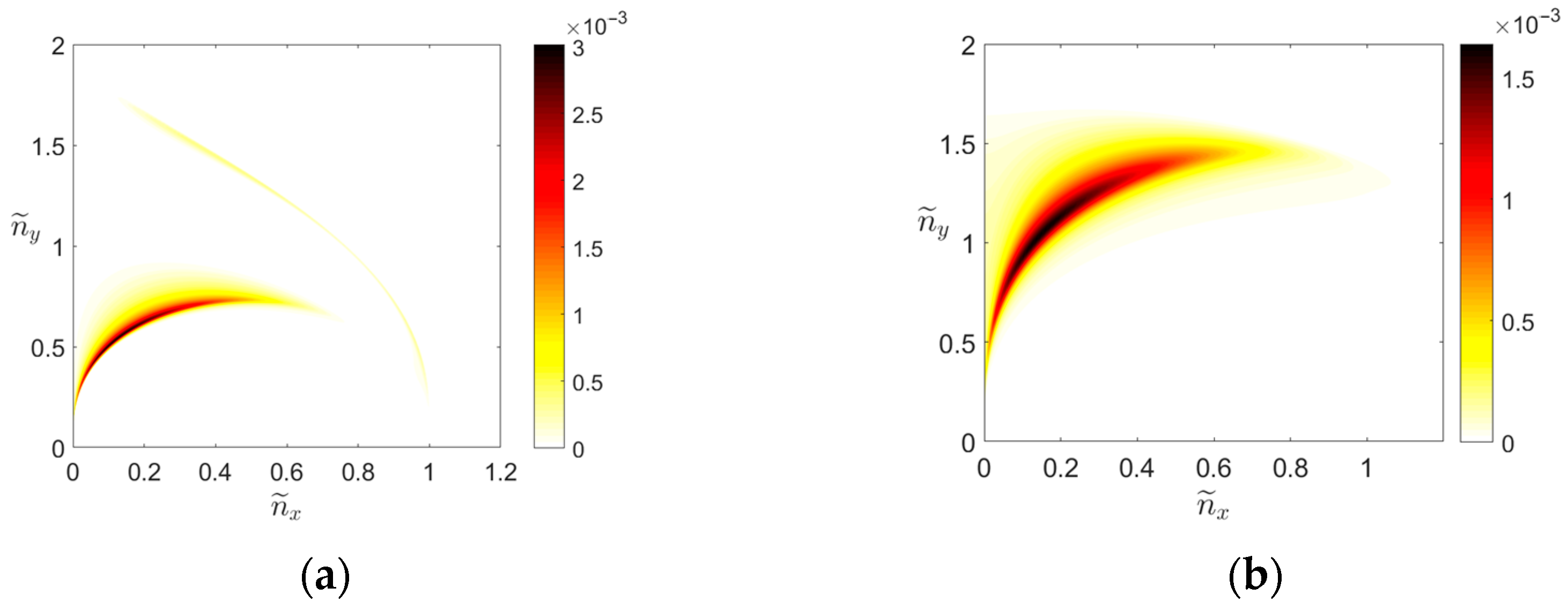
We calculate the feedbacks in the case of large stratification, that is, for $\tilde{\lambda }=\lambda /K_f=1$. Figure 1 and Figure 2 illustrate the barotropic and baroclinic feedbacks, respectively, as a function of the non-dimensional wavenumbers $\tilde{\mathit{n}}=\mathit{n}/K_f$ of the mean flow perturbations for the non-dimensional planetary vorticity gradient $\tilde{\beta }=\beta /\left(r{K}_f\right)=1$. It is noted that the flux feedbacks are symmetric to shifts of the wavevector angle by $\pi /2$. The feedbacks are thus shown only in the first quadrant, that is, for positive values of ${\tilde{n}}_x$ and ${\tilde{n}}_y$.

The organization of the barotropic eddies by the barotropic flow produces a flux feedback $f_t^t$ with positive values for a wide range of wavenumbers with the largest values observed for stationary zonal jets (${\tilde{n}}_x=0)$ with meridional scales close to the excitation scale (${\tilde{n}}_y\le 1$). The organization of the baroclinic eddies by the barotropic mean flow produces a flux feedback $f_t^c$ that has positive values for a ring with a wide range of wavenumbers and is almost isotropic, slightly favoring zonal jets. The largest values correspond to wavenumbers larger than the forcing wavevector. The sum of these two feedbacks (not shown) that are of the same order yields upgradient fluxes that are maximum for zonal flows of slightly larger scale than the forcing, as there is negative interference for scales smaller than the scale of the forcing negating the positive values of $f_t^c$ for these wavenumbers. Thus, zonal jets are expected to dominate the barotropic part of the emerging coherent structures.

The organization of the baroclinic eddies by the baroclinic mean flow produces a flux feedback $f_c^c$ that has positive values for a ring of wavenumbers slightly larger than the forcing wavenumber and is almost isotropic slightly favoring zonal jets. This upgradient flux is of the same order as the magnitude of the barotropic flux feedbacks. The flux feedback that results from the organization of the barotropic eddies by the baroclinic mean flow perturbation is stabilizing for all wavenumbers and is an order of magnitude larger than $f_c^c$. Therefore, their sum is negative, leading the homogeneous equilibrium to be stable with respect to baroclinic perturbations.

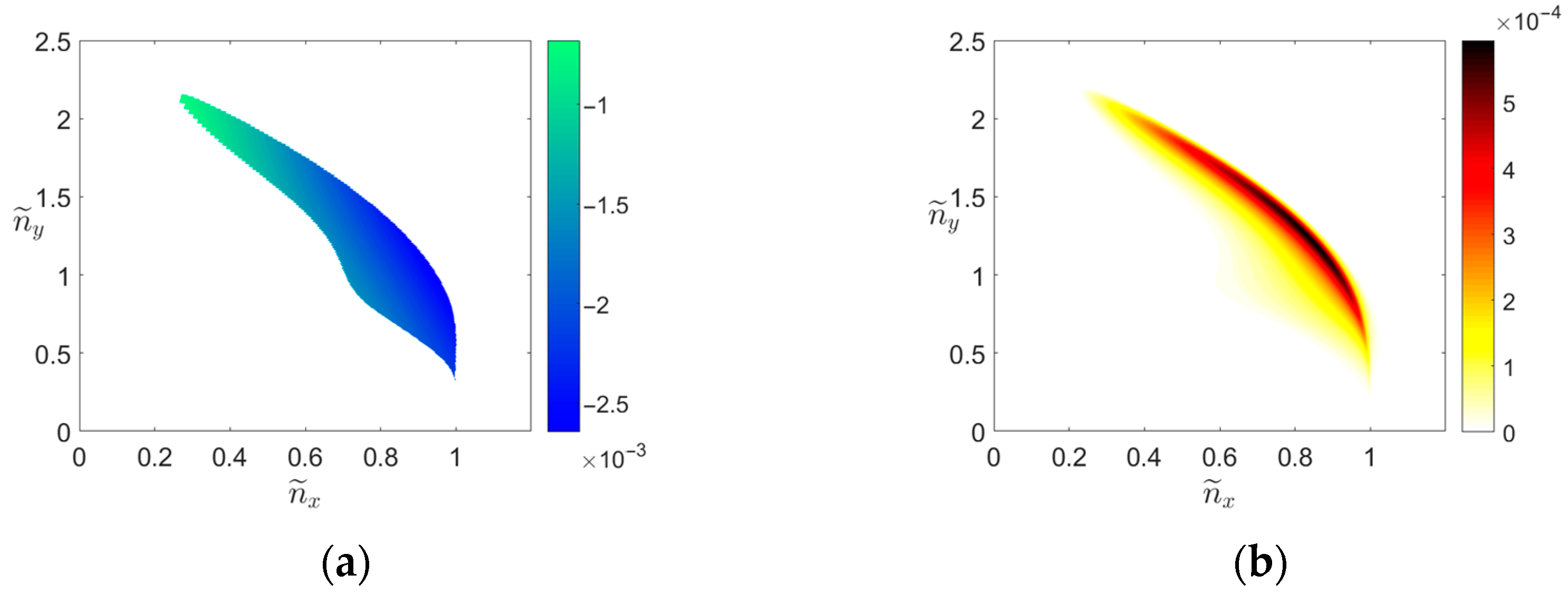
We now consider large values of the planetary vorticity gradient. Figure 3 illustrates the barotropic flux feedbacks for $\tilde{\beta }=100$. In this case, the largest feedback occurs for Rossby waves. The largest positive feedback $f_t^t$ occurs for waves with meridional scales that are twice the scale of the forcing. On the other hand, $f_t^c$ is largest for waves with meridional scale close to the scale of the forcing and its maximum values are approximately half of the corresponding maximum values of $f_t^t$. As there is no significant interference, their sum (not shown) produces two different branches of unstable Rossby waves with small and large meridional scales, respectively. Figure 4 illustrates the baroclinic flux feedbacks in this case. The organization of baroclinic eddies produces upgradient fluxes for baroclinic Rossby waves with scales smaller than the forcing scale. The upgradient $f_c^c$ is cancelled out by the feedback produced by the organization of the barotropic eddies $f_c^t$ that is downgradient for all wavenumbers. This negative interference is found to depend on the stratification of the flow. That is, for this value of $\tilde{\beta }$, the sum of the two flux feedbacks is negative for $\tilde{\lambda }>0.68$. For lower values of $\tilde{\lambda }$, the sum is positive, leading to the emergence of baroclinic structures as well. However, the value of the sum of the baroclinic feedbacks is smaller than its barotropic counterpart, so there is a preference for the emergence of barotropic modes.

4. Conclusions

In this work, we investigated the eddy–mean flow interactions leading to the spontaneous emergence of large-scale coherent structures out of a homogeneous turbulent background in a simplified model of baroclinic turbulence. We utilized the S3T statistical framework that allows for the deterministic description of the interaction between the coherent large-scale structures and the turbulent small-scale eddies. Using this framework, we expressed analytically the momentum and thermal fluxes of the turbulent eddies that drive the instability of the statistical state dynamics of the turbulent flow and which give rise to the large-scale structures. These fluxes express the feedback between the eddy reorganization by the mean flow and the mean flow driving by the eddies and were calculated at the stability boundary for both the barotropic and the baroclinic unstable modes. For large stratification, baroclinic structures were found to be stable due to the stabilizing contribution of the organization of the barotropic eddies by the baroclinic mean flow. This result was found to be independent of the value of the planetary vorticity gradient. For low values of the planetary vorticity gradient, the emerging barotropic modes are zonal jets with meridional scales larger than the forcing scale and arise mainly due to the organization of the barotropic turbulent eddies by the barotropic jet. For large values of the planetary vorticity gradient, the emerging modes are barotropic Rossby waves. The largest flux feedback, whose main contribution is from the organization of barotropic eddies, was found for waves with meridional scales approximately two times the scale of the forcing. A second suboptimal branch of smaller-scale waves was also found and occurs due to the feedback from the baroclinic turbulent eddies and the barotropic Rossby waves.