A Unified Hydrologic Framework for Flood Design Estimation in Ungauged Basins ^†

Abstract

Design flood hydrograph estimation is a key problem in hydrology and is necessary for a variety of applications from the design of hydraulic structures to flood risk mapping processes. Furthermore, in large ungauged basins (>1000 km²), design flood estimation methods mainly rely on single-event theories using digital elevation models, land use/land cover and soil type data, and relevant meteorological information (temperature and rainfall data). The single event-based deterministic approach was adopted based on three modelling components: (i) a synthetic storm generator; (ii) a hydrological simulation model; and (iii) a hydrological routing model. In this study the 100-year design flood (which is assumed equal to 100-year extreme rainfall) was estimated for the Pinios River Basin, Thessaly, Greece, at Larissa outlet station (upstream of the area by about 6500 km²). The hydrological approach is based on semi-distributed modelling of the rainfall–run-off process (at the sub-basin scale) using HEC-HMS v.4.10 software and the SCS-CN method for estimating rainfall excess, as well as the unit hydrograph theory and the Muskingum hydrological flow routing method for propagating the surface run-off to the sub-basin outlets.

1. Introduction

Hydrological extremes such as extreme precipitation and severe floods have consistently posed a risk to human culture. Due to the potential rise in meteorological and hydroclimatological extremes in recent decades, the issue has received significant attention [1]. The primary input for modelling hydrological extremes is rainfall, which has both geographical and temporal characteristics that must be taken into consideration in simulation procedures. In order to design hydraulic structures, design rainfall is often estimated as a univariate variable [2]. Hence, design flood hydrograph estimation is a key problem in hydrology and is necessary for a variety of applications from the design of hydraulic structures to flood risk mapping processes. Furthermore, in large ungauged basins (>1000 km²), design flood estimation methods mainly rely on single-event theories using digital elevation models, land use/land cover and soil type data, and relevant meteorological information (temperature and rainfall data) [3].

The single event-based deterministic approach was adopted based on three modelling components: (i) a synthetic storm generator; (ii) a hydrological simulation model; and (iii) a hydrological routing model [4]. In this study, the 100-year design flood (which is assumed equal to 100-year extreme rainfall) was estimated for the Pinios River Basin, Thessaly, Greece, at Larissa outlet station (upstream area is about 6500 km²). The hydrological approach is based on semi-distributed modelling of the rainfall–run-off process (at the sub-basin scale) using HEC-HMS software and the SCS-CN method for estimating rainfall excess, as well as the unit hydrograph theory for propagating the surface run-off to the sub-basin outlets. Design rainfall at the subbasin scale is estimated from 13 intensity–duration–frequency (IDF) rainfall point curves using thiessen polygons and adjusted to the mean elevation of the sub-basin with the developed precipitation gradients. The design flood hydrograph is estimated by combining the IDF approach with standard time profiles for constructing synthetic rainfall events of a certain probability, the SCS-CN method for extracting the excess from the gross rainfall, and the unit hydrograph theory for propagating the surface run-off to the basin outlet.

2. Materials and Methods

2.1. Study Area

The design flood hydrographs were estimated for a larger part of the Pinios River Basin in Thessaly, Greece. Figure 1 shows the hydrological basin upstream of the point of interest near Larissa City with a total area of 6407 km². The hydrographic network, depicted also shown in Figure 1, was configured in the application of Water Directive 2000/60/EC and includes the main watercourses of the study area. The most extensive and complex network of watercourses develops in the Pinios Basin, and includes, in addition to the main branch of the river, almost all its important tributaries, namely: (a) in the southern part of the basin, Enipeas, Farsaliotis, Sofaditis, and Kalentzis, (b) in the western and southwestern part, Pamisos, Portaikos, Malakasiotiko, and Murgani, and (c) in the northern part, the Litheos and Neochoritis rivers.

Based on the hydrographic network, the study area was divided into 22 watersheds and smaller sub-basins, considering nodes at the entrances of the zones and the confluences of the main watercourses of the study area. The boundaries of the basins are shown in Figure 1 and

Table 1. Sub-watershed characteristics.

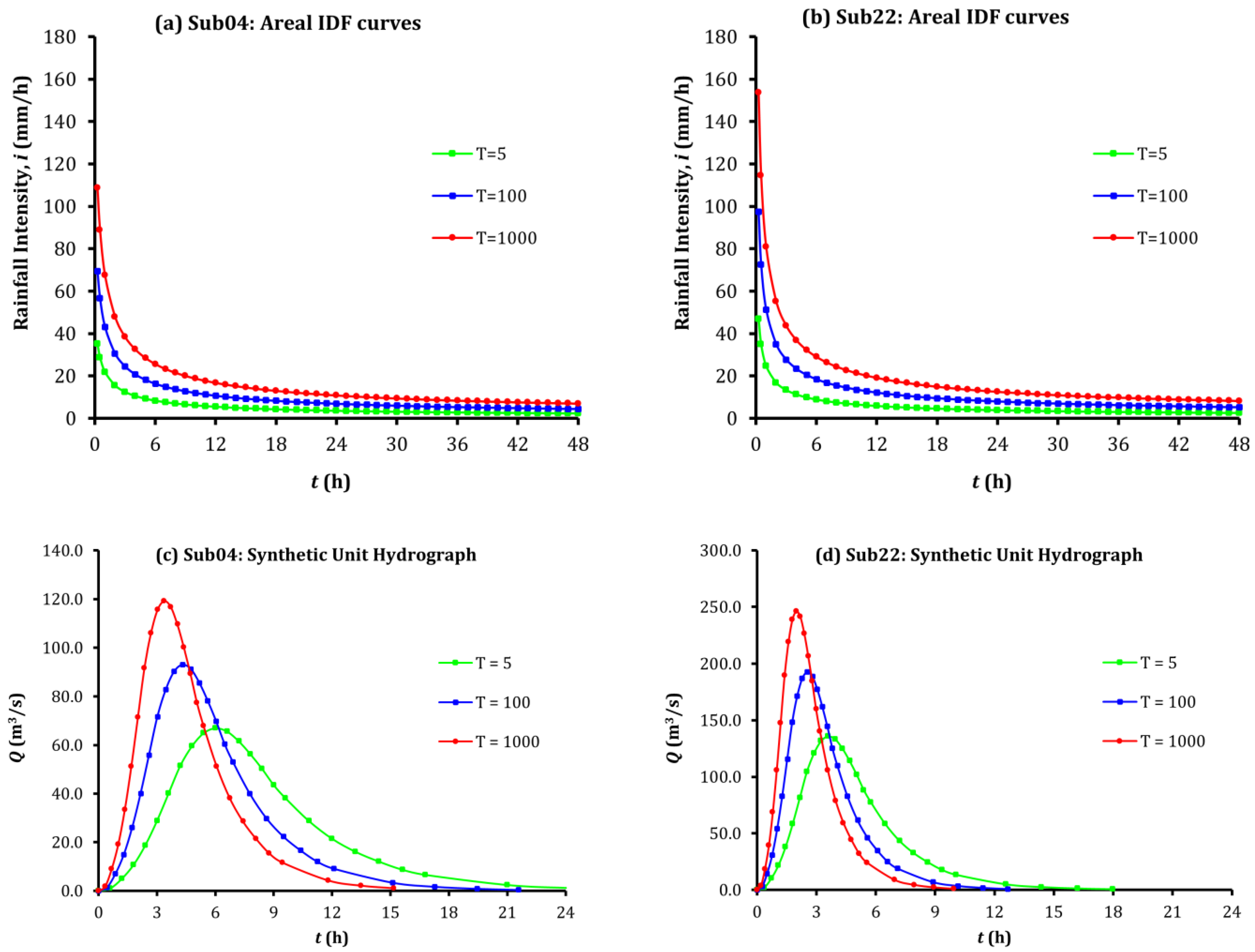
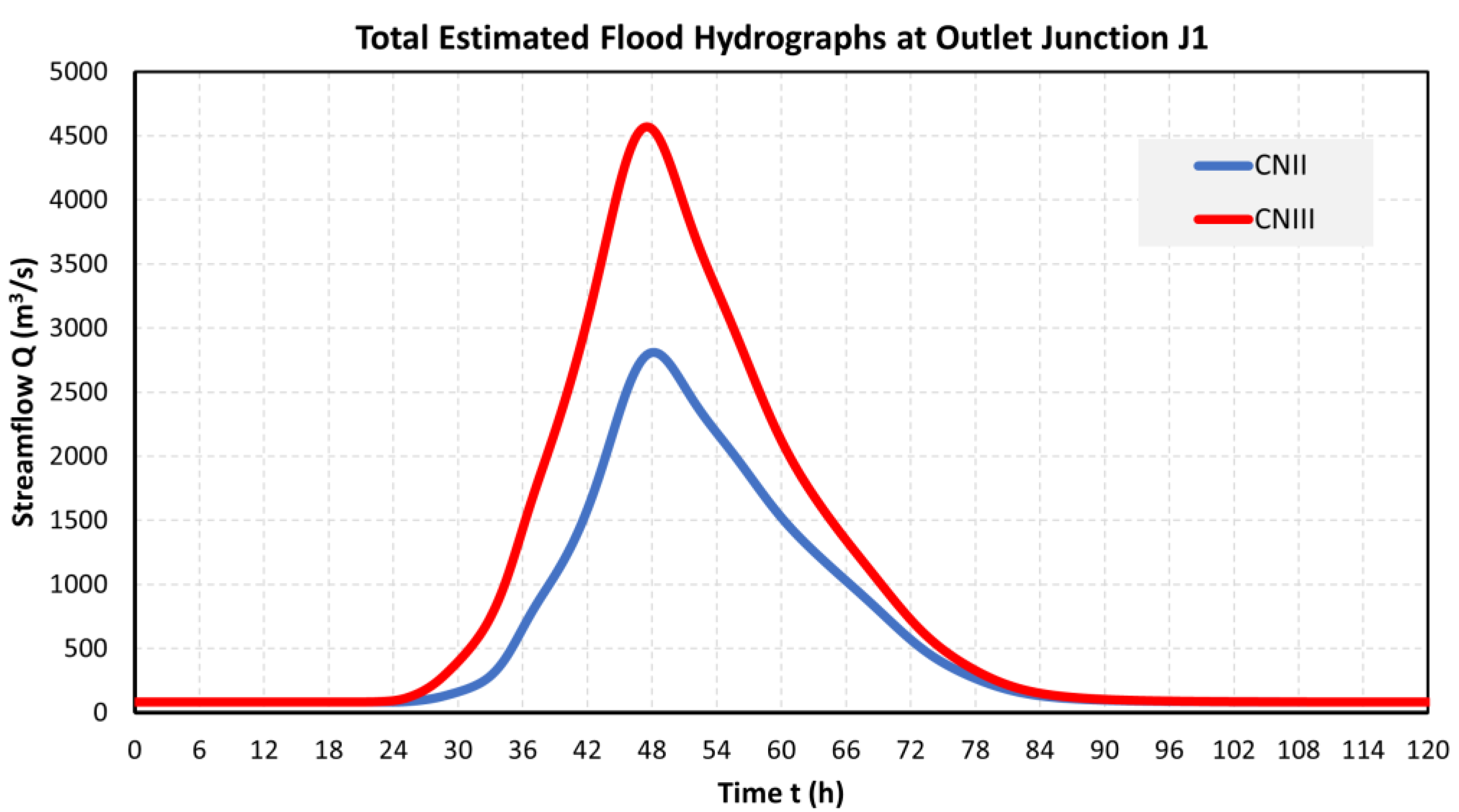
Code	Sub-Watershed	Area (km2)	Mean Elevation (m)	Outlet Elevation (m)	Maximum Flow Length (km)	Curve Number (CNII)	Time of Concentration (h)
Sub01	Larisa	331.15	165.77	61.7	65.617	53.2	20.980
Sub02	Piniada	138.49	193.46	71.4	34.212	55.8	11.130
Sub03	Karditsa-Keramidi	185.09	94.63	79.8	39.607	53.8	36.917
Sub04	Megas	193.14	244.83	92.0	27.504	57.7	9.793
Sub05	Nomi-Mesdani	37.70	98.23	84.2	28.226	54.8	22.327
Sub06	Neochoritis	293.48	489.99	84.2	45.317	70.1	8.470
Sub07	Litheos_2	114.68	246.94	88.4	29.161	52.1	8.596
Sub08	Litheos	321.53	288.80	93.3	62.304	60.4	14.766
Sub09	Pamisos	234.13	568.03	98.0	38.381	59.2	6.848
Sub10	Sarakina-Mesdani	298.87	298.67	98.0	55.542	57.9	13.455
Sub11	Portaikos	296.42	629.41	104.1	34.830	59.1	6.605
Sub12	Malakasiotikos	518.53	962.30	236.6	42.671	62.1	7.196
Sub13	Mourgani	440.46	698.19	236.6	61.254	68.2	10.229
Sub14	Vlochos	92.68	98.42	83.3	23.084	53.1	23.535
Sub15	Kalentzis	398.20	321.06	92.5	41.734	58.2	11.776
Sub16	Enipeas_1	356.69	201.85	86.3	65.253	56.9	20.166
Sub17	Enipeas_2	682.97	524.27	158.9	69.814	59.9	13.685
Sub18	Farsaliotis_1	565.33	140.91	89.0	56.036	53.9	31.078
Sub19	Farsaliotis_2	310.32	299.73	109.3	39.066	55.7	11.690
Sub20	Sofaditis_1	158.58	210.05	93.2	42.671	58.6	13.228
Sub21	Sofaditis_2	203.92	542.48	249.0	34.009	64.7	7.890
Sub22	Sofaditis_3	234.80	739.57	249.0	27.447	64.1	5.783

and present the main characteristics of the sub-watersheds. In the mountainous and semi-mountainous parts of the hydrological basin, the hydrological basins were drawn based on a digital elevation model (DEM) with a pixel size of 25 × 25 m, while in the plain areas, a DEM with 5 m resolution was used across the main bed of the Pinios River combined with National Cadastre maps as well as Google Earth satellite images. The mapping based on higher resolution information was necessary in the c riverbed as well as in the lowland sections, because many of the watercourses are diverted and do not fully follow the natural slope of the ground. In addition, in some cases, embankments have been constructed on either side of the bed to prevent lateral runoff, with the result being that these embankments actually define artificial barriers. Artificial interventions in the flow are also created by other projects, such as road embankments, canals, etc. In addition, the information of the hydrographic network was integrated into the DEM (DEM reconditioning) for more accurate mapping of the flow field in the areas of low and very low gradients and for the correct mapping of the sub-basins and the accurate calculation of the geomorphological characteristics of the sub-basins and watercourses.

The run-off curve number (CN) proposed by the Soil Conservation Service (SCS, 1972) was used to condense the physiographic characteristics of the watershed into a single representative value. In the present study, it is used to estimate the maximum potential retention, which is the input data of the SCS-CN method (the method is applied to estimate hydrological deficits in the context of hydrological modelling with HEC-HMS software). The hydrological simulation model of the Pinios River Basin (just upstream of the confluence of the Pinios River with the Gousbasianiotis stream) includes 22 sub-watersehds, 20 nodes, and 19 river reaches.

2.2. Hydrological Modelling

The surface integration of IDF point measurements at the sub-basin level was carried out using the Thiessen polygon method. The calculated weights resulting from the application of the methodology were used to calculate the intensities (and rainfall heights) for durations of 5 min to 48 h and return periods from 1–1000 years according to the Thiessen method. From the calculated intensities i(d, T) according to the Thiessen method for various durations d and return periods, it is possible to adjust the equation of the rainfall ombrian curves by optimizing the five (5) parameters κ, λ′, ψ′, θ, and η at the sub-basin level. As the objective function of the optimization, the weighted root mean square error (weighted RMSE) was used, resulting from the minimization of the root mean square error of the observed and calculated intensities (RMSEintensity) and rainfall heights (RMSEdepth) for each sub-basin.

In the present study, to estimate the 100-year return period design flood, the flood return period was assumed to be equal to the return period of an extreme typical 100-year return period storm. The duration of the storm was chosen for all sub-basins to be constant and equal to 48 h so that the design storms in the sub-basins had a duration longer than the concentration time of the entire hydrological basin upstream of the point of interest. Finally, the design rainfall was distributed over time using the alternating block method (ABM). Time-distributed precipitation was used as an input hyetograph to the hydrological model to produce the hydrographs for each sub-catchment. Hydrological uncertainty has been expressed in terms of the two typical antecedent moisture conditions (AMC) that are accounted for in the Soil Conservation Service curve number (SCS-CN) approach of moderate (or average—AMC_II) represented by CN_II and wet (or high—AMC_III) represented by CN_III. The transformation of the excess rainfall over each sub-basin to the flood hydrograph at the outlet junction (rainfall–run-off model) was achieved using the dimensionless curvilinear unit hydrograph approach of SCS, which is considered the prevailing modelling approach for ungauged basins. A key assumption of the method was the implementation of the concept of varying the (i.e., run-off-dependent) time of concentration, which affects the shape of unit hydrographs, thus introducing further non-linearities to the overall modelling approach. Hence, in order to take account of the dependence of the response time of the basin against run-off, a kinematic-wave-theory-based semi-empirical formula was employed, considering that t_c is inversely proportional to the design rainfall. Further details are given in [3,4]. HEC-HMS software was used, and the design flood hydrographs from the generated storms were estimated for all scenarios.

3. Results and Discussion

Table 2. Characteristics of SCS synthetic unit hydrographs for the return period of 100 years in all sub-watersheds.

Code	Sub-Watershed	tc (h)	tp (h)	tb (h)	Qp (m3/s)	PD=48h,T=100y (mm)	PD=48h,T=5y (mm)
Sub01	Larisa	14.098	8.584	42.918	80.244	166.2	75.1
Sub02	Piniada	7.754	4.777	23.886	60.300	172.9	83.9
Sub03	Karditsa-Keramidi	25.654	15.517	77.586	24.810	177.3	85.6
Sub04	Megas	6.987	4.317	21.586	93.055	200.0	101.8
Sub05	Nomi-Mesdani	16.171	9.828	49.138	7.979	125.9	66.1
Sub06	Neochoritis	6.483	4.015	20.075	152.038	163.3	95.7
Sub07	Litheos_2	6.439	3.989	19.943	59.804	134.4	75.4
Sub08	Litheos	11.042	6.750	33.751	99.076	135.6	75.8
Sub09	Pamisos	5.158	3.220	16.099	151.245	216.7	122.9
Sub10	Sarakina-Mesdani	10.026	6.141	30.703	101.237	139.3	77.4
Sub11	Portaikos	4.893	3.061	15.304	201.430	226.9	124.5
Sub12	Malakasiotikos	5.575	3.470	17.350	310.816	230.5	138.4
Sub13	Mourgani	7.625	4.700	23.500	194.924	176.7	98.2
Sub14	Vlochos	16.238	9.868	49.338	19.535	195.6	93.1
Sub15	Kalentzis	8.279	5.093	25.463	162.639	211.4	104.5
Sub16	Enipeas_1	13.719	8.356	41.782	88.784	179.6	83.1
Sub17	Enipeas_2	9.696	5.943	29.714	239.040	207.9	104.4
Sub18	Farsaliotis_1	20.668	12.526	62.628	93.879	175.9	77.8
Sub19	Farsaliotis_2	7.810	4.811	24.056	134.160	181.5	81.0
Sub20	Sofaditis_1	8.901	5.465	27.327	60.352	191.6	86.8
Sub21	Sofaditis_2	5.363	3.343	16.714	126.882	210.3	97.2
Sub22	Sofaditis_3	4.016	2.535	12.674	192.678	232.9	112.3

Note: t_c: adjusted time of concentration, t_p: time to peak, t_b: base time, Q_p: peak run-off, P_D=48h,T=100y: extreme rainfall for the rain duration of 48 h and T = 100 years, P_D=48h,T=5y: extreme rainfall for the rain duration of 48 h and T = 5 years.

presents the characteristics of the calculated synthetic unit hydrographs by the SCS-CN method for the 22 sub-watersheds of the study area and for the selected return period T = 100 years. In addition, Figure 2 presents the developed IDF curves and unit hydrographs from the application of the methodology for two sub-basins, the Megas sub-basin with the code name Sub04 and the mountainous sub-basin of Sofaditis (Sofaditis_3) with the code name Sub22, for selected periods return T = 5, 100, and 1000 years.

Application of the hydrological simulation model of the Pinios River Basin, which includes 22 sub-basins, 20 nodes, and 19 river reaches using (i) the developed IDF curves; (ii) the SCS-CN unit hydrograph model; and (iii) the Muskingum hydrological flow routing model, is shown in Figure 3 for the outlet J1 of the study area for the two employed scenarios and the return period of 100 years.

The design flood values for a return period of T = 100 years at the outlet of the study area, which were estimated by the application of semi-distributed methodology, are 2808 m³/s for average soil moisture conditions (CN_II, average scenario) and 4571 m³/s for wet soil moisture conditions (CN_III, adverse scenario). These flood values as well as the flood hydrographs should be used in the design of hydrotechnical projects in the study area.