Design and Experimental Performance Characterization of a Three-Blade Horizontal-Axis Hydrokinetic Water Turbine in a Low-Velocity Channel †
Abstract
1. Introduction
1.1. Rotor Performance
1.2. Blade Design
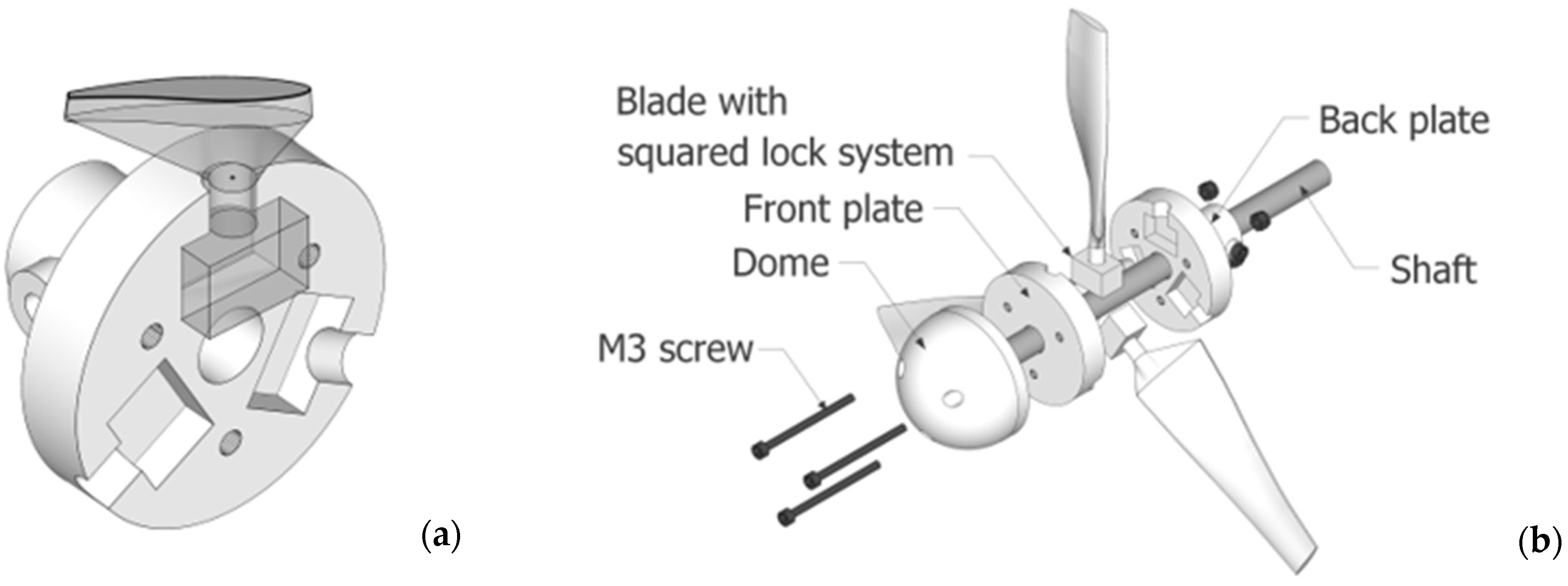
2. Rotor Design and Experimental Set-Up
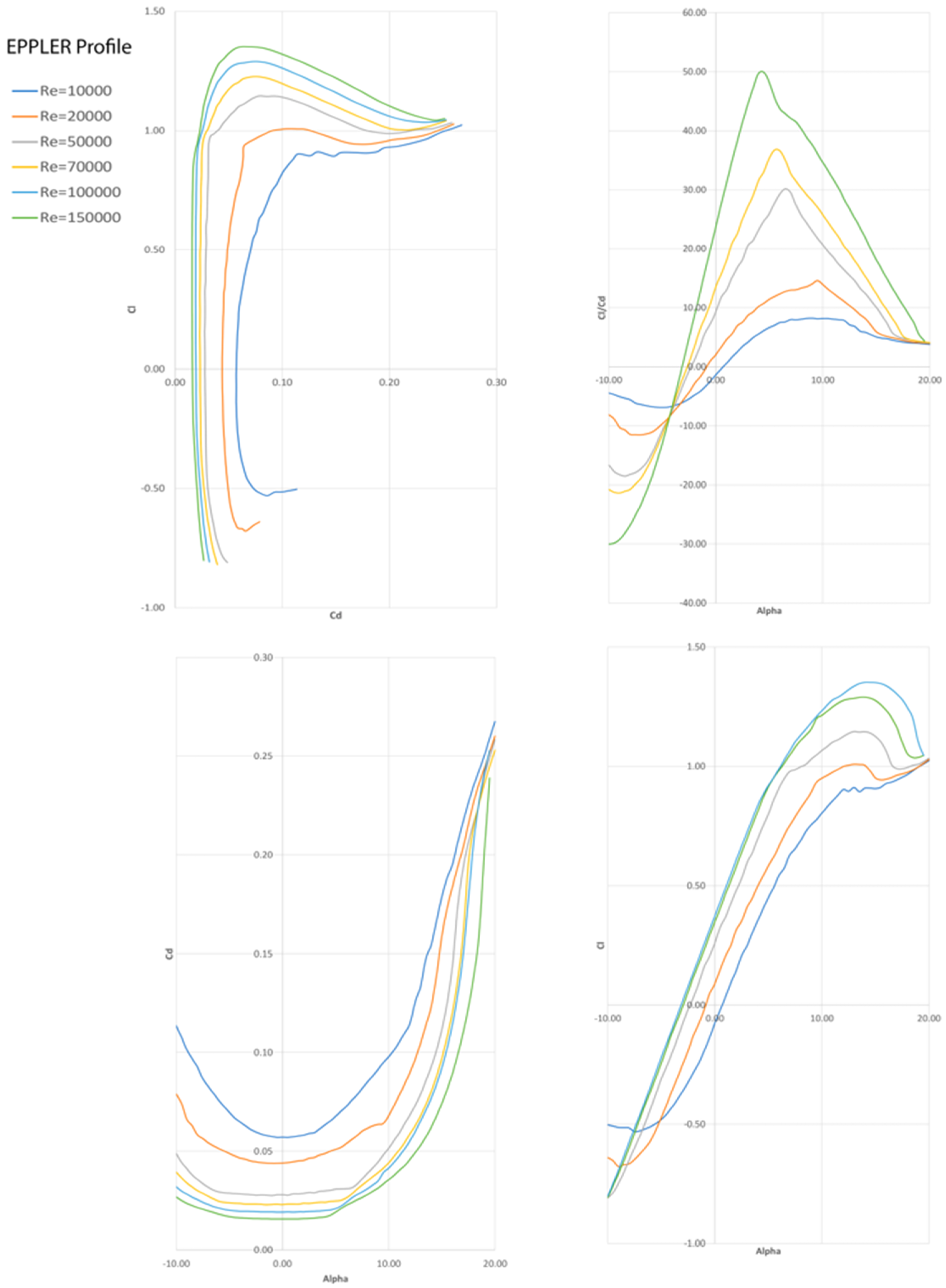
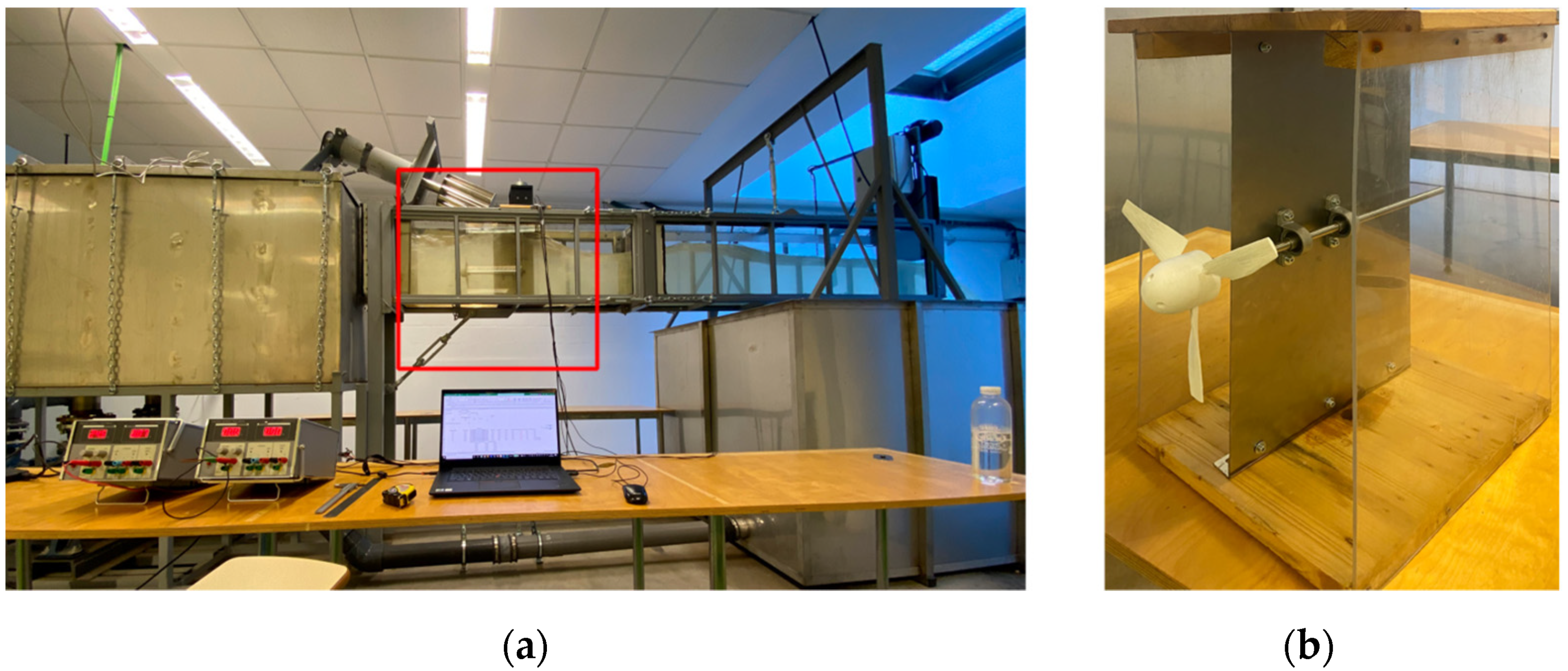
2.1. Methodology
2.2. Results and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- IEA. Renewables 2019—Analysis-IEA; International Energy Agency: Paris, France, 2019. [Google Scholar]
- IEA. Electricity Market Report-July 2021; International Energy Agency: Paris, France, 2021. [Google Scholar]
- Elbatran, A.H.; Yaakob, O.B.; Ahmed, Y.M.; Shabara, H.M. Operation, performance and economic analysis of low head micro-hydropower turbines for rural and remote areas: A review. Renew. Sustain. Energy Rev. 2015, 43, 40–50. [Google Scholar] [CrossRef]
- dos Santos, I.F.S.; Camacho, R.G.R.; Tiago Filho, G.L.; Botan, A.C.B.; Vinent, B.A. Energy potential and economic analysis of hydrokinetic turbines implementation in rivers: An approach using numerical predictions (CFD) and experimental data. Renew. Energy 2019, 143, 648–662. [Google Scholar] [CrossRef]
- Lago, L.I.; Ponta, F.L.; Chen, L. Advances and trends in hydrokinetic turbine systems. Energy Sustain. Dev. 2010, 14, 287–296. [Google Scholar] [CrossRef]
- Loots, I.; Van Dijk, M.; Barta, B.; Van Vuuren, S.J.; Bhagwan, J.N. A review of low head hydropower technologies and applications in a South African context. Renew. Sustain. Energy Rev. 2015, 50, 1254–1268. [Google Scholar] [CrossRef]
- Tian, W.; Mao, Z.; Ding, H. Design, test and numerical simulation of a low-speed horizontal axis hydrokinetic turbine. Int. J. Nav. Archit. Ocean Eng. 2018, 10, 782–793. [Google Scholar] [CrossRef]
- Gasnier, P.; Saoutieff, E.; Soriano, O.; Alessandri, B.; Ojer-Aranguren, J.; Boisseau, S. Cm-scale axial flow water turbines for autonomous flowmeters: An experimental study. Smart Mater. Struct. 2018, 27, 115035. [Google Scholar] [CrossRef]
- Khan, M.J.; Bhuyan, G.; Iqbal, M.T.; Quaicoe, J.E. Hydrokinetic energy conversion systems and assessment of horizontal and vertical axis turbines for river and tidal applications: A technology status review. Appl. Energy 2009, 86, 1823–1835. [Google Scholar] [CrossRef]
- Cardona-Mancilla, C.; Del Río, J.S.; Chica-Arrieta, E.; Hincapié-Zuluaga, D. Horizontal axis hydrokinetic turbines: A literature review. Tecnol. Ciencias Agua 2018, 9, 180–197. [Google Scholar] [CrossRef]
- Castelli, M.R.; Benini, E. Comparison between Lift and Drag-Driven VAWT Concepts on Low-Wind Site AEO. Int. J. Environ. Ecol. Eng. 2011, 5, 1677–1682. [Google Scholar] [CrossRef]
- Barbarić, M.; Guzović, Z. Investigation of the possibilities to improve hydrodynamic performances of micro-hydrokinetic turbines. Energies 2020, 13, 4560. [Google Scholar] [CrossRef]
- Kolekar, N.; Banerjee, A. Performance characterization and placement of a marine hydrokinetic turbine in a tidal channel under boundary proximity and blockage effects. Appl. Energy 2015, 148, 121–133. [Google Scholar] [CrossRef]
- Grasso, F.; Coiro, D.; Bizzarrini, N.; Calise, G. Design of advanced airfoil for stall-regulated wind turbines. Wind Energy Sci. 2017, 2, 403–413. [Google Scholar] [CrossRef]
- Bahaj, A.S.; Molland, A.F.; Chaplin, J.R.; Batten, W.M.J. Power and thrust measurements of marine current turbines under various hydrodynamic flow conditions in a cavitation tunnel and a towing tank. Renew. Energy 2007, 32, 407–426. [Google Scholar] [CrossRef]
- Birjandi, A.H.; Bibeau, E.L.; Chatoorgoon, V.; Kumar, A. Power measurement of hydrokinetic turbines with free-surface and blockage effect. Ocean Eng. 2013, 69, 9–17. [Google Scholar] [CrossRef]
- Benavides-Morán, A.; Rodríguez-Jaime, L.; Laín, S. Numerical Investigation of the Performance, Hydrodynamics, and Free-Surface Effects in Unsteady Flow of a Horizontal Axis Hydrokinetic Turbine. Processes 2022, 10, 69. [Google Scholar] [CrossRef]
- Gharib Yosry, A.; Fernández-Jiménez, A.; Álvarez-Álvarez, E.; Blanco Marigorta, E. Design and characterization of a vertical-axis micro tidal turbine for low velocity scenarios. Energy Convers. Manag. 2021, 237, 114144. [Google Scholar] [CrossRef]


| Velocity Range (m/s) | Blade Radius (m) | Chord R/4 (m) | Hub Radius (m) | Number of Blades (−) |
|---|---|---|---|---|
| 0.43 ÷ 0.68 | 0.084 | 0.03 | 0.025 | 3 |
| Number of Section | Local Solidity σ = cN/2πR | r/R | Chord (m) | Twist (°) |
|---|---|---|---|---|
| 1 | 0.1400 | 0.0000 | 0.03000 | 45.7500 |
| 2 | 0.1200 | 0.0080 | 0.02900 | 41.2500 |
| 3 | 0.1200 | 0.0168 | 0.02800 | 36.2175 |
| 4 | 0.1100 | 0.0252 | 0.02700 | 30.2750 |
| 5 | 0.1100 | 0.0336 | 0.02600 | 23.9550 |
| 6 | 0.1100 | 0.0420 | 0.02500 | 18.5950 |
| 7 | 0.1000 | 0.0504 | 0.02375 | 14.7925 |
| 8 | 0.1000 | 0.0588 | 0.02200 | 11.9775 |
| 9 | 0.0900 | 0.0670 | 0.02100 | 9.0000 |
| 10 | 0.0800 | 0.0755 | 0.01950 | 8.5000 |
| 11 | 0.0800 | 0.0840 | 0.01800 | 8.0000 |
| Test 1 Q1 = 0.052 m3/s | |||||
| H gate (m) | 0.12 | 0.13 | 0.14 | 0.15 | 0.16 |
| H1 upstream (x 0.54 m) | 0.40 | 0.38 | 0.37 | 0.28 | 0.27 |
| V1 upstream (m/s) | 0.43 | 0.45 | 0.47 | 0.62 | 0.64 |
| B = At/Ac | 0.36 | 0.38 | 0.39 | 0.52 | 0.54 |
| Test 2 Q2 = 0.059 m3/s | |||||
| H gate (m) | 0.15 | 0.16 | 0.17 | 0.18 | 0.19 |
| H1 upstream (x 0.54 m) | 0.45 | 0.42 | 0.39 | 0.31 | 0.30 |
| V1 upstream (m/s) | 0.43 | 0.47 | 0.50 | 0.63 | 0.65 |
| B = At/Ac | 0.36 | 0.35 | 0.37 | 0.47 | 0.49 |
| Test 3 Q3 = 0.065 m3/s | |||||
| H gate (m) | 0.16 | 0.17 | 0.18 | 0.19 | 0.20 |
| H1 upstream (x 0.54 m) | 0.50 | 0.44 | 0.43 | 0.33 | 0.32 |
| V1 upstream (m/s) | 0.44 | 0.50 | 0.51 | 0.66 | 0.68 |
| B = At/Ac | 0.29 | 0.33 | 0.34 | 0.44 | 0.46 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferraiuolo, R.; Gharib-Yosry, A.; Fernández-Jiménez, A.; Espina-Valdés, R.; Álvarez-Álvarez, E.; Del Giudice, G.; Giugni, M. Design and Experimental Performance Characterization of a Three-Blade Horizontal-Axis Hydrokinetic Water Turbine in a Low-Velocity Channel. Environ. Sci. Proc. 2022, 21, 62. https://doi.org/10.3390/environsciproc2022021062
Ferraiuolo R, Gharib-Yosry A, Fernández-Jiménez A, Espina-Valdés R, Álvarez-Álvarez E, Del Giudice G, Giugni M. Design and Experimental Performance Characterization of a Three-Blade Horizontal-Axis Hydrokinetic Water Turbine in a Low-Velocity Channel. Environmental Sciences Proceedings. 2022; 21(1):62. https://doi.org/10.3390/environsciproc2022021062
Chicago/Turabian StyleFerraiuolo, Roberta, Ahmed Gharib-Yosry, Aitor Fernández-Jiménez, Rodolfo Espina-Valdés, Eduardo Álvarez-Álvarez, Giuseppe Del Giudice, and Maurizio Giugni. 2022. "Design and Experimental Performance Characterization of a Three-Blade Horizontal-Axis Hydrokinetic Water Turbine in a Low-Velocity Channel" Environmental Sciences Proceedings 21, no. 1: 62. https://doi.org/10.3390/environsciproc2022021062
APA StyleFerraiuolo, R., Gharib-Yosry, A., Fernández-Jiménez, A., Espina-Valdés, R., Álvarez-Álvarez, E., Del Giudice, G., & Giugni, M. (2022). Design and Experimental Performance Characterization of a Three-Blade Horizontal-Axis Hydrokinetic Water Turbine in a Low-Velocity Channel. Environmental Sciences Proceedings, 21(1), 62. https://doi.org/10.3390/environsciproc2022021062











