The New Set Up of Local Performance Indices into WaterNetGen and Application to Santarém’s Network †
Abstract
:1. Introduction
2. Materials and Methods
2.1. Local Performance Indices
2.2. Water Distribution Network Modeling: DDA and PDA Approaches
- DDA: If there is a solution, the requested demand value will always coincide with the provided one.
- PDA: In a good pressure regime, the results will be the same as DDA. Otherwise, in the presence of pressure deficit, since the PDA models the relationship between pressure and demand, the supplied water quantity can be lower than that requested.
3. Results and Discussion
3.1. The Case Study: Santarém’s Water Distribution Network
- Junction and tank characteristics (position and elevation);
- Required water demand and hydraulic load;
- Characteristics of the pipes (geographical location, material, and diameter);
- Network topology.
3.2. Pressure Requested Condition
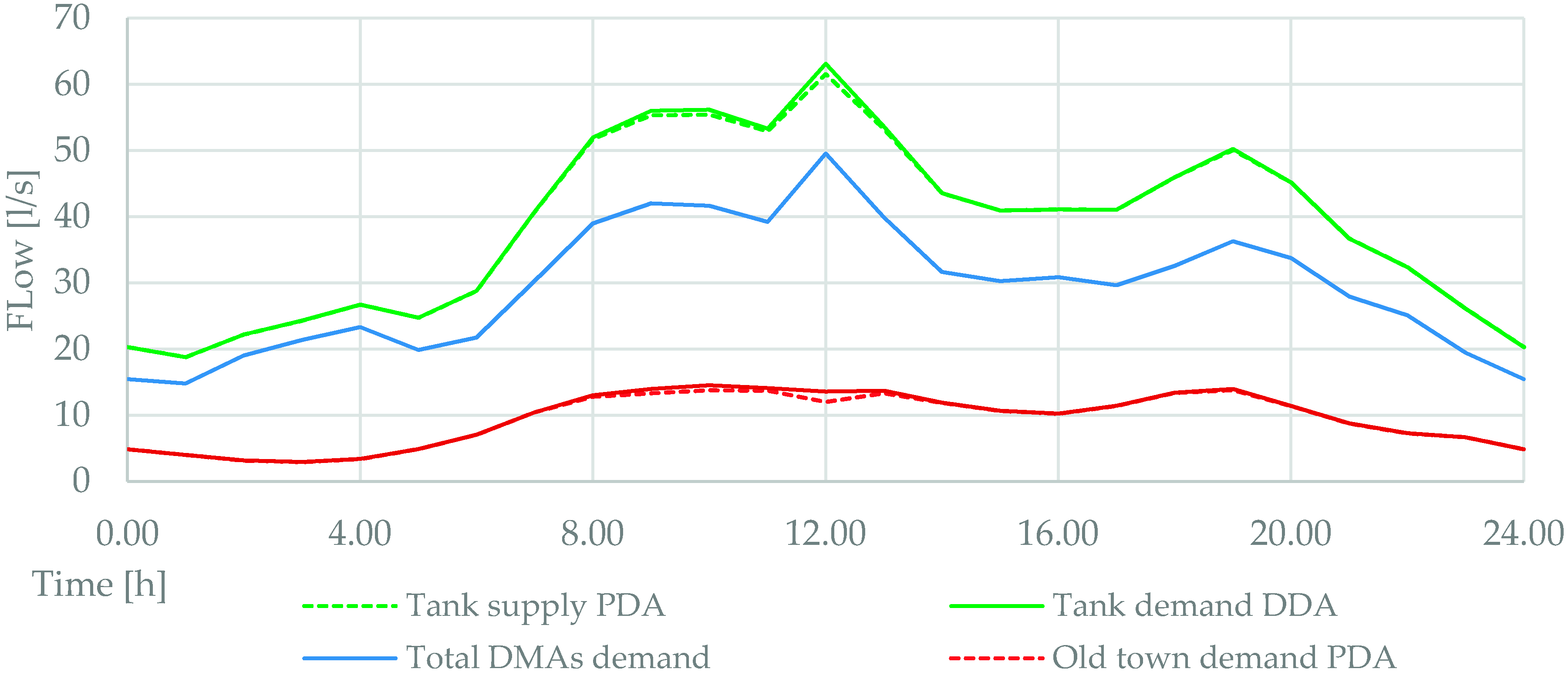
3.3. Water Demand and Water Loss Definition
3.4. Hydraulic Calculations
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Caldarola, F.; Maiolo, M. Local indices within a mathematical framework for urban water distribution systems. Cogent. Eng. 2019, 6, 1643057. [Google Scholar] [CrossRef]
- Muranho, J.; Ferreira, A.; Sousa, J.; Gomes, A.; Sa Marques, A. Waternetgen: An epanet extension for automatic water distribution network models generation and pipe sizing. Water Sci. Technol. Water Supply 2012, 12, 117–123. [Google Scholar] [CrossRef]
- Muranho, J.; Ferreira, A.; Sousa, J.; Gomes, A.; Sa Marques, A. Pressure dependent demand and leakage modelling with an epanet extension—Waternetgen. Procedia Eng. 2014, 89, 632–639. [Google Scholar] [CrossRef]
- Rossman, L.A. EPANET 2: Users Manual. 2000. Available online: https://epanet.es/wp-content/uploads/2012/10/EPANET_User_Guide.pdf (accessed on 28 July 2020).
- Caldarola, F.; Maiolo, M. Algebraic tools and new local indices for water networks: Some numerical examples. In International Conference Numerical Computations: Theory and Algorithms; Sergeyev, Y., Kvasov, D., Eds.; LNCS 11973; Springer: Cham, Switzerland, 2020; pp. 517–524. [Google Scholar] [CrossRef]
- Bonora, M.A.; Caldarola, F.; Muranho, J.; Sousa, J.; Maiolo, M. Numerical experimentations for a new set of local indices of a water network. In International Conference Numerical Computations: Theory and Algorithms; Sergeyev, Y., Kvasov, D., Eds.; LNCS 11973; Springer: Cham, Switzerland, 2020; pp. 495–505. [Google Scholar] [CrossRef]
- Kang, D.; Lansey, K. Revisiting optimal water-distribution system design: Issues and a heuristic hierarchical approach. J. Water Res. Plan. Manag. 2012, 138, 208–217. [Google Scholar] [CrossRef]
- Todini, E. Looped water distribution networks design using a resilience index based heuristic approach. Urban Water J. 2000, 2, 115–122. [Google Scholar] [CrossRef]
- Di Nardo, A.; Di Natale, M. A design support methodology for district metering of water supply networks. Water Distr. Syst. Anal. 2012, 2010, 870–887. [Google Scholar]
- Maiolo, M.; Pantusa, D. A methodological proposal for the evaluation of potable water use risk. Water Pract. Technol. 2015, 10, 152–163. [Google Scholar] [CrossRef]
- Maiolo, M.; Pantusa, D. An optimization procedure for the sustainable management of water resources. Water Sci. Technol. Water Supply 2016, 16, 61–69. [Google Scholar] [CrossRef]
- Maiolo, M.; Pantusa, D. Infrastructure vulnerability index of drinking water supply systems to possible terrorist attacks. Cogent. Eng. 2018, 5, 1456710. [Google Scholar] [CrossRef]
- Maiolo, M.; Pantusa, D.; Chiaravalloti, F.; Carini, M.; Capano, G.; Procopio, A. A new vulnerability measure for water distribution network. Water 2019, 10, 1005. [Google Scholar] [CrossRef]



| Material | Hazen–Williams Roughness |
|---|---|
| Asbestos cement | 140 |
| Ductile Iron | 120 |
| High-density polyethylene | 140 |
| PVC | 140 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bonora, M.A.; Caldarola, F.; Maiolo, M.; Muranho, J.; Sousa, J. The New Set Up of Local Performance Indices into WaterNetGen and Application to Santarém’s Network. Environ. Sci. Proc. 2020, 2, 18. https://doi.org/10.3390/environsciproc2020002018
Bonora MA, Caldarola F, Maiolo M, Muranho J, Sousa J. The New Set Up of Local Performance Indices into WaterNetGen and Application to Santarém’s Network. Environmental Sciences Proceedings. 2020; 2(1):18. https://doi.org/10.3390/environsciproc2020002018
Chicago/Turabian StyleBonora, Marco Amos, Fabio Caldarola, Mario Maiolo, Joao Muranho, and Joaquim Sousa. 2020. "The New Set Up of Local Performance Indices into WaterNetGen and Application to Santarém’s Network" Environmental Sciences Proceedings 2, no. 1: 18. https://doi.org/10.3390/environsciproc2020002018
APA StyleBonora, M. A., Caldarola, F., Maiolo, M., Muranho, J., & Sousa, J. (2020). The New Set Up of Local Performance Indices into WaterNetGen and Application to Santarém’s Network. Environmental Sciences Proceedings, 2(1), 18. https://doi.org/10.3390/environsciproc2020002018









