The Theoretical Approach to the Modelling of Gully Erosion in Cohesive Soil
Abstract
:1. Introduction
2. Materials and Methods
2.1. Gully Erosion by Water
2.2. Instant and Local Rate of Soil Particle Detachment
2.3. Probability Density Function for the Rate of Detachment
2.4. The Main Resistance and Driving Forces
2.5. Probability Density Function for the Factors of Soil Erosion
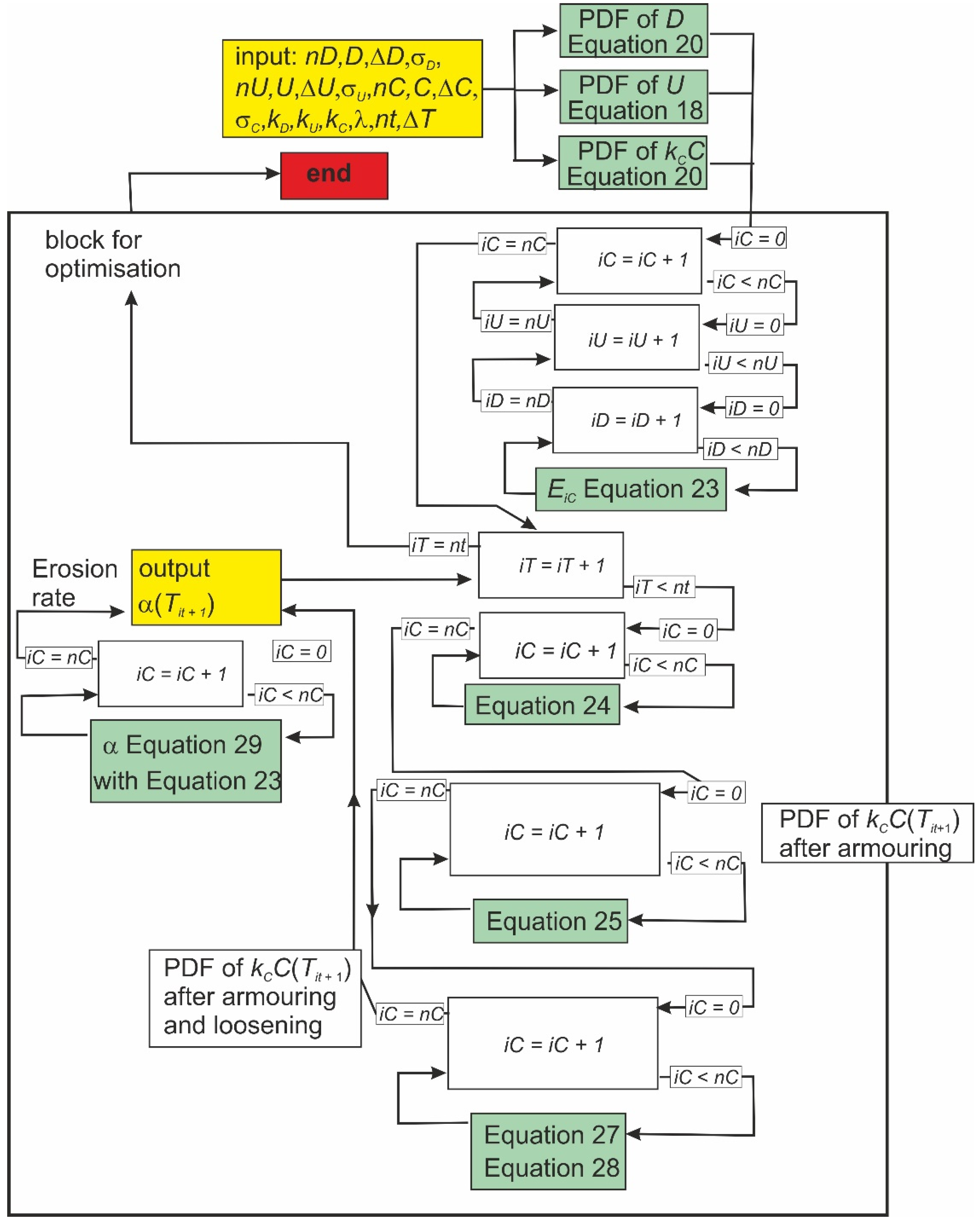
2.6. The Algorithm of Erosion Rate Calculation
- The probability density p(kCC) in the part of the cohesion PDF where resistance forces are less than driving forces decreases due to the erosion EiC of soil with particular cohesion CiCThe initial PDF transforms into intermediate PDF (p*)
- Simultaneously, the intermediate PDF of cohesion is transformed due to the exposition of fresh initial soil in the “windows” of the eroded surface layer to PDF of armored soil (pa)
2.7. The Materials for Comparison of Calculations with Measurements
3. Results
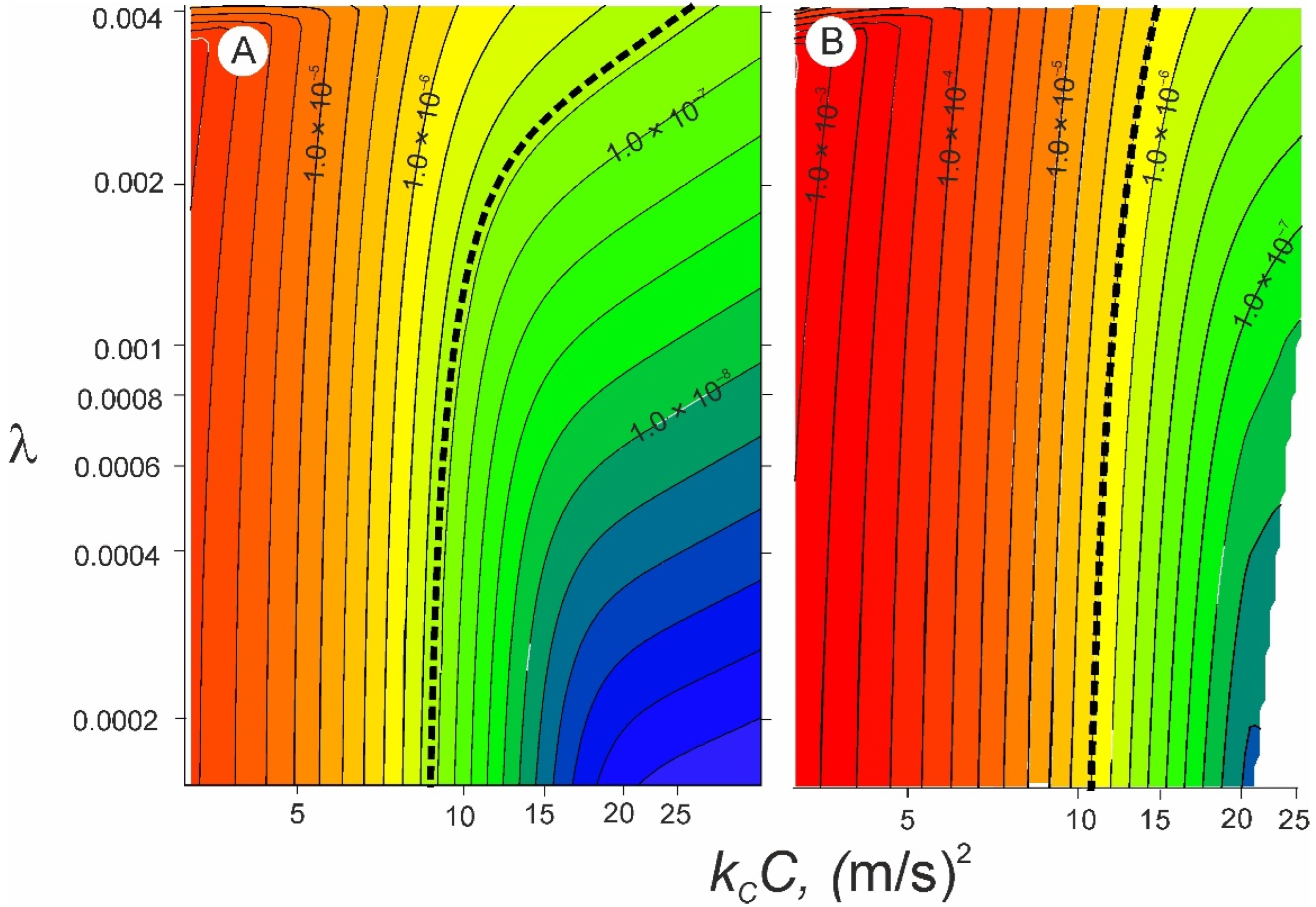
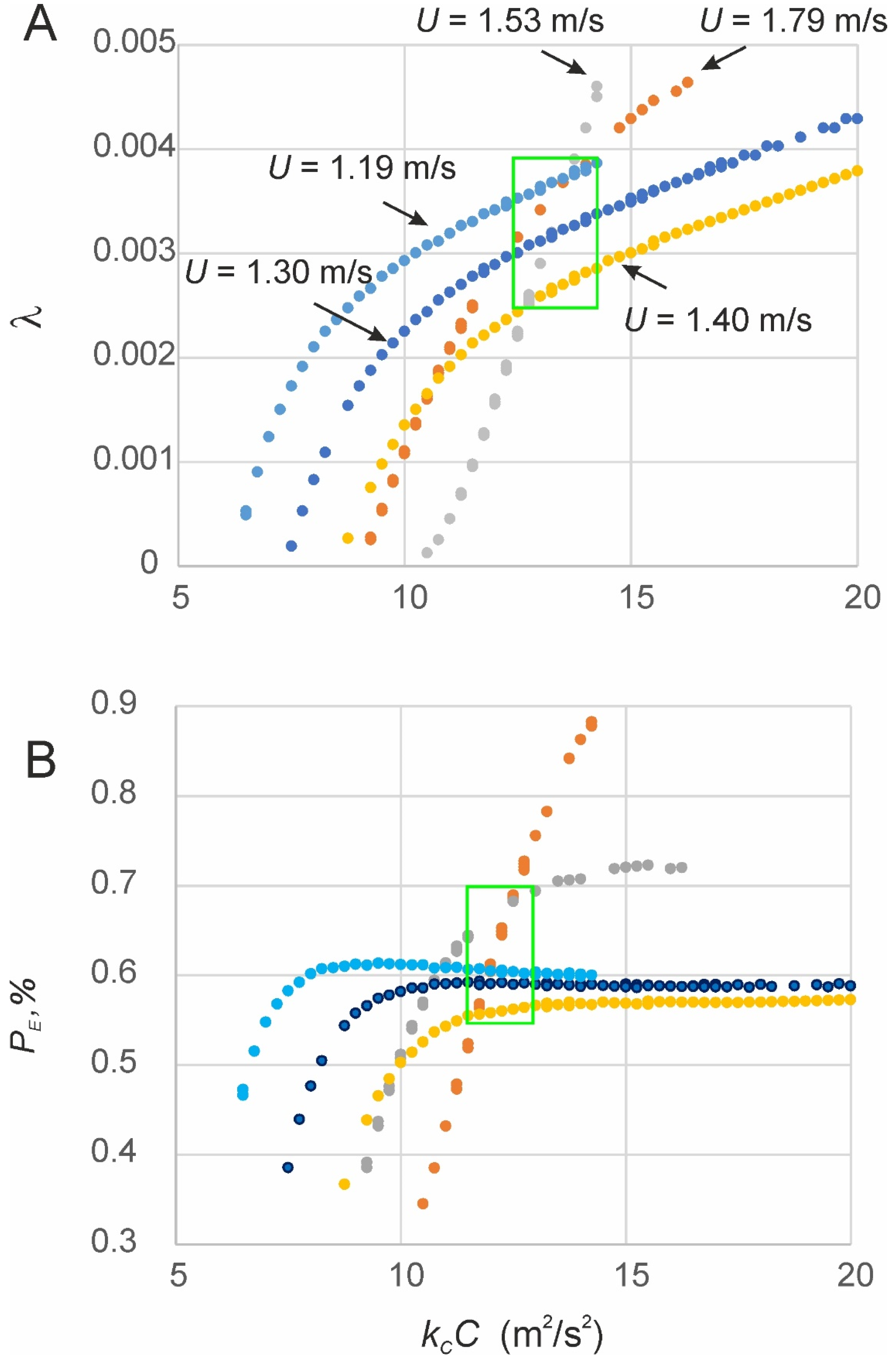
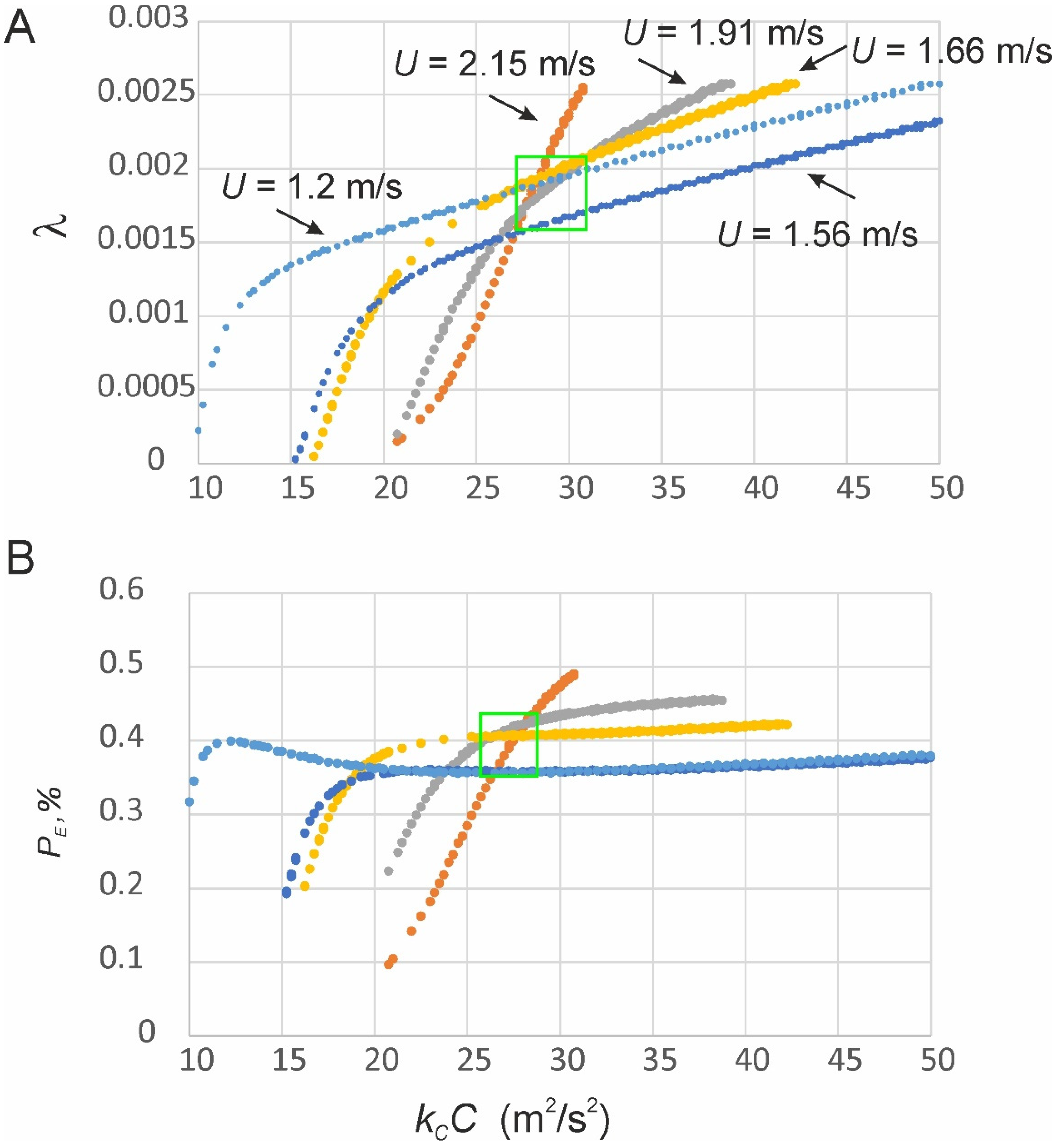
3.1. General Numerical Experiments
3.2. The Comparison of Calculated Erosion Rates with the Measured
4. Discussion
5. Conclusions
Funding
Conflicts of Interest
References
- Ireland, H.A.; Eargle, D.H.; Sharpe, C.F.S. Principles of Gully Erosion in the Piedmont of South Carolina; Technical Bulletins 167374; U.S. Department of Agriculture, Economic Research Service: Washington, DC, USA, 1939. [Google Scholar]
- Watson, D.A.; Laflen, J.M.; Franti, T.G. Estimating Ephemeral Gully Erosion; Paper No. 86-2020; American Society of Agricultural Engineers: St. Joseph, MI, USA, 1986; pp. 1–16. [Google Scholar]
- Kosov, B.F.; Nikolskaya, I.I.; Zorina, Y.F. Experimental research of gullies formation. Exp. Geomorphol. 1978, 3, 113–140. (In Russian) [Google Scholar]
- Sidorchuk, A.Y.; Golosov, V.N. Erosion and sedimentation on the Russian Plain, II: The history of erosion and sedimentation during the period of intensive agriculture. Hydrol. Process. 2003, 17, 3347–3358. [Google Scholar] [CrossRef]
- Montgomery, D.R. Erosional processes at an abrupt channel head: Implications for channel entrenchment and discontinuous gully formation. In Incised River Channels; Darby, S., Simon, A., Eds.; Wiley: New York, NY, USA, 1999; pp. 247–276. [Google Scholar]
- Panin, A.; Borisova, O.; Konstantinov, E.; Belyaev, Y.; Eremenko, E.; Zakharov, A.; Sidorchuk, A. The Late Quaternary evolution of the upper reaches of fluvial systems in the southern East European Plain. Quaternary 2020, 3, 31. [Google Scholar] [CrossRef]
- Poesen, J.W.A.; Torri, D.; Vanwalleghem, T. Gully erosion: Procedures to adopt when modelling soil erosion in landscapes affected by gullying. In Handbook of Erosion Modelling; Morgan, R.P.C., Nearing, M.A., Eds.; Wiley—Blackwell: Chichester, UK, 2011; pp. 360–386. [Google Scholar] [CrossRef]
- Torri, D.; Poesen, J. A review of topographic threshold conditions for gully head development in different environments. Earth Sci. Rev. 2014, 130, 73–85. [Google Scholar] [CrossRef]
- Rose, C.W.; Yu, B.; Ward, D.P.; Saxton, N.E.; Olley, J.M.; Tews, E.K. The erosive growth of hillside gullies. Earth Surf. Process. Landf. 2014, 39, 1989–2001. [Google Scholar] [CrossRef]
- Sidorchuk, A. Models of gully erosion by water. Water 2021, 13, 3293. [Google Scholar] [CrossRef]
- Sidorchuk, A.; Smith, A.; Nikora, V. Probability distribution function approach in stochastic modelling of soil erosion. IAHS Publ. 2004, 288, 345–353. [Google Scholar]
- Du Boys, P. Le Rhône et les Rivières a Lit affouillable. Ann. Ponts Chaussées 1879, 18, 171–195. (In French) [Google Scholar]
- Sidorchuk, A. Stochastic modelling of erosion and deposition in cohesive soils. Hydrol. Process. 2005, 19, 1399–1417. [Google Scholar] [CrossRef]
- Ventsel, E.S. The Probability Theory, 4th ed.; (Teoriya Veroyatnostey); Nauka: Moscow, Russia, 1969; p. 564. (In Russian) [Google Scholar]
- Mirtskhulava, T.E. Principles of Physics and Mechanics of Channel Erosion (Osnovy Fiziki i Mekhaniki Erozii Rusel); Gidrometeoizdat: Leningrad, Russia, 1988; p. 303. (In Russian) [Google Scholar]
- Lamb, M.P.; Dietrich, W.E.; Venditti, J.G. Is the critical Shields stress for incipient sediment motion dependent on channel—bed slope? J. Geophys. Res. 2008, 113, F02008. [Google Scholar] [CrossRef] [Green Version]
- Su, Z.A.; Xiong, D.H.; Dong, Y.F.; Zhang, B.J.; Zhang, S.; Zheng, X.Y.; Fang, H.D. Hydraulic properties of concentrated flow of a bank gully in the dry-hot valley region of southwest China. Earth Surf. Process. Landf. 2015, 40, 1351–1363. [Google Scholar] [CrossRef]
- Nikora, V.I.; Goring, D.G. ADV measurements of turbulence: Can we improve their interpretation? J. Hydraul. Eng. 1998, 124, 630–634. [Google Scholar] [CrossRef]
- Nezu, I.; Nakagawa, H. Turbulence in Open Channel Flows (IAHR Monograph); Balkema: Rotterdam, The Netherlands, 1993; p. 286. [Google Scholar]
- Gardner, W.R. Representation of soil aggregate—Size distribution by a logarithmic—Normal distribution. Soil Sci. Soc. Am. Proc. 1956, 20, 151–153. [Google Scholar] [CrossRef]
- Perfect, E.; Kay, B.D.; Ferguson, J.A.; da Silva, A.P.; Denholm, K.A. Comparison of functions for characterizing the dry aggregate size distribution of tilled soil. Soil Tillage Res. 1993, 28, 123–139. [Google Scholar] [CrossRef]
- Elliot, W.J.; Liebenow, A.M.; Laflen, J.M.; Kohl, K.D. A Compendium of Soil Erodibility Data from WEPP Cropland Soil Field ERODIBILITY Experiments 1987 and 1988; (NSERL Report No. 3); The Ohio State University: Columbus, OH, USA; USDA Agriculture Research Service: Washington, DC, USA, 1989; p. 317. [Google Scholar]
- Barral, M.T.; Arias, M.; Guerif, J. Effects of iron and organic matter on the porosity and structural stability of soil aggregates. Soil Tillage Res. 1998, 46, 261–272. [Google Scholar] [CrossRef]
- Sidorchuk, A.; Nikora, V.; Marsden Fund Project “Stochastic Mechanics of Soil Erosion”. LCR203 Final Report Summary. 2005. Available online: http://fluvial-systems.net/Marsden%20Fund%20award/MarsdenFundReport.html (accessed on 9 January 2022).
- Billinton, R.; Allan, R.N. System reliability evaluation using probability distributions. In Reliability Evaluation of Engineering Systems; Springer: Boston, MA, USA, 1992; Chapter 7; pp. 221–259. [Google Scholar]
- Hewitt, A.E. New Zealand Soil Classification; Landcare Res. Sci. Series 1; Manaaki Whenua Press: Lincoln, New Zealand, 1998; p. 124. [Google Scholar]
- Foster, G.R.; Lane, L.J.; Nearing, M.A.; Finkner, S.C.; Flanagan, D.C. Erosion component. In USDA Water Erosion Prediction Project: Hillslope Profile Model Documentation; NSERL Report No. 2; Lane, L.J., Nearing, M.A., Eds.; National Soil Erosion Research Laboratory, USDA—ARS: Lafayette, IN, USA, 1989; Chapter 10. [Google Scholar]
- Renard, K.G.; Foster, G.R.; Weesies, G.A.; McCool, D.K.; Yoder, D.C. Predicting Soil Erosion by Water: A Guide to Conservation Planning with the Revised Universal Soil Loss Equation (RUSLE). In Agriculture Handbook 703; U.S. Dept. Agriculture: Washington, DC, USA, 1997. [Google Scholar]
- Partheniades, E. Erosion and deposition of cohesive soils. J. Hydraul. Div. Am. Soc. Civ. Eng. 1965, 91, 105–139. [Google Scholar] [CrossRef]
- Nearing, M.A. A probabilistic model of soil detachment by shallow flow. Trans. Am. Soc. Agric. Eng. 1991, 34, 81–85. [Google Scholar] [CrossRef]
- Wilson, B.N. Development of fundamentally based detachment model. Trans. Am. Soc. Agric. Eng. 1993, 36, 1105–1114. [Google Scholar] [CrossRef]
- Winterwerp, J.C.; van Kesteren, W.G.M.; van Prooijen, B.; Jacobs, W. A conceptual framework for shear flow–induced erosion of soft cohesive sediment beds. J. Geophys. Res. 2012, 117, C10020. [Google Scholar] [CrossRef] [Green Version]
- Hairsine, P.B.; Rose, C.W. Modeling water erosion due to overland flow using physical principles: 2. Rill flow. Water Resour. Res. 1992, 28, 245–250. [Google Scholar] [CrossRef]





| N Plot–Soil–Run | Q, l/s | U, m/s | W, m | d, m | U* | σU | E, m/s | Re | Fr |
|---|---|---|---|---|---|---|---|---|---|
| 1–b2–3 | 3.13 | 1.19 | 0.46 | 0.0057 | 0.12 | 0.26 | 2.29 × 10−7 | 6020 | 5.0 |
| 1–b2–4 | 4.01 | 1.30 | 0.46 | 0.0067 | 0.13 | 0.28 | 2.33 × 10−7 | 7670 | 5.1 |
| 1–b2–5 | 5.12 | 1.40 | 0.47 | 0.0077 | 0.14 | 0.30 | 2.29 × 10−7 | 9510 | 5.1 |
| 1–b2–6 | 6.86 | 1.53 | 0.49 | 0.0092 | 0.15 | 0.33 | 5.49 × 10−7 | 12,340 | 5.1 |
| 1–b2–7 | 11.13 | 1.79 | 0.51 | 0.0121 | 0.17 | 0.38 | 1.66 × 10−6 | 19,070 | 5.2 |
| 2–b4–3 | 1.48 | 1.20 | 0.28 | 0.0043 | 0.14 | 0.31 | 3.13 × 10−8 | 4590 | 5.8 |
| 2–b4–4 | 2.22 | 1.56 | 0.29 | 0.0049 | 0.15 | 0.33 | 4.8 × 10−8 | 6750 | 7.1 |
| 2–b4–5 | 3.04 | 1.66 | 0.29 | 0.0062 | 0.17 | 0.38 | 8.54 × 10−8 | 9100 | 6.7 |
| 2–b4–6 | 4.01 | 1.91 | 0.30 | 0.0070 | 0.18 | 0.40 | 1.4 × 10−7 | 11,810 | 7.3 |
| 2–b4–7 | 5.92 | 2.15 | 0.31 | 0.0089 | 0.21 | 0.46 | 3.49 × 10−7 | 16,830 | 7.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sidorchuk, A. The Theoretical Approach to the Modelling of Gully Erosion in Cohesive Soil. Earth 2022, 3, 228-244. https://doi.org/10.3390/earth3010015
Sidorchuk A. The Theoretical Approach to the Modelling of Gully Erosion in Cohesive Soil. Earth. 2022; 3(1):228-244. https://doi.org/10.3390/earth3010015
Chicago/Turabian StyleSidorchuk, Aleksey. 2022. "The Theoretical Approach to the Modelling of Gully Erosion in Cohesive Soil" Earth 3, no. 1: 228-244. https://doi.org/10.3390/earth3010015
APA StyleSidorchuk, A. (2022). The Theoretical Approach to the Modelling of Gully Erosion in Cohesive Soil. Earth, 3(1), 228-244. https://doi.org/10.3390/earth3010015






