Comparison of Static and Dynamic Young’s Modulus of Prasinites ^†

Abstract

This study aimed to investigate the statistical correlation between the static and dynamic Young’s modulus of prasinites, a metabasic rock type that outcrops at various localities in the southern part of the Attica peninsula. A total of 39 cylindrical specimens was prepared and an extensive experimental program was carried out to determine the static and dynamic deformational properties for each specimen. Using ordinary least squares regression techniques, a new empirical linear equation was established between the aforementioned properties that can be used in the study region, or elsewhere where metabasic rocks with similar characteristics are investigated.

1. Introduction

Knowledge of the elastic (or Young’s) modulus is of vital importance in many aspects of geoengineering applications when it comes to the correct design of mining and civil works founded in or on rock formations, as well as in various sectors of the construction industry when natural stones are used as building materials.

Several methods that are categorized as static and dynamic have been developed to determine the Young’s modulus. Static methods are destructive, time-consuming, and expensive, as they require suitable strain measurement devices and specimens of high quality, which are loaded to failure in a uniaxial compression experiment. On the other hand, the dynamic methods, which are non-destructive, are based upon the response of the specimen to the acoustic excitation. In most cases, these non-destructive techniques are less costly and time-consuming.

Young’s modulus determined by dynamic methods (E_d) is usually greater than that determined by static methods (E_s). This difference is attributed to various causes, such as the different strain rates induced by the acoustic waves versus static loading, drainage conditions of the experiments, heterogeny of the material, anisotropy effects, and the different amplitude of the induced strain [1]. The latter is considered to be the dominant cause, where structural features such as cracks and pores can undergo large deformations during a static experiment, but may remain unaffected by the passage of acoustic waves [1,2]. According to extensive data compiled in [2,3], the ratio of E_d to E_s varies between 0.85 up to 3, and this discrepancy tends to decline for rocks that exhibit a higher elastic modulus and lower porosity.

Considerable attention has been paid to the comparison between E_s and E_d for various rock types and several empirical equations are quoted in the literature [4,5,6,7,8,9,10,11]. These equations are either linear in form, matching Equation (1), or non-linear, matching Equation (2).

E_s = a*E_d + b

(1)

E_s = a*(E_d)^b

(2)

where the constant terms a and b are material-dependent.

The usage of such equations allows the estimation of E_s in cases where E_d is known. However, several experiments should be performed to calibrate these equations to obtain reliable results for the petrological type under consideration.

This study aimed to report the results of an experimental program regarding the examination of a possible correlation between E_s and E_d for prasinites, a metabasic rock type outcropping in the Attica peninsula, Greece. As determined from the literature, this is one of the first efforts on this topic and the proposed equation will be a valuable tool for engineers dealing with this petrological type.

2. Materials and Methods

2.1. Materials

Prasinites are basic metamorphic rocks of volcanic origin. In the field, they appear as massive, isolated blocks, slightly weathered to fresh, with a characteristic light to moderately dark oil-green color. Powder X-ray diffraction analyses revealed a mineralogical paragenesis typical of greenschist facies i.e., actinolite, albite, epidote, chlorite, and quartz. The fine-grained matrix of prasinites is cross-cut by a network of calcite veins.

Sample collection was carried out such that the samples included a wide range of physical characteristics that affect the properties of the material, such as the density of the network of calcareous veins, as typically shown in Figure 1.

2.2. Methods

As the content of this study, 39 cylindrical specimens of NX diameter (54.4 mm) and a height-to-diameter ratio between 2.5 and 3.0 were prepared from rock blocks collected from the field. The experimental program included all the tests necessary to determine the dry density (γ_d), the compressional (V_P), and shear (V_S) wave velocities, as well as the static and dynamic Young’s modulus for each specimen.

2.2.1. Dynamic Young’s Modulus

Using the ultrasonic pulse method [2], the wave velocities (V_P, V_S) were determined by dividing the distance traversed by the waves by the travel time. The operating frequency of the transducers was 1 MHz. The dynamic Young’s modulus (E_d) was calculated from the ultrasonic wave velocities and the dry density in accordance with Equation (3).

E_d = ρ_d*(V_S)²*(3*(V_P)² − 4*(V_S)²)/((V_P)² − (V_S)²)

(3)

2.2.2. Static Young’s Modulus and Uniaxial Compressive Strength

For the determination of the static Young’s modulus and uniaxial compressive strength (UCS), the specimens were compressed in a 5000 kN-capacity loading frame. To measure the axial deformation of the specimen, two aluminum rings were attached to the middle third of the specimen, as shown in Figure 2. Three linear variable differential transducers (LVDTs), mounted on the rings at an angle of 120° apart, measured the distance of the rings continuously throughout the experiments. The axial strain of the specimen was evaluated as the average deformation deduced from the three LVDT measurements. Diametral strain was evaluated by the circumferential deformation of the specimen, measured with a circumferential extensometer mounted on the specimen around its mid-height. The tests were executed by lateral displacement control with a constant strain rate of 15 μm/min.

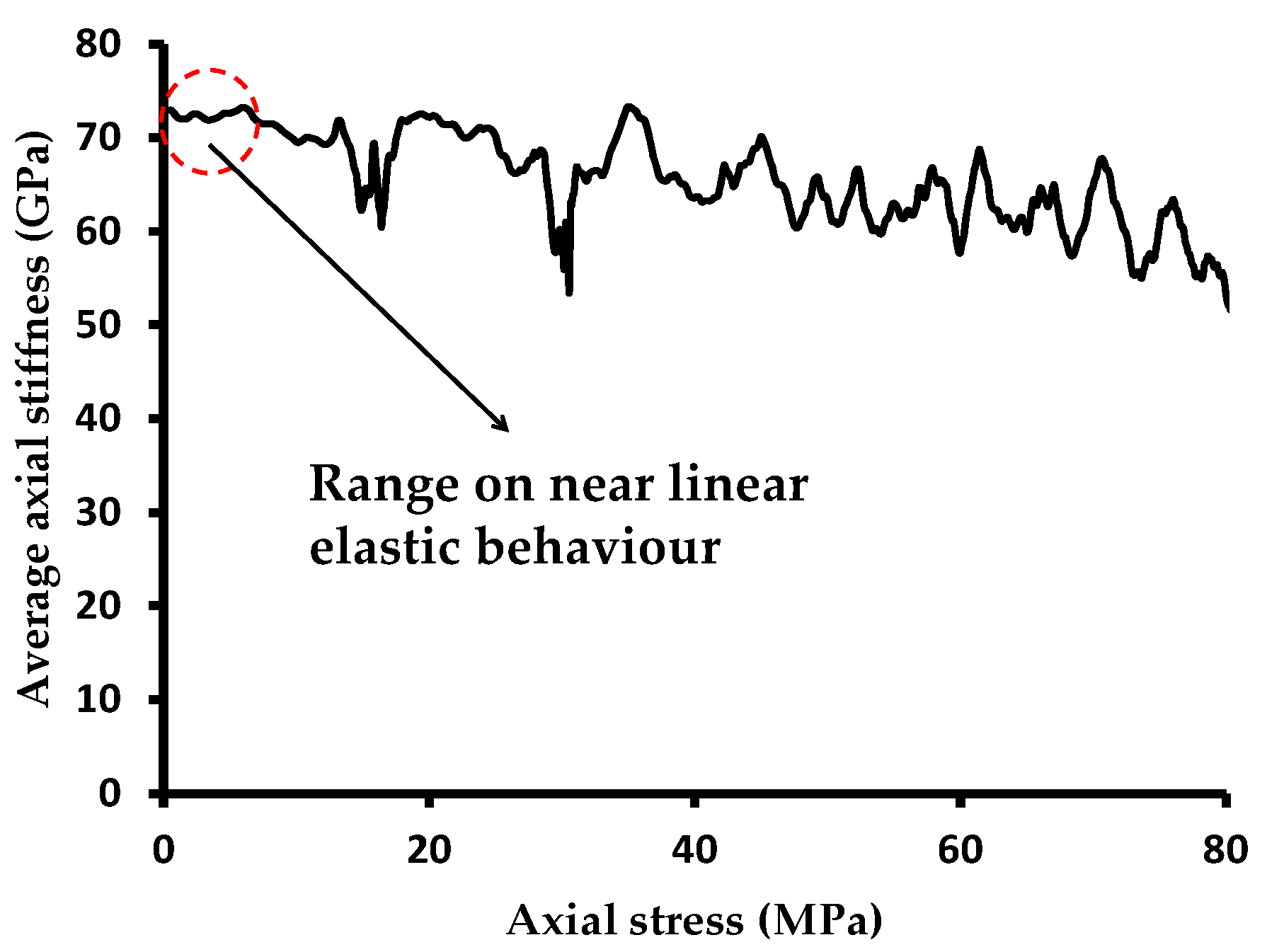
The static Young’s modulus was then calculated as a least-square fit along the near-constant portion of the average axial stiffness–axial stress curve, as shown in Figure 3.

3. Results and Discussion

The descriptive statistics of the deformational properties of prasinites studied are summarized in

Table 1. Dynamical and mechanical properties of prasinites in this study.

	Es (GPa)	Ed (GPa)	UCS (MPa)
Min.	45.6	62.0	88.6
Max.	98.9	106.7	244.1
Mean	75.8	86.4	161.2
S.D. 1	13.4	11.2	40

¹ Standard deviation.

. The table also includes the results for the UCS values, but only for characterization purposes.

According to classification schemes regarding the deformability [12] and the uniaxial compressive strength of the intact rock [13], the prasinites of the study area can be characterized as rocks of low to very low deformability and high to very high strength.

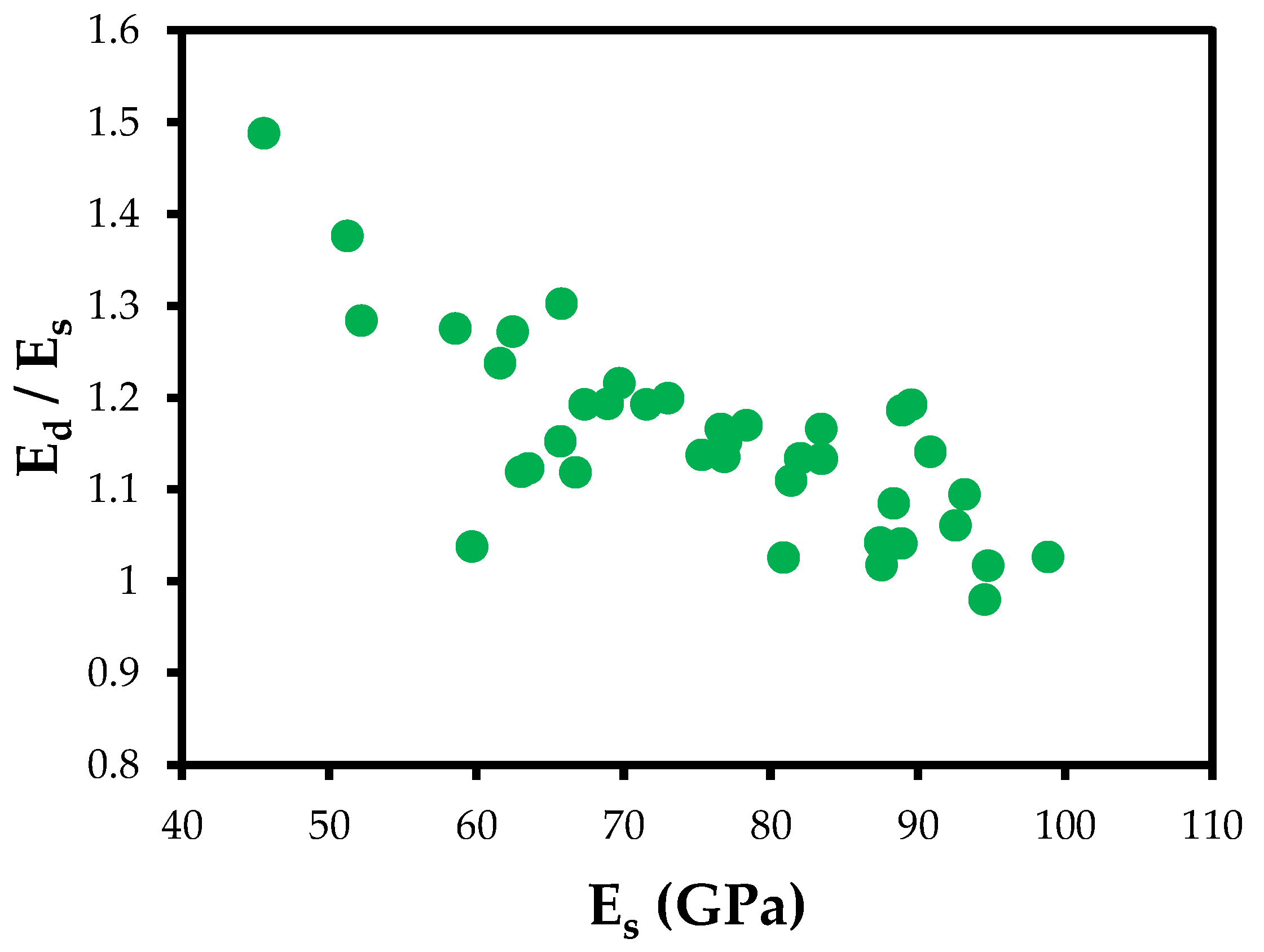
Figure 4 shows the ratio of E_d to E_s for the studied rocks. The ratio varies between 0.98 and 1.49 and tends to have lower values for stiffer specimens. This result agreed with previous findings [2].

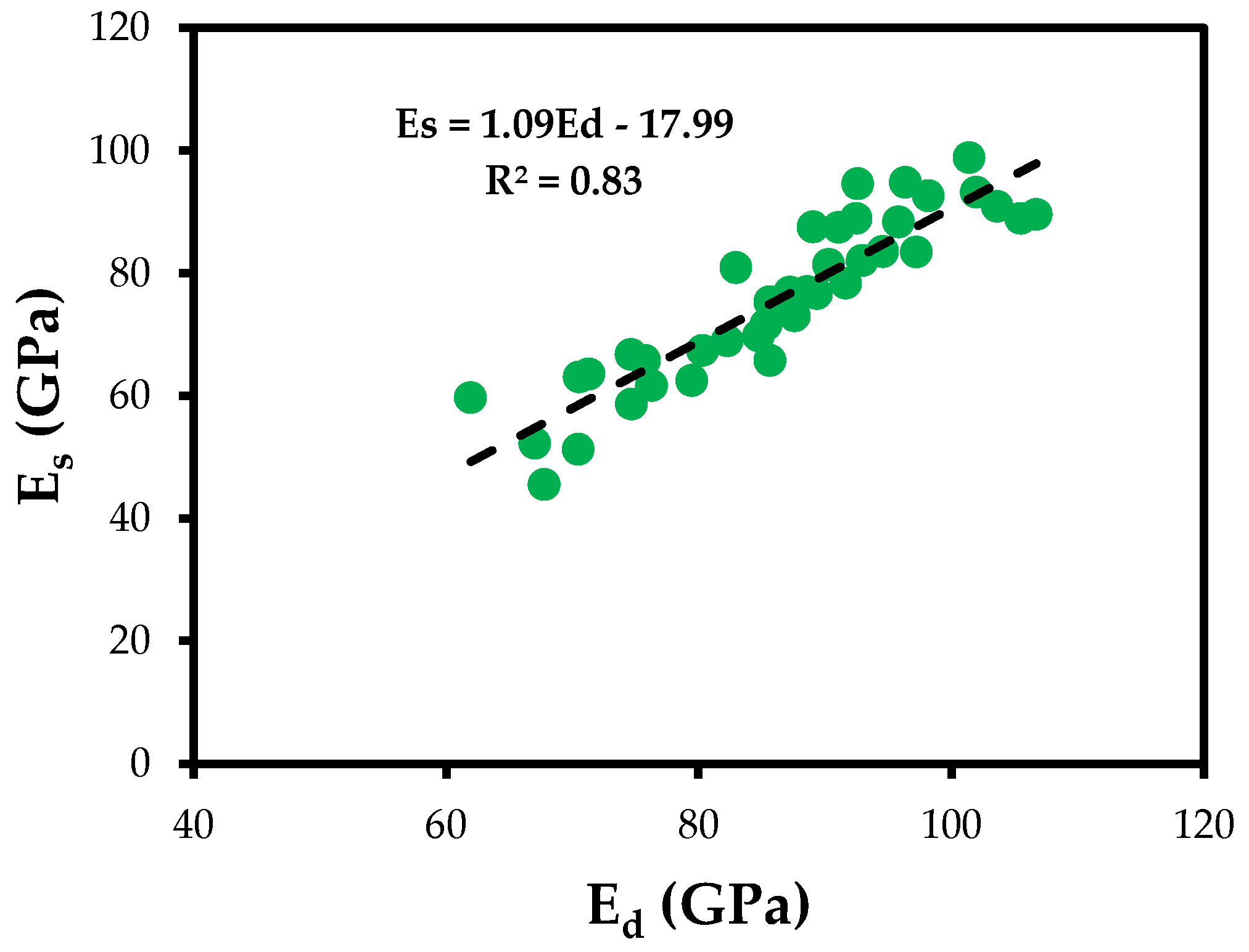
The relationship between static and dynamic Young’s modulus has been investigated for various rock types of sedimentary, metamorphic, and igneous origin [4,5,6,7,8,9,10,11]. Through the results of these studies, linear and non-linear equations were developed with very good coefficients of determination (R²). As illustrated in Figure 5, the relationship between these two properties was also clear for the rocks studied in this work. The empirical relation is characterized by a very good coefficient of determination (R² = 0.83) and is defined by Equation (4).

E_s = 1.09*E_d − 17.99

(4)

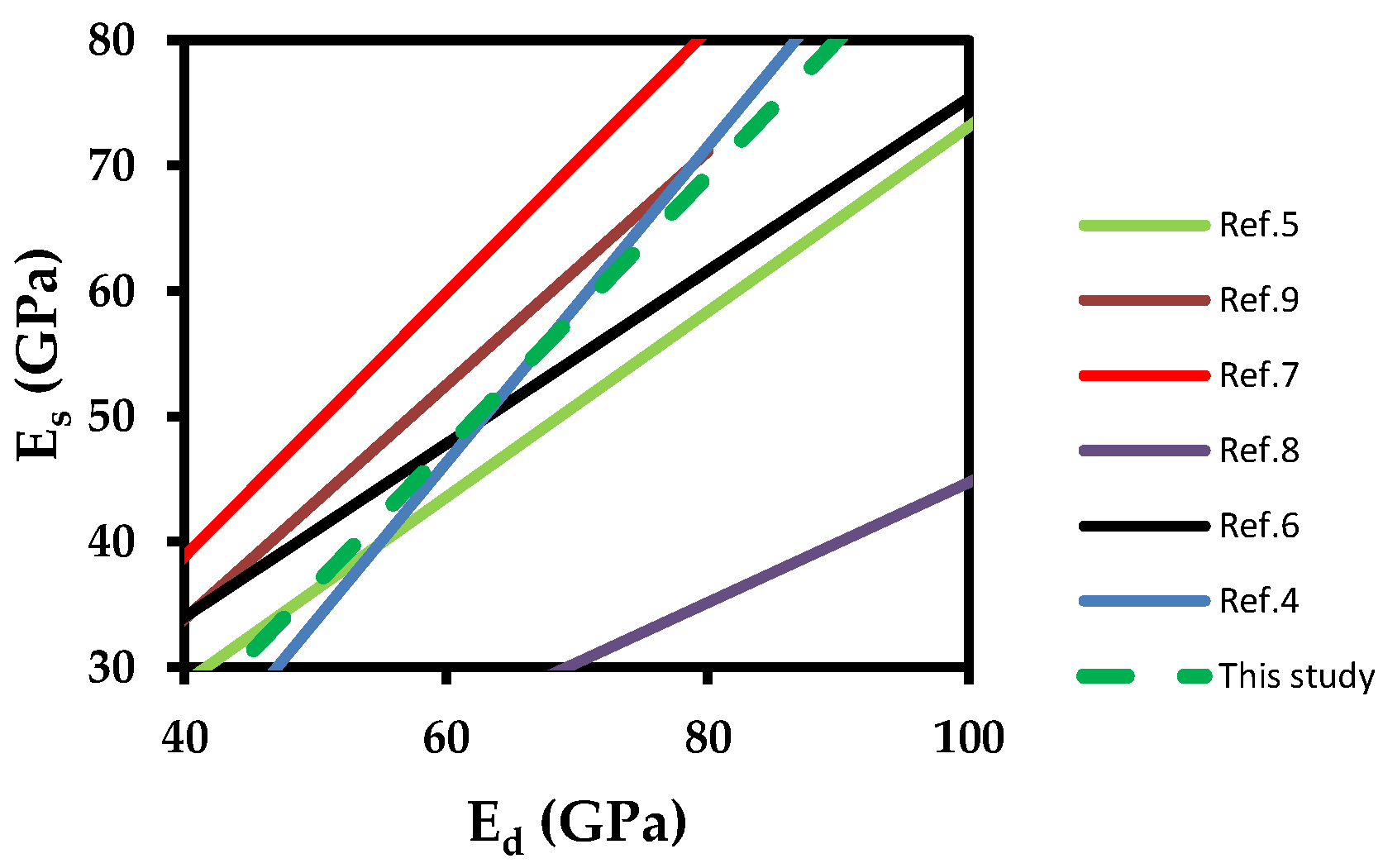
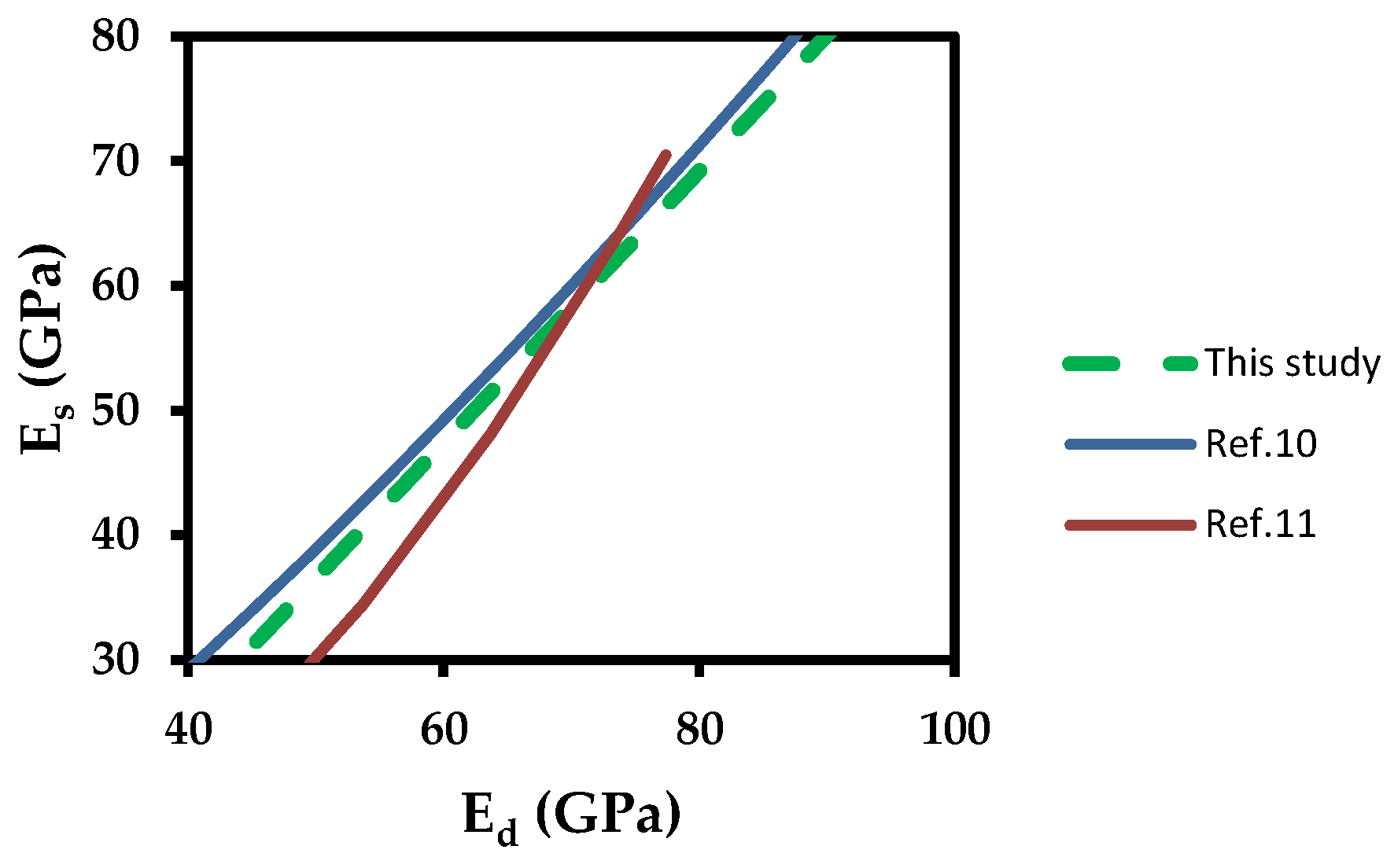
Although the above results confirm the findings of previous studies, the derived mathematical formulations differ from each other, as can been seen in Figure 6 and Figure 7. The random selection of an empirical relationship from the literature may result in underestimation or overestimation of the static Young’s modulus in the study area. The magnitude of these differences seems to depend on the selected equation and the range of the measured values.

4. Conclusions

Bearing in mind that the static methods are time-consuming and costly, it is a challenge to investigate indirect ways of estimating the static Young’s modulus. The purpose of this study was to propose predictive models based on dynamic Young’s modulus values for prasinites.

When applying simple linear regression to the results obtained from the laboratory program, it became clear that E_d was a very good indicator of E_s for this petrological type.

The current findings are in line with the results quoted in the literature, in terms of the applicability of E_d to estimate E_s. However, the derived equations for various rock types are different from each other, suggesting that these relationships are rock type-dependent, a feature that is also frequently reported for relationships between other properties of intact rock.