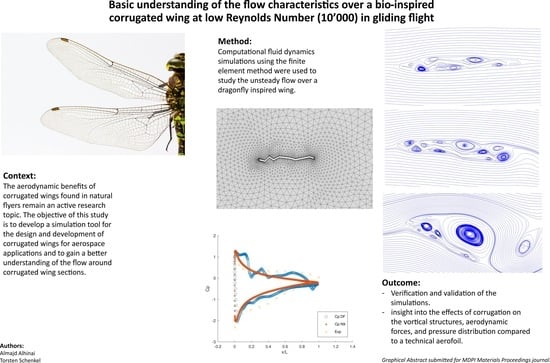
Basic Understanding of the Flow Characteristics over a Bio-Inspired Corrugated Wing at a Low Reynolds Number (10’000) in Gliding Flight †
Abstract
1. Introduction
2. Methods
2.1. Governing Equations
2.2. Solver Setup
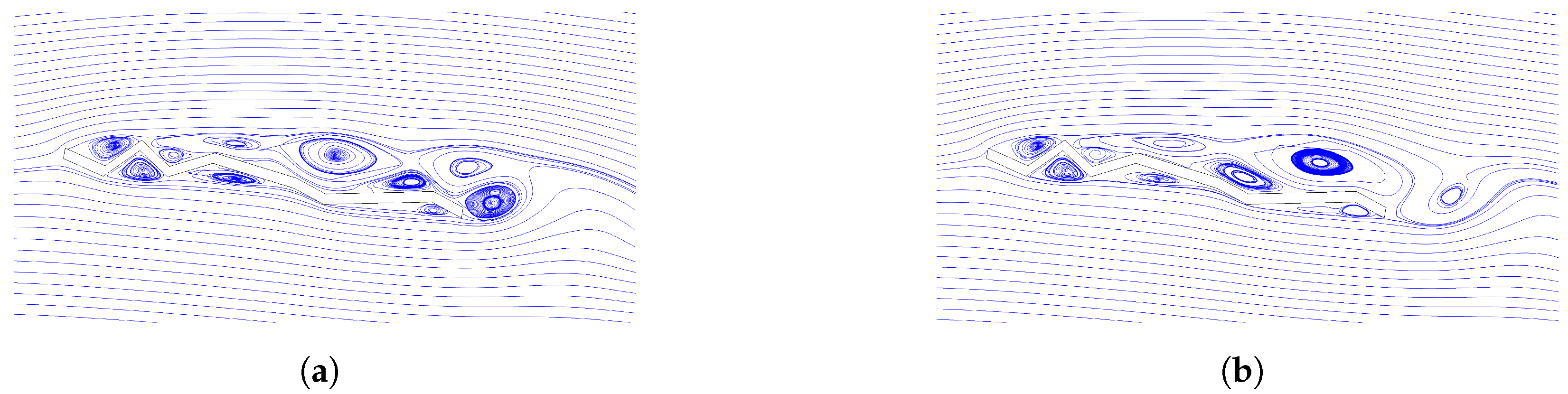
3. Results and Analysis
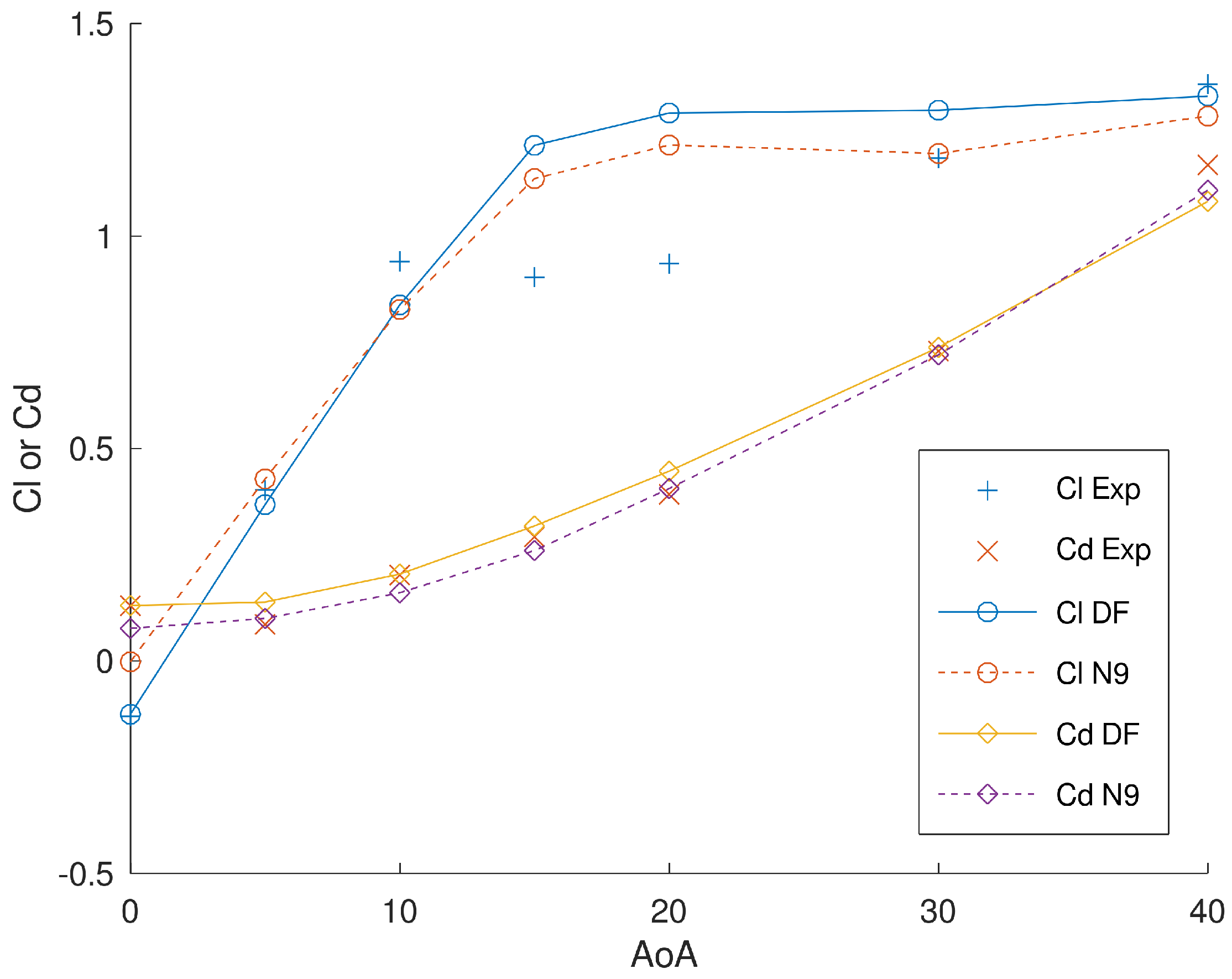
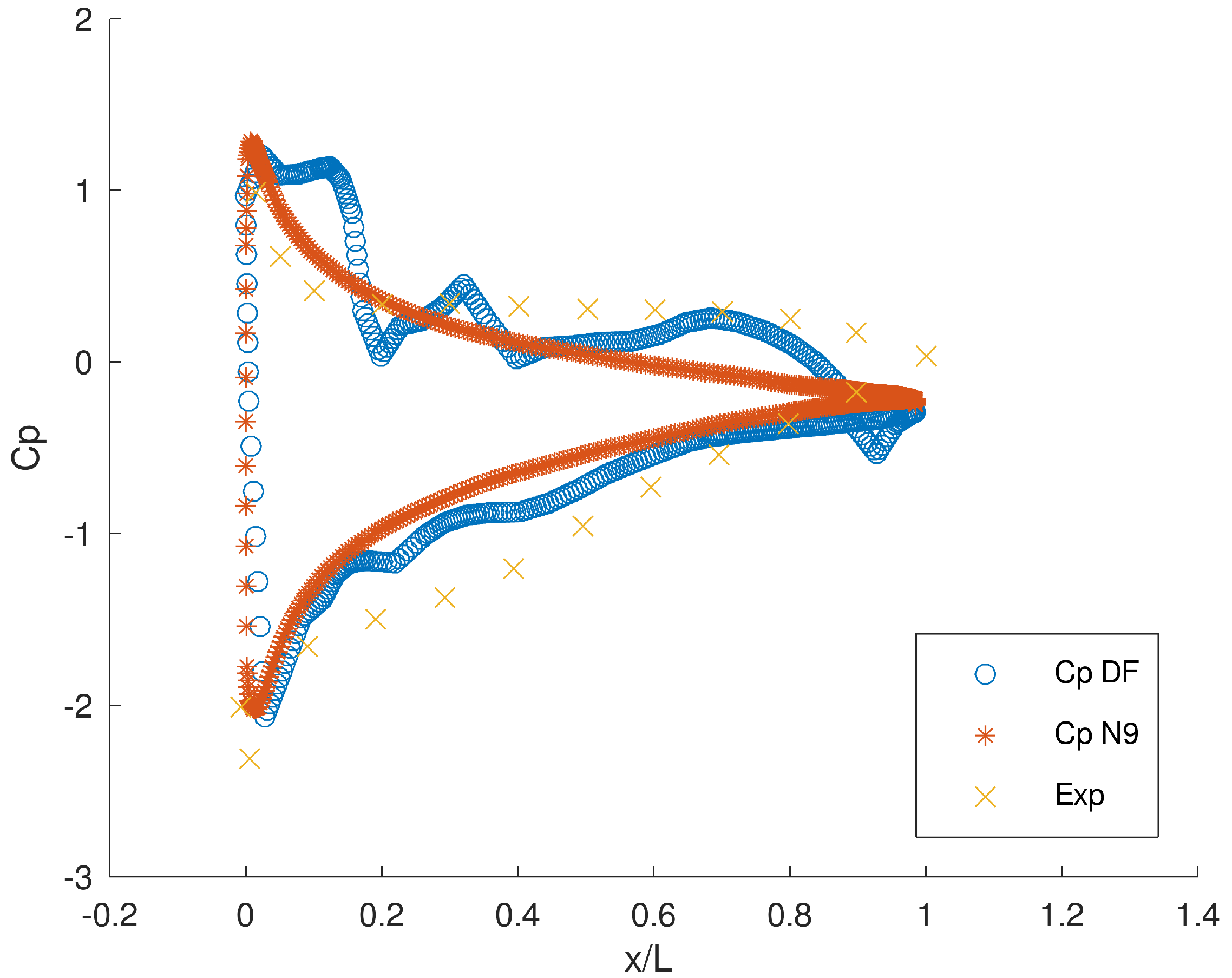
3.1. Verification and Validation
3.2. Angle of Attack Study
3.2.1. Angle of Attack = 0 Degrees
3.2.2. Angle of Attack = 10 Degrees
3.2.3. Angle of Attack = 30 Degrees
3.3. Pressure Distribution
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shyy, W.; Lian, Y.; Tang, J.; Viieru, D.; Liu, H. Aerodynamics of Low Reynolds Number Flyers, 1st ed.; Cambridge University Press: Cambridge, UK, 2009; pp. 22–77. [Google Scholar]
- Zhang, Q.; Xue, R.; Li, H. Aerodynamic Exploration for Tandem Wings with Smooth or Corrugated Surfaces at Low Reynolds Number. Aerospace 2023, 10, 427. [Google Scholar] [CrossRef]
- Rees, C. Form and function in corrugated insect wings. Nature 1975, 256, 200–203. [Google Scholar] [CrossRef]
- Rees, C. Aerodynamic properties of an insect wing section and a smooth aerofoil compared. Nature 1975, 258, 141–142. [Google Scholar] [CrossRef] [PubMed]
- Rudolph, R. Aerodynamic properties of Libellula quadrimaculata L. (Anisoptera: Libelludidea), and the flow around smooth and corrugated wing section models during gliding flight. Odonatologica 1977, 7, 49–58. [Google Scholar]
- Meng, X.; Sun, M. Aerodynamic effects of corrugation in flapping insect wings in forward flight. J. Bionic Eng. 2011, 8, 140–150. [Google Scholar] [CrossRef]
- Kessel, A. Aerodynamic Characteristics of Dragonfly Wing Sections Compared with Technical Aerofoils. J. Exp. Biol. 2000, 203, 3125–3135. [Google Scholar] [CrossRef] [PubMed]
- Vargas, A.; Mittal, R.; Dong, H. A computational study of the aerodynamic performance of a dragonfly wing section in gliding flight. Bioinspir. Biomim. 2008, 3, 026004. [Google Scholar] [CrossRef] [PubMed]
- Winslow, J.; Otsuka, H.; Govindarajan, B.; Chopra, I. Basic Understanding of Airfoil Characteristics at Low Reynolds Numbers (104–105). J. Aircr. 2018, 55, 1050–1061. [Google Scholar] [CrossRef]
- COMSOL Multiphysics® v. 5.0. CFD Module User’s Guide; COMSOL AB: Stockholm, Sweden, 2014; pp. 116–151. [Google Scholar]
- Hughes, T.; Mallet, M. A New Finite Element Formulation for Computational Fluid Dynamics: III. The Generalized Streamline Operator for Multidimensional Advective-Diffusive System. Comput. Methods Appl. Mech. Eng. 1985, 58, 305–328. [Google Scholar] [CrossRef]
- Hauke, G.; Hughes, T. A Unified Approach to Compressible and Incompressible Flows. Comput. Methods Appl. Mech. Eng. 1985, 113, 389–395. [Google Scholar] [CrossRef]
- Schlichting, H.; Gersten, K. Boundary Layer Theory, 8th ed.; Springer: Delhi, India, 2011; pp. 16–18. [Google Scholar]


| DoF | Cl | Cd | ||||
|---|---|---|---|---|---|---|
| 40,971 | −0.152 | 0.091 | ||||
| 68,137 | −0.122 | 0.083 | 0.03 | −0.008 | ||
| 149,886 | −0.121 | 0.111 | 0.001 | 0.028 | 0.033 | −3.5 |
| AoA | Cl | Cl (Exp) | Cd | Cd (Exp) | ||
|---|---|---|---|---|---|---|
| 0 | −0.126 | −0.130 | 3.40 | 0.130 | 0.130 | 0.21 |
| 5 | 0.368 | 0.402 | 8.60 | 0.138 | 0.086 | 37.81 |
| 10 | 0.838 | 0.940 | 10.90 | 0.204 | 0.202 | 1.10 |
| 15 | 1.213 | 0.903 | 25.54 | 0.318 | 0.292 | 8.13 |
| 20 | 1.289 | 0.935 | 27.48 | 0.446 | 0.392 | 12.22 |
| 30 | 1.296 | 1.183 | 8.72 | 0.738 | 0.729 | 1.13 |
| 40 | 1.329 | 1.357 | 2.04 | 1.081 | 1.167 | 7.93 |
| AoA | Corrugated | Aerofoil |
|---|---|---|
| 30 | 0.0067 | 0.0118 |
| 10 | 0.0084 | 0.0311 |
| 0 | 0.0157 | 0.0360 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alhinai, A.; Schenkel, T. Basic Understanding of the Flow Characteristics over a Bio-Inspired Corrugated Wing at a Low Reynolds Number (10’000) in Gliding Flight. Mater. Proc. 2025, 20, 7. https://doi.org/10.3390/materproc2025020007
Alhinai A, Schenkel T. Basic Understanding of the Flow Characteristics over a Bio-Inspired Corrugated Wing at a Low Reynolds Number (10’000) in Gliding Flight. Materials Proceedings. 2025; 20(1):7. https://doi.org/10.3390/materproc2025020007
Chicago/Turabian StyleAlhinai, Almajd, and Torsten Schenkel. 2025. "Basic Understanding of the Flow Characteristics over a Bio-Inspired Corrugated Wing at a Low Reynolds Number (10’000) in Gliding Flight" Materials Proceedings 20, no. 1: 7. https://doi.org/10.3390/materproc2025020007
APA StyleAlhinai, A., & Schenkel, T. (2025). Basic Understanding of the Flow Characteristics over a Bio-Inspired Corrugated Wing at a Low Reynolds Number (10’000) in Gliding Flight. Materials Proceedings, 20(1), 7. https://doi.org/10.3390/materproc2025020007