Evaluation Methodology of Open-Pit Mine Overall Slope Failure Risks ^†

Abstract

Slope failure risks and corresponding probabilities and consequences are related to a large number of causes, thus, their quantification is a complex process. The semi-qualitative method FMEA was modified into a quantitative value method V-FMEA for specific mining requirements, which enabled the risk assessment to perform a constant check of acceptable probabilities of the overall slope failure and the implementation of detection and preventive activities as functions of the present value of consequence costs. The large open-pit mine Field E slope failure served as a good example of the proposed new risk evaluation methodology.

1. Introduction

In all phases of operation and development of an open-pit mine, there is a risk of an unforeseen random occurrence of a mining process failure with significant and even catastrophic consequences [1]. Risks arise from a series of natural–external, technical, and technological–internal characteristic variables.

The stability of slopes in open-pit mines is extremely important considering the safety, economic, and environmental aspects [1,2], which include a series of discrete stochastic processes and relatively frequent changes in the internal and external environment [3,4]. The influence of unstable ground in the slope zone of the surface mine must not be ignored because it can lead to temporary or permanent closure of the mine.

One of the generally accepted and most frequently applied methods for risk management is the FMEA (Failure Mode and Effect Analysis) method [5,6,7,8,9]. The risk ranking is most often shown through risk matrices with the risk priority numbers (RPN), by multiplying S (Severity), O (Occurrence), and D (Detection) modes (RPN = S × O × D). However, it should be noted that the conventional methods of FMEA suffered a lot of criticism from the scientific public, especially referring to the standard applied expert subjective procedure of relative risk ranking and prioritization via the RPN number.

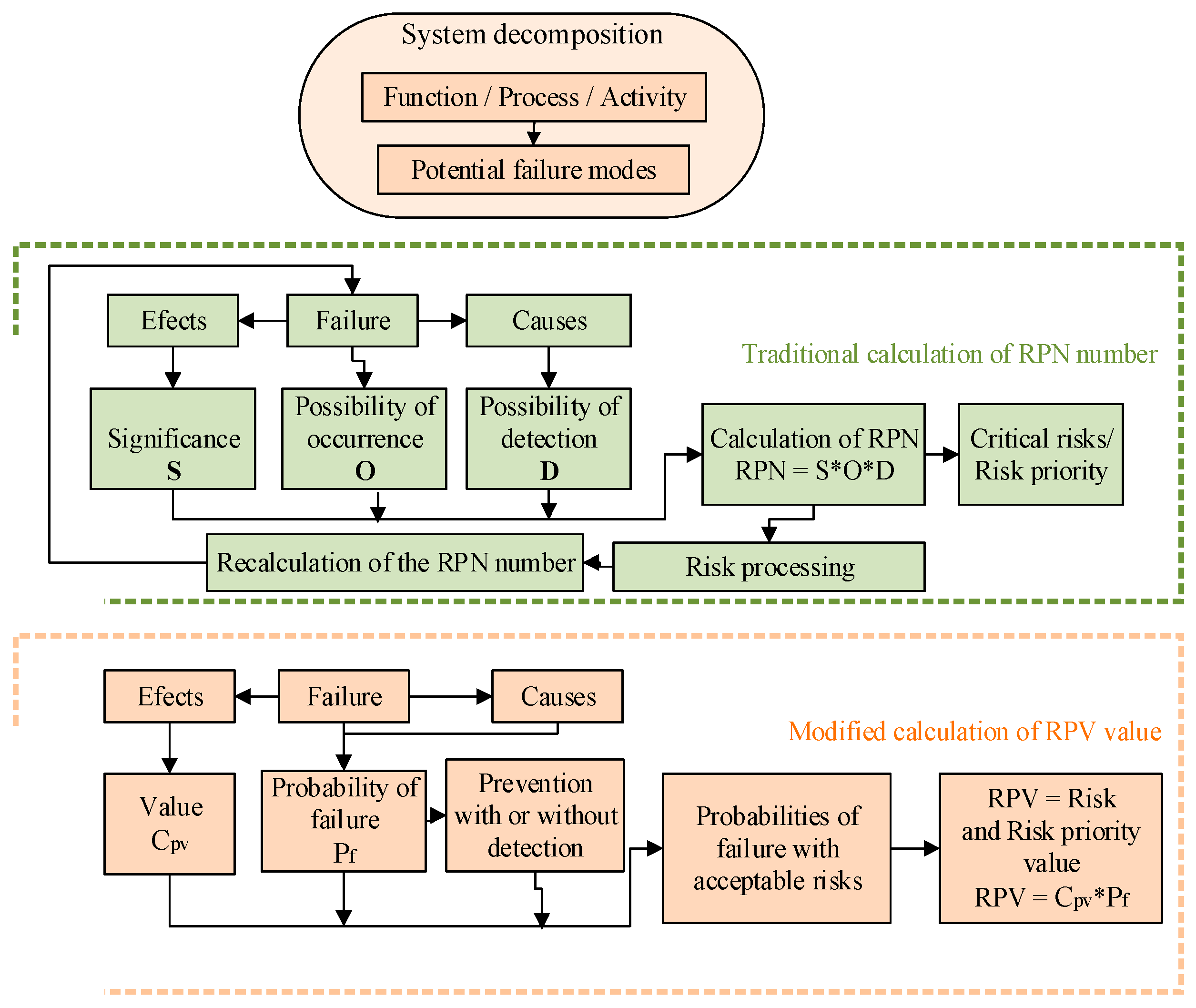
The quantitative method V-FMEA (Value-Failure Mode and Effect Analysis) is modified in comparison to the conventional method FMEA in regard to risk assessment. There, a risk priority value (RPV) is proposed. V-FMEA is based on the reliability theory and maintains the simplicity of the FMEA method while eliminating the criticized shortcomings of conventional RPV. Figure 1 shows a methodological comparison of the FMEA method and the new modified V-FMEA method.

The open-pit mine Field E is situated in the Kolubara coal basin in Serbia. Evaluations of the Field E overall slope failure risks were carried out for the condition before its occurrence, in the rehabilitation phase after its occurrence (Figure 2a) and also for future mine development.

2. Materials and Methods

Analysis of the risk (R) of failure of random eco-processes, facilities or equipment in open-pit mining is methodologically based on the basic and well-known principle, same as FMEA, with an addition of a valuable quantitative approach with the analysis of possible variants of failure probability P_f. The value of risk is obtained by multiplying the probability of failure and the corresponding present monetary value of the consequences on the functioning and economy of a mine for any cause and for each variant (C_PV), which, simultaneously with its size, defines the priority in taking measures for elimination or reduction of risk [4,10,11]:

RPV = P_f × C_PV,

(1)

Stable and safe ecosystem probability prior to the time of analysis, or prior to the appropriate preventive activities can be defined by the most-used exponential distribution expressed over the appropriate time of stable and safe functioning until failure (T_s) [10]: P_s (t) = exp(−a × t_a), where: t_a—planned time of eco-system reliability analysis and a—distribution parameter (failure intensity). The average time until the failure of an open-pit mine eco-system T_s is: T_s = ∫P_s(t)dt = ∫exp(−a × t_a)dt = 1/a. That means:

a = 1/T_s,

(2)

On the other hand, the risk or probability of failure after time t, according to the exponential distribution law, amounts to: P_f (t) = 1 − exp(−a × t_a) = 1 − P_s(t).

2.1. Probabilities of Failure Occurrence According to the Exponential Law

If the continuous random variable of any failure cause/influencing factor N_i (i = 1, …, n) as event T_si at time t has a negative exponential distribution with parameter a_i, then its density is: f_i(t) = a_i × exp(−a_i × t), t > 0. If it is certain that no failure occurred in time t_pi until the successful execution of preventive activities, then the probability of that event is (Figure 2): P_pi = 1 − ∫₀^t_pi f(t)dt = exp(a_i × t_pi). Then, the conditional density f_pi(t) of the time remaining until the analysis time is: f_pi(t) = f_i(t)/P_pi. From this, it can be concluded that: f_pi(t) = a_i × exp(−a_i × t)/exp(−a_i × t_pi) = a × exp(−a_i(t_ai − t_pi)), t_ai > t_pi.

The time interval (t_a − t_pi), as a random variable, has the same but conditional density as the random event T_si for ta > 0 (Figure 2b). The conditional distribution of the time remaining until the failure of this random variable does not depend on the previously elapsed time, which is characteristic of the exponential distribution with a continuous random variable, the exclusive property of being memoryless.

There are two paths for calculating the probability of failure of elements or the eco-system of open-pit mines as a whole: Path 1—without detection and preventive activities (P_fs) and Path 2—after successful preventive activities with or without detection (P_fp) for any cause N_i. Without the possibility of implementing preventive activities (t_p = 0), the detection time of a possible system failure was ignored as irrational, and Equation (4) was reduced to Equation (3). In conclusion, Path 1 (Figure 2b) is:

P_fi = 1 − exp(−a_i × t_ai),

(3)

When preventive activities are successfully carried out in time t_pi with or without detection (t_d = 0), and depending on the adopted acceptable risk, the probability of failure for Path 2 (Figure 2b) is:

P_fpi = 1 − exp(−a_i(t_ai − t_pi)),

(4)

2.2. Open-Pit Mine Eco-System Failure Consequences

Total expected costs Ci for each cause of failure Ni represents the sum of all types of costs M_j (j = 1, …, m) and generally amount to [4,11,12]:

C_ij = C_pi1 + C_si2 + C_ri3 + C_li4 + C_hij5 + C_dpi +…+ C_im = ∑ _{j = 1}^m C_ij, (Euro),

(5)

where: C_p—preparatory organizational costs after failure, C_s—operational costs of removing all direct failure consequences, C_r—renewal of facilities and equipment, C_l—possible costs due to losses in production, C_h—costs of endangering health and safety, and C_dpj—the necessary costs of detection and prevention.

The dynamics of the occurrence of failure costs are variable over time, so the financial impact on the costs incurred in this way is very important for risk assessment [2,4]. Therefore, it is good to use present value (PV—Present Value) in the calculations for the total costs (C_i) over a time of n years and with the interest rate of return (r) through the well-known equation:

PV_i = C_i/(1 + r)ⁿ,

(6)

2.3. Maximum Acceptable Failure Risks

If RPV_max is the maximum acceptable risk of failure of one of the elements in the eco-system, and if P_fmax is its maximum acceptable probability of failure, then the limit probability of failure according to the basic traditional risk equation (1) is: P_f ≤ P_fmax = RPV_max/C_PV. This means that the limit probability of failure must be less than or equal to the maximum acceptable share of risk in relation to the total costs due to the failure of the corresponding cause [2,7,11].

Determining the execution time of preventive activities t_p if there is no detection (Path 2) is crucial for the analysis of acceptable risks. Depending on the minimum acceptable system reliability in time t, expressed as (4), when (t_ai − t_pi) = −ln P_smaxi/a_i, then the acceptable probability of failure is: P_fpmaxi = 1 − exp(ln P_smaxi)

The time of executing preventive activities in time t for function f_i(t) amounts to:

t_pi = (−ln P_smaxi)/a_i.

(7)

The planned start of prevention in time t is determined by subtracting the mean value of the prevention duration T_pi from the time t_pi (Figure 2b). The final risk calculation is performed according to Equation (1) for all causes and paths with established acceptable risks.

3. Results

Although the open-pit mine Field E overall slope was designed following the current legal regulations in the field of mining, an unwanted overall slope failure occurred (Figure 3b). The worst consequences were large amounts of collapsed overburden material, coal losses, equipment damage, and the drop in overburden excavation, but the workshop facilities for auxiliary equipment, the assembly site under construction, the electrical substation, the displaced riverbed, and the road were also endangered.

The four most common causes of slope failure are the reliability of geological parameters as a basis for the slope design process, the degree of implementing planned mining technology and dewatering activities, as well as the occurrence of extreme rainfall as a random process (

Table 1. Estimated consequences as costs and losses due to failure (M€).

Costs/Losses	Cpj	Csj	Crj	Clj	Chj	∑j=1m Cj	∑j=1m Cdp
Geological data and design	0.1	1.4	-	-	-	1.5	1.4
Wrong mining technology	0.2	2	0.7	2	0.1	5	2
Wrong dewatering system	0.3	2.5	1	2	0.2	7	3
Extreme weather	0.5	5	2	4	0.5	12	4

,

Table 2. Risk evaluation and ranking by causes of failure without preventive activities (initial risk).

Cause/Process	ai (2)	Pfi (3)	Ci (M€) (5)	RPVi (M€) (1)	Risk Priority
Geological Input data/Design	0.50	0.78	1.5	1.17	3
Technology/Mining	0.25	0.53	5	2.65	4
Water/Dewatering	0.33	0.63	7	4.41	5
Extreme weather	0.05	0.14	12	1.68	3

and

Table 3. Risk mitigation and ranking with successful preventive activities.

Cause/Process	ai (2)	tpi (7)	CPVi (M€) (6)	RPVi (M€) (1)	Risk Priority
Geological input data/Design	0.50	0.71	1.05	0.32	2
Technology/Mining	0.25	1.43	5.26	1.58	3
Water/Dewatering	0.33	1.05	7.52	2.27	4
Extreme weather	0.05	3	12	1.68	3

). All causes relate to an appropriate time-dependent cost of failure consequences.

The subsequent geomechanical analysis of the condition before slope failure shows great instability of the overall slope because Factor of Safety FS = 0.9 (Figure 3a) even with the minimum value of pore pressure Ru = 0.2. According to the Serbian state Rulebook (2010), the FS from 1.3 to 1.5 is allowed. It was concluded that for the overall slope stabilization, it was necessary to build a supporting embankment in order to achieve overall slope stability of FS = 1.3, under a slope angle of 9 degrees for a height of 50 m. The necessary volume of bulk material was about V = 1,500,000 m³. The calculated amount of coal loss in this mine boundary was about 700,000 t.

The preliminary stability check showed that for the next phase of mining to the west, the overall slope angle should not be greater than 8 degrees for the total overall slope height of 60 m when FS = 1.5 (Figure 3b) with the implementation of proper mining technology with an active and adequately supplemented dewatering system. In terms of the operating parameters, the mine should be further developed based on a completely new mining project.

The estimated consequences of costs and losses are shown in Table 1, where the four previously mentioned important possible causes of slope failure are highlighted.

A preliminary initial risk analysis with risk ranking was conducted for the phase before the occurrence of slope failure with recognized consequence values C_i (Table 1) and for the option without detection and preventive activities (Path 1) (Table 2). The option with risk mitigation and ranking was also examined, which included Path 2 with the successful implementation of preventive activities with and without successful detections (Table 3) with consequence values C_dp (Table 1). The selected analysis time for all causes was t_a = 3 years, and the interest rate of return (6) was r = 10%. The maximum acceptable probability of failure (P_fmaxi = 0.3) was used for each of the causes of failure (1). In

Table 4. Distribution into priorities, risks intervals and description of risk values (M€).

Priority 1	Estimated risks in the interval 0 < RPV ≤ 0.2	Very low risk
Priority 2	Estimated risks in the interval 0.2 < RPV ≤ 0.5	Low risk
Priority 3	Estimated risks in the interval 0.5 < RPV ≤ 2	Medium risk
Priority 4	Estimated risks in the interval 2 < RPV ≤ 4	High risk
Priority 5	Estimated risks in the interval RPV > 4	Very high risk

, a description of the distribution into priorities, risk intervals, and description of risk values (M€) is given, with risk priority for preventive activities as well as in the FMEA method.

The obtained results indicate a significant reduction of risk in Path 2 for all failure causes (Table 3), which was employed for risk mitigation and ranking with successful preventive activities in relation to Path 1 (Table 2). The water and dewatering design represents the highest priority with high risk (Table 3 and Table 4), which in the initial risk option Path 1 had very high risk (Table 2) and demands maximum technical activities with serious investments. Therefore, in the Path 2 option, funds for preventive activities of 3 M€ are provided (Table 1) in order to reduce the risk of occurrence of this cause.

4. Discussion

As a part of the analysis of the reliable operation of the opencast mines as an eco-system, the risk evaluation method V-FMEA was effectively applied to the determination of open-pit coal mine Field E overall slope failure risks. Two options (Path 1 and Path 2) were analyzed for the four selected important possible causes. The probability of failure was calculated with the established acceptable maximum probability of slope failure and the present value of consequences/costs of full slope sanation. Thus, when potential failure modes are identified, preventive and corrective action can be taken to eliminate them or to continually reduce the potential occurrence.

As an example, with extensive measures taken, the biggest priority risk of failure due to the weaknesses in the dewatering system was reduced by 52% in the Path 2 option with the possibilities of preventive activities, compared to the total cost in the Path 1 option without detection and preventive activities.

It was also suggested that an urgent geotechnical and hydro-geological investigation of the entire area must be carried out and a monitoring system should be installed in order to respond in a timely manner in case of detection of land sliding occurrences.

The modified V-FMEA method, compared to the FME(C)A method, is fully aligned with the set Risk Management Process in the overall structure and approach of the ISO 31,000:2018 standard and the recommended traditional FME(C)A method while removing or mitigating its shortcomings.

While a valuable tool in various fields, including mining, no method is without flaws, and V-FMEA is no exception:

• The accuracy and reliability of quantitative analysis depends heavily on the quality of the data used, which in geology and mining are extremely variable random variables, and their expert analysis and adoption requires good practical and theoretical knowledge of external and internal hard-to-predict influencing parameters, in space and time of each deposit and each mine;
• When using many variables that are often difficult to predict spatially and temporally, there is a risk of overfitting the data and poor generalizing;
• Sensitivity to assumptions such as normality of data distribution or independence of variables. If these assumptions are violated, the results can be misleading;
• Experts may manipulate data or choose data points selectively to support a particular hypothesis or agenda, leading to biased results;
• The variables’ sensitivity to change is pronounced, but also common for stochastic processes in mining and was included in the assessment of acceptable risks;
• Human errors in data entry, analysis or interpretation can introduce significant flaws into quantitative analysis.

It is essential to be aware of these limitations and exercise caution when interpreting quantitative results, and use the practical experience of good experts in the field of geology and mining.

The V-FMEA method provides promising results, taking into account the specific requirements of the mining industry in the domain of risk management, and eliminates or at least reduces the effect of the shortcomings of the conventional FMEA method with the risk priority metric as a priority decision with optimization possibilities.