Design of a Light-Weight Vertical Tail for a Hybrid High-Altitude Pseudo-Satellite †
Abstract
1. Introduction
2. Materials and Methods
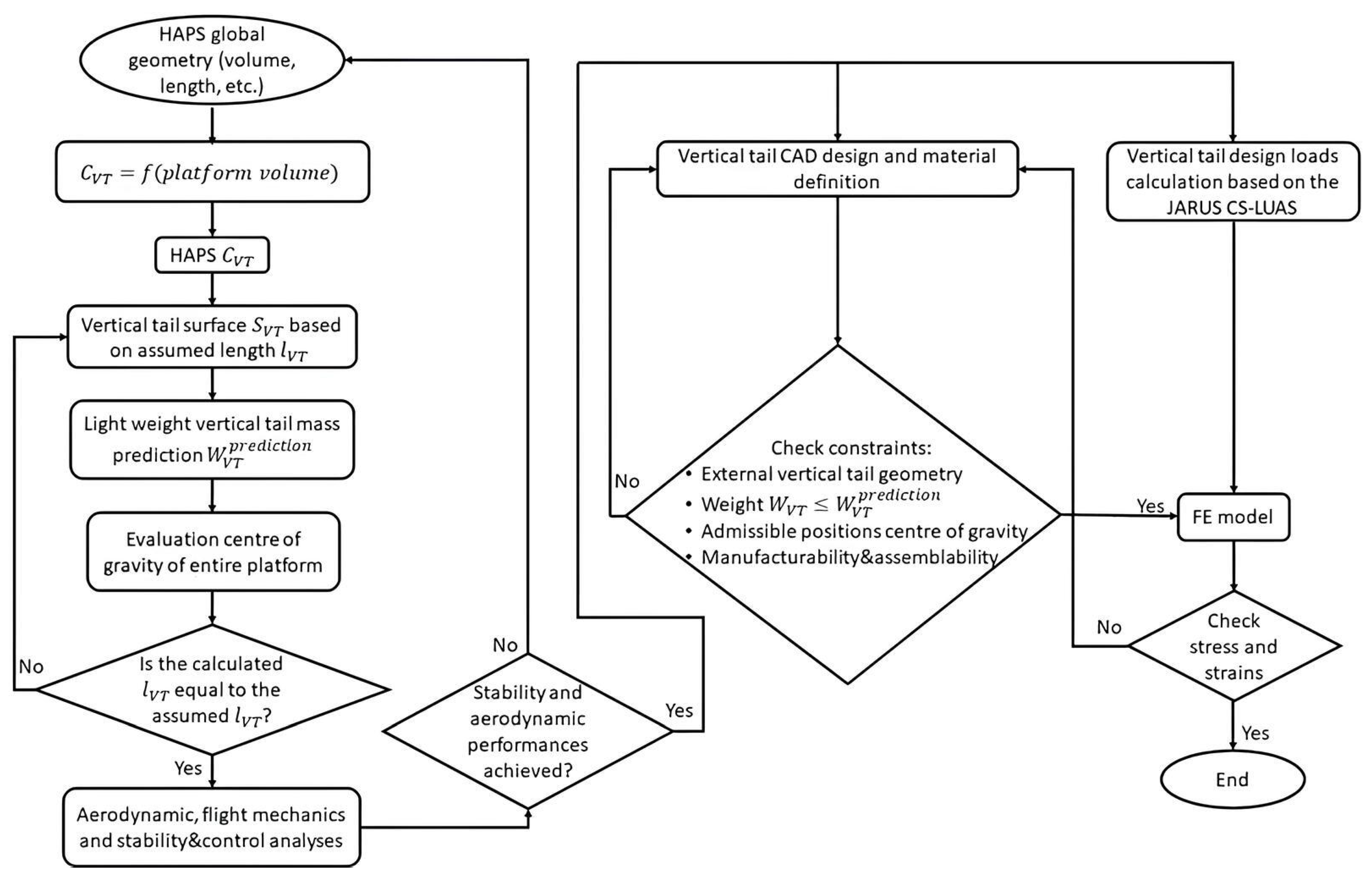
2.1. Design Process
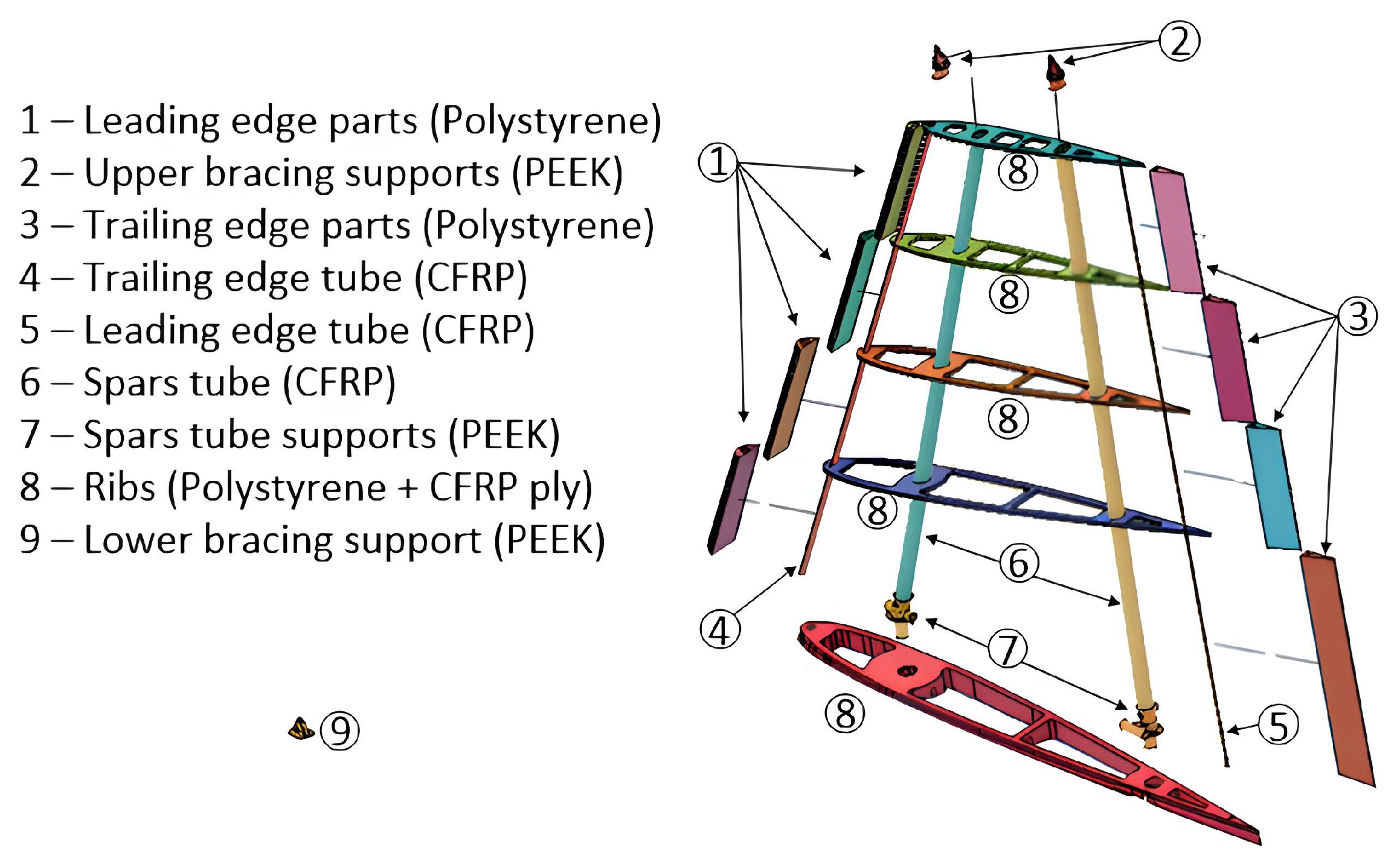
2.2. CAD Model
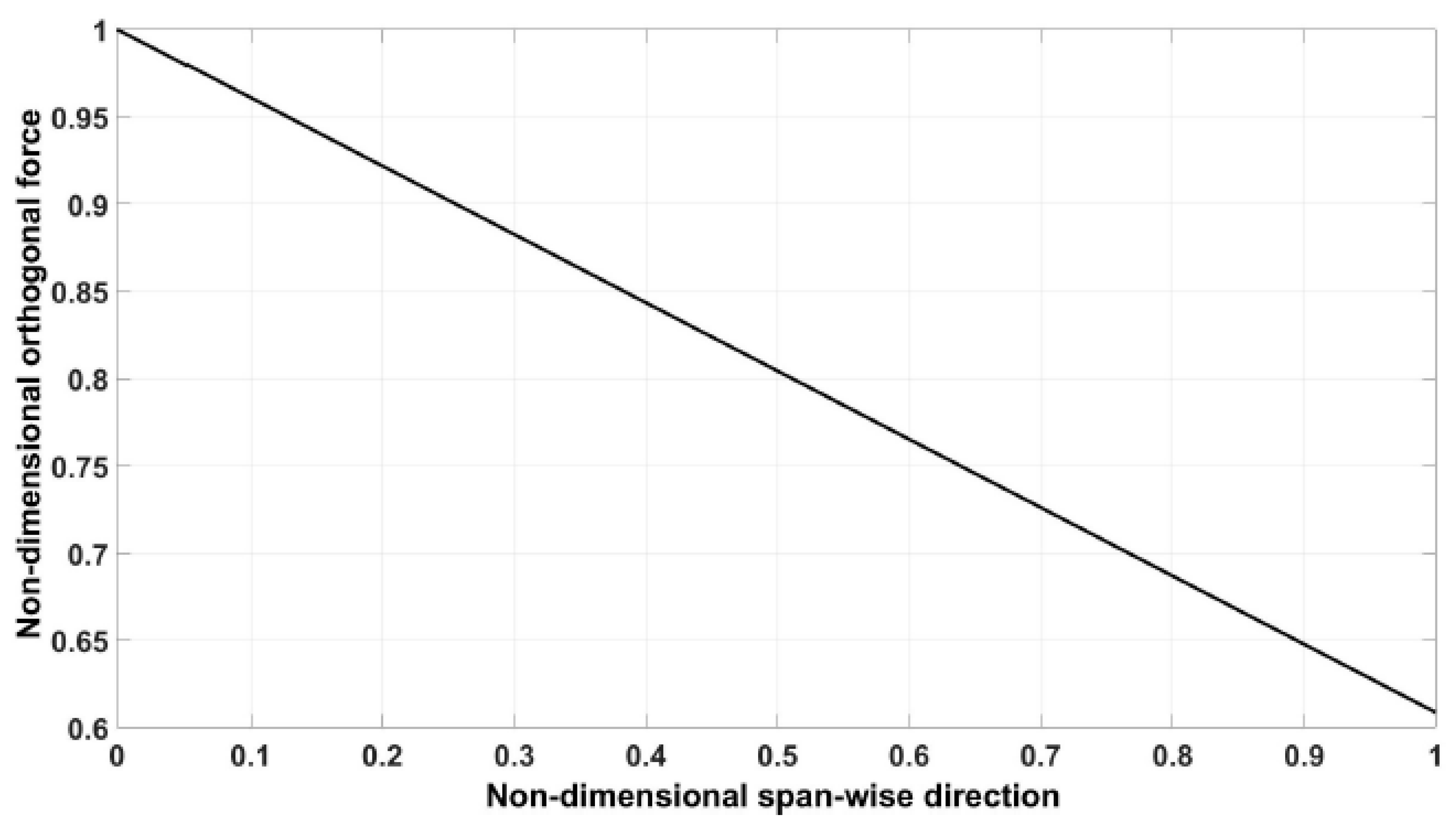
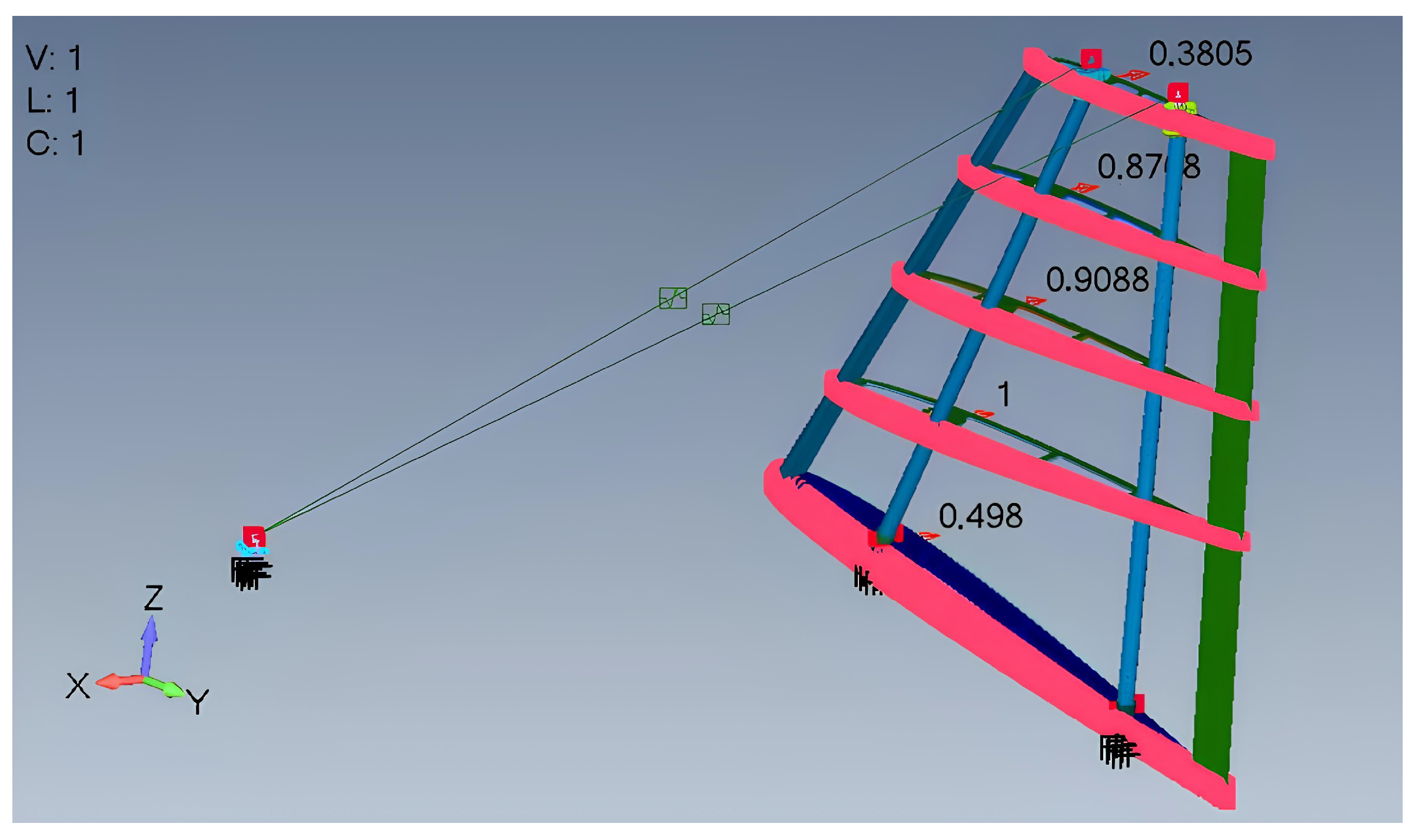
2.3. FE Model
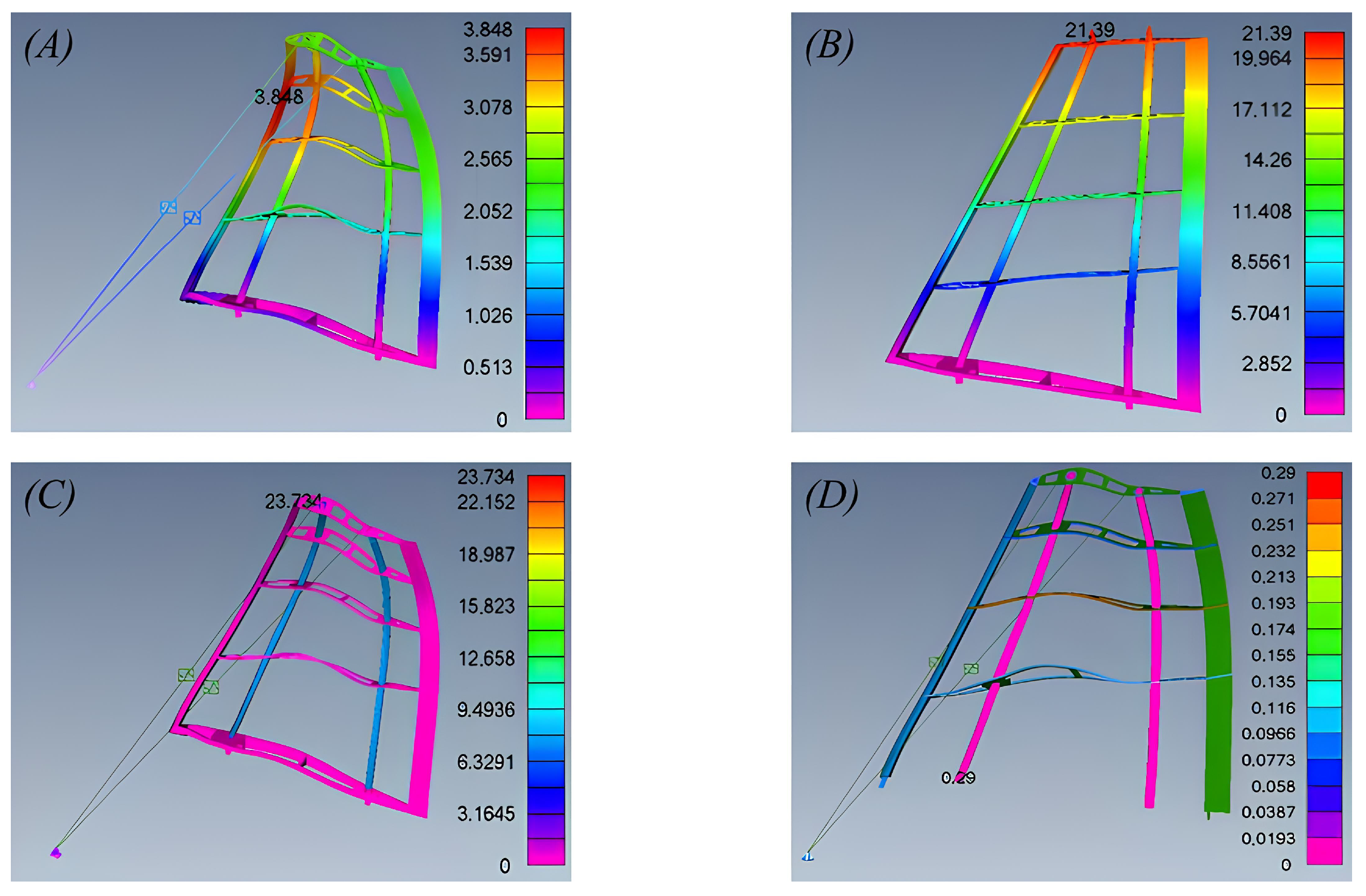
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Baraniello, V.R.; Persechino, G.; Borsa, R. Tools for the Conceptual Design of a Stratospheric Hybrid Platform. In SAE Technical Paper; No. 2020-01-0025; SAE International: Warrendale, PA, USA, 2020. [Google Scholar]
- Araripe d’Oliveira, F.; Cristovão Lourenço de Melo, F.; Campos Devezas, T. High-altitude platforms—Present situation and technology trends. J. Aerosp. Technol. Manag. 2016, 8, 249–262. [Google Scholar] [CrossRef]
- Gonzalo, J.; Lopez, D.; Dominguez, D.; García, A.; Escapa, A.; Borsa, R. On the capabilities and limitations of high altitude pseudo-satellites. Prog. Aerosp. Sci. 2018, 98, 37–56. [Google Scholar] [CrossRef]
- Sinko, J. High altitude powered platform—A microwave powered airship. In Proceedings of the 3rd Lighter-Than-Air Systems Technology Conference, American Institute of Aeronautics and Astronautics, Palo Alto, CA, USA, 11–13 July 1979. [Google Scholar]
- De Grado, J.G.; Tascon, C.S. On the development of a digital meteorological model for simulating future air traffic management automation. In Proceedings of the 2011 20th IEEE International Workshops on Enabling Technologies: Infrastructure for Collaborative Enterprises Art, Paris, France, 27–29 June 2011; pp. 223–228. [Google Scholar]
- Khoury, G.A. Airship Technology, 2nd ed.; Cambridge University Press: New York, NY, USA, 2012; p. 5. [Google Scholar]
- ul Haque, A.; Asrar, W.; Sulaeman, E.; Omar, A.; Ali, J.S.M. Pugh Analysis for Configuration Selection of a Hybrid Buoyant Aircraft. Technical Report. In SAE Technical Paper; SAE International: Warrendale, PA, USA, 2015. [Google Scholar] [CrossRef]
- Gudmundsson, S. Requirements for Static Directional Stability, In General Aviation Aircraft Design: Applied Methods and Procedures; Elsevier: Amsterdam, The Netherlands; Publishing House: Oxford, UK, 2014; pp. 460, 476–477. [Google Scholar]
- Grant Carichner, E.; Leland Nicolai, M. Subsystems and Weights, In Fundamentals of Aircraft and Airship Design, Volume II—Airship Design and Case Studies; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2013; Chapters 7, 9, 10. [Google Scholar]
- Etkin, B.; Reid, L.D. Dynamics of Flight: Stability and Control; John Wiley & Sons: Hoboken, NJ, USA, 1995; pp. 79–93. [Google Scholar]
- Farner, M. JARUS CS-LUAS. Recommendations for Certification Specification for Light Unmanned Aeroplane Systems; JAR_DEL_WG3_D.04. Available online: http://jarus-rpas.org/wp-content/uploads/2023/06/jar_07_doc_CS_LUAS.pdf (accessed on 2 September 2024).
- Zhai, H.; Euler, A. Material challenges for lighter-than-air systems in high altitude applications. In Proceedings of the AIAA 5th Aviation, Technology, Integration, and Operations Conference, Arlington, VA, USA, 27 September 2005. [Google Scholar]
- Hexcel Corporation. Prepreg Data Sheet. HexPly 8552. Available online: https://www.hexcel.com/user_area/content_media/raw/HexPly_8552_eu_DataSheet(1).pdf (accessed on 2 September 2024).
- Matweb. Overview of Materials for Polyetheretherketone. Unreinforced. Available online: https://www.matweb.com/search/datasheet_print.aspxmatguid=2164cacabcde4391a596640d553b2ebe (accessed on 2 September 2024).
- Matweb. Overview of Materials for Polystyrene, Molded. Unreinforced. Available online: https://www.matweb.com/search/datasheettext.aspx?matguid=48055b1b098041ba8e8eb06c64064e93 (accessed on 2 September 2024).
- Obert, E. Aerodynamic Design of Transport Aircraft; IOS Press: Amsterdam, The Netherlands, 2009; pp. 413–422. [Google Scholar]
- Oskar, S. A simple approximation method for obtaining the spanwise lift distribution. Aeronaut. J. 1941, 45, 331–336. [Google Scholar]
- Kamal, N.N.M.; Basri, A.A.; Basri, E.I.; Basri, I.S.; Abas, M.F. Comparison study between Schrenk’s approximation method and computational fluid dynamics of aerodynamic loading on UAV NACA 4415 wing. J. Adv. Res. Fluid Mech. Therm. Sci. 2019, 64, 283–292. [Google Scholar]
- Tsai, S.W.; Wu, E.M. A General Theory of Strength for Anisotropic Materials. J. Compos. Mater. 1971, 5, 58–80. [Google Scholar] [CrossRef]


| Geometrical Parameter | Value |
|---|---|
| Geometric mean chord | 1.3 [m] |
| 75.0° | |
| / | 1.2 |
| Material | Young Module [MPa] | Young Module [MPa] | Poisson’s Ratio | Density [kg/m3] |
|---|---|---|---|---|
| Prepreg | 163,000.0 | 12,000.0 | 0.28 | 1570.0 |
| PEEK | 3600.0 | - | 0.35 | 1320.0 |
| Polystyrene | 3200.0 | - | 0.35 | 1021.0 |
| Dyneema | 44,000.0 | - | - | 970.0 |
| Structure | Weight [kg] |
|---|---|
| Light-weight classical | 3.47 |
| Innovative proposed | 1.10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Biase, F.; Varricchio, D.; Baraniello, V.R.; Persechino, G. Design of a Light-Weight Vertical Tail for a Hybrid High-Altitude Pseudo-Satellite. Eng. Proc. 2025, 90, 19. https://doi.org/10.3390/engproc2025090019
Di Biase F, Varricchio D, Baraniello VR, Persechino G. Design of a Light-Weight Vertical Tail for a Hybrid High-Altitude Pseudo-Satellite. Engineering Proceedings. 2025; 90(1):19. https://doi.org/10.3390/engproc2025090019
Chicago/Turabian StyleDi Biase, Feliciano, Domenico Varricchio, Vincenzo Rosario Baraniello, and Giuseppe Persechino. 2025. "Design of a Light-Weight Vertical Tail for a Hybrid High-Altitude Pseudo-Satellite" Engineering Proceedings 90, no. 1: 19. https://doi.org/10.3390/engproc2025090019
APA StyleDi Biase, F., Varricchio, D., Baraniello, V. R., & Persechino, G. (2025). Design of a Light-Weight Vertical Tail for a Hybrid High-Altitude Pseudo-Satellite. Engineering Proceedings, 90(1), 19. https://doi.org/10.3390/engproc2025090019






