Validation of Chlorine Decay Equation for Water Quality Analysis in Distribution Networks †
Abstract
:1. Introduction
2. Research Methodology
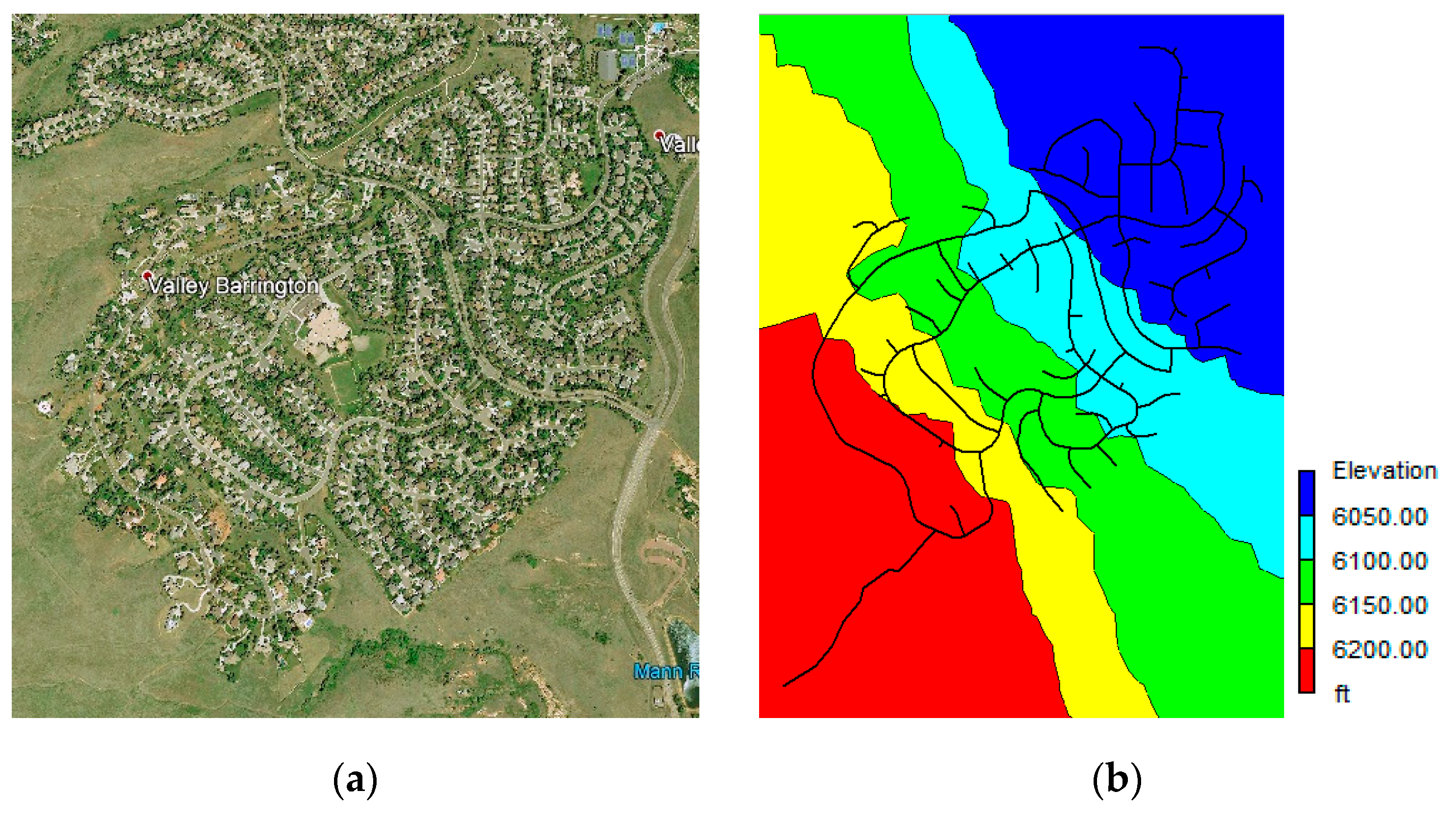
2.1. Study Area
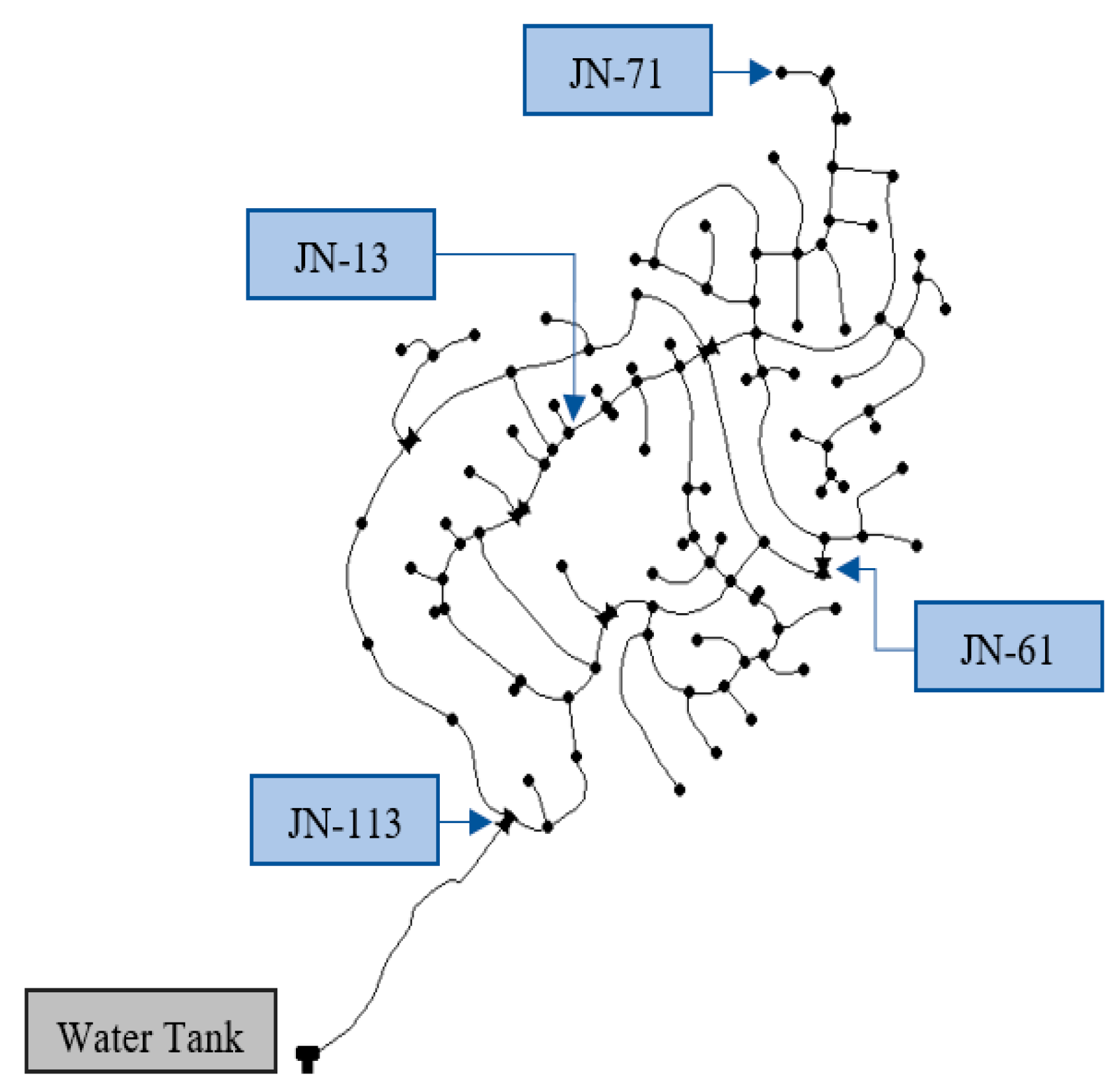
2.2. Hydraulic Analysis Parameters
3. Results and Discussion
3.1. Contaminant Spread
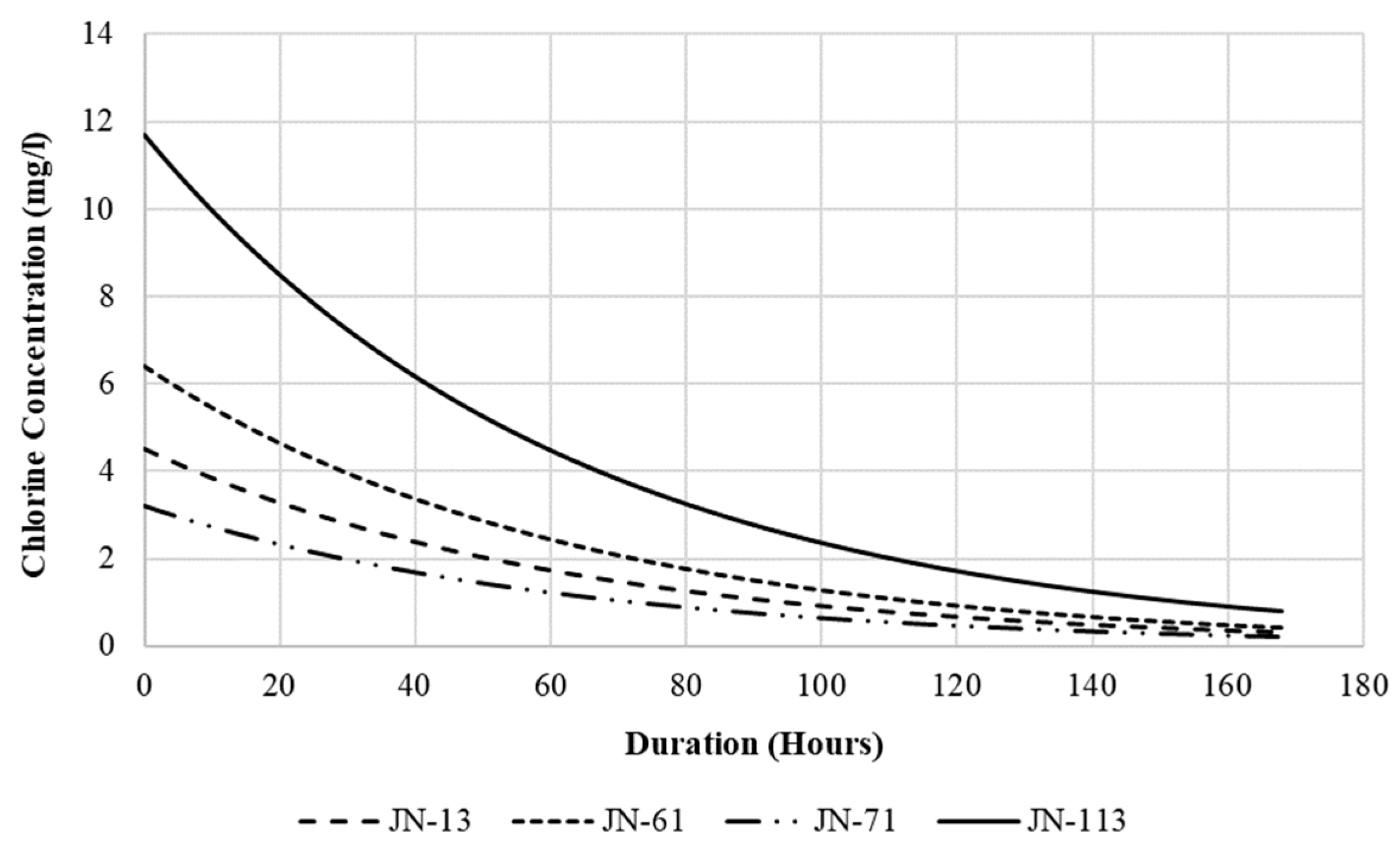
3.2. Chlorine Decay Rate
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Boyd, G.R.; Wang, H.; Britton, M.D.; Howie, D.C.; Wood, D.J.; Funk, J.E.; Friedman, M.J. Intrusion within a Simulated Water Distribution System due to Hydraulic Transients I: Volumetric Method and Comparison of Results. J. Environ. Eng. 2004, 130, 774–777. [Google Scholar] [CrossRef]
- LeChevallier, M.W.; Gullick, R.W.; Karim, M.R.; Friedman, M.; Funk, J.E. The Potential for Health Risks from Intrusion of Contaminants into the Distribution System from Pressure Transients. J. Water Health 2003, 1, 3–14. [Google Scholar] [CrossRef] [PubMed]
- Gong, J.; Guo, X.; Yan, X.; Hu, C. Review of Urban Drinking Water Contamination Source Identification Methods. Energies 2023, 16, 705. [Google Scholar] [CrossRef]
- Qasem, A.; Jamil, R. GIS-Based Financial Analysis Model for Integrated Maintenance and Rehabilitation of Underground Pipe Networks. J. Perform. Constr. Facil. 2021, 35, 1–8. [Google Scholar] [CrossRef]
- Rodríguez, J.M.; Galván, X.D.; Ramos, H.M.; Amparo, P.A.L. An overview of leaks and intrusion for different pipe materials and failures. Urban Water J. 2014, 11, 1–10. [Google Scholar] [CrossRef]
- Karim, M.R.; Abbaszadegan, M.; Lechevallier, M. Potential for Pathogen Intrusion during Pressure Transients. J. Am. Water Work. Assoc. 2003, 95, 134–146. [Google Scholar] [CrossRef]
- Tamminen, S.; Ramos, H.; Covas, D. Water Supply System Performance for Different Pipe Materials Part I: Water Quality Analysis. Water Resour. Manag. 2008, 22, 1579–1607. [Google Scholar] [CrossRef]
- Keramat, A.; Payesteh, M.; Brunone, B.; Meniconi, S. Interdependence of flow and pipe characteristics in transient induced contamination intrusion: Numerical Analysis. J. Hydroinform. 2020, 22, 473–490. [Google Scholar] [CrossRef]
- Darweesh, M. Impact of variable speed pumps on water quality in distribution systems. Water SA 2018, 44, 419–427. [Google Scholar] [CrossRef]
- Ponti, A.; Candelieri, A.; Giordani, I.; Archetti, F. Intrusion Detection in Networks by Wasserstein Enabled Many-Objective Evolutionary Algorithms. Mathematics 2023, 11, 2342. [Google Scholar] [CrossRef]
- Li, Z.; Liu, H.; Zhang, C.; Fu, G. Generative adversarial networks for detecting contamination events in water distribution systems using multi-parameter, multi-site water quality monitoring. Environ. Sci. Ecotechnol. 2023, 14, 100231. [Google Scholar] [CrossRef] [PubMed]
- Aquaveo, L.L.C. 2023. Available online: https://www.aquaveo.com/ (accessed on 13 April 2023).
- Jamil, R. Frictional Head Loss Relation between Hazen-Williams and Darcy-Weisbach Equations for Various Water Supply Pipe Materials. Int. J. Water 2019, 13, 333–347. [Google Scholar] [CrossRef]
- Jamil, R. Performance of a New Pipe Material UHMWPE against Disinfectant Decay in Water Distribution Networks. Clean Technol. Environ. Policy 2018, 20, 1287–1296. [Google Scholar] [CrossRef]
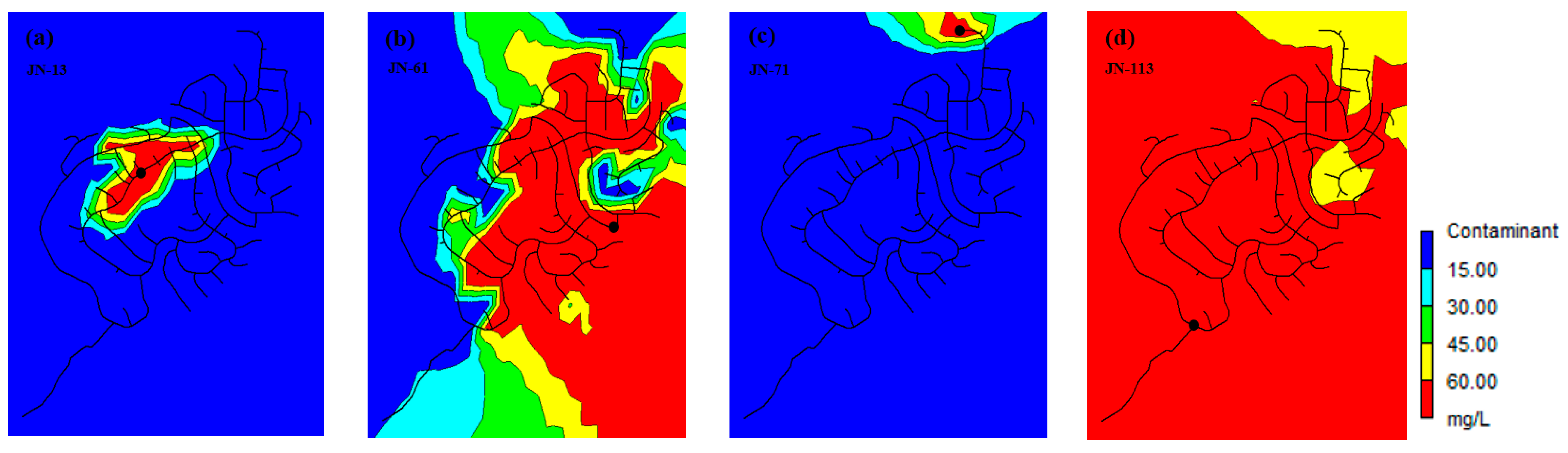
| Critical Pipe Junctions | Contamination Spread (%) |
|---|---|
| JN-13 | 5.01 |
| JN-61 | 21.58 |
| JN-71 | 3.07 |
| JN-113 | 79.61 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jamil, R.; Aziz, H.A.; Murshed, M.F. Validation of Chlorine Decay Equation for Water Quality Analysis in Distribution Networks. Eng. Proc. 2023, 44, 11. https://doi.org/10.3390/engproc2023044011
Jamil R, Aziz HA, Murshed MF. Validation of Chlorine Decay Equation for Water Quality Analysis in Distribution Networks. Engineering Proceedings. 2023; 44(1):11. https://doi.org/10.3390/engproc2023044011
Chicago/Turabian StyleJamil, Rehan, Hamidi Abdul Aziz, and Mohamad Fared Murshed. 2023. "Validation of Chlorine Decay Equation for Water Quality Analysis in Distribution Networks" Engineering Proceedings 44, no. 1: 11. https://doi.org/10.3390/engproc2023044011
APA StyleJamil, R., Aziz, H. A., & Murshed, M. F. (2023). Validation of Chlorine Decay Equation for Water Quality Analysis in Distribution Networks. Engineering Proceedings, 44(1), 11. https://doi.org/10.3390/engproc2023044011







