Numerical Prediction of the Fatigue Life of Complex Riveted Structures †
Abstract
1. Introduction
2. Material and Methods
2.1. Aluminum Alloy and Rivet Descriptions
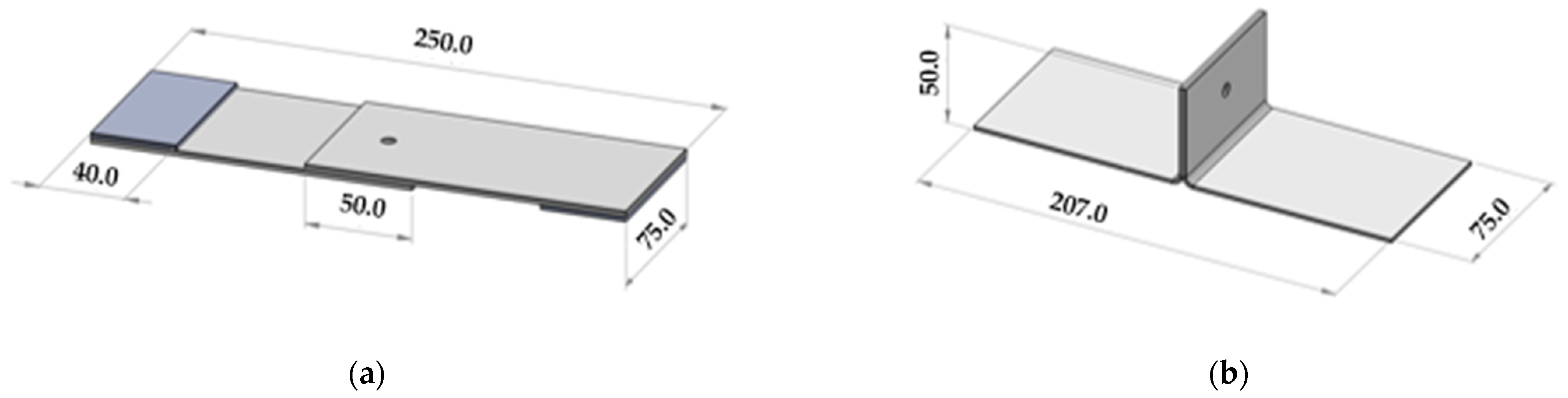
2.2. Single-Riveted Specimen Preparation and Mechanical Testing
2.3. Multi Riveted Specimen Preparation and Mechanical Testing
2.4. Module E Specimen Preparation and Mechanical Testing
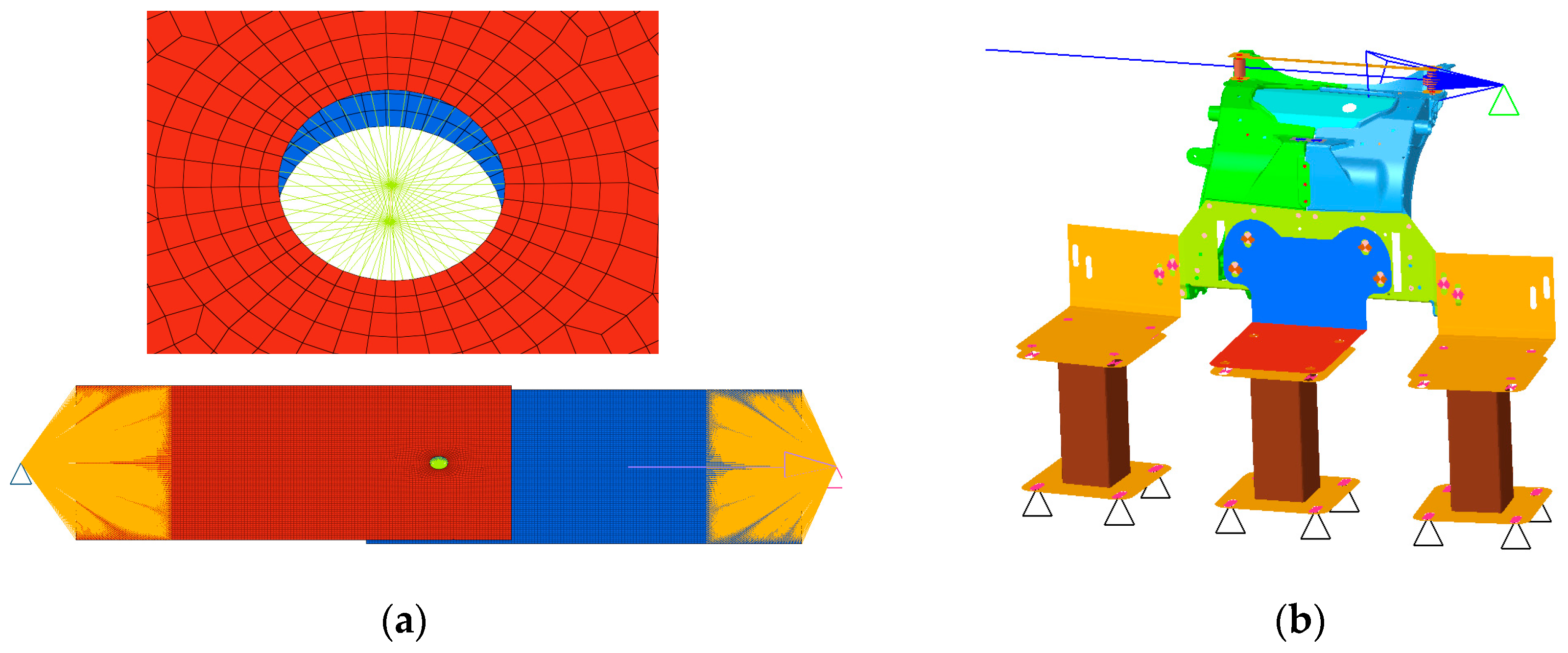
2.5. Numerical Model
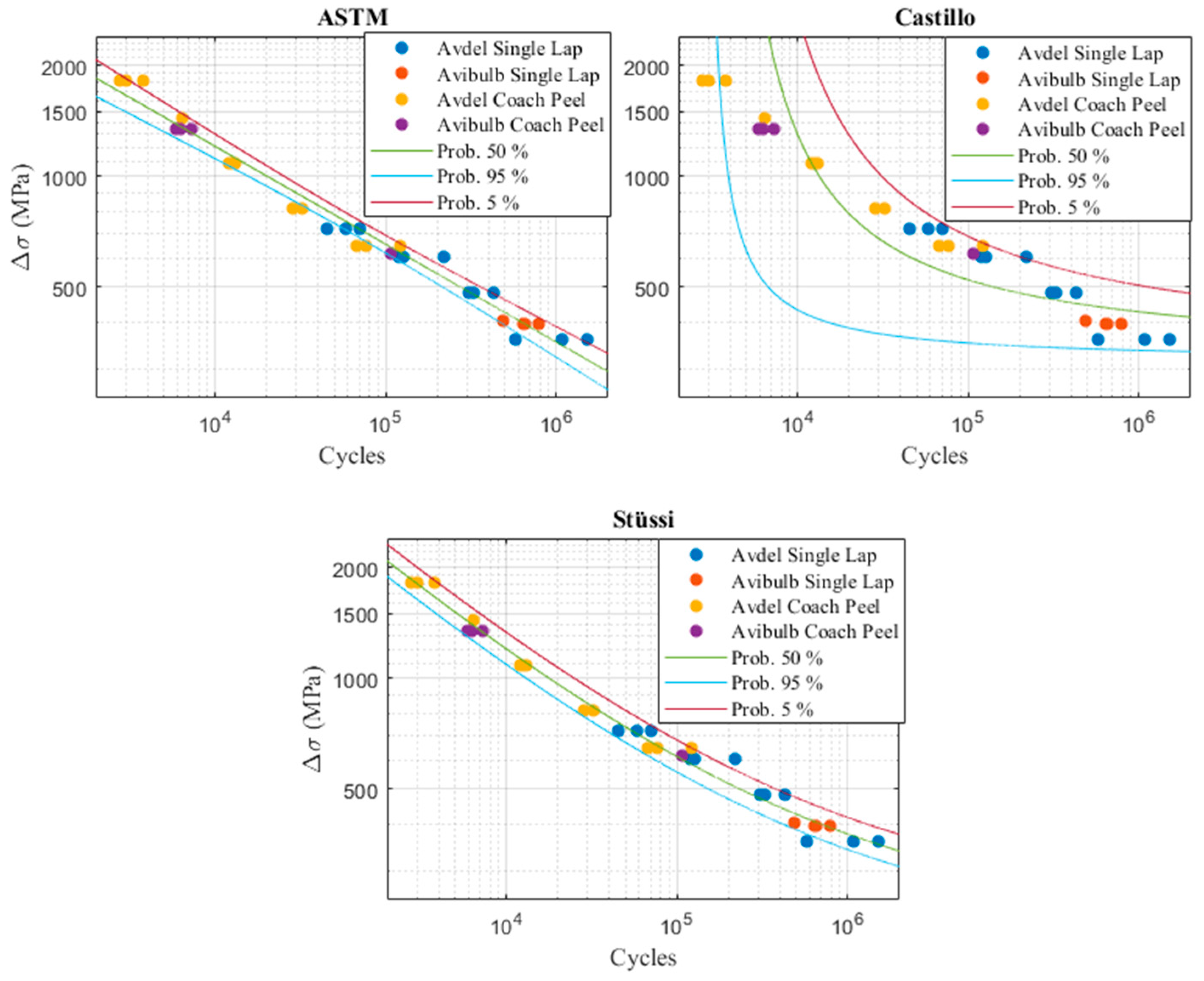
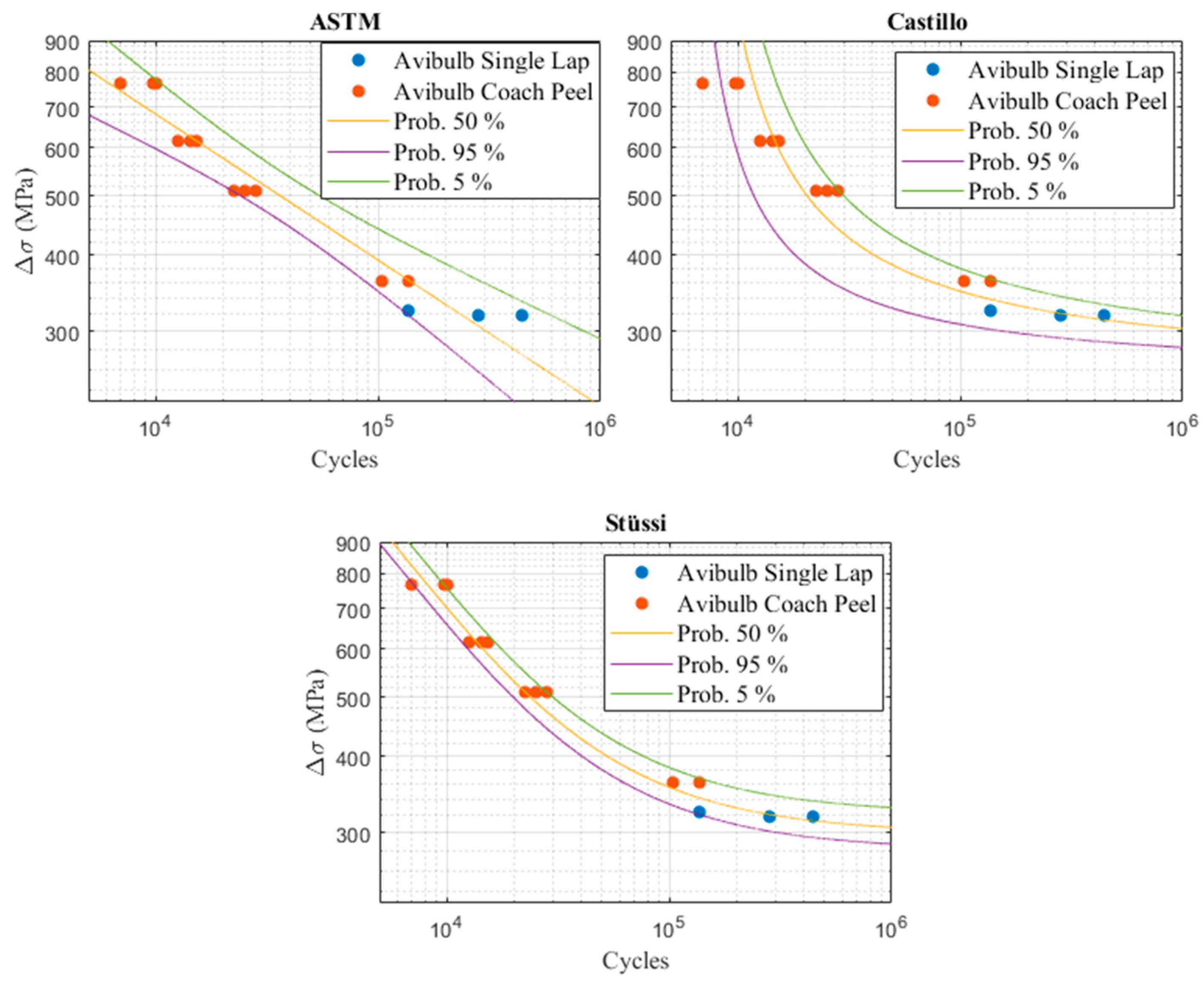
3. Results and Discussions
3.1. Single-Rivet Joint
3.2. Complex Assembly Fatigue Life Prediction
4. Conclusions
- The simplified structural stress evaluation method is effective in combining on a single (N) curve the experimental results of several specimen configurations with distinct characteristics (rivet diameter, covering and peeling configurations, sheet thickness);
- Stüssi’s probabilistic model is the most effective in expressing the fatigue behavior of riveted joints in fatigue;
- Miner’s law is effective in evaluating the accumulated fatigue damage of complex riveted assemblies.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Alam, M. A Study of the Fatigue Behaviour of Laser and Hybrid Laser Welds. Doctoral Dissertation, Luleå University of Technology, Luleå, Sweden, 2009. [Google Scholar]
- Cui, W. A state-of-the-art review on fatigue life prediction methods for metal structures. J. Mar. Sci. Technol. 2002, 7, 43–56. [Google Scholar] [CrossRef]
- ASTM E739-10(2015); Standard Practice for Statistical Analysis of Linear or Linearized Stress-Life (S-N) and Strain-Life (ε-N) Fatigue Data. ASTM: West Conshohocken, PA, USA, 2009; pp. 1–7.
- Castillo, E.; Canteli, A.C.F. A Unified Statistical Methodology for Modeling Fatigue Damage; Springer: Berlin/Heidelberg, Germany, 2009; pp. 1–232. [Google Scholar]
- Barbosa, J.F.; Correia, J.A.; Júnior, R.F.; Zhu, S.-P.; De Jesus, A.M. Probabilistic S-N fields based on statistical distributions applied to metallic and composite materials: State of the art. Adv. Mech. Eng. 2019, 11, 1–22. [Google Scholar] [CrossRef]
- Fatemi, A.; Shamsaei, N. Multiaxial fatigue: An overview and some approximation models for life estimation. Int. J. Fatigue 2011, 33, 948–958. [Google Scholar] [CrossRef]
- Santecchia, E.; Hamouda, A.M.S.; Musharavati, F.; Zalnezhad, E.; Cabibbo, M.; El Mehtedi, M.; Spigarelli, S. A Review on Fatigue Life Prediction Methods for Metals. Adv. Mater. Sci. Eng. 2016, 2016, 9573524. [Google Scholar] [CrossRef]
- Rami, K. Modern Fatigue Analysis Methodology for Laser Welded Joints. Master’s Thesis, University of Oulu, Oulu, Finland, 2018. [Google Scholar]
- Maddox, S. Review of fatigue assessment procedures for welded aluminium structures. Int. J. Fatigue 2003, 25, 1359–1378. [Google Scholar] [CrossRef]
- Caccese, V.; Blomquist, P.; Berube, K.; Webber, S.; Orozco, N. Effect of weld geometric profile on fatigue life of cruciform welds made by laser/GMAW processes. Mar. Struct. 2006, 19, 1–22. [Google Scholar] [CrossRef]
- Fricke, W. Fatigue analysis of welded joints: State of development. Mar. Struct. 2003, 16, 185–200. [Google Scholar] [CrossRef]
- Hobbacher, A.F. Recommendations for Fatigue Design of Welded Joints and Components, 2nd ed.; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Dong, P. A structural stress definition and numerical implementation for fatigue analysis of welded joints. Int. J. Fatigue 2001, 23, 865–876. [Google Scholar] [CrossRef]
- Wu, X.; Wei, Z.; Kang, H.; Khosrovaneh, A. A Structural Stress Recovery Procedure for Fatigue Life Assessment of Welded Structures; SAE Technical Papers; SAE International: Warrendale, PA, USA, 2017; Volume 2017. [Google Scholar]
- Shen, W.; Yan, R.; Barltrop, N.; Liu, E.; Song, L. A method of determining structural stress for fatigue strength evaluation of welded joints based on notch stress strength theory. Int. J. Fatigue 2016, 90, 87–98. [Google Scholar] [CrossRef]
- Xiao, Z.-G.; Yamada, K. A method of determining geometric stress for fatigue strength evaluation of steel welded joints. Int. J. Fatigue 2004, 26, 1277–1293. [Google Scholar] [CrossRef]
- Hong, J.K.; Forte, T.P. Fatigue evaluation procedures for multiaxial loading in welded structures using battelle structural stress approach. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering—OMAE, San Francisco, CA, USA, 8–13 June 2014; Volume 5, pp. 1–9. [Google Scholar]
- Kang, H.T.; Dong, P.; Hong, J. Fatigue analysis of spot welds using a mesh-insensitive structural stress approach. Int. J. Fatigue 2007, 29, 1546–1553. [Google Scholar] [CrossRef]
- Seyedi, A.; Guler, M.A. Mesh Insensitive Structural Stress Method for Fatigue Analysis of Welded Joints Using the Finite Element Method. In Proceedings of the International Advanced Technologies Symposium (IATS’17), Elazığ, Turkey, 19–22 October 2017. [Google Scholar]
- Cox, A.; Hong, J. Fatigue Evaluation Procedure Development for Self-Piercing Riveted Joints Using the Battelle Structural Stress Method; SAE Technical Papers; SAE International: Warrendale, PA, USA, 2016. [Google Scholar]
- Kang, H.T.; Khosrovaneh, A.; Su, X.; Lee, Y.-L.; Guo, M.; Jiang, C.; Li, Z. A Fatigue Life Prediction Method of Laser Assisted Self-Piercing Rivet Joint for Magnesium Alloys. SAE Int. J. Mater. Manuf. 2015, 8, 789–794. [Google Scholar] [CrossRef]
- Rao, H.M.; Kang, J.; Huff, G.; Avery, K. Structural Stress Method to Evaluate Fatigue Properties of Similar and Dissimilar Self-Piercing Riveted Joints. Metals 2019, 9, 359. [Google Scholar] [CrossRef]
- Kang, H.-T.; Boorgu, S. Fatigue Life Prediction of Self-Piercing Rivet Joints Between Magnesium and Aluminum Alloys. MATEC Web Conf. 2018, 165, 10004. [Google Scholar] [CrossRef]
- Turlier, D.; Facchinetti, M.; Wolf, S.; Raoult, I.; Delattre, B.; Magnin, A.; Grimonprez, N. Seam weld shell element model for thin walled structure FE fatigue design. MATEC Web Conf. 2018, 165, 21007. [Google Scholar] [CrossRef][Green Version]
- Hong, J.K. The Development of a Simplified Spot Weld Model for Battelle Stuctural Stress Calculation. SAE Int. J. Mater. Manuf. 2011, 4, 602–612. [Google Scholar] [CrossRef]
- Schneider, N.; Bödecker, J.; Berger, C.; Oechsner, M. Frequency effect and influence of testing technique on the fatigue behaviour of quenched and tempered steel and aluminium alloy. Int. J. Fatigue 2016, 93, 224–231. [Google Scholar] [CrossRef]
- Mayer, H.; Papakyriacou, M.; Pippan, R.; Stanzl-Tschegg, S. Influence of loading frequency on the high cycle fatigue properties of AlZnMgCu1.5 aluminium alloy. Mater. Sci. Eng. A 2001, 314, 48–54. [Google Scholar] [CrossRef]



| Tested Material | Young Modulus (GPa) | Yield Strength (MPa) | Max. Tensile Strength (MPa) | Maximum Elongation (%) |
|---|---|---|---|---|
| AA5052-H36 | 70000 | 230 | 280 | 7 |
| Description | Units | Avibulb BN01-00611 | Avdel Hemlock 2221-00813 |
|---|---|---|---|
| Body diameter | mm | 4.8 (3/16″) | 6.4 (1/4″) |
| Head diameter | mm | 9.6 | 13.4 |
| Hole tolerance | mm | 4.9–5.1 | 6.7–6.9 |
| Max shear strength | kN | 3.6 | 12.0 |
| Max tensile strength | kN | 3.8 | 8.8 |
| Features | Config. 1 | Config. 2 | Config. 3 | Config. 4 |
|---|---|---|---|---|
| Configuration | Single lap | Single lap | Peel | Peel |
| Sheet thickness | 2.0 mm | 2.0 mm | 1.6 mm | 1.6 mm |
| Rivet | Avdel | Avibulb | Avdel | Avibulb |
| Rivet diameter | 6.35 mm | 4.87 mm | 6.35 mm | 4.87 mm |
| Clamping surface (hydraulic jaws) | 75 mm × 40 mm | 75 mm × 40 mm | 75 mm × 40 mm | 75 mm × 40 mm |
| Features | Config. 1 | Config. 2 |
|---|---|---|
| Configuration | Single lap | Peel |
| Sheet thickness | 1.6 mm | 1.6 mm |
| Rivet | Avibulb | Avibulb |
| Rivet diameter | 4.87 mm | 4.87 mm |
| Clamping surface (hydraulic jaws) | 75 mm × 40 mm | 75 mm × 40 mm |
| Samples | Maximal Force | Frequency |
|---|---|---|
| Units | N | Hz |
| Single lap #1 | 10,000 | 5 |
| Single lap #2 | 11,000 | 5 |
| Single lap #3 | 9000 | 5 |
| Coach peel #1 | 600 | 3 |
| Coach peel #2 | 800 | 3 |
| Coach peel #3 | 500 | 3 |
| Load Level | Single Lap | Coach Peel 1 & 2 | Coach Peel 3 | Cycles |
|---|---|---|---|---|
| Load 1 | 10,500 | 600 | 675 | 1000 |
| Load 2 | 10,000 | 650 | 700 | 1000 |
| Load 3 | 9500 | 550 | 600 | 1000 |
| Load 4 | 10,500 | 600 | 650 | 1000 |
| Load 5 | 10,000 | 650 | 700 | 1000 |
| Load 6 | 9500 | 550 | 600 | 1000 |
| Load 7 | 9000 | 500 | 575 | 1000 |
| Load 8 | 11,000 | 700 | 750 | 1000 |
| Load 9 | 9000 | 500 | 575 | 1000 |
| Load 10 | 11,000 | 700 | 750 | 1000 |
| Load 11 | 9000 | 500 | 575 | 1000 |
| Load 12 | 11,000 | 700 | 750 | 1000 |
| Load 13 | 9750 | 625 | 675 | 1000 |
| Load 14 | 10,250 | 600 | 650 | 1000 |
| Load 15 | 10,750 | 625 | 675 | 1000 |
| Load 16 | 10,250 | 650 | 700 | 1000 |
| Load 17 | 10,750 | 625 | 675 | 1000 |
| Load 18 | 9750 | 550 | 600 | 1000 |
| Name | # | Material | Thickness |
|---|---|---|---|
| Casting #1 | 1 | Casting Al alloys | 3 mm |
| Casting #2 | 2 | Casting Al alloys | 3 mm |
| Sheet #1 | 3 | AA5052-H36 | 1.6 mm |
| Sheet #2 | 4 | AA5052-H36 | 1.6 mm |
| Test #1 | Test #2 | Test #3 | |||||
|---|---|---|---|---|---|---|---|
| Load Step | Max Force (N) | Number of Cycles | Max Force (N) | Number of Cycles | Max Force (N) | Number of Cycles | Frequency (Hz) |
| Load step #1 | 1300 | 195,000 | 1500 | 1000 | 1500 | 2000 | 2 |
| Load step #2 | 1500 | 20,000 | 1350 | 1000 | 1400 | 2000 | 2 |
| Load step #3 | 1600 | To failure | 1300 | 1000 | 1300 | 2000 | 2 |
| Load step #4 | 1500 | 1000 | 1500 | 2000 | 2 | ||
| Load step #5 | 1350 | 1000 | 1400 | 2000 | 2 | ||
| Load step #6 | 1200 | 1000 | 1800 | 2000 | 2 | ||
| Load step #7 | 1300 | 1000 | 1350 | 2000 | 2 | ||
| Load step #8 | 1250 | 1000 | 1800 | 2000 | 2 | ||
| Load step #9 | 1300 | 1000 | 1350 | 2000 | 2 | ||
| Load step #10 | 1200 | 1000 | 1800 | 2000 | 2 | ||
| Load step #11 | 1800 | 1000 | 1600 | 2000 | 2 | ||
| Load step #12 | 1300 | 1000 | 1200 | 2000 | 2 | ||
| Load step #13 | 1800 | 1000 | 2000 | 2000 | 2 | ||
| Load step #14 | 1300 | 1000 | 1400 | 2000 | 2 | ||
| Load step #15 | 1800 | 1000 | 1700 | 2000 | 2 | ||
| Load step #16 | 1150 | 1000 | 2 | ||||
| Load step #17 | 1200 | 1000 | 2 | ||||
| Load step #18 | 1250 | 1000 | 2 | ||||
| Load step #19 | 1300 | 1000 | 2 | ||||
| Load step #20 | 1400 | 1000 | 2 | ||||
| Load step #21 | 1300 | 1000 | 2 | ||||
| Load step #22 | 1150 | 1000 | 2 | ||||
| Load step #23 | 2000 | 1000 | 2 | ||||
| Load step #24 | 1200 | 1000 | 2 | ||||
| Load step #25 | 1650 | 1000 | 2 | ||||
| Samples | Test Config. | Type of Failure | Experimental Results | Predict 5% Survival | Predict 95% Survival |
|---|---|---|---|---|---|
| Variable #1 | Coach peel | Rivet | 153,500 | 146,530 | 66,000 |
| Variable #2 | Coach peel | Rivet | 136,900 | 146,530 | 66,000 |
| Variable #3 | Coach peel | Rivet | 94,000 | 117,000 | 53,000 |
| Variable #4 | Single lap | Sheet metal | 205,000 | 375,000 | 152,000 |
| Variable #5 | Single lap | Sheet metal | 193,000 | 375,000 | 152,000 |
| Variable #6 | Single lap | Sheet metal | 188,000 | 375,000 | 152,000 |
| Module E #1 | Module E | Rivet | 233,300 | 278,000 | 146,530 |
| Module E #2 | Module E | Rivet | 206,400 | 207,010 | 89,203 |
| Module E #3 | Module E | Rivet | 100,100 | 106,050 | 53,478 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Corriveau, F.; Desrochers, A.; Maslouhi, A. Numerical Prediction of the Fatigue Life of Complex Riveted Structures. Eng. Proc. 2023, 43, 6. https://doi.org/10.3390/engproc2023043006
Corriveau F, Desrochers A, Maslouhi A. Numerical Prediction of the Fatigue Life of Complex Riveted Structures. Engineering Proceedings. 2023; 43(1):6. https://doi.org/10.3390/engproc2023043006
Chicago/Turabian StyleCorriveau, Francis, Alain Desrochers, and Ahmed Maslouhi. 2023. "Numerical Prediction of the Fatigue Life of Complex Riveted Structures" Engineering Proceedings 43, no. 1: 6. https://doi.org/10.3390/engproc2023043006
APA StyleCorriveau, F., Desrochers, A., & Maslouhi, A. (2023). Numerical Prediction of the Fatigue Life of Complex Riveted Structures. Engineering Proceedings, 43(1), 6. https://doi.org/10.3390/engproc2023043006






