High Gain Observer Based Active Disturbance Estimation ADE for Second Order Nonlinear Uncertain Systems (ex:Induction Motor) †
Abstract
:1. Introduction
2. State Observation Scheme with Active Disturbance Estimation (ADE)
2.1. Problem Statement
2.2. High-Gain Observer with Active Disturbance Estimator
3. Ade Observer for the Induction Motor
3.1. Induction Motor System Modeling
3.2. High-Gain Observer with Active Disturbance Estimator
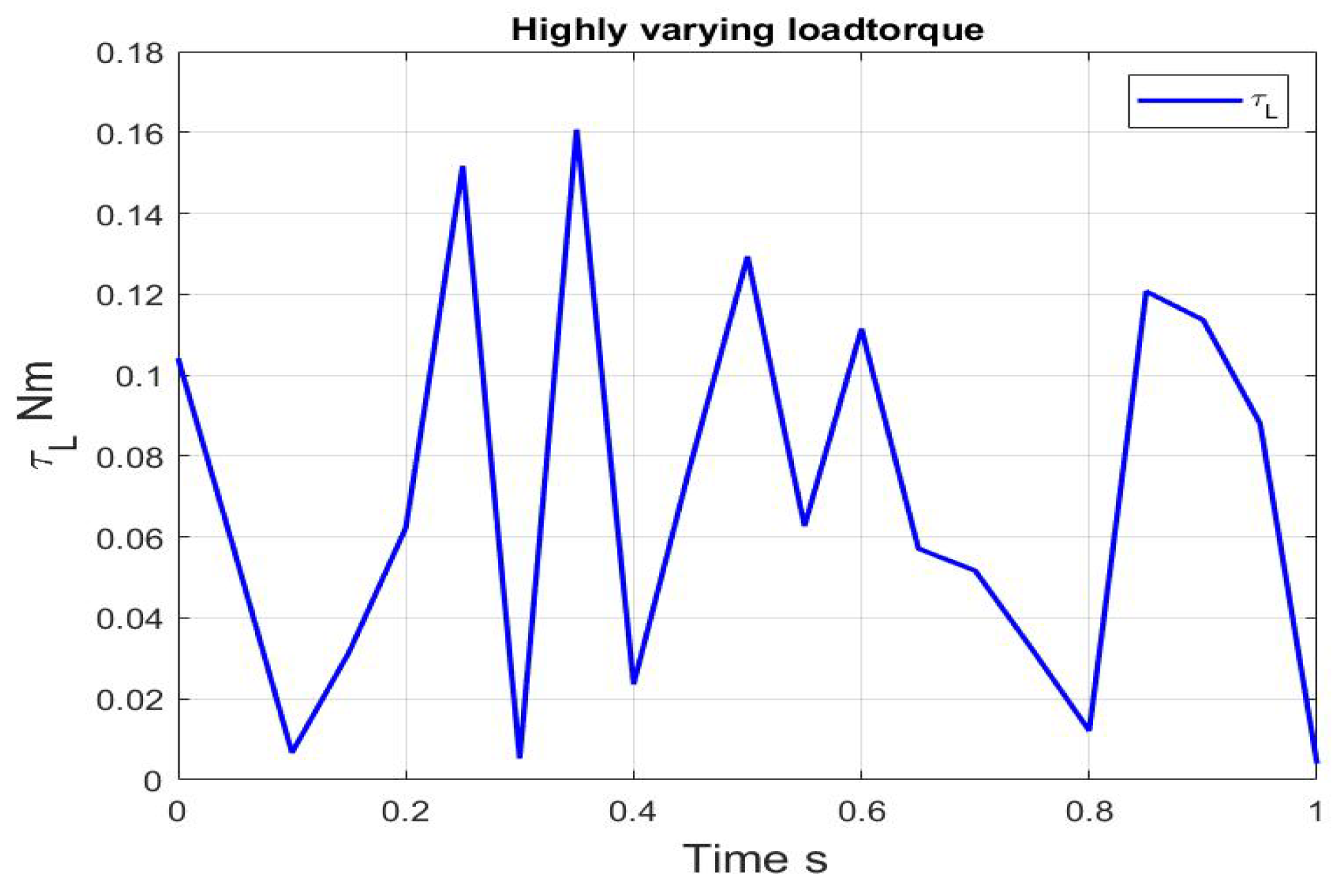
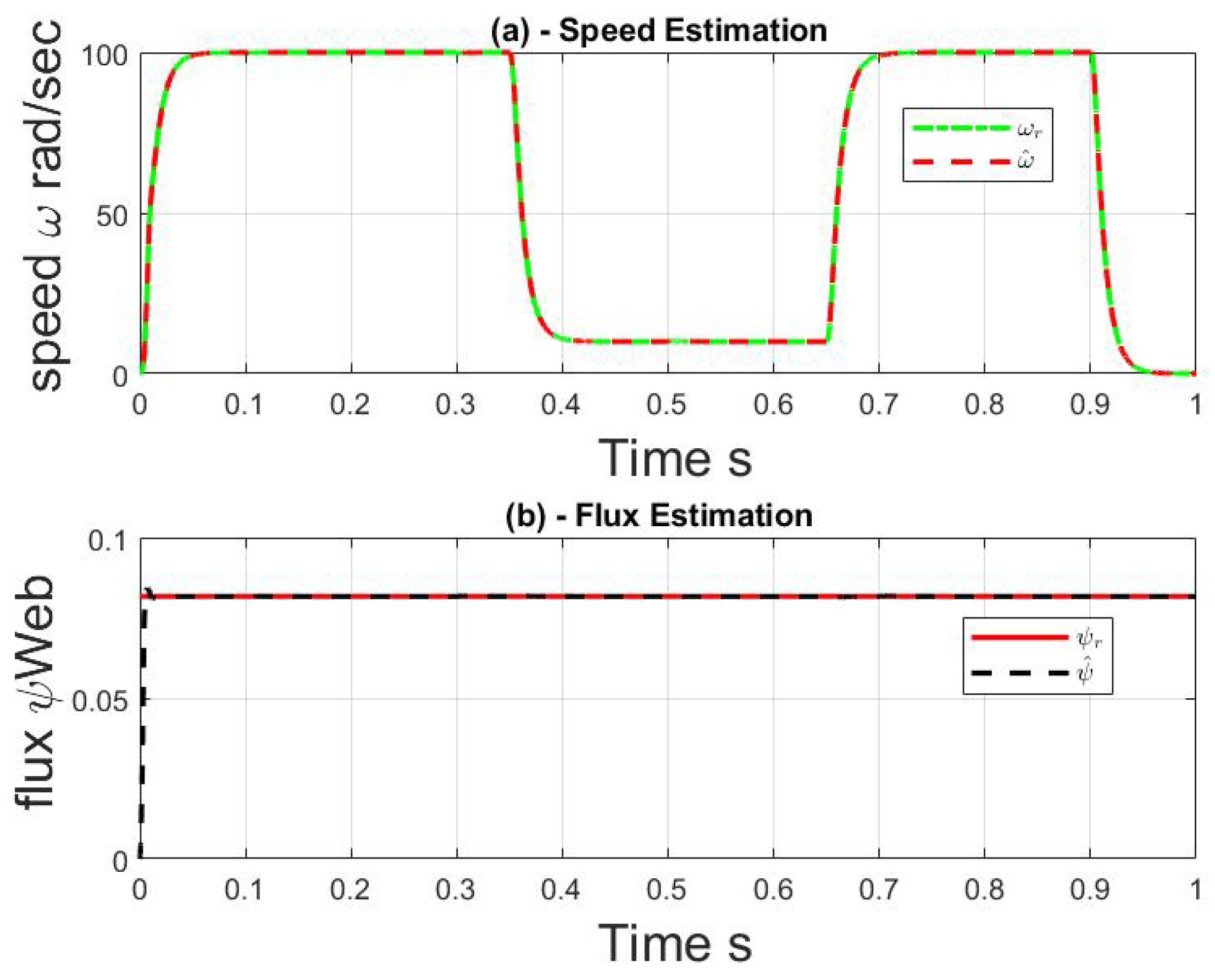
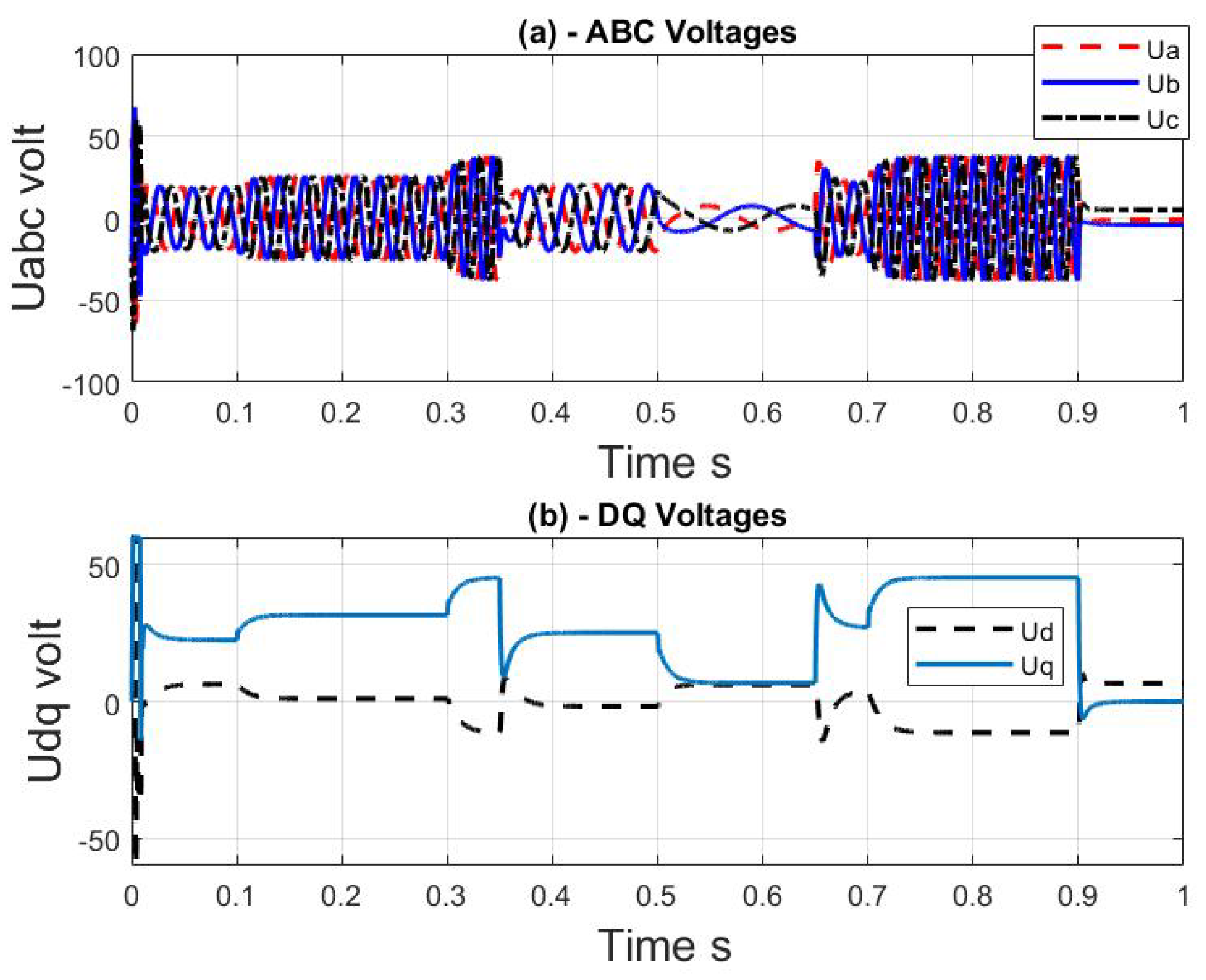
4. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| FOC | Field-Oriented Control |
| IM | Induction motor |
| ADE | Active Disturbance Estimation |
References
- Sira-Ramírez, H.; Luviano-Juárez, A.; Ramírez-Neria, M.; Zurita-Bustamante, E.W. Active Disturbance Rejection Control of Dynamic Systems: A Flatness-Based Approach; Elsevier Ergonomics Book Series; Butterworth-Heinemann: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Ammar, A.; Kheldoun, A.; Metidji, B.; Ameid, T.; Azzoug, Y. Feedback linearization based sensorless direct torque control using stator flux MRAS-sliding mode observer for induction motor drive. ISA Trans. 2020, 98, 382–392. [Google Scholar] [CrossRef] [PubMed]
- Regaya, C.B.; Farhani, F.; Zaafouri, A.; Chaari, A. A novel adaptive control method for induction motor based on Backstepping approach using dSpace DS 1104 control board. Mech. Syst. Signal Process. 2018, 100, 466–481. [Google Scholar] [CrossRef]
- Regaya, C.B.; Farhani, F.; Zaafouri, A.; Chaari, A. An adaptive sliding-mode speed observer for induction motor under backstepping control. Int. J. Innov. Comput. I 2017, 11, 763–771. [Google Scholar]
- Khalil, H.K. High-Gain Observers in Nonlinear Feedback Control; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2017. [Google Scholar]
- Petersen, I.R.; Holot, C.V. High-gain observers applied to problems in disturbance attenuation, H-infinity optimization and the stabilization of uncertain linear systems. In Proceedings of the 1988 American Control Conference, Atlanta, GA, USA, 15–17 June 1988; pp. 2490–2496. [Google Scholar]
- Esfandiari, F.; Khalil, H.K. Observer-based design of uncertain systems: Recovering state feedback robustness under matching conditions. In Proceedings of the 1989 American Control Conference, Pittsburgh, PA, USA, 21–23 June 1989. [Google Scholar]
- Khalil, H.; Saberi, A. Adaptive stabilization of a class of nonlinear systems using high-gain feedback. IEEE Trans. Autom. Contr. 1987, 32, 1031–1035. [Google Scholar] [CrossRef]
- Saberi, A.; Sannuti, P. Observer design for loop transfer recovery and for uncertain dynamical systems. IEEE Trans. Autom. Contr. 1990, 35, 878–897. [Google Scholar] [CrossRef]
- Tornambe, A. Use of asymptotic observers having high-gains in the state and parameter estimation. In Proceedings of the 28th IEEE Conference on Decision and Control, Tampa, FL, USA, 13–15 December 1989; pp. 1792–1794. [Google Scholar]
- Yin, Z.; Zhang, Y.; Du, C.; Liu, J.; Sun, X.; Zhong, Y. Research on Anti-Error Performance of Speed and Flux Estimation for Induction Motors Based on Robust Adaptive State Observer. IEEE Trans. Ind. Electron. 2016, 63, 3499–3510. [Google Scholar] [CrossRef]
- Guo, B.-Z.; Zhao, Z.-L. On convergence of the nonlinear active disturbance rejection control for MIMO systems. SIAM J. Control Optim. 2013, 51, 1727–1757. [Google Scholar] [CrossRef]
- Guo, B.-Z.; Zhao, Z.-L. On the convergence of an extended state observer for nonlinear systems with uncertainty. Syst. Control Lett. 2011, 60, 420–430. [Google Scholar] [CrossRef]
- Zheng, Q.; Gao, L.; Gao, Z. On stability analysis of active disturbance rejection control for nonlinear time-varying plants with unknown dynamics. In Proceedings of the 2007 46th IEEE Conference on Decision and Control, New Orleans, LA, USA, 12–14 December 2007; pp. 12–14. [Google Scholar]
- Chiasson, J. : Modeling and High Performance Control of Electric Machines; John Wiley and Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Abbas, H.A.; Belkheiri, M.; Zegnini, B. Feedback Linearization Control of an Induction Machine Augmented by Single Hidden Layer Neural Networks. Int. J. Control 2016, 89, 140–155. [Google Scholar] [CrossRef]
- Zhang, Y.; Jiang, Z.; Yu, X. Indirect Field-Oriented Control of Induction Machines Based on Synergetic Control Theory. In Proceedings of the IEEE Power and Energy Society General Meeting—Conversion and Delivery of Electrical Energy in the 21st Century, Pittsburgh, PA, USA, 20–24 July 2008; pp. 1–7. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kelam, S.; Chennafa, M.; Belkheiri, M.; Zaafrane, M.A. High Gain Observer Based Active Disturbance Estimation ADE for Second Order Nonlinear Uncertain Systems (ex:Induction Motor). Eng. Proc. 2023, 29, 14. https://doi.org/10.3390/engproc2023029014
Kelam S, Chennafa M, Belkheiri M, Zaafrane MA. High Gain Observer Based Active Disturbance Estimation ADE for Second Order Nonlinear Uncertain Systems (ex:Induction Motor). Engineering Proceedings. 2023; 29(1):14. https://doi.org/10.3390/engproc2023029014
Chicago/Turabian StyleKelam, Saad, Mohamed Chennafa, Mohamed Belkheiri, and Mohamed Amine Zaafrane. 2023. "High Gain Observer Based Active Disturbance Estimation ADE for Second Order Nonlinear Uncertain Systems (ex:Induction Motor)" Engineering Proceedings 29, no. 1: 14. https://doi.org/10.3390/engproc2023029014
APA StyleKelam, S., Chennafa, M., Belkheiri, M., & Zaafrane, M. A. (2023). High Gain Observer Based Active Disturbance Estimation ADE for Second Order Nonlinear Uncertain Systems (ex:Induction Motor). Engineering Proceedings, 29(1), 14. https://doi.org/10.3390/engproc2023029014





