Large-Scale Tungsten Fibre-Reinforced Tungsten and Its Mechanical Properties
Abstract
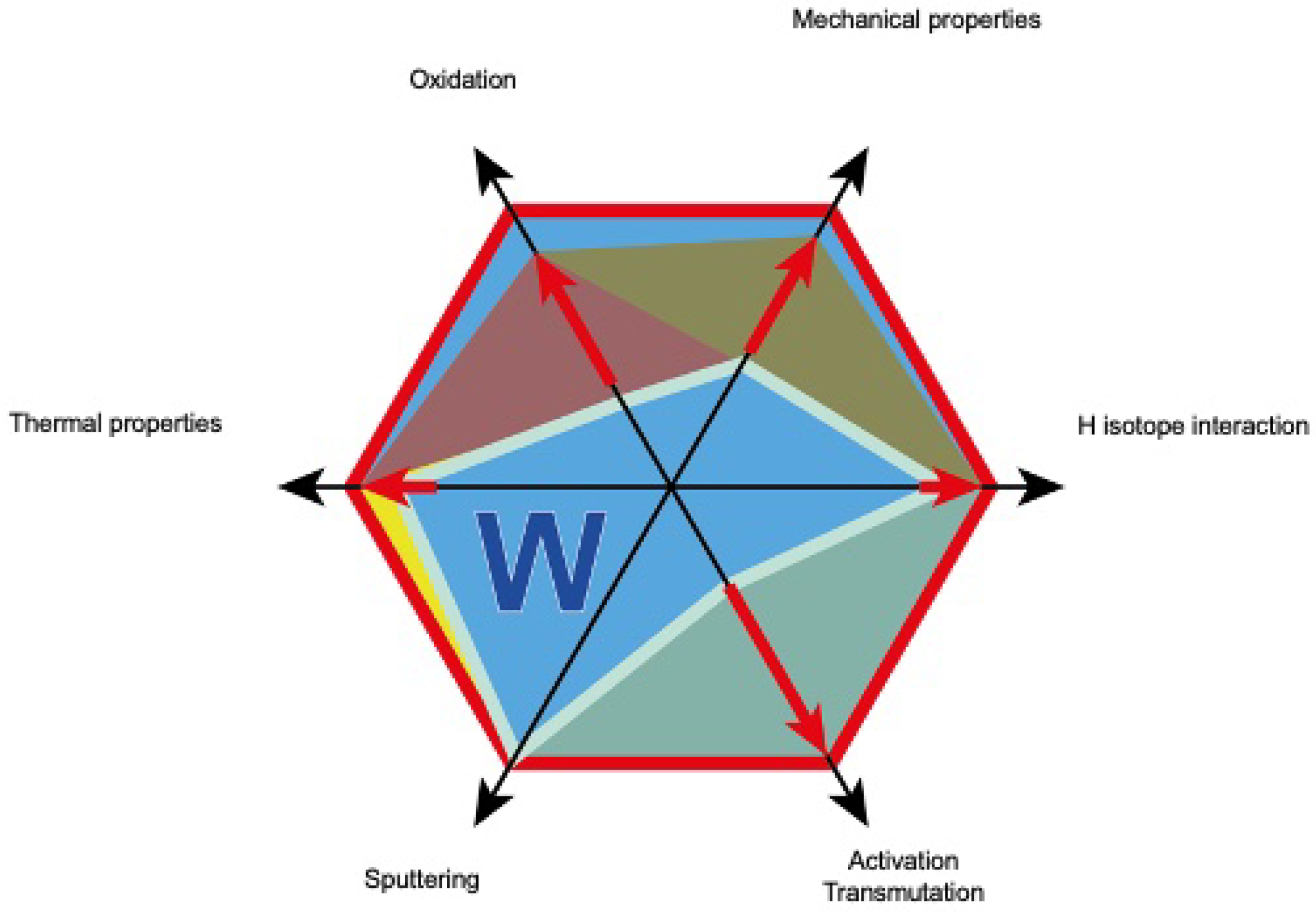
1. Introduction
2. Tungsten-Fibre-Reinforced Tungsten
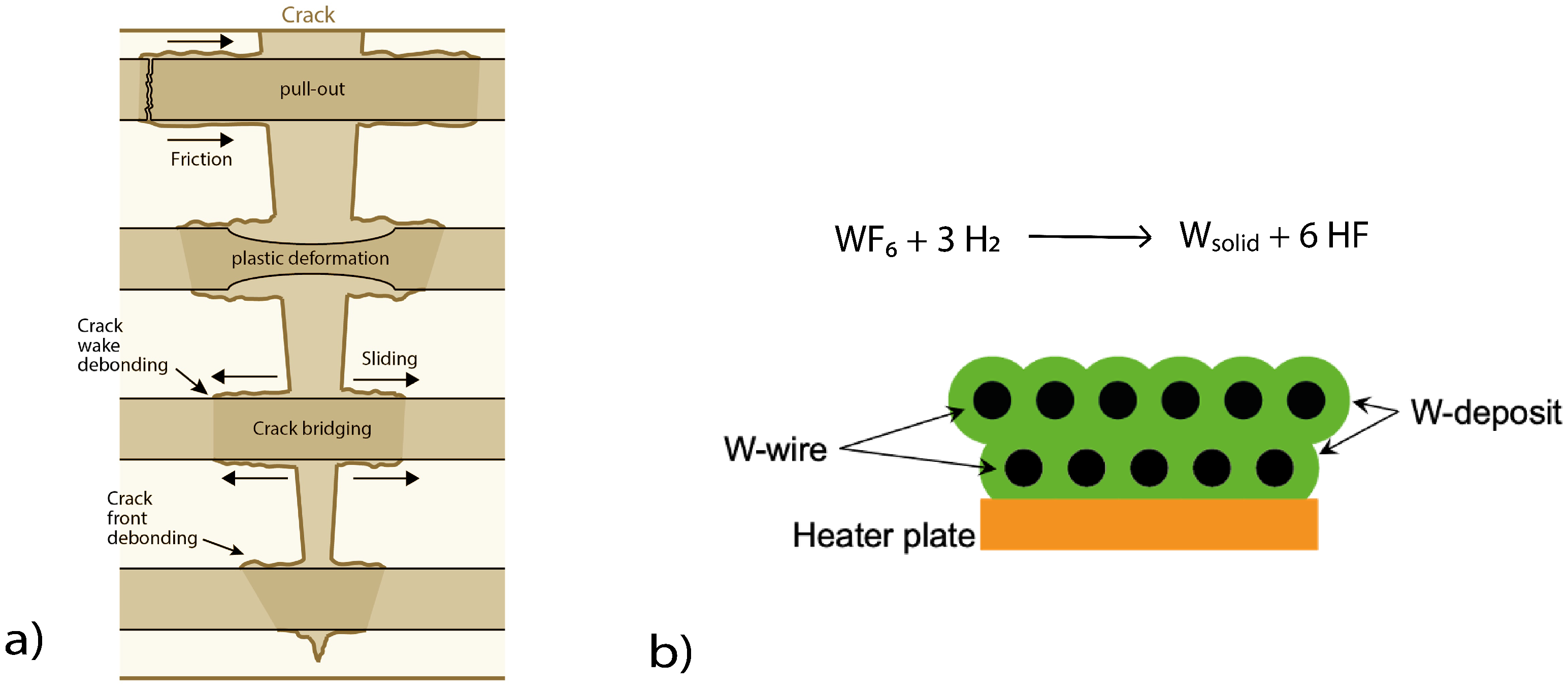
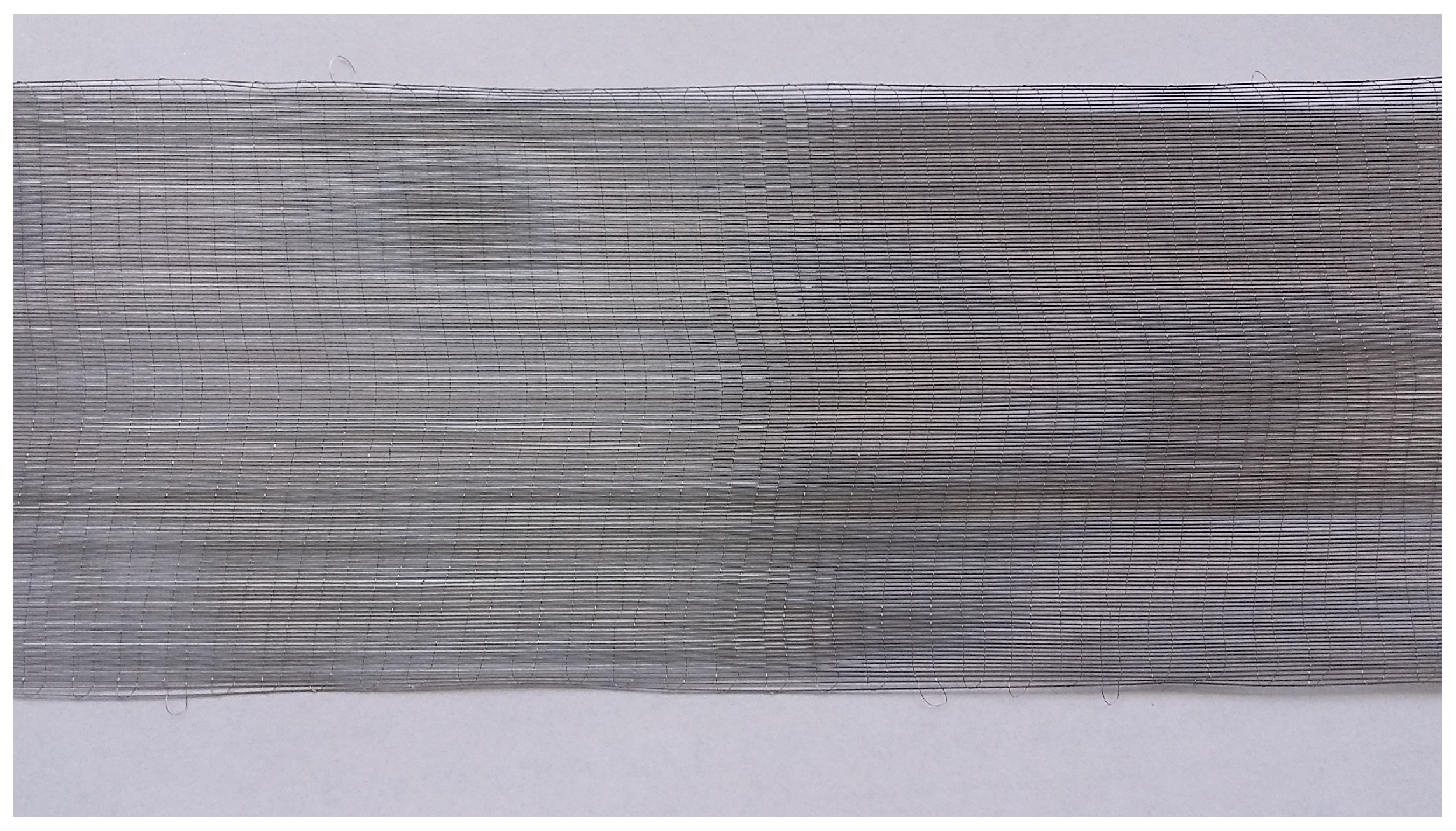
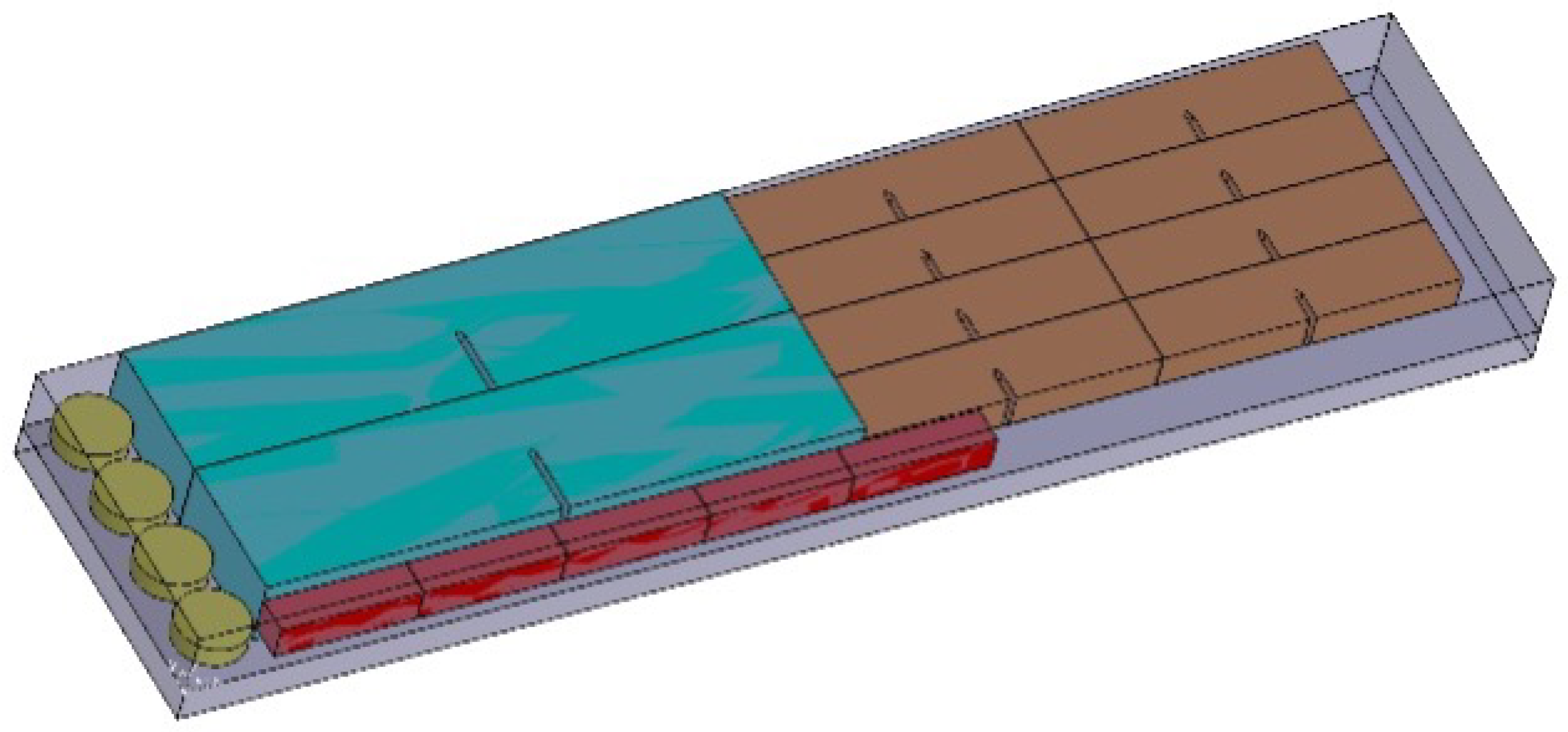
3. Materials and Methods of Sample Production
4. Mechanical Tests
5. Fracture Toughness Determination
5.1. ASTM E399
5.2. ASTM 1820
6. Experimental Results and Discussion
6.1. Large Sized Sample
6.2. Medium-Sized Samples
7. Summary and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Pitts, R.; Carpentier, S.; Escourbiac, F.; Hirai, T.; Komarov, V.; Lisgo, S.; Kukushkin, A.; Loarte, A.; Merola, M.; Naik, A.S.; et al. A full tungsten divertor for ITER: Physics issues and design status. J. Nucl. Mater. 2013, 438, S48. [Google Scholar] [CrossRef]
- Linsmeier, C.; Rieth, M.; Aktaa, J.; Chikada, T.; Hoffmann, A.; Hoffmann, J.; Houben, A.; Kurishita, H.; Jin, X.; Li, M.; et al. Development of advanced high heat flux and plasma-facing materials. Nucl. Fusion 2017, 57, 092007. [Google Scholar] [CrossRef]
- Philipps, V. Tungsten as material for plasma-facing components in fusion devices. J. Nucl. Mater. 2011, 415, 2–9. [Google Scholar] [CrossRef]
- Ueda, Y.; Schmid, K.; Balden, M.; Coenen J., W.; Loewenhoff, T.; Ito, A.; Hasegawa, A.; Hardie, c.; Porton, M.; Gilbert, M. Baseline high heat flux and plasma facing materials for fusion. Nucl. Fusion 2017, 57, 092006. [Google Scholar] [CrossRef]
- You, J.; Visca, E.; Barrett, T.; Böswirth, B.; Crescenzi, F.; Domptail, F.; Fursdon, M.; Gallay, F.; Ghidersa, B.E.; Greuner, H.; et al. European divertor target concepts for DEMO: Design rationales and high heat flux performance. Nucl. Mater. Energy 2018, 16, 1–11. [Google Scholar] [CrossRef]
- Bachmann, C. Initial DEMO Tokamak Design Configuration Studies. Fusion Eng. Des. 2014, 98, 1423–1426. [Google Scholar] [CrossRef]
- Coenen, J.; Antusch, S.; Aumann, M.; Biel, W.; Du, J.; Engels, J.; Heuer, S.; Houben, A.; Hoeschen, T.; Jasper, B.; et al. Materials for DEMO and reactor applications—Boundary conditions and new concepts. Phys. Scr. 2016, 2016, 014002. [Google Scholar] [CrossRef]
- Coenen, J.W. Fusion Materials Development at Forschungszentrum Juelich. Adv. Eng. Mater. 2020, 22, 1901376. [Google Scholar] [CrossRef]
- Gandhi, C.; Ashby, M. Overview no. 5. Acta Metall. 1979, 27, 1565–1602. [Google Scholar] [CrossRef]
- Lassner, E.; Schubert, W.D. Tungsten; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar] [CrossRef]
- Yih, W. Tungsten: Sources, Metallurgy, Properties, and Applications; Springer: Berlin/Heidelberg, Germany, 1979; p. 500. [Google Scholar]
- Barabash, V.; Federici, G.; Rödig, M.; Snead, L.; Wu, C. Neutron irradiation effects on plasma facing materials. J. Nucl. Mater. 2000, 283–287, 138–146. [Google Scholar] [CrossRef]
- Steichen, J. Tensile properties of neutron irradiated TZM and tungsten. J. Nucl. Mater. 1976, 60, 13–19. [Google Scholar] [CrossRef]
- Gietl, H.; Olbrich, S.; Riesch, J.; Holzner, G.; Höschen, T.; Coenen, J.; Neu, R. Estimation of the fracture toughness of tungsten fibre-reinforced tungsten composites. Eng. Fract. Mech. 2020, 232, 107011. [Google Scholar] [CrossRef]
- Garrison, L.M.; Katoh, Y.; Snead, L.L.; Byun, T.S.; Reiser, J.; Rieth, M. Irradiation effects in tungsten-copper laminate composite. J. Nucl. Mater. 2016, 481, 134–146. [Google Scholar] [CrossRef]
- Reiser, J.; Rieth, M.; Moeslang, A.; Greuner, H.; Armstrong, D.; Denk, T.; Gruening, T.; Hering, W.; Hoffmann, A.; Hoffmann, J.; et al. Tungsten (W) Laminate pipes for innovative high temperature energy conversion systems. Adv. Eng. Mater. 2014, 17, 491–501. [Google Scholar] [CrossRef]
- Rieth, M.; Dudarev, S.; Gonzalez De Vicente, S.; Aktaa, J.; Ahlgren, T.; Antusch, S.; Armstrong, D.; Balden, M.; Baluc, N.; Barthe, M.F.; et al. A brief summary of the progress on the EFDA tungsten materials program. J. Nucl. Mater. 2013, 442, 173–180. [Google Scholar] [CrossRef]
- Fujitsuka, M.; Tsuchiya, B.; Mutoh, I.; Tanabe, T.; Shikama, T. Effect of neutron irradiation on thermal diffusivity of tungsten–rhenium alloys. J. Nucl. Mater. 2000, 283–287, 1148–1151. [Google Scholar] [CrossRef]
- Qiu, N.N.; Zhang, Y.; Zhang, C.; Tong, H.; Song, X.P. Tensile properties of tungsten-rhenium wires with nanofibrous structure. Int. J. Miner. Metall. Mater. 2018, 25, 1055–1059. [Google Scholar] [CrossRef]
- Antusch, S.; Armstrong, D.E.; Britton, T.B.; Commin, L.; Gibson, J.S.L.; Greuner, H.; Hoffmann, J.; Knabl, W.; Pintsuk, G.; Rieth, M.; et al. Mechanical and microstructural investigations of tungsten and doped tungsten materials produced via powder injection molding. Nucl. Mater. Energy 2015, 3-4, 22–31. [Google Scholar] [CrossRef]
- Rieth, M.; Dudarev, S.; De Vicente, S.G.; Aktaa, J.; Ahlgren, T.; Antusch, S.; Armstrong, D.; Balden, M.; Baluc, N.; Barthe, M.F.; et al. Recent progress in research on tungsten materials for nuclear fusion applications in Europe. J. Nucl. Mater. 2013, 432, 482–500. [Google Scholar] [CrossRef]
- Riesch, J.; Buffiere, J.Y.; Hoeschen, T.; di Michiel, M.; Scheel, M.; Linsmeier, C.; You, J.H. In situ synchrotron tomography estimation of toughening effect by semi-ductile fibre reinforcement in a tungsten-fibre-reinforced tungsten composite system. Acta Mater. 2013, 61, 7060–7071. [Google Scholar] [CrossRef]
- Riesch, J.; Hoeschen, T.; Linsmeier, C.; Wurster, S.; You, J.H. Enhanced toughness and stable crack propagation in a novel tungsten fibre-reinforced tungsten composite produced by chemical vapour infiltration. Phys. Scr. 2014, 2014, 014031. [Google Scholar] [CrossRef]
- Mao, Y.; Coenen, J.; Sistla, S.; Liu, C.; Terra, A.; Tan, X.; Riesch, J.; Hoeschen, T.; Wu, Y.; Broeckmann, C.; et al. Design of tungsten fibre-reinforced tungsten composites with porous matrix. Mater. Sci. Eng. A 2021, 817, 141361. [Google Scholar] [CrossRef]
- Mao, Y.; Coenen, J.W.; Riesch, J.; Sistla, S.; Almanstötter, J.; Terra, A.; Chen, C.; Wu, Y.; Raumann, L.; Höschen, T.; et al. Fibre Volume Fraction Influence on Randomly Distributed Short Fibre Tungsten Fibre Reinforced Tungsten Composites. Adv. Eng. Mater. 2020, 22, 1901242. [Google Scholar] [CrossRef]
- Mao, Y.; Coenen, J.W.; Sistla, S.; Tan, X.; Riesch, J.; Raumann, L.; Schwalenberg, D.; Höschen, T.; Chen, C.; Wu, Y.; et al. Development of tungsten fibre-reinforced tungsten with a porous matrix. Phys. Scr. 2020, T171, 014030. [Google Scholar] [CrossRef]
- Jasper, B.; Coenen, J.W.; Riesch, J.; Höschen, T.; Bram, M.; Linsmeier, C. Powder Metallurgical Tungsten Fibre-Reinforced Tungsten. Mater. Sci. Forum 2015, 825, 125–133. [Google Scholar] [CrossRef]
- Jasper, B.; Schoenen, S.; Du, J.; Hoeschen, T.; Koch, F.; Linsmeier, C.; Neu, R.; Riesch, J.; Terra, A.; Coenen, J. Behavior of tungsten fibre-reinforced tungsten based on single fibre push-out study. Nucl. Mater. Energy 2016, 9, 416–421. [Google Scholar] [CrossRef]
- Raumann, L.; Coenen, J.; Riesch, J.; Mao, Y.; Schwalenberg, D.; Wegener, T.; Gietl, H.; Höschen, T.; Linsmeier, C.; Guillon, O. Modeling and experimental validation of a Wf/W-fabrication by chemical vapor deposition and infiltration. Nucl. Mater. Energy 2021, 28, 101048. [Google Scholar] [CrossRef]
- Raumann, L.; Coenen, J.W.; Riesch, J.; Mao, Y.; Schwalenberg, D.; Gietl, H.; Linsmeier, C.; Guillon, O. Improving the W Coating Uniformity by a COMSOL Model-Based CVD Parameter Study for Denser Wf/W Composites. Metals 2021, 11, 1089. [Google Scholar] [CrossRef]
- Raumann, L.; Coenen, J.; Riesch, J.; Mao, Y.; Gietl, H.; Höschen, T.; Linsmeier, C.; Guillon, O. Modeling and validation of chemical vapor deposition of tungsten for tungsten fibre reinforced tungsten composites. Surf. Coat. Technol. 2020, 381, 124745. [Google Scholar] [CrossRef]
- Coenen, J.; Mao, Y.; Sistla, S.; Müller, A.; Pintsuk, G.; Wirtz, M.; Riesch, J.; Hoeschen, T.; Terra, A.; You, J.H.; et al. Materials development for new high heat-flux component mock-ups for DEMO. Fusion Eng. Des. 2019, 146, 1431–1436. [Google Scholar] [CrossRef]
- Gietl, H.; Müller, A.V.; Coenen, J.; Decius, M.; Ewert, D.; Höschen, T.; Huber, P.; Milwich, M.; Riesch, J.; Neu, R. Textile preforms for tungsten fibre-reinforced composites. J. Compos. Mater. 2018, 52, 3875–3884. [Google Scholar] [CrossRef]
- Mao, Y.; Coenen, J.; Riesch, J.; Sistla, S.; Almanstötter, J.; Jasper, B.; Terra, A.; Höschen, T.; Gietl, H.; Linsmeier, C.; et al. Influence of the interface strength on the mechanical properties of discontinuous tungsten fibre-reinforced tungsten composites produced by field assisted sintering technology. Compos. Part A Appl. Sci. Manuf. 2018, 107, 342–353. [Google Scholar] [CrossRef]
- Riesch, J.; Aumann, M.; Coenen, J.; Gietl, H.; Holzner, G.; Hoeschen, T.; Huber, P.; Li, M.; Linsmeier, C.; Neu, R. Chemically deposited tungsten fibre-reinforced tungsten – The way to a mock-up for divertor applications. Nucl. Mater. Energy 2016, 9, 75–83. [Google Scholar] [CrossRef]
- Riesch, J.; Han, Y.; Almanstötter, J.; Coenen, J.; Höschen, T.; Jasper, B.; Zhao, P.; Linsmeier, C.; Neu, R. Development of tungsten fibre-reinforced tungsten composites towards their use in DEMO—Potassium doped tungsten wire. Phys. Scr. 2016, T167, 014006. [Google Scholar] [CrossRef]
- Gietl, H.; Riesch, J.; Coenen, J.; Höschen, T.; Linsmeier, C.; Neu, R. Tensile deformation behavior of tungsten fibre-reinforced tungsten composite specimens in as-fabricated state. Fusion Eng. Des. 2017, 124, 396–400. [Google Scholar] [CrossRef]
- Mao, Y.; Coenen, J.W.; Riesch, J.; Sistla, S.; Almanstötter, J.; Jasper, B.; Terra, A.; Höschen, T.; Gietl, H.; Bram, M.; et al. Development and characterization of powder metallurgically produced discontinuous tungsten fibre reinforced tungsten composites. Physica Scripta 2017, T170, 014005. [Google Scholar] [CrossRef]
- Coenen, J.; Riesch, J.; You, J.H.; Rieth, M.; Pintsuk, G.; Gietl, H.; Jasper, B.; Klein, F.; Litnovsky, A.; Mao, Y.; et al. Advanced materials for a damage resilient divertor concept for DEMO: Powder-metallurgical tungsten-fibre reinforced tungsten. Fusion Eng. Des. 2017, 124, 964–968. [Google Scholar] [CrossRef]
- Mao, Y.; Engels, J.; Houben, A.; Rasinski, M.; Steffens, J.; Terra, A.; Linsmeier, C.; Coenen, J. The influence of annealing on yttrium oxide thin film deposited by reactive magnetron sputtering: Process and microstructure. Nucl. Mater. Energy 2017, 10, 1–8. [Google Scholar] [CrossRef]
- ASTM E399-90; Test Method for Linear-Elastic Plane-Strain Fracture Toughness KIc of Metallic Materials. ASTM: West Conshohocken, PA, USA, 1997. [CrossRef]
- Marshall, D.; Cox, B.; Evans, A. The mechanics of matrix cracking in brittle-matrix fibre composites. Acta Metall. 1985, 33, 2013–2021. [Google Scholar] [CrossRef]
- Rice, J.R. A Path Independent Integral and the Approximate Analysis of Strain Concentration by Notches and Cracks. J. Appl. Mech. 1968, 35, 379–386. [Google Scholar] [CrossRef]
- Gross, D.; Seelig, T. Bruchmechanik; Springer: Berlin/Heidelberg, Germany, 2006; p. 327. [Google Scholar]
- Evans, A.; Marshall, D. Overview no. 85 The mechanical behavior of ceramic matrix composites. Acta Metall. 1989, 37, 2567–2583. [Google Scholar] [CrossRef]
- ASTM E1830-11; Test Method for Measurement of Fracture Toughness. ASTM: West Conshohocken, PA, USA, 2013. [CrossRef]
- Coenen, J.W.; Huber, P.; Lau, A.; Raumann, L.; Schwalenberg, D.; Mao, Y.; Riesch, J.; Terra, A.; Linsmeier, C.; Neu, R. Tungsten fibre reinforced tungsten (Wf/W) using yarn based textile preforms. Phys. Scr. 2021, 96, 124063. [Google Scholar] [CrossRef]
- Coenen, J.W.; Treitz, M.; Gietl, H.; Huber, P.; Hoeschen, T.; Raumann, L.; Schwalenberg, D.; Mao, Y.; Riesch, J.; Terra, A.; et al. The use of tungsten yarns in the production for W f /W. Phys. Scr. 2020, T171, 014061. [Google Scholar] [CrossRef]
- Anderson, T. Fracture Mechanics; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- Bloyer, D.R.; Ritchie, R.O.; Rao, K.T.V. Fracture toughness and R-Curve behavior of laminated brittle-matrix composites. Metall. Mater. Trans. A 1998, 29, 2483–2496. [Google Scholar] [CrossRef]
- Pitts, R.; Carpentier, S.; Escourbiac, F.; Hirai, T.; Komarov, V.; Kukushkin, A.; Lisgo, S.; Loarte, A.; Merola, M.; Mitteau, R.; et al. Physics basis and design of the ITER plasma-facing components. J. Nucl. Mater. 2011, 415, S957–S964. [Google Scholar] [CrossRef]
- You, J.; Mazzone, G.; Visca, E.; Greuner, H.; Fursdon, M.; Addab, Y.; Bachmann, C.; Barrett, T.; Bonavolontà, U.; Böswirth, B.; et al. Divertor of the European DEMO: Engineering and technologies for power exhaust. Fusion Eng. Des. 2022, 175, 113010. [Google Scholar] [CrossRef]



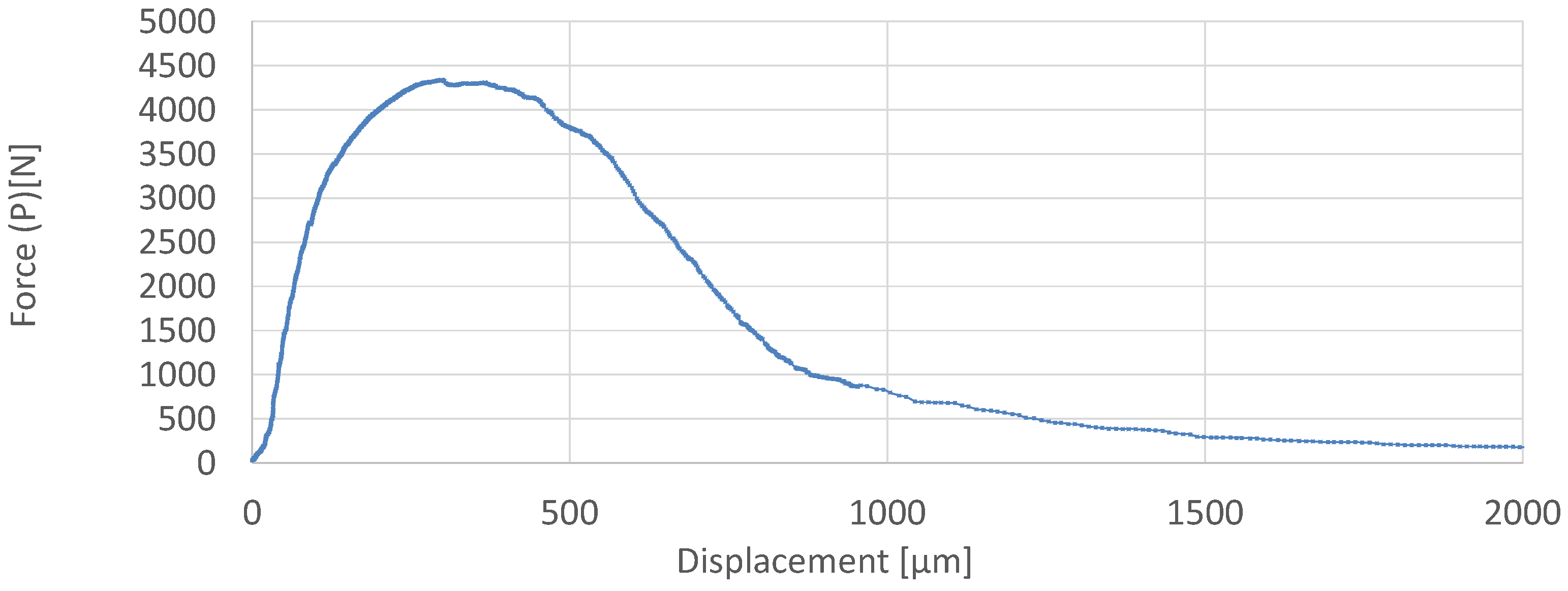
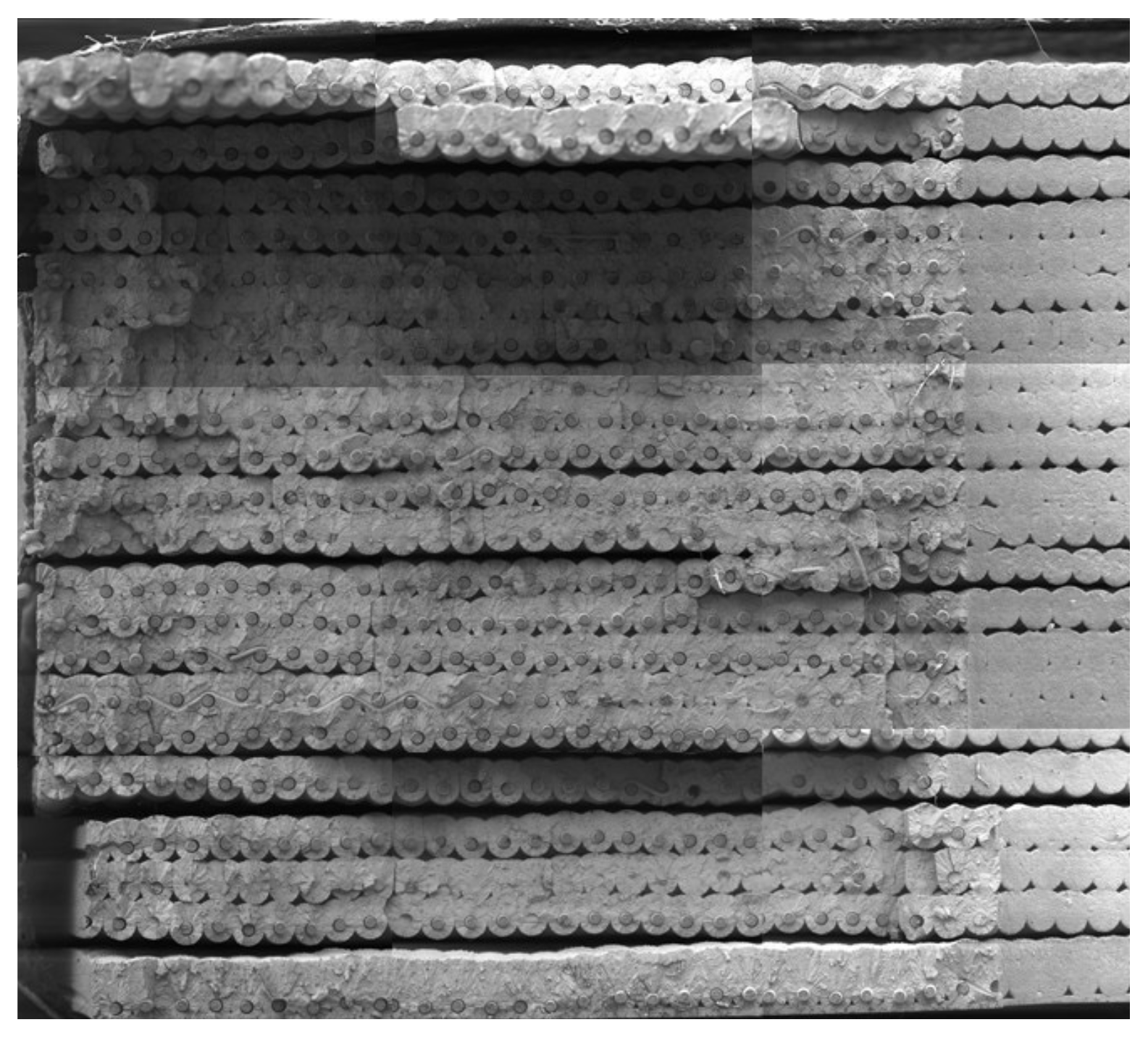
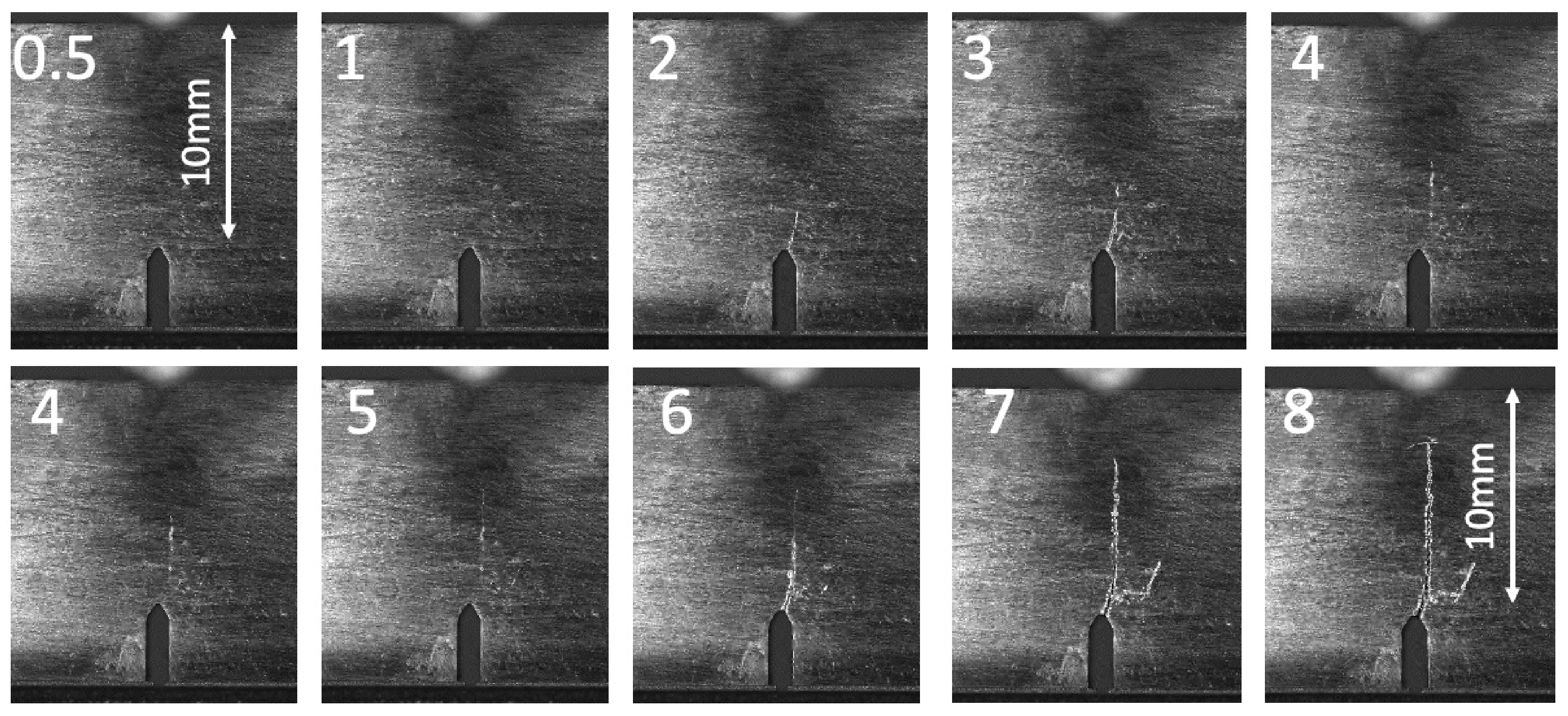


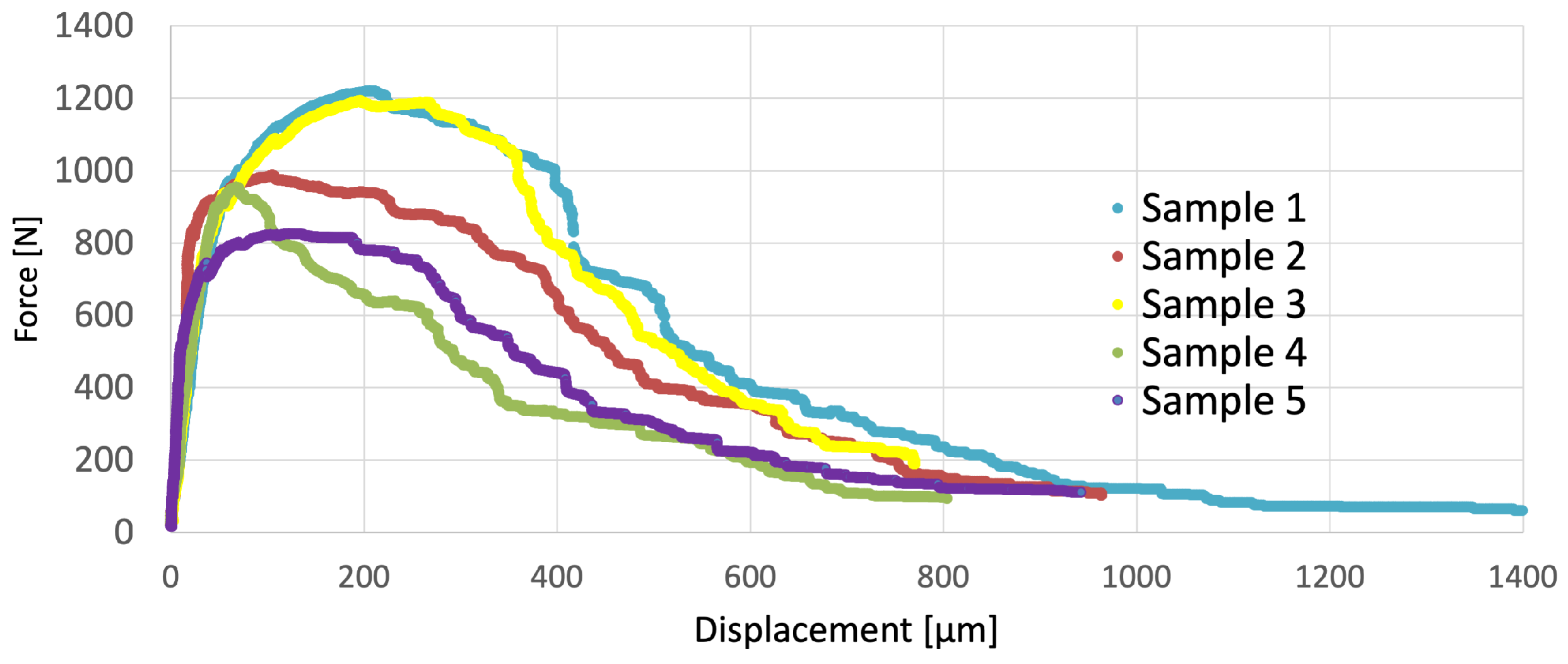

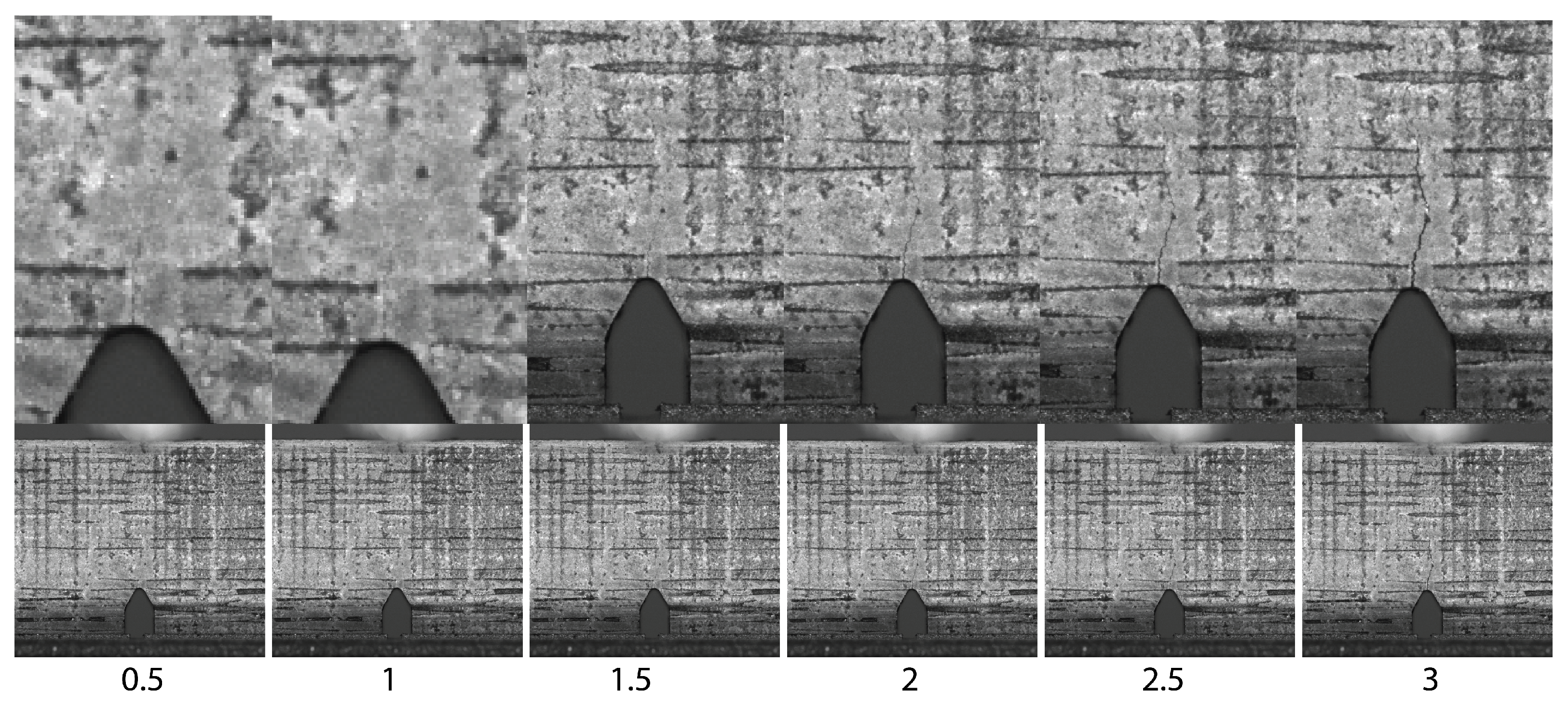

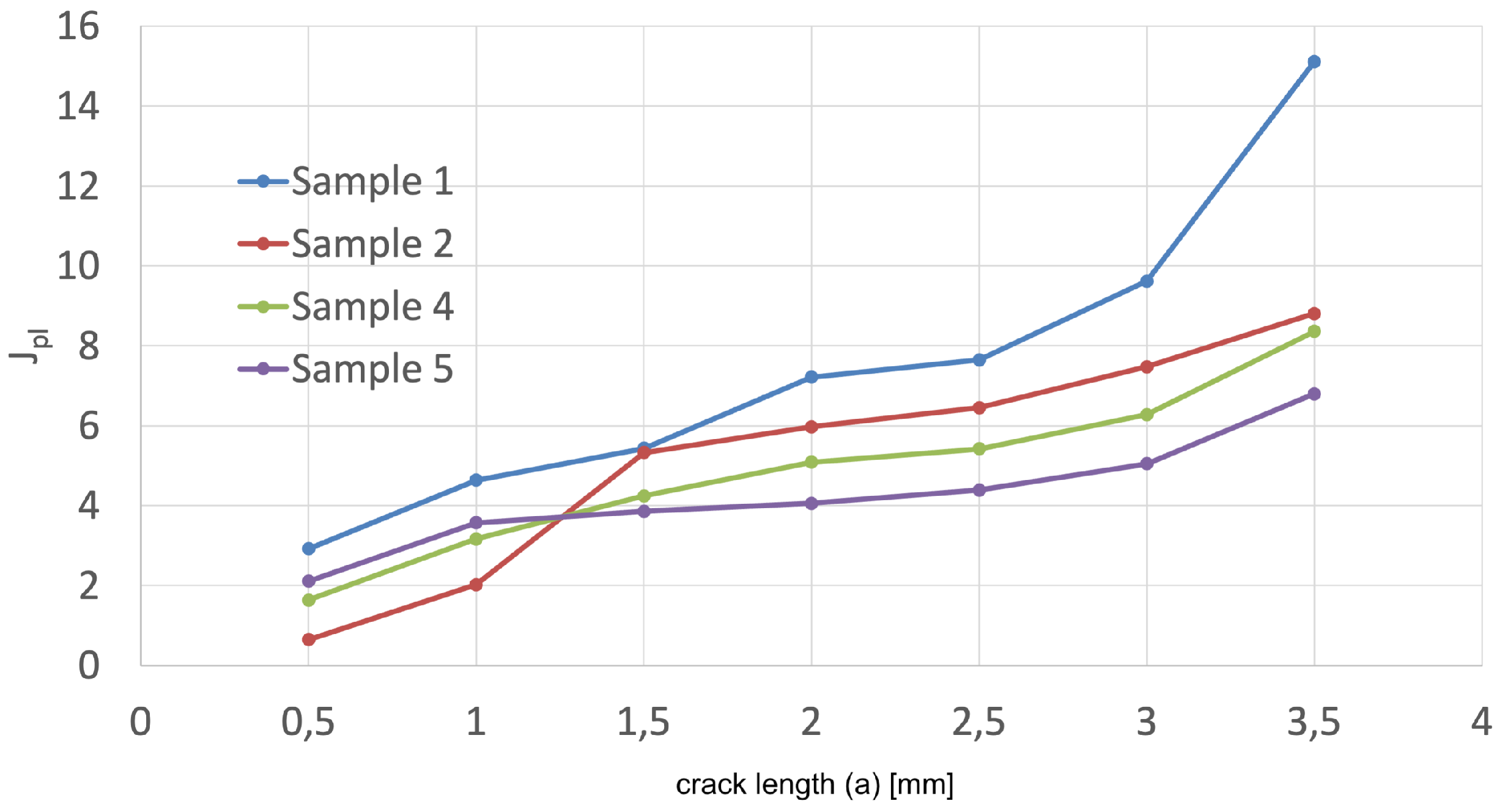
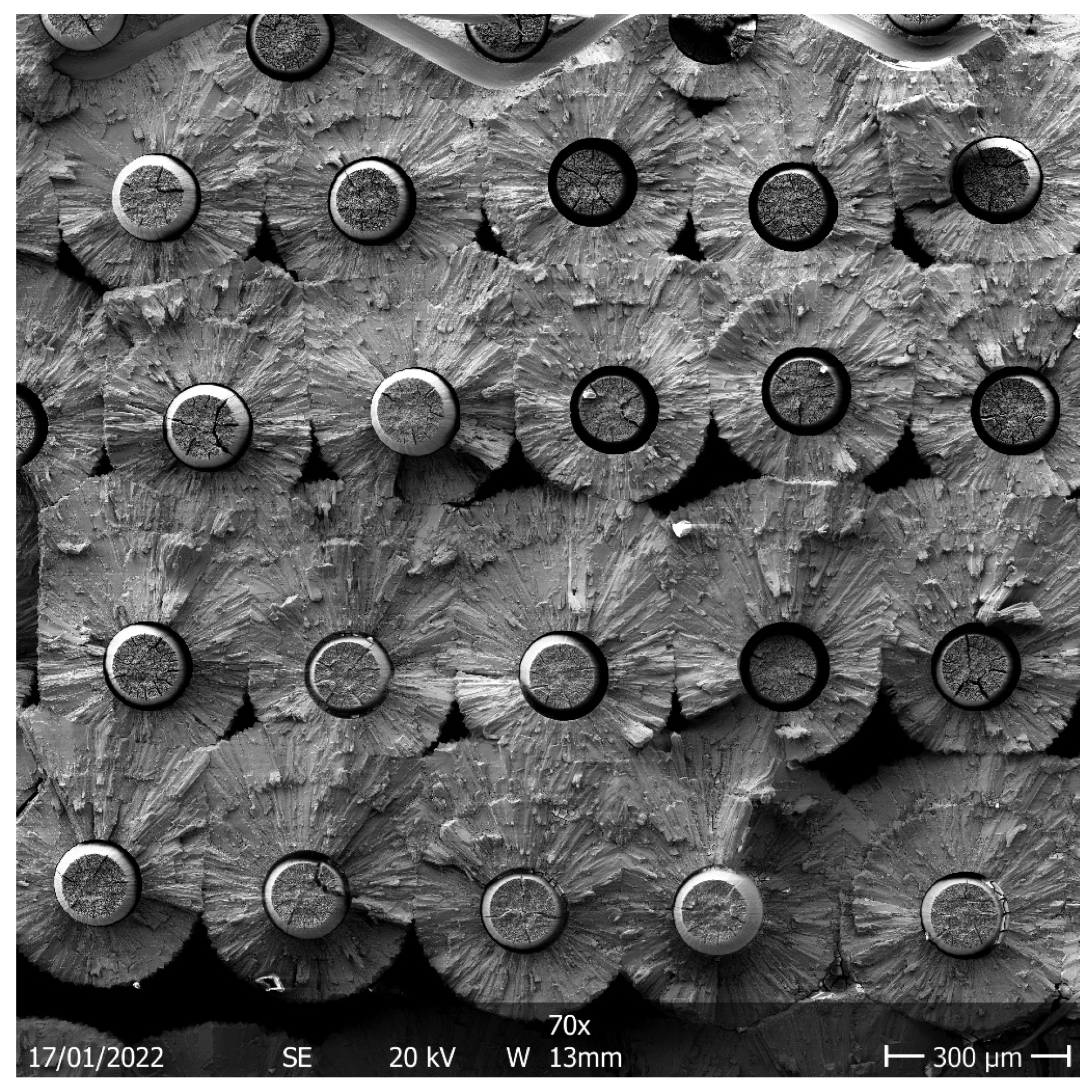
| Sample Type | Dimension (Length × Width × Height) | Amount | Relative Density | Fibre-Volume-Fraction |
|---|---|---|---|---|
| Large | 84 × 24 × 10 | 1 | 92 | 10.4 |
| Medium | 42 × 12 × 5 | 5 | 88–93 | 10.4–12.16 |
| Sample Type | B [mm] | H [mm] | f(a/W) | ||||
|---|---|---|---|---|---|---|---|
| Large Sample | 84 | 10 | 10 | 8 | 30.83 | 4333.53 | 346.68 |
| Medium Sample 1 | 42 | 12 | 5 | 3.5 | 16.69 | 1218.38 | 162.68 |
| Medium Sample 2 | 42 | 12 | 5 | 3.5 | 16.69 | 939.03 | 125.38 |
| Medium Sample 3 | 42 | 12 | 5 | 3.75 | 22.03 | 1218.93 | 214.78 |
| Medium Sample 4 | 42 | 12 | 5 | 3.5 | 16.69 | 790.86 | 105.6 |
| Medium Sample 5 | 42 | 12 | 5 | 3.5 | 16.69 | 806.25 | 107.65 |
| Sample Type | B mm | H (mm) | (mm) | [(mm)] | ||
|---|---|---|---|---|---|---|
| Large | 84 | 10 | 10 | 8 | 40.84 | 134.82 |
| Medium 1 | 42 | 12 | 5 | 3.5 | 15.11 | 82.01 |
| Medium 2 | 42 | 12 | 5 | 3.5 | 8.81 | 62.61 |
| Medium 4 | 42 | 12 | 5 | 3.5 | 8.36 | 61.01 |
| Medium 5 | 42 | 12 | 5 | 3.5 | 6.8 | 55.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schwalenberg, D.; Coenen, J.W.; Riesch, J.; Hoeschen, T.; Mao, Y.; Lau, A.; Gietl, H.; Raumann, L.; Huber, P.; Linsmeier, C.; et al. Large-Scale Tungsten Fibre-Reinforced Tungsten and Its Mechanical Properties. J. Nucl. Eng. 2022, 3, 306-320. https://doi.org/10.3390/jne3040018
Schwalenberg D, Coenen JW, Riesch J, Hoeschen T, Mao Y, Lau A, Gietl H, Raumann L, Huber P, Linsmeier C, et al. Large-Scale Tungsten Fibre-Reinforced Tungsten and Its Mechanical Properties. Journal of Nuclear Engineering. 2022; 3(4):306-320. https://doi.org/10.3390/jne3040018
Chicago/Turabian StyleSchwalenberg, Daniel, Jan Willem Coenen, Johann Riesch, Till Hoeschen, Yiran Mao, Alexander Lau, Hanns Gietl, Leonard Raumann, Philipp Huber, Christian Linsmeier, and et al. 2022. "Large-Scale Tungsten Fibre-Reinforced Tungsten and Its Mechanical Properties" Journal of Nuclear Engineering 3, no. 4: 306-320. https://doi.org/10.3390/jne3040018
APA StyleSchwalenberg, D., Coenen, J. W., Riesch, J., Hoeschen, T., Mao, Y., Lau, A., Gietl, H., Raumann, L., Huber, P., Linsmeier, C., & Neu, R. (2022). Large-Scale Tungsten Fibre-Reinforced Tungsten and Its Mechanical Properties. Journal of Nuclear Engineering, 3(4), 306-320. https://doi.org/10.3390/jne3040018









