Validation of the MORET 5 Monte Carlo Transport Code on Reactor Physics Experiments
Abstract
1. Introduction
2. Selection of Criticality and Reactor Physics Benchmarks
2.1. Selection of Benchmarks
2.2. Methodology for Validation
3. Analysis of KEFF Results
4. Sensitivity/Uncertainty Analysis
5. Other Parameters
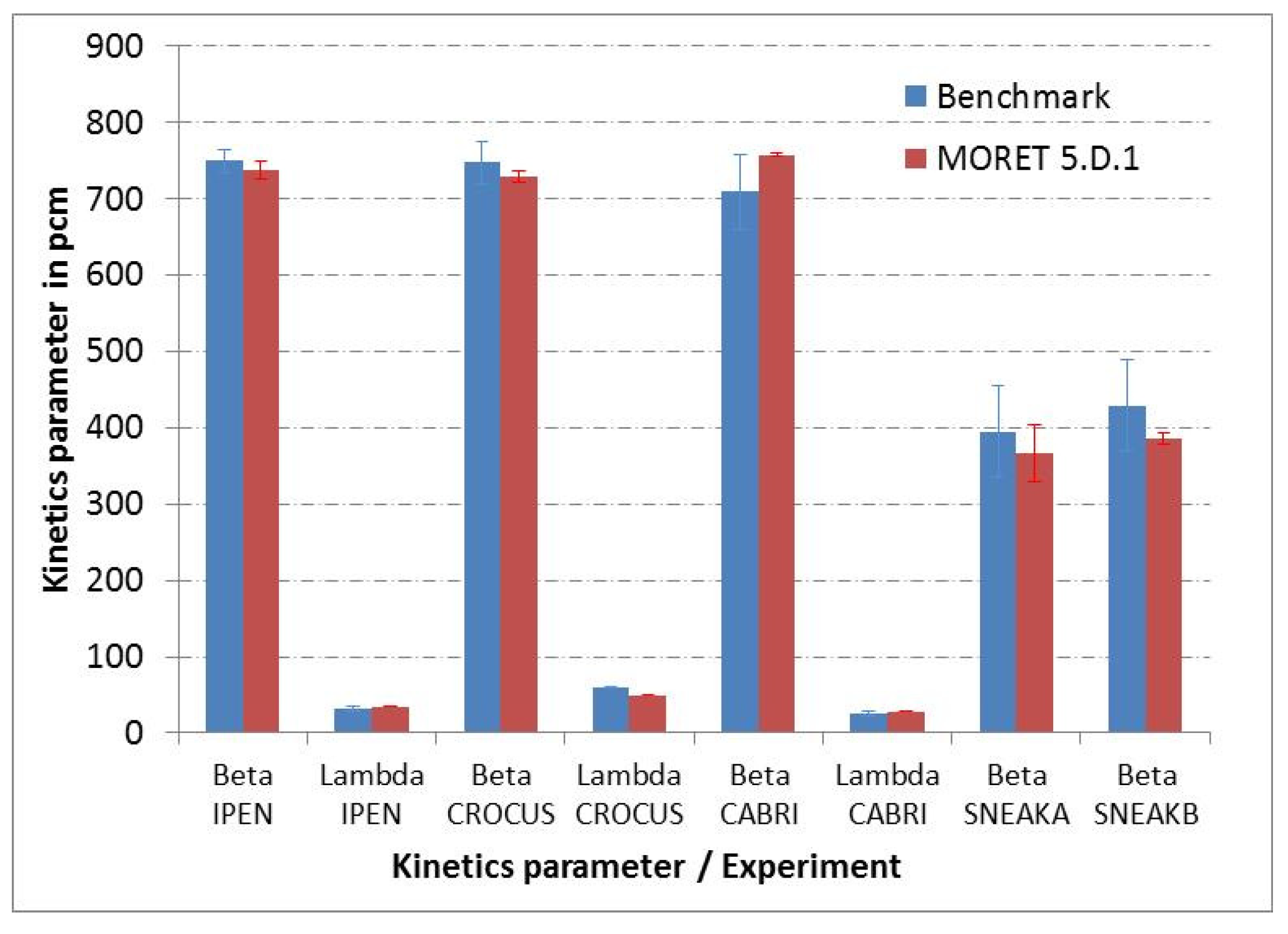
5.1. Kinetics Parameters
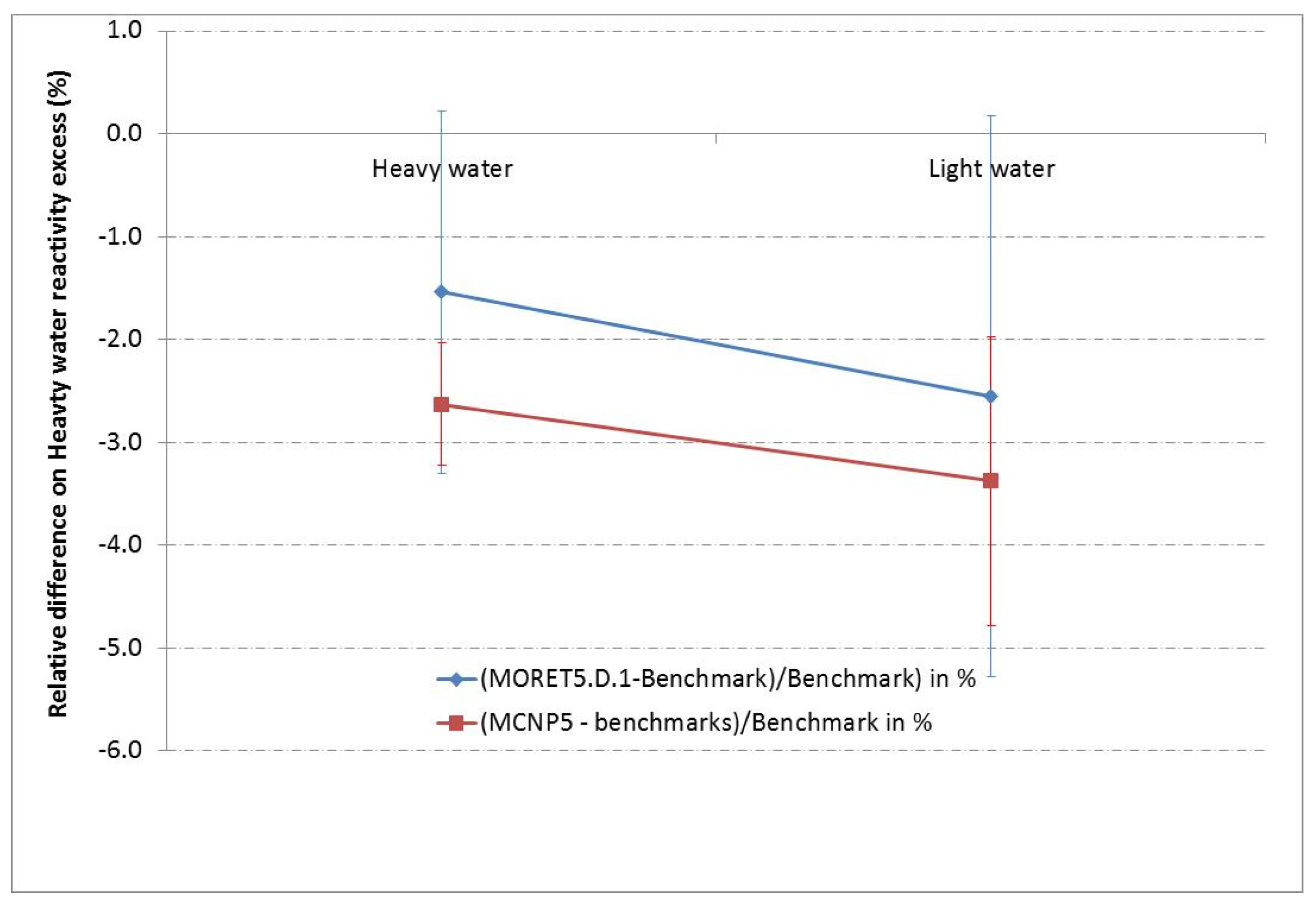
5.2. Reactivity Parameters
6. Conclusions
Author Contributions
Funding
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Cochet, B.; Jinaphanh, A.; Heulers, L.; Jacquet, O. Capabilities overview of the MORET 5 Monte Carlo code. Ann. Nucl. Energy 2015, 82, 74–84. [Google Scholar] [CrossRef]
- Sanchez, R.; Zmijarevic, I.; Coste-Delclaux, M.; Masiello, E.; Santandrea, S.; Martinolli, E.; Villate, L.; Schwartz, N.; Guler, N. APOLLO2 year 2010. Nucl. Eng. Technol. 2010, 42, 474–499. [Google Scholar] [CrossRef]
- Gomit, J.M.; Duhamel, I.; Richet, Y.; Entringer, A.; Magnaud, C.; Malouch, F.; Carmouze, C. CRISTAL V2: New package for criticality calculations. In Proceedings of the Nuclear Criticality Safety Division Topical Meeting (NCSD 2017), Carlsbad, CA, USA, 11–14 September 2017. [Google Scholar]
- International Criticality Safety Benchmark Evaluation Project. International Handbook of Evaluated Criticality Safety Benchmark Experiments, Organization of Economic Cooperation and Development-Nuclear Energy; NEA/NSC/DOC(95)03; United States Department of Energy: Washington, DC, USA, 2016. [Google Scholar]
- International Reactor Physics Experiment Evaluation Project. International Handbook of Evaluated Reactor Physics Benchmark Experiments, Organization of Economic Cooperation and Development-Nuclear Energy; NEA/NSC/DOC(2006)01; United States Department of Energy: Washington, DC, USA, 2018. [Google Scholar]
- Ritter, G.; Rodiac, F.; Beretz, D.; Jammes, C.; Guéton, O. Neutron Commissioning in the new CABRI Water Loop Facility. In Proceedings of the International Group on Research Reactors 13th Conference, Knoxville, TN, USA, 19–23 September 2010. [Google Scholar]
- Jinaphanh, A.; LeClaire, N.; Cochet, B. Continuous-Energy Sensitivity Coefficients in the MORET Code. Nucl. Sci. Eng. 2016, 184, 53–68. [Google Scholar] [CrossRef]
- Ichou, R.; Jeannesson, C.; Haeck, W. Feedback on JEFF-3.3 Processing with GAIA 1.1; JEFDOC 1930; OECD: Paris, France, 2018. [Google Scholar]
- MacFarlane, R.; Kahler, A. Methods for Processing ENDF/B-VII with NJOY. Nucl. Data Sheets 2010, 111, 2739–2890. [Google Scholar] [CrossRef]
- Rearden, B.T.; Reed, D.A.; Lefebvre, R.A.; Mueller, D.; Marshall, W. SCALE/TSUNAMI Sensitivity Data for ICSBEP Evaluations. In Proceedings of the International Conference on Nuclear Criticality 2011, Edinburgh, UK, 19–22 September 2011. [Google Scholar]
- Petrie, L.M.; Jordon, W.C.; Edwards, A.L.; Williams, P.T.; Ryman, J.C.; Hermann, O.W.; Landers, N.F.; Bucholz, J.A.; Knight, J.R.; Parks, C.V.; et al. SCALE: A Modular Code System for Performing Standardized Computer Analyses for Licensing Evaluations; NUREG/CR-0200, Rev. 7 (ORNL/NUREG/CSD-2/R7); Radiation Safety Information Computational Center: Oak Ridge, TN, USA, 2004; Volume 1–3. [Google Scholar]
- Brown, F.; Kiedrowski, B.; Bull, J. MCNP5-1.60 Release Notes; LA UR 10 06235; US Department of Energy: Washington, DC, USA, 2010. [Google Scholar]
- Jinaphanh, A.; Fernex, F.; Leclaire, N. Uncertainty and Bias Quantification with the MACSENS Software; EGUACSA-2017; OECD NEA: Paris, France, 2017. [Google Scholar]
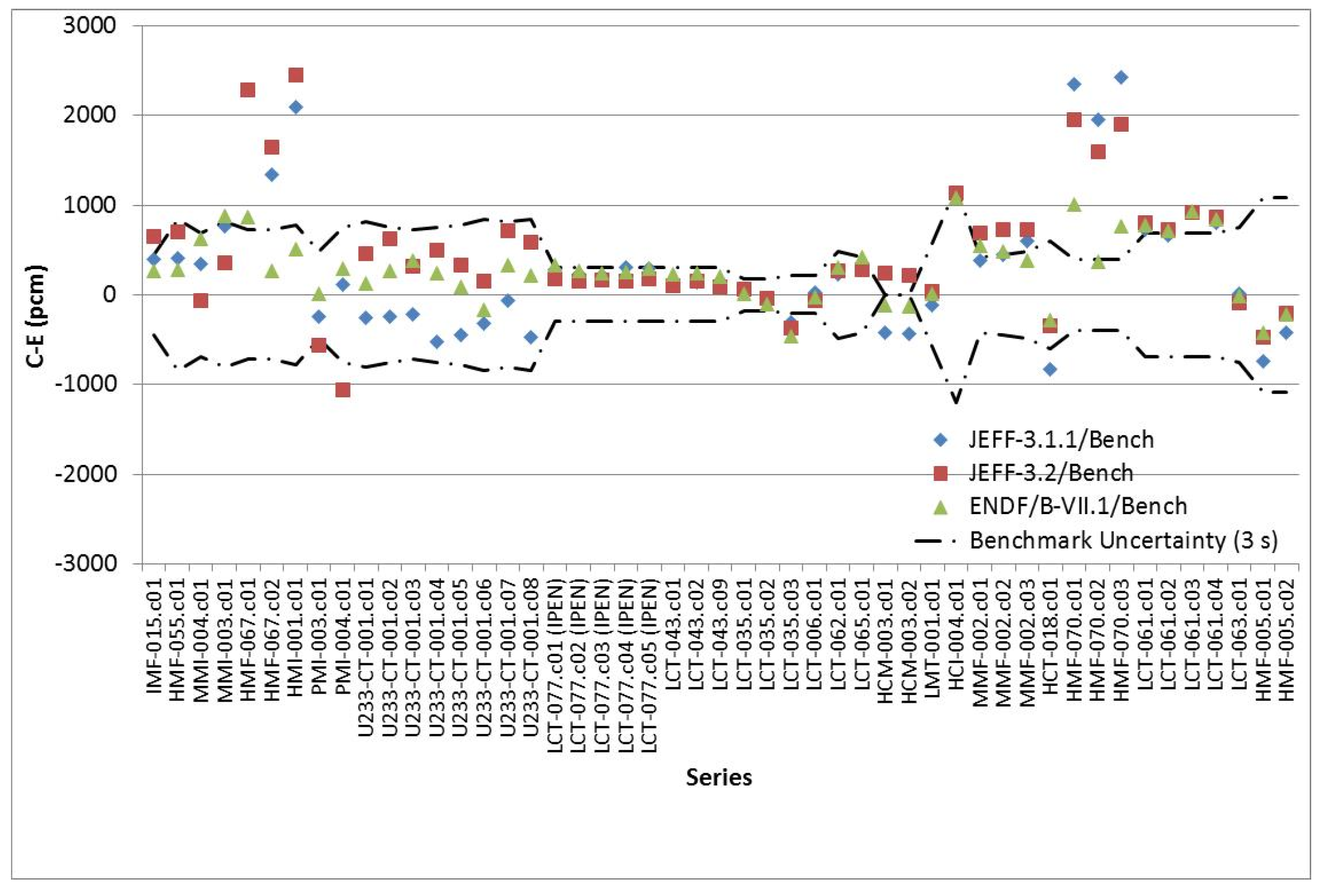
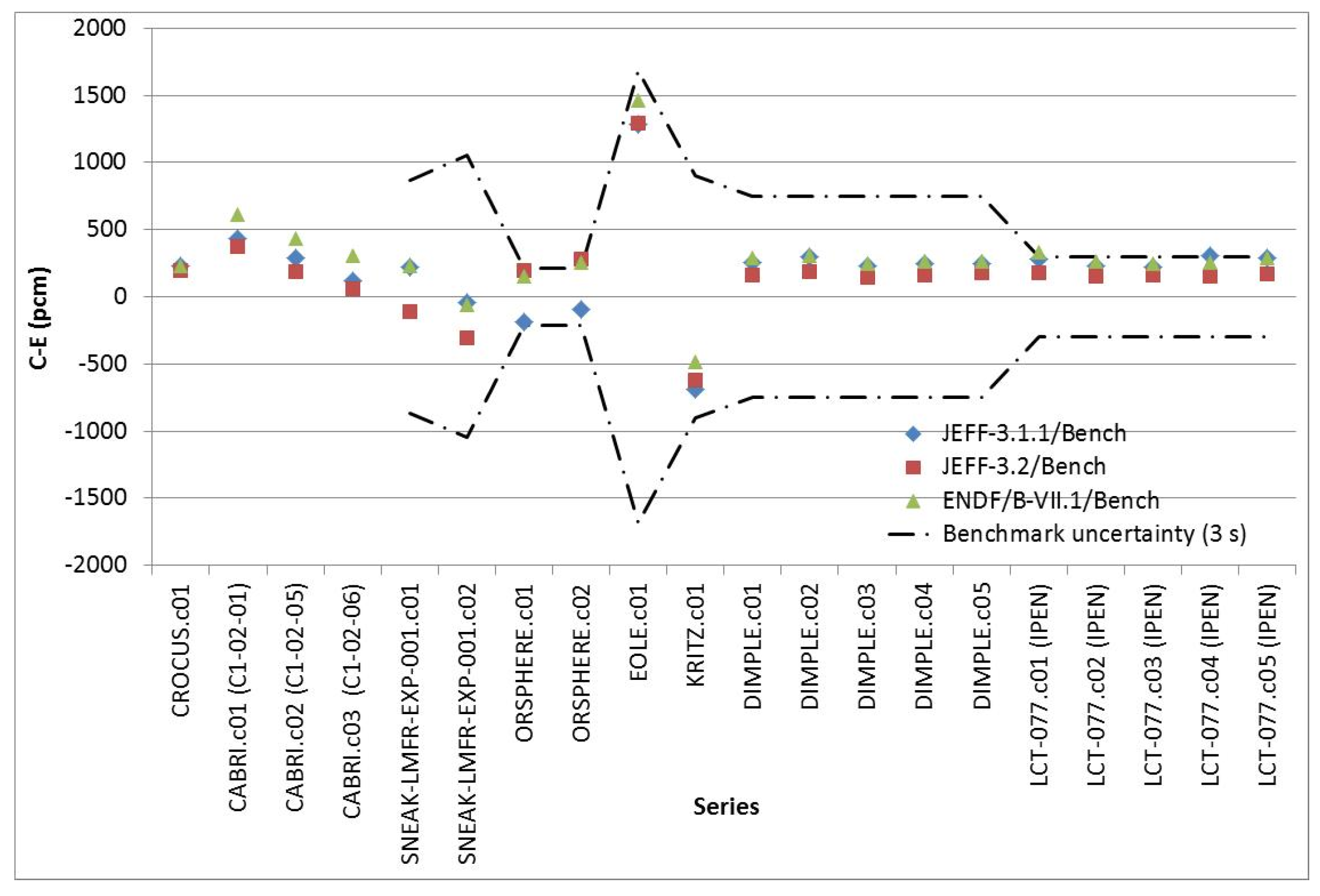


| Series of Experiments | Description | Parameter of Interest |
|---|---|---|
| IPEN1—LCT-077 | Critical loading configurations of the IPEN/MB-01 reactor | keff, spectral indices, reactivity coefficients, reaction rates |
| SNEAK-LMFR-EXP-001 | SNEAK 7A and 7B Pu-fueled fast critical assemblies in the Karlsruhe fast critical facility | keff, spectral indices, reactivity coefficients, kinetics parameters |
| CROCUS-LWR-RESR-001 | Benchmark on Kinetics Parameters in CROCUS | keff, reactivity coefficients, kinetics parameters |
| CABRI | Studies on the French CABRI reactor to determine the behavior of fuel rods (cladding) during a reactivity insertion accident in PWR | keff, kinetics parameters, control rods’ reactivity worth, isothermal temperature coefficient |
| ORSPHERE-FUND-EXP-001—HMF-100 | Physics measurements for bare, HEU(93.2)-metal sphere | keff, reactivity effects, kinetics parameters |
| EOLE-PWR-EXP-001—MCI-005 | Under-moderated MOX (11 wt.% PuO2) lattice in the EOLE reactor | keff |
| KRITZ-LWR-RESR-001 | KRITZ-2:19 experiment on regular H2O/fuel pin lattices with mixed oxide fuel at temperatures 21.1 °C and 235.9 °C | keff, reaction rates distribution |
| DIMPLE-LWR-EXP-001—LCT-048 | Light water moderated and reflected low Enriched uranium (3 wt.% 235U) dioxide rod lattices DIMPLE s01 | keff, reaction rates distribution |
| Benchmarks | Total Prior Uncertainty 44groupcov (pcm) | Total Prior Uncertainty 56groupcov7.1 (pcm) |
|---|---|---|
| HCT-018-001 | 506 | 555 |
| KRITZ-LWR-RESR-001 (cold) | 1160 | 720 |
| IPEN1—LCT-077-001 | 655 | 671 |
| EOLE-PWR-EXP-001 | 976 | 735 |
| CABRI program | 518 | 553 |
| CROCUS—Erbium | 553 | 597 |
| DIMPLE—LCT-048-005 | 578 | 616 |
| SNEAK A | 1150 | 872 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leclaire, N.; Duhamel, I. Validation of the MORET 5 Monte Carlo Transport Code on Reactor Physics Experiments. J. Nucl. Eng. 2021, 2, 65-73. https://doi.org/10.3390/jne2010007
Leclaire N, Duhamel I. Validation of the MORET 5 Monte Carlo Transport Code on Reactor Physics Experiments. Journal of Nuclear Engineering. 2021; 2(1):65-73. https://doi.org/10.3390/jne2010007
Chicago/Turabian StyleLeclaire, Nicolas, and Isabelle Duhamel. 2021. "Validation of the MORET 5 Monte Carlo Transport Code on Reactor Physics Experiments" Journal of Nuclear Engineering 2, no. 1: 65-73. https://doi.org/10.3390/jne2010007
APA StyleLeclaire, N., & Duhamel, I. (2021). Validation of the MORET 5 Monte Carlo Transport Code on Reactor Physics Experiments. Journal of Nuclear Engineering, 2(1), 65-73. https://doi.org/10.3390/jne2010007





